Natural Fractals as Irreversible Disorder: Entropy Approach from Cracks in the Semi Brittle-Ductile Lithosphere and Generalization
Abstract
1. Introduction
- Earthquakes spatial distributions [24],
- Earthquake slip patterns [25],
- Structural geology [27],
- Galaxies clustering [28],
- Self-organized criticality (SOC) systems [29],
- High energy collisions data [32],
- Fractal electrodynamics [33],
- Fractal structures of spacetime and mass [34],
- Snowflakes dendrites distribution [35],
- Neuropsychiatric disorders [38],
- Ecology [39],
- Economics [40],
- Urbanism [41],
- Laws [42],
2. Results and Discussion
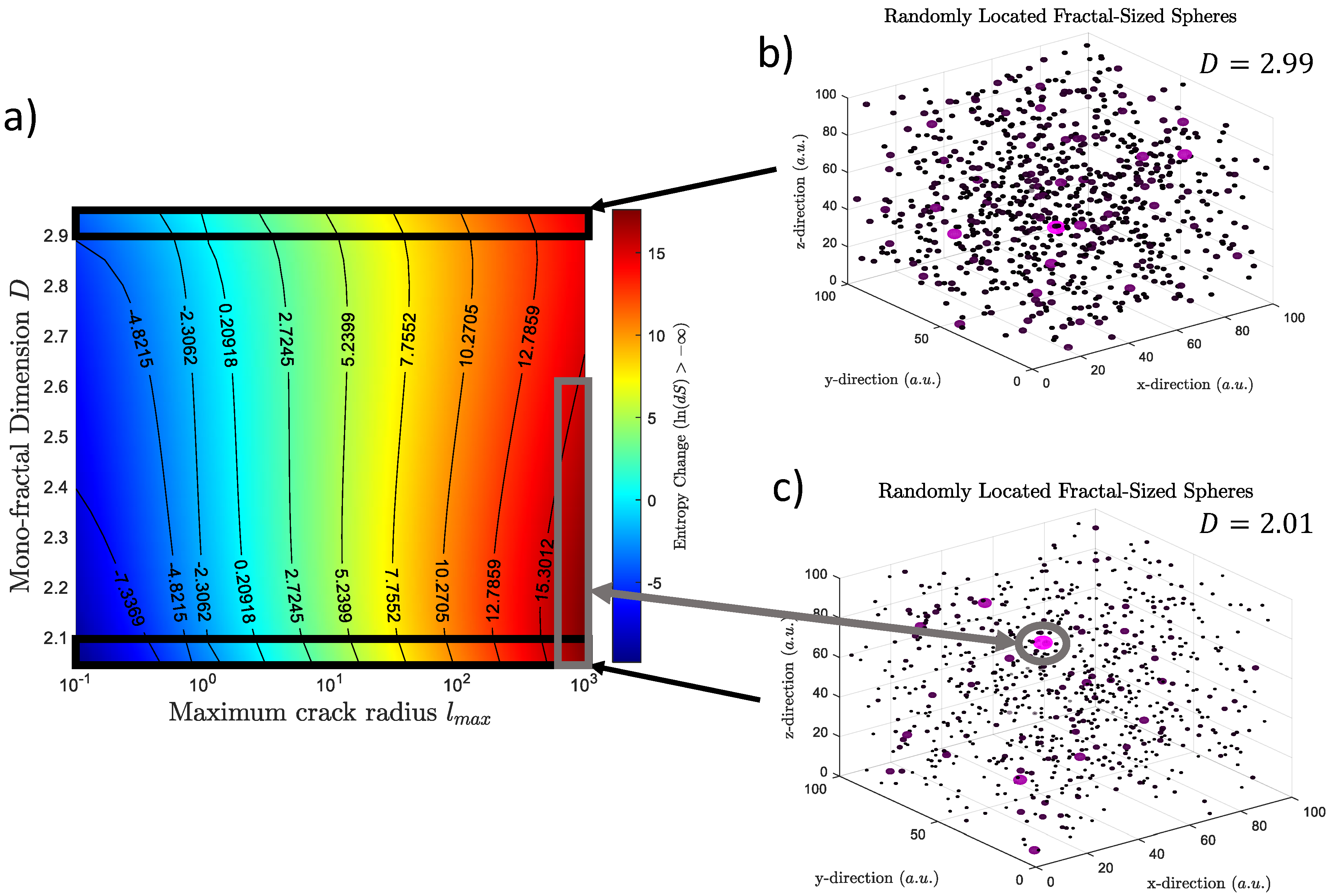
2.1. Entropy of Fractals Cracks Distribution
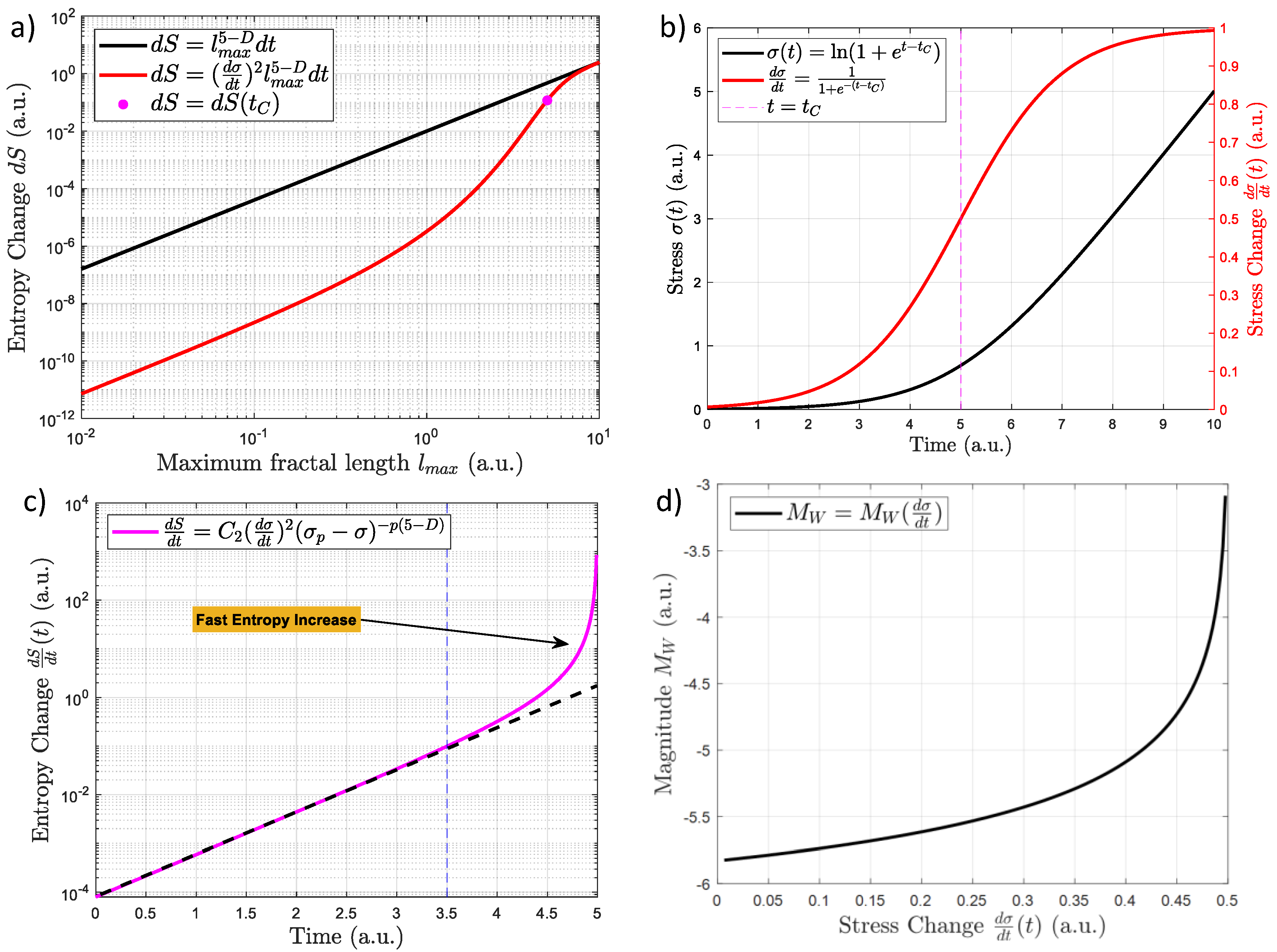
Entropy Change in Terms of Spatial Parameters
2.2. Entropy Change in Terms of External Stress Change
Seismic Moment and Entropy
2.3. Entropy and Fractal Geometry Generalization for Linear Nonequilibrium Thermodynamics
Multifractal Entropy for Linear Nonequilibrium Thermodynamics
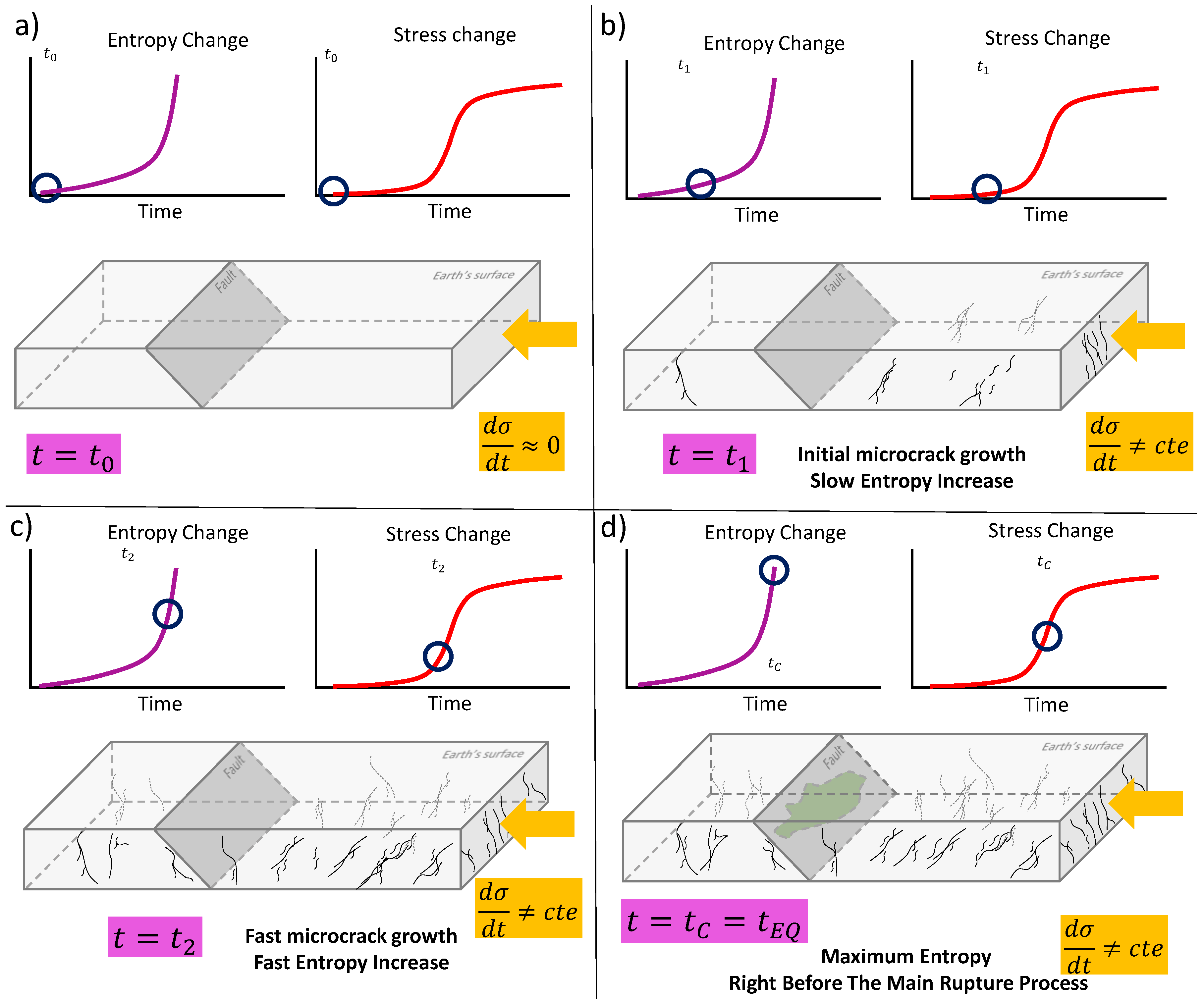
2.4. Discussion
3. Conclusions
- As Equation (8) is always positive, it is implied that the generation of cracks are the manifestation of irreversible process.
- The pre-failure and failure process can be linked by means of the entropy changes.
- The seismic moment and magnitude exist if external stress, that increases of the entropy of the lithosphere, and increases in the number of cracks and electromagnetic signals also exist.
- It is possible to estimate an expected seismic magnitude in terms of the entropy change/stress change.
- Entropy rapidly increases before earthquakes.
- No entropy increase, no earthquake.
- The seismo-electromagnetic theory explains the non-seismic pre-earthquakes signals and gives physical foundations to the generation of earthquakes.
- The tendency in which nature creates fractals corresponds to a geometrical manifestation of that tendency in which the universe increases the entropy.
- Fractals rising in several fields and topics reveals the increase of ‘disorder’ of those systems.
- The phenomenological coefficients can describe geometrical properties of forces and fluxes.
- The Constructal law is one geometrical application of Onsager’s relations.
- The entropy density is defined as , which represents the quadratic time derivative of those forces () that generate the fractal geometry . No changing force implies no fractality.
- More work must be done in order to link metric tensor, fractal entropy and multiscale thermodynamics.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Aki, K.; Richards, P.G. Quantitative Seismology: Theory and Methods; W. H. Freeman & Co.: New York, NY, USA, 2002. [Google Scholar]
- Fernández, J. (Ed.) Geodetic and Geophysical Effects Associated with Seismic and Volcanic Hazards. In Pageoph Topical Volumes; Birkhäuser: Basel, Switzerland, 2004. [Google Scholar] [CrossRef]
- Vallianatos, F.; Tzanis, A. Electric Current Generation Associated with the Deformation Rate of a Solid: Preseismic and Coseismic Signals. Phys. Chem. Earth 1998, 23, 933–938. [Google Scholar] [CrossRef]
- Rabinovitch, A.; Frid, V.; Bahat, D. Use of electromagnetic radiation for potential forecast of earthquakes. Geol. Mag. 2018, 155, 992–996. [Google Scholar] [CrossRef]
- Geller, R.J.; Jackson, D.D.; Kagan, Y.Y.; Mulargia, F. Earthquakes Cannot Be Predicted. Science 1997, 275, 1616. [Google Scholar] [CrossRef]
- Zhuang, J.; Matsu’ura, M.; Han, P. Critical zone of the branching crack model for earthquakes: Inherent randomness, earthquake predictability, and precursor modelling. Eur. Phys. J. Spec. Top. 2021, 230, 409–424. [Google Scholar] [CrossRef]
- McBeck, J.A.; Zhu, W.; Renard, F. The competition between fracture nucleation, ropagation, and coalescence in dry and water-saturated crystalline rock. Solid Earth 2021, 12, 375–387. [Google Scholar] [CrossRef]
- McBeck, J.; Ben-Zion, Y.; Renard, F. Fracture Network Localization Preceding Catastrophic Failure in Triaxial Compression Experiments on Rocks. Front. Earth Sci. 2021, 9, 778811. [Google Scholar] [CrossRef]
- Triantis, D.; Stavrakas, I.; Anastasiadis, C.; Kyriazopoulos, A.; Vallianatos, F. An analysis of Pressure Stimulated Currents (PSC), in marble samples under mechanical stress. Phys. Chem. Earth Parts A/B/C 2006, 31, 234–239. [Google Scholar] [CrossRef]
- Stroh, A.N. The Formation of Cracks in Plastic Flow II. Proc. R. Soc. London. Ser. A Math. Phys. Sci. 1955, 232, 548–560. [Google Scholar]
- Ma, L.; Zhao, J.; Ni, B. A Zener-Stroh crack interacting with an edge dislocation. Theor. Appl. Mech. Lett. 2011, 2, 021003. [Google Scholar] [CrossRef]
- Triantis, D.; Vallianatos, F.; Stavrakas, I.; Hloupis, G. Relaxation phenomena of electrical signal emissions from rock following application of abrupt mechanical stress. Ann. Geophys. 2012, 55. [Google Scholar] [CrossRef]
- Li, D.; Wang, E.; Li, Z.; Ju, Y.; Wang, D.; Wang, X. Experimental investigations of pressure stimulated currents from stressed sandstone used as precursors to rock fracture. Int. J. Rock Mech. Min. Sci. 2021, 145, 104841. [Google Scholar] [CrossRef]
- De Santis, A.; Balasis, G.; Pavón-Carrasco, F.J.; Cianchini, G.; Mandea, M. Potential earthquake precursory pattern from space: The 2015 Nepal event as seen by magnetic Swarm satellites. Earth Planet. Sci. Lett. 2017, 461, 119–126. [Google Scholar] [CrossRef]
- Marchetti, D.; Akhoondzadeh, M. Analysis of Swarm satellites data showing seismo-ionospheric anomalies around the time of the strong Mexico (Mw D 8:2) earthquake of 08 September 2017. Adv. Space Res. 2018, 62, 614–623. [Google Scholar] [CrossRef]
- Cordaro, E.G.; Venegas, P.; Laroze, D. Long-term magnetic anomalies and their possible relationship to the latest greater Chilean earthquakes in the context of the seismo-electromagnetic theory. Nat. Hazards Earth Syst. Sci. 2021, 21, 1785–1806. [Google Scholar] [CrossRef]
- Venegas-Aravena, P.; Cordaro, E.G.; Laroze, D. A review and upgrade of the lithospheric dynamics in context of the seismo-electromagnetic theory. Nat. Hazards Earth Syst. Sci. 2019, 19, 1639–1651. [Google Scholar] [CrossRef]
- Frid, V.; Rabinovitch, A.; Bahat, D. Earthquake forecast based on its nucleation stages and the ensuing electromagnetic radiations. Phys. Lett. A 2020, 384, 126102. [Google Scholar] [CrossRef]
- Marchetti, D.; Zhu, K.; De Santis, A.; Campuzano, S.A.; Zhang, D.; Soldani, M.; Wang, T.; Cianchini, G.; D’Arcangelo, S.; Di Mauro, D.; et al. Multiparametric and multilayer investigation of global earthquakes in the World by a statistical approach. In Proceedings of the EGU General Assembly 2022, Vienna, Austria, 23–27 May 2022. [Google Scholar] [CrossRef]
- De Santis, A.; Cianchini, G.; Favali, P.; Beranzoli, L.; Boschi, E. The Gutenberg–Richter Law and Entropy of Earthquakes: Two Case Studies in Central Italy. Bull. Seismol. Soc. Am. 2011, 101, 1386–1395. [Google Scholar] [CrossRef]
- Venegas-Aravena, P.; Cordaro, E.G.; Laroze, D. The spatial–temporal total friction coefficient of the fault viewed from the perspective of seismo-electromagnetic theory. Nat. Hazards Earth Syst. Sci. 2020, 20, 1485–1496. [Google Scholar] [CrossRef]
- Posadas, A.; Morales, J.; Posadas-Garzon, A. Earthquakes and entropy: Characterization of occurrence of earthquakes in southern Spain and Alboran Sea. Chaos 2021, 31, 043124. [Google Scholar] [CrossRef] [PubMed]
- Amiri, M.; Khonsari, M.M. On the Thermodynamics of Friction and Wear―A Review. Entropy 2010, 12, 1021–1049. [Google Scholar] [CrossRef]
- Pastén, D.; Munoz, V.; Cisternas, A.; Rogan, J.; Valdivia, J.A. Monofractal and multifractal analysis of the spatial distribution of earthquakes in the central zone of Chile. Phys. Rev. E 2011, 84, 066123. [Google Scholar] [CrossRef] [PubMed]
- Mai, P.M.; Beroza, G.C. A spatial random field model to characterize complexity in earthquake slip. J. Geophys. Res. 2022, 107, 2308. [Google Scholar] [CrossRef]
- Borodich, F.M. Fractals and fractal scaling in fracture mechanics. Int. J. Fract. 1999, 95, 239–259. [Google Scholar] [CrossRef]
- Johnston, J.D.; McCaffrey, J.W. Fractal geometries of vein systems and the variation of scaling relationships with mechanism. J. Struct. Geol. 1996, 18, 349–358. [Google Scholar] [CrossRef]
- Ribeiro, M.B.; Miguelote, A.Y. Fractals and the Distribution of Galaxies. Braz. J. Phys. 1998, 28, 132–160. [Google Scholar] [CrossRef]
- Bak, P.; Tang, C.; Wiesenfeld, K. Self-Organized Criticality: An Explanation of 1/f Noise. Phys. Rev. Lett. 1987, 59, 381–384. [Google Scholar] [CrossRef]
- Li, J.; Pelliciari, J.; Mazzoli, C.; Catalano, S.; Simmons, F.; Sadowski, J.T.; Levitan, A.; Gibert, M.; Carlson, E.; Triscone, J.-M.; et al. Scale-invariant magnetic textures in the strongly correlated oxide NdNiO3. Nat. Commun. 2019, 10, 4568. [Google Scholar] [CrossRef]
- Xu, X.Y.; Wang, X.W.; Chen, D.Y.; Smith, C.M.; Jin, X.-M. Quantum transport in fractal networks. Nat. Photon. 2021, 15, 703–710. [Google Scholar] [CrossRef]
- Deppman, A.; Megías, E. Fractal Structure in Gauge Fields. Physics 2019, 1, 103–110. [Google Scholar] [CrossRef]
- Jaggard, D.L. On Fractal Electrodynamics. In Recent Advances in Electromagnetic Theory; Kritikos, H.N., Jaggard, D.L., Eds.; Springer: New York, NY, USA, 1990. [Google Scholar] [CrossRef]
- Argyris, J.; Ciubotariu, C.; Matuttis, H.G. Fractal space, cosmic strings and spontaneous symmetry breaking. Chaos Solitons Fractals 2001, 12, 1–48. [Google Scholar] [CrossRef]
- Libbrecht, K.G. The physics of snow crystals. Rep. Prog. Phys. 2005, 68, 855–895. [Google Scholar] [CrossRef]
- Weibel, E.R. Fractal geometry: A design principle for living organisms. Am. J. Phys. 1991, 261, L361–L369. [Google Scholar] [CrossRef] [PubMed]
- Buldyrev, S.V. Fractals in Biology. In Encyclopedia of Complexity and Systems Science; Meyers, R., Ed.; Springer: New York, NY, USA, 2009. [Google Scholar] [CrossRef]
- Racz, F.S.; Stylianou, O.; Mukli, P.; Eke, A. Multifractal and Entropy-Based Analysis of Delta Band Neural Activity Reveals Altered Functional Connectivity Dynamics in Schizophrenia. Front. Syst. Neurosci. 2020, 14, 49. [Google Scholar] [CrossRef] [PubMed]
- Brown, J.H.; Gupta, V.K.; Li, B.-L.; Milne, B.T.; Restrepo, C.; West, G.B. The fractal nature of nature: Power laws, ecological complexity and biodiversity. Phil. Trans. R. Soc. Lond. B 2002, 357, 619–626. [Google Scholar] [CrossRef]
- Takayasu, M.; Takayasu, H. Fractals and Economics. In Complex Systems in Finance and Econometric; Meyers, R., Ed.; Springer: New York, NY, USA, 2009. [Google Scholar] [CrossRef]
- Frankhauser, P. From Fractal Urban Pattern Analysis to Fractal Urban Planning Concepts. In Computational Approaches for Urban Environments, Geotechnologies and the Environment; Helbich, M., Arsanjani, J., Leitner, M., Eds.; Springer: Cham, Switzerland, 2015; Volume 13. [Google Scholar] [CrossRef]
- Morrison, A.S. The Law is a Fractal: The Attempt to Anticipate Everything (1 March 2013); U of Michigan Public Law Research Paper, No. 292; 44 Loyola University Chicago L.J.: Chicago, IL, USA, 2013; Volume 649, Available online: https://ssrn.com/abstract=2157804 (accessed on 12 September 2022).
- Mandelbrot, B.B. The Fractal Geometry of Nature; W. H. Freeman and Company: New York, NY, USA, 1982. [Google Scholar]
- Bejan, A.; Lorente, S. Constructal law of design and evolution: Physics, biology, technology, and society. J. Appl. Phys. 2013, 113, 151301. [Google Scholar] [CrossRef]
- Annila, A. All in Action. Entropy 2010, 12, 2333–2358. [Google Scholar] [CrossRef]
- Annila, A. Evolution of the universe by the principle of least action. Phys. Essays 2017, 30, 248–254. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal Relations in Irreversible Processes. I. Phys. Rev. 1931, 37, 405. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal Relations in Irreversible Processes. II. Phys. Rev. 1931, 38, 2265. [Google Scholar] [CrossRef]
- Slifkin, L. Seismic electric signals from displacement of charged dislocations. Tectonophysics 1993, 224, 149–152. [Google Scholar] [CrossRef]
- Fan, H. Interfacial Zener-Stroh Crack. J. Appl. Mech. 1994, 61, 829–834. [Google Scholar] [CrossRef]
- Freund, F. Rocks That Crackle and Sparkle and Glow: Strange Pre-Earthquake Phenomena. J. Sci. Explor. 2003, 17, 37–71. [Google Scholar]
- Anastasiadis, C.; Triantis, D.; Stavrakas, I.; Vallianatos, F. Pressure Stimulated Currents (PSC) in marble samples. Ann. Geophys. 2004, 47, 21–28. [Google Scholar] [CrossRef]
- Vallianatos, F.; Triantis, D. Scaling in Pressure Stimulated Currents related with rock fracture. Phys. A 2008, 387, 4940–4946. [Google Scholar] [CrossRef]
- Cartwright-Taylor, A.; Vallianatos, F.; Sammonds, P. Superstatistical view of stress-induced electric current fluctuations in rocks. Phys. A 2014, 414, 368–377. [Google Scholar] [CrossRef]
- Zhang, X.; Li, Z.; Wang, E.; Li, B.; Song, J.; Niu, Y. Experimental investigation of pressure stimulated currents and acoustic emissions from sandstone and gabbro samples subjected to multi-stage uniaxial loading. Bull. Eng. Geol. Environ. 2021, 80, 7683–7700. [Google Scholar] [CrossRef]
- Hayakawa, M.; Schekotov, A.; Potirakis, S.; Eftaxias, K. Criticality features in ULF magnetic fields prior to the 2011 Tohoku earthquake. Proc. Jpn. Acad. Ser. B, Phys. Biol. Sci. 2015, 91, 25–30. [Google Scholar] [CrossRef]
- Cordaro, E.G.; Venegas, P.; Laroze, D. Latitudinal variation rate of geomagnetic cutoff rigidity in the active Chilean convergent margin. Ann. Geophys. 2018, 36, 275–285. [Google Scholar] [CrossRef]
- De Santis, A.; Marchetti, D.; Pavón-Carrasco, F.J.; Cianchini, G.; Perrone, L.; Abbattista, C.; Alfonsi, L.; Amoruso, L.; Campuzano, S.A.; Carbone, M.; et al. Precursory worldwide signatures of earthquake occurrences on Swarm satellite data. Sci. Rep. 2019, 9, 20287. [Google Scholar] [CrossRef]
- Blackett, M.; Wooster, M.J.; Malamud, B.D. Exploring land surface temperature earthquake precursors: A focus on the Gujarat (India) earthquake of 2001. Geophys. Res. Lett. 2011, 38, 1–7. [Google Scholar] [CrossRef]
- Tzanis, A.; Vallianatos, F. A physical model of electrical earthquake precursors due to crack propagation and the motion of charged edge dislocations. In Seismo Electromagnetics (Lithosphere–Atmosphere–Ionosphere-Coupling); TerraPub: Tokyo, Japan, 2002; pp. 117–130. [Google Scholar]
- Lerner, L.S. Physics for Scientists and Engineers; Jones and Bartlett Publishers: Sudbury, MA, USA, 1997; Volume 2. [Google Scholar]
- Griffiths, D.J. Introduction to electrodynamics. Am. Assoc. Phys. Teach. 2005, 73, 574. [Google Scholar] [CrossRef]
- Nosonovsky, M.; Amano, R.; Luccia, J.M.; Rohatgi, P.K. Physical chemistry of self-organization and self-healing in metals. Phys. Chem. Chem. Phys. 2009, 11, 9530–9536. [Google Scholar] [CrossRef] [PubMed]
- Xie, H.; Sanderson, D.J. Fractal kinematics of crack propagation in geomaterials. Eng. Fract. Mech. 1995, 50, 529–536. [Google Scholar] [CrossRef]
- Uritsky, V.; Smirnova, N.; Troyan, V.; Vallianatos, F. Critical dynamics of fractal fault systems and its role in the generation of pre-seismic electromagnetic emissions. Phys. Chem. Earth 2004, 29, 473–480. [Google Scholar] [CrossRef]
- Turcotte, D.L. Fractals and Chaos in Geology and Geophysics, 2nd ed.; Cambridge University Press: Cambridge, UK, 1997; p. 397. [Google Scholar]
- Thouless, D. Condensed matter physics in less than three dimensions. In The New Physics; Davies, P., Ed.; Cambridge University Press: Cambridge, UK, 1989; pp. 209–235. [Google Scholar]
- Bruce, A.; Wallace, D. Critical point phenomena: Universal physics at large length scales. In The New Physics; Davies, P., Ed.; Cambridge University Press: Cambridge, UK, 1989; pp. 236–267. [Google Scholar]
- Cartwright-Taylor, A.; Main, I.G.; Butler, I.B.; Fusseis, F.; Flynn, M.; King, A. Catastrophic Failure: How and When? Insights From 4-D In Situ X-ray Microtomography. J. Geophys. Res. Solid Earth 2020, 125, e2020JB019642. [Google Scholar] [CrossRef]
- Lucia, U. Maximum entropy generation and k-exponential model. Phys. A Stat. Mech. Its Appl. 2010, 389, 4558–4563. [Google Scholar] [CrossRef]
- Murotani, S.; Satake, K.; Fujii, Y. Scaling relations of seismic moment, rupture area, average slip, and asperity size for M~9 subduction-zone earthquakes. Geophys. Res. Lett. 2013, 40, 5070–5074. [Google Scholar] [CrossRef]
- Demirel, Y. Chapter 3—Linear nonequilibrium thermodynamics, in Nonequilibrium Thermodynamics. Transp. Rate Processes Phys. Biol. Syst. 2002, 59–83. [Google Scholar] [CrossRef]
- Demirel, Y. Chapter 3—Fundamentals of Nonequilibrium Thermodynamics, Nonequilibrium Thermodynamics (Third Edition). Transp. Rate Processes Phys. Chem. Biol. Syst. 2014, 119–176. [Google Scholar] [CrossRef]
- Alvarez, F.X.; Jou, D.; Sellitto, A. Pore-size dependence of the thermal conductivity of porous silicon: A phonon hydrodynamic approach. Appl. Phys. Lett. 2010, 97, 033103. [Google Scholar] [CrossRef]
- Wang, M.; Guo, Z.Y. Understanding of temperature and size dependences of effective thermal conductivity of nanotubes. Phys. Lett. A 2010, 374, 4312–4315. [Google Scholar] [CrossRef]
- Wang, M.; Yang, N.; Guo, Z.Y. Non-Fourier heat conductions in nanomaterials. J. Appl. Phys. 2011, 110, 064310. [Google Scholar] [CrossRef]
- Wang, H.F. Theory of Linear Poroelasticity with Applications to Geomechanics and Hydrogeology; Princeton University Press: Princeton, NJ, USA, 2017. [Google Scholar]
- Beretta, G.P. Steepest entropy ascent model for far-nonequilibrium thermodynamics: Unified implementation of the maximum entropy production principle. Phys. Rev. E 2014, 90, 042113. [Google Scholar] [CrossRef] [PubMed]
- Nosonovsky, M.; Mortazavi, V. Friction-Induced Vibrations and Self-Organization, Mechanics and Non-Equilibrium Thermodynamics of Sliding Contact, 1st ed.; CRC Press: Boca Raton, FL, USA, 2013. [Google Scholar] [CrossRef]
- Xie, H. Fractals in Rock Mechanics, 1st ed.; CRC Press: Boca Raton, FL, USA, 1993. [Google Scholar]
- Basirat, R.; Goshtasbi, K.; Ahmadi, M. Scaling geological fracture network from a micro to a macro scale. Frat. Integrità Strutt. 2020, 51, 71–80. [Google Scholar] [CrossRef]
- Pappachen, J.P.; Sathiyaseelan, R.; Gautam, P.K.; Pal, S.K. Crustal velocity and interseismic strain-rate on possible zones for large earthquakes in the Garhwal–Kumaun Himalaya. Sci. Rep. 2021, 11, 21283. [Google Scholar] [CrossRef]
- Bedford, J.R.; Moreno, M.; Deng, Z.; Oncken, O.; Schurr, B.; John, T.; Báez, J.C.; Bevis, M. Months-long thousand-kilometre-scale wobbling before great subduction earthquakes. Nature 2020, 580, 628–635. [Google Scholar] [CrossRef]
- Anagnostopoulos, G. On the Origin of ULF Magnetic Waves Before the Taiwan Chi-Chi 1999 Earthquake. Front. Earth Sci. 2021, 9, 730162. [Google Scholar] [CrossRef]
- Nelson, R.A. 1—Evaluating Fractured Reservoirs: Introduction. In Geologic Analysis of Naturally Fractured Reservoirs, 2nd ed.; Elsevier: Amsterdam, The Netherlands, 2001; pp. 1–100. [Google Scholar] [CrossRef]
- Darcy, H. Les Fontaines Publiques de la Ville de Dijon; Victor Dalmond: Paris, France, 1856. [Google Scholar]
- Finkbeiner, T.; Zoback, M.; Flemings, P.; Stump, B. Stress, ore pressure, and dynamically constrained hydrocarbon columns in the South Eugene Island 330 field, northern Gulf of Mexico. AAPG Bull. 2001, 85, 1007–1031. [Google Scholar] [CrossRef]
- Donzé, F.V.; Tsopela, A.; Guglielmi, Y.; Henry, P.; Gout, C. Fluid migration in faulted shale rocks: Channeling below active faulting threshold. Eur. J. Environ. Civ. Eng. 2020, 1–15. [Google Scholar] [CrossRef]
- Pulinets, S.A.; Ouzounov, D.P. Lithosphere–Atmosphere–Ionosphere Coupling (LAIC) model—An unified concept for earthquake precursors validation. J. Asian Earth Sci. 2011, 41, 371–382. [Google Scholar] [CrossRef]
- Pulinets, S.A.; Ouzounov, D.P.; Karelin, A.V.; Davidenko, D.V. Physical bases of the generation of short-term earthquake precursors: A complex model of ionization-induced geophysical processes in the lithosphere-atmosphere-ionosphere-magnetosphere system. Geomagn. Aeron. 2015, 55, 521–538. [Google Scholar] [CrossRef]
- Daneshvar, M.R.M.; Freund, F.T. Remote sensing of atmospheric and ionospheric signals prior to the Mw 8.3 Illapel earthquake, Chile 2015. Pure Appl. Geophys. 2017, 174, 11–45. [Google Scholar] [CrossRef]
- Mahmood, I. Anomalous variations of air temperature prior to earthquakes. Geocarto Int. 2019, 36, 1396–1408. [Google Scholar] [CrossRef]
- D’Incecco, S.; Petraki, E.; Priniotakis, G.; Papoutsidakis, M.; Yannakopoulos, P.; Nikolopoulos, D. CO2 and Radon Emissions as Precursors of Seismic Activity. Earth Syst. Environ. 2021, 5, 655–666. [Google Scholar] [CrossRef]
- Freund, F. Toward a unified solid state theory for pre-earthquake signals. Acta Geophys. 2010, 58, 719–766. [Google Scholar] [CrossRef]
- Xiong, P.; Long, C.; Zhou, H.; Battiston, R.; De Santis, A.; Ouzounov, D.; Zhang, X.; Shen, X. Pre-Earthquake Ionospheric Perturbation Identification Using CSES Data via Transfer Learning. Front. Environ. Sci. 2021, 9, 779255. [Google Scholar] [CrossRef]
- He, Y.; Yang, D.; Qian, J.; Parrot, M. Anomaly of the ionospheric electron density close to earthquakes: Case studies of Pu’er and Wenchuan earthquakes. Earthq. Sci. 2011, 24, 549–555. [Google Scholar] [CrossRef][Green Version]
- Triantis, D.; Pasiou, E.D.; Stavrakas, I.; Kourkoulis, S.K. Hidden Affinities Between Electric and Acoustic Activities in Brittle Materials at Near-Fracture Load Levels. Rock Mech. Rock Eng. 2022, 55, 1325–1342. [Google Scholar] [CrossRef]
- Basak, T. The law of life: The bridge between Physics and Biology. Phys. Life Rev. 2011, 8, 249–252. [Google Scholar] [CrossRef]
- Bejan, A.; Lorente, S. Thermodynamic Formulation of the Constructal Law. In Proceedings of the ASME 2003 International Mechanical Engineering Congress and Exposition, Washington, DC, USA, 15–21 November 2003; IMECE2003-41167. pp. 163–172. [Google Scholar] [CrossRef]
- Grmela, M. Multiscale Thermodynamics. Entropy 2021, 23, 165. [Google Scholar] [CrossRef]
- Das, D.; Dutta, S.; Al Mamon, A.; Chakraborty, S. Does fractal universe describe a complete cosmic scenario? Eur. Phys. J. C 2018, 78, 849. [Google Scholar] [CrossRef]
- Benedetti, D. Fractal Properties of Quantum Spacetime. Phys. Rev. Lett. 2009, 102, 111303. [Google Scholar] [CrossRef] [PubMed]
- Hu, B.L. Fractal spacetimes in stochastic gravity?—Views from anomalous diffusion and the correlation hierarchy. IOP Conf. Ser. J. Phys. Conf. Ser. 2017, 880, 012004. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Venegas-Aravena, P.; Cordaro, E.G.; Laroze, D. Natural Fractals as Irreversible Disorder: Entropy Approach from Cracks in the Semi Brittle-Ductile Lithosphere and Generalization. Entropy 2022, 24, 1337. https://doi.org/10.3390/e24101337
Venegas-Aravena P, Cordaro EG, Laroze D. Natural Fractals as Irreversible Disorder: Entropy Approach from Cracks in the Semi Brittle-Ductile Lithosphere and Generalization. Entropy. 2022; 24(10):1337. https://doi.org/10.3390/e24101337
Chicago/Turabian StyleVenegas-Aravena, Patricio, Enrique G. Cordaro, and David Laroze. 2022. "Natural Fractals as Irreversible Disorder: Entropy Approach from Cracks in the Semi Brittle-Ductile Lithosphere and Generalization" Entropy 24, no. 10: 1337. https://doi.org/10.3390/e24101337
APA StyleVenegas-Aravena, P., Cordaro, E. G., & Laroze, D. (2022). Natural Fractals as Irreversible Disorder: Entropy Approach from Cracks in the Semi Brittle-Ductile Lithosphere and Generalization. Entropy, 24(10), 1337. https://doi.org/10.3390/e24101337





