The Evolution Characteristics of Systemic Risk in China’s Stock Market Based on a Dynamic Complex Network
Abstract
1. Introduction
2. Related Works
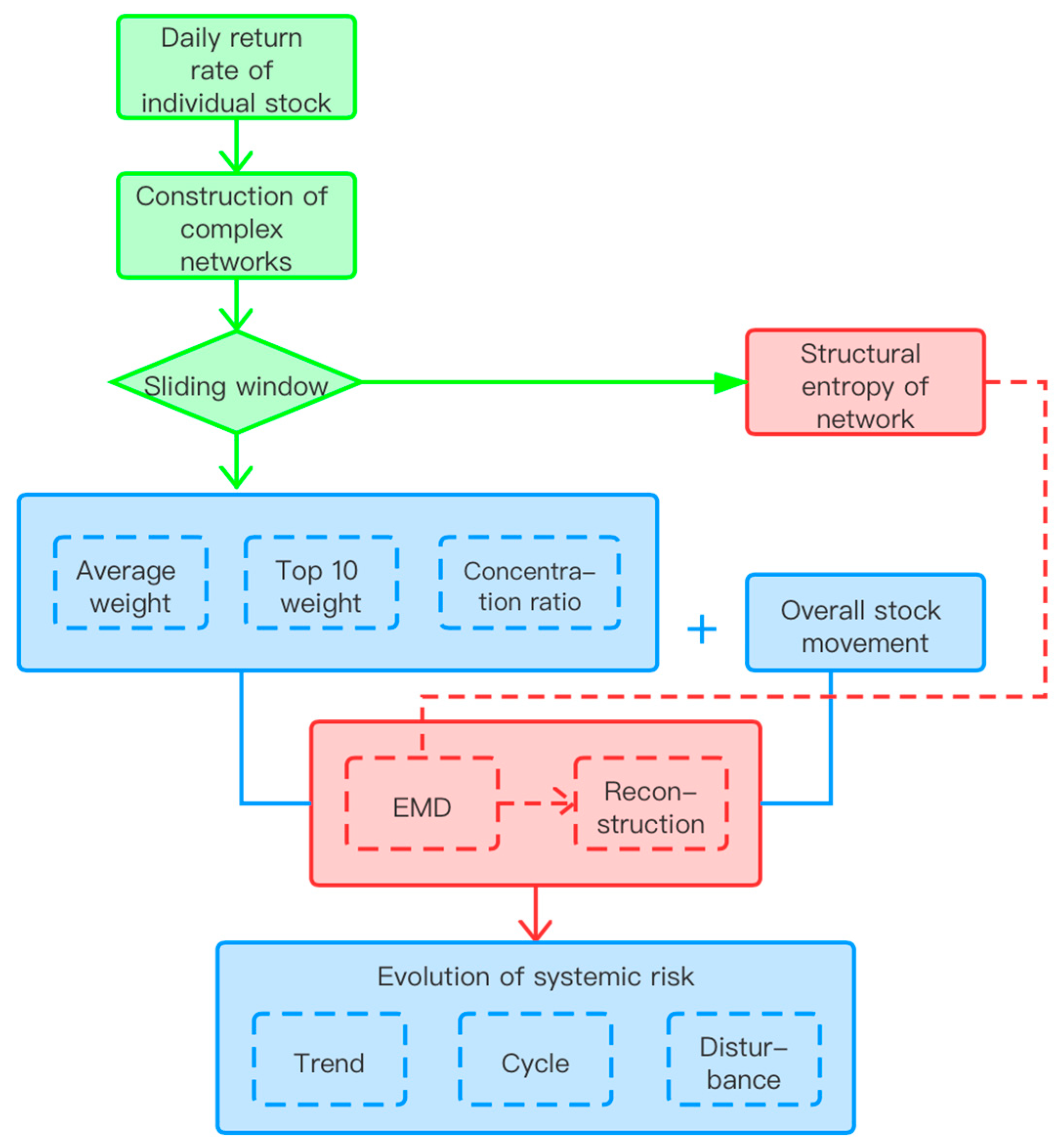
3. Data and Methodology
3.1. Construction of the Complex Network
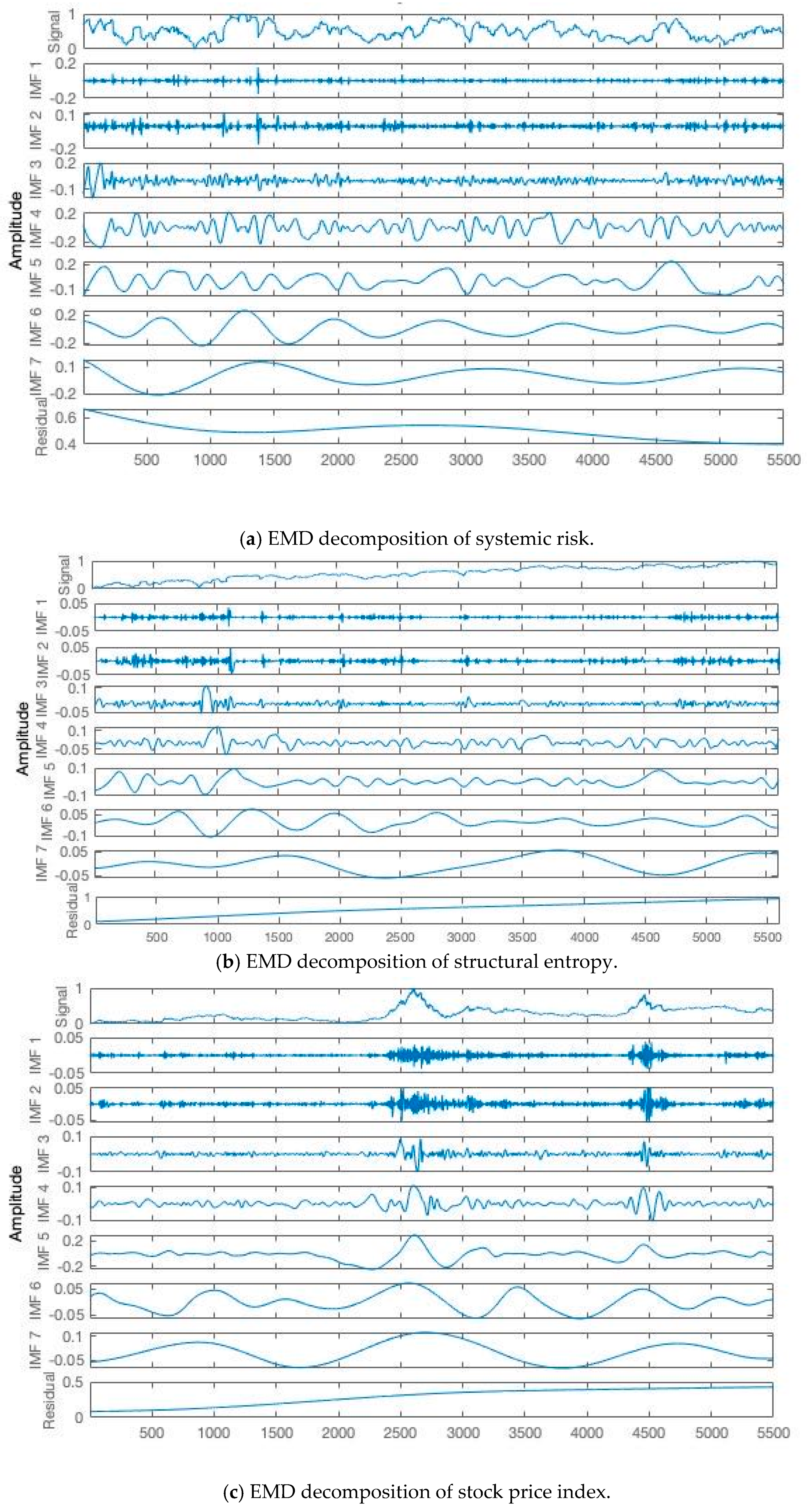
3.2. Empirical Mode Decomposition
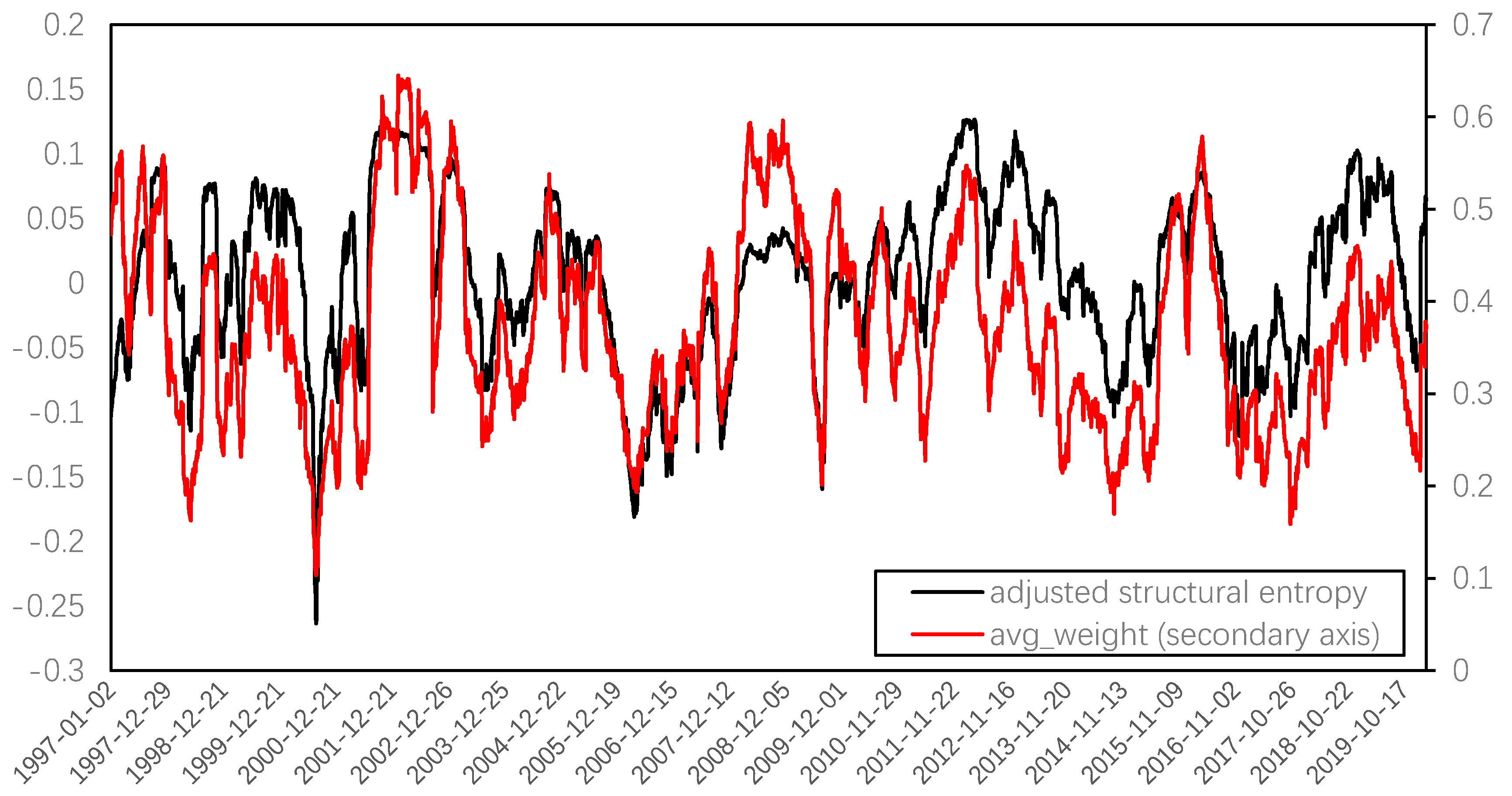
3.3. Grey Relational Analysis
4. Empirical Analysis
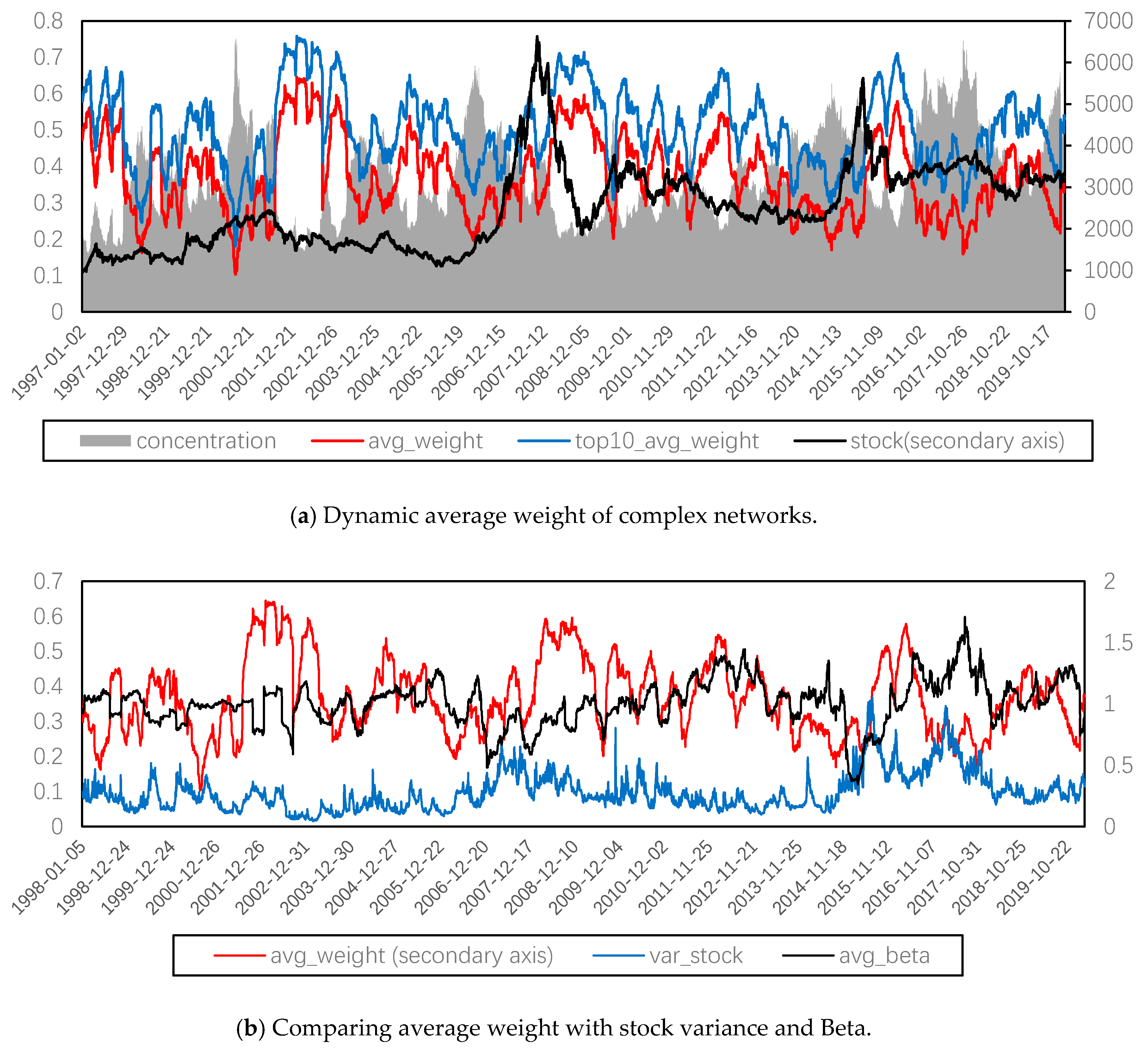
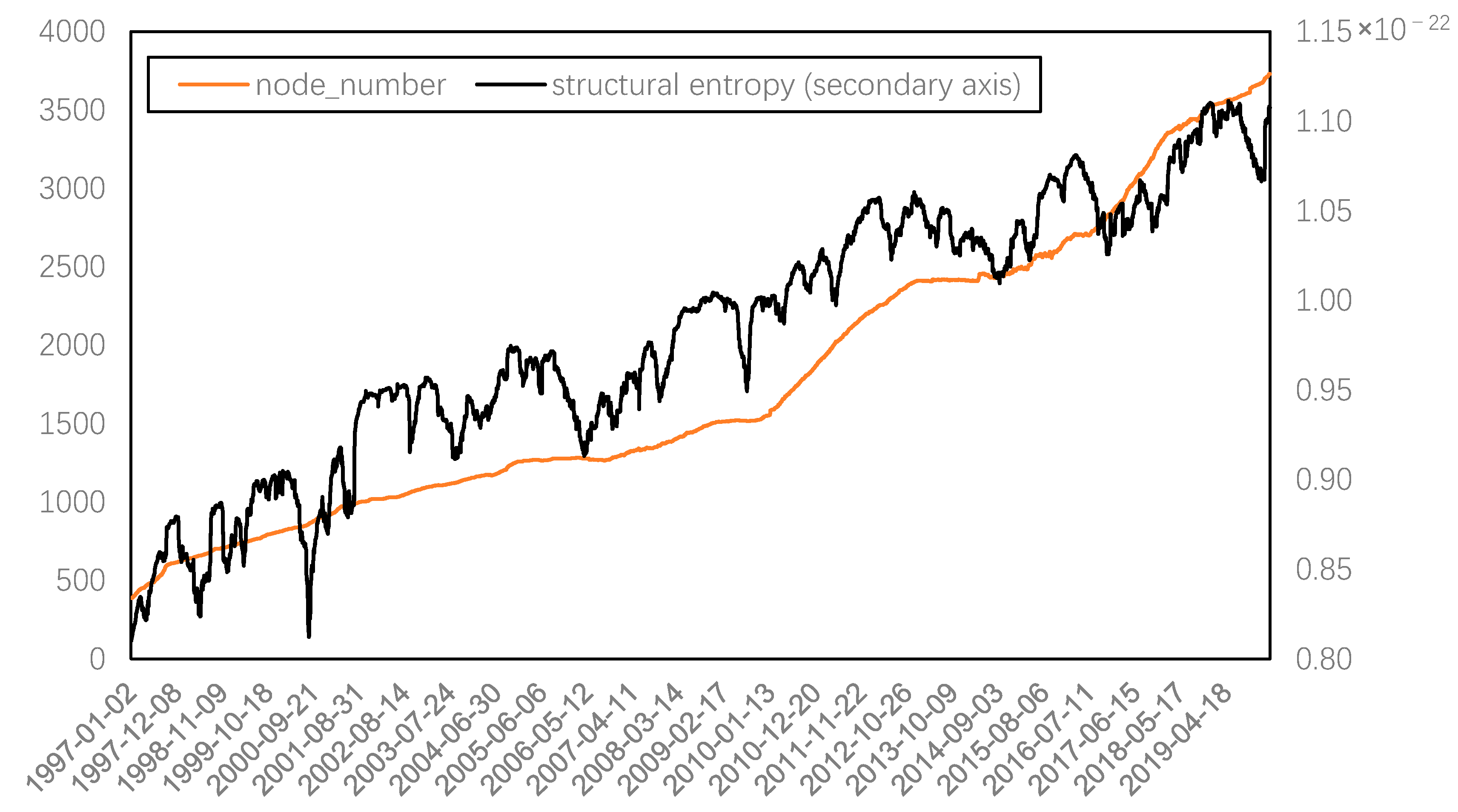
4.1. Dynamic Characteristics of Complex Networks
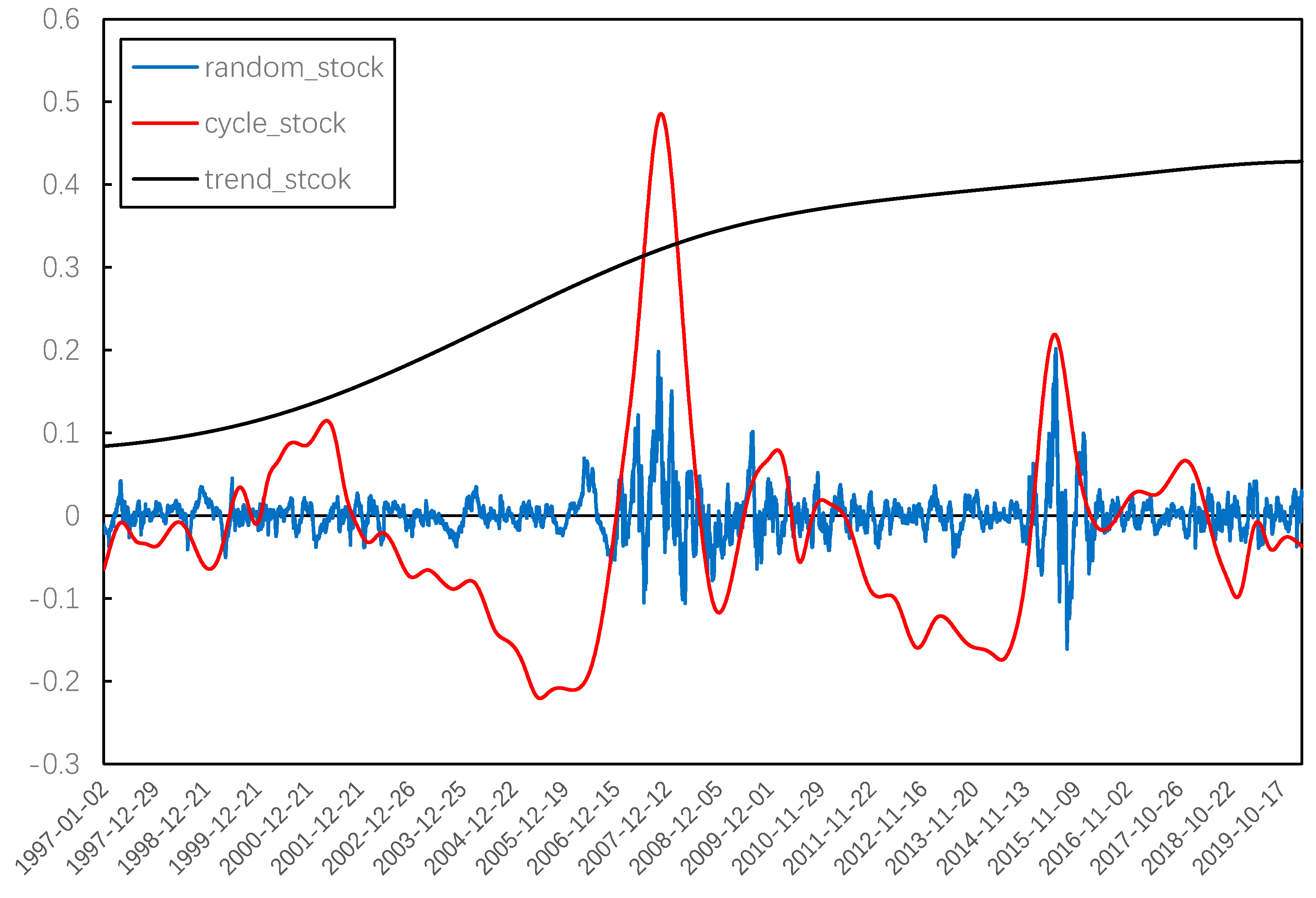
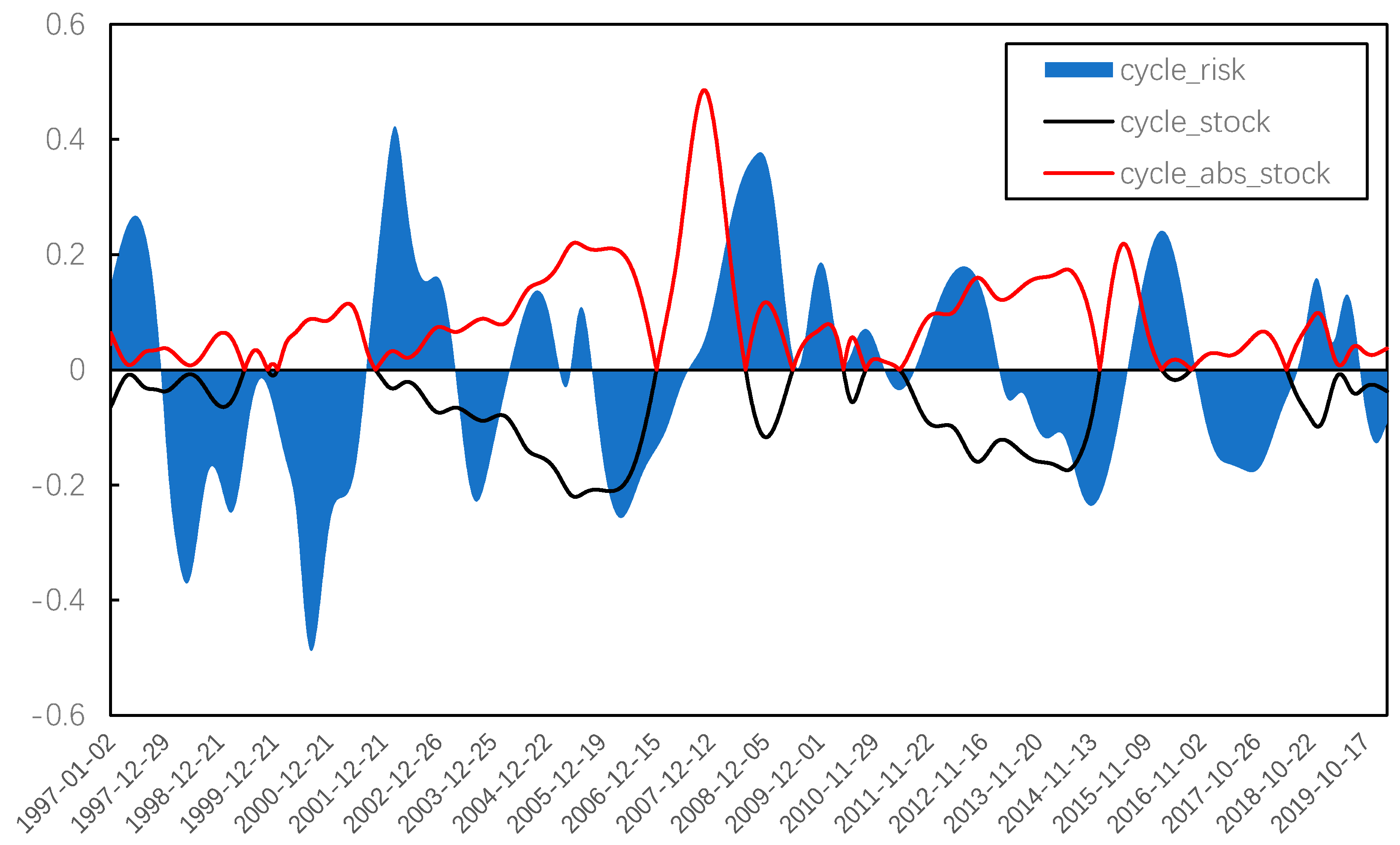
4.2. Decomposition and Reconstructione
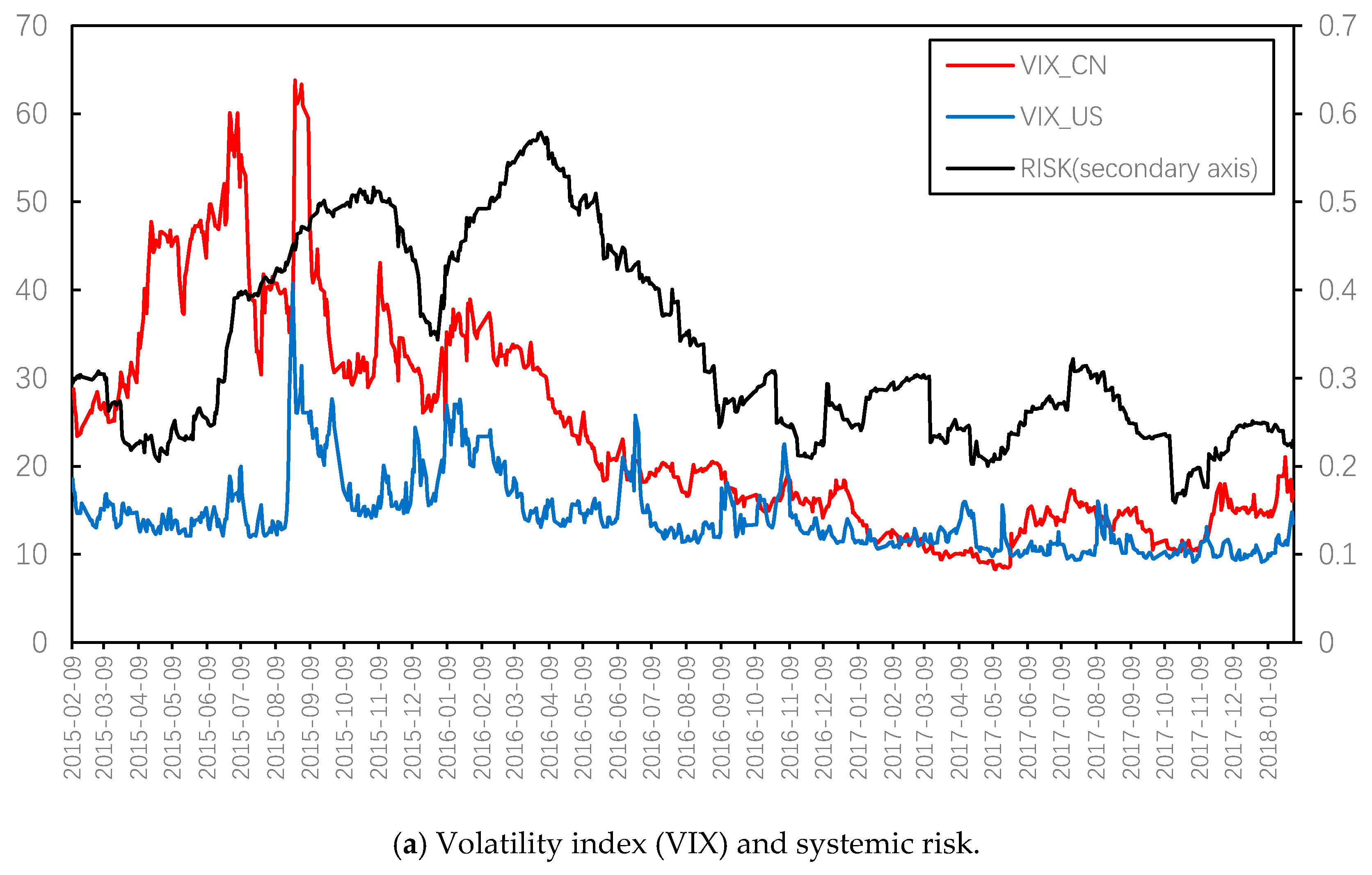
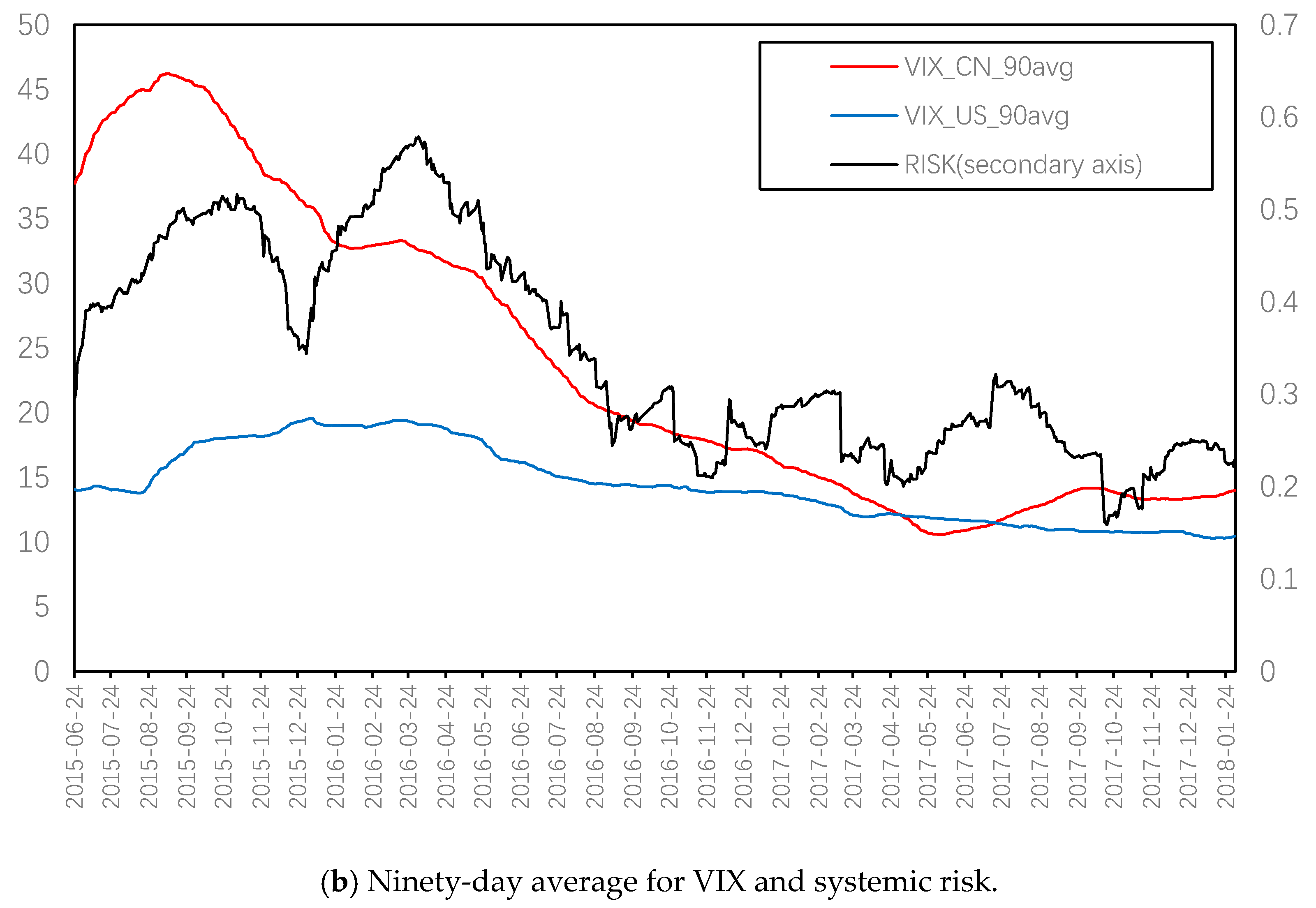
5. Discussion
Author Contributions
Funding
Conflicts of Interest
References
- Scharfstein, D.S.; Stein, J.C. Herd behavior and investment. Am. Econ. Rev. 1990, 80, 4654–4679. [Google Scholar]
- Bikhchandani, S.; Sharma, S. Herd behavior in financial markets. IMF Staff Pap. 2000, 47, 279–310. [Google Scholar]
- Li, X.; Sullivan, R.N.; Garcia, F.L. The low-volatility anomaly: Market evidence on systematic risk vs. mispricing. Financ. Anal. J. 2016, 72, 36–47. [Google Scholar] [CrossRef]
- De, S.R.A. Unobservable systematic risk, economic activity and stock market. J. Bank. Financ. 2018, 97, 51–69. [Google Scholar]
- Price, K.; Price, B.; Nantell, T.J. Variance and lower partial moment measures of systematic risk: Some analytical and empirical results. J. Financ. 1982, 37, 843–855. [Google Scholar] [CrossRef]
- Estrada, J. Systematic risk in emerging markets: The D-CAPM. Emerg. Mark. Rev. 2002, 3, 365–379. [Google Scholar] [CrossRef]
- Caporale, T. Time varying CAPM betas and banking sector risk. Econ. Lett. 2012, 115, 293–295. [Google Scholar] [CrossRef]
- Iqbal, M.J.; Shah, S.Z.A. Determinants of systematic risk. J. Commer. 2012, 4, 47. [Google Scholar]
- Mantegna, R.N.; Stanley, H.E. An Introduction to Econophysics: Correlations and Complexity in Finance; Cambridge University: Cambridge, UK, 1999. [Google Scholar]
- Kwapień, J.; Drożdż, S. Physical approach to complex systems. Phys. Rep. 2012, 515, 115–226. [Google Scholar] [CrossRef]
- Costa, L.F.; Oliveira, J.O.N.; Travieso, G.; Rodrigues, F.A.; Boas, P.R.V.; Antiqueira, L.; Viana, M.R.; Enrique, L. Analyzing. and modeling real-world phenomena with complex networks: A survey of applications. Adv. Phys. 2011, 60, 329–412. [Google Scholar] [CrossRef]
- D’Arcangelis, A.M.; Rotundo, G. Complex networks in finance. Lect. Notes Econ. Math. Cham. 2016, 683, 209–235. [Google Scholar]
- Nagurney, A. Networks in economics and finance in Networks and beyond: A half century retrospective. Networks 2019, 14–15. [Google Scholar] [CrossRef]
- Mantegna, R.N. Hierarchical structure in financial markets. Eur. Phys. J. B 1999, 11, 193–197. [Google Scholar] [CrossRef]
- Onnela, J.P.; Chakraborti, A.; Kaski, K.; Kertesz, J.; Kanto, A. Dynamics of market correlations: Taxonomy and portfolio analysis. Phys. Rev. E 2003, 68, 056110. [Google Scholar] [CrossRef]
- McDonald, M.; Suleman, O.; Williams, S.; Howison, S.; Johnson, N.F. Detecting a currency’s dominance or dependence using foreign exchange network trees. Phys. Rev. E 2005, 72, 046106. [Google Scholar] [CrossRef]
- Zhuang, X.; Min, Z.; Chen, S. Characteristic analysis of complex network for Shanghai Stock Market. J. Northeast. Univ. Nat. Sci. 2007, 28, 1053. [Google Scholar]
- Chi, K.; Liu, T.J.; Lau, C.M.F. A network perspective of the stock market. J. Empir. Financ. 2010, 17, 659–667. [Google Scholar]
- Nobi, A.; Lee, J.W. Systemic risk and hierarchical transitions of financial networks. Chaos 2017, 27, 063107. [Google Scholar] [CrossRef]
- Liao, Z.; Wang, Z.; Guo, K. The dynamic evolution of the characteristics of exchange rate risks in countries along “The Belt and Road” based on network analysis. PLoS ONE 2019, 14. [Google Scholar] [CrossRef]
- Miccichè, S.; Bonanno, G.; Lillo, F.; Mantegna, R.N. Degree stability of a minimum spanning tree of price return and volatility. Physica A 2003, 324, 66–73. [Google Scholar]
- Kwapień, J.; Oświęcimka, P.; Forczek, M.; Drożdż, S. Minimum spanning tree filtering of correlations for varying time scales and size of fluctuations. Phys. Rev. E 2017, 95, 052313. [Google Scholar] [CrossRef]
- Wang, Y.; Zheng, S.; Zhang, W.; Wang, G.; Wang, J. Fuzzy entropy complexity and multifractal behavior of statistical physics financial dynamics. Physica A 2018, 506, 486–498. [Google Scholar] [CrossRef]
- Caraiani, P. Characterizing emerging European stock markets through complex networks: From local properties to self-similar characteristics. Physica A 2012, 391, 3629–3637. [Google Scholar] [CrossRef]
- He, J.; Deem, M.W. Structure and response in the world trade network. Phys. Rev. Lett. 2010, 105, 198701. [Google Scholar] [CrossRef]
- Wang, Z.; Yan, Y.; Chen, X. Time and frequency structure of causal correlation networks in the China bond market. Eur. Phys. J. B 2017, 90, 137. [Google Scholar] [CrossRef]
- Billio, M.; Getmansky, M.; Lo, A.W.; Pelizzon, L. Econometric measures of connectedness and systemic risk in the finance and insurance sectors. J. Financ. Econ. 2012, 104, 535–559. [Google Scholar] [CrossRef]
- Tu, C. Cointegration-based financial networks study in Chinese stock market. Physica A 2014, 402, 245–254. [Google Scholar] [CrossRef]
- Long, H.; Zhang, J.; Tang, N. Does network topology influence systemic risk contribution? A perspective from the industry indices in Chinese stock market. PLoS ONE 2017, 12. [Google Scholar] [CrossRef]
- Kwapień, J.; Gworek, S.; Drożdż, S.; Górski, A. Analysis of a network structure of the foreign currency exchange market. J. Econ. Interact. Coord. 2009, 4, 55. [Google Scholar] [CrossRef]
- Bonanno, G.; Caldarelli, G.; Lillo, F.; Mantegna, R.N. Topology of correlation-based minimal spanning trees in real and model markets. Phys. Rev. E 2003, 68, 046130. [Google Scholar] [CrossRef]
- Konishi, M. A global network of stock markets and home bias puzzle. Appl. Financ. Econ. Lett. 2007, 3, 197–199. [Google Scholar] [CrossRef]
- Lee, K.E.; Lee, J.W.; Hong, B.H. Complex networks in a stock market. Comput. Phys. Commun. 2007, 177, 186. [Google Scholar] [CrossRef]
- Li, P.; Wang, B. An approach to Hang Seng Index in Hong Kong stock market based on network topological statistics. Chin. Sci. Bull. 2006, 51, 624–629. [Google Scholar] [CrossRef]
- Yalamova, R.; McKelvey, B. Explaining what leads up to stock market crashes: A phase transition model and scalability dynamics. J. Behav. Financ. 2011, 12, 169–182. [Google Scholar] [CrossRef]
- Bardoscia, M.; Battiston, S.; Caccioli, F.; Caldarelli, G. Pathways towards instability in financial networks. Nat. Commun. 2017, 8, 14416. [Google Scholar] [CrossRef]
- Lu, K.; Yang, Q.; Chen, G. Singular cycles and chaos in a new class of 3D three-zone piecewise affine systems. Chaos J. Nonlin. Sci. 2019, 29, 043124. [Google Scholar] [CrossRef]
- Deng, J. Introduction to grey system theory. J. Grey Syst. 1989, 1, 1–24. [Google Scholar]
- Mei, Z. The concept and computation method of grey absolute correlation degree. Syst. Eng. 1992, 10, 43–44. [Google Scholar]

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, Y.; Zheng, Y.; Guo, K.; Jin, Z.; Huang, Z. The Evolution Characteristics of Systemic Risk in China’s Stock Market Based on a Dynamic Complex Network. Entropy 2020, 22, 614. https://doi.org/10.3390/e22060614
Shi Y, Zheng Y, Guo K, Jin Z, Huang Z. The Evolution Characteristics of Systemic Risk in China’s Stock Market Based on a Dynamic Complex Network. Entropy. 2020; 22(6):614. https://doi.org/10.3390/e22060614
Chicago/Turabian StyleShi, Yong, Yuanchun Zheng, Kun Guo, Zhenni Jin, and Zili Huang. 2020. "The Evolution Characteristics of Systemic Risk in China’s Stock Market Based on a Dynamic Complex Network" Entropy 22, no. 6: 614. https://doi.org/10.3390/e22060614
APA StyleShi, Y., Zheng, Y., Guo, K., Jin, Z., & Huang, Z. (2020). The Evolution Characteristics of Systemic Risk in China’s Stock Market Based on a Dynamic Complex Network. Entropy, 22(6), 614. https://doi.org/10.3390/e22060614




