Entropy Generation and Thermoelastic Damping in the In-plane Vibration of Microring Resonators
Abstract
:1. Introduction
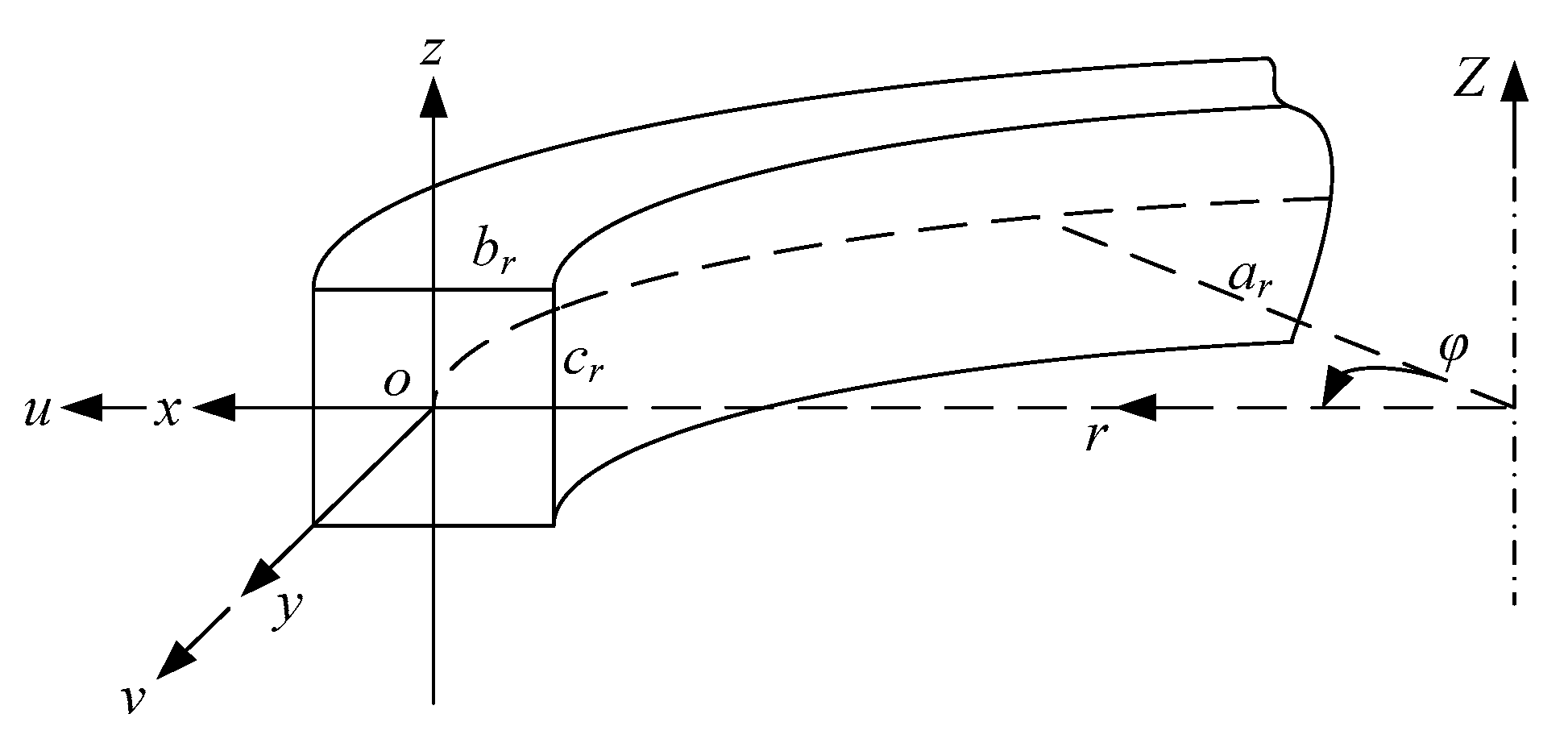
2. Problem Formulation
2.1. Heat Conduction Governing Equation
2.2. Entropy Generation
2.3. Thermoelastic Damping
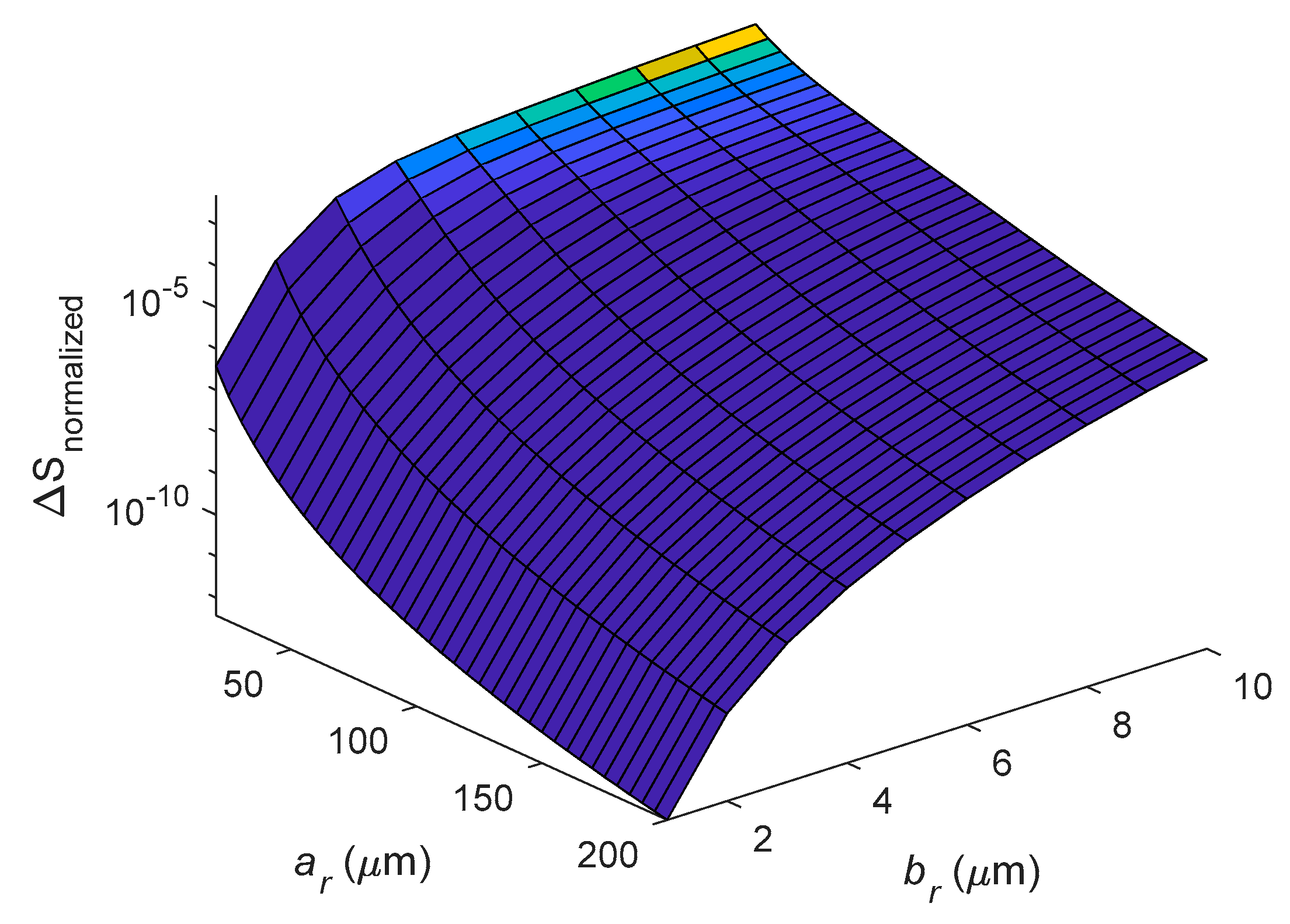
3. Results and Discussions
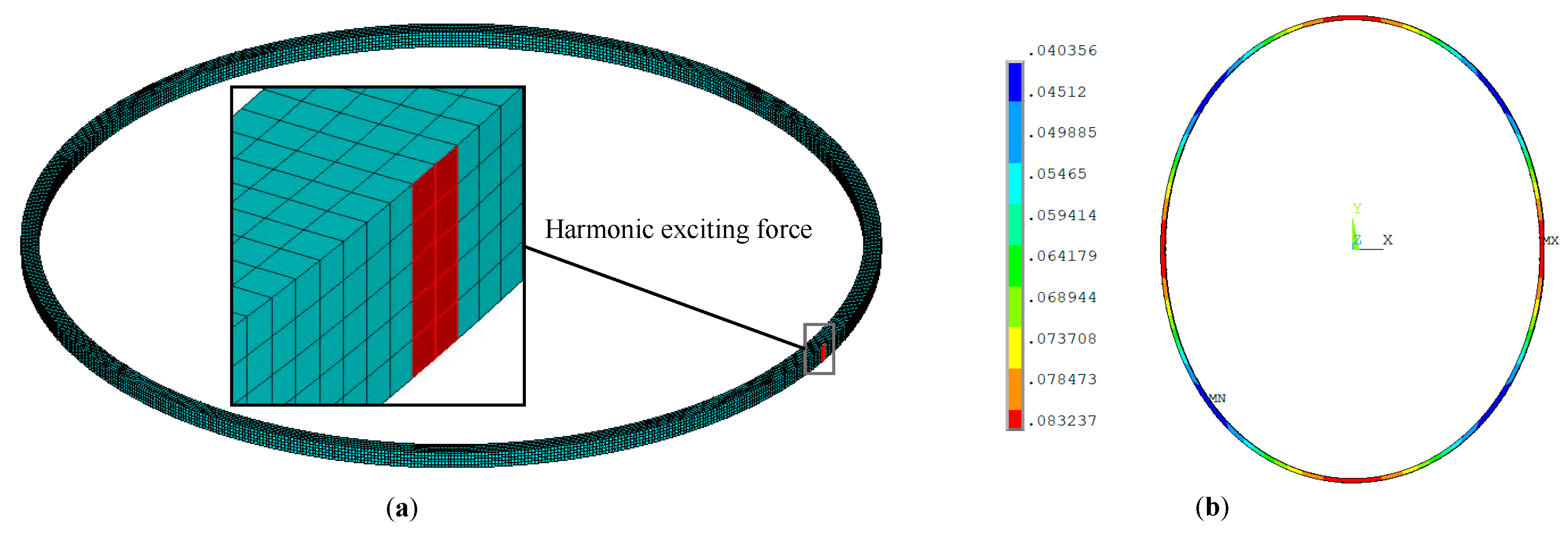
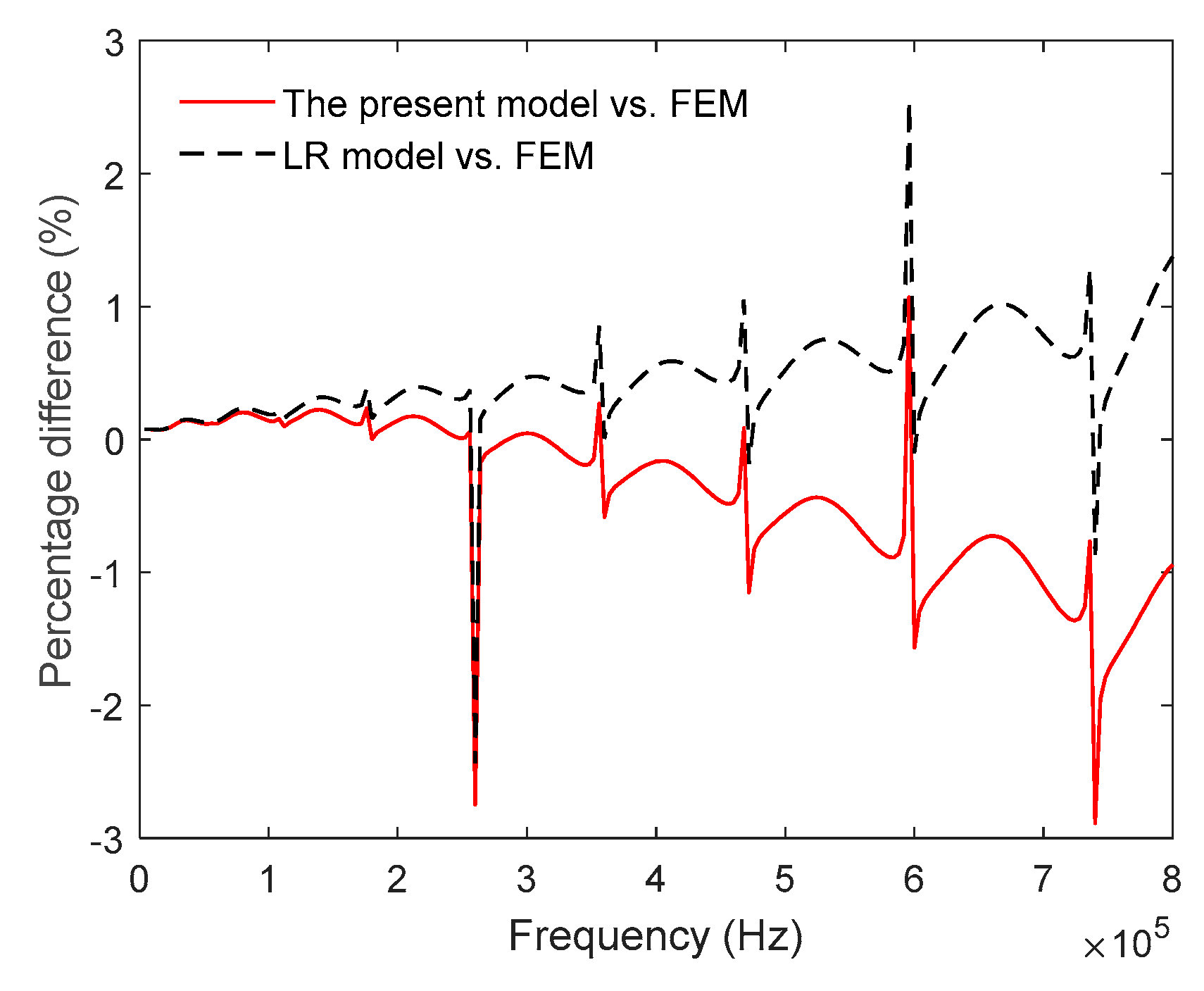
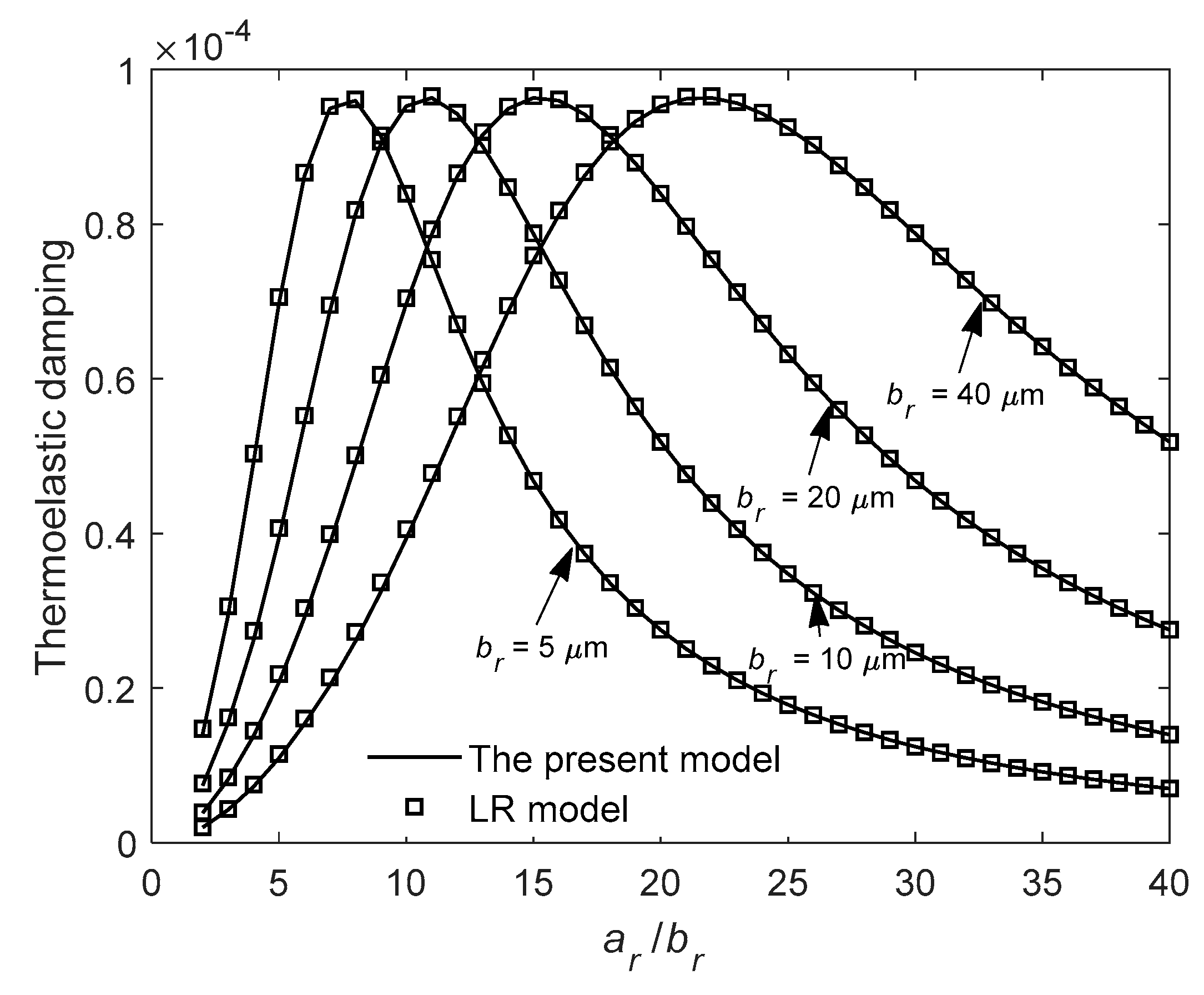
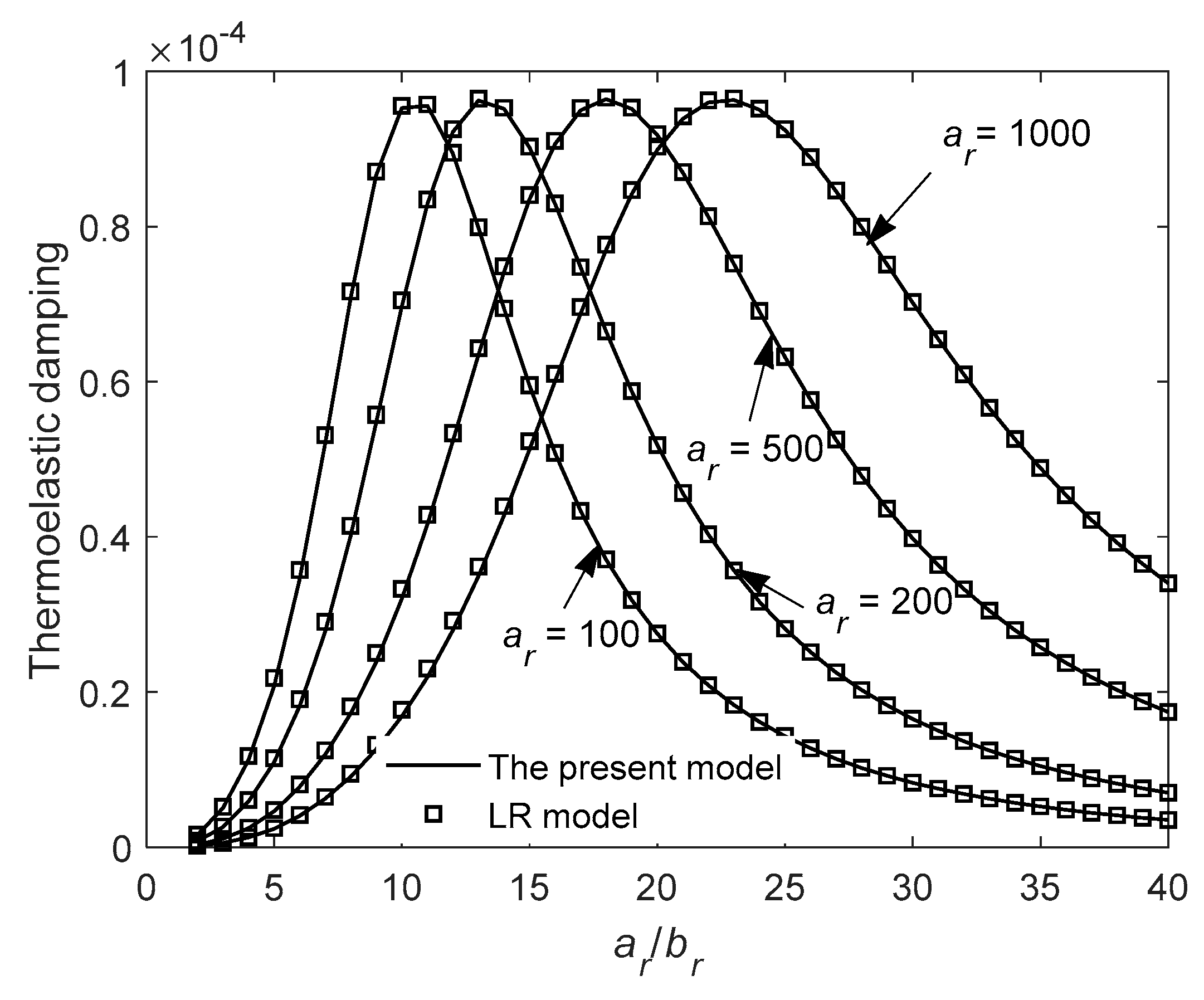
3.1. Verification of the Present Model
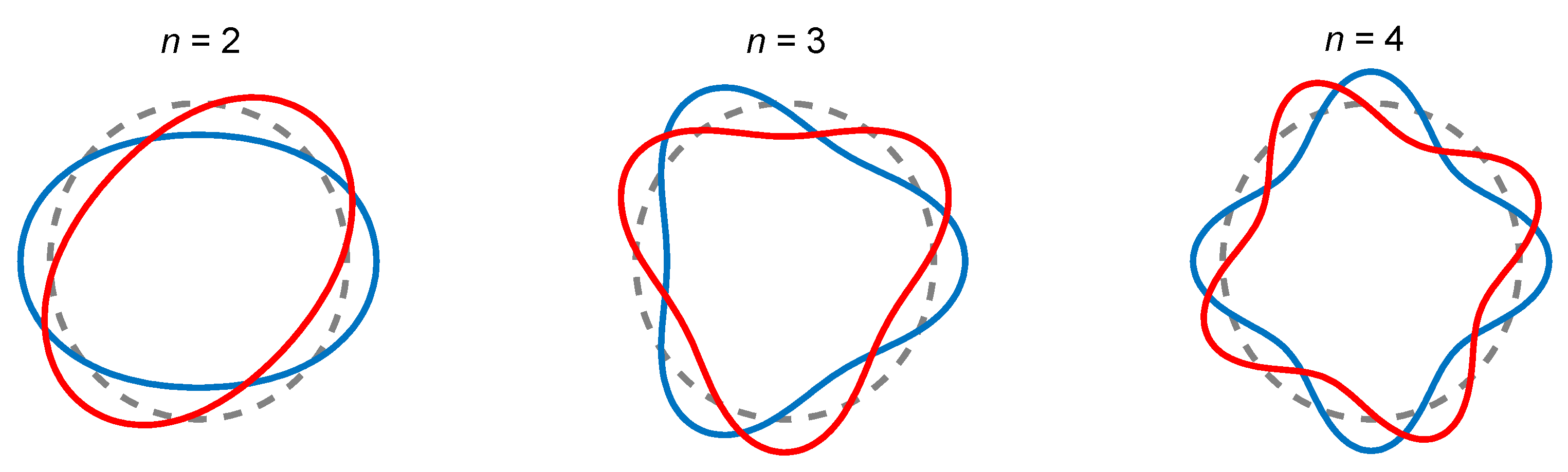
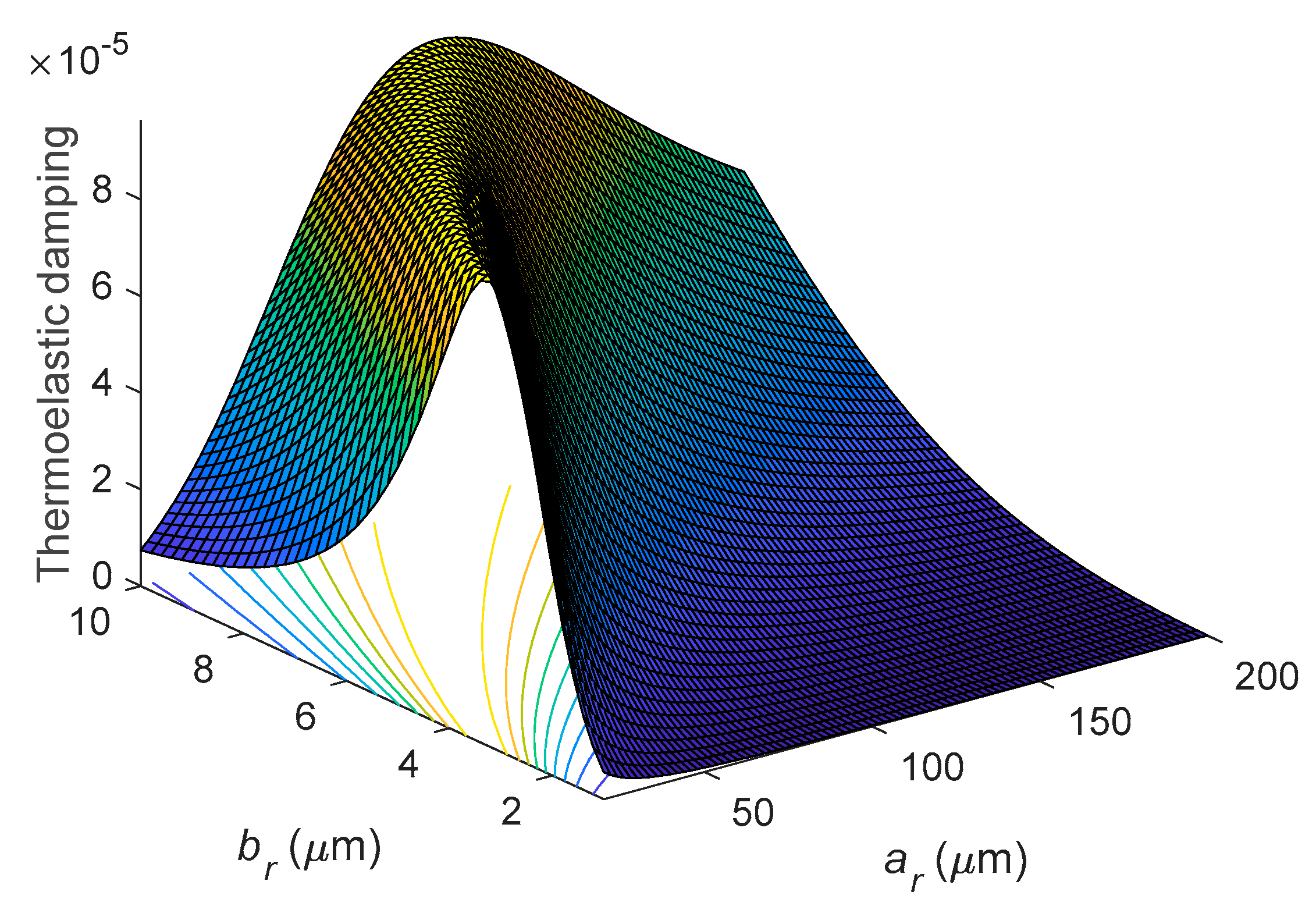
3.2. Geometry effect on TED
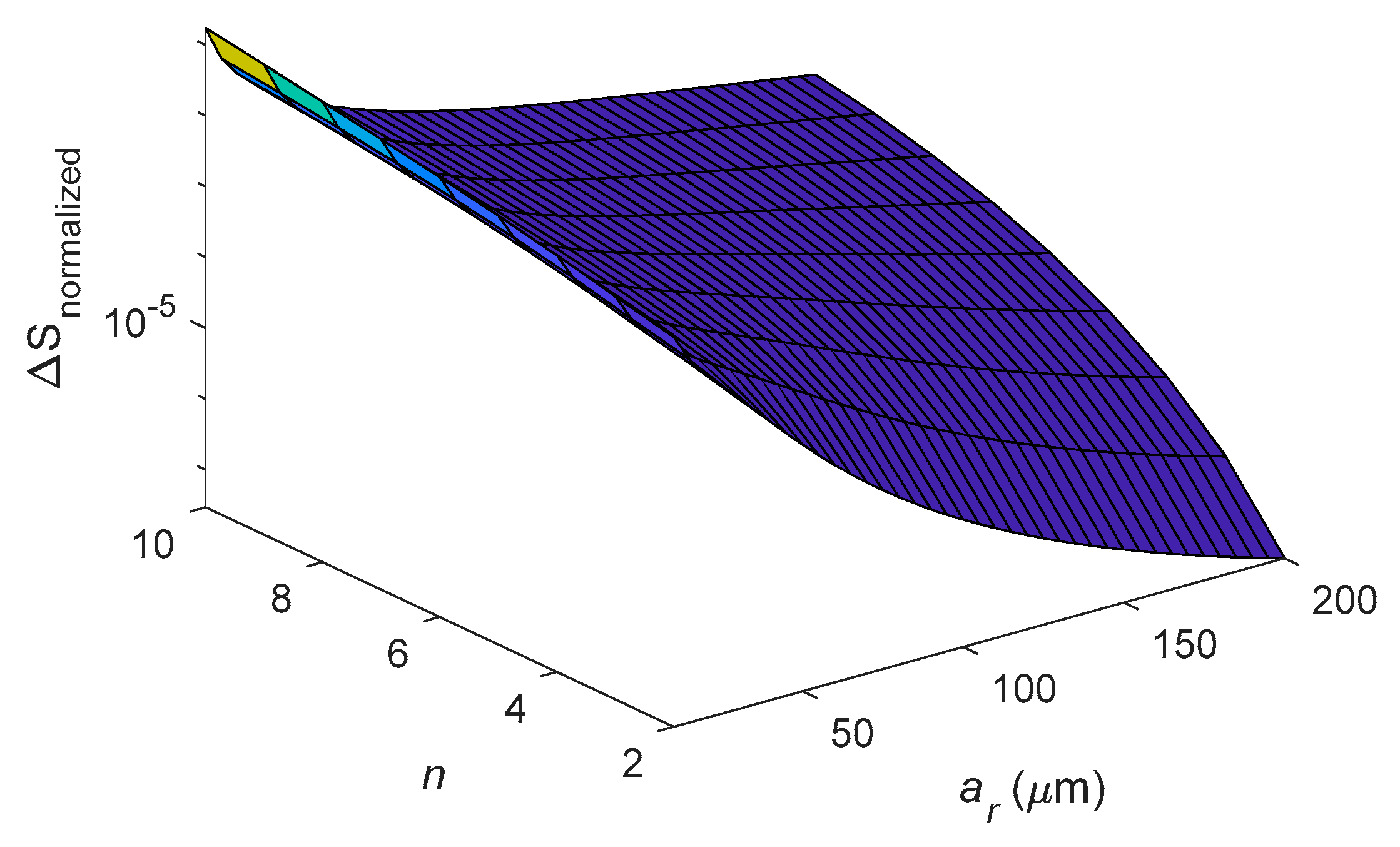
3.3. Characteristics of Entropy Generation
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix
References
- Rourke, A.K.; McWilliam, S.; Fox, C.H.J. Frequency trimming of a vibrating ring-based multi-axis rate sensor. J. Sound Vib. 2005, 280, 495–530. [Google Scholar] [CrossRef]
- Eley, R.; Fox, C.H.J.; McWilliam, S. CORIOLIS COUPLING EFFECTS ON THE VIBRATION OF ROTATING RINGS. J. Sound Vib. 2000, 238, 459–480. [Google Scholar] [CrossRef]
- Eley, R.; Fox, C.H.J.; Mcwilliam, S. The dynamics of a vibrating-ring multi-axis rate gyroscope. J. Mech. Eng. Sci. 2000, 214, 1503–1513. [Google Scholar] [CrossRef]
- Li, M.-H.; Chen, C.-Y.; Li, C.-S.; Chin, C.-H.; Li, S.-S. Design and Characterization of a Dual-Mode CMOS-MEMS Resonator for TCF Manipulation. J. Microelectromech. S. 2015, 24, 446–457. [Google Scholar] [CrossRef]
- Zener, C. Internal Friction in Solids. I. Theory of Internal Friction in Reeds. Phys. Rev. 1937, 52, 230–235. [Google Scholar] [CrossRef]
- Zener, C. Internal Friction in Solids II. General Theory of Thermoelastic Internal Friction. Phys. Rev. 1938, 53, 90–99. [Google Scholar] [CrossRef]
- Lifshitz, R.; Roukes, M.L. Thermoelastic damping in micro- and nanomechanical systems. Phys. Rev. B 2000, 61, 5600–5609. [Google Scholar] [CrossRef] [Green Version]
- Zhou, H.Y.; Li, P.; Fang, Y.M. Thermoelastic damping in circular cross-section micro/nanobeam resonators with single-phase-lag time. Int. J. Mech. Sci. 2018, 142, 583–594. [Google Scholar] [CrossRef]
- Wong, S.J.; Fox, C.H.J.; McWilliam, S. Thermoelastic damping of the in-plane vibration of thin silicon rings. J. Sound Vib. 2006, 293, 266–285. [Google Scholar] [CrossRef]
- Zuo, W.; Li, P.; Du, J.; Huang, J. Thermoelastic damping in trilayered microplate resonators. Int. J. Mech. Sci. 2019, 151, 595–608. [Google Scholar] [CrossRef]
- Darvishian, A.; Nagourney, T.; Cho, J.Y.; Shiari, B.; Najafi, K. Thermoelastic Dissipation in Micromachined Birdbath Shell Resonators. J. Microelectromech. S. 2017, 26, 758–772. [Google Scholar] [CrossRef]
- Nayfeh, A.H.; Younis, M.I. Modeling and simulations of thermoelastic damping in microplates. J. Micromech. Microeng. 2004, 14, 1711–1717. [Google Scholar] [CrossRef]
- Hao, Z. Thermoelastic damping in the contour-mode vibrations of micro- and nano-electromechanical circular thin-plate resonators. J. Sound Vib. 2008, 313, 77–96. [Google Scholar] [CrossRef]
- Sun, Y.X.; Tohmyoh, H. Thermoelastic damping of the axisymmetric vibration of circular plate resonators. J. Sound Vib. 2009, 319, 392–405. [Google Scholar] [CrossRef]
- Li, P.; Fang, Y.; Hu, R. Thermoelastic damping in rectangular and circular microplate resonators. J. Sound Vib. 2012, 331, 721–733. [Google Scholar] [CrossRef]
- Hossain, S.T.; Mcwilliam, S.; Popov, A.A. An investigation on thermoelastic damping of high-Q ring resonators. Int. J. Mech. Sci. 2015, 106, 209–219. [Google Scholar] [CrossRef]
- Fang, Y.M.; Li, P. Thermoelastic damping in thin microrings with two-dimensional heat conduction. Phys. E 2015, 69, 198–206. [Google Scholar] [CrossRef]
- Wong, S.J.; Fox, C.H.J.; McWilliam, S.; Fell, C.P.; Eley, R. A preliminary investigation of thermo-elastic damping in silicon rings. J. Micromech. Microeng. 2004, 14, S108–S113. [Google Scholar] [CrossRef]
- Li, P.; Fang, Y.M.; Zhang, J.R. Thermoelastic damping in microrings with circular cross-section. J. Sound Vib. 2016, 361, 341–354. [Google Scholar] [CrossRef]
- Kim, J.-H.; Kim, J.-H. Thermoelastic damping effect of the micro-ring resonator with irregular mass and stiffness. J. Sound Vib. 2016, 369, 168–177. [Google Scholar] [CrossRef]
- Tai, Y.; Chen, N. Thermoelastic damping in the out-of-plane vibration of a microring resonator with rectangular cross-section. Int. J. Mech. Sci. 2019, 151, 684–691. [Google Scholar] [CrossRef]
- Tai, Y.; Li, P. An Analytical Model for Thermoelastic Damping in Microresonators Based on Entropy Generation. J. Vib. Acoust. 2014, 136. [Google Scholar] [CrossRef]
- Kinra, V.K.; Milligan, K.B. A 2nd-Law Analysis of Thermoelastic Damping. J. Appl. Mech-T Asme 1994, 61, 71–76. [Google Scholar] [CrossRef]
- Bishop, J.E.; Kinra, V.K. Elastothermodynamic damping in laminated composites. Int. J. Solids Struct. 1997, 34, 1075–1092. [Google Scholar] [CrossRef]
- Chandorkar, S.A.; Candler, R.N.; Duwel, A.; Melamud, R.; Agarwal, M.; Goodson, K.E.; Kenny, T.W. Multimode thermoelastic dissipation. J. Appl. Phys. 2009, 105, 043505. [Google Scholar] [CrossRef] [Green Version]
- Hao, Z.L.; Xu, Y.; Durgam, S.K. A thermal-energy method for calculating thermoelastic damping in micromechanical resonators. J. Sound Vib. 2009, 322, 870–882. [Google Scholar] [CrossRef]
- Soedel, W. Vibrations of Shells and Plates; Marcel Dekker: New York, NY, USA, 1993. [Google Scholar]
- Boley, B.A.; Weiner, J.H. Theory of Thermal Stresses; Wiley: New York, NY, USA, 1960. [Google Scholar]
- Prabhakar, S.; Vengallatore, S. Theory of thermoelastic damping in micromechanical resonators with two-dimensional heat conduction. J. Microelectromech. S. 2008, 17, 494–502. [Google Scholar] [CrossRef]
- Duwel, A.; Candler, R.N.; Kenny, T.W.; Varghese, M. Engineering MEMS resonators with low thermoelastic damping. J. Microelectromech. S. 2006, 15, 1437–1445. [Google Scholar] [CrossRef]



| Model | Expression | Features | Simplification |
|---|---|---|---|
| Zener model | τ = b2/(π2χ) | • Modal superposition temperature field • The first thermal mode is dominant. • Method of mechanical work loss or heat increment | High order thermal modes |
| LR model | • Complex temperature field • Method of mechanical work loss | Complex resonant frequency or thermal stress | |
| The present model | • Complex temperature field • Method of heat increment related to entropy generation | Temperature |
| Parameters | Polysilicon |
|---|---|
| Young’s modulus, E (GPa) | 157 |
| Poisson’s ratio, υ | 0.22 |
| Density, ρ (kg m−3) | 2330 |
| Thermal conductivity, κ (W m−1 K−1) | 90 |
| Specific heat, Cp (J kg−1 K−1) | 699 |
| Thermal expansion coefficient, α (K−1) | 2.6 × 10−6 |
| ar (mm) | br (μm) | Mode (n = 2) (kHz) | Q−1 | % Error | |
|---|---|---|---|---|---|
| Measured [18] | The Present Model | ||||
| 3 | 120 | 13.8 | 9.5238 × 10−5 | 9.2843 × 10−5 | −2.51 |
| 3 | 117 | 13.49 | 1.0000 × 10−5 | 9.5178 × 10−5 | −4.82 |
| 2 | 50 | 12.97 | 4.1667 × 10−5 | 4.5396 × 10−5 | 8.95 |
| 2 | 52 | 13.49 | 4.5455 × 10−5 | 5.0340 × 10−5 | 10.75 |
| 2 | 38 | 9.85 | 2.0833 × 10−5 | 2.0830 × 10−5 | −0.01 |
| Item | Value | ||||
|---|---|---|---|---|---|
| Temperature (K) | 240 | 258 | 298 | 320 | 348 |
| α (×10−6 K−1) | 1.99 | 2.24 | 2.60 | 2.85 | 3.06 |
| Cv (×10−6 J m−3 K−1) | 1.51 | 1.52 | 1.64 | 1.68 | 1.73 |
| χ (×10−5 m2 s−1) | 14.3 | 11.7 | 8.60 | 7.92 | 6.97 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tai, Y.; Li, P.; Zheng, Y.; Tian, J. Entropy Generation and Thermoelastic Damping in the In-plane Vibration of Microring Resonators. Entropy 2019, 21, 631. https://doi.org/10.3390/e21070631
Tai Y, Li P, Zheng Y, Tian J. Entropy Generation and Thermoelastic Damping in the In-plane Vibration of Microring Resonators. Entropy. 2019; 21(7):631. https://doi.org/10.3390/e21070631
Chicago/Turabian StyleTai, Yongpeng, Pu Li, Yan Zheng, and Jie Tian. 2019. "Entropy Generation and Thermoelastic Damping in the In-plane Vibration of Microring Resonators" Entropy 21, no. 7: 631. https://doi.org/10.3390/e21070631
APA StyleTai, Y., Li, P., Zheng, Y., & Tian, J. (2019). Entropy Generation and Thermoelastic Damping in the In-plane Vibration of Microring Resonators. Entropy, 21(7), 631. https://doi.org/10.3390/e21070631




