Adaptive Synchronization Strategy between Two Autonomous Dissipative Chaotic Systems Using Fractional-Order Mittag–Leffler Stability
Abstract
1. Introduction
2. Fractional-Order Chaotic System Description and Chaotic Behavior Analysis
2.1. The Fractional Calculus and the Mittag–Leffler Stability Theorem
2.2. Description of the Two Fractional-Order Chaotic Systems
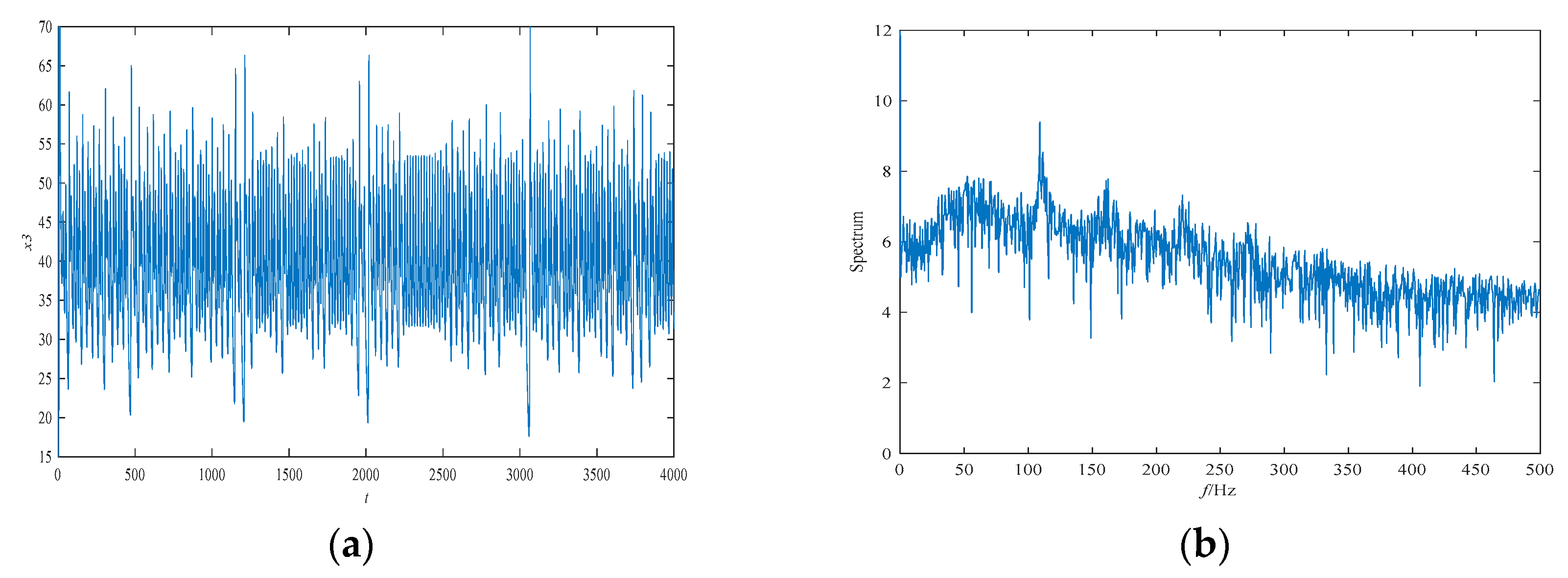
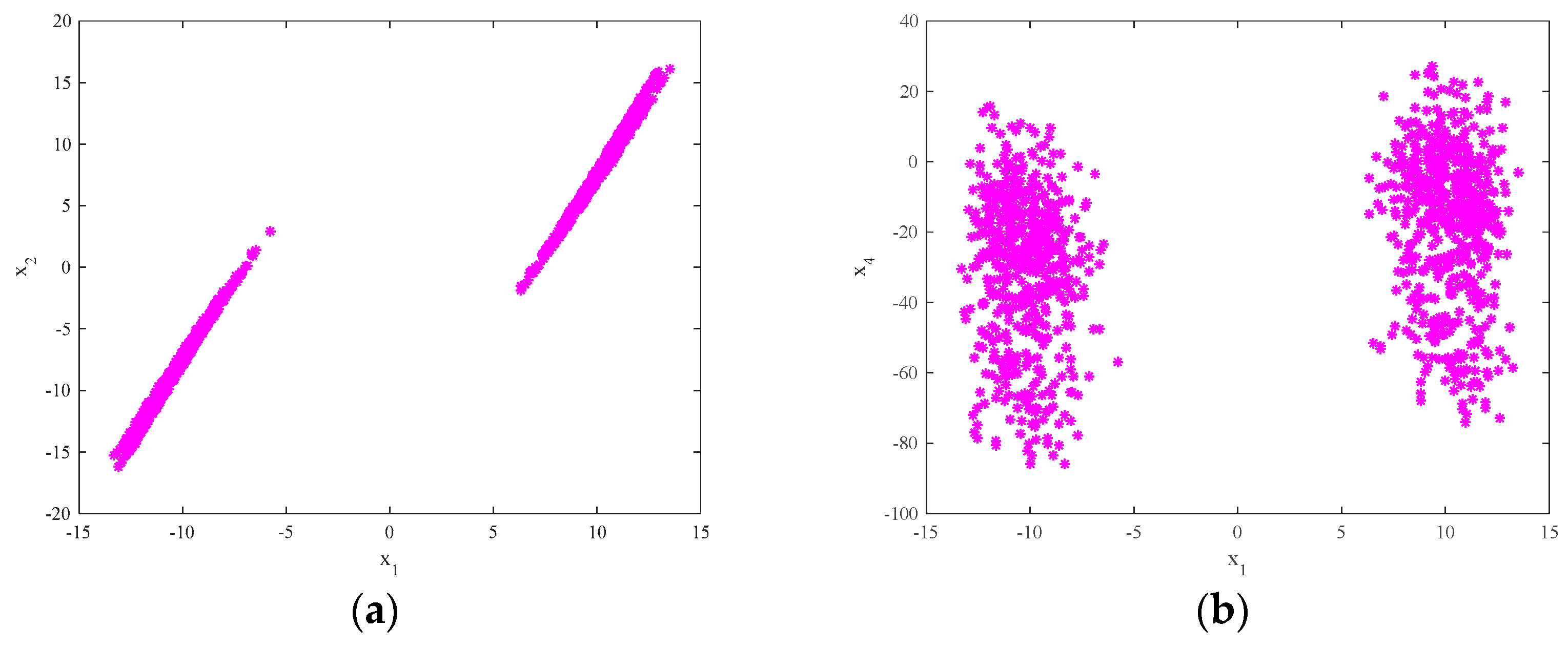
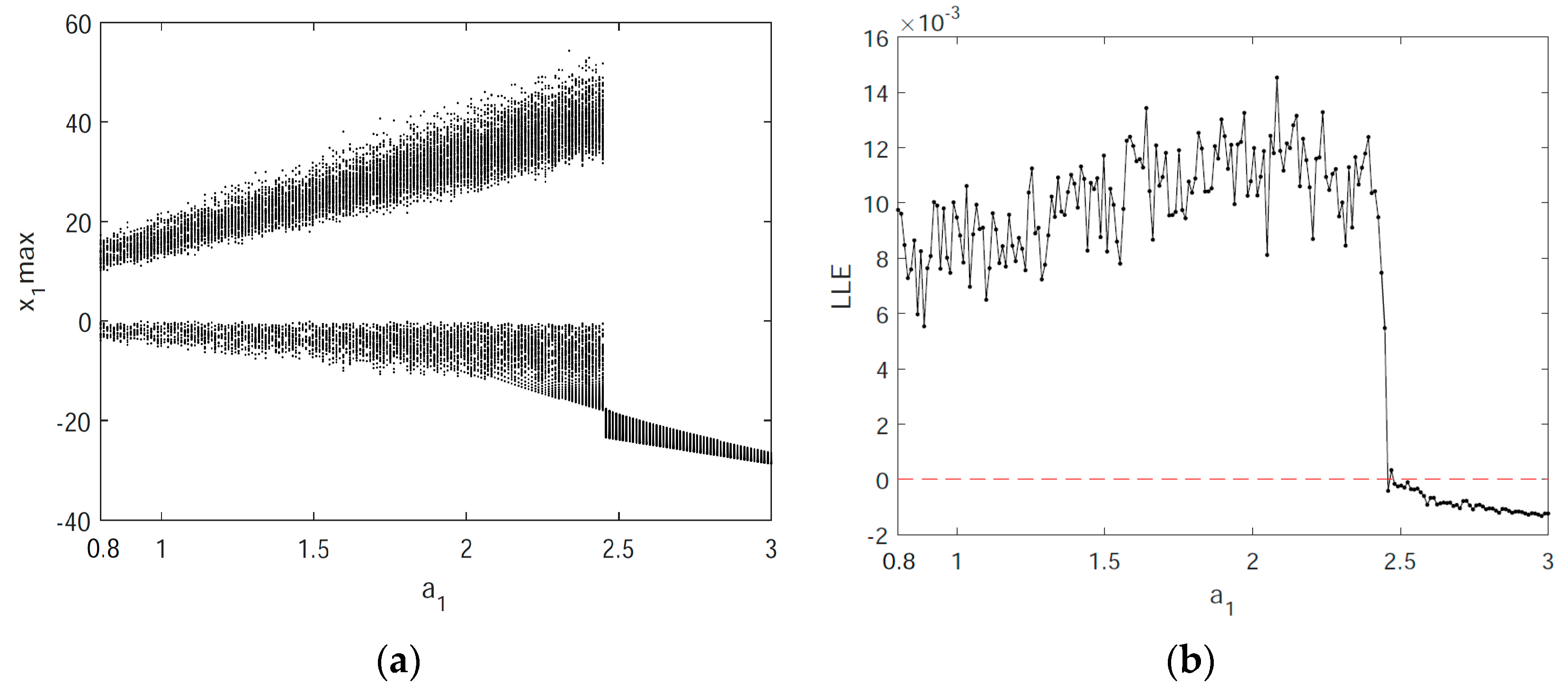
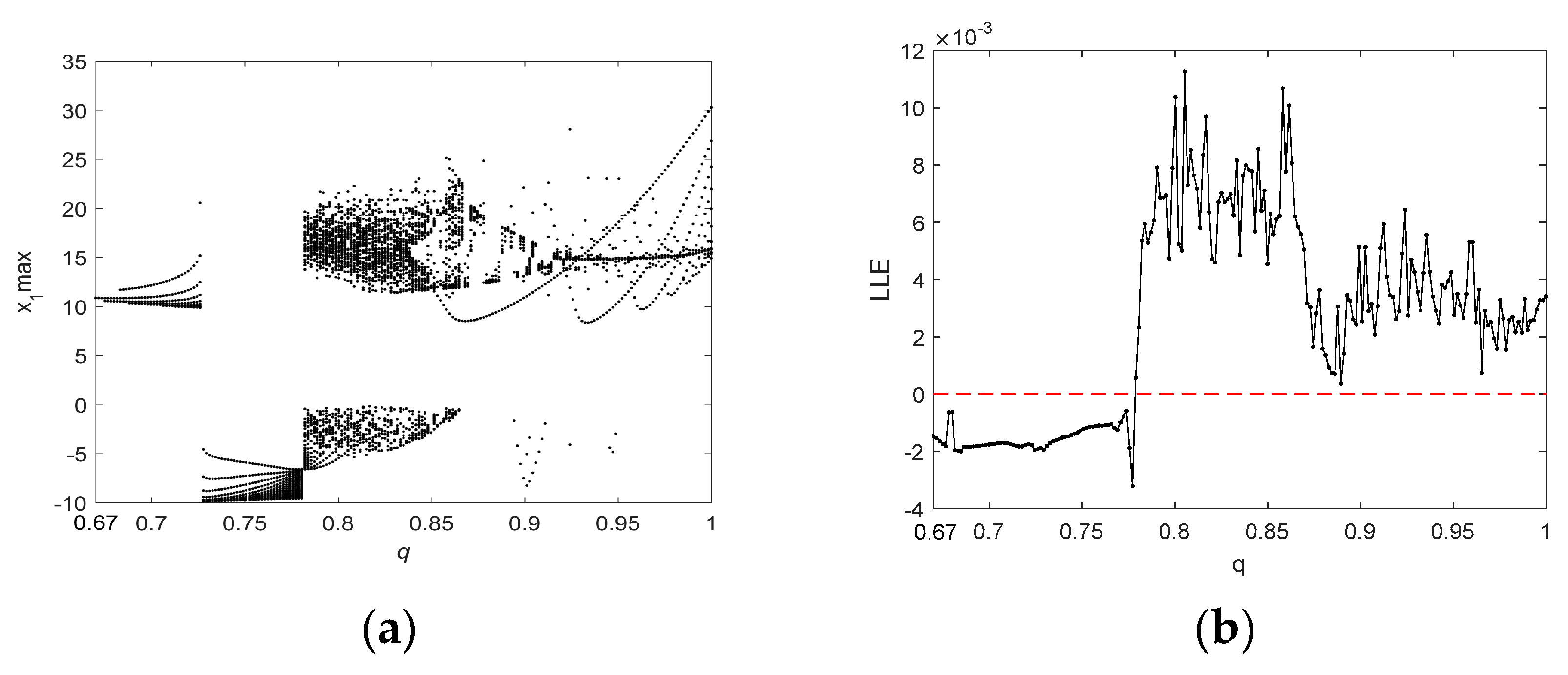
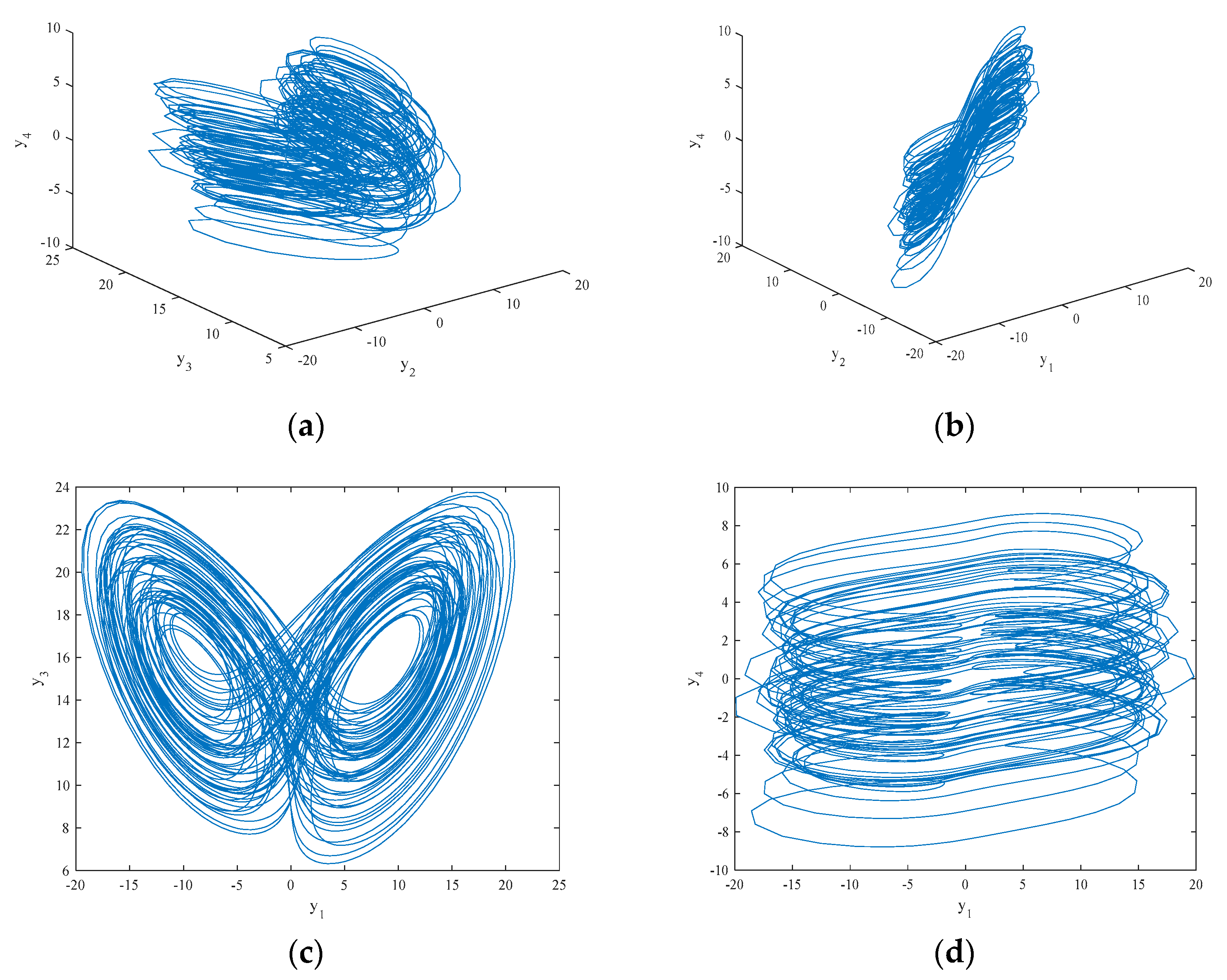
2.3. Analysis of the Chaotic Behavior in the Two Fractional-Order Chaotic Systems
3. Entropy Analysis
3.1. Description of the Complexity Algorithm
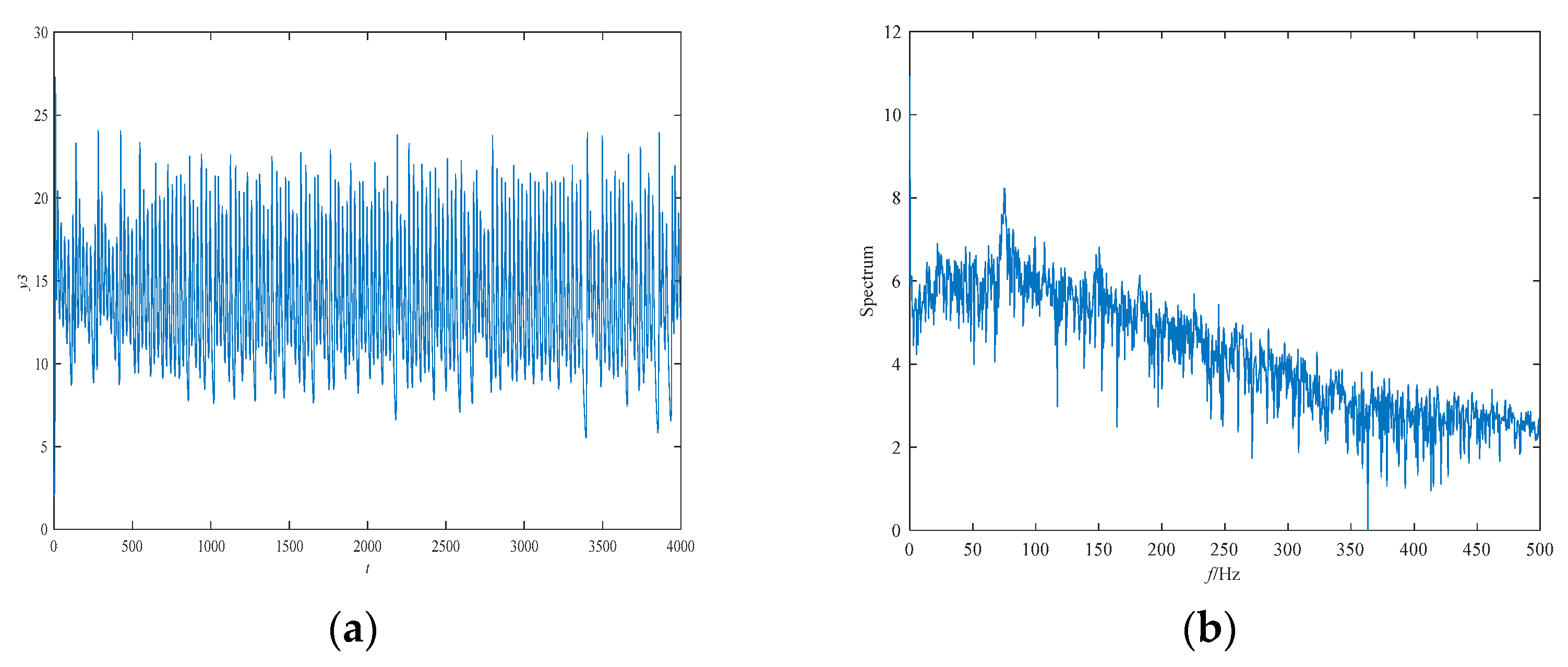
3.2. Influence of the Number of Simulation Points on Entropy
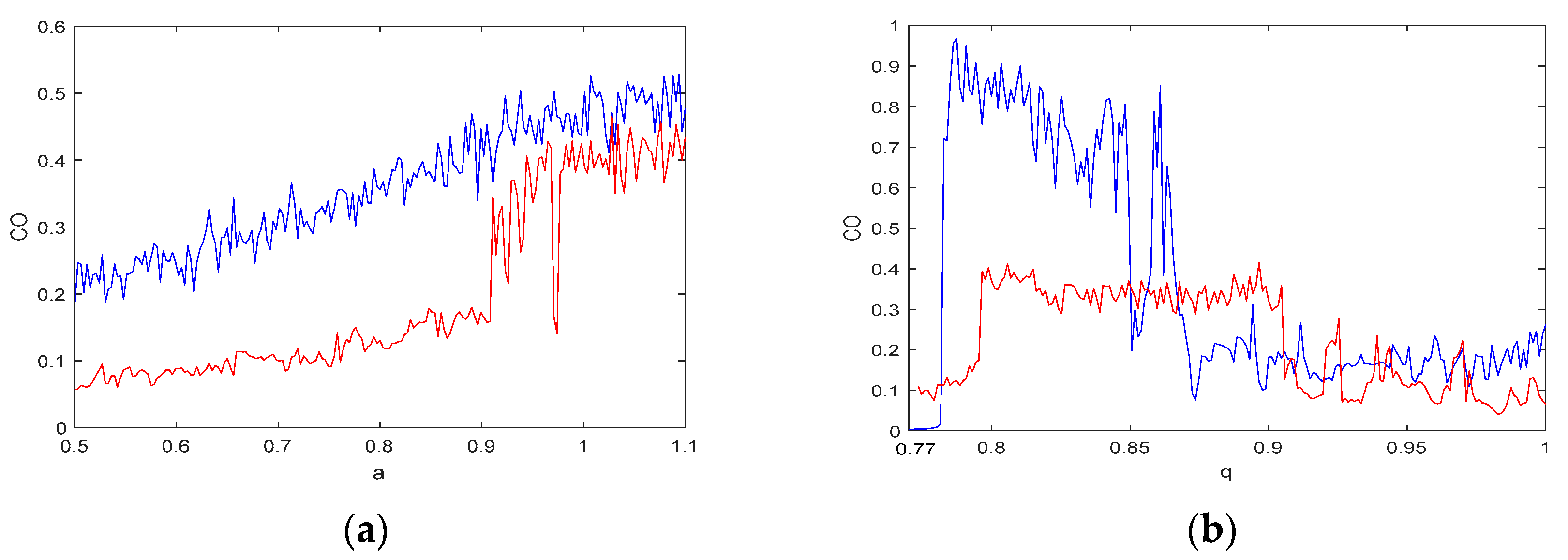
3.3. Influence of System Parameters on Entropy
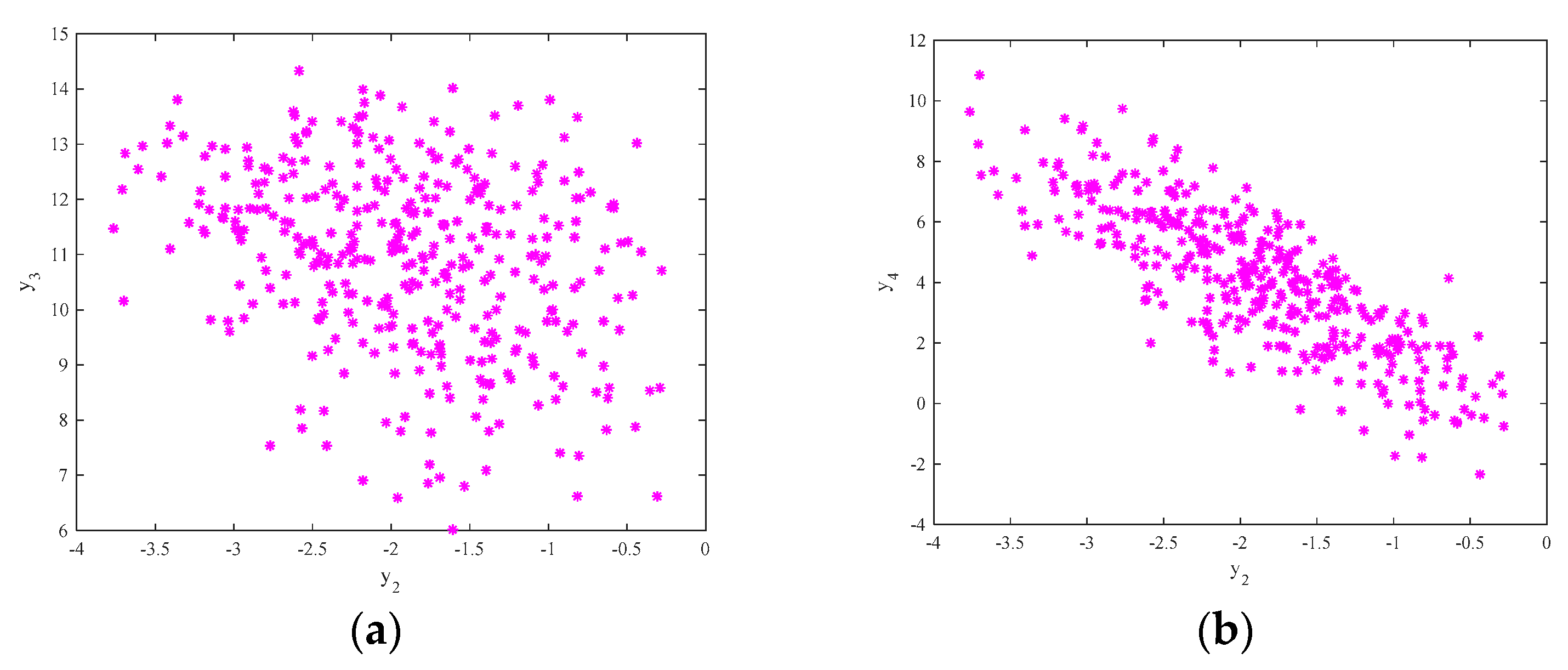
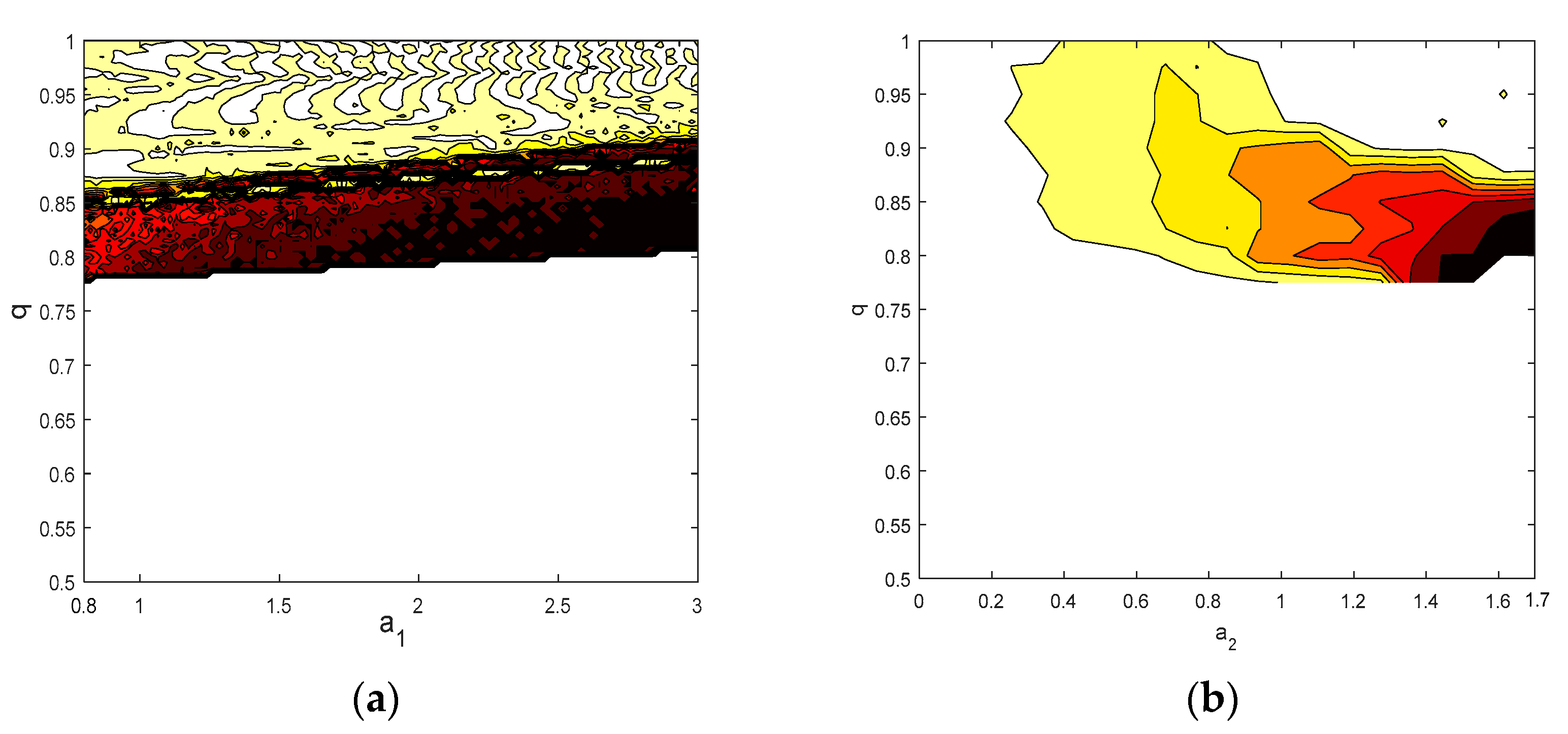
3.4. Analysis of the Chaotic Diagram of System Entropy
4. Adaptive Synchronization of Fractional-Order Systems
4.1. Adaptive Synchronization for Determined Parameters
4.2. Adaptive Synchronization for Uncertain Parameters
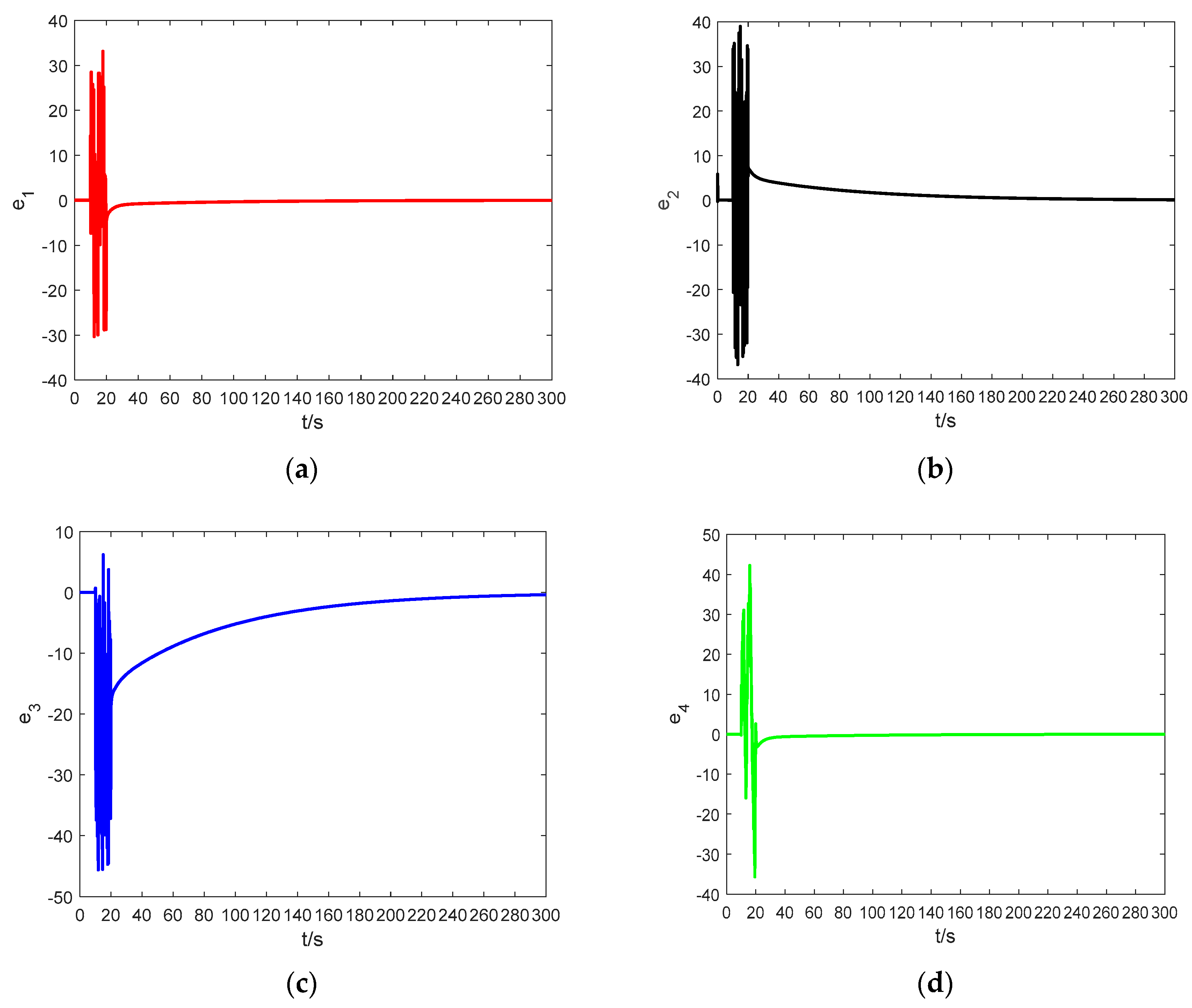
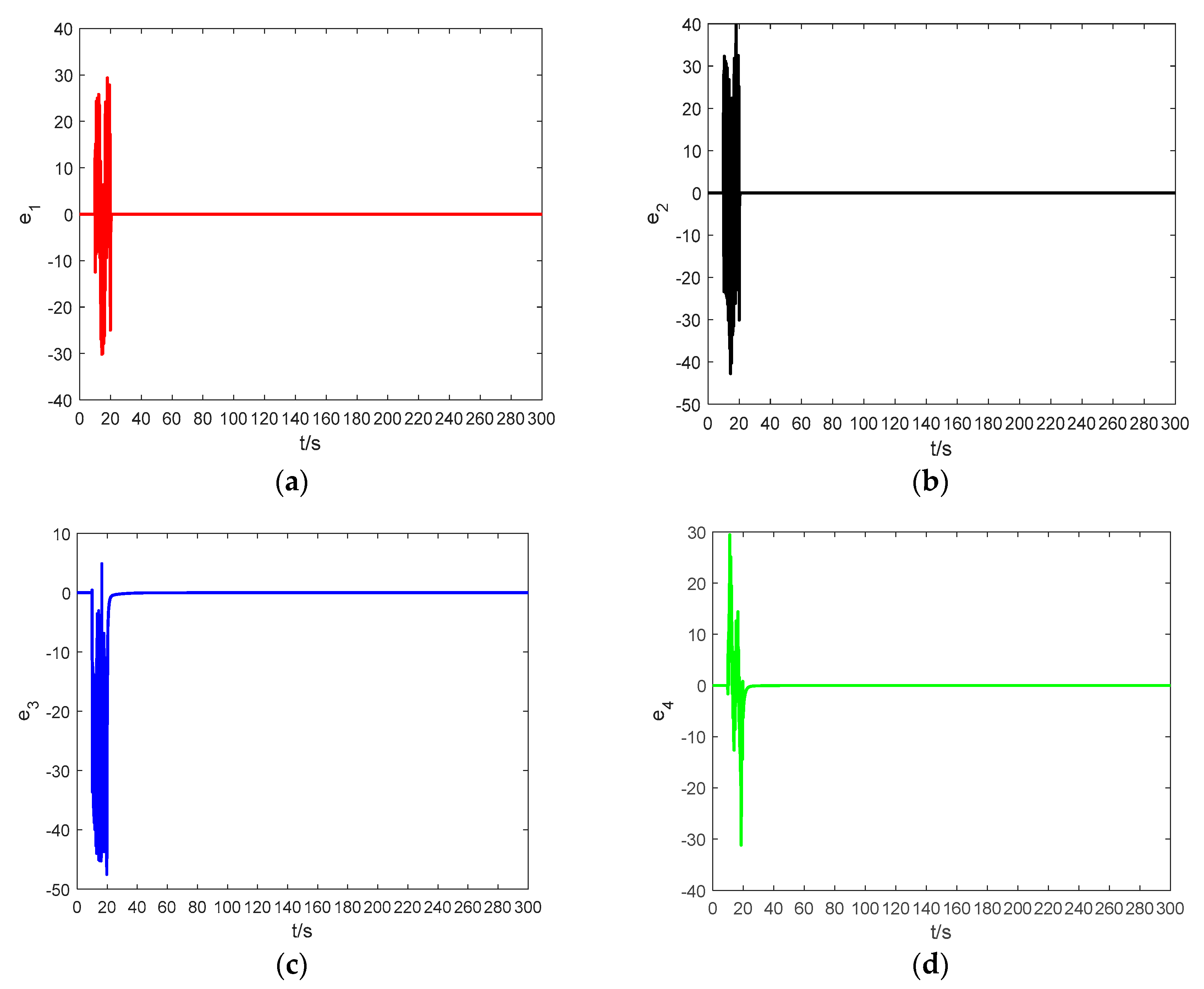
4.3. Numerical Simulation
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Mischaikow, K.; Mrozek, M. Chaos in the Lorenz Equations: A Computer Assisted Proof. Part II: Details. Math. Comput. 1998, 67, 1023–1046. [Google Scholar] [CrossRef]
- Lorenz, E.N. Deterministic nonperiodic flow. J. Atmos. Sci. 1963, 20, 130–141. [Google Scholar] [CrossRef]
- Stewart, I.I. Mathematics. The Lorenz attractor exists. Nature 2000, 406, 948–949. [Google Scholar] [CrossRef] [PubMed]
- Ueta, T.; Chen, G. Bifurcation analysis of chen’s equation. Int. J. Bifurc. Chaos 2000, 10, 1917–1931. [Google Scholar] [CrossRef]
- Liu, C.; Liu, T.; Liu, L.; Liu, K. A new chaotic attractor. Chaos Solitons Fractals 2004, 22, 1031–1038. [Google Scholar] [CrossRef]
- Rössler, O.E. An equation for continuous chaos. Phys. Lett. A 1976, 57, 397–398. [Google Scholar] [CrossRef]
- Xu, G.; Shekofteh, Y.; Akgül, A.; Li, C.; Panahi, S. A New Chaotic System with a Self-Excited Attractor: Entropy Measurement, Signal Encryption, and Parameter Estimation. Entropy 2018, 20, 86. [Google Scholar] [CrossRef]
- Lai, Q.; Akgul, A.; Li, C.; Xu, G.; Çavuşoğlu, Ü. A New Chaotic System with Multiple Attractors: Dynamic Analysis, Circuit Realization and S-Box Design. Entropy 2017, 20, 12. [Google Scholar] [CrossRef]
- Leonov, G.A.; Kuznetsov, N.V.; Mokaev, T.N. Homoclinic orbits, and self-excited and hidden attractors in a Lorenz-like system describing convective fluid motion. Eur. Phys. J. Spec. Top. 2015, 224, 1421–1458. [Google Scholar] [CrossRef]
- Piper, J.R.; Sprott, J.C. Simple Autonomous Chaotic Circuits. IEEE Trans. Circuits Syst. II Express Briefs 2010, 57, 730–734. [Google Scholar] [CrossRef]
- Duane, G.S. Synchronized chaos in extended systems and meteorological teleconnections. Phys. Rev. E 1997, 56, 6475–6493. [Google Scholar] [CrossRef]
- Tsonis, A.A.; Elsner, J.B. Chaos, Strange Attractors, and Weather. Bull. Am. Meteorol. Soc. 2010, 70, 14–23. [Google Scholar] [CrossRef]
- Peterka, F.; Vacík, J. Transition to chaotic motion in mechanical systems with impacts. J. Sound Vib. 1992, 154, 95–115. [Google Scholar] [CrossRef]
- Jalnine, A.Y.; Kuznetsov, S.P. Autonomous strange nonchaotic oscillations in a system of mechanical rotators. Regul. Chaotic Dyn. 2017, 22, 210–225. [Google Scholar] [CrossRef]
- Fiori, S.; Di Filippo, R. An Improved Chaotic Optimization Algorithm Applied to a DC Electrical Motor Modeling. Entropy 2017, 19, 665. [Google Scholar] [CrossRef]
- Hussein, A.M.; Rahma, T.F.; Al-Suhail, G.A.; Thanh, P.V. An adaptive observer synchronization using chaotic time-delay system for secure communication. Nonlinear Dyn. 2017, 90, 2583–2598. [Google Scholar]
- Pano-Azucena, A.D.; de Jesus Rangel-Magdaleno, J.; Tlelo-Cuautle, E.; de Jesus Quintas-Valles, A. Arduino-based chaotic secure communication system using multi-directional multi-scroll chaotic oscillators. Nonlinear Dyn. 2016, 87, 2203–2217. [Google Scholar] [CrossRef]
- Hyun, C.H.; Park, C.W.; Kim, J.H.; Park, M. Synchronization and secure communication of chaotic systems via robust adaptive high-gain fuzzy observer. Chaos Solitons Fractals 2009, 40, 2200–2209. [Google Scholar] [CrossRef]
- Fiori, S. Non-delayed synchronization of non-autonomous dynamical systems on Riemannian manifolds and its applications. Nonlinear Dyn. 2018, 94, 3077–3100. [Google Scholar] [CrossRef]
- Lu, J.G. Chaotic dynamics of the fractional-order Lü system and its synchronization. Phys. Lett. A 2006, 354, 305–311. [Google Scholar] [CrossRef]
- Megherbi, O.; Hamiche, H.; Djennoune, S.; Bettayeb, M. A new contribution for the impulsive synchronization of fractional-order discrete-time chaotic systems. Nonlinear Dyn. 2017, 90, 1519–1533. [Google Scholar] [CrossRef]
- Danca, M.F. Hidden chaotic attractors in fractional-order systems. Nonlinear Dyn. 2017, 89, 577–586. [Google Scholar] [CrossRef]
- Luo, C.; Wang, X. Chaos Generated from the Fractional-Order Complex Chen System and Its Application to Digital Secure Communication. Int. J. Mod. Phys. C 2013, 24. [Google Scholar] [CrossRef]
- Pecora, L.M.; Carroll, T.L. Synchronization in chaotic systems. Phys. Rev. Lett. 1996, 6, 821–824. [Google Scholar]
- Carroll, T.L.; Pecora, L.M. Synchronizing chaotic circuits. IEEE Trans. Circuits Syst. Video Technol. 2002, 38, 453–456. [Google Scholar] [CrossRef]
- Vepa, R. Active–passive decomposition with application to arrays of chaotic systems. Int. J. Bifurcation Chaos 2001, 11, 1593–1606. [Google Scholar] [CrossRef]
- Li, D.; Lu, J.A.; Wu, X. Linearly coupled synchronization of the unified chaotic systems and the Lorenz systems. Chaos Solitons Fractals 2005, 23, 79–85. [Google Scholar]
- Sarasola, C.; Torrealdea, F.J.; D'anjou, A.; Moujahid, A.; Grana, M. Feedback synchronization of chaotic systems. Int. J. Bifurcation Chaos 2003, 13, 177–191. [Google Scholar] [CrossRef]
- Zhou, X.; Xiong, L.; Cai, W.; Cai, X. Adaptive Synchronization and Antisynchronization of a Hyperchaotic Complex Chen System with Unknown Parameters Based on Passive Control. J. Appl. Math. 2013, 2013. [Google Scholar] [CrossRef]
- Vaidyanathan, S.; Volos, C. Analysis and adaptive control of a novel 3-D conservative no-equilibrium chaotic system. Arch. Control. Sci. 2015, 25, 333–353. [Google Scholar] [CrossRef]
- Feki, M. An adaptive chaos synchronization scheme applied to secure communication. Chaos Solitons Fractals 2003, 18, 141–148. [Google Scholar] [CrossRef]
- Bowong, S. Adaptive synchronization between two different chaotic dynamical systems. Commun. Nonlinear Sci. Numer. Simul. 2007, 12, 976–985. [Google Scholar] [CrossRef]
- Jahanshahi, H.; Shahriari-Kahkeshi, M.; Alcaraz, R.; Wang, X.; Singh, V.P.; Pham, V.T. Entropy Analysis and Neural Network-based Adaptive Control of a Non-Equilibrium Four-Dimensional Chaotic System with Hidden Attractors. Entropy 2019, 21, 156. [Google Scholar] [CrossRef]
- Kilic, R.; Alci, M.; Günay, E. Two impulsive synchronization studies using sc-cnn-based circuit and chua's circuit. Int. J. Bifurcation Chaos 2004, 14, 3277–3293. [Google Scholar] [CrossRef]
- Guo, R. Projective synchronization of a class of chaotic systems by dynamic feedback control method. Nonlinear Dyn. 2017, 90, 53–64. [Google Scholar] [CrossRef]
- Kengne, R.; Tchitnga, R.; Mezatio, A.; Fomethe, A.; Litak, G. Finite-time synchronization of fractional-order simplest two-component chaotic oscillators. Eur. Phys. J. B 2017, 90, 88. [Google Scholar] [CrossRef]
- Sun, J.; Wang, Y.; Wang, Y.; Shen, Y. Finite-time synchronization between two complex-variable chaotic systems with unknown parameters via nonsingular terminal sliding mode control. Nonlinear Dyn. 2016, 85, 1105–1117. [Google Scholar] [CrossRef]
- Khan, A.; Tyagi, A. Analysis and hyper-chaos control of a new 4-D hyper-chaotic system by using optimal and adaptive control design. Int. J. Dyn. Control 2017, 5, 1147–1155. [Google Scholar] [CrossRef]
- Saberi Nik, H.; Effati, S.; Saberi-Nadjafi, J. New ultimate bound sets and exponential finite-time synchronization for the complex Lorenz system. J. Complex. 2015, 31, 715–730. [Google Scholar] [CrossRef]
- Liu, Z. Design of nonlinear optimal control for chaotic synchronization of coupled stochastic neural networks via Hamilton-Jacobi-Bellman equation. Neural Netw. 2018, 99, 166–177. [Google Scholar] [CrossRef]
- Naderi, B.; Kheiri, H.; Heydari, A.; Mahini, R. Optimal Synchronization of Complex Chaotic T-Systems and Its Application in Secure Communication. Int. J. Control Autom. Syst. 2016, 27, 379–390. [Google Scholar] [CrossRef]
- Fiori, S. Synchronization of first-order autonomous oscillators on Riemannian manifolds. Discret. Cont. Dyn. Syst. B 2019, 24, 1725–1741. [Google Scholar] [CrossRef]
- Odibat, Z.M. Adaptive feedback control and synchronization of non-identical chaotic fractional order systems. Nonlinear Dyn. 2009, 60, 479–487. [Google Scholar] [CrossRef]
- Shao, S.; Chen, M.; Yan, X. Adaptive sliding mode synchronization for a class of fractional-order chaotic systems with disturbance. Nonlinear Dyn. 2015, 83, 1855–1866. [Google Scholar] [CrossRef]
- Hassan Hosseinnia, R.G.; ARanjbar, N.; Sadati, S.J. Synchronization of Chaotic Fractional-Order Systems via Fractional-Order Adaptive Controller. Appl. Mech. Mater. 2011, 109, 333–339. [Google Scholar]
- Huang, L.; Shi, S.; Zhang, J. Dislocation Synchronization of the Different Complex Value Chaotic Systems Based on Single Adaptive Sliding Mode Controller. Math. Prob. Eng. 2015, 2015. [Google Scholar] [CrossRef]
- Ni, J.; Liu, L.; Liu, C.; Hu, X. Fractional order fixed-time nonsingular terminal sliding mode synchronization and control of fractional order chaotic systems. Nonlinear Dyn. 2017, 89, 2065–2083. [Google Scholar] [CrossRef]
- Harry, B. Book Reviews: Tables of Integral Transforms. Bull. Am. Math. Soc. 1954, 60, 491–493. [Google Scholar]
- Mainardi, F.; Gorenflo, R. On Mittag-Leffler-type functions in fractional evolution processes. J. Comput. Appl. Math. 2000, 118, 283–299. [Google Scholar] [CrossRef]
- Agarwal, R.P.; Belmekki, M.; Benchohra, M. A Survey on Semilinear Differential Equations and Inclusions Involving Riemann-Liouville Fractional Derivative. Adv. Differ. Equ. 2009, 2009, 981728. [Google Scholar] [CrossRef]
- Gallegos, J.A.; Duarte-Mermoud, M.A. A dissipative approach to the stability of multi-order fractional systems. IMA J. Math. Control Inf. 2018, 36. [Google Scholar] [CrossRef]
- Caputo, M. Linear Models of Dissipation whose Q is almost Frequency Independent-II. Geophys. J. Int. 1967, 13, 529–539. [Google Scholar] [CrossRef]
- Soukkou, A.; Boukabou, A.; Leulmi, S. Prediction-based feedback control and synchronization algorithm of fractional-order chaotic systems. Nonlinear Dyn. 2016, 85, 2183–2206. [Google Scholar] [CrossRef]
- Gallegos, J.A.; Duarte-Mermoud, M.A. Convergence of fractional adaptive systems using gradient approach. ISA Trans. 2017, 69, 31–42. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Chen, Y.; Podlubny, I. Stability of fractional-order nonlinear dynamic systems: Lyapunov direct method and generalized Mittag—Leffler stability. Comput. Math. Appl. 2010, 59, 1810–1821. [Google Scholar] [CrossRef]
- Yu, Y.; Li, H.; Wang, S.; Yu, J. Dynamic analysis of a fractional-order Lorenz chaotic system. Chaos Solitons Fractals 2009, 42, 1181–1189. [Google Scholar] [CrossRef]
- Aguilera, E.D.; Mermoud, M.D. Synchronization of Fractional-Order Systems of the Lorenz type: The Non-adaptive Case. IEEE Lat. Am. Trans. 2014, 12, 410–415. [Google Scholar] [CrossRef]
- Kai, D.; Ford, N.J.; Freed, A.D. A Predictor-Corrector Approach for the Numerical Solution of Fractional Differential Equations. Nonlinear Dyn. 2002, 29, 3–22. [Google Scholar]
- Kapitaniak, T.; Mohammadi, S.; Mekhilef, S.; Alsaadi, F.; Hayat, T.; Pham, V.T. A New Chaotic System with Stable Equilibrium: Entropy Analysis, Parameter Estimation, and Circuit Design. Entropy 2018, 20, 670. [Google Scholar] [CrossRef]
- Munoz-Pacheco, J.; Zambrano-Serrano, E.; Volos, C.; Jafari, S.; Kengne, J.; Rajagopal, K. A New Fractional-Order Chaotic System with Different Families of Hidden and Self-Excited Attractors. Entropy 2018, 20, 564. [Google Scholar] [CrossRef]




© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, L.; Du, C.; Zhang, X.; Li, J.; Shi, S. Adaptive Synchronization Strategy between Two Autonomous Dissipative Chaotic Systems Using Fractional-Order Mittag–Leffler Stability. Entropy 2019, 21, 383. https://doi.org/10.3390/e21040383
Liu L, Du C, Zhang X, Li J, Shi S. Adaptive Synchronization Strategy between Two Autonomous Dissipative Chaotic Systems Using Fractional-Order Mittag–Leffler Stability. Entropy. 2019; 21(4):383. https://doi.org/10.3390/e21040383
Chicago/Turabian StyleLiu, Licai, Chuanhong Du, Xiefu Zhang, Jian Li, and Shuaishuai Shi. 2019. "Adaptive Synchronization Strategy between Two Autonomous Dissipative Chaotic Systems Using Fractional-Order Mittag–Leffler Stability" Entropy 21, no. 4: 383. https://doi.org/10.3390/e21040383
APA StyleLiu, L., Du, C., Zhang, X., Li, J., & Shi, S. (2019). Adaptive Synchronization Strategy between Two Autonomous Dissipative Chaotic Systems Using Fractional-Order Mittag–Leffler Stability. Entropy, 21(4), 383. https://doi.org/10.3390/e21040383



