Exergy Analysis of a Parallel-Plate Active Magnetic Regenerator with Nanofluids
Abstract
1. Introduction
2. One-Dimensional Numerical Method
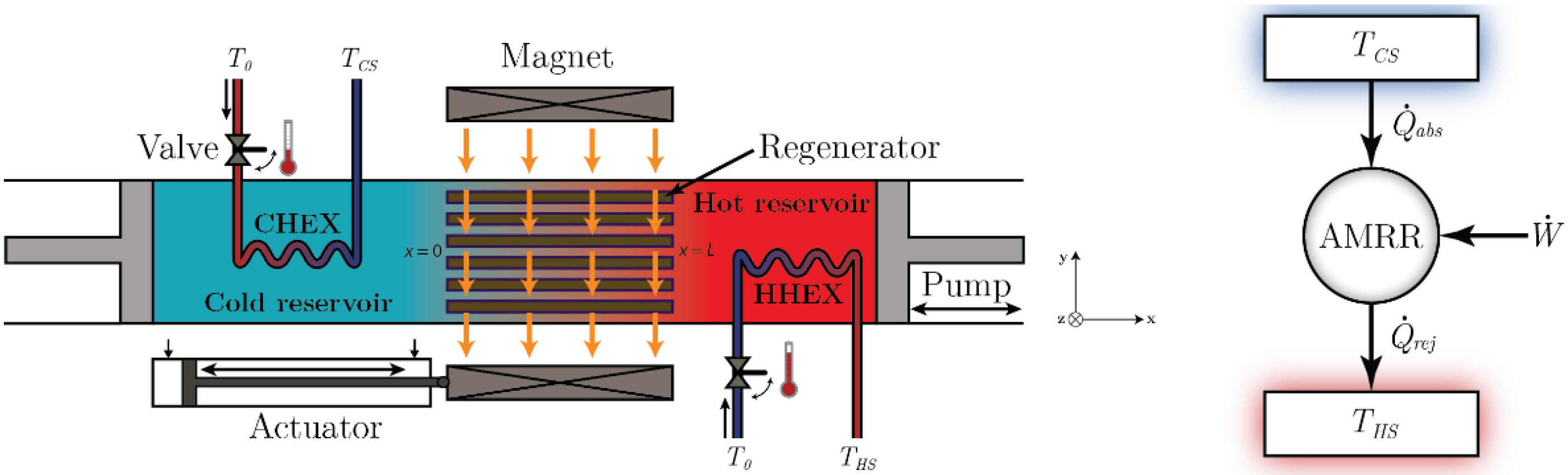
2.1. Description of the System
2.2. Numerical Modeling
2.2.1. Assumptions
- The system operating near room temperature, adiabatic conditions may be assumed. It has been carefully checked that including losses to the surroundings leads to similar results.
- The plates are made of gadolinium (Gd), which is the most common material used in magnetic refrigeration near room temperature. To model the magnetocaloric effect (MCE), the experimental data of Dankov et al. [18] are used showing better results compared to the Weiss-Debye-Sommerfeld model. The properties of Gd are temperature- and pressure-dependent thanks to Coolprop library.
- The magnetic field is applied in the y-direction (Figure 1). As a first step, the demagnetization is neglected. The reader can refer to the works of Nielsen et al. [19], Engelbrecht et al. [20] and Mugica et al. [15] for details on the impact of the demagnetization effect on the performance of the AMR.
- The magnetic field is assumed equally applied throughout the entire length of the regenerator. The parasitic losses are neglected. Their influence on the AMR performance has been discussed in [20].
- The time for magnetization or demagnetization is fixed to tmag = tdemag = 0.01 s and no idle time between each step of the cycle is considered.
- The flow is supposed to be laminar, fully-developed and steady-state with only one uniform velocity component V in the streamwise direction. The impact of flow maldistribution discussed in [20] is not taken into account here.
- Nanofluids are assumed to be single-phase fluids with constant volumetric concentration in nanoparticles throughout the domain. Their thermophysical properties depend on the fluid and nanoparticle properties, and the local temperature T.
2.2.2. Energy Equations
2.2.3. Heat Transfer Fluid Properties
2.2.4. Numerical Method and Parameters
2.3. Thermodynamic Analysis
3. Results and Discussion
3.1. Influence of the Nanoparticle Concentration
3.2. Influence of the Blowing Time
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Bouchekara, H. Recherche sur les Systèmes de Réfrigération Magnétique: Modélisation Numérique, Conception et Optimisation. Ph.D. Thesis, Institut National Polytechnique de Grenoble, Grenoble, France, 2008. (In French). [Google Scholar]
- Kitanovski, A.; Tušek, J.; Tomc, U.; Plaznik, U.; Ožbolt, M.; Poredoš, A. Magnetocaloric Energy Conversion: From Theory to Applications, Green Energy and Technology; Springer: New York, NY, USA, 2015. [Google Scholar]
- Pecharsky, V.V.; Cui, J.; Johnson, D.D. (Magneto) caloric refrigeration: Is there light at the end of the tunnel? Philos. Trans. R. Soc. A 2016, 374. [Google Scholar] [CrossRef] [PubMed]
- Trevizoli, P.V.; Christiaanse, T.V.; Govindappa, P.; Niknia, I.; Teyber, R.; Barbosa, J.R., Jr.; Rowe, A. Magnetic heat pumps: An overview of design principles and challenges. Sci. Technol. Built Environ. 2016, 22, 507–519. [Google Scholar] [CrossRef]
- Yu, B.; Liu, M.; Egolf, P.W.; Kitanovski, A. A review of magnetic refrigerator and heat pump prototypes built before the year 2010. Int. J. Refrig. 2010, 33, 1029–1060. [Google Scholar] [CrossRef]
- Tusek, J.; Kitanovsky, A.; Zupan, S.; Prebil, I.; Poredos, A. A comprehensive experimental analysis of gadolinium active magnetic regenerators. Appl. Therm. Eng. 2013, 53, 57–66. [Google Scholar] [CrossRef]
- Trevizoli, P.V.; Nakashima, A.T.; Peixer, G.F.; Barbosa, J.R., Jr. Performance assessment of different porous matrix for active regenerators. Appl. Energy 2017, 187, 847–861. [Google Scholar] [CrossRef]
- Tagliafico, G.; Scarpa, F.; Canepa, F. A dynamic 1-D model for a reciprocating active magnetic regenerator; influence of the main working parameters. Int. J. Refrig. 2010, 33, 286–293. [Google Scholar] [CrossRef]
- Tura, A.; Nielsen, K.K.; Rowe, A. Experimental and modeling results of a parallel plate-based active magnetic regenerator. Int. J. Refrig. 2012, 35, 1518–1527. [Google Scholar] [CrossRef]
- Wu, J.; Liu, C.; Hou, P.; Huang, Y.; Ouyang, G.; Chen, Y. Fluid choice and test standardization for magnetic regenerators operating at near room temperature. Int. J. Refrig. 2014, 37, 135–146. [Google Scholar] [CrossRef]
- Nielsen, K.; Tušek, J.; Engelbrecht, K.; Schopfer, S.; Kitanovski, A.; Bahl, C.; Smith, A.; Pryds, N.; Poredoš, A. Review on numerical modeling of active magnetic regenerators for room temperature applications. Int. J. Refrig. 2011, 34, 603–616. [Google Scholar] [CrossRef]
- Trevizoli, P.V.; Nakashima, A.T.; Barbosa, J.R., Jr. Performance evaluation of an active magnetic regenerator for cooling applications—Part II: Mathematical modeling and thermal losses. Int. J. Refrig. 2016, 72, 206–217. [Google Scholar] [CrossRef]
- Niknia, I.; Campbell, O.; Christiaanse, T.V.; Govindappa, P.; Teyber, R.; Trevizoli, P.V.; Rowe, A. Impacts of configuration losses on active magnetic regenerator device performance. Appl. Therm. Eng. 2016, 106, 601–612. [Google Scholar] [CrossRef]
- Roy, S.; Poncet, S.; Sorin, M. Sensitivity analysis and multiobjective optimization of a parallel-plate active magnetic regenerator using a genetic algorithm. Int. J. Refrig. 2017, 75, 276–285. [Google Scholar] [CrossRef]
- Mugica, I.; Poncet, S.; Bouchard, J. Entropy generation in a parallel-plate active magnetic regenerator with insulator layers. J. Appl. Phys. 2017, 121, 074901. [Google Scholar] [CrossRef]
- Lei, T.; Engelbrecht, K.; Nielsen, K.K.; Veje, C.T. Study of geometries of active magnetic regenerators for room temperature magnetocaloric refrigeration. Appl. Therm. Eng. 2017, 111, 1232–1243. [Google Scholar] [CrossRef]
- Roudaut, J. Modélisation et Conception de Systèmes de Réfrigération Magnétique autour de la Température Ambiante. Ph.D. Thesis, Université de Grenoble, Grenoble, France, 2012. (In French). [Google Scholar]
- Dankov, S.Y.; Tishin, A.; Pecharsky, V.; Gschneidner, K. Magnetic phase transitions and the magnetothermal properties of gadolinium. Phys. Rev. B 1998, 57, 1–13. [Google Scholar] [CrossRef]
- Nielsen, K.K.; Smith, A.; Bahl, C.R.H.; Olsen, U.L. The influence of demagnetizing effects on the performance of active magnetic regenerators. J. Appl. Phys. 2012, 112, 094905. [Google Scholar] [CrossRef]
- Engelbrecht, K.; Tusek, J.; Nielsen, K.K.; Kitanovski, A.; Bahl, C.R.H.; Poredos, A. Improved modelling of a parallel plate active magnetic regenerator. J. Phys. D 2013, 46, 255002. [Google Scholar] [CrossRef]
- Rohsenow, W.M.; Hartnett, J.P.; Cho, Y.L. Handbook of Heat Transfer; McGraw-Hill: New York, NY, USA, 1998. [Google Scholar]
- Garby, L.; Larsen, P.S. Bioenergetics: Its Thermodynamic Foundations; Cambridge University Press: Cambridge, UK, 1991. [Google Scholar]
- Bianco, V.; Manca, O.; Nardini, S.; Vafai, K. Heat Transfer Enhancement with Nanofluids; CRC Press: New York, NY, USA, 2015. [Google Scholar]
- Maïga, S.E.B.; Palm, S.M.; Nguyen, C.T.; Roy, G.; Galanis, N. Heat transfer enhancement by using nanofluids in forced convection flows. Int. J. Heat Fluid Flow 2005, 26, 530–546. [Google Scholar] [CrossRef]
- Mintsa, H.A.; Roy, G.; Nguyen, C.T.; Doucet, D. New temperature dependent thermal conductivity data for water-based nanofluids. Int. J. Therm. Sci. 2009, 48, 363–371. [Google Scholar] [CrossRef]
- Sekrani, G.; Poncet, S. Further investigation on Laminar forced convection of nanofluid flows in a uniformly heated pipe using direct numerical simulations. Appl. Sci. 2016, 6, 1–24. [Google Scholar] [CrossRef]
- Prasher, R.; Song, D.; Phelan, J.W. Measurements of nanofluid viscosity and its implications for thermal applications. Appl. Phys. Lett. 2006, 89, 133108. [Google Scholar] [CrossRef]
- Kitanovski, A.; Egolf, P.W. Application of magnetic refrigeration and its assessment. J. Magn. Magn. Mater. 2009, 321, 777–781. [Google Scholar] [CrossRef]
- Rowe, A. Thermodynamics of active magnetic regenerators: Part I. Cryogenics 2012, 52, 111–118. [Google Scholar] [CrossRef]
- Rowe, A. Thermodynamics of active magnetic regenerators: Part II. Cryogenics 2012, 52, 119–128. [Google Scholar] [CrossRef]
- Trevizoli, P.V.; Barbosa, J.R., Jr. Entropy generation minimization analysis of oscillating-flow regenerators. Int. J. Heat Mass Transf. 2015, 87, 347–358. [Google Scholar] [CrossRef]
- Plaznik, U.; Tusek, J.; Kitanovski, A.; Poredos, A. Numerical and experimental analyses of different magnetic thermodynamic cycles with an active magnetic regenerator. Appl. Therm. Eng. 2013, 59, 52–59. [Google Scholar] [CrossRef]
- Li, P.; Gong, M.; Yao, G.; Wu, J. A practical model for analysis of active magnetic regenerative refrigerators for room temperature applications. Int. J. Refrig. 2006, 29, 1259–1266. [Google Scholar] [CrossRef]



| Material | B | L | ef | es | ∆THX |
|---|---|---|---|---|---|
| 0.2 kg (Gd) | 1.5 T | 0.1 m | 0.15 mm | 0.5 mm | 5 K |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mugica, I.; Roy, S.; Poncet, S.; Bouchard, J.; Nesreddine, H. Exergy Analysis of a Parallel-Plate Active Magnetic Regenerator with Nanofluids. Entropy 2017, 19, 464. https://doi.org/10.3390/e19090464
Mugica I, Roy S, Poncet S, Bouchard J, Nesreddine H. Exergy Analysis of a Parallel-Plate Active Magnetic Regenerator with Nanofluids. Entropy. 2017; 19(9):464. https://doi.org/10.3390/e19090464
Chicago/Turabian StyleMugica, Ibai, Steven Roy, Sébastien Poncet, Jonathan Bouchard, and Hakim Nesreddine. 2017. "Exergy Analysis of a Parallel-Plate Active Magnetic Regenerator with Nanofluids" Entropy 19, no. 9: 464. https://doi.org/10.3390/e19090464
APA StyleMugica, I., Roy, S., Poncet, S., Bouchard, J., & Nesreddine, H. (2017). Exergy Analysis of a Parallel-Plate Active Magnetic Regenerator with Nanofluids. Entropy, 19(9), 464. https://doi.org/10.3390/e19090464






