Second Law Analysis for Variable Viscosity Hydromagnetic Boundary Layer Flow with Thermal Radiation and Newtonian Heating
Abstract
:1. Introduction
2. Mathematical Model
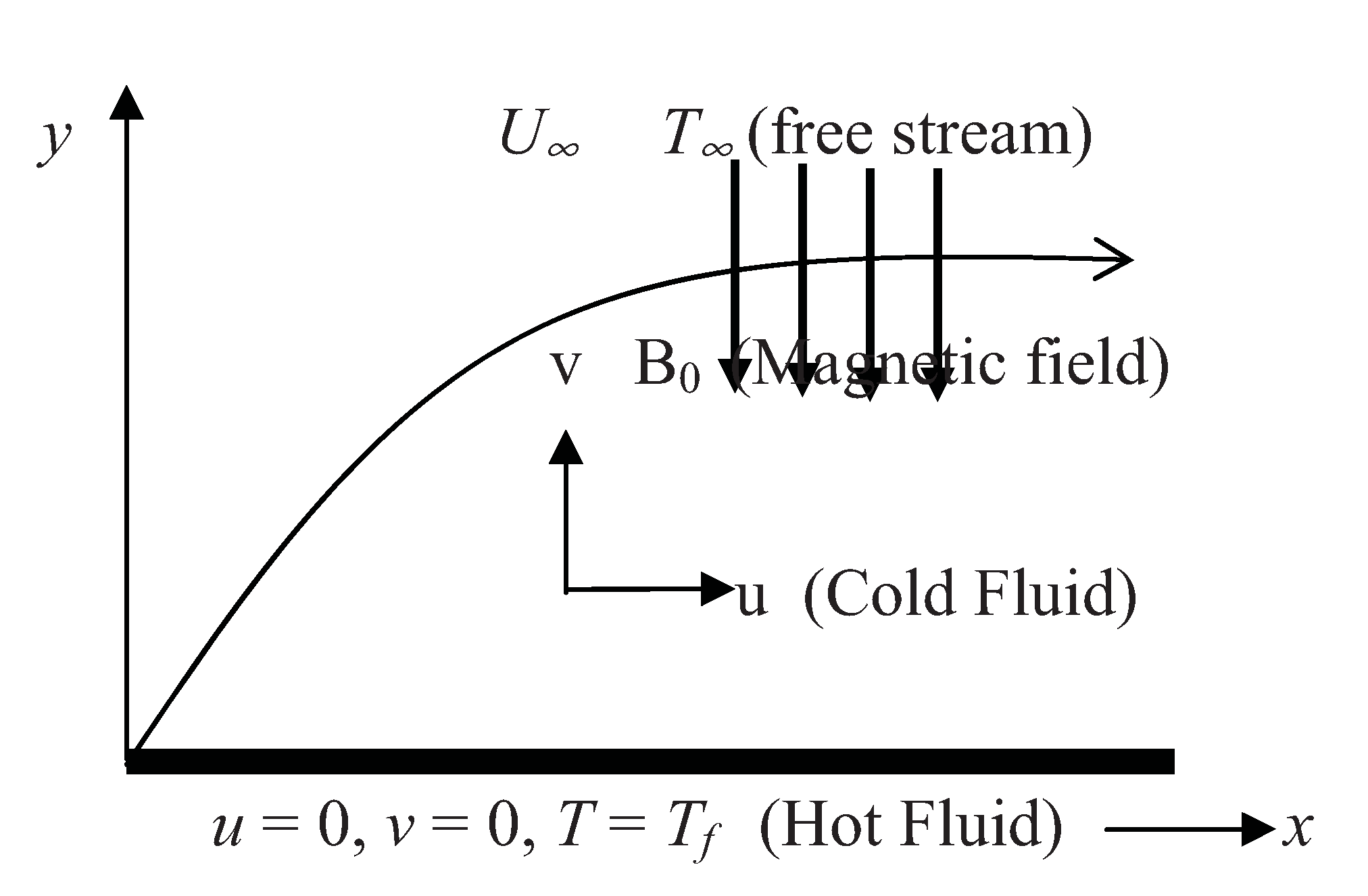
2.1. Numerical Procedure
3. Entropy Generation Analysis
4. Results and Discussion
Bi | Aziz [18] | − Aziz [18] | Present | − Present |
|---|---|---|---|---|
| 0.05 | 0.1447 | 0.0428 | 0.14466 | 0.04276 |
| 0.60 | 0.6699 | 0.1981 | 0.66991 | 0.19805 |
| 1.00 | 0.7718 | 0.2282 | 0.77182 | 0.22817 |
| 5.00 | 0.9441 | 0.2791 | 0.94417 | 0.27913 |
| 20.00 | 0.9854 | 0.2913 | 0.98543 | 0.29132 |
| Bi | a | Br | Ra | Pr | Ha | − | ||
|---|---|---|---|---|---|---|---|---|
| 0.1 | 0.1 | 0.1 | 0.7 | 0.72 | 0.1 | 0.46167359 | 0.06417756 | 0.35822438 |
| 1.0 | 0.1 | 0.1 | 0.7 | 0.72 | 0.1 | 0.47460353 | 0.16490527 | 0.83509472 |
| 10 | 0.1 | 0.1 | 0.7 | 0.72 | 0.1 | 0.47847240 | 0.19577586 | 0.98042241 |
| 0.1 | 0.5 | 0.1 | 0.7 | 0.72 | 0.1 | 0.49847466 | 0.06463133 | 0.35368667 |
| 0.1 | 1.0 | 0.1 | 0.7 | 0.72 | 0.1 | 0.53980796 | 0.06510562 | 0.34894370 |
| 0.1 | −0.1 | 0.1 | 0.7 | 0.72 | 0.1 | 0.44170340 | 0.06391808 | 0.36081912 |
| 0.1 | −0.5 | 0.1 | 0.7 | 0.72 | 0.1 | 0.39774382 | 0.06331171 | 0.36688289 |
| 0.1 | 0.1 | 1.0 | 0.7 | 0.72 | 0.1 | 0.46903194 | 0.03335240 | 0.66647595 |
| 0.1 | 0.1 | 10 | 0.7 | 0.72 | 0.1 | 0.52982570 | 0.24134507 | 3.41345073 |
| 0.1 | 0.1 | 0.1 | 5.0 | 0.72 | 0.1 | 0.46104375 | 0.06820145 | 0.31798541 |
| 0.1 | 0.1 | 0.1 | 10.0 | 0.72 | 0.1 | 0.46100454 | 0.06856032 | 0.31439674 |
| 0.1 | 0.1 | 0.1 | 0.7 | 3.00 | 0.1 | 0.45924014 | 0.07604897 | 0.23951024 |
| 0.1 | 0.1 | 0.1 | 0.7 | 7.10 | 0.1 | 0.45801360 | 0.08146073 | 0.18539265 |
| 0.1 | 0.1 | 0.1 | 0.7 | 0.72 | 0.5 | 0.78699049 | 0.06242200 | 0.37577998 |
| 0.1 | 0.1 | 0.1 | 0.7 | 0.72 | 1.0 | 1.06625574 | 0.06065042 | 0.39349571 |
| 0.1 | 0.1 | 0.1 | 0.7 | 0.72 | 2.0 | 1.47684794 | 0.05773749 | 0.42262501 |
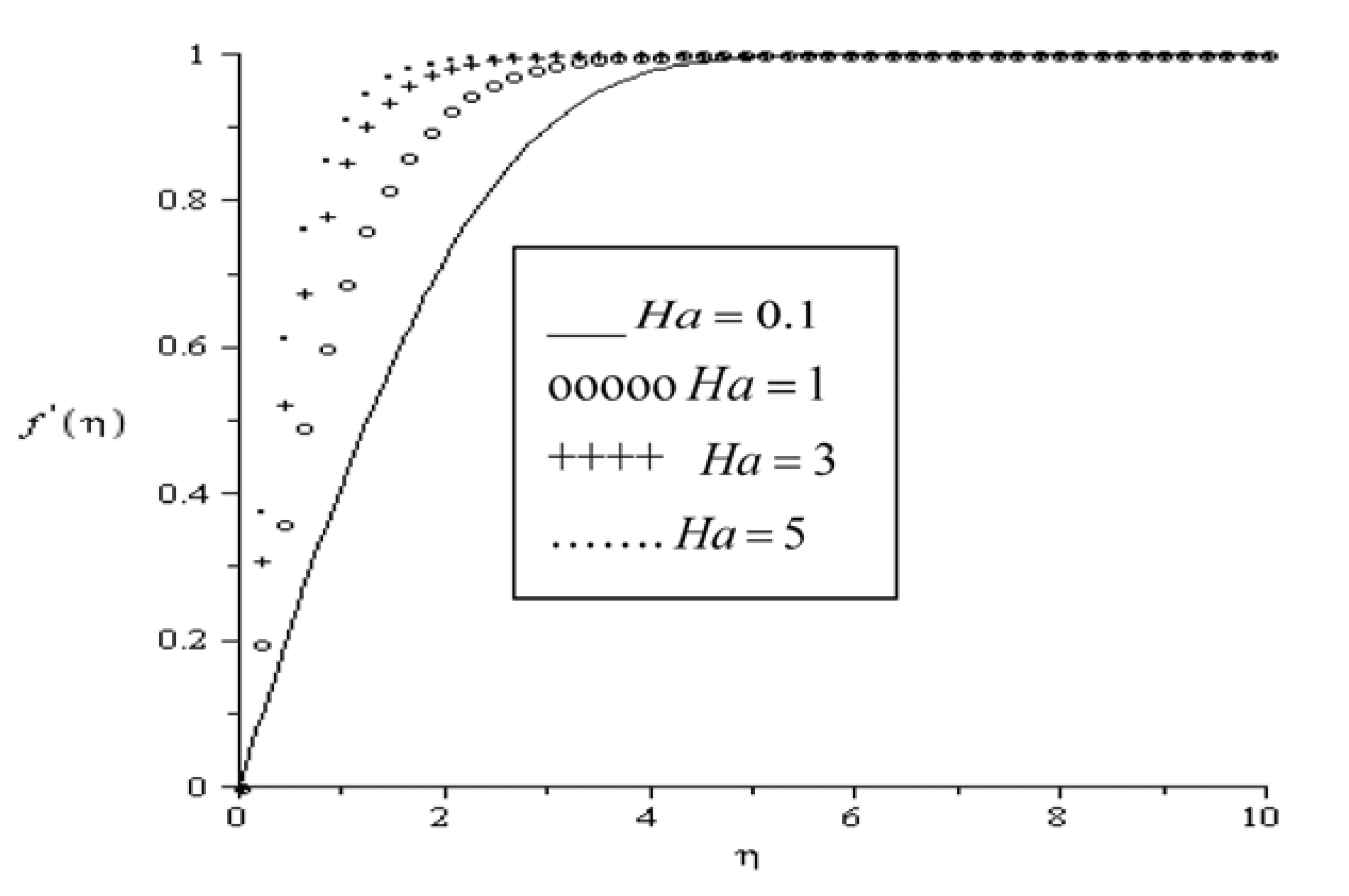
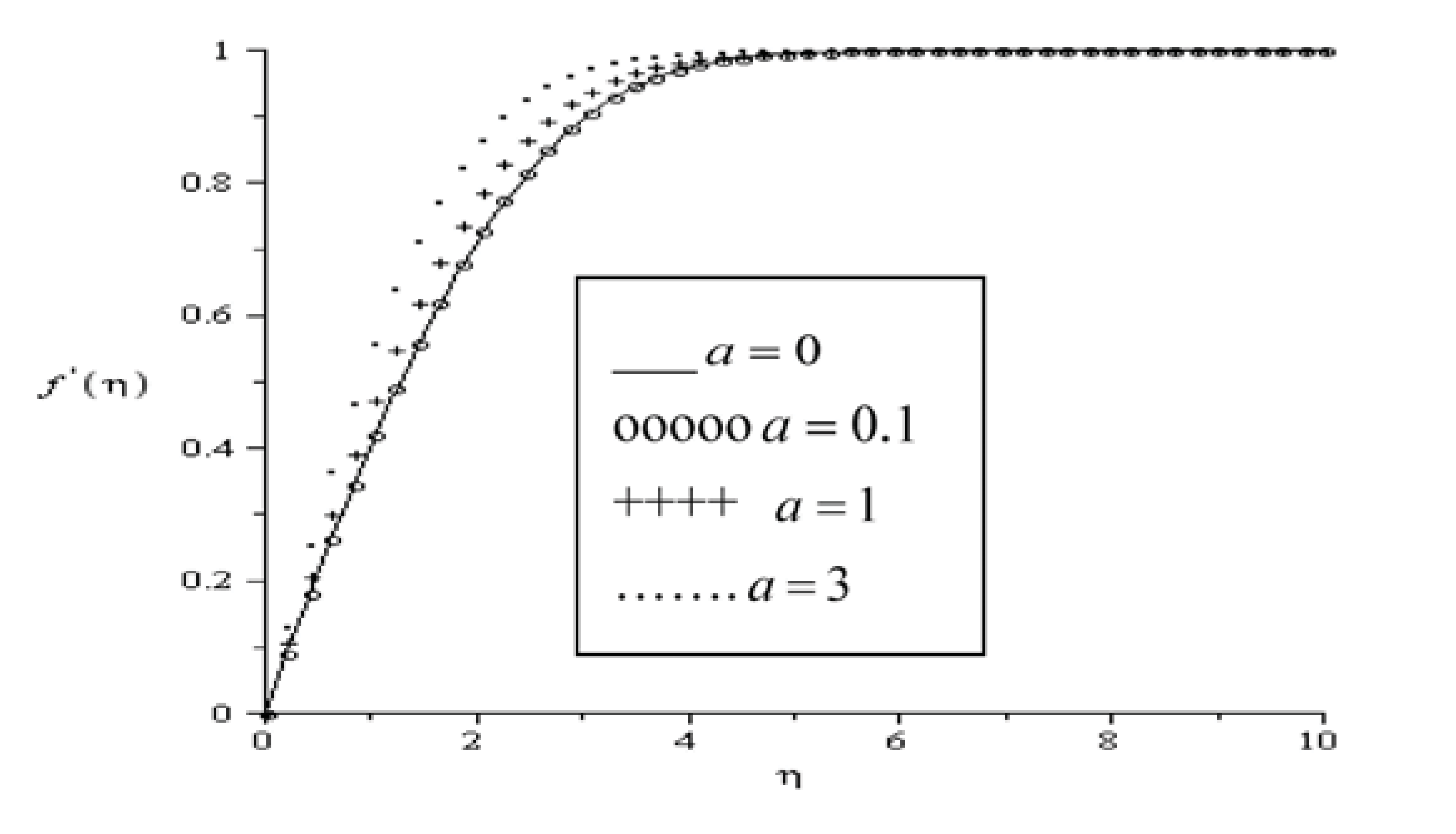
4.1. Effect of Parameter Variation on Velocity Profiles
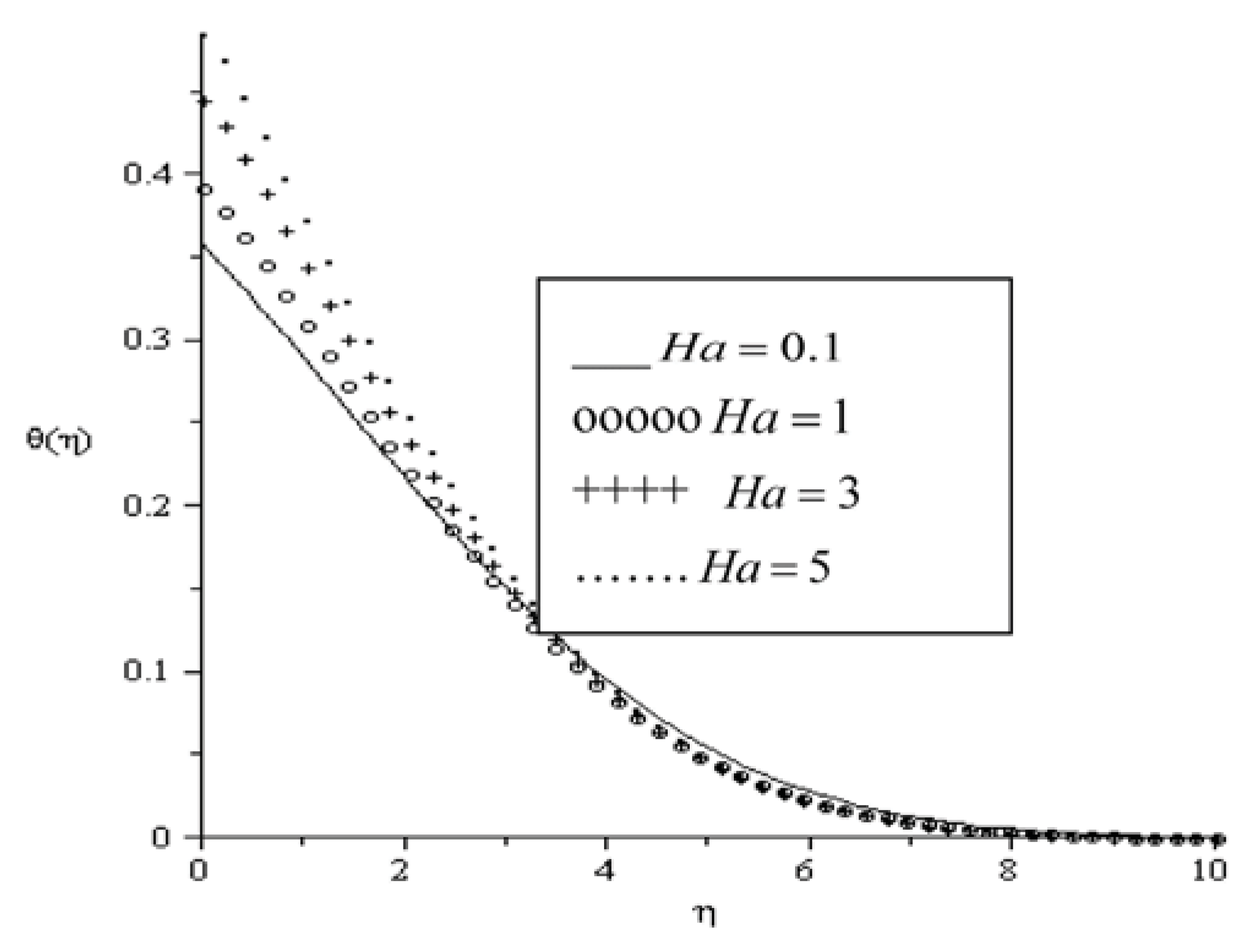
4.2. Effects of Parameter Variation on Temperature Profiles
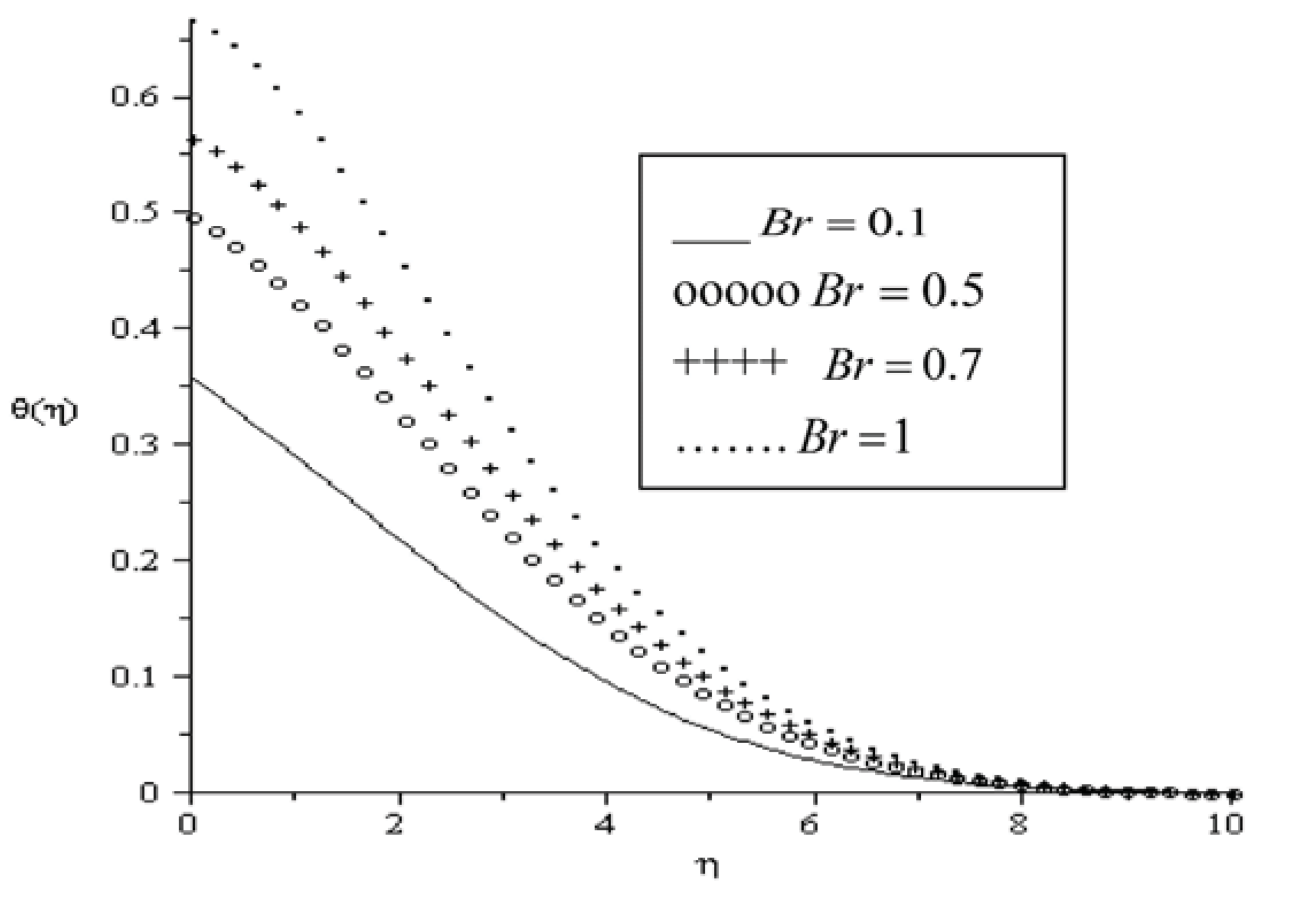
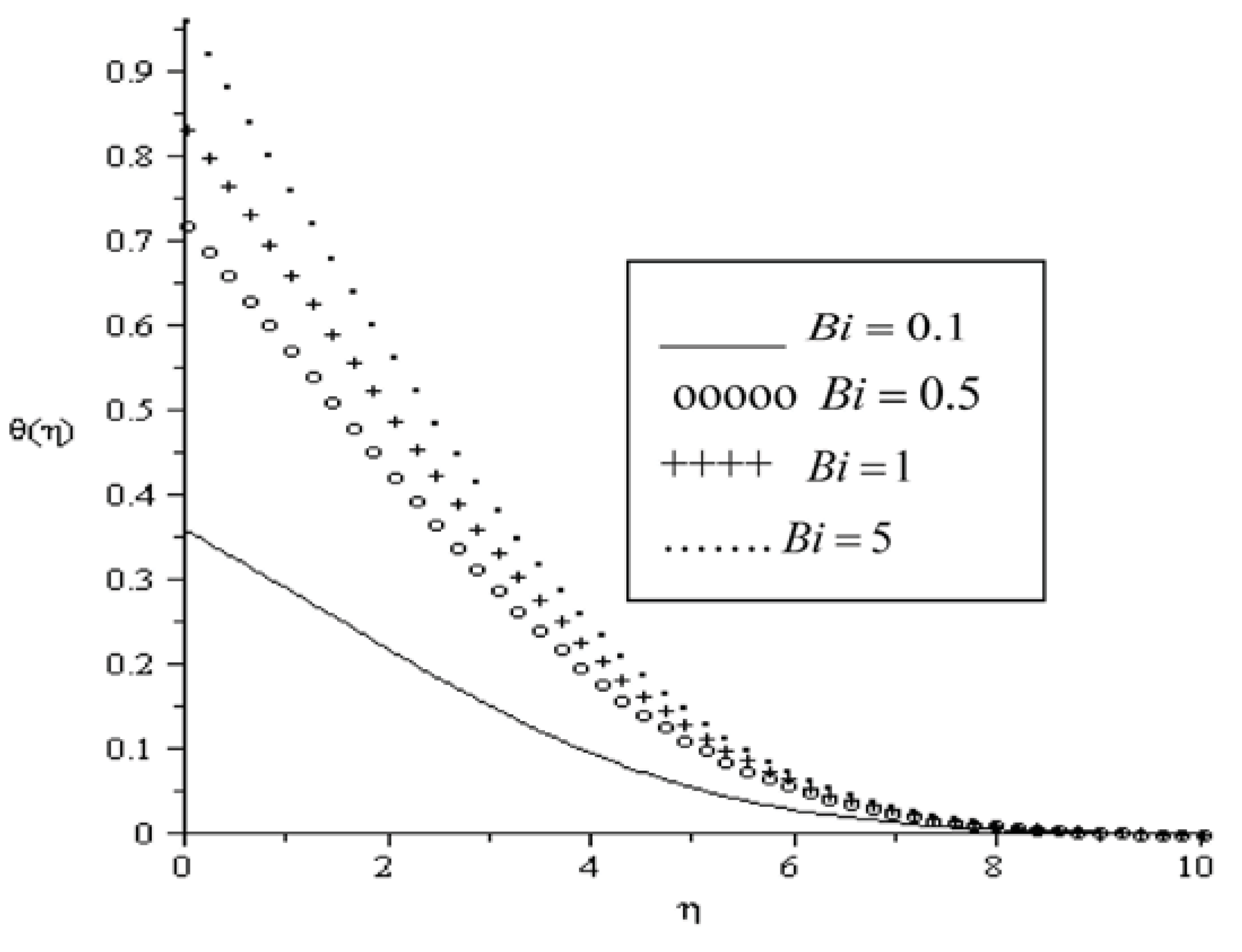
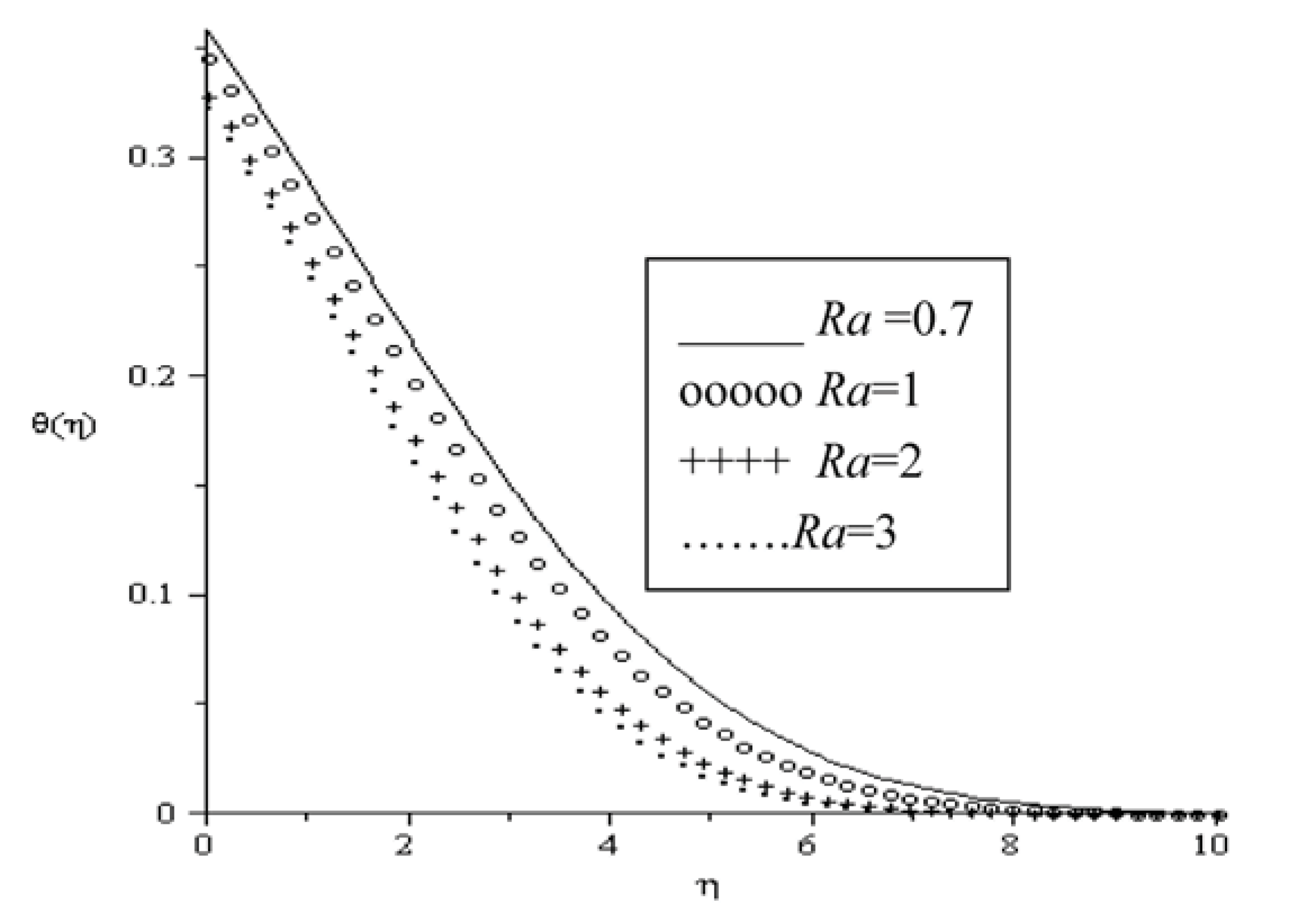
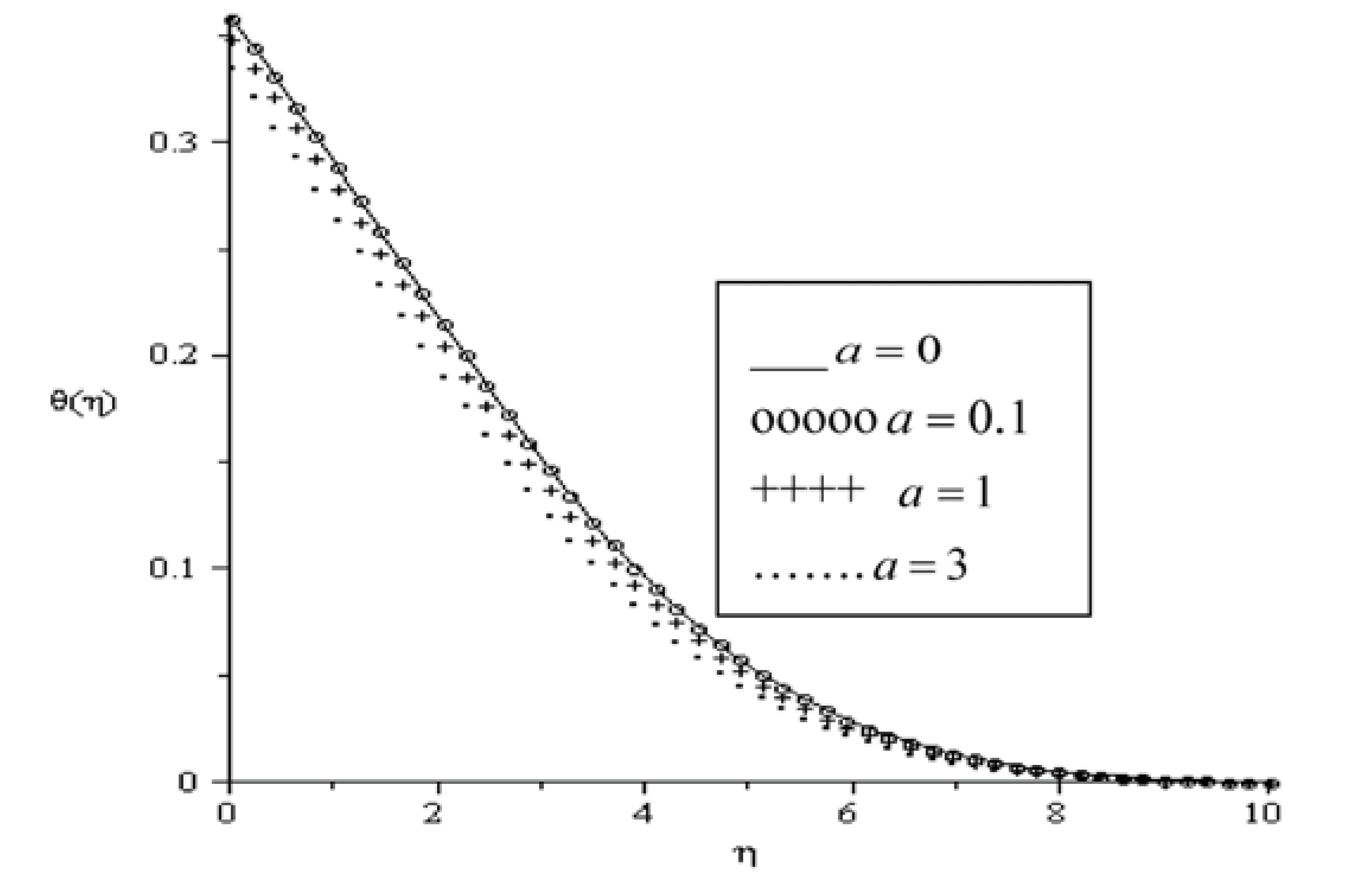

4.3. Effects of Parameter Variation on Entropy Generation Rate
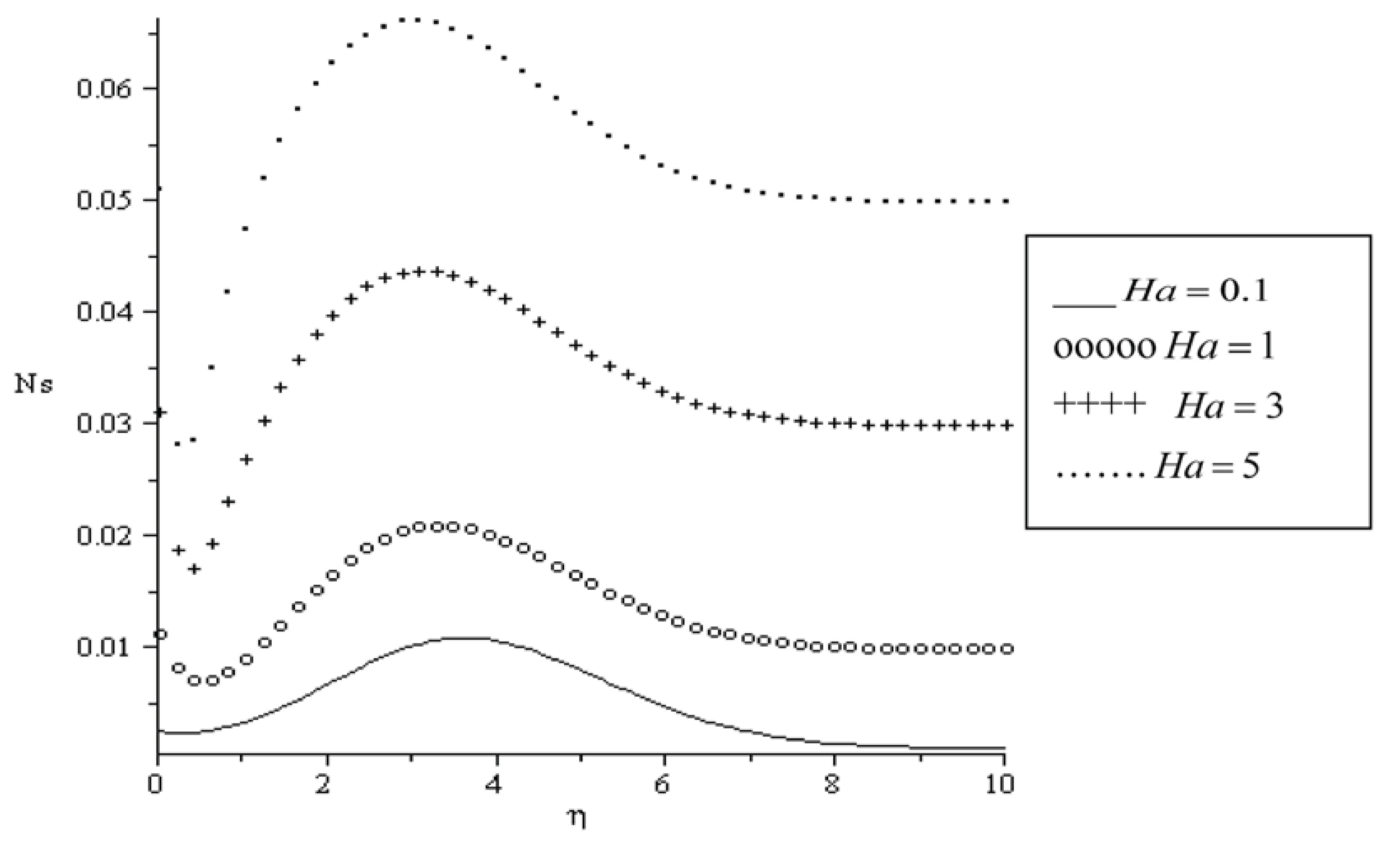
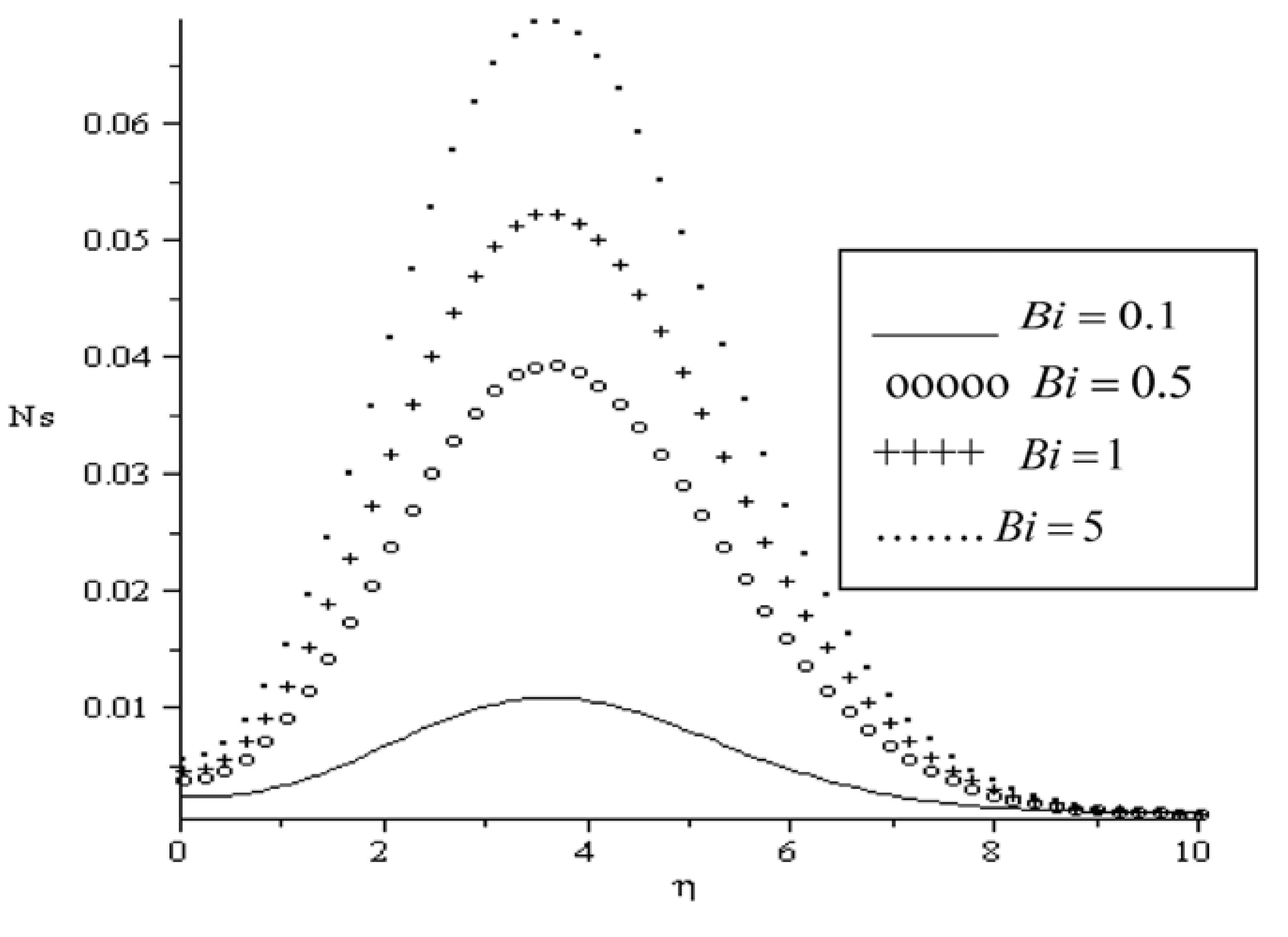
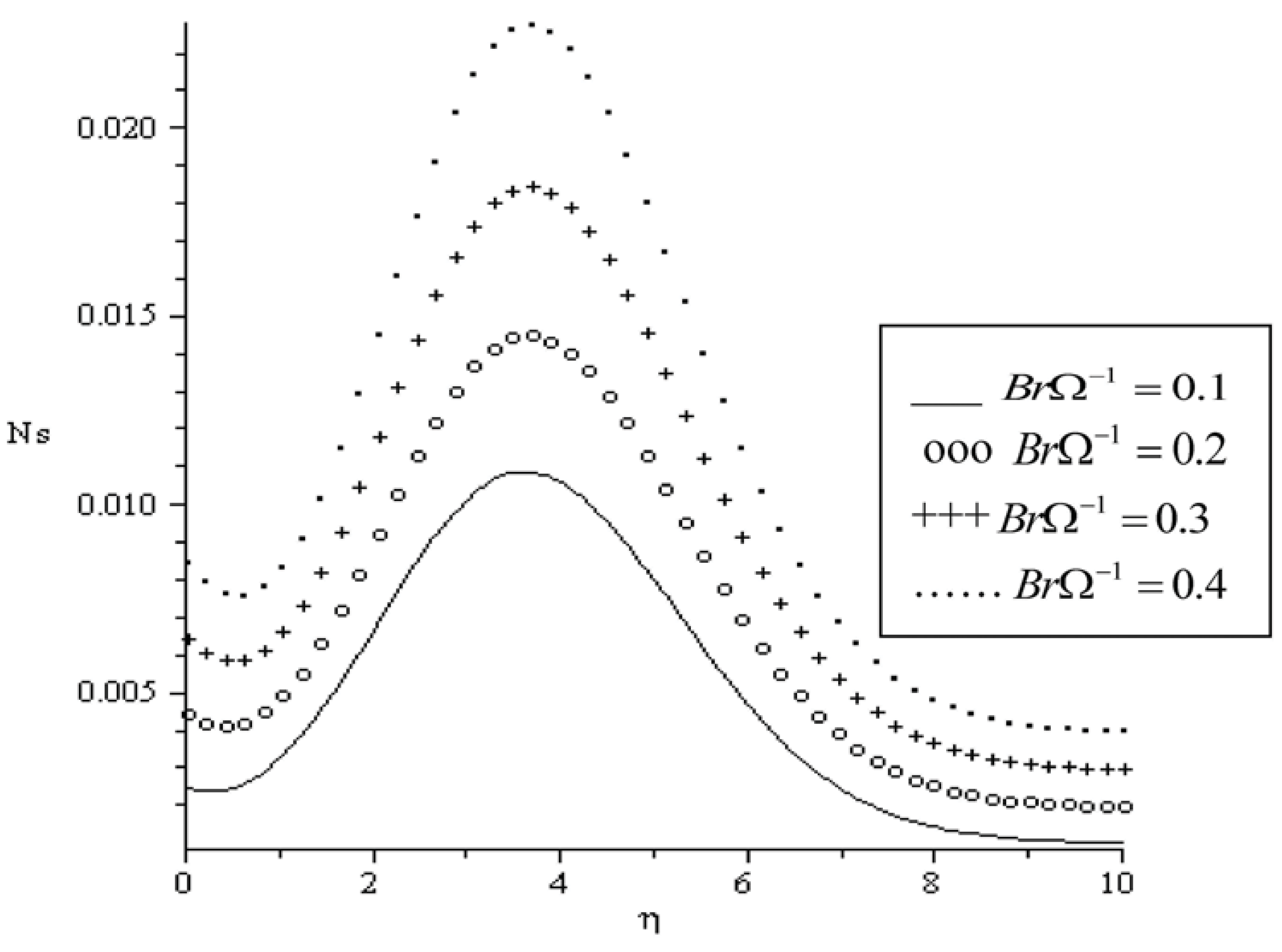
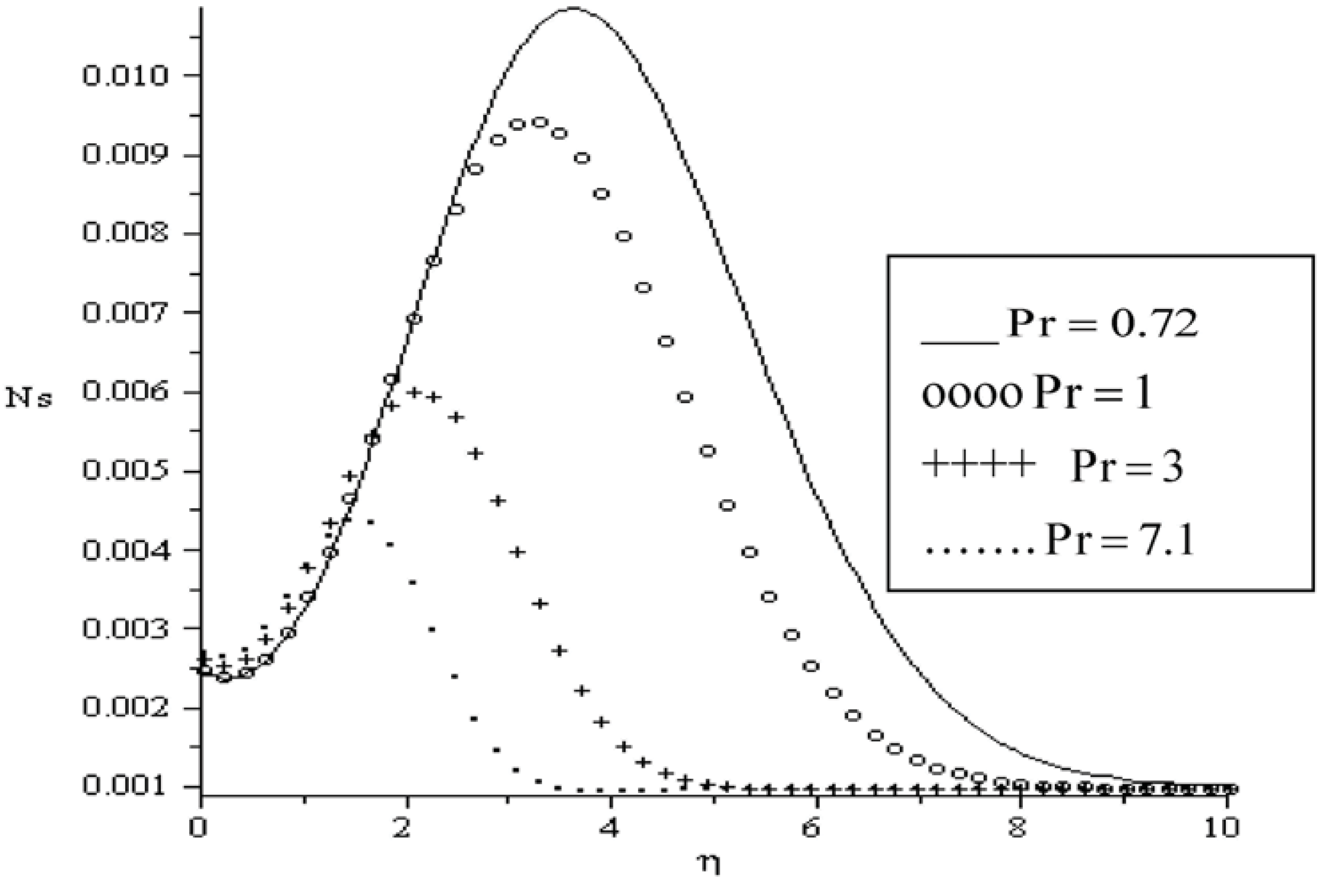
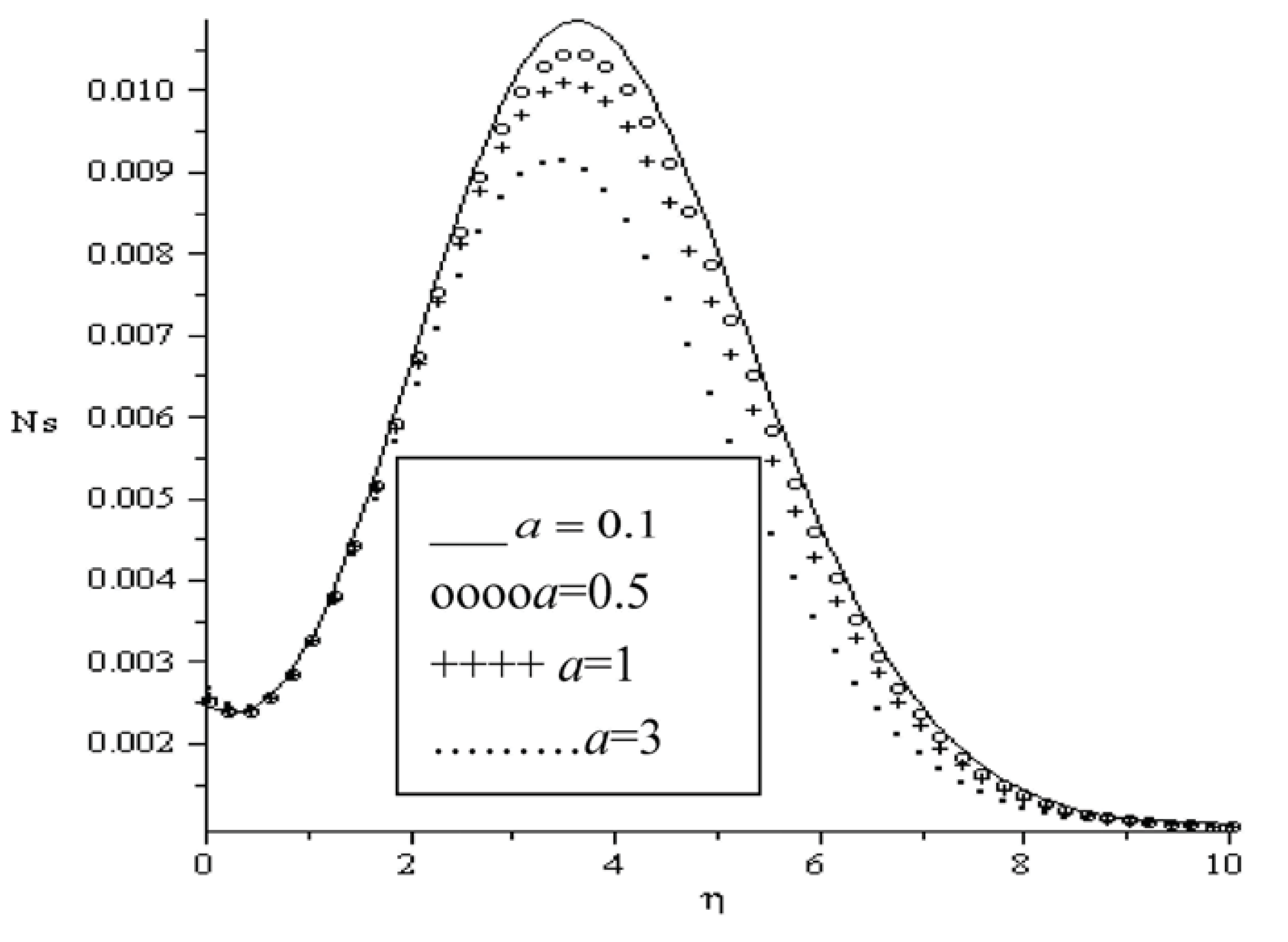
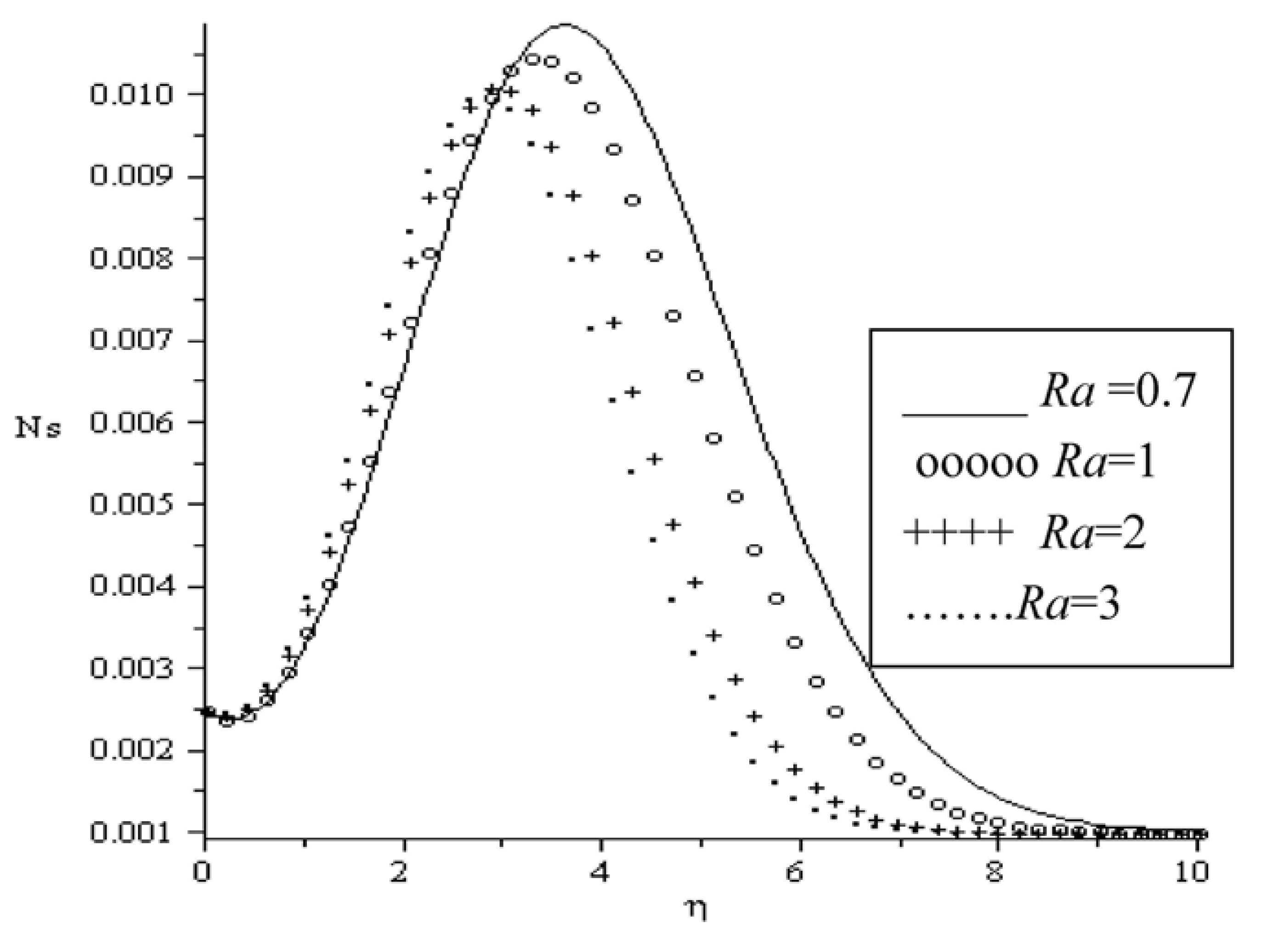
4.4. Effects of Parameter Variation on Bejan Number


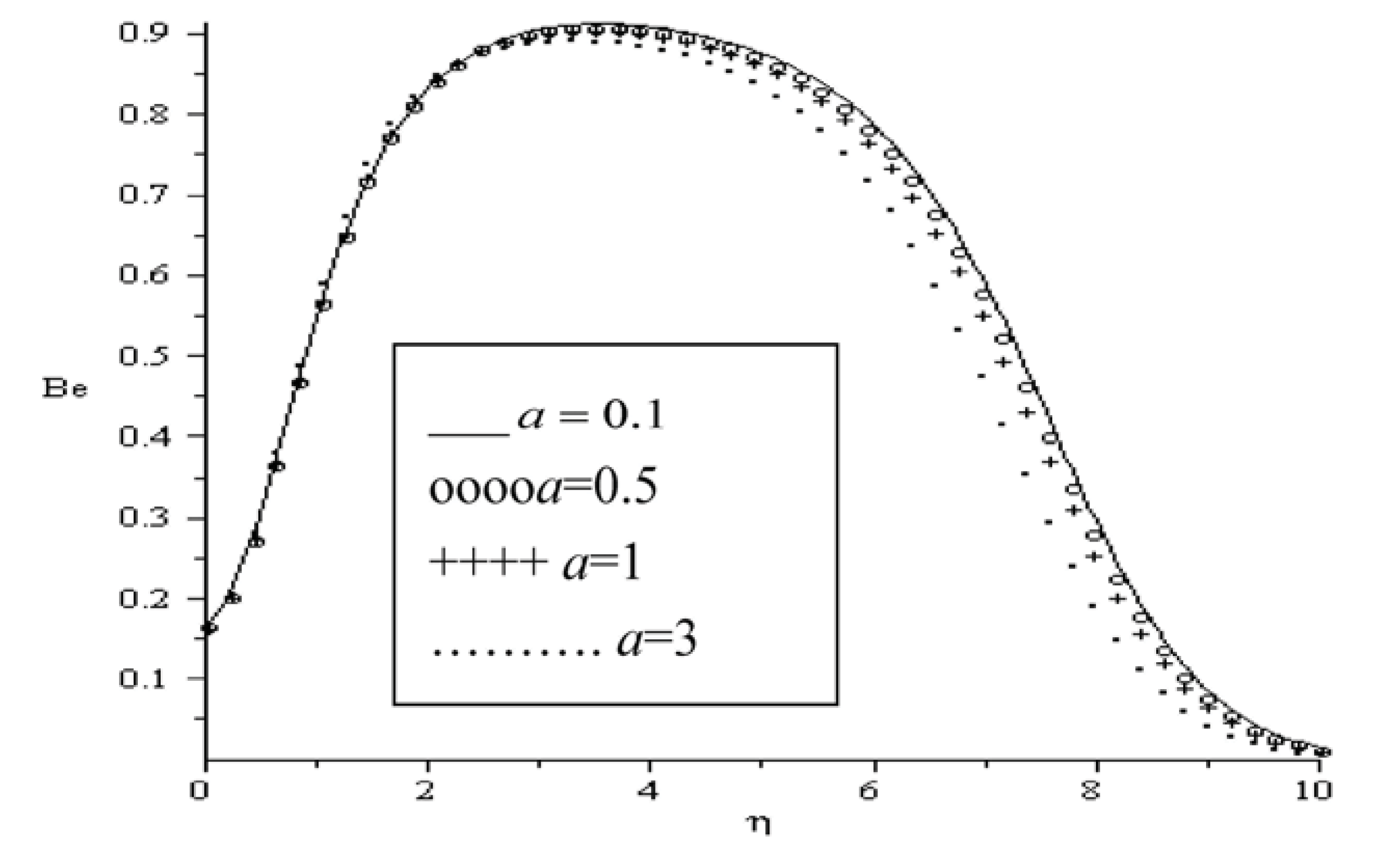



5. Conclusions
- The skin friction and the Nusselt number increase with increasing values of Bi, Br, a. An increase Ha causes a decrease in the Nusselt number and an increase in the skin friction. As Ra and Pr increase, the skin friction decreases while the Nusselt number increases.
- The velocity boundary layer thickness decreases with Ha, a.
- The thermal boundary layer thickness increases with Ha, Br, Bi and decreases with Ra, Pr, a.
- The plate surface act as a strong source of entropy generation and heat transfer irreversibility. However, the peak of entropy generation rate is attained within the boundary layer region.
- The entropy generation number increases as Ha, Bi and BrΩ−1 increase while it decreases as the Pr, Ra and a increase.
- The optimum design and efficient performance of the flow system can be enhanced by the ability to clearly identify the source and location of entropy generation. The present results show that minimum entropy generation in the flow system can be achieved with appropriate choice and combination of the various thermophysical parameters.
Acknowledgements
References
- Woods, L.C. Thermodynamics of Fluid Systems; Oxford University Press: Oxford, UK, 1975. [Google Scholar]
- Aıboud, S.; Saouli, S. Entropy analysis for viscoelastic magnetohydrodynamic flow over a stretching surface. Int. J. Non-Linear Mech. 2010, 45, 482–489. [Google Scholar] [CrossRef]
- Sparrow, E.M.; Cess, R.D. Radiation Heat Transfer; Harpercollins College Div: New York, NY, USA, 1978; pp. 7–10. [Google Scholar]
- Raptis, A.; Perdikis, C.; Takhar, H.S. Effect of thermal radiation on MHD flow. Appl. Math. Comput. 2004, 153, 645–649. [Google Scholar] [CrossRef]
- Mbeledogu, I.U.; Amakiri, A.R.C.; Ogulu, A. Unsteady MHD free convection flow of a compressible fluid past a moving vertical plate in the presence of radiative heat transfer. Int. J. Heat Mass Transf. 2007, 50, 1668–1674. [Google Scholar] [CrossRef]
- Bataller, R.C. Radiation effects for the Blasius and Sakiadis flows with a convective surface boundary condition. Appl. Math. Comput. 2008, 206, 832–840. [Google Scholar] [CrossRef]
- Makinde, O.D.; Aziz, A. MHD mixed convection from a vertical plate embedded in a porous medium with a convective boundary condition. Int. J. Therm. Sci. 2010, 49, 1813–1820. [Google Scholar] [CrossRef]
- Makinde, O.D. On MHD heat and mass transfer over a moving vertical plate with a convective surface boundary condition. Can. J. Chem. Eng. 2010, 88, 983–990. [Google Scholar] [CrossRef]
- Narusawa, U. The second-law analysis of mixed convection in rectangular ducts. Heat Mass Transf. 1998, 37, 197–203. [Google Scholar] [CrossRef]
- Sahin, A.Z. Second law analysis of laminar viscous flow through a duct subjected to constant wall temperature. J. Heat Transf. 1998, 120, 76–83. [Google Scholar] [CrossRef]
- Bejan, A. Second-law analysis in heat transfer and thermal design. Adv. Heat Transf. 1982, 15, 1–58. [Google Scholar]
- Bejan, A. Entropy Generation Minimization; CRC: Boca Raton, FL, USA, 1996. [Google Scholar]
- Kobo, N.S.; Makinde, O.D. Second law analysis for a variable viscosity reactive Couette flow under Arrhenius kinetics. Math. Probl. Eng. 2010, 2010, 278104. [Google Scholar] [CrossRef]
- Jalaal, M.; Ganji, D.D.; Ahmadi, G. Analytical investigation on acceleration motion of a vertically falling spherical particle in incompressible Newtonian media. Adv. Powder Technol. 2010, 21, 298–304. [Google Scholar] [CrossRef]
- Jalaal, M.; Ganji, D.D. An analytical study on motion of a sphere rolling down an inclined plane submerged in a Newtonian fluid. Powder Technol. 2010, 198, 82–92. [Google Scholar] [CrossRef]
- Makinde, O.D.; Aziz, A. Second law analysis for a variable viscosity plane Poiseuille flow with asymmetric convective cooling. Comput. Math. Appl. 2010, 60, 3012–3019. [Google Scholar] [CrossRef]
- Makinde, O.D. Thermodynamic second law analysis for a gravity driven variable viscosity liquid film along an inclined heated plate with convective cooling. J. Mech. Sci. Technol. 2010, 24, 899–908. [Google Scholar] [CrossRef]
- Mahmud, S; Tasnim, S.H.; Mamun, H.A.A. Thermodynamic analysis of mixed convection in a channel with transverse hydromagnetic effect. Int. J. Therm. Sci. 2003, 42, 731–740. [Google Scholar] [CrossRef]
- Makinde, O.D.; Beg, O.A. On inherent irreversibility in a reactive hydromagnetic channel flow. J. Therm. Sci. 2010, 19, 72–79. [Google Scholar] [CrossRef]
- Chen, S.; Tolke, J.; Krafczyk, M. Numerical investigation of double-diffusive (natural) convection in vertical annuluses with opposing temperature and concentration gradients. Int. J. Heat Fluid Flow 2010, 31, 217–226. [Google Scholar] [CrossRef]
- Chen, S.; Zheng, C. Entropy generation in impinging flow confined by planar opposing jets. Int. J. Therm. Sci. 2010, 49, 2067–2075. [Google Scholar] [CrossRef]
- Chen, S.; Du, R. Entropy generation of turbulent double-diffusive natural convection in a rectangle cavity. Energy 2011, 36, 1721–1734. [Google Scholar] [CrossRef]
- Chen, S. Analysis of entropy generation in counter-flow premixed hydrogen-air combustion. Int. J. Hydrogen Energy 2010, 35, 1401–1411. [Google Scholar] [CrossRef]
- Chen, S.; Liu, Z.; Liu, J.; Li, J.; Wang, L.; Zheng, C. Analysis of entropy generation in hydrogen-enriched ultra-lean counter-flow methane–air non-premixed combustion. Int. J. Hydrogen Energy 2010, 35, 12491–12501. [Google Scholar] [CrossRef]
- Aziz, A. A similarity solution for laminar thermal boundary layer over a flat plate with a convective surface boundary condition. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 1064–1068. [Google Scholar] [CrossRef]
- Lai, F.C.; Kulacki, F.A. The effect of variable viscosity on convective heat and mass transfer in saturated porous media. Int. J. Heat Mass Transf. 1991, 33, 1028–1031. [Google Scholar] [CrossRef]
- Nachtsheim, P.R.; Swigert, P. Satisfaction of the Asymptotic Boundary Conditions in Numerical Solution of the System of Nonlinear Equations of Boundary Layer Type; NASA TND-3004; NASA: Washington, DC, USA, 1965. [Google Scholar]
© 2011 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Makinde, O.D. Second Law Analysis for Variable Viscosity Hydromagnetic Boundary Layer Flow with Thermal Radiation and Newtonian Heating. Entropy 2011, 13, 1446-1464. https://doi.org/10.3390/e13081446
Makinde OD. Second Law Analysis for Variable Viscosity Hydromagnetic Boundary Layer Flow with Thermal Radiation and Newtonian Heating. Entropy. 2011; 13(8):1446-1464. https://doi.org/10.3390/e13081446
Chicago/Turabian StyleMakinde, Oluwole Daniel. 2011. "Second Law Analysis for Variable Viscosity Hydromagnetic Boundary Layer Flow with Thermal Radiation and Newtonian Heating" Entropy 13, no. 8: 1446-1464. https://doi.org/10.3390/e13081446
APA StyleMakinde, O. D. (2011). Second Law Analysis for Variable Viscosity Hydromagnetic Boundary Layer Flow with Thermal Radiation and Newtonian Heating. Entropy, 13(8), 1446-1464. https://doi.org/10.3390/e13081446




