Diffuser and Nozzle Design Optimization by Entropy Generation Minimization
Abstract
:1. Introduction

2. Head Loss, Dissipation and Entropy Generation
- A -value, defined for all kinds of conduit components like bends, trijunctions but also straight pipe segments, often is defined in terms of pressure differences that occur along that component. For example a straight pipe of diameter D and length L is characterized by a friction factor f withassuming that the flow is fully developed and therefore holds. This definition, as well as all definitionswith as “pressure loss” are appropriate only in special situations. We recommend to use the definition of according to (1) for all conduit components and as the only version to define a head loss coefficient, see [1] for more details, as well as the following point.
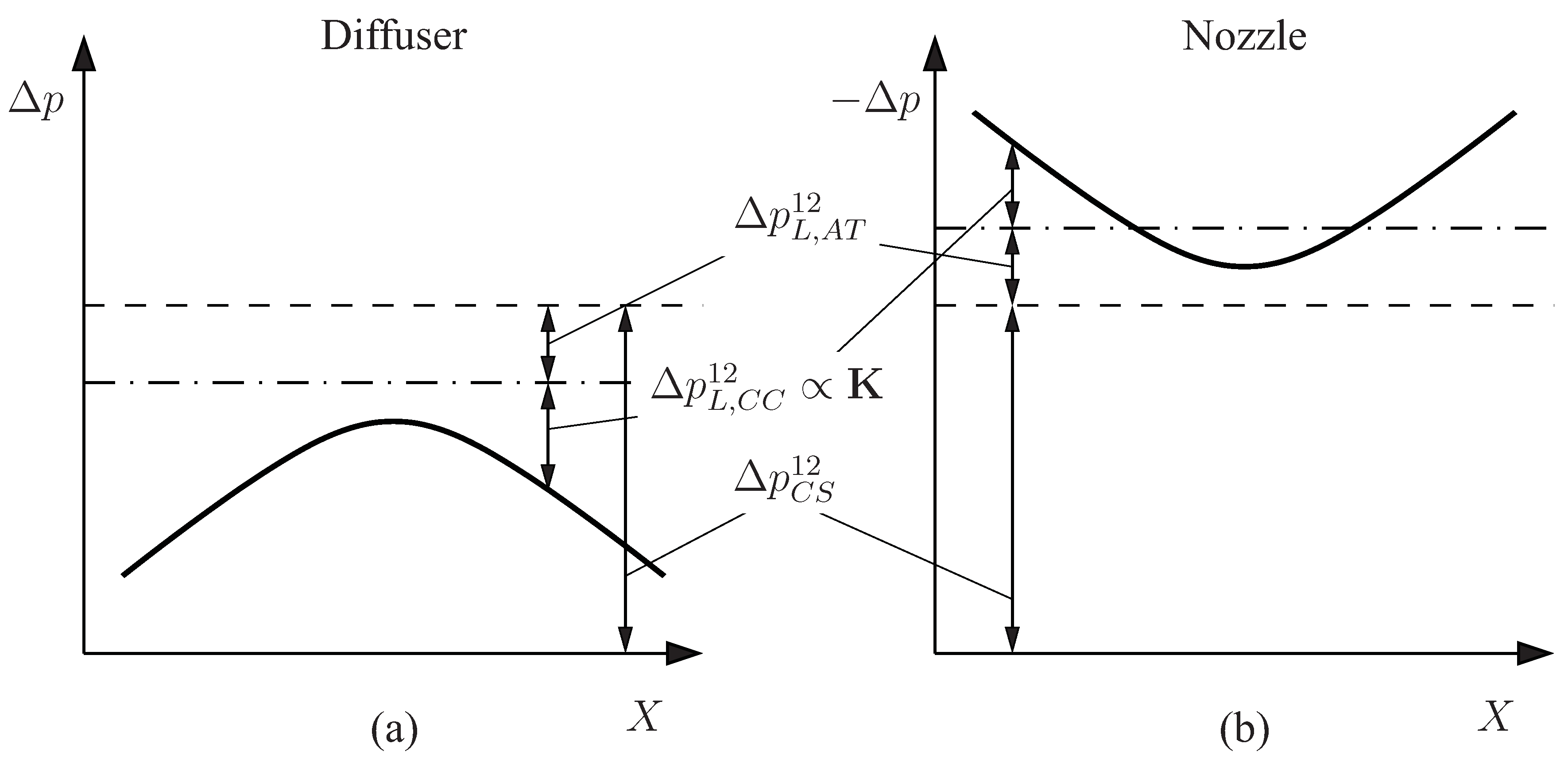
- For conduit components with different cross section areas upstream and downstream (like with diffusers and nozzles, c.f. Figure 1) there should be a careful interpretation with respect to pressure differences which are often measured in order to characterize the components. If is measured with and , being the pressure in cross sections ① and ② in Figure 1, there is
- a change of pressure due to the change of cross section, , corresponding to the difference in kinetic energy in both cross sections.
- a loss of total head due to losses in the flow field, . This can formally be subdivided into two parts, i.e., . Here, is the frictional loss in the adjacent tangents (index: AT) from the conduit component to the cross section ① and ②, respectively. It is determined under the assumption of a fully developed and undisturbed flow in the tangents upstream and downstream. Then corresponds to the loss of total head due to (not in) the conduit component (index: CC). It can be uniquely linked to the head loss coefficient . It is the only part inthat is subject to changes when the shape of the diffuser or nozzle is changed (keeping and unchanged), i.e., when an optimization is performed.This is sketched in Figure 2 where X is a parameter of the geometry that can be altered during the optimization process. With the definition of given above this part of the measured (corresponding to the head loss coefficient) is independent of the exact location of ① and ② in Figure 1, provided both cross sections are outside the zones of influence upstream and downstream of the component.In Figure 2b it has been anticipated that in a nozzle can be negative, i.e., the nozzle may reduce the head loss in the adjacent tangents compared to the assumed loss due to a fully developed and undisturbed flow.
- Losses from a thermodynamic point of view are best described as losses of exergy, sometimes called losses of available work (exergy: maximum theoretical work obtainable from the energy interacting with the environment to equilibrium). In a power cycle, for example, this is especially important, since lost exergy is no longer available in the turbine of that cycle resulting in a reduced cycle efficiency, see [2] for a comprehensive discussion of this issue.Dissipation of mechanical energy, which is directly linked to the head loss coefficient in (2), iswith as mean temperature at which the dissipation process occurs. The specific entropy generated, s, is related to the corresponding specific exergy loss byso that the relation between φ and isHere is the environmental temperature which occurs in the so-called Gouy-Stodola theorem. For these basic thermodynamic relations see standard textbooks like [3,4,5]. Note that all specific values introduced so far are their absolute values referred to the mass or more convenient for an interpretation their absolute values per time, i.e., rates , referred to the mass flux in the flow system.Since according to (8) it depends on the temperature level how much exergy is lost with a certain dissipation the head loss coefficient defined in (1) is not a general measure for the exergy lost in a conduit component.Hence, in addition to a second coefficient called exergy loss coefficient should be introduced. With defined asthere now are two loss coefficients and related to each other byIf, for example, the head loss occurs in a power cycle at an upper temperature level , the temperature ratio may be . Thus, the exergy loss is only one third of what it would be if the same flow would have the ambient temperature instead of .
- During the optimization process described hereafter, -values of the diffusers and nozzles will have to be determined. According to (2) this can either be done by the determination of φ (dissipation rate per mass flux) or of s (entropy generation rate per mass flux). We definitely prefer s since it is the more fundamental quantity of the conversion process (mechanical → thermal energy). A further argument in favor of the specific entropy is that then a common quantity exists when also losses (of exergy) in a superimposed heat transfer process are accounted for, see [1] for more details of this convective heat transfer situation.
3. Review of Literature
3.1. Literature about Entropy in General
3.2. Literature about Entropy Generation
3.3. Literature about Optimization Processes
4. Entropy Generation as an Optimization Criterion
4.1. Determination of the Overall Entropy Generation Rate
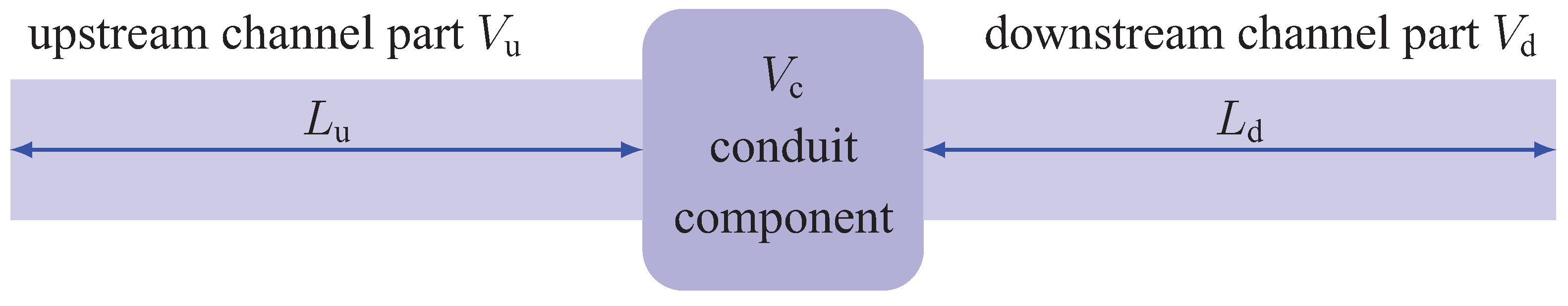
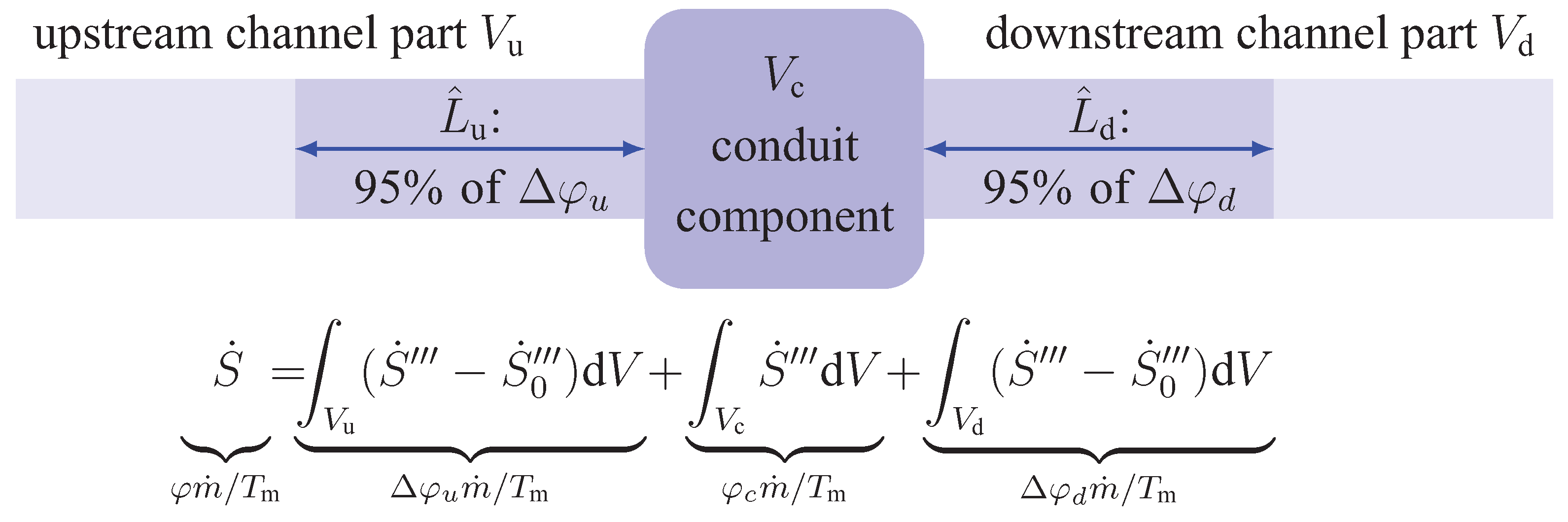
4.2. An Example
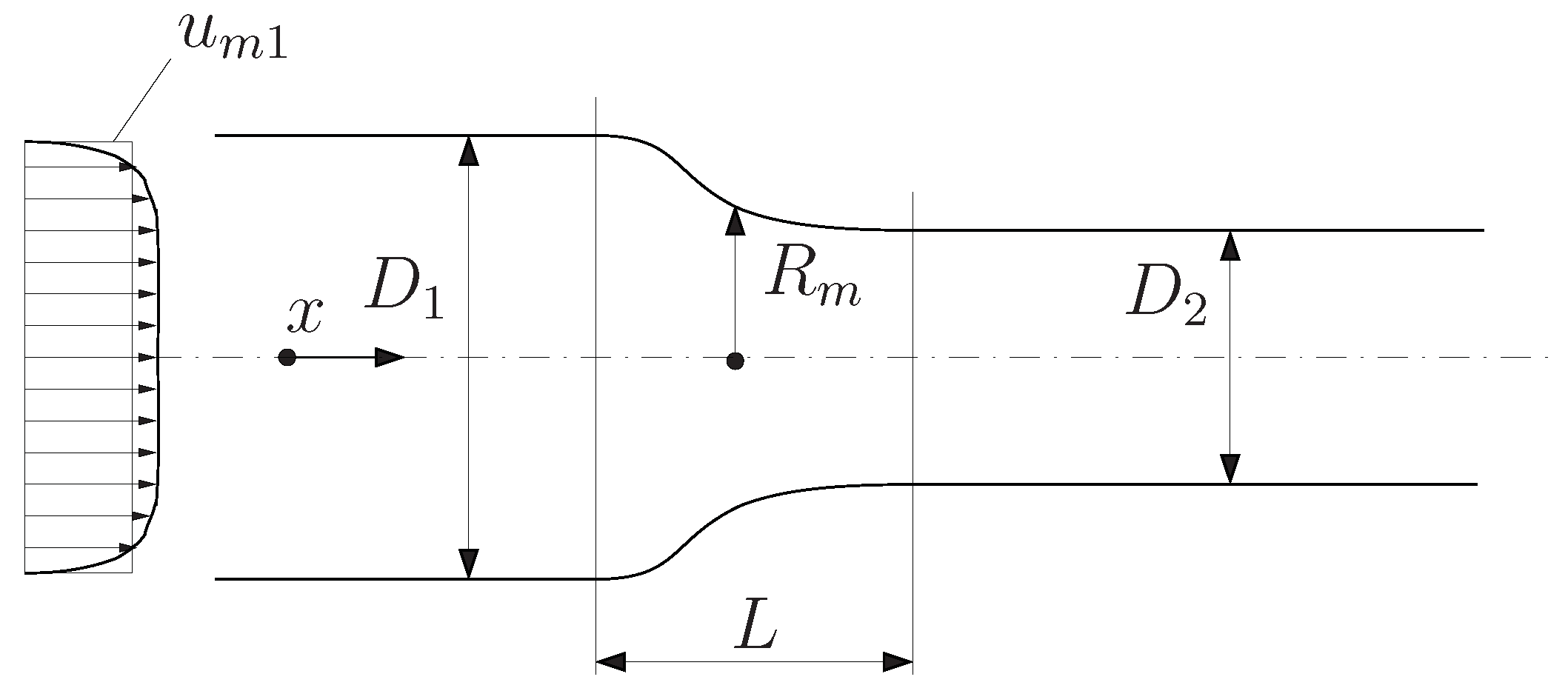
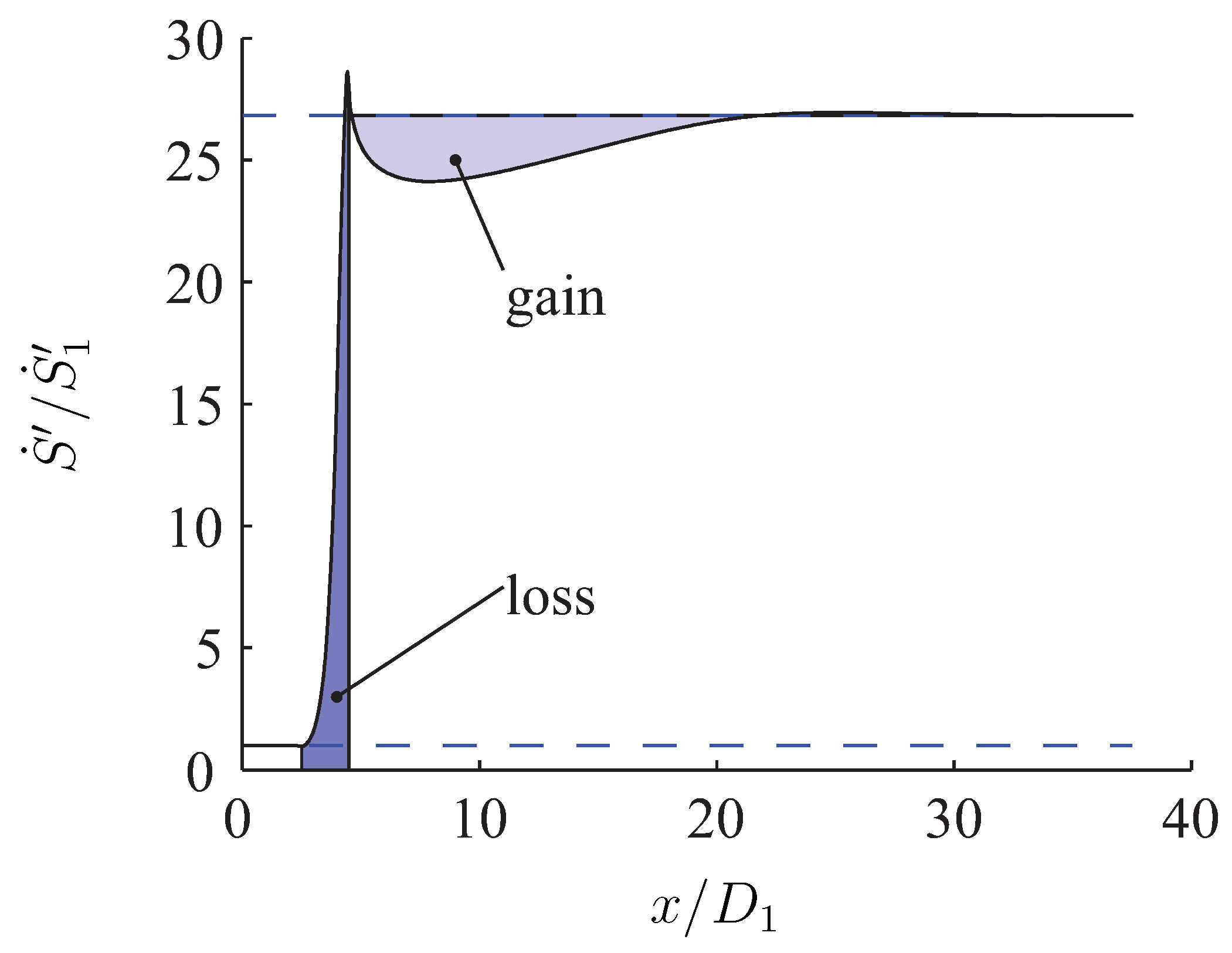
- the additional entropy generation upstream,
- the entropy generation in the component (nozzle),
- the additional entropy generation downstream, , which here is negative
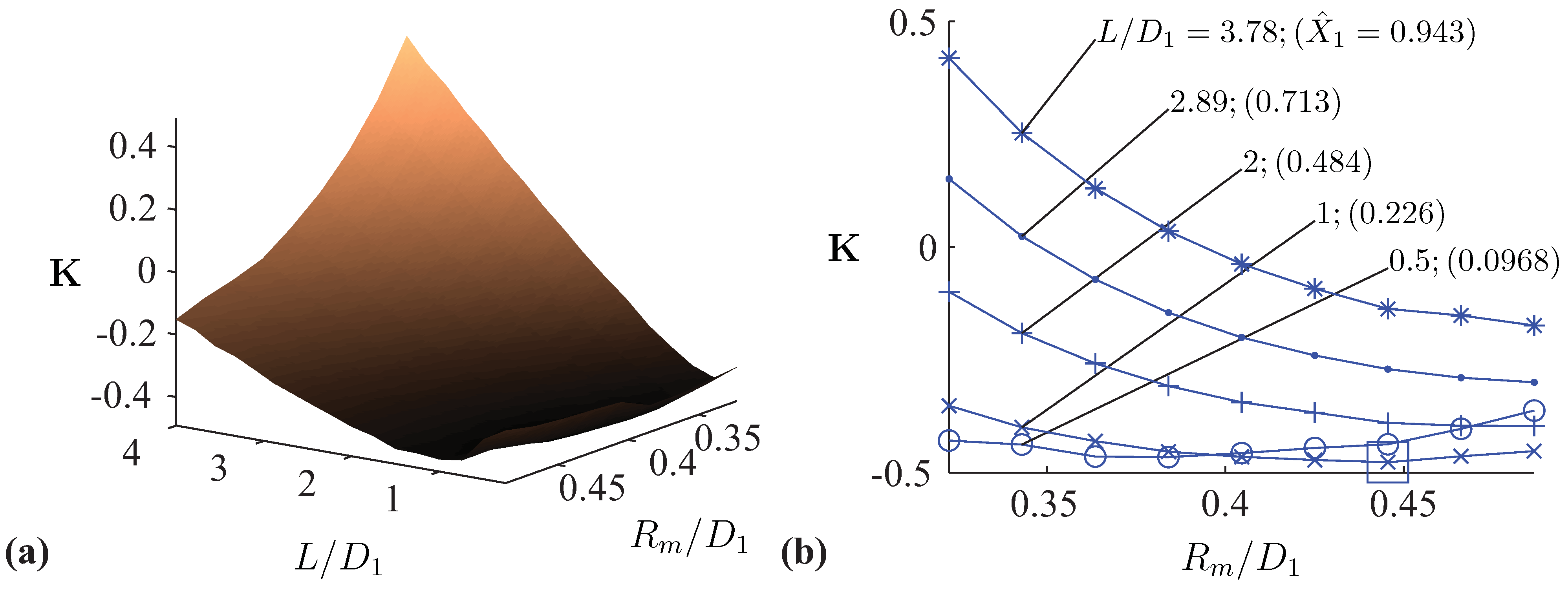
| K | |||
|---|---|---|---|
| (24) | 1.088 | 1.069 | −0.125 |
| (24) and | 1.077 | 1.060 | 0.018 |
| 1.0 | 1.0 | 0.897 |
5. Nozzle Optimization
5.1. Numerical Details

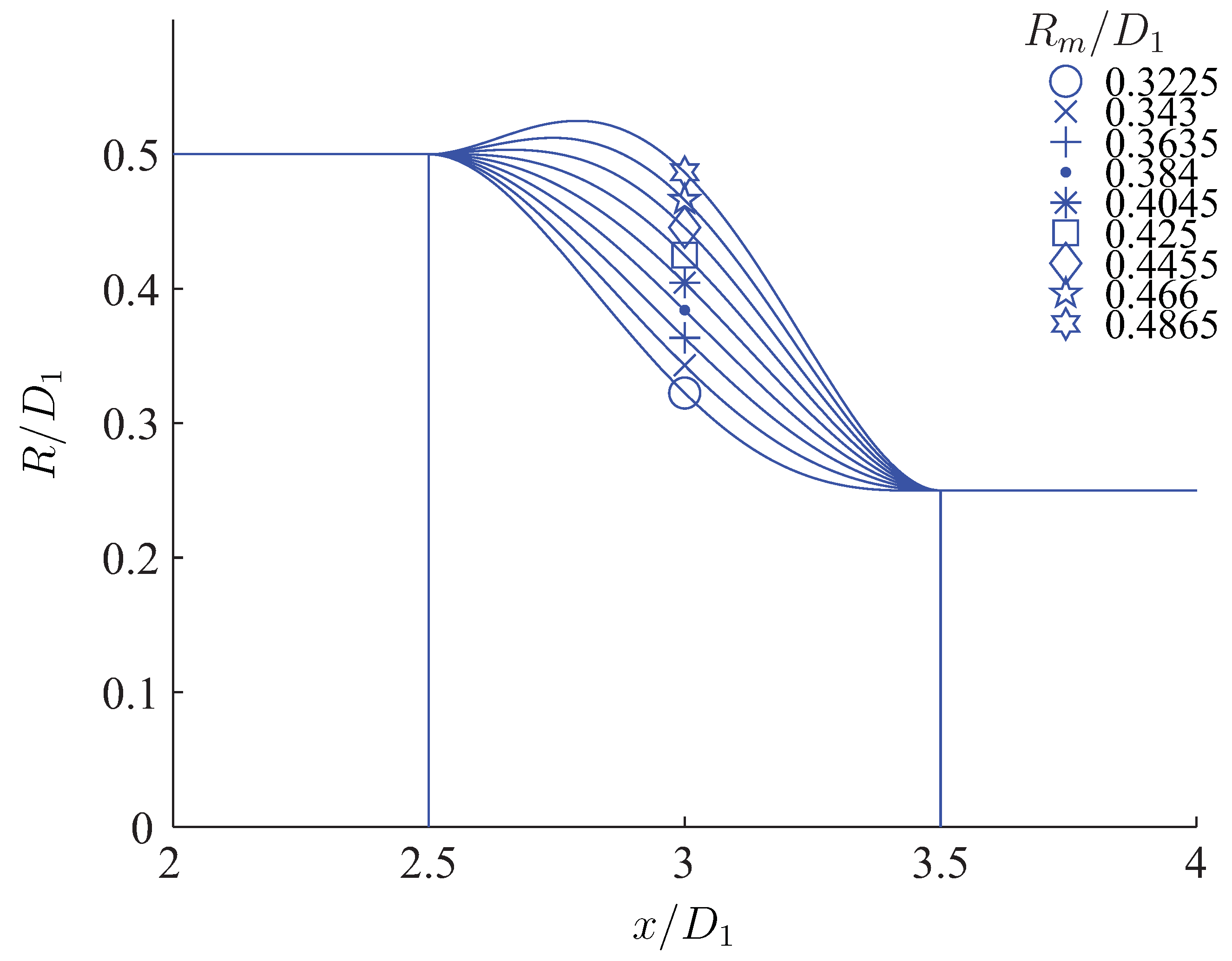
5.2. Nozzle Optimization for the Polynomial Wall Shape,
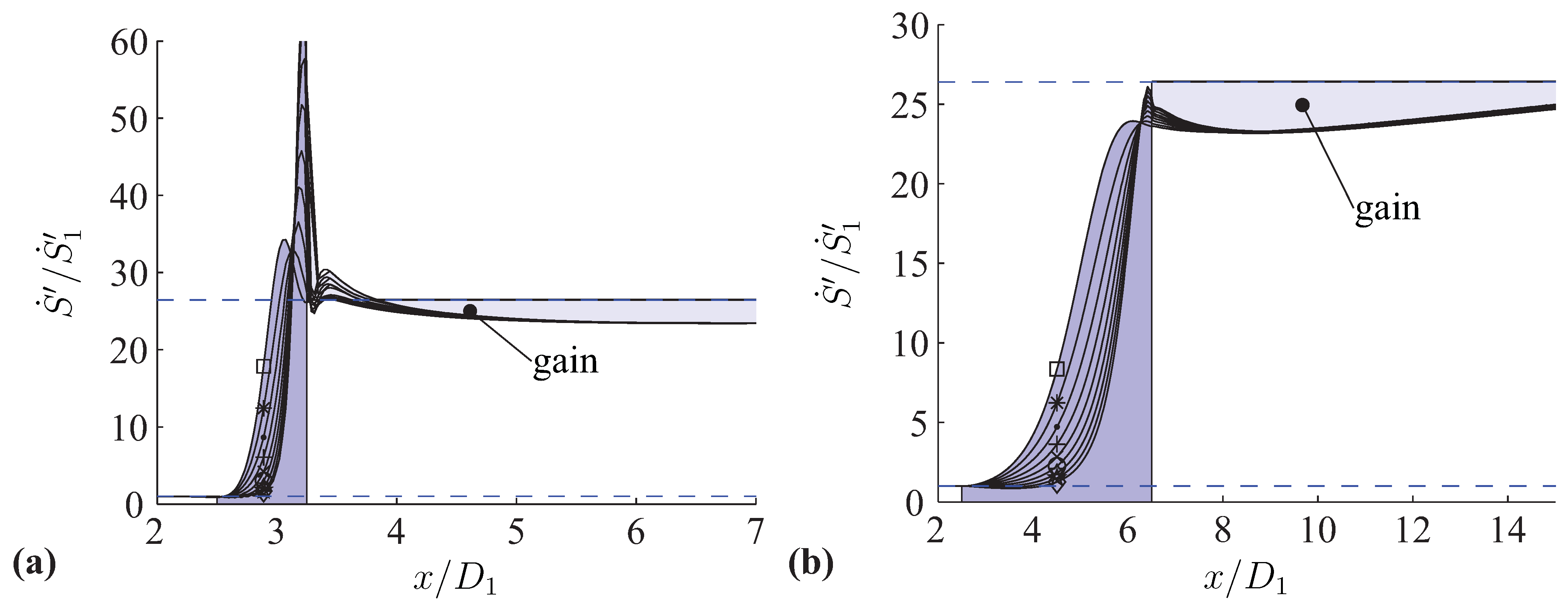

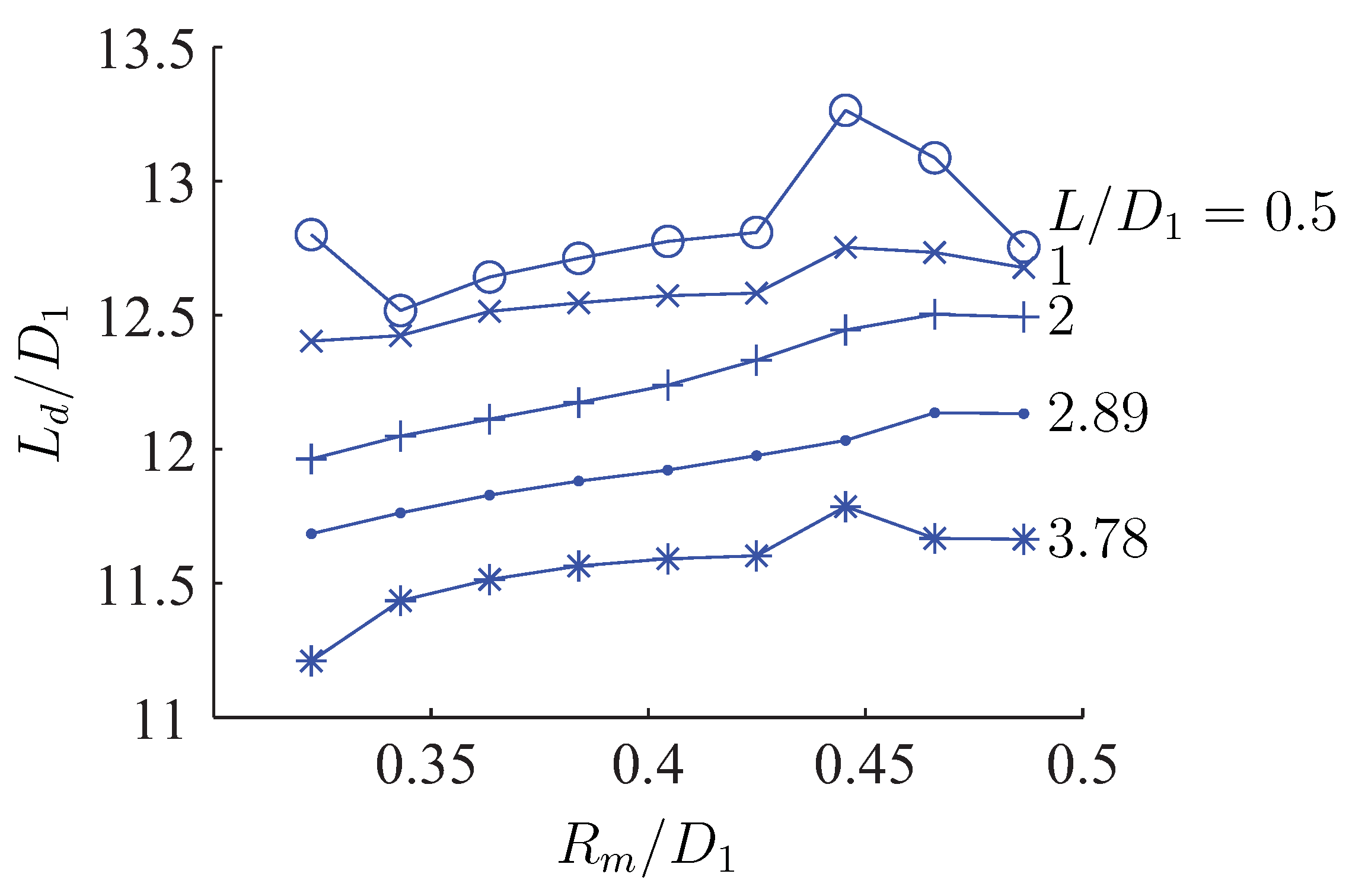
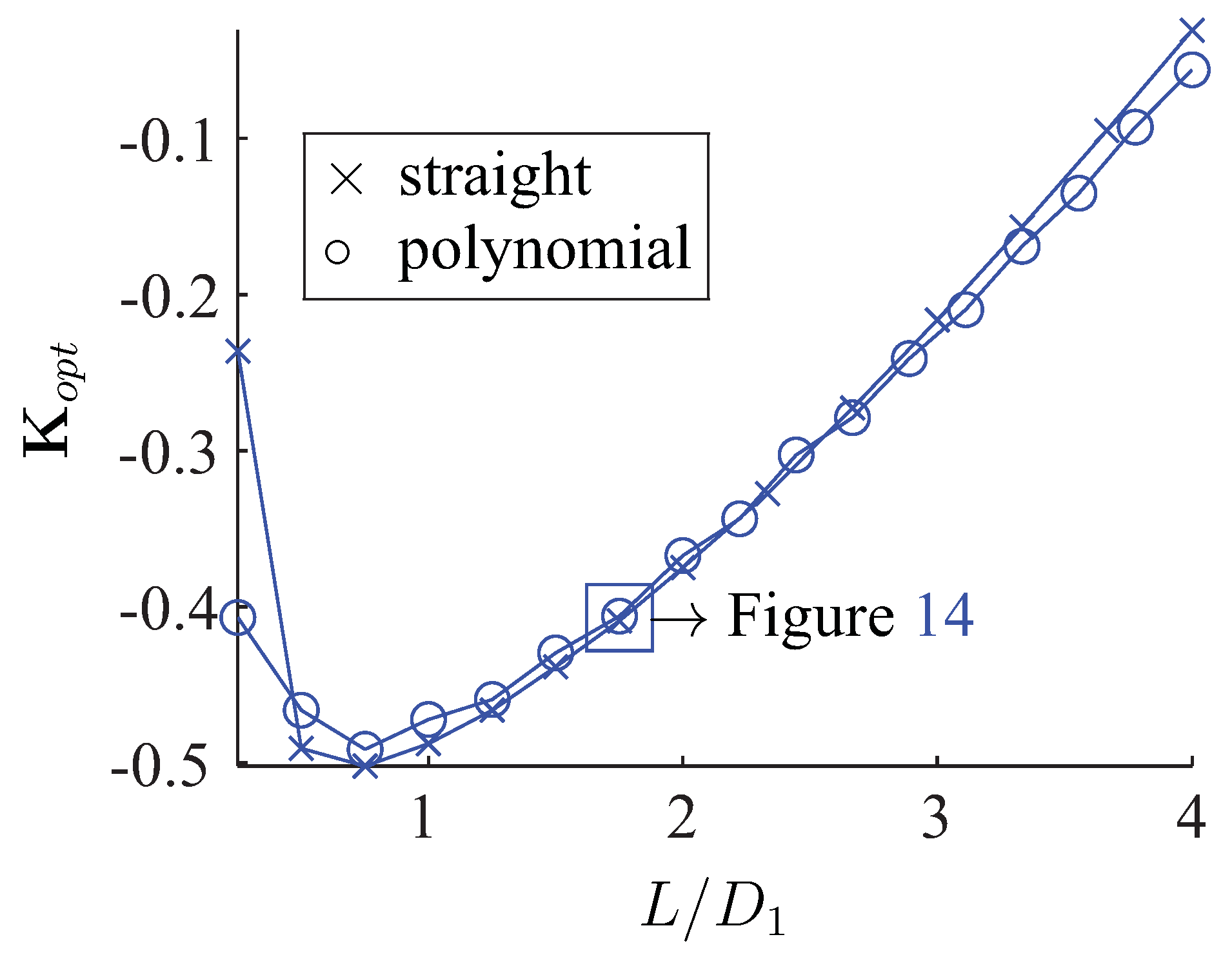
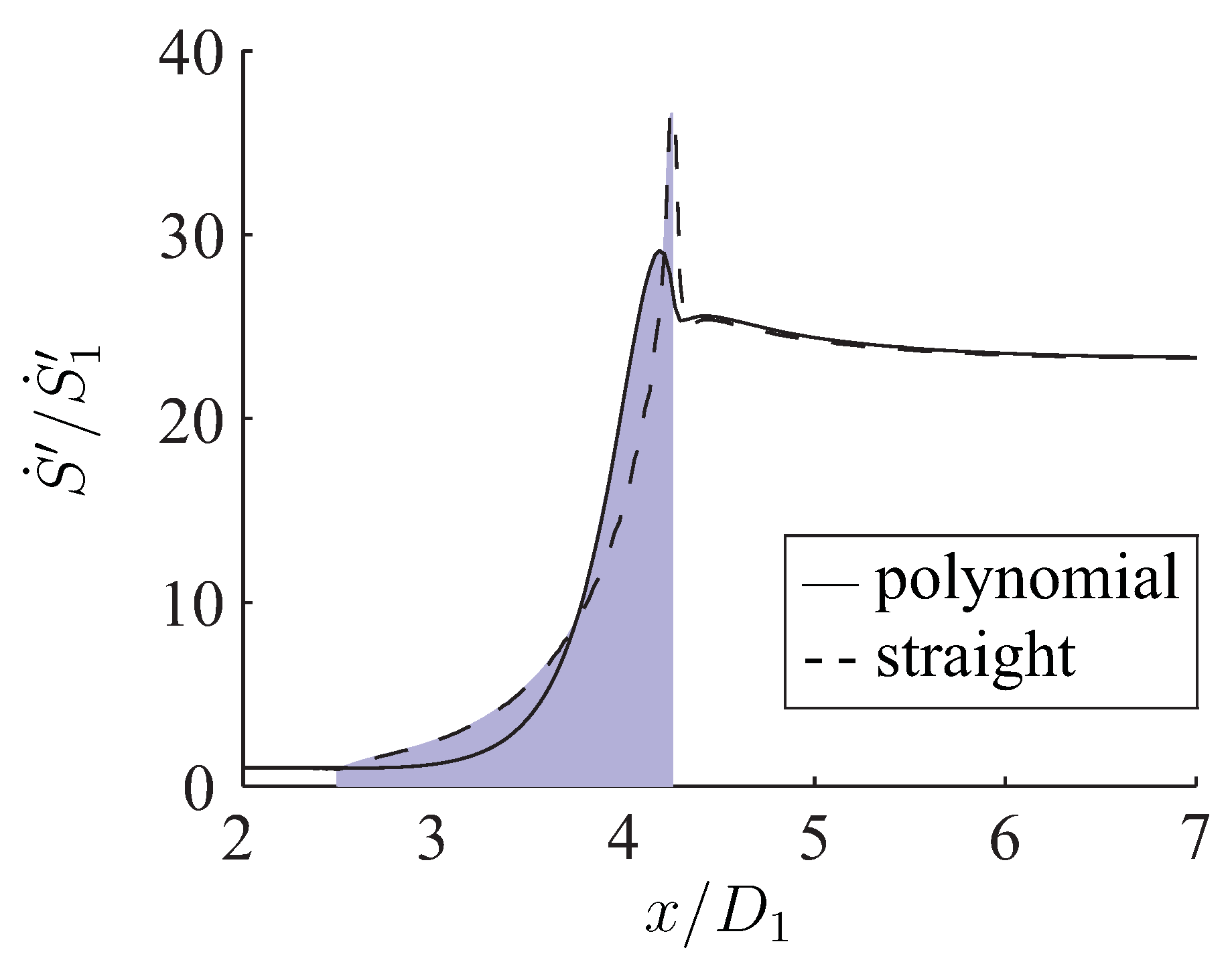
6. Diffuser Optimization

6.1. Diffuser Optimization for the Polynomial Wall Shape;

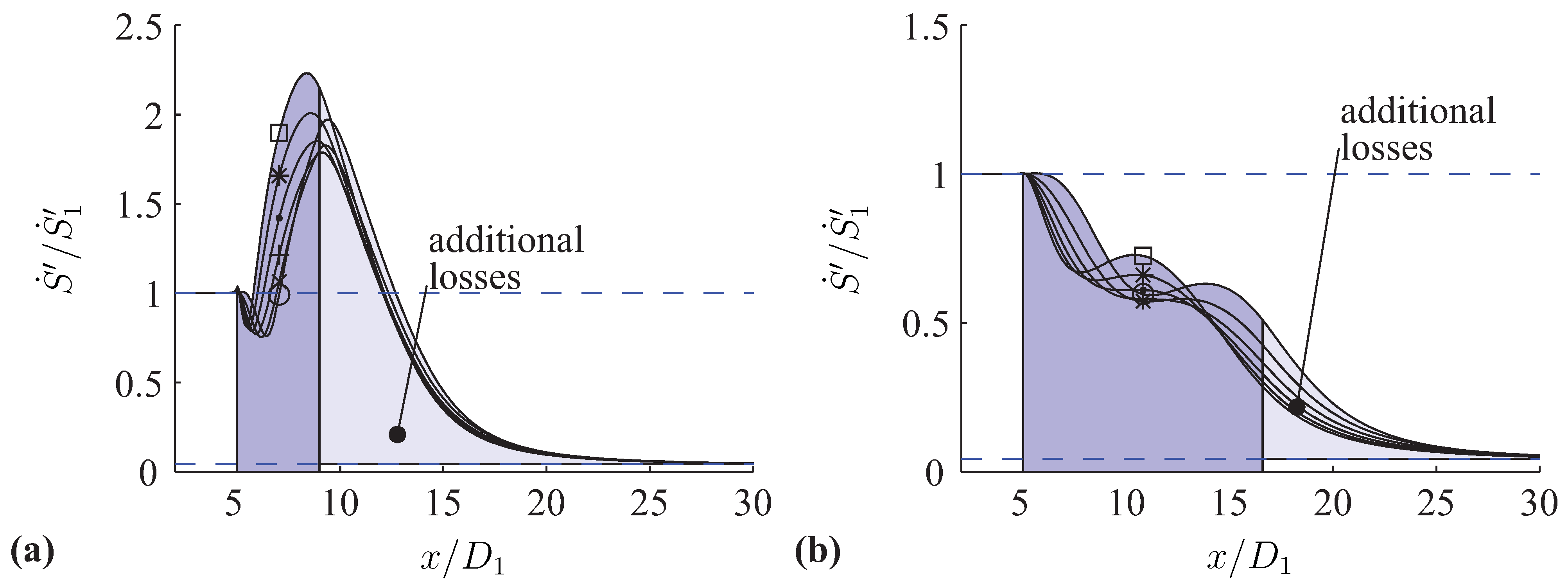

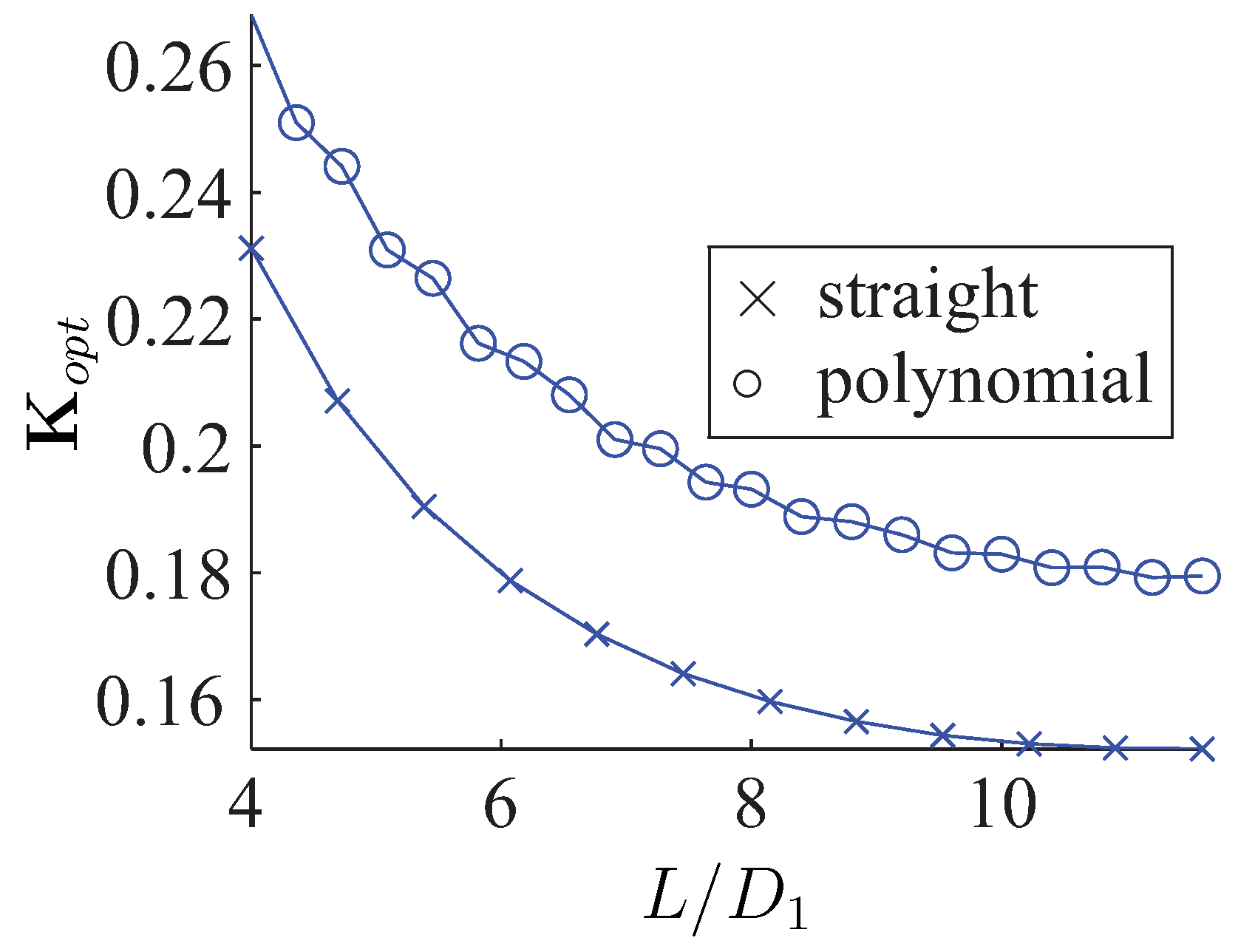
6.2. Diffuser Optimization for a Non-Straight Wall Shape;
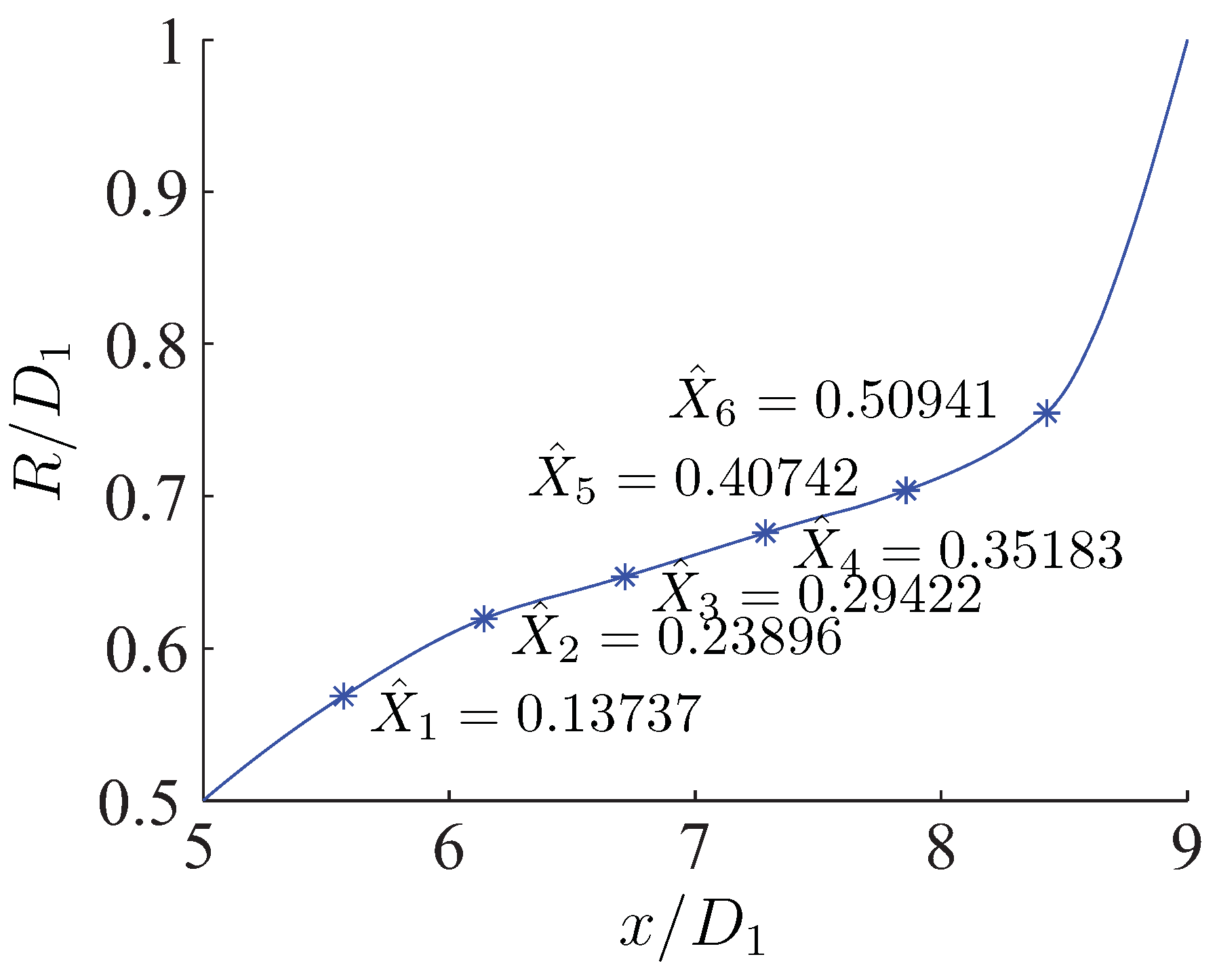
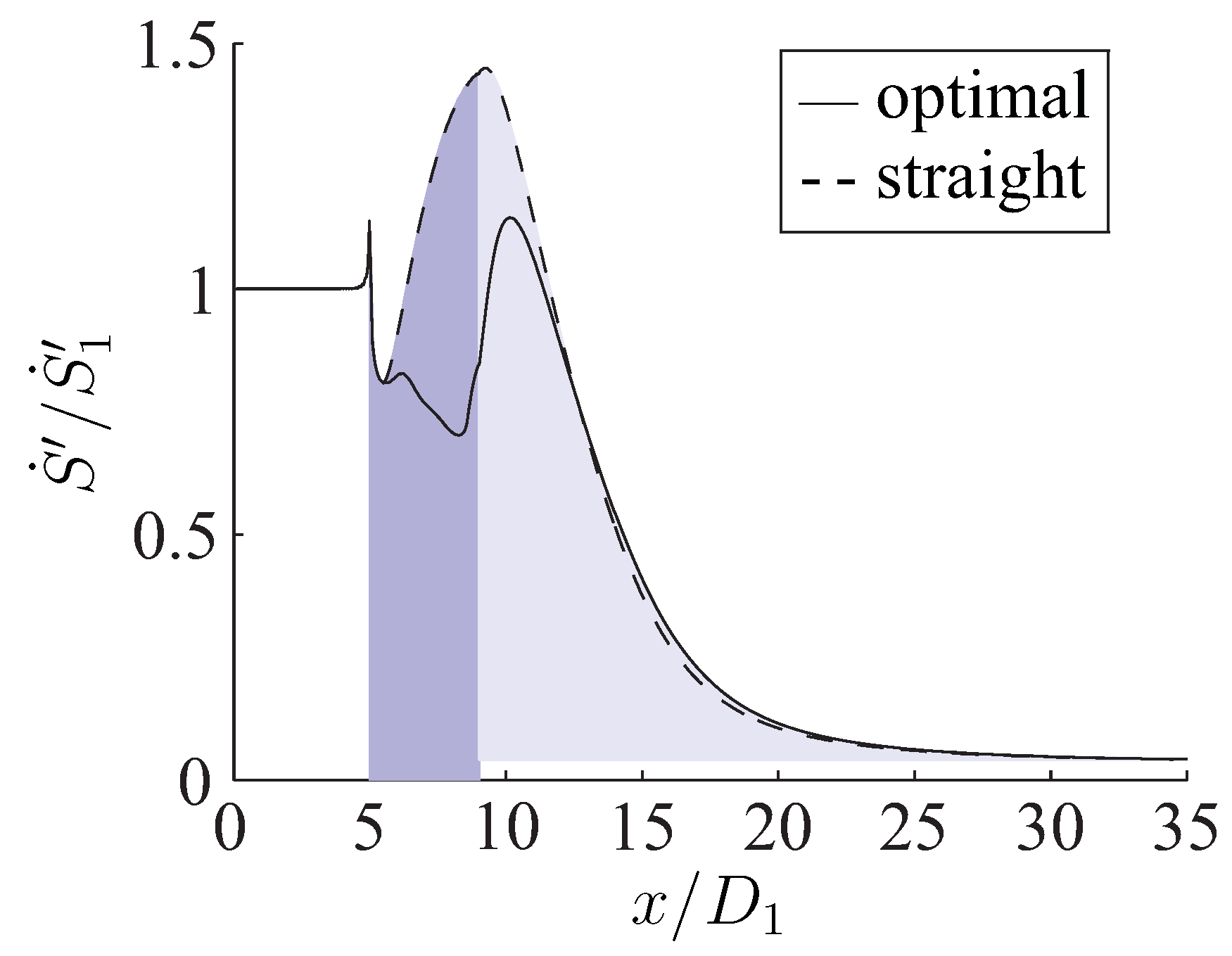


7. Conclusions
Acknowledgements
References
- Herwig, H. The role of entropy generation in momentum and heat transfer. In Proceedings of the International Heat Transfer Conference, Washington D.C., USA, 8–13 August 2010. No. IHTC14-23348.
- Schmandt, B.; Herwig, H. Internal flow losses: A fresh look at old concepts. J. Fluids Eng. 2011, 133, 051201. [Google Scholar] [CrossRef]
- Moran, M.; Shapiro, H. Fundamentals of Engineering Thermodynamics, 3rd ed.; John Wiley & Sons, Inc.: New York, NY, USA, 1996. [Google Scholar]
- Baehr, H. Thermodynamik, 14th ed.; Springer-Verlag: Berlin, Germany, 2009. [Google Scholar]
- Herwig, H.; Kautz, C. Technische Thermodynamik; Pearson Studium: München, Germany, 2007. [Google Scholar]
- Dugdale, J. Entropy and Its Physical Meaning.; Cambridge University Press: Cambridge, UK, 1996. [Google Scholar]
- Atkins, P. The Second Law; Scientific American Books, W.H. Freeman and Company: New York, NY, USA, 1984. [Google Scholar]
- Goldstein, M.; Goldstein, I. The Refrigerator and the Universe; Harvard University Press: Cambridge, MA, USA, 1993. [Google Scholar]
- Falk, G.; Ruppel, W. Energie und Entropie; Springer-Verlag: Berlin, Germany, 1976. [Google Scholar]
- Lieb, E.; Yngvason, J. A fresh look at entropy and the second law of thermodynamics. Physics Today 2000, 11, 106. [Google Scholar]
- Beretta, G.; Ghoniem, A.; Hatsopoulos, G. Meeting the entropy challenge. AIP Conference Proceedings 2008, CP 1033, 382. [Google Scholar]
- Bejan, A. The concept of irreversibility in heat exchanger design: counter-flow heat exchangers for gas-to-gas applications. J. Heat Tran. 1977, 99, 274–380. [Google Scholar] [CrossRef]
- Sekulic, D. Entropy generation in a heat exchanger. Heat Tran. Eng. 1986, 7, 83–88. [Google Scholar] [CrossRef]
- Gaggioli, R. Second law analysis for process and energy engineering. In Efficiency and Costing, Second Laws Analysis of Processes; American Chemical Society: New York, NY, USA, 1983. [Google Scholar]
- Bejan, A. A study of entropy generation in fundamental convective heat transfer. J. Heat Tran. 1979, 101, 718–725. [Google Scholar] [CrossRef]
- Bejan, A. Entropy Generation through Heat and Fluid Flow; John Wiley & Sons: New York, NY, USA, 1982. [Google Scholar]
- Bejan, A. Entropy Generation Minimization; CRC Press: Boca Raton, New York, NY, 1996. [Google Scholar]
- Hesselgreaves, J. Rationalisation of second law analysis of heat exchangers. J. Heat Mass Tran. 2000, 43, 4189–4204. [Google Scholar] [CrossRef]
- Herwig, H.; Kock, F. Direct and indirect methods of calculating entropy generation rates in turbulent convective heat transfer problems. Heat Mass Tran. 2007, 43, 207–215. [Google Scholar] [CrossRef]
- Anand, D. Second law analysis of solar powered absorption cooling cycles and systems. J. Sol. Energy Eng. 1984, 106, 291–298. [Google Scholar] [CrossRef]
- Nuwayhid, R.; Moukalled, F.; Noueihed, N. On entropy generation in thermoelectric devices. Energ. Conv. Manage. 2000, 41, 891–914. [Google Scholar] [CrossRef]
- Assad, E. Thermodynamic analysis of an irreversible MHD power plant. Int. J. Energ. Res. 2000, 24, 865–875. [Google Scholar] [CrossRef]
- Shiba, T.; Bejan, A. Thermodynamic optimization of geometric structure in the counterflow heat exchanger for an environmental control system. Energy 2001, 26, 493–511. [Google Scholar] [CrossRef]
- Saidi, M.; Yazdi, M. Exergy model of a vortex tube system with experimental results. Energy 1999, 24, 625–632. [Google Scholar] [CrossRef]
- San, J.; Jan, C. Second law analysis of a wet crossflow heat exchanger. Energy 2000, 25, 939–955. [Google Scholar] [CrossRef]
- Ko, T.; Ting, K. Entropy generation and optimal analysis for laminar forced convection in curved rectangular ducts: A numerical study. Int. J. Therm. Sci. 2006, 45, 138–150. [Google Scholar] [CrossRef]
- Madsen, J.I.; Shyy, W. Response surface techniques for diffuser shape optimization. AIAA J. 2000, 38, 1512–1518. [Google Scholar] [CrossRef]
- Ghosh, S.; Pratihat, D.; Das, P. An evolutionary optimization of diffuser shapes based on CFD simulations. Int. J. Numer. Meth. Fluids 2010, 63, 1147–1166. [Google Scholar] [CrossRef]
- Thevenin, D.; Janiga, D. Optimization and Computational Fluid Dynamics; Springer-Verlag: Berlin, Heidelberg, Germany, 2008. [Google Scholar]
- Kock, F.; Herwig, H. Local entropy production in turbulent shear flows: A high Reynolds number model with wall functions. Int. J. Heat Mass Tran. 2004, 47, 2205–2215. [Google Scholar] [CrossRef]
- Menter, F. Improved two-equation k-omega turbulence models for aerodynamic flows. NASA Tech. Memorand. 1992, 103975. [Google Scholar]
- OpenFOAM User Guide; Version 1.6; OpenCFD, Ltd.: Reading, Berkshire, UK, 2009.
- Matlab 7: Mathematics, from Mathworks Userguide 2010a; The MathWorks, Inc.: Natick, MA, USA, 2010.
© 2011 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Schmandt, B.; Herwig, H. Diffuser and Nozzle Design Optimization by Entropy Generation Minimization. Entropy 2011, 13, 1380-1402. https://doi.org/10.3390/e13071380
Schmandt B, Herwig H. Diffuser and Nozzle Design Optimization by Entropy Generation Minimization. Entropy. 2011; 13(7):1380-1402. https://doi.org/10.3390/e13071380
Chicago/Turabian StyleSchmandt, Bastian, and Heinz Herwig. 2011. "Diffuser and Nozzle Design Optimization by Entropy Generation Minimization" Entropy 13, no. 7: 1380-1402. https://doi.org/10.3390/e13071380
APA StyleSchmandt, B., & Herwig, H. (2011). Diffuser and Nozzle Design Optimization by Entropy Generation Minimization. Entropy, 13(7), 1380-1402. https://doi.org/10.3390/e13071380





