An Analytical Technique to Solve the System of Nonlinear Fractional Partial Differential Equations
Abstract
:1. Introduction
2. Definitions and Preliminary Concepts
3. Idea of Fractional Laplace–Adomian Decomposition Method
4. Results
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Helal, M.A.; Mehanna, M.S. A comparative study between two different methods for solving the general Kortweg–de Vries equation (GKdV). Chaos Solitons Fractals 2007, 33, 725–739. [Google Scholar] [CrossRef]
- Jibran, M.; Nawaz, R.; Khan, A.; Afzal, S. Iterative Solutions of Hirota Satsuma Coupled KDV and Modified Coupled KDV Systems. Math. Probl. Eng. 2018, 2018, 9042039. [Google Scholar] [CrossRef]
- Biazar, J.; Eslami, M. A new homotopy perturbation method for solving systems of partial differential equations. Comput. Math. Appl. 2011, 62, 225–234. [Google Scholar] [CrossRef] [Green Version]
- Kumar, A.; Pankaj, R.D. Laplace-Modified Decomposition Method for the Generalized Hirota-Satsuma Coupled KdV Equation. Can. J. Basic Appl. Sci. 2015, 3, 126–133. [Google Scholar]
- Wang, M.; Zhou, Y.; Li, Z. Application of a homogeneous balance method to exact solutions of nonlinear equations in mathematical physics. Phys. Lett. A 1996, 216, 67–75. [Google Scholar] [CrossRef]
- Gokdogan, A.; Yildirim, A.; Merdan, M. Solving coupled-KdV equations by differential transformation method. World Appl. Sci. J. 2012, 19, 1823–1828. [Google Scholar]
- Jafari, H.; Firoozjaee, M.A. Homotopy analysis method for solving KdV equations. Surv. Math. Its Appl. 2010, 5, 89–98. [Google Scholar]
- Mohamed, M.A.; Torky, M.S. Numerical solution of nonlinear system of partial differential equations by the Laplace decomposition method and the Pade approximation. Am. J. Comput. Math. 2013, 3, 175. [Google Scholar] [CrossRef]
- Seadawy, A.R.; El-Rashidy, K. Water wave solutions of the coupled system Zakharov-Kuznetsov and generalized coupled KdV equations. Sci. World J. 2014, 2014, 724759. [Google Scholar] [CrossRef]
- González-Gaxiola, O. The Laplace–Adomian Decomposition Method Applied to the Kundu-Eckhaus Equation. arXiv 2017, arXiv:1704.07730. [Google Scholar]
- Alhendi, F.A.; Alderremy, A.A. Numerical Solutions of Three-Dimensional Coupled Burgers’ Equations by Using Some Numerical Methods. J. Appl. Math. Phys. 2016, 4, 2011. [Google Scholar] [CrossRef]
- Jafari, H.; Khalique, C.M.; Nazari, M. Application of the Laplace decomposition method for solving linear and nonlinear fractional diffusion–wave equations. Appl. Math. Lett. 2011, 24, 1799–1805. [Google Scholar] [CrossRef]
- Khan, M.; Hussain, M.; Jafari, H.; Khan, Y. Application of Laplace decomposition method to solve nonlinear coupled partial differential equations. World Appl. Sci. J. 2010, 9, 13–19. [Google Scholar]
- Khan, M.; Hussain, M. Application of Laplace decomposition method on semi-infinite domain. Numer. Algorithms 2011, 56, 211–218. [Google Scholar] [CrossRef]
- Mohamed, M.Z. Comparison between the Laplace Decomposition Method and Adomian Decomposition in Time-Space Fractional Nonlinear Fractional Differential Equations. Appl. Math. 2018, 9, 448. [Google Scholar] [CrossRef]
- Al-Zurigat, M. Solving nonlinear fractional differential equation using a multi-step Laplace Adomian decomposition method. Ann. Univ. Craiova-Math. Comput. Sci. Ser. 2012, 39, 200–210. [Google Scholar]
- Haq, F.; Shah, K.; ur Rahman, G.; Shahzad, M. Numerical solution of fractional order smoking model via laplace Adomian decomposition method. Alex. Eng. J. 2018, 57, 1061–1069. [Google Scholar] [CrossRef]
- Haq, F.; Shah, K.; Khan, A.; Shahzad, M.; Rahman, G. Numerical solution of fractional order epidemic model of a vector born disease by Laplace Adomian decomposition method. Punjab Univ. J. Math. 2017, 49, 13–22. [Google Scholar]
- Mahmood, S.; Shah, R.; Arif, M. Laplace Adomian Decomposition Method for Multi Dimensional Time Fractional Model of Navier-Stokes Equation. Symmetry 2019, 11, 149. [Google Scholar] [CrossRef]
- Shah, R.; Khan, H.; Arif, M.; Kumam, P. Application of Laplace–Adomian Decomposition Method for the Analytical Solution of Third-Order Dispersive Fractional Partial Differential Equations. Entropy 2019, 21, 335. [Google Scholar] [CrossRef]
- Thabet, H.; Kendre, S.; Chalishajar, D. New analytical technique for solving a system of nonlinear fractional partial differential equations. Mathematics 2017, 5, 47. [Google Scholar] [CrossRef]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; Wiley-Interscience: New York, NY, USA, 1993. [Google Scholar]
- Hilfer, R. Applications of Fractional Calculus in Physics; World Sci. Publishing: River Edge, NJ, USA, 2000. [Google Scholar]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Elsevier: New York, NY, USA, 1998; Volume 198. [Google Scholar]
- Naghipour, A.; Manafian, J. Application of the Laplace Adomian decomposition and implicit methods for solving Burgers’ equation. TWMS J. Pure Appl. Math. 2015, 6, 68–77. [Google Scholar]
- Kaya, D. An application of the decomposition method for the KdVB equation. Appl. Math. Comput. 2004, 152, 279–288. [Google Scholar] [CrossRef]
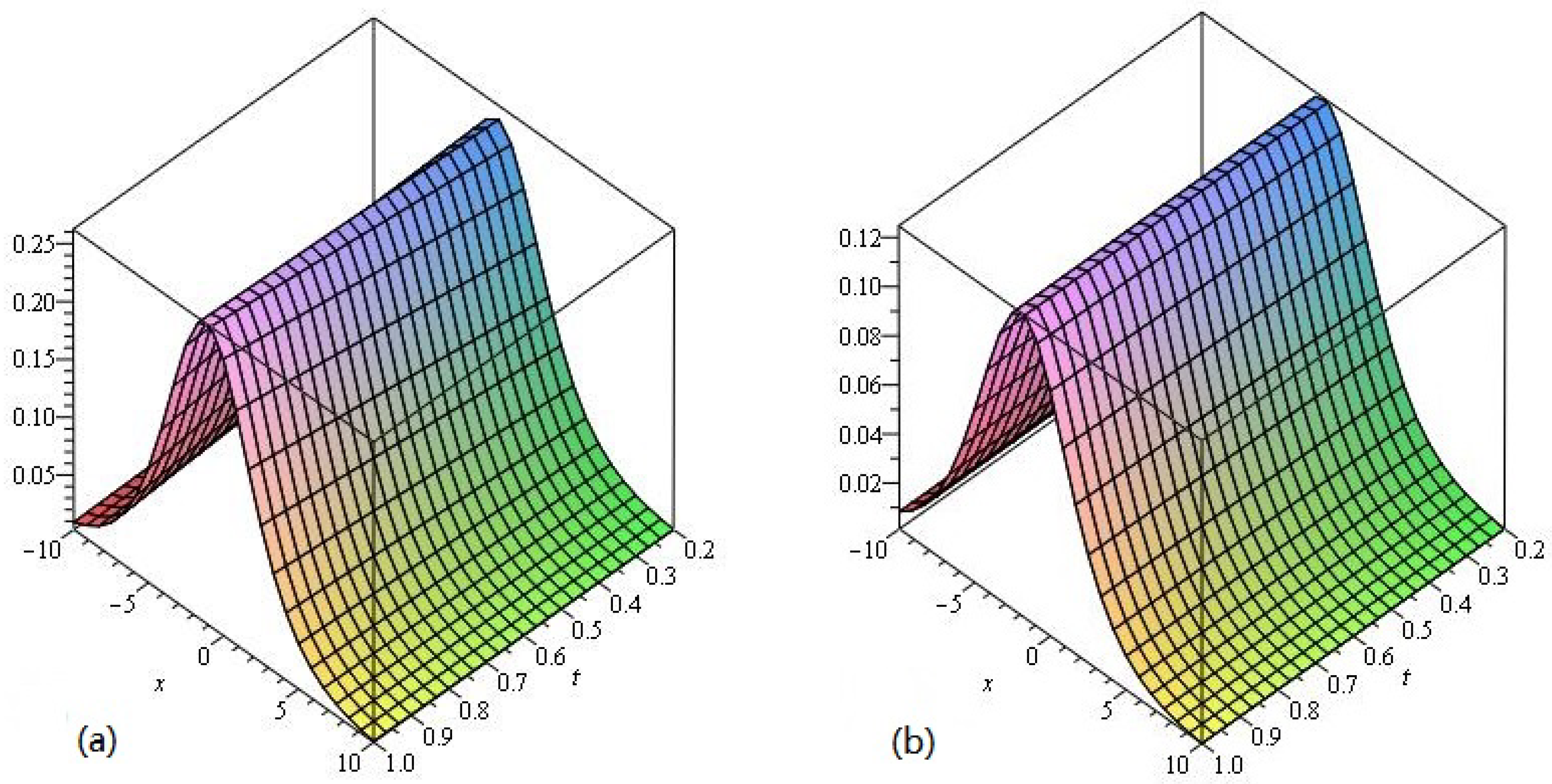
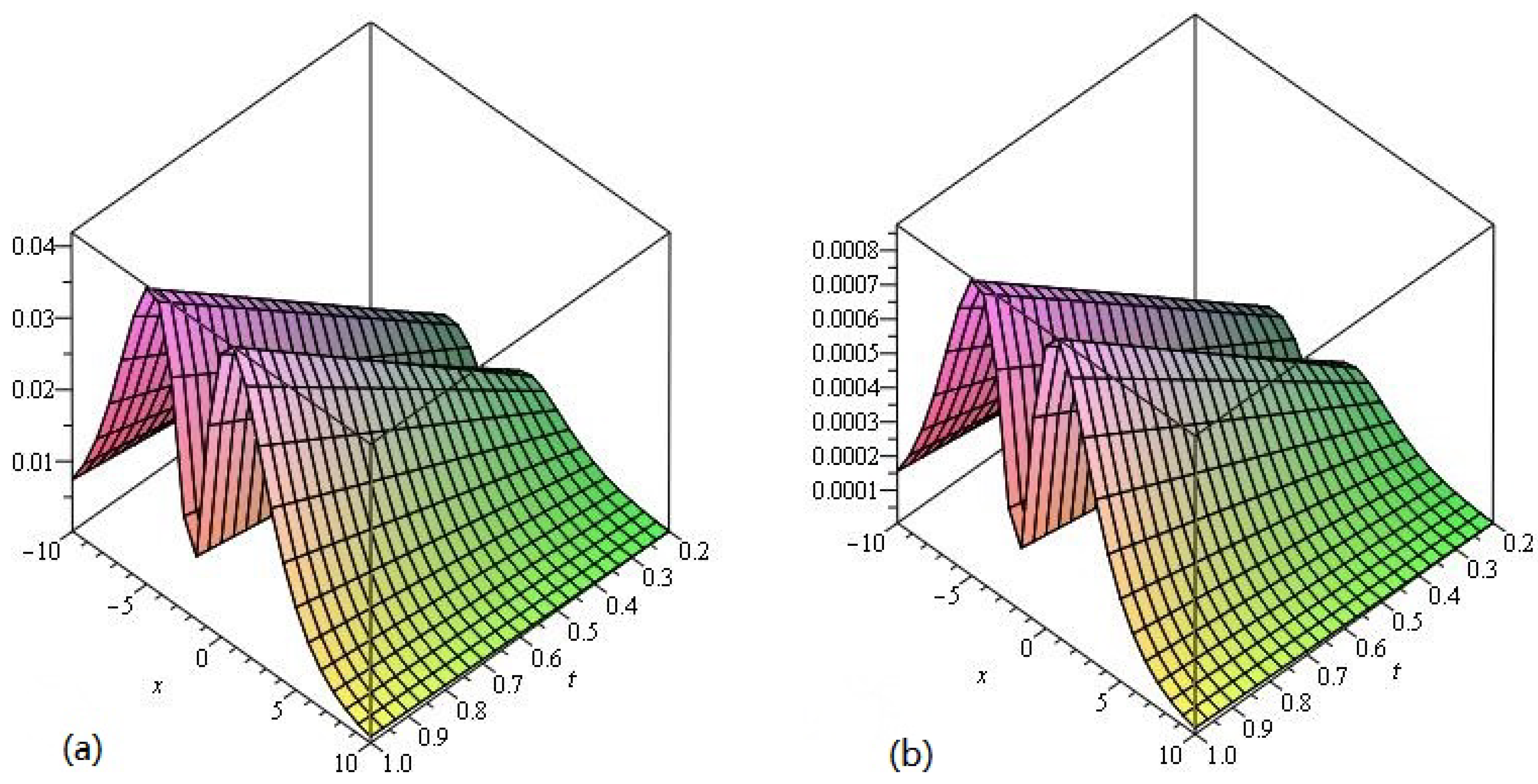
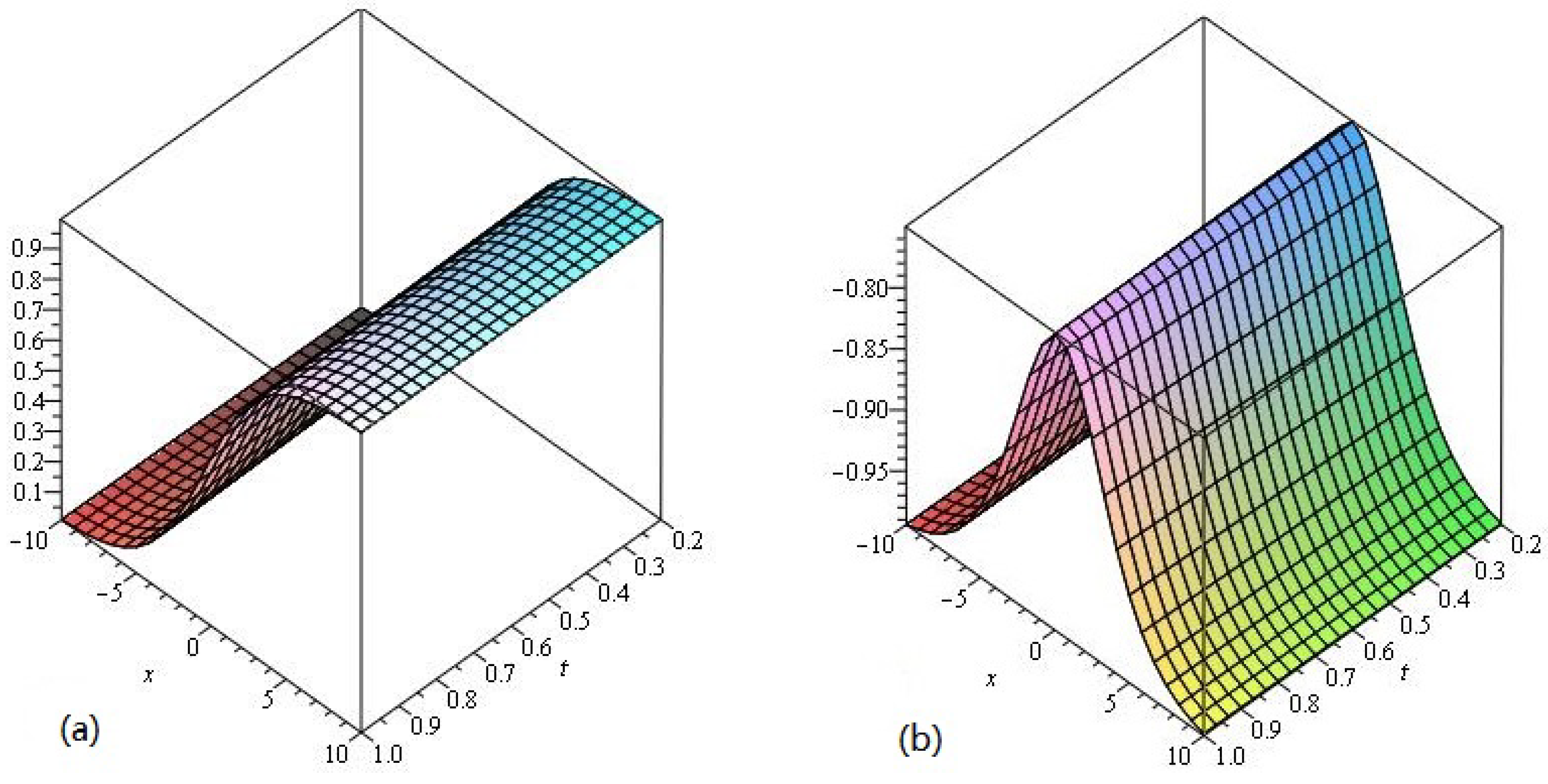
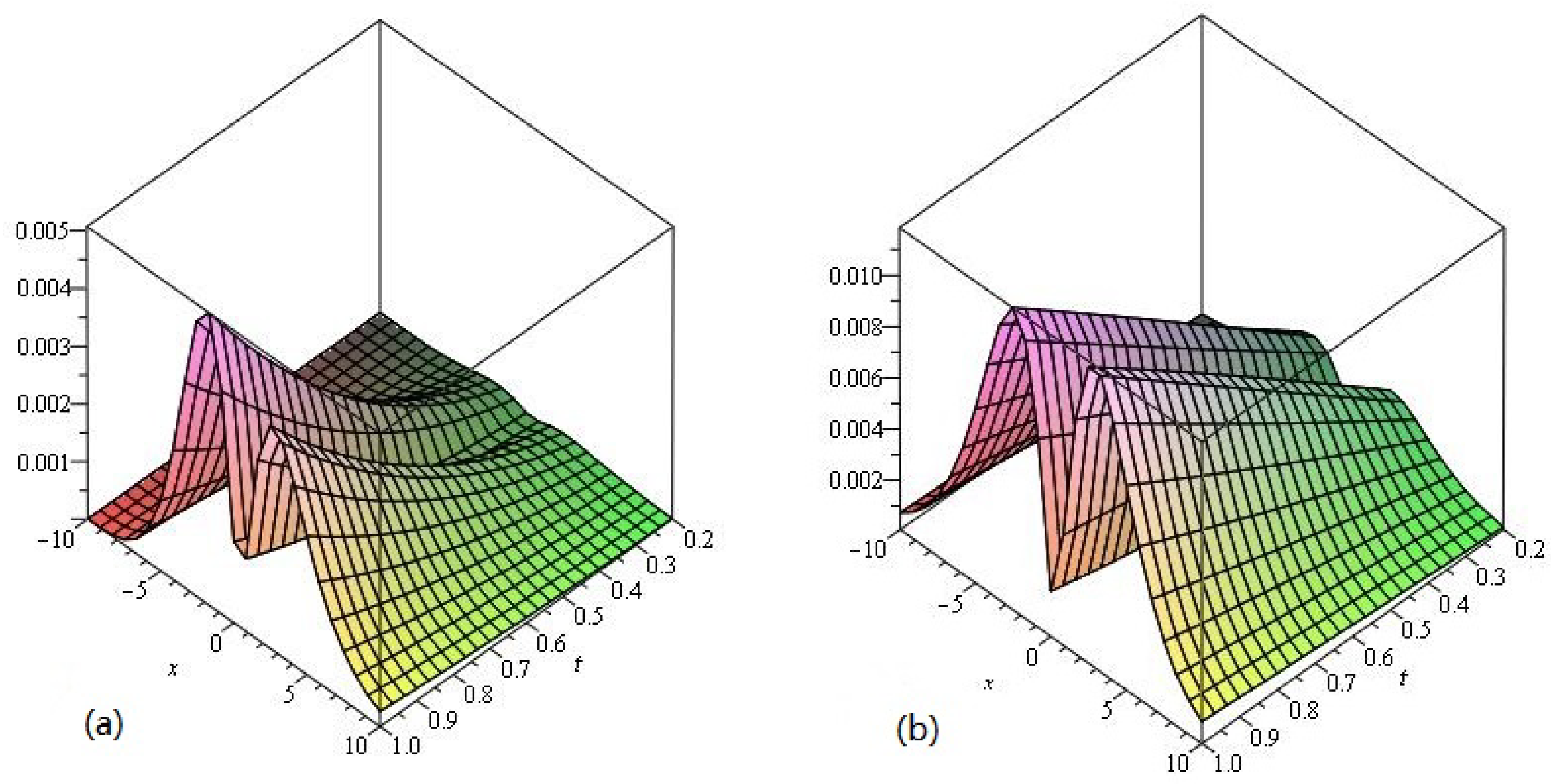
| LADM | Ex() | AE | |||||||
|---|---|---|---|---|---|---|---|---|---|
| −10 | 0.1 | 0.017635 | 0.002788 | 0.017654 | 0.002791 | 0.017661 | 0.002791 | 7.449 | 4.928 |
| 0.3 | 0.017613 | 0.002785 | 0.017637 | 0.002789 | 0.017659 | 0.002787 | 2.238 | 1.478 | |
| 0.5 | 0.017597 | 0.002783 | 0.017620 | 0.002786 | 0.017657 | 0.002784 | 3.727 | 2.464 | |
| 0 | 0.1 | 0.196755 | 0.031107 | 0.196657 | 0.031093 | 0.196617 | 0.031096 | 3.975 | 2.629 |
| 0.3 | 0.196875 | 0.031123 | 0.196748 | 0.031106 | 0.196628 | 0.031114 | 1.192 | 7.889 | |
| 0.5 | 0.196961 | 0.031135 | 0.196839 | 0.031118 | 0.196640 | 0.031131 | 1.987 | 1.314 | |
| 10 | 0.1 | 0.002470 | 0.000390 | 0.002467 | 0.000390 | 0.002466 | 0.000390 | 1.073 | 7.100 |
| 0.3 | 0.002473 | 0.000390 | 0.002470 | 0.000390 | 0.002466 | 0.000390 | 3.221 | 2.131 | |
| 0.5 | 0.002475 | 0.000391 | 0.002472 | 0.000390 | 0.002467 | 0.000391 | 5.368 | 3.552 |
| LADM | Ex() | AE | |||||||
|---|---|---|---|---|---|---|---|---|---|
| −10 | 0.1 | 0.010173 | −0.98953 | 0.010718 | −0.98926 | 0.010718 | −0.98939 | 1.210 | 1.312 |
| 0.3 | 0.009571 | −0.98982 | 0.010200 | −0.98951 | 0.010201 | −0.98990 | 6.351 | 3.848 | |
| 0.5 | 0.009181 | −0.99001 | 0.009707 | −0.98975 | 0.009708 | −0.99038 | 5.301 | 6.274 | |
| 0 | 0.1 | 0.603381 | −0.76294 | 0.616554 | −0.76429 | 0.616566 | −0.76358 | 1.230 | 7.041 |
| 0.3 | 0.586890 | −0.76158 | 0.604564 | −0.76297 | 0.604679 | −0.76095 | 1.141 | 2.015 | |
| 0.5 | 0.574744 | −0.76081 | 0.592339 | −0.76177 | 0.592666 | −0.75858 | 3.267 | 3.188 | |
| 10 | 0.1 | 0.995627 | −0.99577 | 0.995829 | −0.99589 | 0.995827 | −0.99584 | 2.502 | 5.088 |
| 0.3 | 0.995404 | −0.99562 | 0.995636 | −0.99579 | 0.995614 | −0.99563 | 2.271 | 1.566 | |
| 0.5 | 0.995261 | −0.99550 | 0.995454 | −0.99567 | 0.995390 | −0.99541 | 6.364 | 2.680 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shah, R.; Khan, H.; Kumam, P.; Arif, M. An Analytical Technique to Solve the System of Nonlinear Fractional Partial Differential Equations. Mathematics 2019, 7, 505. https://doi.org/10.3390/math7060505
Shah R, Khan H, Kumam P, Arif M. An Analytical Technique to Solve the System of Nonlinear Fractional Partial Differential Equations. Mathematics. 2019; 7(6):505. https://doi.org/10.3390/math7060505
Chicago/Turabian StyleShah, Rasool, Hassan Khan, Poom Kumam, and Muhammad Arif. 2019. "An Analytical Technique to Solve the System of Nonlinear Fractional Partial Differential Equations" Mathematics 7, no. 6: 505. https://doi.org/10.3390/math7060505
APA StyleShah, R., Khan, H., Kumam, P., & Arif, M. (2019). An Analytical Technique to Solve the System of Nonlinear Fractional Partial Differential Equations. Mathematics, 7(6), 505. https://doi.org/10.3390/math7060505







