Signal Amplification by Means of a Dickson Charge Pump: Analysis and Experimental Validation
Abstract
1. Introduction
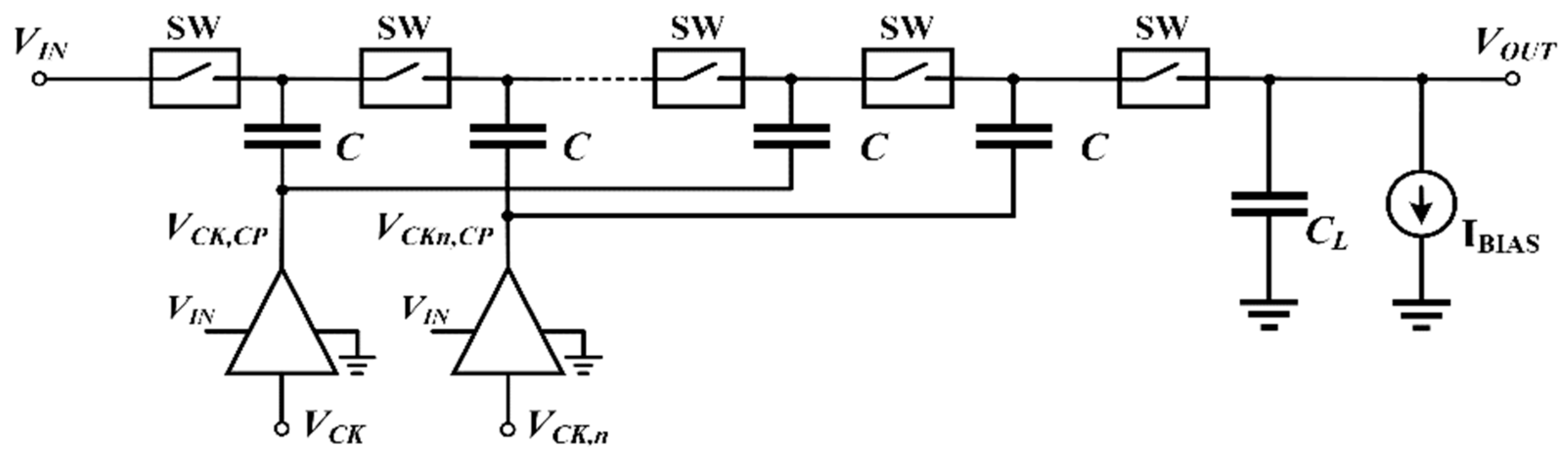
2. The Equivalent Model of the CP
2.1. General Considerations
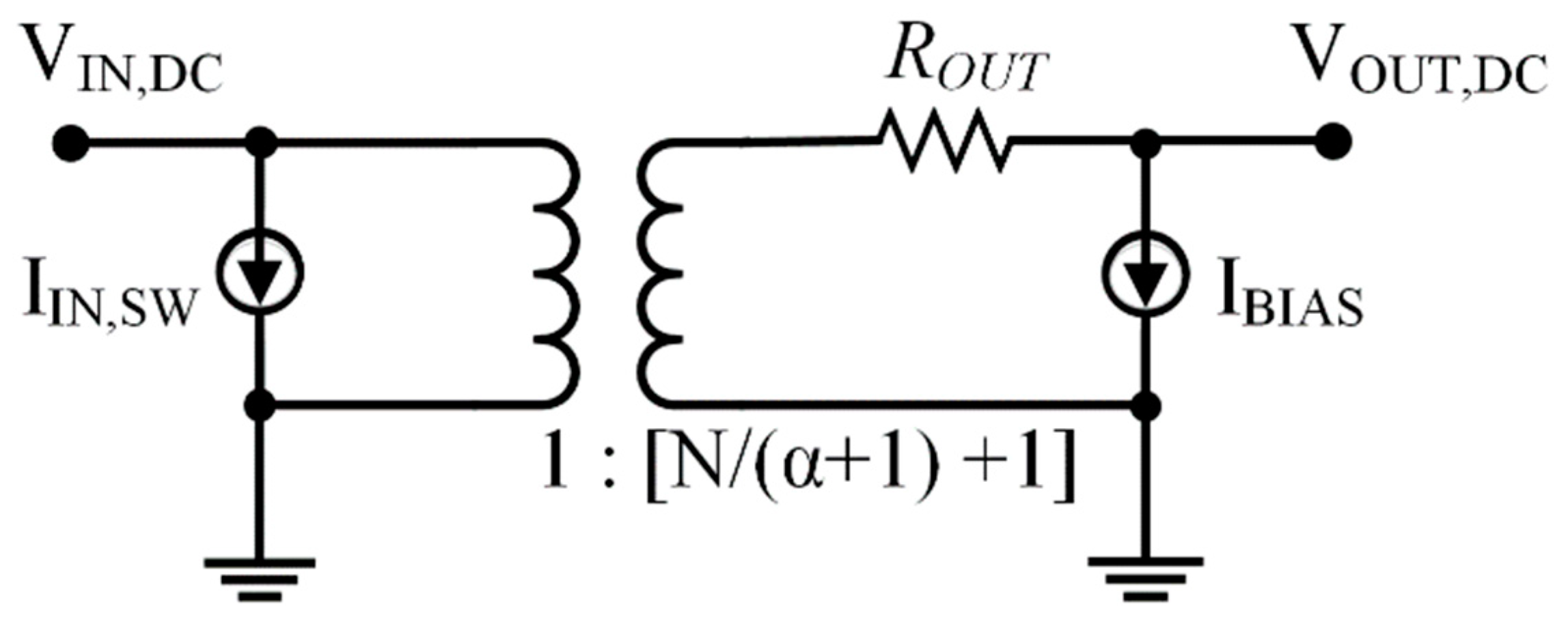
2.2. DC Behavior
2.3. AC Behavior in SSL
2.4. Small- and Large-Signal Analysis
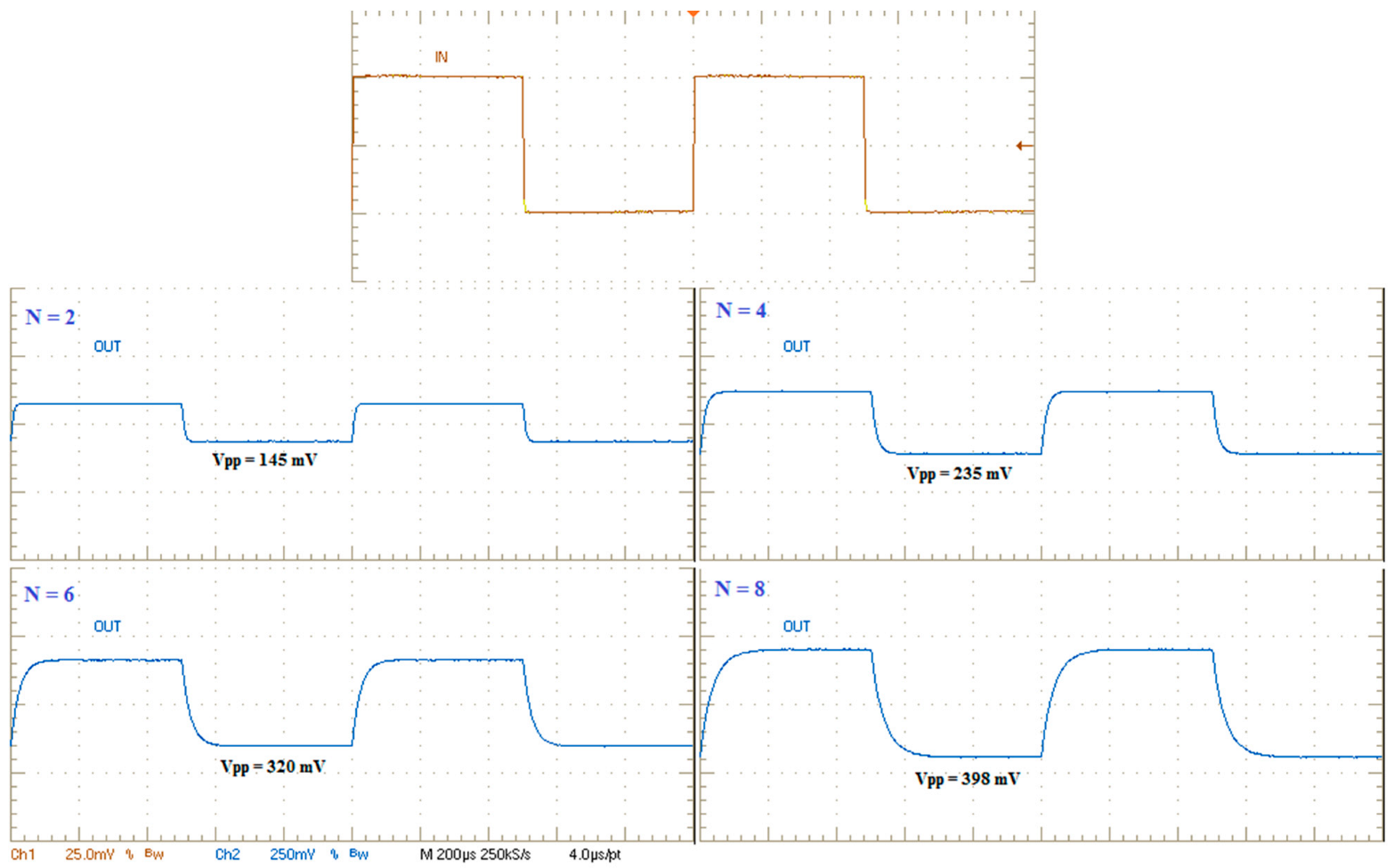
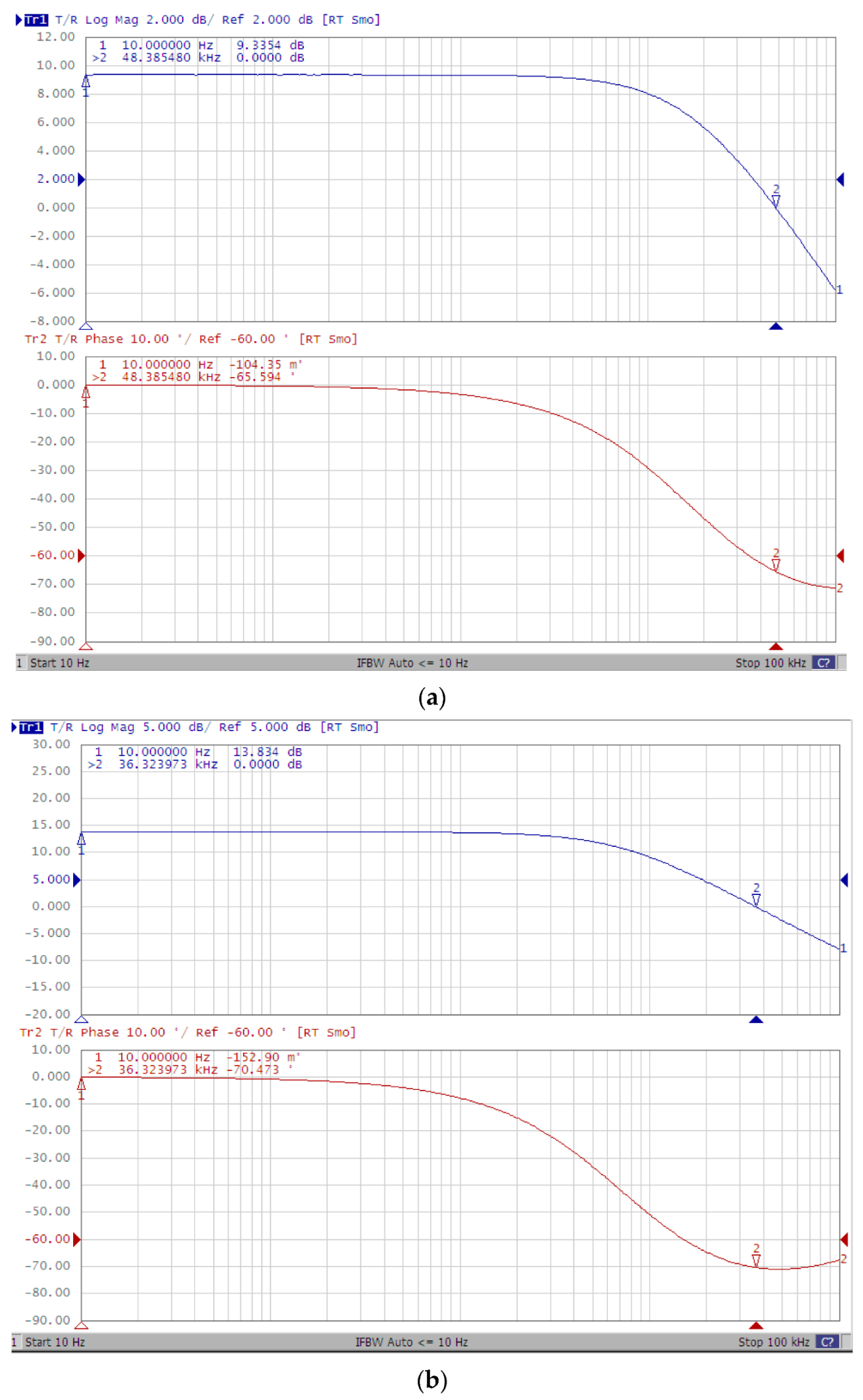
3. Experimental Validation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dickson, J. On-chip high-voltage generation in MNOS integrated circuits using an improved voltage multiplier technique. IEEE J. Solid-State Circuits 1976, 11, 374–378. [Google Scholar] [CrossRef]
- Fan, S.; Dong, J.; Zhang, R.; Xue, Z.; Geng, L. A reconfigurable multi-ratio charge pump with wide input/output voltage range for wireless energy harvesting system. In Proceedings of the 2018 IEEE MTT-S International Wireless Symposium (IWS), Chengdu, China, 6–10 May 2018; pp. 1–3. [Google Scholar] [CrossRef]
- Pulvirenti, F. 3-V Input, 70-V Output, Fully Integrated Hybrid Charge Pump. IEEE Access 2022, 10, 44062–44075. [Google Scholar] [CrossRef]
- Tan, Y.; Zhan, C.; Wang, G. A Fully-On-Chip Analog Low-Dropout Regulator with Negative Charge Pump for Low-Voltage Applications. IEEE Trans. Circuits Syst. II Express Briefs 2019, 66, 1361–1365. [Google Scholar] [CrossRef]
- Seeman, M.D.; Sanders, S.R. Analysis and Optimization of Switched-Capacitor DC–DC Converters. IEEE Trans. Power Electron. 2008, 23, 841–851. [Google Scholar] [CrossRef]
- Ballo, A.; Grasso, A.D.; Palumbo, G. A Review of Charge Pump Topologies for the Power Management of IoT Nodes. Electronics 2019, 8, 480. [Google Scholar] [CrossRef]
- Ueno, F.; Inoue, T.; Oota, I.; Harada, I. Emergency power supply for small computer systems. In Proceedings of the 1991 IEEE International Sympoisum on Circuits and Systems, Singapore, 11–14 June 1991; Volume 2, pp. 1065–1068. [Google Scholar] [CrossRef]
- Brugler, J. Theoretical performance of voltage multiplier circuits. IEEE J. Solid-State Circuits 1971, 6, 132–135. [Google Scholar] [CrossRef]
- Ker, M.-D.; Chen, S.-L.; Tsai, C.-S. Design of Charge Pump Circuit With Consideration of Gate-Oxide Reliability in Low-Voltage CMOS Processes. IEEE J. Solid-State Circuits 2006, 41, 1100–1107. [Google Scholar] [CrossRef][Green Version]
- Ballo, A.; Grasso, A.D.; Palumbo, G.; Tanzawa, T. Charge Pumps for Ultra-Low-Power Applications: Analysis, Design, and New Solutions. IEEE Trans. Circuits Syst. II Express Briefs 2021, 68, 2895–2901. [Google Scholar] [CrossRef]
- Palumbo, G.; Barniol, N.; Bethaoui, M. Improved behavioral and design model of an Nth-order charge pump. IEEE Trans. Circuits Syst. I Regul. Pap. 2000, 47, 264–268. [Google Scholar] [CrossRef]
- Tanzawa, T.; Tanaka, T. A dynamic analysis of the Dickson charge pump circuit. IEEE J. Solid-State Circuits 1997, 32, 1231–1240. [Google Scholar] [CrossRef]
- Aloqlah, A.S.; Alhawari, M. A Resistor-Network Model of Dickson Charge Pump Using Steady-State Analysis. Energies 2022, 15, 1899. [Google Scholar] [CrossRef]
- Palumbo, G.; Pappalardo, D. Charge pump circuits with only capacitive loads: Optimized design. IEEE Trans. Circuits Syst. II Express Briefs 2006, 53, 128–132. [Google Scholar] [CrossRef]
- Ballo, A.; Grasso, A.D.; Palumbo, G.; Tanzawa, T. Linear distribution of capacitance in Dickson charge pumps to reduce rise time. Int. J. Circuit Theory Appl. 2020, 48, 555–566. [Google Scholar] [CrossRef]
- Lin, Y.; Yang, J.; Mui, T.-W.; Zhou, Y.; Leung, K.-N. Optimization of Charge Pump Based on Piecewise Modeling of Output-Voltage Ripple. Energies 2021, 14, 4809. [Google Scholar] [CrossRef]
- Ballo, A.; Grasso, A.D.; Palumbo, G. A simple and effective design strategy to increase power conversion efficiency of linear charge pumps. Int. J. Circuit Theory Appl. 2020, 48, 157–161. [Google Scholar] [CrossRef]
- Ballo, A.; Grasso, A.D.; Palumbo, G. A Memory-Targeted Dynamic Reconfigurable Charge Pump to Achieve a Power Consumption Reduction in IoT Nodes. IEEE Access 2021, 9, 41958–41964. [Google Scholar] [CrossRef]
- Koketsu, K.; Tanzawa, T. Design of a Charge Pump Circuit and System with Input Impedance Modulation for a Flexible-Type Thermoelectric Generator with High-Output Impedance. Electronics 2021, 10, 1212. [Google Scholar] [CrossRef]
- Ballo, A.; Grasso, A.D.; Palumbo, G. A Subthreshold Cross-Coupled Hybrid Charge Pump for 50-mV Cold-Start. IEEE Access 2020, 8, 188959–188969. [Google Scholar] [CrossRef]
- Yong, J.K.; Ramiah, H.; Churchill, K.K.P.; Chong, G.; Mekhilef, S.; Chen, Y.; Mak, P.-I.; Martins, R.P. A 0.1-V VIN Subthreshold 3-Stage Dual-Branch Charge Pump with 43.4% Peak Power Conversion Efficiency Using Advanced Dynamic Gate-Bias. IEEE Trans. Circuits Syst. II Express Briefs 2022, 1. [Google Scholar] [CrossRef]
- Potocny, M.; Kovac, M.; Arbet, D.; Sovcik, M.; Nagy, L.; Stopjakova, V.; Ravasz, R. Low-Voltage DC-DC Converter for IoT and On-Chip Energy Harvester Applications. Sensors 2021, 21, 5721. [Google Scholar] [CrossRef]
- Zhu, X.; Fu, Q.; Yang, R.; Zhang, Y. A High Power-Conversion-Efficiency Voltage Boost Converter with MPPT for Wireless Sensor Nodes. Sensors 2021, 21, 5447. [Google Scholar] [CrossRef] [PubMed]
- Esmailiyan, A.; Schembari, F.; Staszewski, R.B. A 0.36-V 5-MS/s Time-Mode Flash ADC With Dickson-Charge-Pump-Based Comparators in 28-nm CMOS. IEEE Trans. Circuits Syst. I Regul. Pap. 2020, 67, 1789–1802. [Google Scholar] [CrossRef]
- Esmailiyan, A.; Du, J.; Siriburanon, T.; Schembari, F.; Staszewski, R.B. Dickson-Charge-Pump-Based Voltage-to-Time Conversion for Time-Based ADCs in 28-nm CMOS. IEEE Open J. Circuits Syst. 2021, 2, 23–31. [Google Scholar] [CrossRef]
- Gokdag, M.; Akbaba, M.; Gulbudak, O. Switched-capacitor converter for PV modules under partial shading and mismatch conditions. Sol. Energy 2018, 170, 723–731. [Google Scholar] [CrossRef]
- Wang, Y.; Yan, N.; Min, H.; Shi, C.-J.R. A High-Efficiency Split–Merge Charge Pump for Solar Energy Harvesting. IEEE Trans. Circuits Syst. II Express Briefs 2017, 64, 545–549. [Google Scholar] [CrossRef]
- Woo, K.-C.; Yang, B.-D. A 0.35V 90nA Quiescent Current Output-Capacitor-Less NMOS Low-Dropout Regulator Using a Coarse-Fine Charge-Pump Circuit. IEEE Trans. Circuits Syst. II Express Briefs 2020, 67, 3118–3122. [Google Scholar] [CrossRef]
- Ballo, A.; Grasso, A.D.; Palumbo, G. The Dickson Charge Pump as a Signal Amplifier. IEEE Trans. Circuits Syst. Regul. Pap. 2022, 1–14. [Google Scholar] [CrossRef]
- Nakagome, Y.; Tanaka, H.; Takeuchi, K.; Kume, E.; Watanabe, Y.; Kaga, T.; Kawamoto, Y.; Murai, F.; Izawa, R.; Hisamoto, D.; et al. An experimental 1.5-V 64-Mb DRAM. IEEE J. Solid-State Circuits 1991, 26, 465–472. [Google Scholar] [CrossRef]
- Gariboldi, R.; Pulvirenti, F. A monolithic quad line driver for industrial applications. IEEE J. Solid-State Circuits 1994, 29, 957–962. [Google Scholar] [CrossRef]
- Gariboldi, R.; Pulvirenti, F. A 70 mΩ Intelligent High Side Switch with Full Diagnostics. In Proceedings of the ESSCIRC ’95: Twenty-first European Solid-State Circuits Conference, Lille, France, 19–21 September 1995; pp. 262–265. [Google Scholar]



| Parameter | 2-Stage CP | 4-Stage CP | 6-Stage CP | 8-Stage CP | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| μ/σ | Model | εP2M% | μ/σ | Model | εP2M% | μ/σ | Model | εP2M% | μ/σ | Model | εP2M% | |
| UGF (kHz) | 41.3/1.3 | 43.5 | −5.3 | 33.6/1.2 | 34 | −1.1 | 30.3/0.9 | 29.9 | 1.5 | 28.8/0.5 | 27.2 | 5.6 |
| DC gain (dB) | 9.28/0.07 | 9.32 | −0.4 | 13.71/0.13 | 13.71 | 0.02 | 16.63/0.14 | 16.61 | 0.1 | 18.81/0.14 | 18.78 | 0.2 |
| Settling time a (ms) | 0.34/0.02 | 0.31 | 9.1 | 0.68/0.03 | 0.66 | 3.5 | 1.14/0.06 | 1.04 | 8.5 | 1.56/0.08 | 1.47 | 5.8 |
| SR a (V/ms) | 15.75/0.19 | 13.67 | 13.2 | 11.26/0.15 | 10.67 | 5.2 | 10.35/0.08 | 9.38 | 9.4 | 9.12/0.11 | 8.55 | 6.3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ballo, A.; Grasso, A.D.; Palumbo, G. Signal Amplification by Means of a Dickson Charge Pump: Analysis and Experimental Validation. Chips 2022, 1, 72-82. https://doi.org/10.3390/chips1020007
Ballo A, Grasso AD, Palumbo G. Signal Amplification by Means of a Dickson Charge Pump: Analysis and Experimental Validation. Chips. 2022; 1(2):72-82. https://doi.org/10.3390/chips1020007
Chicago/Turabian StyleBallo, Andrea, Alfio Dario Grasso, and Gaetano Palumbo. 2022. "Signal Amplification by Means of a Dickson Charge Pump: Analysis and Experimental Validation" Chips 1, no. 2: 72-82. https://doi.org/10.3390/chips1020007
APA StyleBallo, A., Grasso, A. D., & Palumbo, G. (2022). Signal Amplification by Means of a Dickson Charge Pump: Analysis and Experimental Validation. Chips, 1(2), 72-82. https://doi.org/10.3390/chips1020007








