Experimental and Simulative Investigation of Deterministic Lateral Displacement and Dielectrophoresis Methods for Continuous Multi-Property Particle Sorting
Abstract
1. Introduction
1.1. Deterministic Lateral Displacement
1.2. Dielectrophoresis
1.3. Combined Systems
2. Materials and Methods
2.1. Deterministic Lateral Displacement
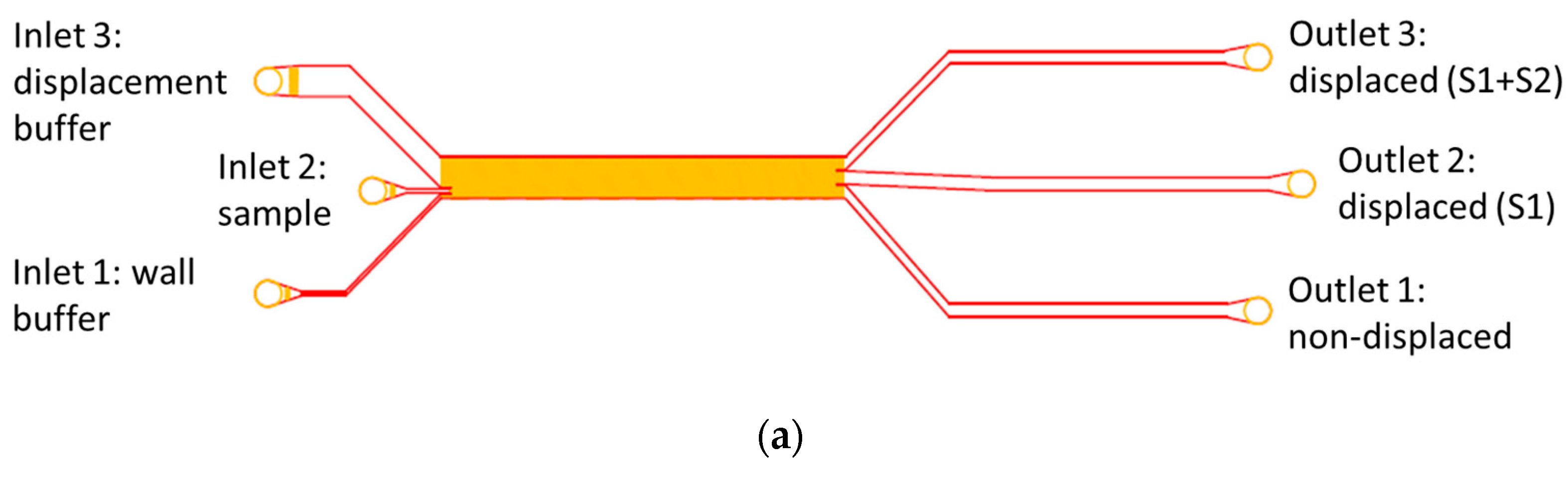
2.1.1. Designs of DLD Arrays
2.1.2. Fabrication of the DLD Arrays
2.1.3. Experimental Setup for DLD Experiments
Variation of the Particle Shape and Density
Fractionation of Polydisperse Particle Suspensions
2.2. Dielectrophoresis
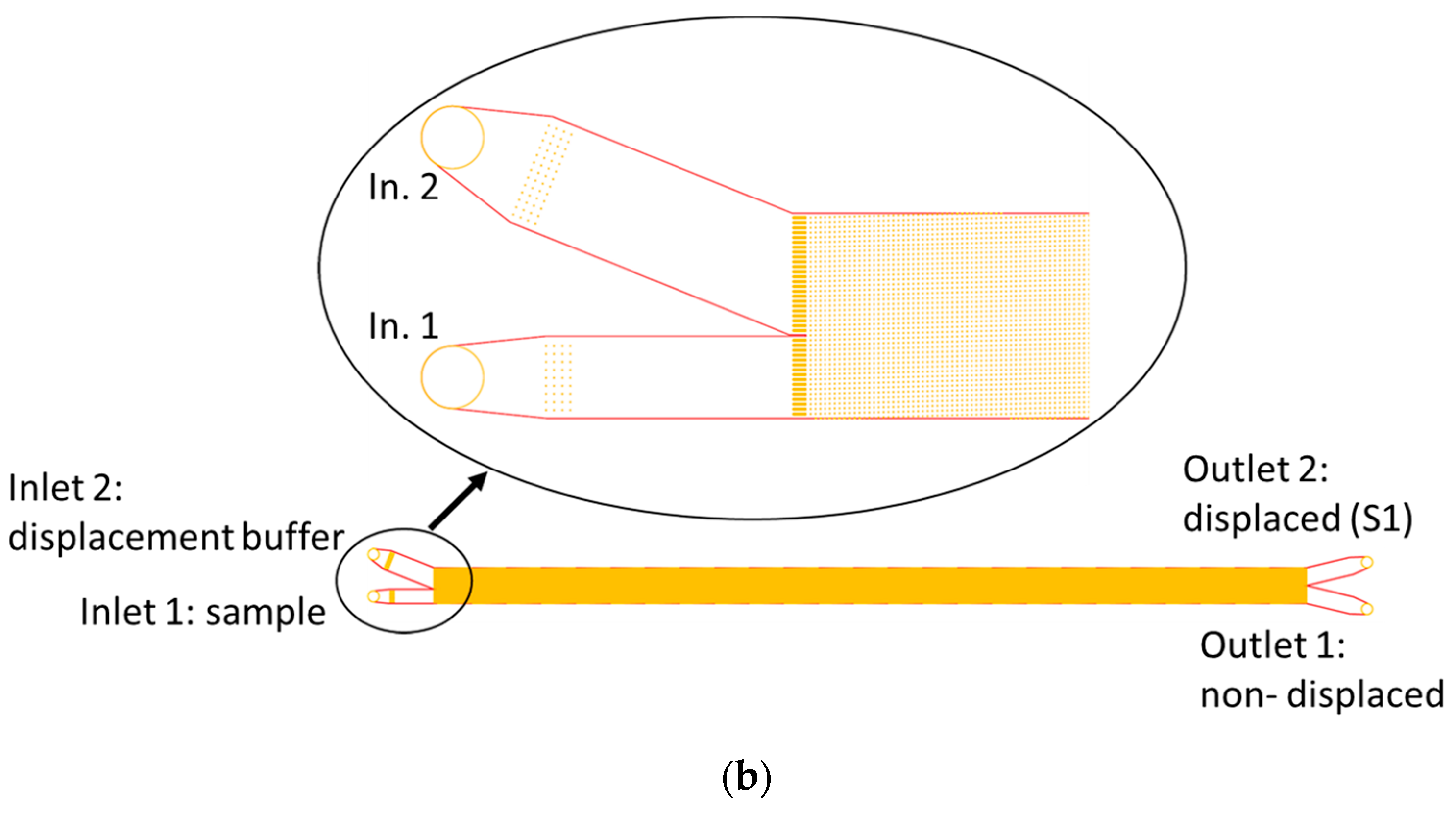
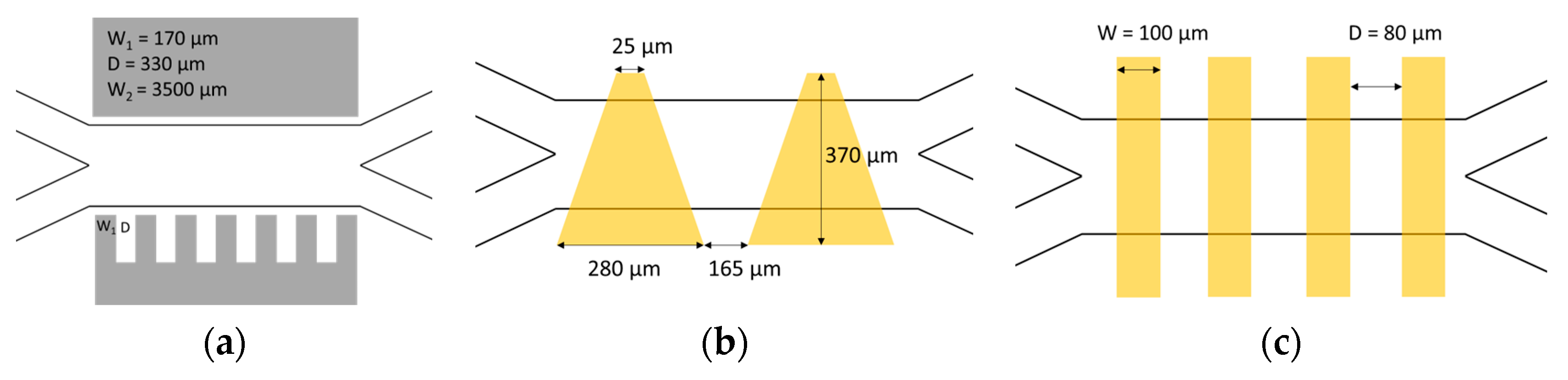
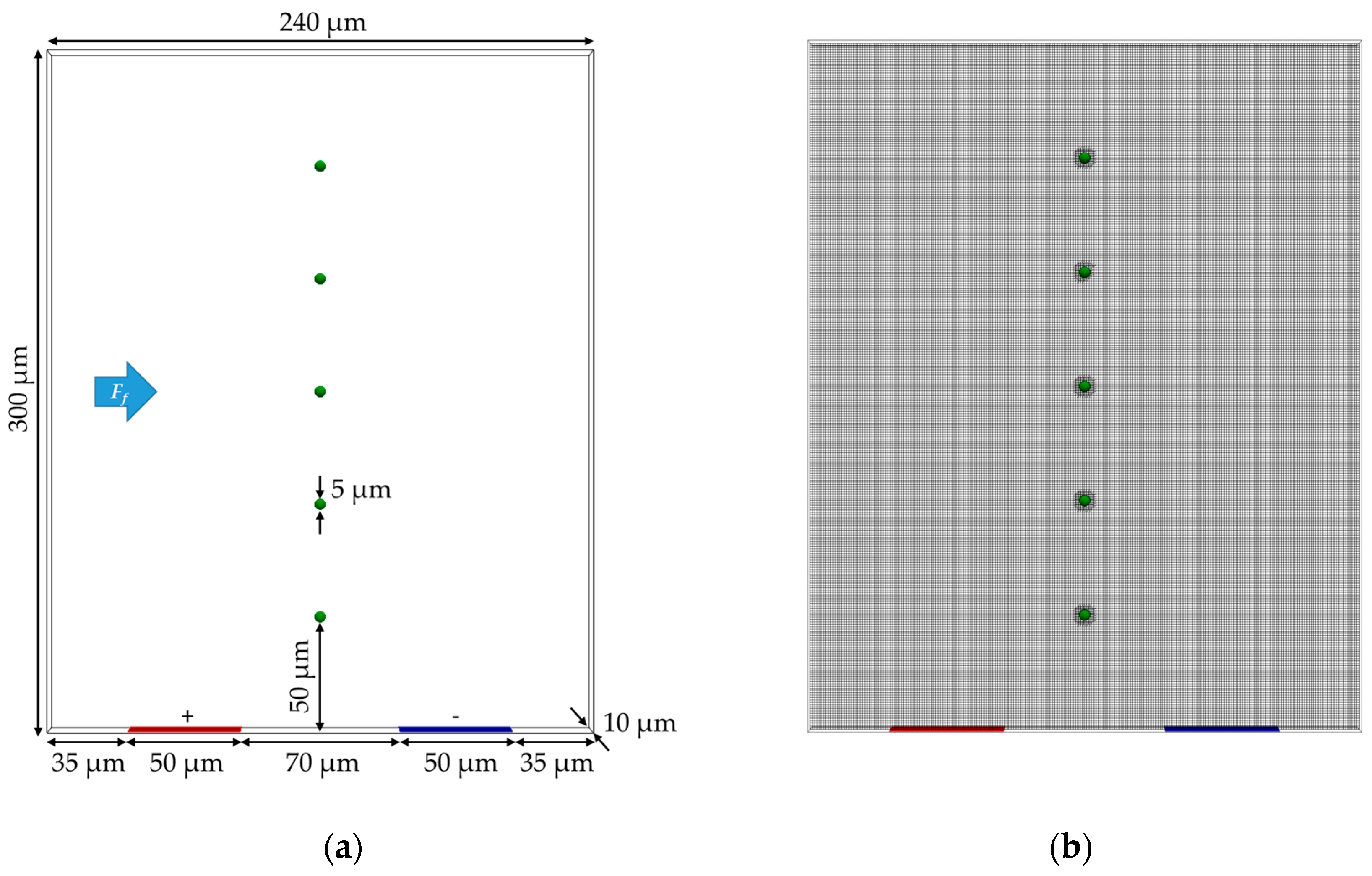
2.2.1. Designs of DEP Systems
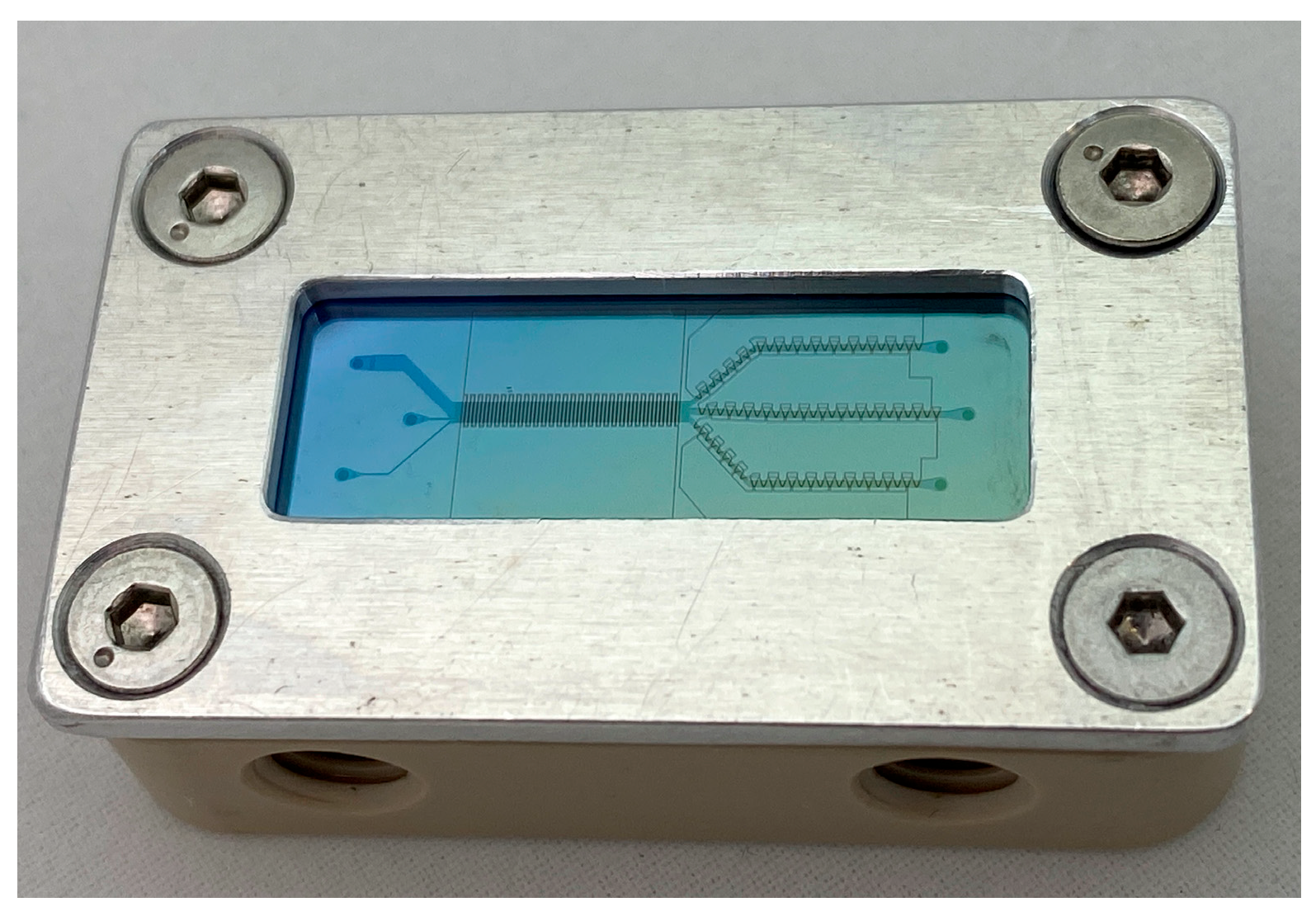
2.2.2. Fabrication of the DEP Systems
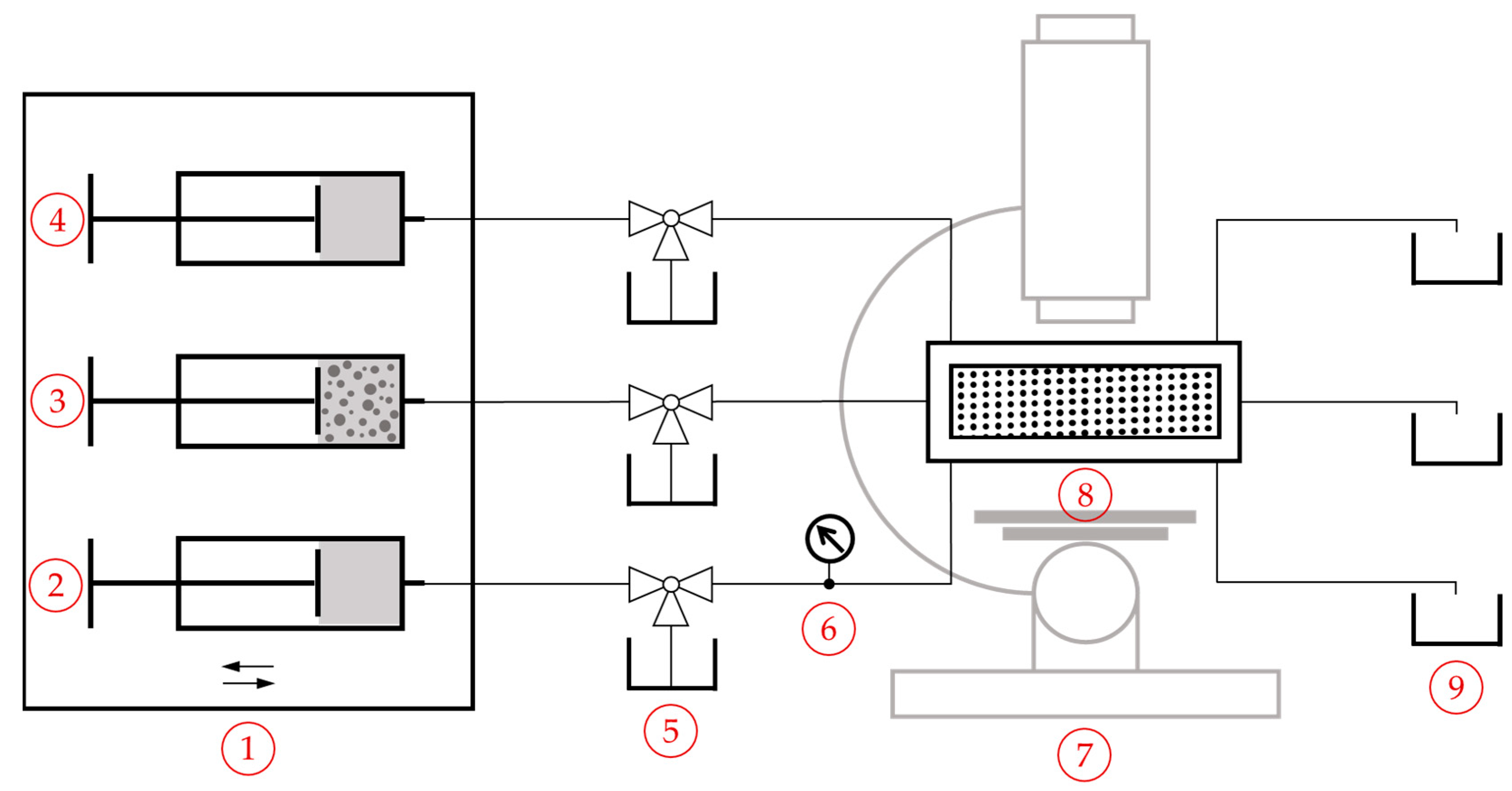
2.2.3. Experimental Setup
2.2.4. Description of the Numerical CFD-DEM Model
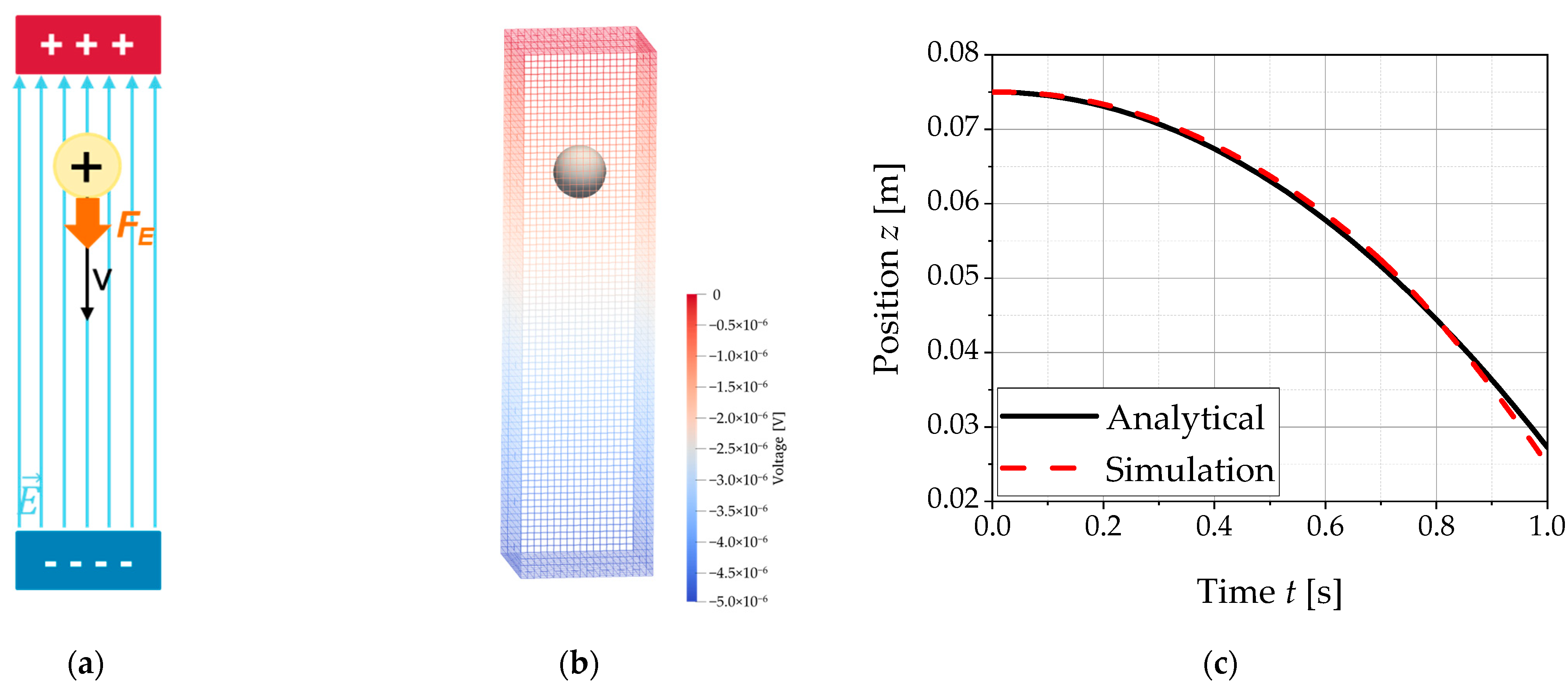
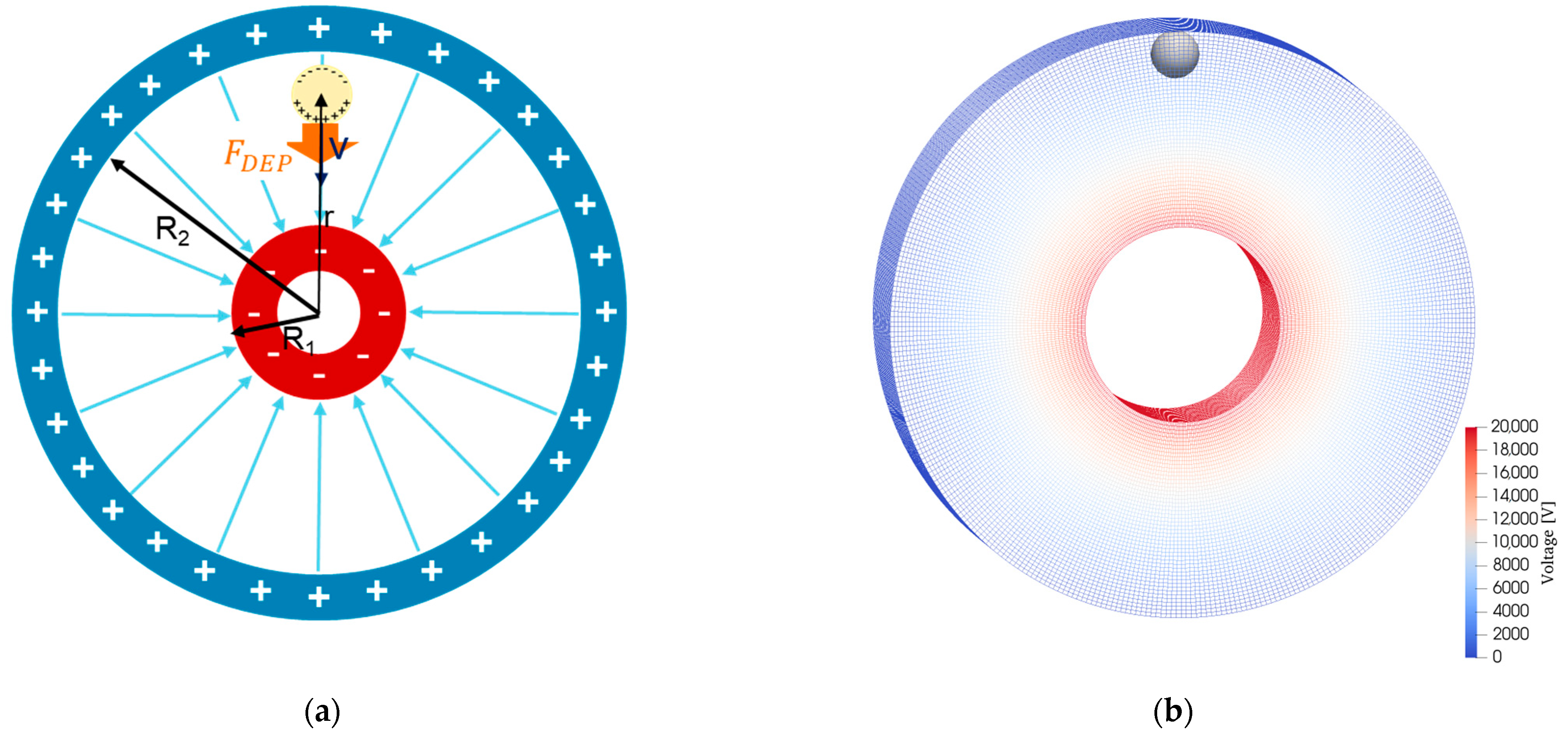
2.2.5. Validation of the Numerical CFD-DEM Model
Electrical Force on a Charged Particle
Dielectrophoretic Force on an Uncharged Particle
2.2.6. Model Description of the (combined) DEP Fractionation
3. Results and Discussion
3.1. Deterministic Lateral Displacement
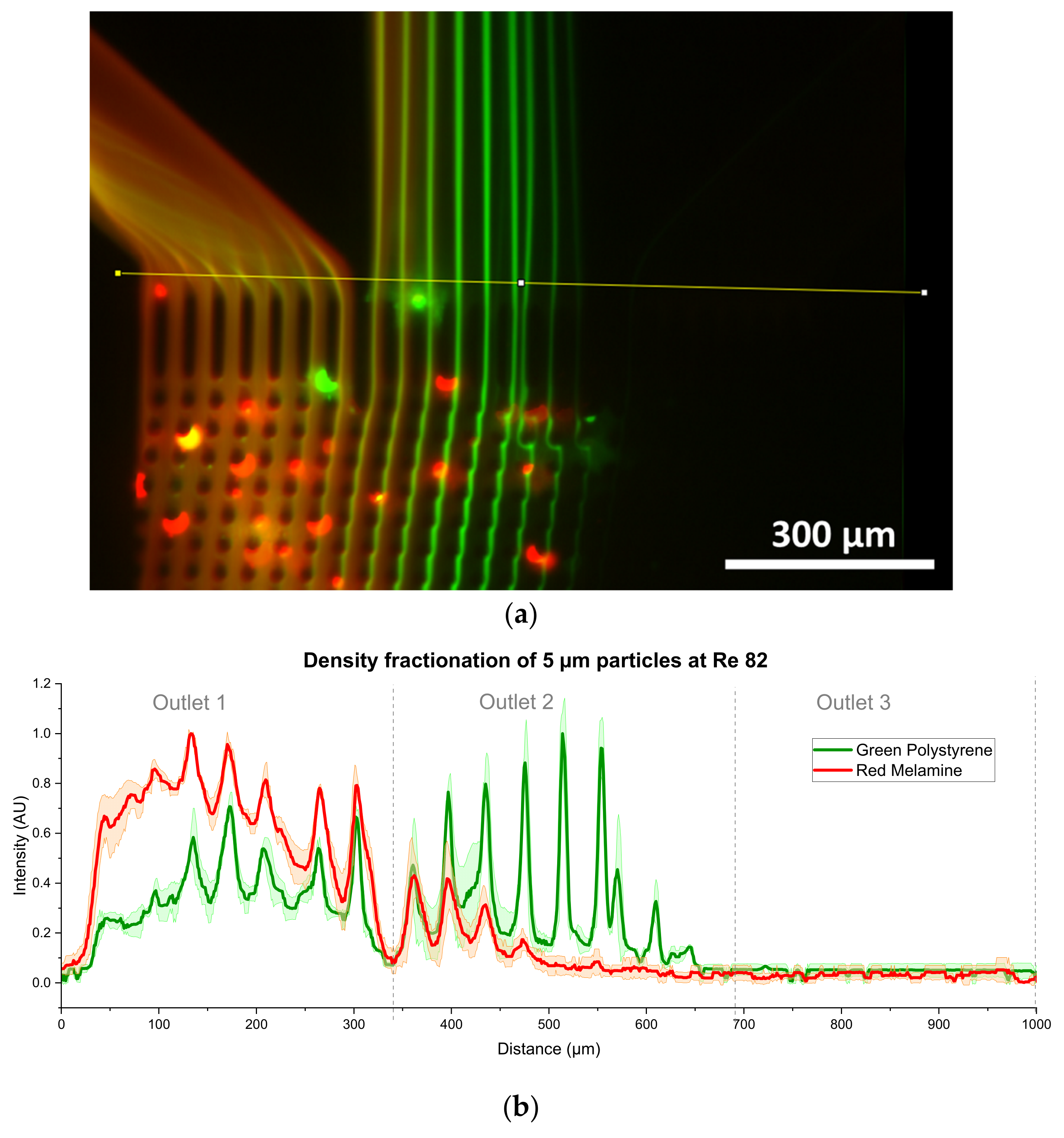
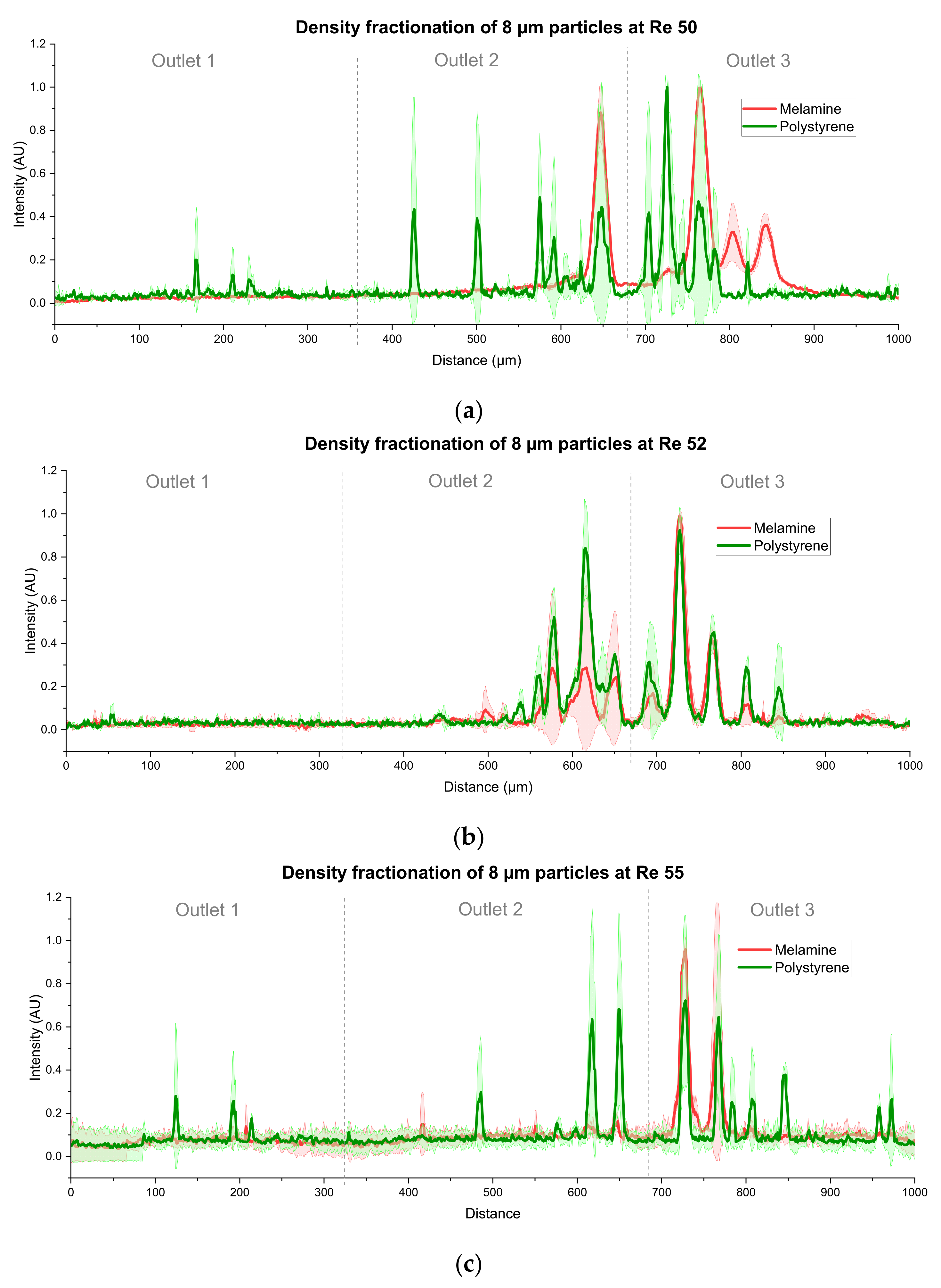
3.1.1. Particle Density Variation
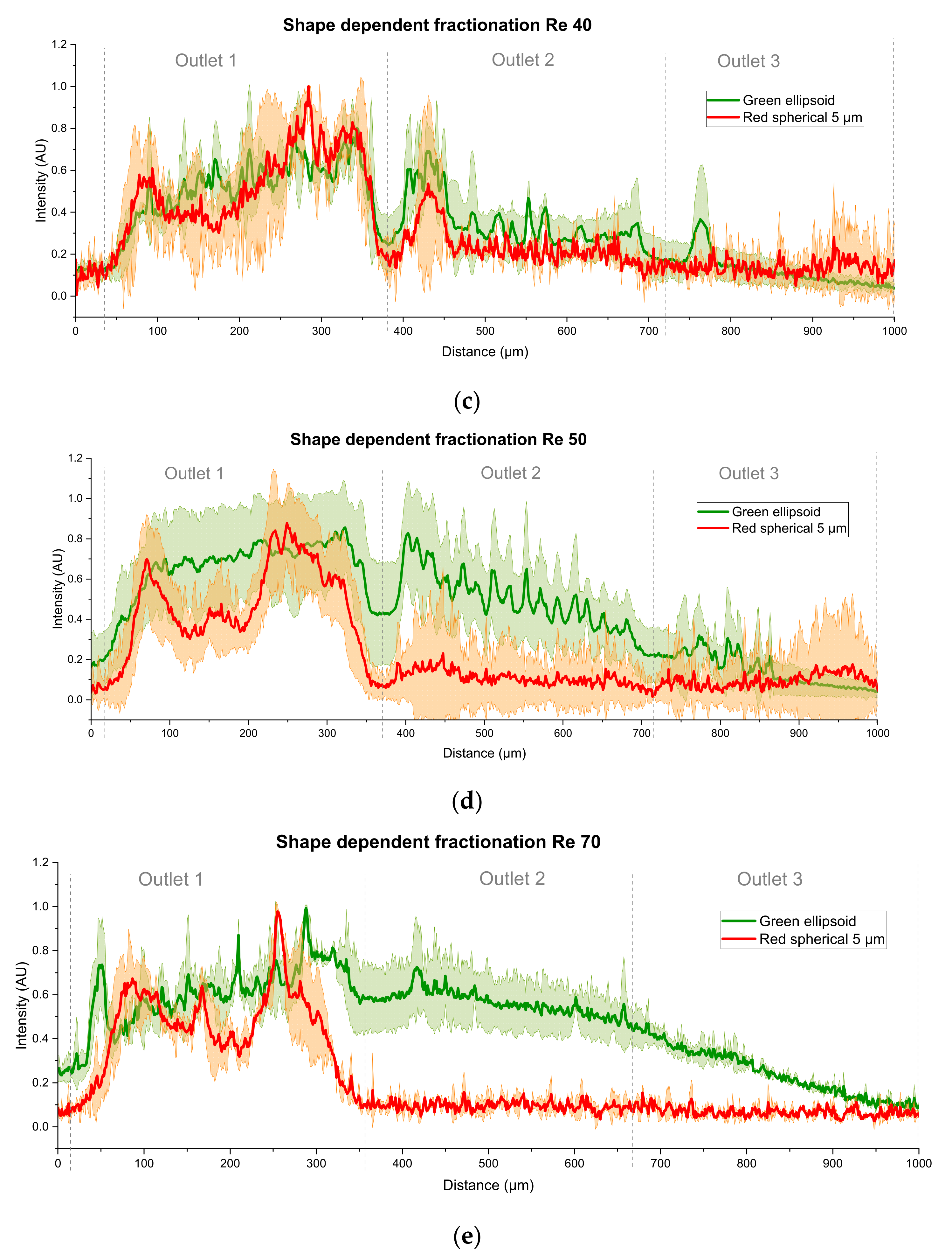
3.1.2. Variation of Particle Shape
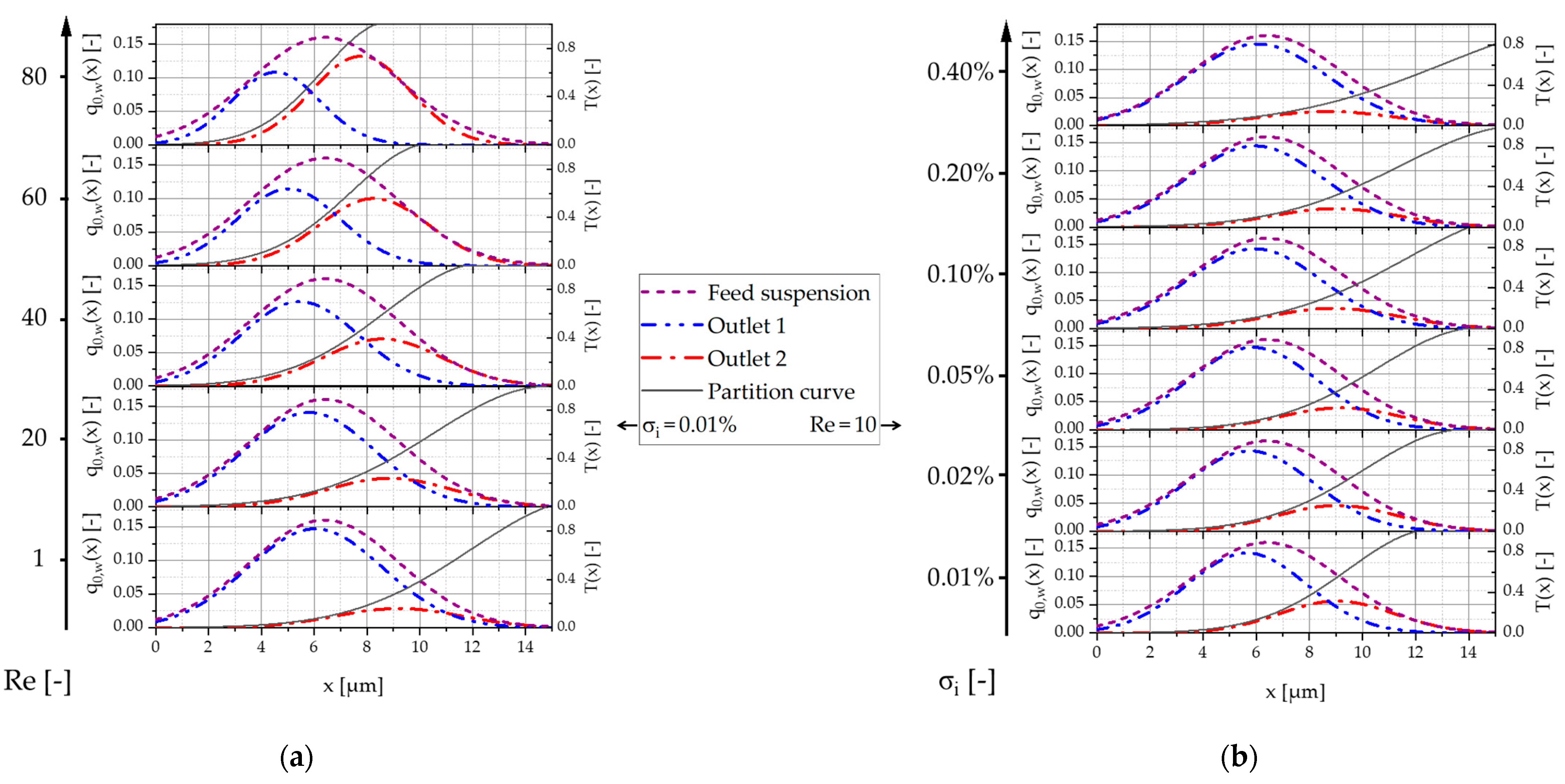
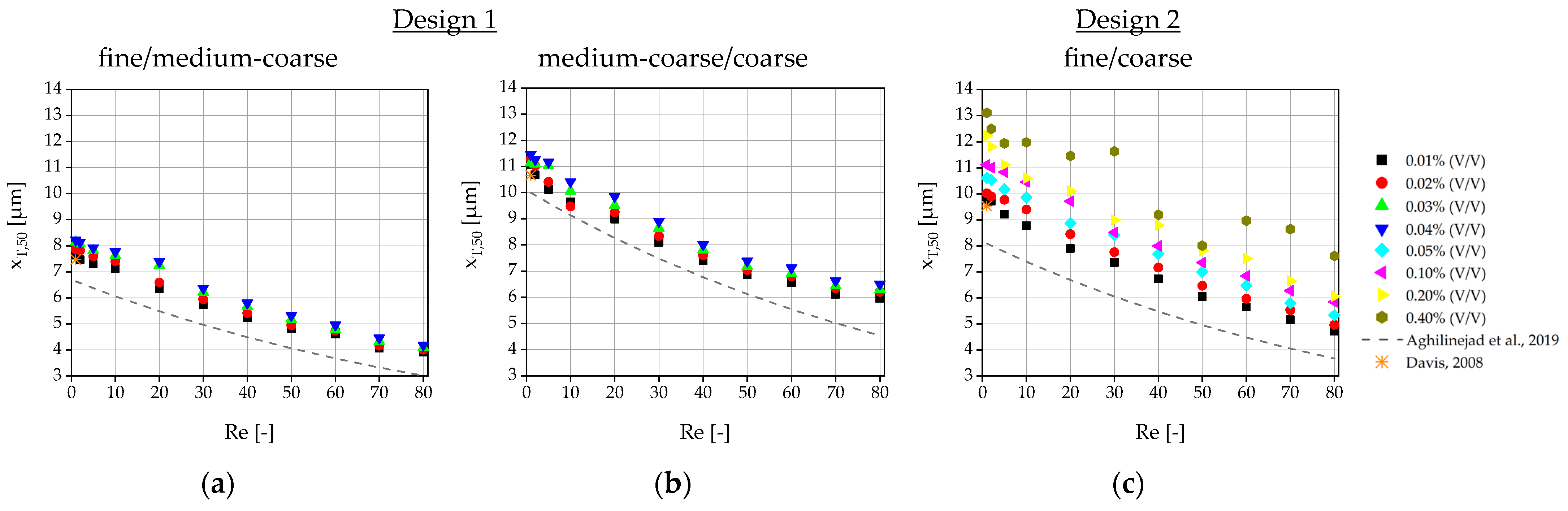
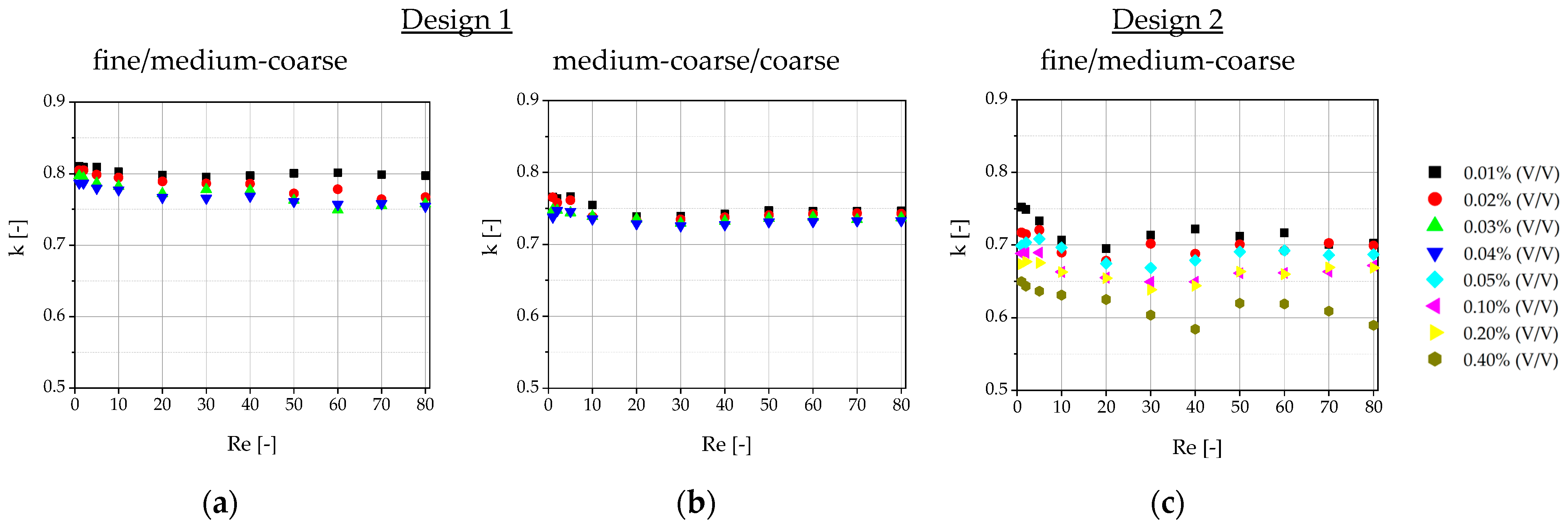
3.1.3. Fractionation of Polydisperse Particle Suspensions
3.2. Dielectrophoresis
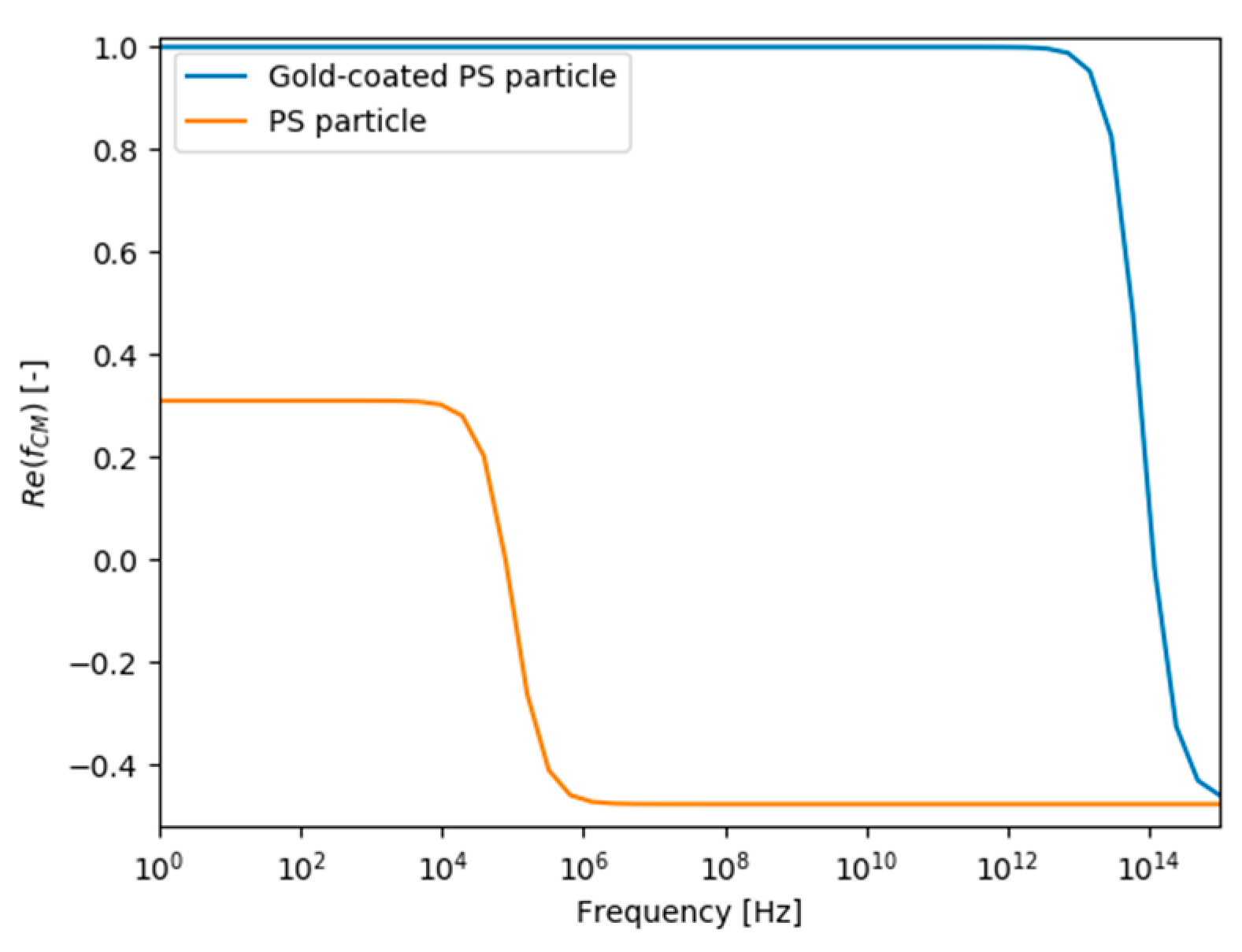
3.2.1. Simulations
3.2.2. Experimental Results Dielectrophoresis and Combined Systems
4. Conclusions
- In the DLD, a density-dependent fractionation was experimentally visible for melamine and polystyrene particles of 5 µm at Re = 82, which appeared to be very sensitive to this certain flow conditions.
- Elliptical particles of size 5 × 10 µm could be experimentally separated from spherical melamine particles in the DLD.
- Polydisperse silica particles were separated in two different DLD designs under the influence of different Reynolds numbers and particle concentrations.
- In general, the separation size decreased with increasing Reynolds number, but increased with increasing particle concentration due to the intensifying particle interactions. The separation efficiency decreased with increasing particle concentration, but showed no clear dependence on the Reynolds number.
- Design 1 (three inlets including sheath flow) showed higher separation efficiencies, but allowed lower concentrations than design 2 (two inlets; significantly wider particle inlet; flatter tilt angle with significantly enlarged gap).
- The IB-CFD-DEM software has been extended to include the calculation of electric fields in the FV cells and, based on this, the calculation of electric and dielectric forces on spherical particles. The implemented force models were validated by comparing simple test cases with the analytical solutions.
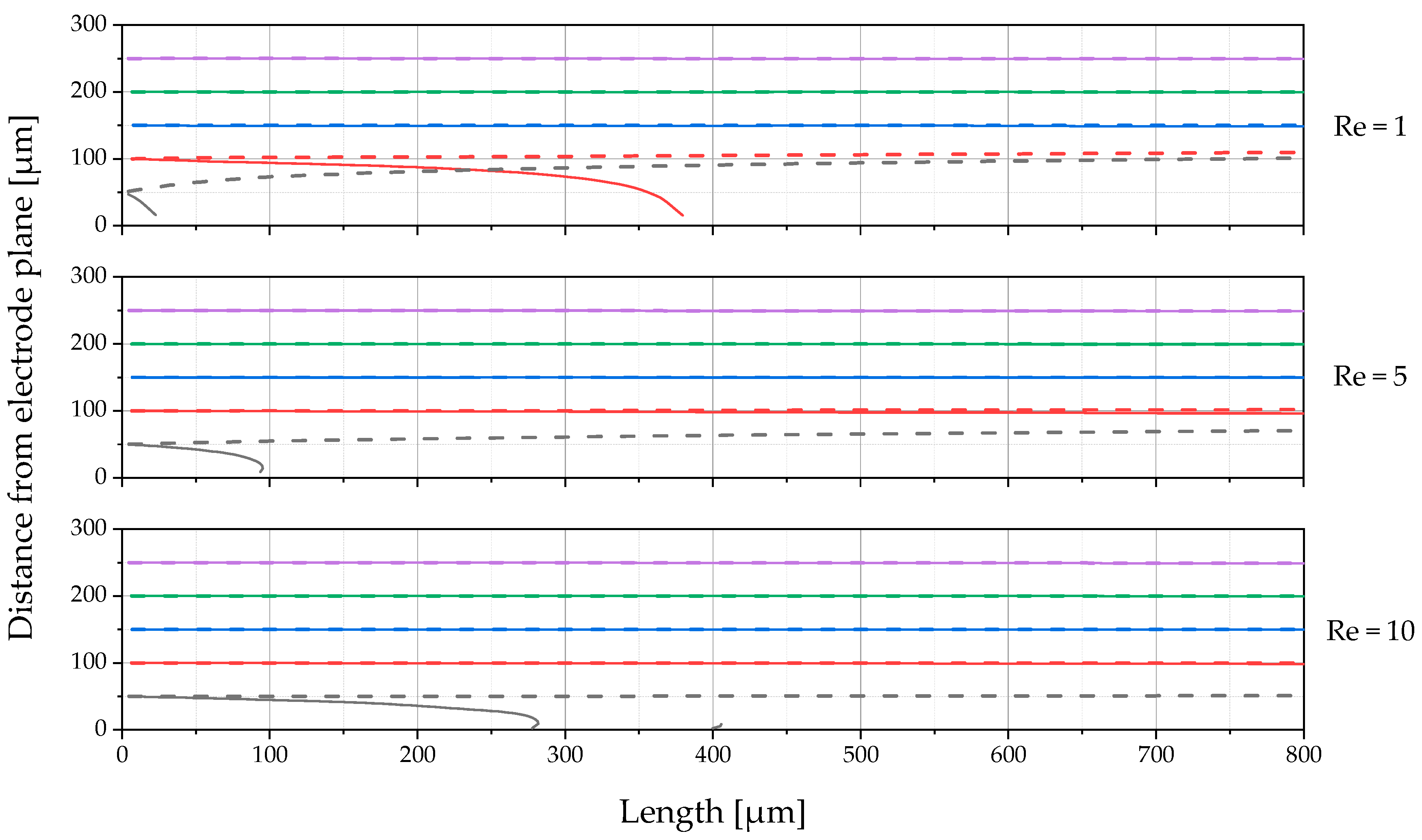
- The DEP section of the serial-combined DLD-DEP design was simulated using this software at different Reynolds numbers for the separation of 5 µm polystyrene particles from gold-coated polystyrene particles. A good deflection of the core-shell particles was observed in the half of the channel near the electrodes, which decreased with increasing Reynolds number. The half of the channel averted from the electrodes showed negligible deflection, which is why the inclusion of an additional particle-focusing section between the DLD and DEP sections is recommended to concentrate the particles to the electrodes.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shekunov, B.Y.; Chattopadhyay, P.; Tong, H.H.Y.; Chow, A.H.L. Particle size analysis in pharmaceutics: Principles, methods and applications. Pharm. Res. 2007, 24, 203–227. [Google Scholar] [CrossRef] [PubMed]
- Salafi, T.; Zhang, Y.; Zhang, Y. A Review on Deterministic Lateral Displacement for Particle Separation and Detection. Nano-Micro Lett. 2019, 11, 77. [Google Scholar] [CrossRef] [PubMed]
- Aghilinejad, A.; Aghaamoo, M.; Chen, X. On the transport of particles/cells in high-throughput deterministic lateral displacement devices: Implications for circulating tumor cell separation. Biomicrofluidics 2019, 13, 34112. [Google Scholar] [CrossRef] [PubMed]
- Vernekar, R.; Krüger, T. Breakdown of deterministic lateral displacement efficiency for non-dilute suspensions: A numerical study. Med. Eng. Phys. 2015, 37, 845–854. [Google Scholar] [CrossRef]
- Dincau, B.M.; Aghilinejad, A.; Hammersley, T.; Chen, X.; Kim, J.-H. Deterministic lateral displacement (DLD) in the high Reynolds number regime: High-throughput and dynamic separation characteristics. Microfluid. Nanofluid. 2018, 22, 59. [Google Scholar] [CrossRef]
- Kottmeier, J.; Wullenweber, M.S.; Kampen, I.; Kwade, A.; Dietzel, A. A High-Aspect-Ratio Deterministic Lateral Displacement Array for High-Throughput Fractionation. Micromachines 2024, 15, 802. [Google Scholar] [CrossRef]
- Mallorie, C.; Vernekar, R.; Owen, B.; Inglis, D.W.; Krüger, T. Numerical analysis of flow anisotropy in rotated-square deterministic lateral displacement devices at moderate Reynolds number. Phys. Rev. Fluids 2024, 9, 024203. [Google Scholar] [CrossRef]
- Reinecke, S.R.; Blahout, S.; Rosemann, T.; Kravets, B.; Wullenweber, M.; Kwade, A.; Hussong, J.; Kruggel-Emden, H. DEM-LBM simulation of multidimensional fractionation by size and density through deterministic lateral displacement at various Reynolds numbers. Powder Technol. 2021, 385, 418–433. [Google Scholar] [CrossRef]
- Bowman, T.J.; Drazer, G.; Frechette, J. Inertia and scaling in deterministic lateral displacement. Biomicrofluidics 2013, 7, 64111. [Google Scholar] [CrossRef]
- Holm, S.H.; Beech, J.P.; Tegenfeldt, J.O. Combined density and size-based sorting in deterministic lateral displacement devices. In Proceedings of the 17th International Conference on Miniaturized Systems for Chemistry and Life Sciences (MicroTAS 2013), Freiburg, Germany, 27–31 October 2013; Zengerle, R., Ed.; Curran: Red Hook, NY, USA, 2014; pp. 1224–1226, ISBN 9781632666246. [Google Scholar]
- Holm, S.H.; Beech, J.P.; Barrett, M.P.; Tegenfeldt, J.O. Simplifying microfluidic separation devices towards field-detection of blood parasites. Anal. Methods 2016, 8, 3291–3300. [Google Scholar] [CrossRef]
- Beech, J.P.; Ho, B.D.; Garriss, G.; Oliveira, V.; Henriques-Normark, B.; Tegenfeldt, J.O. Separation of pathogenic bacteria by chain length. Anal. Chim. Acta 2018, 1000, 223–231. [Google Scholar] [CrossRef] [PubMed]
- Di Carlo, D. Inertial microfluidics. Lab. Chip 2009, 9, 3038–3046. [Google Scholar] [CrossRef] [PubMed]
- Wullenweber, M.S.; Kottmeier, J.; Kampen, I.; Dietzel, A.; Kwade, A. Numerical Study on High Throughput and High Solid Particle Separation in Deterministic Lateral Displacement Microarrays. Processes 2023, 11, 2438. [Google Scholar] [CrossRef]
- Lubbersen, Y.S.; Schutyser, M.; Boom, R.M. Suspension separation with deterministic ratchets at moderate Reynolds numbers. Chem. Eng. Sci. 2012, 73, 314–320. [Google Scholar] [CrossRef]
- Pethig, R. Dielectrophoresis: Theory, Methodology and Biological Applications, 1st ed.; Wiley: Hoboken, NJ, USA; Chichester, UK, 2017; ISBN 9781118671450. [Google Scholar]
- Giesler, J.; Weirauch, L.; Thöming, J.; Baune, M.; Pesch, G.R. Separating microparticles by material and size using dielectrophoretic chromatography with frequency modulation. Sci. Rep. 2021, 11, 16861. [Google Scholar] [CrossRef]
- Douglas, T.A.; Alinezhadbalalami, N.; Balani, N.; Schmelz, E.M.; Davalos, R.V. Separation of Macrophages and Fibroblasts Using Contactless Dielectrophoresis and a Novel ImageJ Macro. Bioelectricity 2019, 1, 49–55. [Google Scholar] [CrossRef]
- Al-Ali, A.; Waheed, W.; Abu-Nada, E.; Alazzam, A. A review of active and passive hybrid systems based on Dielectrophoresis for the manipulation of microparticles. J. Chromatogr. A 2022, 1676, 463268. [Google Scholar] [CrossRef]
- Yan, S.; Zhang, J.; Yuan, D.; Li, W. Hybrid microfluidics combined with active and passive approaches for continuous cell separation. Electrophoresis 2017, 38, 238–249. [Google Scholar] [CrossRef]
- Cha, H.; Fallahi, H.; Dai, Y.; Yuan, D.; An, H.; Nguyen, N.-T.; Zhang, J. Multiphysics microfluidics for cell manipulation and separation: A review. Lab. Chip 2022, 22, 423–444. [Google Scholar] [CrossRef]
- Peng, T.; Zhang, Z.; Yuan, S.; Qiang, J.; Jia, Y. Investigation on sheathless inertial focusing within low-aspect ratio spiral microchannel for cascaded microfluidic tumor cell separation. Phys. Fluids 2024, 36, 077153. [Google Scholar] [CrossRef]
- Zhou, Y.; Ma, Z.; Ai, Y. Hybrid microfluidic sorting of rare cells based on high throughput inertial focusing and high accuracy acoustic manipulation. RSC Adv. 2019, 9, 31186–31195. [Google Scholar] [CrossRef] [PubMed]
- Weirauch, L.; Giesler, J.; Pesch, G.R.; Baune, M.; Thöming, J. Sorting microparticle mixtures by multiple properties in a single dielectrophoretic filter. Results Eng. 2024, 23, 102641. [Google Scholar] [CrossRef]
- Dittami, G.M.; Sethi, M.; Rabbitt, R.D.; Ayliffe, H.E. Determination of mammalian cell counts, cell size and cell health using the Moxi Z mini automated cell counter. J. Vis. Exp. 2012, 64, 3842. [Google Scholar] [CrossRef]
- Stieß, M. Mechanische Verfahrenstechnik—Partikeltechnologie 1; Springer: Berlin/Heidelberg, Germany, 2009; ISBN 978-3-540-32551-2. [Google Scholar]
- Li, H. Dielectrophoretic separation and manipulation of live and heat-treated cells of Listeria on microfabricated devices with interdigitated electrodes. Sens. Actuators B Chem. 2002, 86, 215–221. [Google Scholar] [CrossRef]
- Choi, S.; Park, J.-K. Microfluidic system for dielectrophoretic separation based on a trapezoidal electrode array. Lab. Chip 2005, 5, 1161–1167. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, J.; Wu, X.; Jiang, Z.; Wang, W. Dielectrophoretic separation of microalgae cells in ballast water in a microfluidic chip. Electrophoresis 2019, 40, 969–978. [Google Scholar] [CrossRef]
- Al-Halhouli, A.; Albagdady, A.; Al-Faqheri, W.; Kottmeier, J.; Meinen, S.; Frey, L.J.; Krull, R.; Dietzel, A. Enhanced inertial focusing of microparticles and cells by integrating trapezoidal microchambers in spiral microfluidic channels. RSC Adv. 2019, 9, 19197–19204. [Google Scholar] [CrossRef]
- Hager, A.; Kloss, C.; Pirker, S.; Goniva, C. Parallel Resolved Open Source CFD-DEM: Method, Validation and Application. J. Comput. Multiph. Flows 2014, 6, 13–27. [Google Scholar] [CrossRef]
- Goniva, C.; Kloss, C.; Deen, N.G.; Kuipers, J.A.; Pirker, S. Influence of rolling friction on single spout fluidized bed simulation. Particuology 2012, 10, 582–591. [Google Scholar] [CrossRef]
- Wang, Z.; Liu, M.; Yang, X. A four-way coupled CFD-DEM modeling framework for charged particles under electrical field with applications to gas insulated switchgears. Powder Technol. 2020, 373, 433–445. [Google Scholar] [CrossRef]
- Ferziger, J.H.; Perić, M. Numerische Strömungsmechanik; Springer: Berlin/Heidelberg, Germany, 2008; ISBN 978-3-540-68228-8. [Google Scholar]
- Moayedi, H.; Amanifard, N.; Deylami, H.M. Evaluation of using micropolar fluid approach for the EHD-enhanced forced convection through a rectangular channel using multiple electrode arrangements. Appl. Therm. Eng. 2019, 159, 113857. [Google Scholar] [CrossRef]
- Aycock, K.I.; Campbell, R.L.; Manning, K.B.; Craven, B.A. A resolved two-way coupled CFD/6-DOF approach for predicting embolus transport and the embolus-trapping efficiency of IVC filters. Biomech. Model. Mechanobiol. 2017, 16, 851–869. [Google Scholar] [CrossRef] [PubMed]
- Cetin, B.; Li, D. Dielectrophoresis in microfluidics technology. Electrophoresis 2011, 32, 2410–2427. [Google Scholar] [CrossRef] [PubMed]
- Zielina, M.; Bielski, A.; Młyńska, A. Leaching of chromium and lead from the cement mortar lining into the flowing drinking water shortly after pipeline rehabilitation. J. Clean. Prod. 2022, 362, 132512. [Google Scholar] [CrossRef]
- Lubbersen, Y.S.; Dijkshoorn, J.P.; Schutyser, M.; Boom, R.M. Visualization of inertial flow in deterministic ratchets. Sep. Purif. Technol. 2013, 109, 33–39. [Google Scholar] [CrossRef]
- Lubbersen, Y.S.; Boom, R.M.; Schutyser, M. High throughput particle separation with a mirrored deterministic ratchet design. Chem. Eng. Process. Process Intensif. 2014, 77, 42–49. [Google Scholar] [CrossRef]
- Wullenweber, M.S.; Kottmeier, J.; Kampen, I.; Dietzel, A.; Kwade, A. Simulative Investigation of Different DLD Microsystem Designs with Increased Reynolds Numbers Using a Two-Way Coupled IBM-CFD/6-DOF Approach. Processes 2022, 10, 403. [Google Scholar] [CrossRef]
- Kottmeier, J.; Wullenweber, M.; Blahout, S.; Hussong, J.; Kampen, I.; Kwade, A.; Dietzel, A. Accelerated Particle Separation in a DLD Device at Re 1 Investigated by Means of µPIV. Micromachines 2019, 10, 768. [Google Scholar] [CrossRef]
- Dincau, B.M.; Aghilinejad, A.; Chen, X.; Moon, S.Y.; Kim, J.-H. Vortex-free high-Reynolds deterministic lateral displacement (DLD) via airfoil pillars. Microfluid. Nanofluid. 2018, 22, 137. [Google Scholar] [CrossRef]
- Holm, S.H.; Zhang, Z.; Beech, J.P.; Gompper, G.; Fedosov, D.A.; Tegenfeldt, J.O. Microfluidic Particle Sorting in Concentrated Erythrocyte Suspensions. Phys. Rev. Appl. 2019, 12, 014051. [Google Scholar] [CrossRef]
- Beech, J. Microfluidics Separation and Analysis of Biological Particles; Lund University: Lund, Sweden, 2011. [Google Scholar]
- Hochstetter, A.; Vernekar, R.; Austin, R.H.; Becker, H.; Beech, J.P.; Fedosov, D.A.; Gompper, G.; Kim, S.-C.; Smith, J.T.; Stolovitzky, G.; et al. Deterministic Lateral Displacement: Challenges and Perspectives. ACS Nano 2020, 14, 10784–10795. [Google Scholar] [CrossRef] [PubMed]
- Davis, J.A. Microfluidic Separation of Blood Components through Deterministic Lateral Displacement. Ph.D. Thesis, Princeton University, Princeton, NJ, USA, 2008. [Google Scholar]
- Pariset, E.; Pudda, C.; Boizot, F.; Verplanck, N.; Berthier, J.; Thuaire, A.; Agache, V. Anticipating Cutoff Diameters in Deterministic Lateral Displacement (DLD) Microfluidic Devices for an Optimized Particle Separation. Small 2017, 13, 1701901. [Google Scholar] [CrossRef] [PubMed]
- Kulrattanarak, T.; van der Sman, R.G.M.; Schroën, C.G.P.H.; Boom, R.M. Analysis of mixed motion in deterministic ratchets via experiment and particle simulation. Microfluid. Nanofluid. 2011, 10, 843–853. [Google Scholar] [CrossRef]
- Kulrattanarak, T.; van der Sman, R.G.M.; Lubbersen, Y.S.; Schroën, C.G.P.H.; Pham, H.T.M.; Sarro, P.M.; Boom, R.M. Mixed motion in deterministic ratchets due to anisotropic permeability. J. Colloid. Interface Sci. 2011, 354, 7–14. [Google Scholar] [CrossRef]
- Kim, S.-C.; Wunsch, B.H.; Hu, H.; Smith, J.T.; Austin, R.H.; Stolovitzky, G. Broken flow symmetry explains the dynamics of small particles in deterministic lateral displacement arrays. Proc. Natl. Acad. Sci. USA 2017, 114, E5034–E5041. [Google Scholar] [CrossRef]
- Vernekar, R.; Krüger, T.; Loutherback, K.; Morton, K.; Inglis, D.W. Anisotropic permeability in deterministic lateral displacement arrays. Lab. Chip 2017, 17, 3318–3330. [Google Scholar] [CrossRef]
- Inglis, D.W. Efficient microfluidic particle separation arrays. Appl. Phys. Lett. 2009, 94, 13510. [Google Scholar] [CrossRef]
- Crews, N.; Darabi, J.; Voglewede, P.; Guo, F.; Bayoumi, A. An analysis of interdigitated electrode geometry for dielectrophoretic particle transport in micro-fluidics. Sens. Actuators B Chem. 2007, 125, 672–679. [Google Scholar] [CrossRef]
- Sadeghian, H.; Hojjat, Y.; Soleimani, M. Interdigitated electrode design and optimization for dielectrophoresis cell separation actuators. J. Electrost. 2017, 86, 41–49. [Google Scholar] [CrossRef]
- Wang, Y.; Du, F.; Baune, M.; Thöming, J. Dielectrophoresis in aqueous suspension: Impact of electrode configuration. Microfluid. Nanofluid. 2014, 17, 499–507. [Google Scholar] [CrossRef]
- Xuan, X.; Zhu, J.; Church, C. Particle focusing in microfluidic devices. Microfluid. Nanofluid. 2010, 9, 1–16. [Google Scholar] [CrossRef]
- Adamíková, J.; Antošová, M.; Polakovič, M. Chromatographic purification of recombinant human erythropoietin. Biotechnol. Lett. 2019, 41, 483–493. [Google Scholar] [CrossRef] [PubMed]
- de Lima, J.M.; Furlani, I.L.; da Silva, L.R.G.; Valverde, A.L.; Cass, Q.B. Micro- and nano-sized amine-terminated magnetic beads in a ligand fishing assay. Anal. Methods 2020, 12, 4116–4122. [Google Scholar] [CrossRef] [PubMed]
- Cao, J.; Cheng, P.; Hong, F. A numerical analysis of forces imposed on particles in conventional dielectrophoresis in microchannels with interdigitated electrodes. J. Electrost. 2008, 66, 620–626. [Google Scholar] [CrossRef]







| Design 1 | Design 2 | ||
|---|---|---|---|
| General | Gap | 23 µm | 40 µm |
| Segment 1 | Tilt angle | 3° | 1° |
| Periodicity (N) | 20 | 40 | |
| Dc1 (Equation (1)) | 8 µm | 8 µm | |
| Segment 2 | Tilt angle | 7° | |
| Periodicity (N) | 8 | ||
| Dc2 (Equation (1)) | 11 µm |
| Experiment | Inlet 1 | Inlet 2 | Inlet 3 |
|---|---|---|---|
| Density variation (Design 1) | 25 mL | 10 mL | 10 mL |
| Shape variation (Design 1) | 25 mL | 10 mL | 10 mL |
| Polydisperse suspensions (Design 1) | 50 mL | 25 mL | 10 mL |
| Polydisperse suspensions (Design 2) | 50 mL | 25 mL | - |
| Experiment | Green Fluorescent | Density PS | Red Fluorescent | Density Melamine | Re Number |
|---|---|---|---|---|---|
| Density | 5 µm PS | 5 µm MF | 82 | ||
| 8 µm PS | 1.01 g/cm3 | 8 µm MF | 1.51 g/cm3 | 52 | |
| Shape | 5 µm × 10 µm elliptical PS | 5 µm MF | 10–80 (inc.: 10) |
| Re | Design 1 | Design 2 | |||||||
|---|---|---|---|---|---|---|---|---|---|
| [mm/s] | [µL/min] | [µL/min] | [µL/min] | [µL/min] | [mm/s] | [µL/min] | [µL/min] | [µL/min] | |
| 1 | 43 | 164 | 127 | 18 | 18 | 25 | 250 | 149 | 101 |
| 2 | 87 | 327 | 253 | 37 | 37 | 50 | 500 | 299 | 202 |
| 5 | 217 | 818 | 633 | 92 | 92 | 125 | 1250 | 747 | 504 |
| 10 | 435 | 1636 | 1266 | 185 | 185 | 250 | 2501 | 1493 | 1008 |
| 20 | 870 | 3271 | 2532 | 370 | 370 | 500 | 5003 | 2986 | 2017 |
| 30 | 1304 | 4907 | 3797 | 555 | 555 | 750 | 7504 | 4479 | 3025 |
| 40 | 1739 | 6542 | 5063 | 740 | 740 | 1000 | 10,006 | 5972 | 4034 |
| 50 | 2174 | 8178 | 6329 | 925 | 925 | 1250 | 12,507 | 7465 | 5042 |
| 60 | 2609 | 9814 | 7595 | 1110 | 1110 | 1500 | 15,009 | 8958 | 6050 |
| 70 | 3043 | 11,449 | 8860 | 1294 | 1294 | 1750 | 17,510 | 10,451 | 7059 |
| 80 | 3478 | 13,085 | 10,126 | 1479 | 1479 | 2000 | 20,012 | 11,944 | 8067 |
| Design | Volume Concentration σi [% mL/mL] | Mass Concentration γi [g/mL] |
|---|---|---|
| 1 | 0.01 | 0.00022 |
| 0.02 | 0.00044 | |
| 0.03 | 0.00066 | |
| 0.04 | 0.00088 | |
| 2 | 0.01 | 0.00022 |
| 0.02 | 0.00044 | |
| 0.05 | 0.00110 | |
| 0.10 | 0.00220 | |
| 0.20 | 0.00440 | |
| 0.40 | 0.00880 |
| Buffer | Particle Size | Material |
|---|---|---|
| 1 µS/cm | 8 µm | PS |
| 8 µm | PS, Au coated | |
| 5 µm | PS | |
| 10 µm | PS | |
| 1 mS/cm | 10 µm | PS |
| 5 mS/cm | 10 µm | PS |
| Description | Symbol | Value | Unit |
|---|---|---|---|
| Particle diameter | dp | 0.01 | m |
| Particle charge | qp | 1 | C |
| Particle mass | mp | 5.236 × 10−4 | kg |
| Voltage (DC) | Uφ | −5.0 × 10−6 | V |
| Electrode separation | d | 0.1 | m |
| Description | Symbol | Value | Unit |
|---|---|---|---|
| Particle diameter | dp | 0.01 | m |
| Particle volume | Vp | 5.236 × 10−7 | m3 |
| Particle mass | mp | 5.236 × 10−4 | kg |
| Radius electrode | R1 | 0.02 | m |
| Radius grounding | R2 | 0.06 | m |
| Medium permittivity | εm | 8.85 × 10−12 | F/m |
| Voltage (DC) | Uφ | 20,000 | V |
| Clausius–Mossotti factor | fCM | 1 | [-] |
| Inlet Velocity | Reynolds Number DLD Channel (Re) | Reynolds Number DEP Channel (Re) | Particle Diameter (dp) | Domain Depth | Core-Shell |
|---|---|---|---|---|---|
| 0.02580 m/s | 1 | 4 | 5 µm | 10 µm | Yes |
| 0.12900 m/s | 5 | 22 | |||
| 0.25800 m/s | 10 | 44 | |||
| 0.02580 m/s | 1 | 4 | 5 µm | 10 µm | No |
| 0.12900 m/s | 5 | 22 | |||
| 0.25800 m/s | 10 | 44 |
| Description | Symbol | Value | Unit |
|---|---|---|---|
| Coefficient of restitution | e | 0.1 | [-] |
| Poisson ratio | ν | 0.5 | [-] |
| Static friction coefficient | μ | 0.1 | [-] |
| Young’s modulus | E | 3 × 108 | Pa |
| Particle radius | rp | 5 × 10−6 | m |
| Particle density | ρp | 1000 | kg/m3 |
| Fluid density | Ρ | 1000 | kg/m3 |
| Kinematic viscosity | υ | 1 × 10−6 | m2/s |
| Voltage (AC) (peek-to-peek) | Uφ,pp | 200 | V |
| Voltage (root mean squared) | Uφ,RMS | 141.41 | V |
| Relative permittivity of the medium | εrm | 78.5 | [-] |
| Conductivity of the medium | σm | 0.0002 | S/m |
| Relative permittivity of the particle | εrp | 2.55 | [-] |
| Conductivity of the particle | σp | 4.7 × 10−4 | S/m |
| Relative permittivity of the core-shell particle shell | εrs | 6.9 | [-] |
| Conductivity of the core-shell particle shell | σs | 4.5 × 106 | S/m |
| Core-shell particle shell width | Δs | 2 × 10−7 | m |
| Frequency of the AC | f | 1 × 106 | 1/s |
| Time step CFD | 1 × 10−7 | s | |
| Time step DEM | 5 × 10−11 | s | |
| Mesh size | 1 × 10−6 | m | |
| Mesh size (dynamically refined) | 5 × 10−7 | m | |
| Number of cells (without dynamic refinement) | 720,000 | [-] | |
| Number of processors | 16 | [-] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kottmeier, J.; Wullenweber, M.S.; Liu, Z.; Kampen, I.; Kwade, A.; Dietzel, A. Experimental and Simulative Investigation of Deterministic Lateral Displacement and Dielectrophoresis Methods for Continuous Multi-Property Particle Sorting. Powders 2025, 4, 13. https://doi.org/10.3390/powders4020013
Kottmeier J, Wullenweber MS, Liu Z, Kampen I, Kwade A, Dietzel A. Experimental and Simulative Investigation of Deterministic Lateral Displacement and Dielectrophoresis Methods for Continuous Multi-Property Particle Sorting. Powders. 2025; 4(2):13. https://doi.org/10.3390/powders4020013
Chicago/Turabian StyleKottmeier, Jonathan, Maike Sophie Wullenweber, Zhen Liu, Ingo Kampen, Arno Kwade, and Andreas Dietzel. 2025. "Experimental and Simulative Investigation of Deterministic Lateral Displacement and Dielectrophoresis Methods for Continuous Multi-Property Particle Sorting" Powders 4, no. 2: 13. https://doi.org/10.3390/powders4020013
APA StyleKottmeier, J., Wullenweber, M. S., Liu, Z., Kampen, I., Kwade, A., & Dietzel, A. (2025). Experimental and Simulative Investigation of Deterministic Lateral Displacement and Dielectrophoresis Methods for Continuous Multi-Property Particle Sorting. Powders, 4(2), 13. https://doi.org/10.3390/powders4020013








