Influence of Underlying Topography on Post-Monsoon Cyclonic Systems over the Indian Peninsula
Abstract
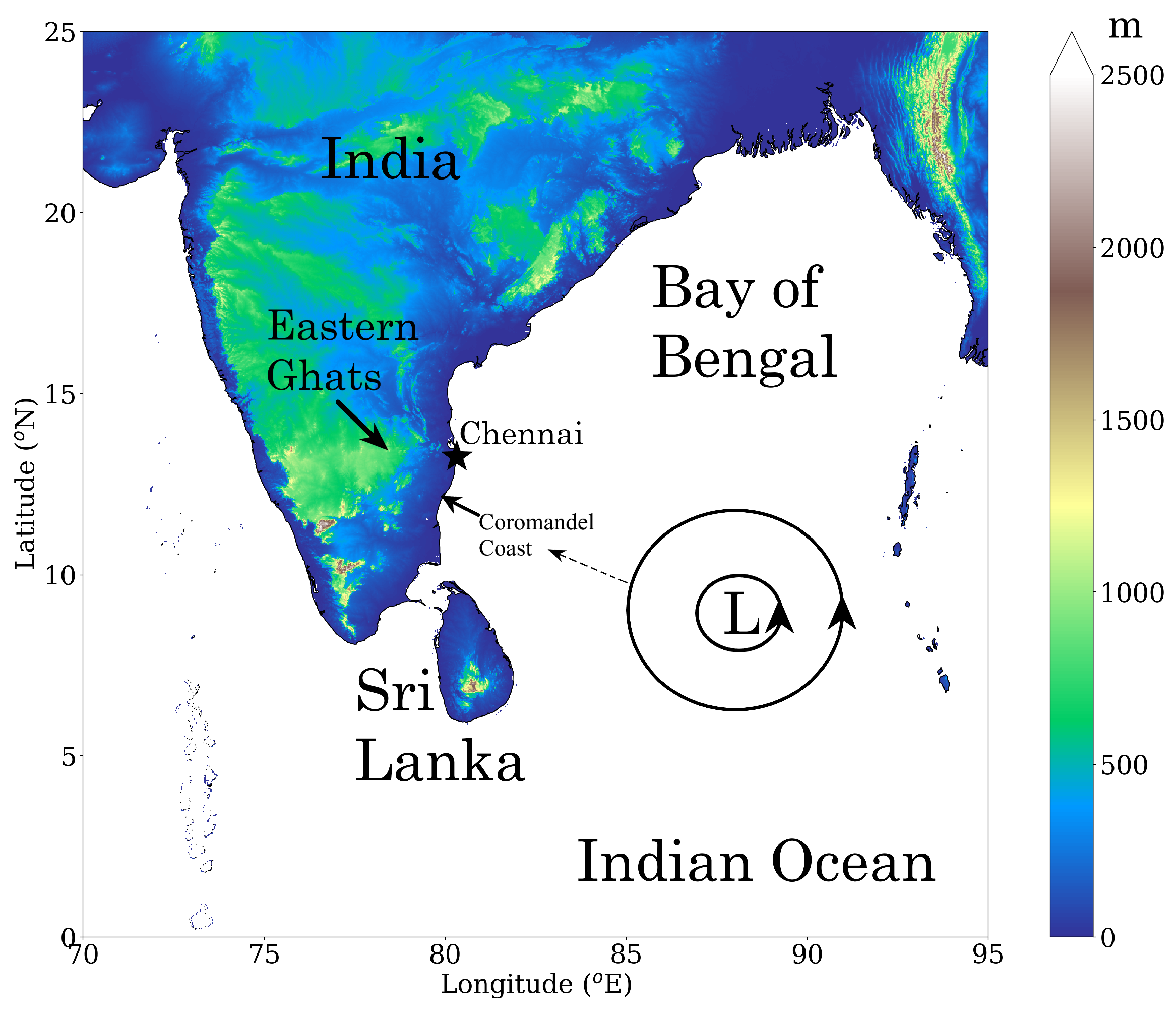
1. Introduction
2. Data
2.1. Dynamics
2.2. Convection and Rainfall
3. Event Description
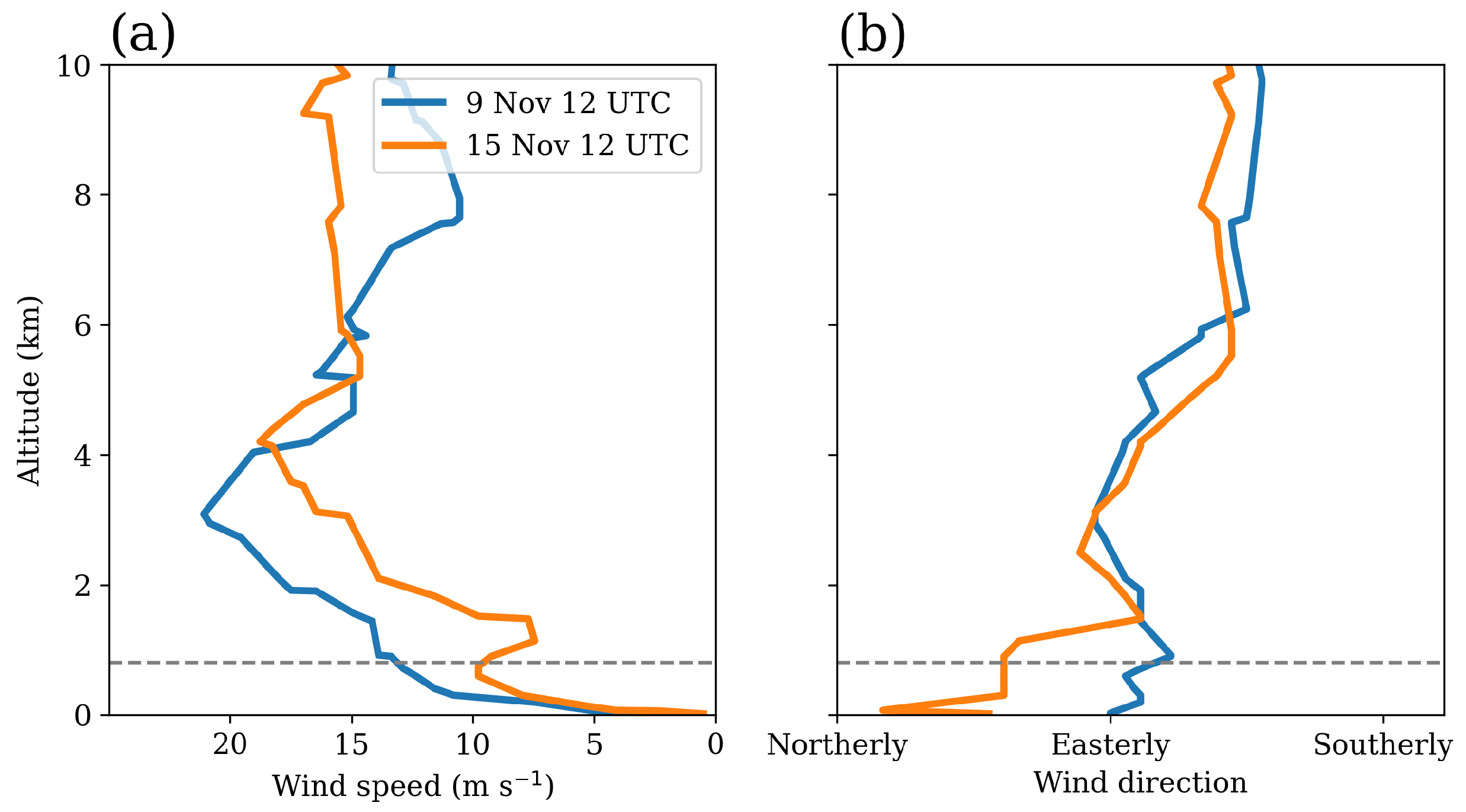
3.1. Propagation of Vortices
3.2. Convection and Rainfall
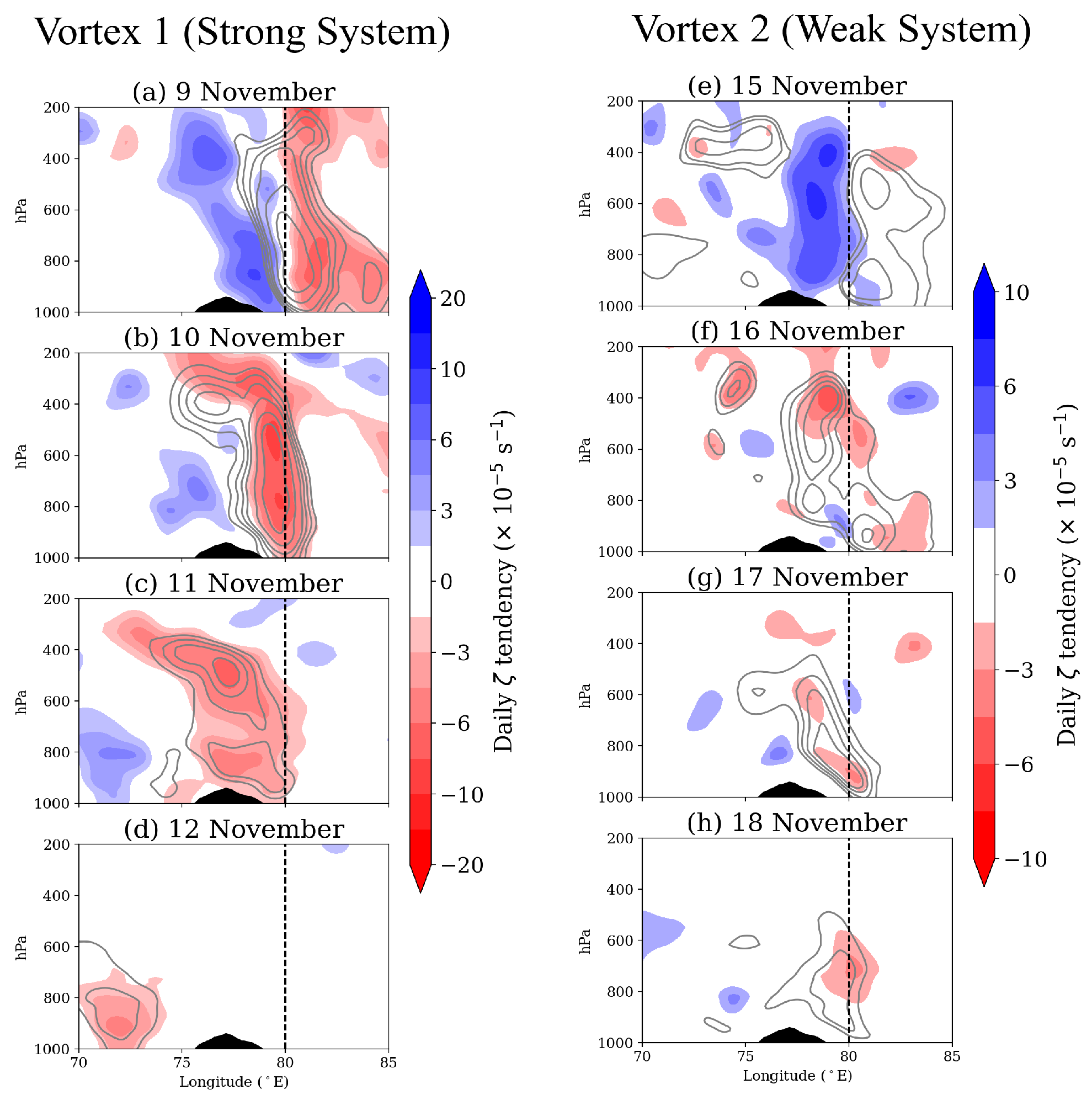
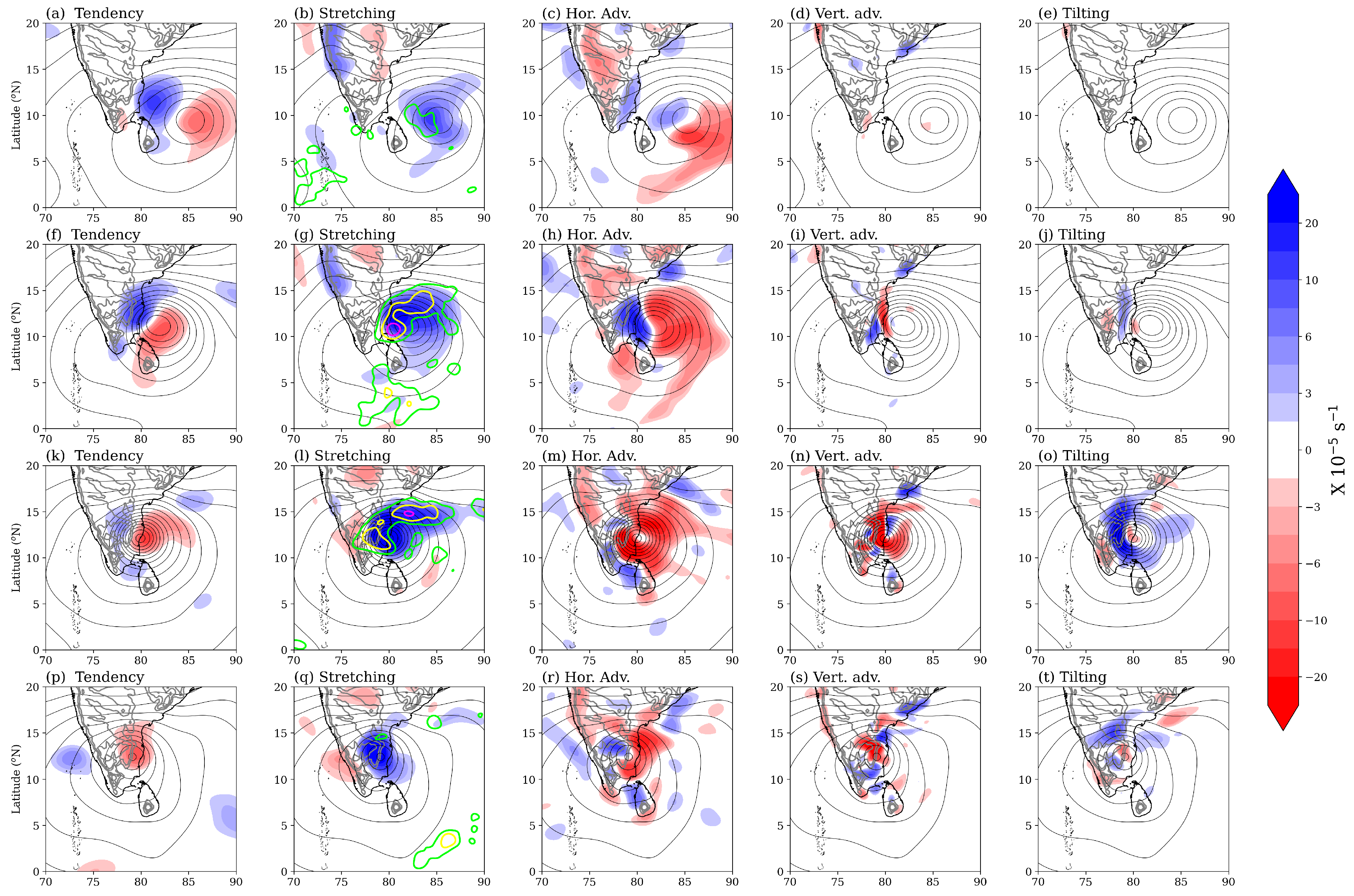
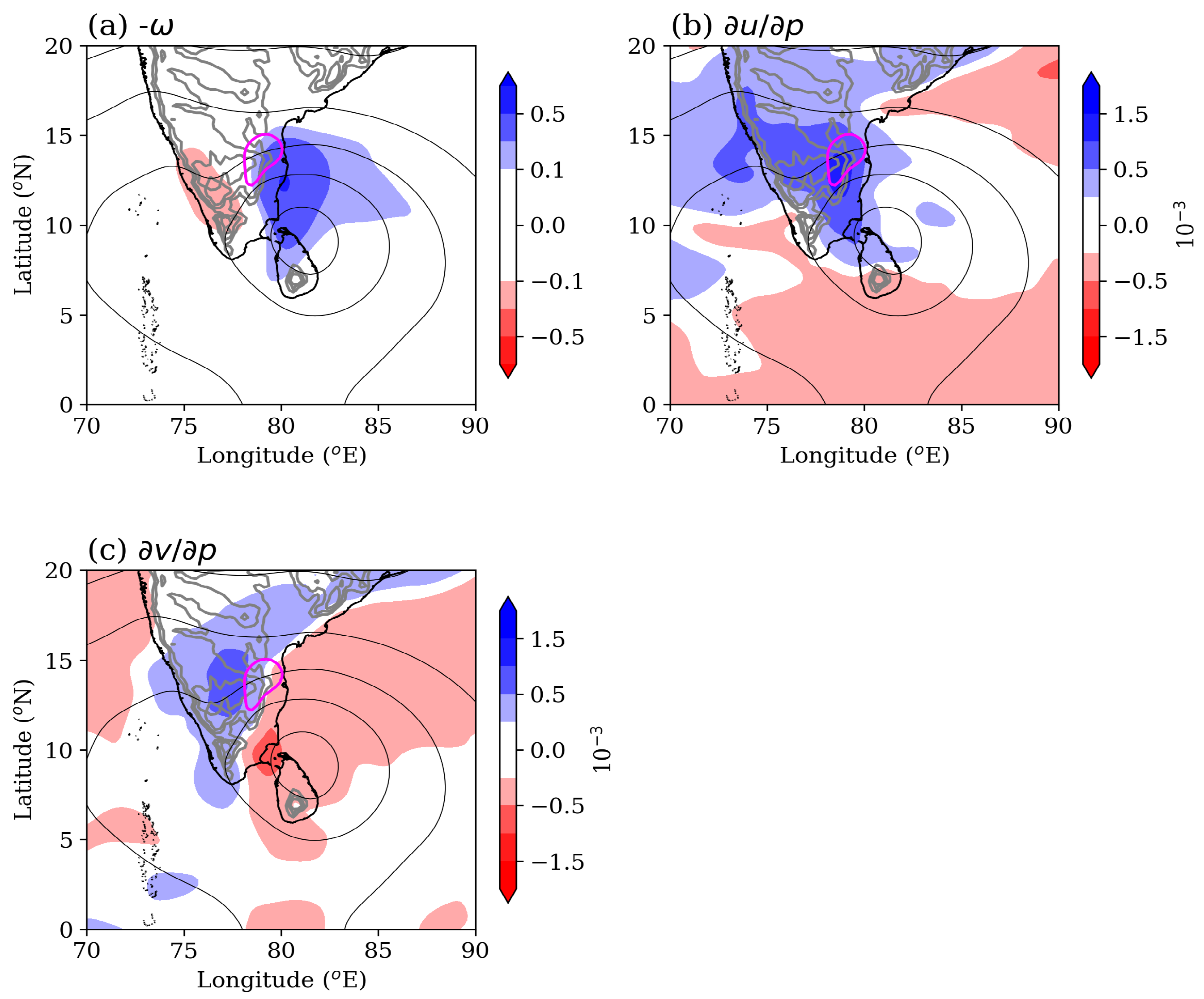
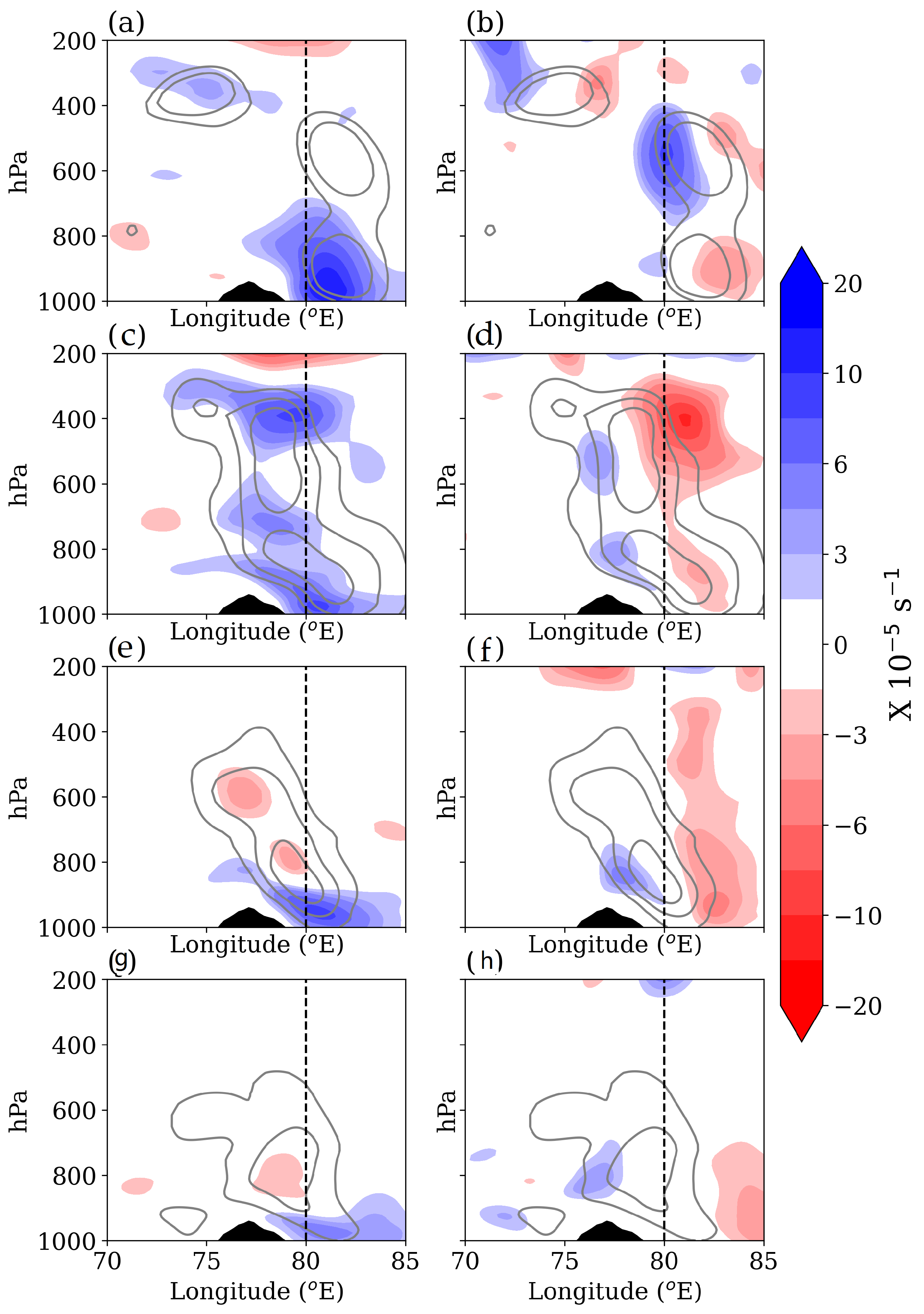
4. Vorticity Budget
4.1. Vortex-1: Strong Vortex
4.2. Vortex-2: Weak Vortex
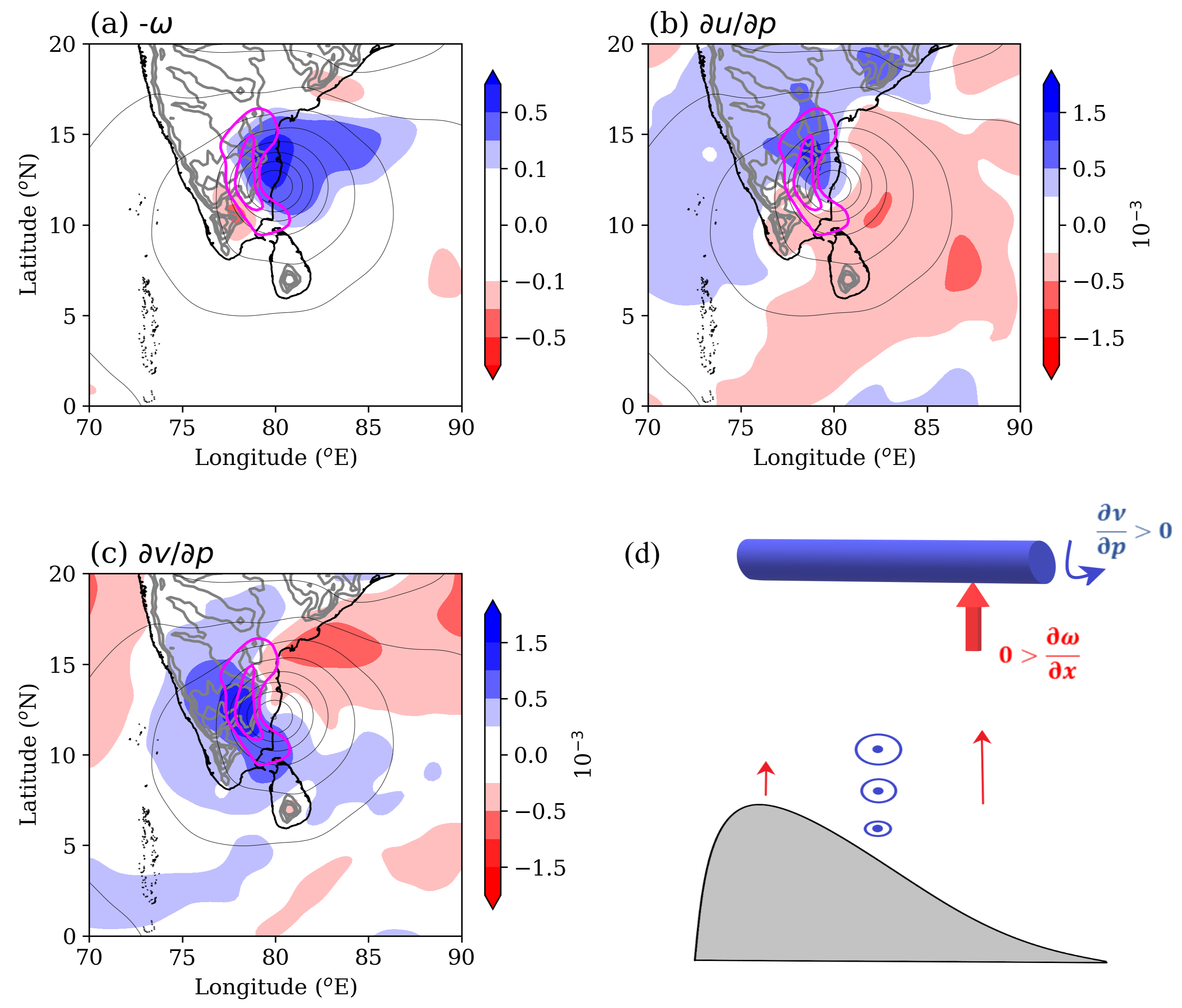
4.3. Orographic Blocking
5. Conclusions and Discussion
- Vortex stretching and horizontal advection were the dominant terms in the vorticity budgets of these vortices. The horizontal vorticity advection was positive on the western side and negative on the eastern side of the vortices. This dipole favored the westward progression of the vortices.
- The vertical advection of vorticity was significant only over the orographic slope. This quantity was negative in the ascending zone and positive in the descending zone due to the westward tilt of the vortex near the orography.
- The tilting of the vorticity filaments was stronger in Vortex-1 due to the stronger ascent over the coast. This resulted in a cyclonic vorticity tendency due to tilting over the orographic slopes in Vortex-1. In the case of (the weaker) Vortex-2, the vorticity filaments were tilted due to the barrier jet in a manner so that an anticyclonic vorticity tendency appeared over the orographic slope on the western side of the vortex.
- The vortex stretching always occurred upstream of the orography in Vortex-2 due to the convergence caused by upstream orographic blocking. Hence, Vortex-2 remained upstream of the orography. The stronger winds in Vortex-1 resulted in convergence and vortex stretching over the orography, notwithstanding its barrier effect. Therefore, Vortex-1 moved over the orography swiftly.
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Charney, J.G.; Eliassen, A. On the growth of the hurricane depression. J. Atmos. Sci. 1964, 21, 68–75. [Google Scholar] [CrossRef]
- Chan, J.C.; Williams, R. Analytical and numerical studies of the beta-effect in tropical cyclone motion. Part I: Zero mean flow. J. Atmos. Sci. 1987, 44, 1257–1265. [Google Scholar] [CrossRef]
- Fiorino, M.; Elsberry, R.L. Some aspects of vortex structure related to tropical cyclone motion. J. Atmos. Sci. 1989, 46, 975–990. [Google Scholar] [CrossRef]
- Wang, Y.; Wu, C.C. Current understanding of tropical cyclone structure and intensity changes—A review. Meteorl. Atmos. Phys. 2004, 87, 257–278. [Google Scholar] [CrossRef]
- Chan, J.C. The physics of tropical cyclone motion. Annu. Rev. Fluid Mech. 2005, 37, 99–128. [Google Scholar] [CrossRef]
- Tamarin, T.; Kaspi, Y. The poleward motion of extratropical cyclones from a potential vorticity tendency analysis. J. Atmos. Sci. 2016, 73, 1687–1707. [Google Scholar] [CrossRef]
- Sengupta, D.; Goddalehundi, B.R.; Anitha, D. Cyclone-induced mixing does not cool SST in the post-monsoon North Bay of Bengal. Atmos. Sci. Lett. 2008, 9, 1–6. [Google Scholar] [CrossRef]
- Van Oldenborgh, G.J.; Van Der Wiel, K.; Sebastian, A.; Singh, R.; Arrighi, J.; Otto, F.; Haustein, K.; Li, S.; Vecchi, G.; Cullen, H. Attribution of extreme rainfall from Hurricane Harvey, August 2017. Environ. Res. Lett. 2017, 12, 124009. [Google Scholar] [CrossRef]
- Chakraborty, A. A synoptic-scale perspective of heavy rainfall over Chennai in November 2015. Curr. Sci. 2016, 111, 201–207. [Google Scholar] [CrossRef]
- Phadtare, J. Role of Eastern Ghats Orography and Cold Pool in an Extreme Rainfall Event over Chennai on 1 December 2015. Mon. Weather Rev. 2018, 146, 943–965. [Google Scholar] [CrossRef]
- Stevens, J.; Carlowicz, M. Historic Rainfall Floods Southeast India. 2015. Available online: https://earthobservatory.nasa.gov/images/87131/historic-rainfall-floods-southeast-india (accessed on 12 March 2023).
- Boyaj, A.; Ashok, K.; Ghosh, S.; Devanand, A.; Dandu, G. The Chennai extreme rainfall event in 2015: The Bay of Bengal connection. Clim. Dyn. 2018, 50, 2867–2879. [Google Scholar] [CrossRef]
- Zubair, L.; Ropelewski, C.F. The strengthening relationship between ENSO and northeast monsoon rainfall over Sri Lanka and southern India. J. Clim. 2006, 19, 1567–1575. [Google Scholar] [CrossRef]
- Kumar, P.; Kumar, K.R.; Rajeevan, M.; Sahai, A. On the recent strengthening of the relationship between ENSO and northeast monsoon rainfall over South Asia. Clim. Dyn. 2007, 28, 649–660. [Google Scholar] [CrossRef]
- Geethalakshmi, V.; Yatagai, A.; Palanisamy, K.; Umetsu, C. Impact of ENSO and the Indian Ocean Dipole on the north-east monsoon rainfall of Tamil Nadu State in India. Hydrol. Process. Int. J. 2009, 23, 633–647. [Google Scholar] [CrossRef]
- Yadav, R. Why is ENSO influencing Indian northeast monsoon in the recent decades? Int. J. Climatol. 2012, 32, 2163–2180. [Google Scholar] [CrossRef]
- Rajeevan, M.; Unnikrishnan, C.; Bhate, J.; Niranjan Kumar, K.; Sreekala, P. Northeast monsoon over India: Variability and prediction. Meteorol. Appl. 2012, 19, 226–236. [Google Scholar] [CrossRef]
- Sreekala, P.; Rao, S.V.B.; Rajeevan, M. Northeast monsoon rainfall variability over south peninsular India and its teleconnections. Theor. Appl. Climatol. 2012, 108, 73–83. [Google Scholar] [CrossRef]
- Krishnamurthy, L.; Vecchi, G.A.; Yang, X.; van der Wiel, K.; Balaji, V.; Kapnick, S.B.; Jia, L.; Zeng, F.; Paffendorf, K.; Underwood, S. Causes and probability of occurrence of extreme precipitation events like Chennai 2015. J. Clim. 2018, 31, 3831–3848. [Google Scholar] [CrossRef]
- van Oldenborgh, G.; Otto, F.; Haustein, K.; Achuta Rao, K. The heavy precipitation event of December 2015 in Chennai, India. B Am. Meteorol. Soc. 2016, 97, S87–S91. [Google Scholar] [CrossRef]
- Leroux, M.D.; Wood, K.; Elsberry, R.; Cayanan, E.; Hendricks, E.; Kucas, M.; Otto, P.; Rogers, R.; Sampson, B.; Yu, Z. Recent Advances in Research and Forecasting of Tropical Cyclone Track, Intensity, and Structure at Landfall. Trop. Cyclone Res. Rev. 2018, 7, 85–105. [Google Scholar] [CrossRef]
- Vaidya, S.; Mukhopadhyay, P.; Trivedi, D.; Sanjay, J.; Singh, S. Prediction of tropical systems over Indian region using mesoscale model. Meteorl. Atmos. Phys. 2004, 86, 63–72. [Google Scholar] [CrossRef]
- Mohapatra, M.; Nayak, D.; Sharma, R.; Bandyopadhyay, B. Evaluation of official tropical cyclone track forecast over north Indian Ocean issued by India Meteorological Department. J. Earth Syst. Sci. 2013, 122, 589–601. [Google Scholar] [CrossRef]
- Osuri, K.K.; Mohanty, U.; Routray, A.; Niyogi, D. Improved prediction of Bay of Bengal tropical cyclones through assimilation of Doppler weather radar observations. Mon. Weather Rev. 2015, 143, 4533–4560. [Google Scholar] [CrossRef]
- Mohanty, U.; Osuri, K.K.; Pattanayak, S. Tropical Cyclone Research over the North Indian Ocean: Impact of Data and Vortex Initialization in High Resolution Mesoscale Models. In Advanced Numerical Modeling and Data Assimilation Techniques for Tropical Cyclone Prediction; Springer Dordrecht: Berlin/Heidelberg, Germany, 2016; pp. 465–495. [Google Scholar] [CrossRef]
- Kumkar, Y.V.; Sen, P.; Chaudhari, H.S.; Oh, J.H. Tropical cyclones over the North Indian Ocean: Experiments with the high-resolution global icosahedral grid point model GME. Meteorl. Atmos. Phys. 2018, 130, 23–37. [Google Scholar] [CrossRef]
- Routray, A.; Dutta, D.; George, J.P. Evaluation of Track and Intensity Prediction of Tropical Cyclones Over North Indian Ocean Using NCUM Global Model. Pure Appl. Geophys. 2018, 176, 421–440. [Google Scholar] [CrossRef]
- Daggupaty, S.M.; Sikka, D.R. On the vorticity budget and vertical velocity distribution associated with the life cycle of a monsoon depression. J. Atmos. Sci. 1977, 34, 773–792. [Google Scholar] [CrossRef]
- Sanders, F. Quasi-geostrophic diagnosis of the monsoon depression of 5–8 July 1979. J. Atmos. Sci. 1984, 41, 538–552. [Google Scholar] [CrossRef]
- Douglas, M.W. Structure and dynamics of two monsoon depressions. Part I: Observed structure. Mon. Weather Rev. 1992, 120, 1524–1547. [Google Scholar] [CrossRef]
- Chen, T.C.; Yoon, J.H.; Wang, S.Y. Westward propagation of the Indian monsoon depression. Tellus A Dyn. Meteorol. Oceanogr. 2005, 57, 758–769. [Google Scholar] [CrossRef][Green Version]
- Boos, W.; Hurley, J.; Murthy, V. Adiabatic westward drift of Indian monsoon depressions. Q. J. R. Meteorol. Soc. 2015, 141, 1035–1048. [Google Scholar] [CrossRef]
- Raymond, D.; Jiang, H. A theory for long-lived mesoscale convective systems. J. Atmos. Sci. 1990, 47, 3067–3077. [Google Scholar] [CrossRef]
- Stoelinga, M.T. A potential vorticity-based study of the role of diabatic heating and friction in a numerically simulated baroclinic cyclone. Mon. Weather Rev. 1996, 124, 849–874. [Google Scholar] [CrossRef]
- Zehnder, J.A. The influence of large-scale topography on barotropic vortex motion. J. Atmos. Sci. 1993, 50, 2519–2532. [Google Scholar] [CrossRef]
- Harold, F.P.; Gutro, R. NASA Measures India’s Deadly Flooding Rains. 2015. Available online: https://www.nasa.gov/feature/goddard/97b-north-indian-ocean (accessed on 12 March 2023).
- Hersbach, H.; Bell, B.; Berrisford, P.; Biavati, G.; Horányi, A.; Muñoz Sabater, J.; Nicolas, J.; Peubey, C.; Radu, R.; Rozum, I.; et al. ERA5 Hourly Data on Pressure Levels from 1979 to Present. Copernicus Climate Change Service (C3S) Climate Data Store (CDS). 2018. Available online: https://cds.climate.copernicus.eu/ (accessed on 12 November 2022).
- Pierrehumbert, R.; Wyman, B. Upstream effects of mesoscale mountains. J. Atmos. Sci. 1985, 42, 977–1003. [Google Scholar] [CrossRef]
- Phadtare, J.A.; Fletcher, J.K.; Ross, A.N.; Turner, A.G.; Schiemann, R.K. Froude-number-based rainfall regimes over the Western Ghats mountains of India. Q. J. R. Meteorol. Soc. 2022, 148, 3388–3405. [Google Scholar] [CrossRef]
- Huffman, G.J.; Bolvin, D.T.; Nelkin, E.J.; Tan, J. Integrated Multi-satellitE Retrievals for GPM (IMERG) technical documentation. NASA/GSFC Code 2015, 612, 2019. [Google Scholar]
- Adames, Á.F.; Ming, Y. Interactions between water vapor and potential vorticity in synoptic-scale monsoonal disturbances: Moisture vortex instability. J. Atmos. Sci. 2018, 75, 2083–2106. [Google Scholar] [CrossRef]
- Phadtare, J.; Bhat, G. Characteristics of deep cloud systems under weak and strong synoptic forcing during the Indian summer monsoon season. Mon. Weather Rev. 2019, 147, 3741–3758. [Google Scholar] [CrossRef]
- Holton, J.R.; Hakim, G.J. Circulation, Vorticity, and Potential Vorticity. In An Introduction to Dynamic Meteorology, 5th ed.; Academic Press: Cambridge, MA, USA, 2013; pp. 95–125. [Google Scholar]
- Lin, Y.L.; Wang, T.A. Flow regimes and transient dynamics of two-dimensional stratified flow over an isolated mountain ridge. J. Atmos. Sci. 1996, 53, 139–158. [Google Scholar] [CrossRef]
- Hurley, J.V.; Boos, W.R. A global climatology of monsoon low-pressure systems. Q. J. R. Meteorol. Soc. 2015, 141, 1049–1064. [Google Scholar] [CrossRef]
- Hunt, K.M.; Turner, A.G.; Inness, P.M.; Parker, D.E.; Levine, R.C. On the structure and dynamics of Indian monsoon depressions. Mon. Weather Rev. 2016, 144, 3391–3416. [Google Scholar] [CrossRef]
- Kushwaha, P.; Sukhatme, J.; Nanjundiah, R. A Global Tropical Survey of Midtropospheric Cyclones. Mon. Weather Rev. 2021, 149, 2737–2753. [Google Scholar] [CrossRef]
- Thomas, T.M.; Bala, G.; Srinivas, V.V. Characteristics of the monsoon low pressure systems in the Indian subcontinent and the associated extreme precipitation events. Clim. Dyn. 2021, 56, 1859–1878. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Phadtare, J. Influence of Underlying Topography on Post-Monsoon Cyclonic Systems over the Indian Peninsula. Meteorology 2023, 2, 329-343. https://doi.org/10.3390/meteorology2030020
Phadtare J. Influence of Underlying Topography on Post-Monsoon Cyclonic Systems over the Indian Peninsula. Meteorology. 2023; 2(3):329-343. https://doi.org/10.3390/meteorology2030020
Chicago/Turabian StylePhadtare, Jayesh. 2023. "Influence of Underlying Topography on Post-Monsoon Cyclonic Systems over the Indian Peninsula" Meteorology 2, no. 3: 329-343. https://doi.org/10.3390/meteorology2030020
APA StylePhadtare, J. (2023). Influence of Underlying Topography on Post-Monsoon Cyclonic Systems over the Indian Peninsula. Meteorology, 2(3), 329-343. https://doi.org/10.3390/meteorology2030020






