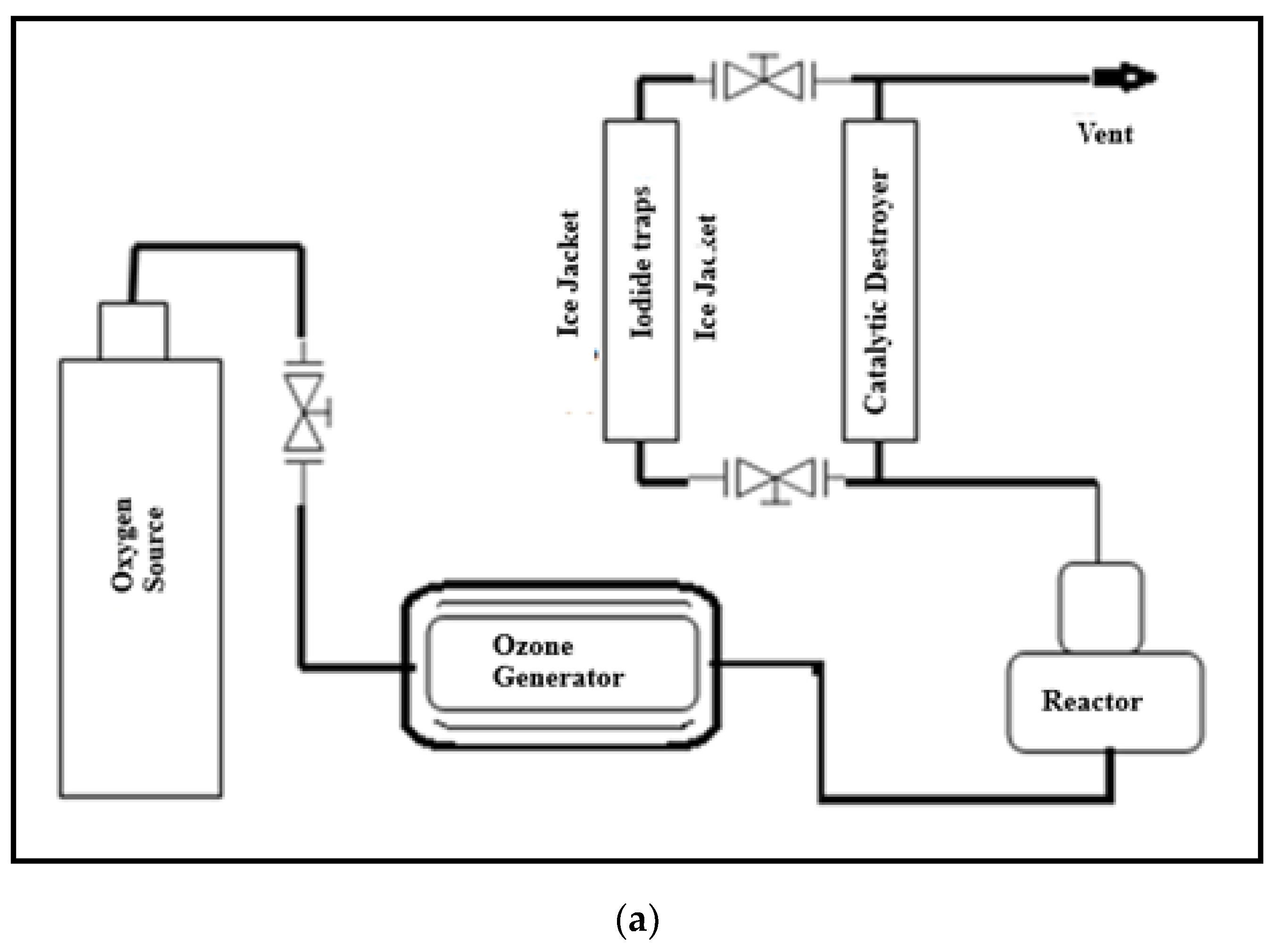
1. Introduction
In the last twenty years, there has been a huge growth in the consumption of liquid fuels rather than fossil fuels. Liquid fuels are generally derived from lignocellulosic biomass wastes that are used to produce energy. They are originally found in the agricultural or animal substances. The sources of biomass are many, including but not limited to, forest or wood residues, wastes from foodstuff processing and livestock farming, or wastes from water treatment plants [
1]. The main ingredients of agricultural residues are cellulose, hemicellulose and lignin. The disposition of these components in the structure is that lignin occupies the spaces in the cell wall, which mediates the cellulose and hemicellulose. Explaining the nature for lignocellulosic in terms of chemistry, lignin is covalently bonded to hemicellulose and cross-linked with cellulose. In general, lignin acts as a hindrance to the biofuel (bioethanol) production process, which is produced from cellulose and hemicellulose only, and therefore requires removal by pretreatments.
The literature shows that there are four sets of pretreatments, grouped into physical, chemical, biological, and physiochemical [
2]. The major principle of physical pretreatment is to reduce the particle size, to enhance the surface area of a substrate and degrade the polymer chains and crystallinity [
3]. This turns out to be more effective and trouble-free for the downstream processes [
4] The major drawbacks lie in the amount of investment in mechanical equipment and high-energy consumption [
5]. Additionally, chemical pretreatment is a good alternative with respect to the physical pretreatment, which includes treatments with alkali, acids and ionic liquids, or deep eutectic solvents. However, the disadvantage of the chemical pretreatment is the use of expensive, non-environmentally friendly and sometimes highly flammable solvents [
6]. Moreover, the biological pretreatment, including cellular and enzymatic, is a promising eco-friendly option with lower energy consumption and requires no inhibitor during the process. On the other hand, ammonia fiber explosion (AFEX), CO
2 or steam explosion (SE), liquid hot water (LHW), wet oxidation by hydrogen peroxide or ozonation are all considered physio-chemical pretreatments, and each of them has its pros and cons. For many years, ozonation pretreatment has been a topic of interest for many researchers [
7,
8,
9,
10,
11,
12]. This may be attributed to the fact that ozone produces wastes that are less hazardous to the environment compared to conventional pretreatments. Nevertheless, to the best of our knowledge, no one has studied the reaction between ozone and wheat straw.
Based on our earlier findings, it is better to moisten the wheat straw before the start of ozonation because the reaction was very slow with the dry wheat straw, and this may lead to issues related to ozone decomposition. We presumed that the absorbed water on wheat straw causes swelling and improves ozone accessibility to the inner cells. In this paper, we provide a novel technique that is simple and environmentally friendly. The proposed technique is a step-by-step treatment procedure that involves the use of sodium hydroxide (NaOH) solution to remove the natural waxy layer on the wheat straw surface and let the ozone interact with the lignin. Our preliminary work showed if the surface is not cleaned, there will be no reaction of ozone. The main purpose of this technique is to increase the accessibility of ozone for reaction, which leads to an easy lignin removal. It also increases the cellulose exposure to enzymatic hydrolysis and favors benign byproducts.
The study goal is to understand the kinetics governing lignin removal from wheat straw after ozonation pretreatment. Based on a vigorous literature survey, the kinetics of lignin removal using pure oxygen has been widely studied in the paper and pulp industry. In fact, the low contents of lignin, the homogenous distribution of lignin and the gas reactant’s easy access to lignin in pulp helped both the first-order kinetic model and the pseudo-first-order kinetic model to describe the kinetics of delignification. Yun reported the removal of lignin from pulp by oxygen and caustic treatments [
13]. The delignification reaction rate was a first-order type with residual lignin contents and without hexuronic acid. Roncero et al. investigated the kinetics of ozone bleaching on dried materials using the oven and at room temperature [
14]. The ozonation took place in a reactor volume of 0.5 L, perfect mixing was assumed, and the amount of ozone consumed by pulp was tracked every second. In addition, an ozone diffuser was used to provide a consistent and uniform supply of ozone to the fiber suspension. The mass flow rate of ozone into the reactor was 85 mg/min, the flow rate was controlled by a valve and measured by an automatic UV detector. The results showed that the lignin degradation followed first-order kinetics. Other studies have addressed the lignin model to better understand the dynamics of the delignification reaction. For instance, Kishimoto and Sano reported a lignin model comprising phenolic β-o-4 type guaiacylglycerol-β-guaiacyl ether at 160–200 °C to investigate the pathways of lignin removal using high boiling solvent (HBS) [
15]. The results showed that the phenolic β-o-4 linkage was broken down into two or more fragments and the scission of β-o-4 linkage revealed a pseudo-first-order reaction rate. Gasper et al. studied the aerobic lignin removal from Eucalyptus globulus kraft pulp [
16]. The reaction was catalyzed by Mn-molybdovanado-phosphate polyoxoanion (HPA-5-Mn
II), and the measured rates of lignin removal was a pseudo-first-order model. Kim and Holtzapple, treated corn stover with an excess of CaOH
2 under oxidative and non-oxidative conditions and at various temperatures (25, 35, 45 and 55 °C) [
17]. The results revealed first-order reactions for the delignification of corn stoves. Mbachu and Manley [
18] studied the ozonolysis of spruce wood in aqueous acid media at room temperature. The degradation rate of lignin in spruce wood also followed a first-order kinetics. In this paper, the first-order model for delignification reactions, proposed by Mbachu and Manley, was followed for simplicity. However, this was an unsuccessful attempt and modifications were made to the proposed model [
18]. The purpose of the present study is to determine the degradation rate of lignin on wheat straw after ozonation and to go into the mechanism of ozone transportation into the wheat straw matrix.
3. Results and Discussion
In general, the lignin constituents of wheat straw samples began to steadily decrease as the ozonation reaction started.
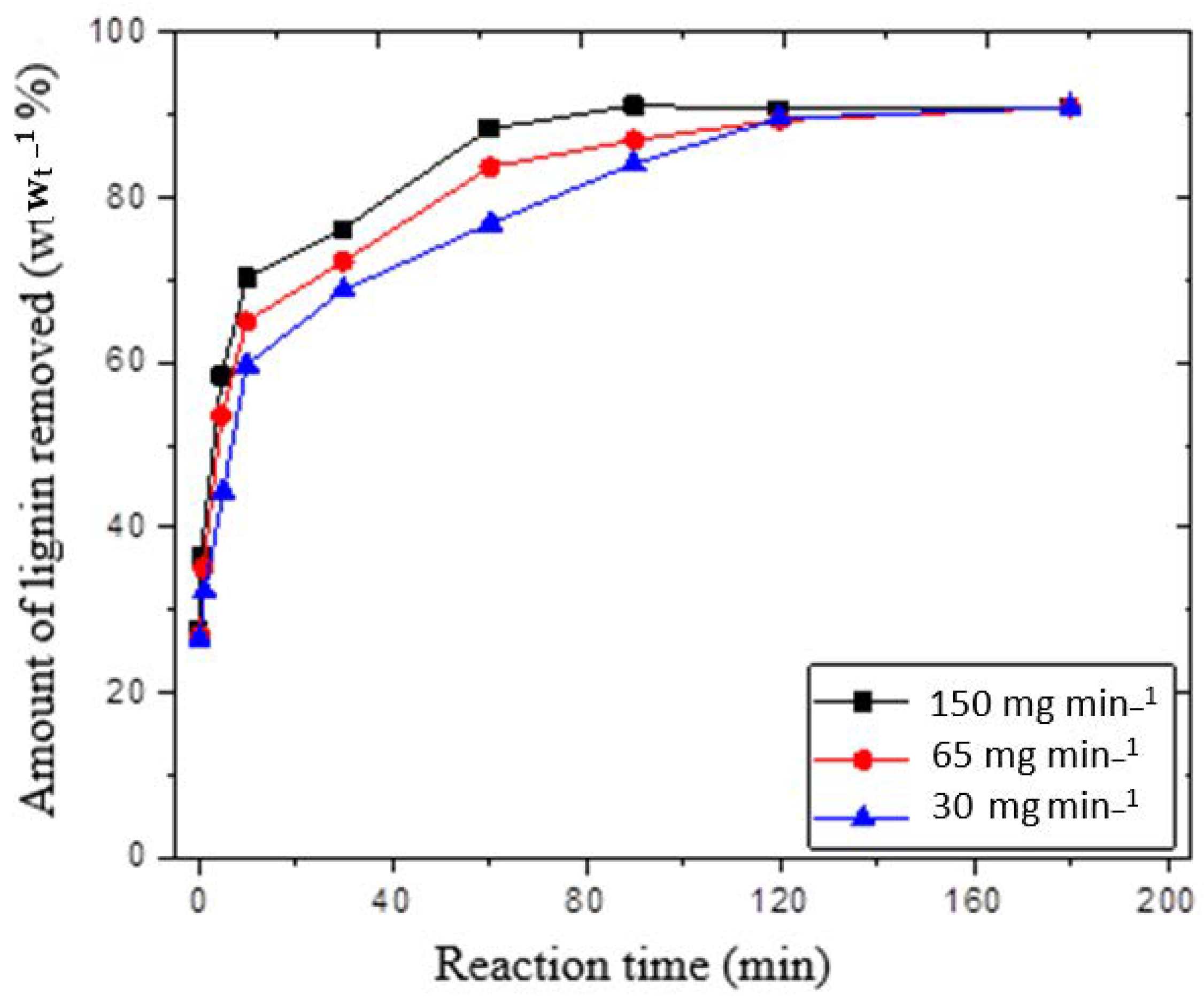
Figure 2 depicts the amount of lignin that was eliminated at various ozone flow rates, ranging from 6.55 to 150 mg min
−1. The ozone in the inlet ozone–oxygen streamline was 2% wt. wt.
−1, and the reaction time (i.e., time of contact between ozone and wheat straw) ranged from 1 to 120 min. In the beginning, a very fast degradation rate was observed, as the ozone had easy access to the lignin. At 65 mg min
−1 ozone supply, the results showed that 65% and then over 90% of lignin was removed in the first 10 min and 110 min, respectively. In fact, the reaction rate varied with respect to the ease of access to lignin content in the pretreated samples. For instance, after a 90 min reaction, there was a clear reduction in the delignification rate because most of the lignin within accessible regions was consumed. Moreover, after a 60-min reaction, approximately 88% of the lignin was removed. Furthermore, the results also revealed that only a 3–5% change in lignin occurred over a long period of time (ranging between 60 to 120 min). The findings showed that 90% of the insoluble lignin was removed from the total lignin content of natural wheat straw after 90 min of contact time at the ozone flow rate of 150 mg min
−1. After a 120-min reaction, the amount of lignin removed was almost the same for ozone flow rates of 30 and 65 mg min
−1. Later, the reaction time was increased from 120 to 180 min and no significant difference in lignin removal was detected for all ozone flow rates. Interestingly, the remaining 8–9% lignin resisted removal under the current experimental conditions.
Whether it is possible to completely remove lignin from wheat straw is a subject of debate. Some of them claim that all the lignin constituents can react with ozone, while others say that a certain amount of lignin is unable to react [
14,
21,
22,
23]. Our experimental study supports the belief that a certain amount of lignin is left behind after ozonation. Kristensen et al. reported the use of scanning electron microscope (SEM) images to present the accessibility routes for ozone [
24]. The images were taken after ozone pretreatment of wheat straw and showed lignin shells, which are hollow parts containing cellulose. Our findings stipulate that, under certain experimental conditions, ozone would not reach the deepest lignin sites. This is not to say that ozone cannot diffuse to the desired point; rather, ozone may damage the component of interest, in this case, cellulose.
In this study, a pseudo-first-order model and pseudo-second-order model were used for the first time to investigate the kinetics of ozonation on wheat straw that has been carried out in a solid–gas reactor. For each order of reaction, the coefficients were computed as a number between 0 and 1 and represented as r
2 to evaluate the delignification process. In general, the higher the value of the determined coefficient, the better the fit is thought to be. Mbachu and Manley [
18] presented the linear mode of a pseudo-first-order model, as shown in Equation (1):
where
Lt and
L0 represent the amount of lignin removed (mg g
−1) from wheat straw at any time ‘
t’ (min), and the initial amount of lignin in the biomass, respectively.
K1 is the reaction rate constant for a pseudo-first-order model.
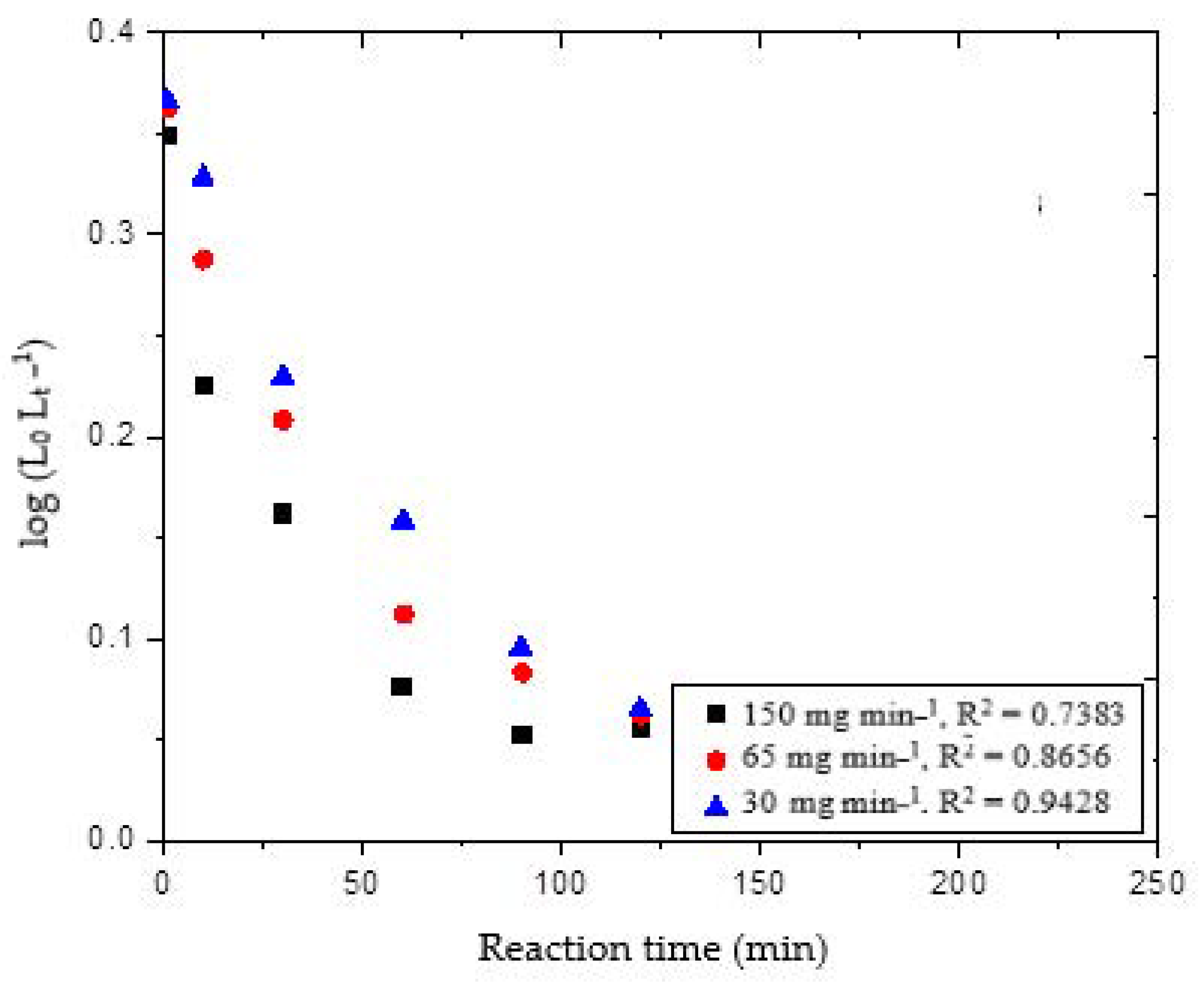
Figure 3 shows the linear mode plot of the pseudo-first-order model for the delignification of wheat straw under different ozone flow rates of 30, 65 and 150 mg min
−1.
Theoretically, the plots should be straight lines while the number of experimental datapoints deviated from the ideal lines; therefore, the correlation coefficients were in the range of 0.74 to 0.94. The r
2 value indicates that the variation in reaction time can explain approximately from 74 to 94% of the variation in the concentration of lignin removal [log (L
o L
t−1)], and that the variables have a moderate degree of correlation [
25]. The correlation factor elucidated that the reaction model was not suitable for the present study. The slope and intercept of these plots were used to determine the reaction constant
K1, and the amount of lignin removed at any given time L
t.
Table 1 presents a comparison of the predicted and experimental values of L
t for the corresponding flow rates. Additionally, we found that lignin degradation rate occurred approximately 1.25 times faster at an ozone supply of 30 mg min
−1 than at a flow rate of 150 mg min
−1. However, the pseudo-first-order model showed poor fitting, with correlation values of 0.74, 0.87 and 0.94 for 30, 65 and 150 mg min
−1, respectively. Based on the results, the pseudo second-order-model was next chosen for the ozone-lignin reaction.
Iribarne and Schroeder suggested lignin removal model from kraft pulp using oxygen [
26]. In Equation (2), a power law relationship is presented, which describes the kinetics govern lignin removal by oxidation, and also indicates the impact of the main reaction parameters such as caustic concentration, oxygen concentration (pressure) and process temperature.
where
E is the activation energy;
R is the ideal gas constant. Moreover, the reaction constants are represented by
α,
β and
n, while
k is the reaction rate coefficient, which depends on the reaction temperature in terms of the Arrhenius law, as shown in Equation (3):
When no acid or alkali were used in the system, the change in [
OH−] was insignificant. The supply of ozone was deemed excessive; therefore, Equation (2) turns into Equation (4).
Equation (5) is the pseudo-second-order model modified from Equation (4) and used to study the kinetics of lignin removal.
where
k is the delignification reaction rate constant (mg
−1 min
−1).
The amount of lignin reacted and removed by ozone in wheat straw at any time
t is represented as
Lt, while the maximum amount of lignin available for removal is
Lmax, which is equal to (
Lo −
Lr).
Lr represents the amount of lignin that could be removed under experimental conditions. The driving force for the delignification of wheat straw is related to (L
max − L
t). As a result, Equation (5) is rearranged by separating the variables, as given in Equation (6):
In addition, the integration of Equation (6) for the boundary conditions where t is 0 to
t and L is
Lmax to (
Lmax−Lt) gives Equation (7):
Again, rearrange the variables in Equation (8). This results in the solution of delignification kinetics, where Equation (5) has the mode of pseudo-second-order model and is presented as follows in Equation (9):
k2 is the reaction rate constant for the pseudo-second-order.
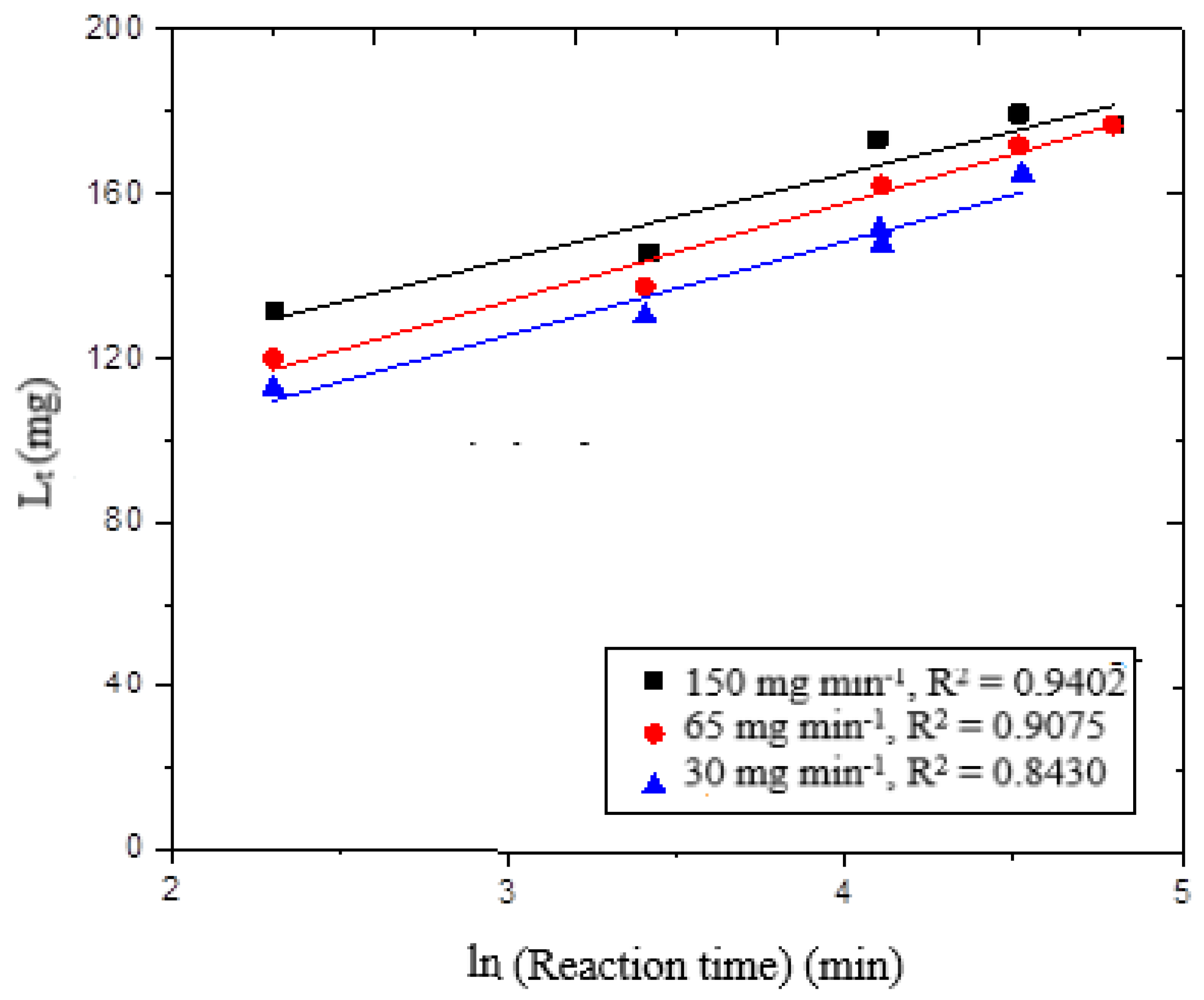
Figure 4 shows the plot of t/L
t versus t for wheat straw. The values of L
t and k
2 are obtained by the slope and intercept.
The correlation coefficient (r
2) values obtained from the pseudo-second-order model were 0.9883, 0.9940 and 0.9977 for ozone supply of 30, 65 and 150 mg min
−1, respectively. We found that the r
2 value obtained here is greater than the one in the pseudo-first-order model. In other words, the lower the error in the prediction of L
max, the kinetics of ozone reaction with lignin in wheat straw likely follows a pseudo-second-order reaction model.
Table 2 shows a further test that was conducted to test the model prediction, in which an ozone reaction with wheat straw was performed for 60 min. This indicated that the prediction error decreased from 4.5 to 0.5% when the ozone supply increased from 30 to 150 mg min
−1.
It is well-known that wheat straw is a biological material made up of various components. Therefore, it is necessary to understand thoroughly the transport mechanism of the delignification reaction in this complicated pattern. First, the kinetics of delignification are explained by the Elovich kinetic model, which assumes that the wheat straw–ozone reaction takes place on the surface. The model holds true when the reversible rate of reaction is negligible. Equation (10) expresses the linear mode of the Elovich kinetic model [
27,
28]
where the initial delignification rate is α (mg g
−1 min
−1) and the parameter β represents the extent of surface coverage by ozone (g mg
−1). To simplify the Elovich equation, it is assumed that αβt << 1. The model is useful in describing chemisorption on highly heterogeneous adsorbents such as agricultural waste materials (wheat straw). On the L
t plot against ln (t), shown in
Figure 5, the kinetic results were linear. The delignification curve showed poor suitability for the r
2 values, which varied from 0.84 to 0.94. From these results, it is clear that the delignification of wheat straw by ozone is affected by more than just a surface reaction; other reactions may also be involved.
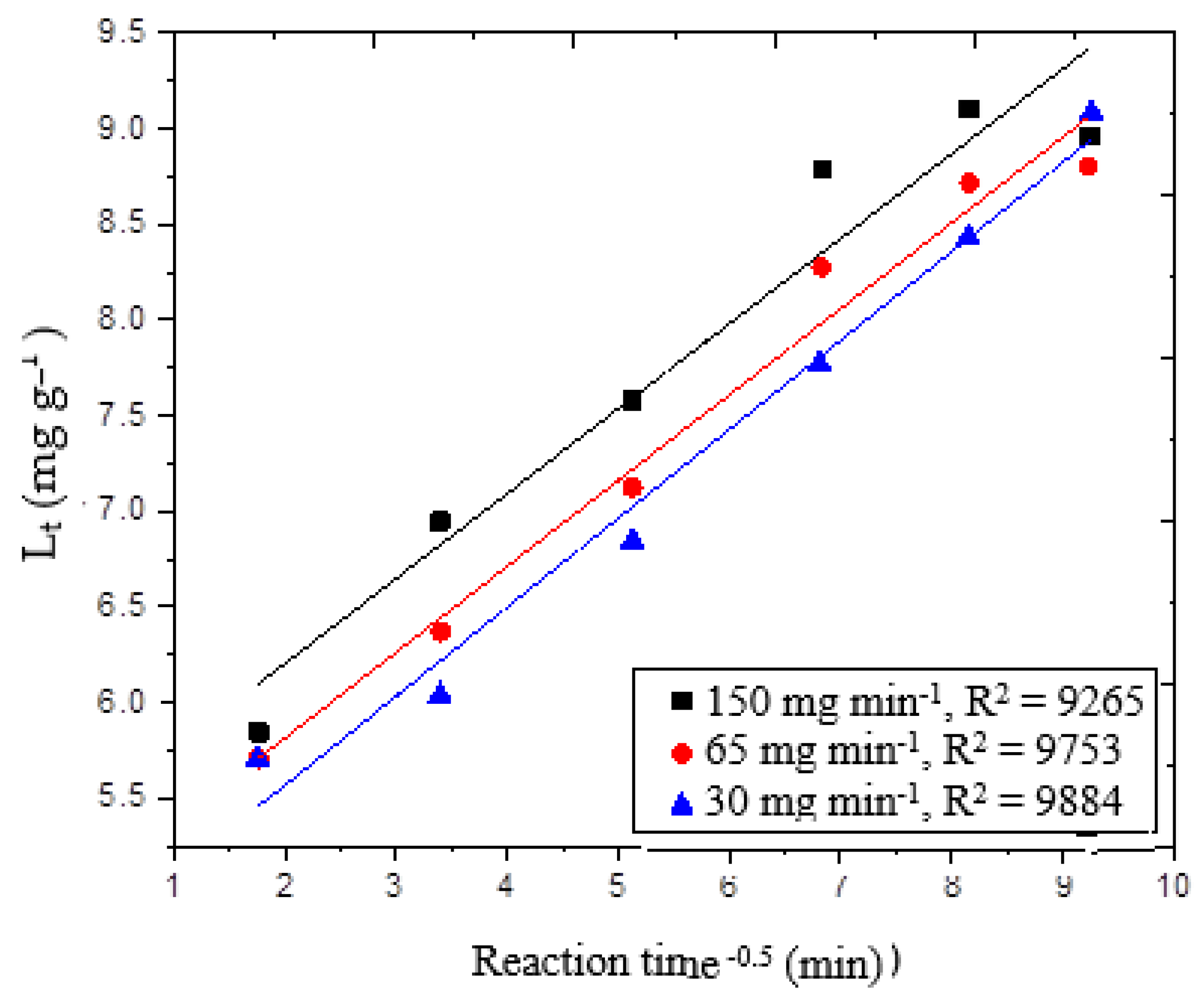
The experimental data were further examined using the intra-particle diffusion model. Alkan et al. found that, in many chemical reactions, solute uptake varies proportionally with t
0.5 rather than the total reaction time [
29], as shown in Equation (11):
where C is the intercept (mg g
−1) and k
3 is the rate of intra-particle diffusion (mg g
−1 min
−0.5). According to the model, if the ozone reaction with wheat straw was solely controlled by the intra-particle diffusion, the plot of L
t against t
0.5 would be linear. This was not the case here, since the intra-particle diffusion was one of the factors contributing delignification reactions.
Figure 6 depicts a single-stage straight line. We think this may be attributed to the rapid covering of the accessible sites on the wheat straw surface. The correlation coefficient for intra-particle diffusion into wheat straw is a straight line with an r
2 greater than 0.92. This means that while the intra-particle diffusion was the primary reaction, it was not the only one that contributed to wheat straw delignification. At 30, 65 and 150 mg min
−1 ozone supply, the predicted value of lignin removed from L
t was evaluated when wheat straw was reacted with ozone for 60 min. The estimated error varied from 0.8 to 7%. The data were further examined in order to reveal more about pore diffusion during the delignification process.
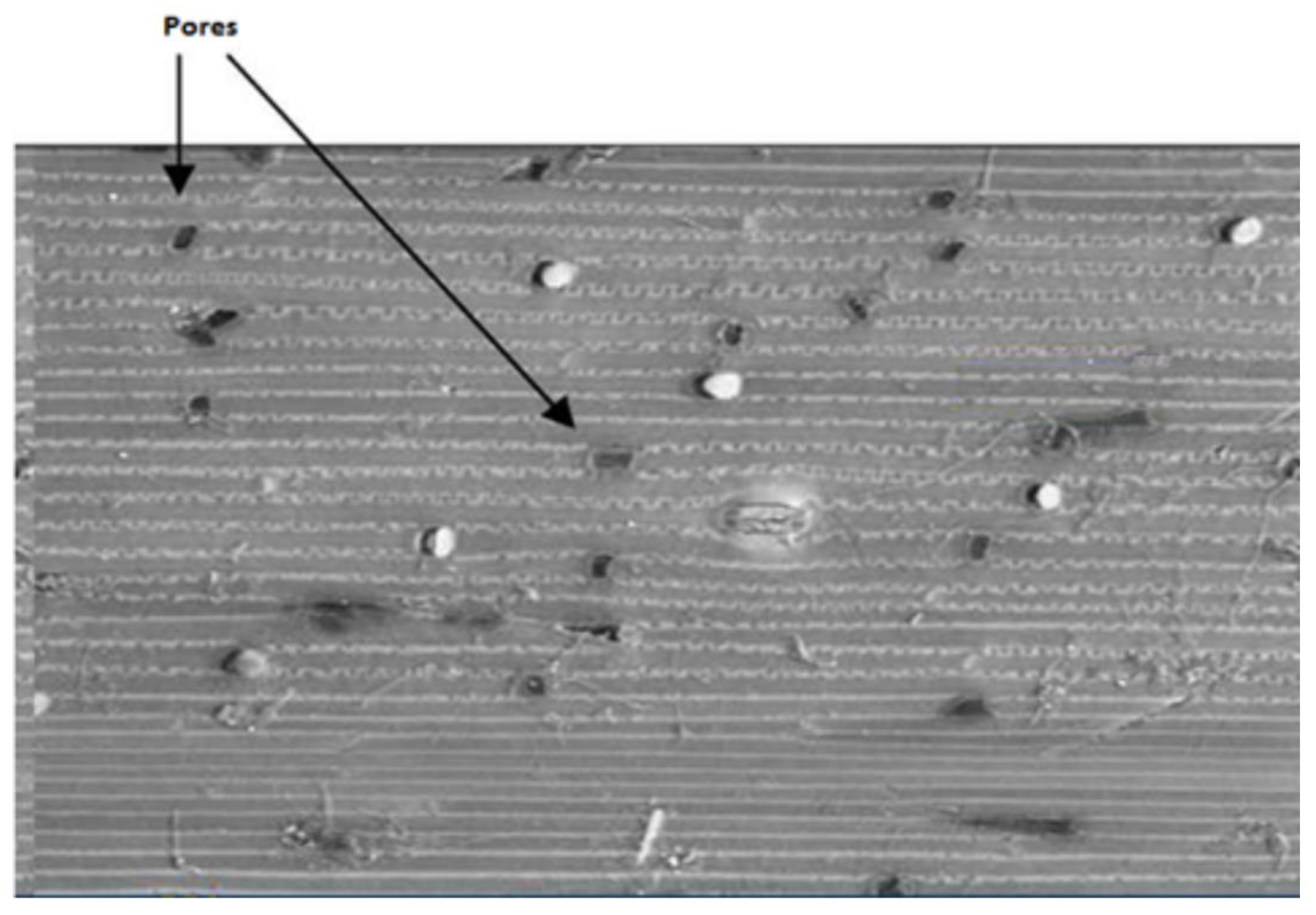
An interesting finding is that at the high ozone supply of 150 mg min−1, ozone may react with lignin on the surface and through intra-particle diffusion. Moreover, at a low ozone supply of 30 mg min−1, ozone may react with the sites of contact, then move to the next site, and so on, until it loses the ability to react or accesses fewer sites.
Figure 7 shows an SEM image of pores on the surface of wheat straw. These pores help ozone to diffuse and react with available lignin sites within wheat straw.
Few researchers have studied the reactivity of lignin compounds with ozone and cover the kinetic models that describe various reaction mechanisms [
30,
31]. On the basis of the literature survey and the experimental results presented in various Tables and Figures in this article, it can be concluded that various reaction pathways may exist due to the existence of various morphological patterns of wheat straw components (lignin, cellulose and hemicellulose), inaccessible lignin sites hidden beneath the layers of other components, the cross-linking of reaction products, and reaction types (physical, chemical). Furthermore, we found that there are various lignin removal pathways involving ozonation, such as adsorption, surface reaction, surface diffusion, and/or intraarticular diffusion. On the basis of this study, the author proposes an ozonation mechanism of lignin removal: (i) ozone adsorbed onto the surface of wheat straw that chemically reacts with the available reaction sites at the surface, (ii) one molecule of ozone reacts with one site; another molecule reaches to the next site through intra-particle diffusion, and (iii) ozone moves through the pores (
Figure 7) in the wheat straw surface (pore diffusion occur) and reacts with other available sites as the lignin is removed. Further studies are required to conduct a physical evaluation of the proposed mechanism.