Fundamental Characteristics of Wind Loading on Vaulted-Free Roofs
Abstract
:1. Introduction
2. Experimental Arrangement and Procedure
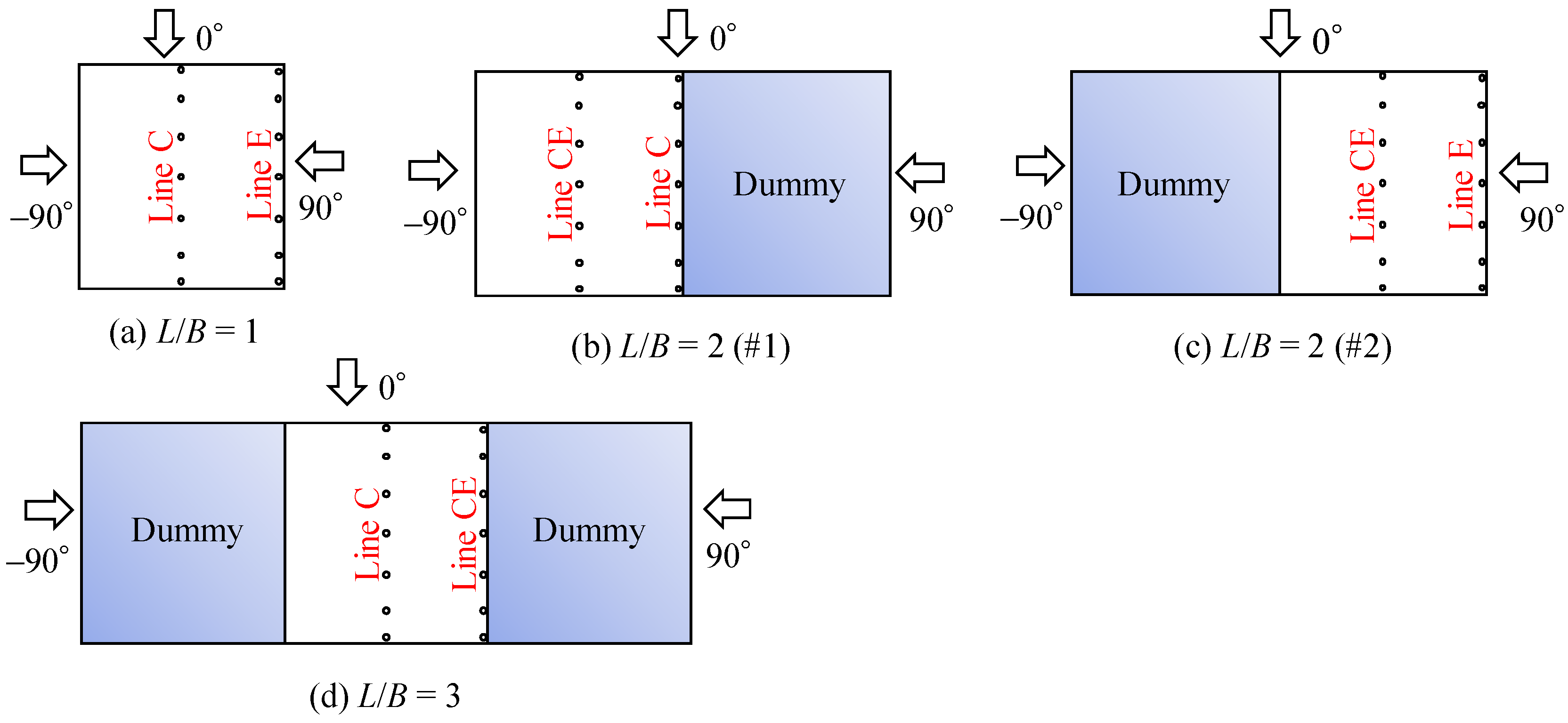
2.1. Investigated Building
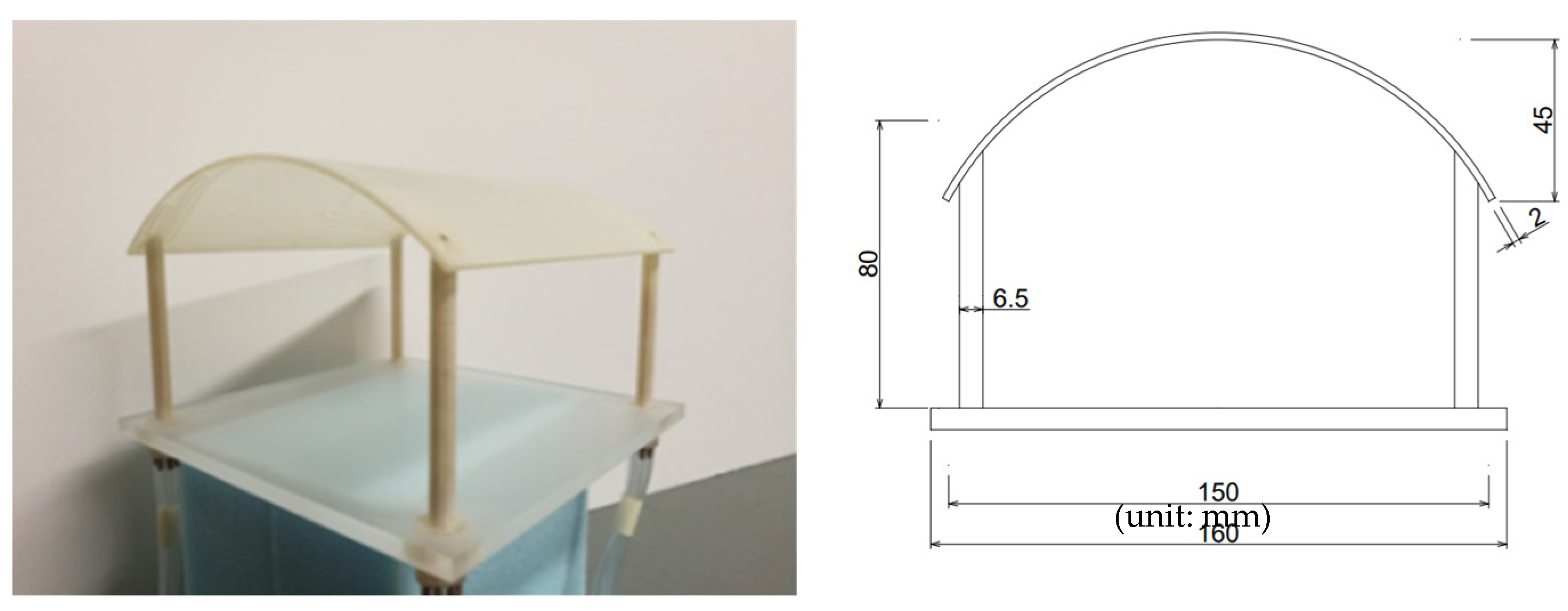
2.2. Wind Tunnel Model
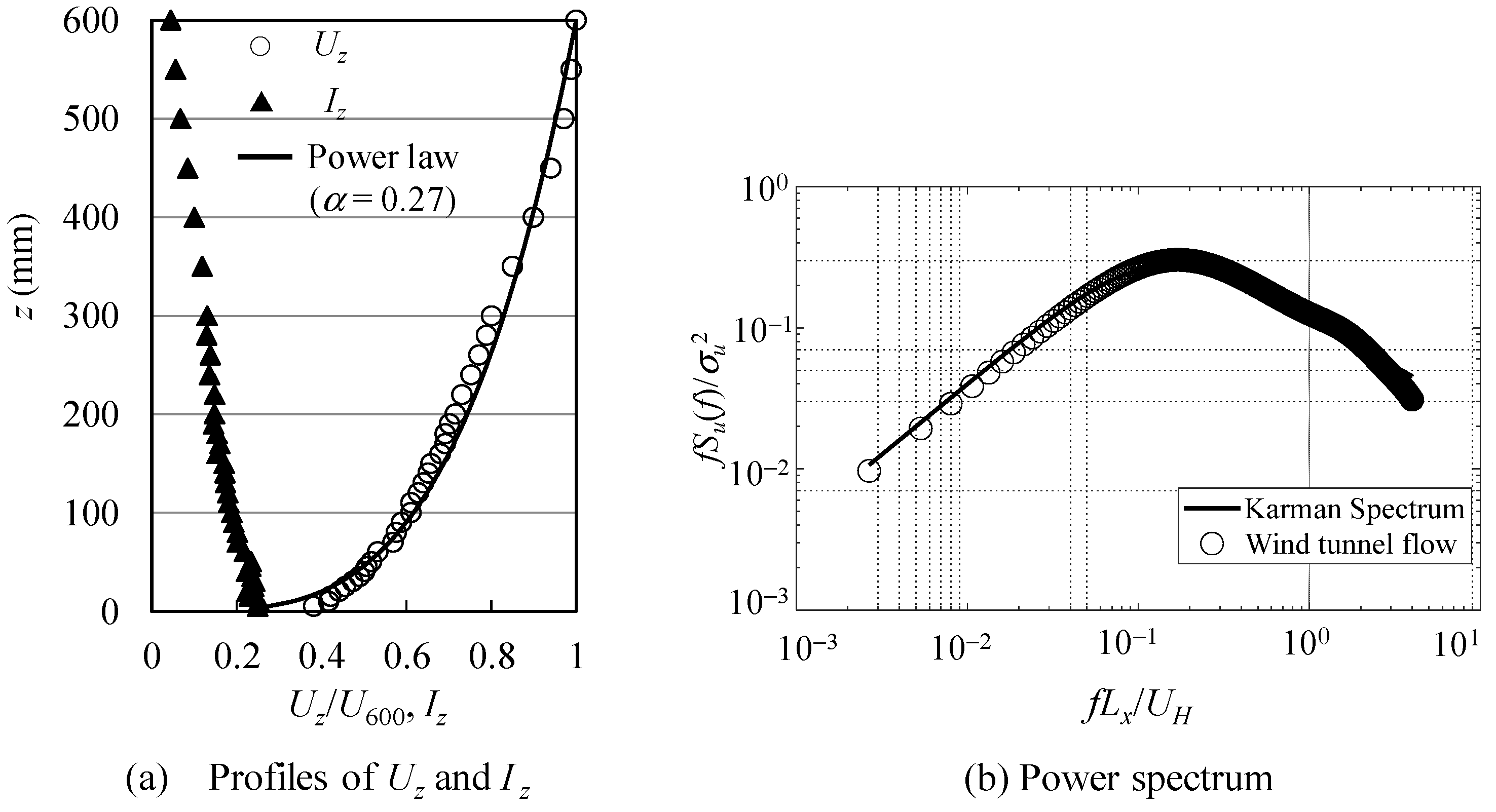
2.3. Wind Tunnel Flow
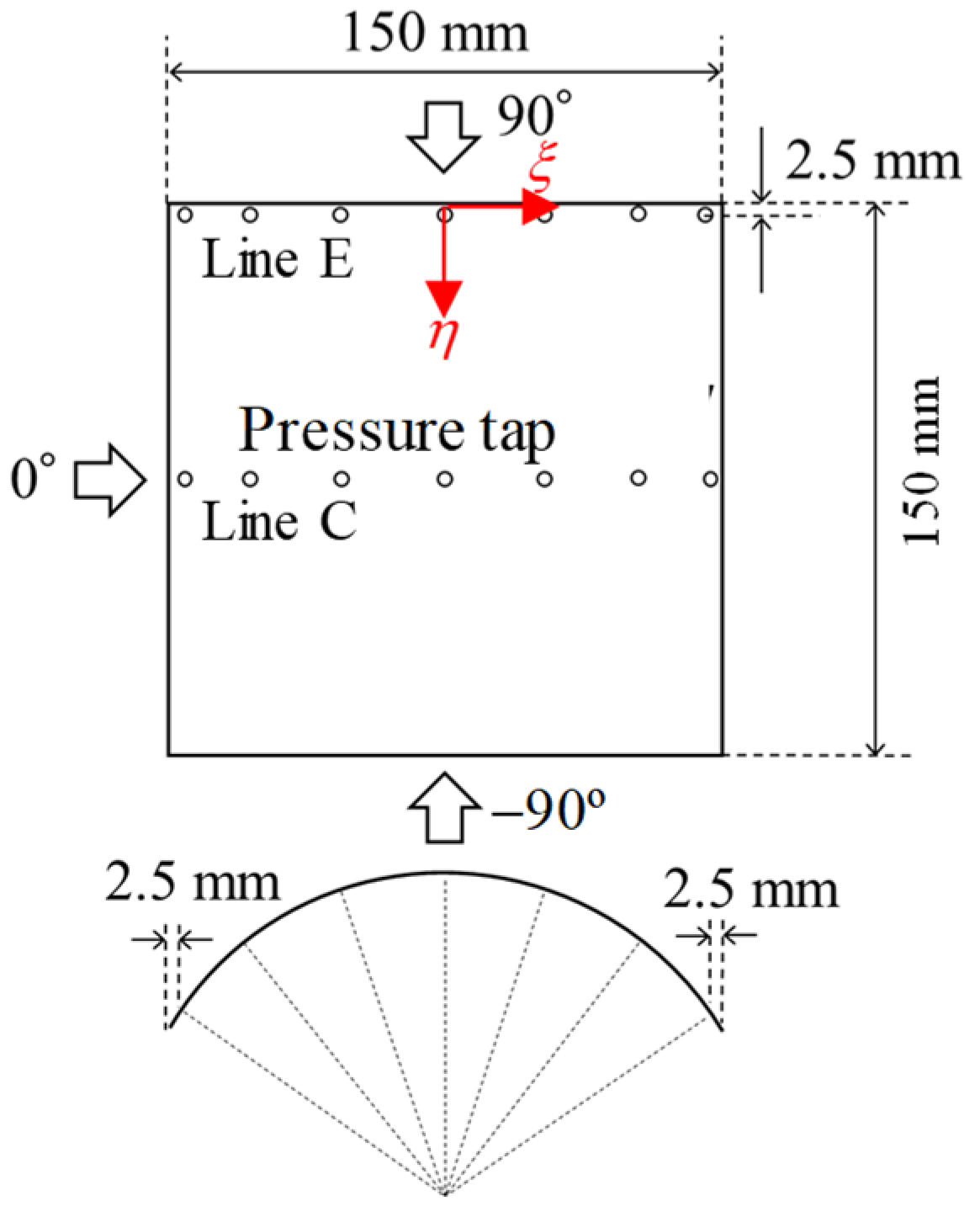
2.4. Experimental Procedure of Pressure Measurements
3. CFD Simulation
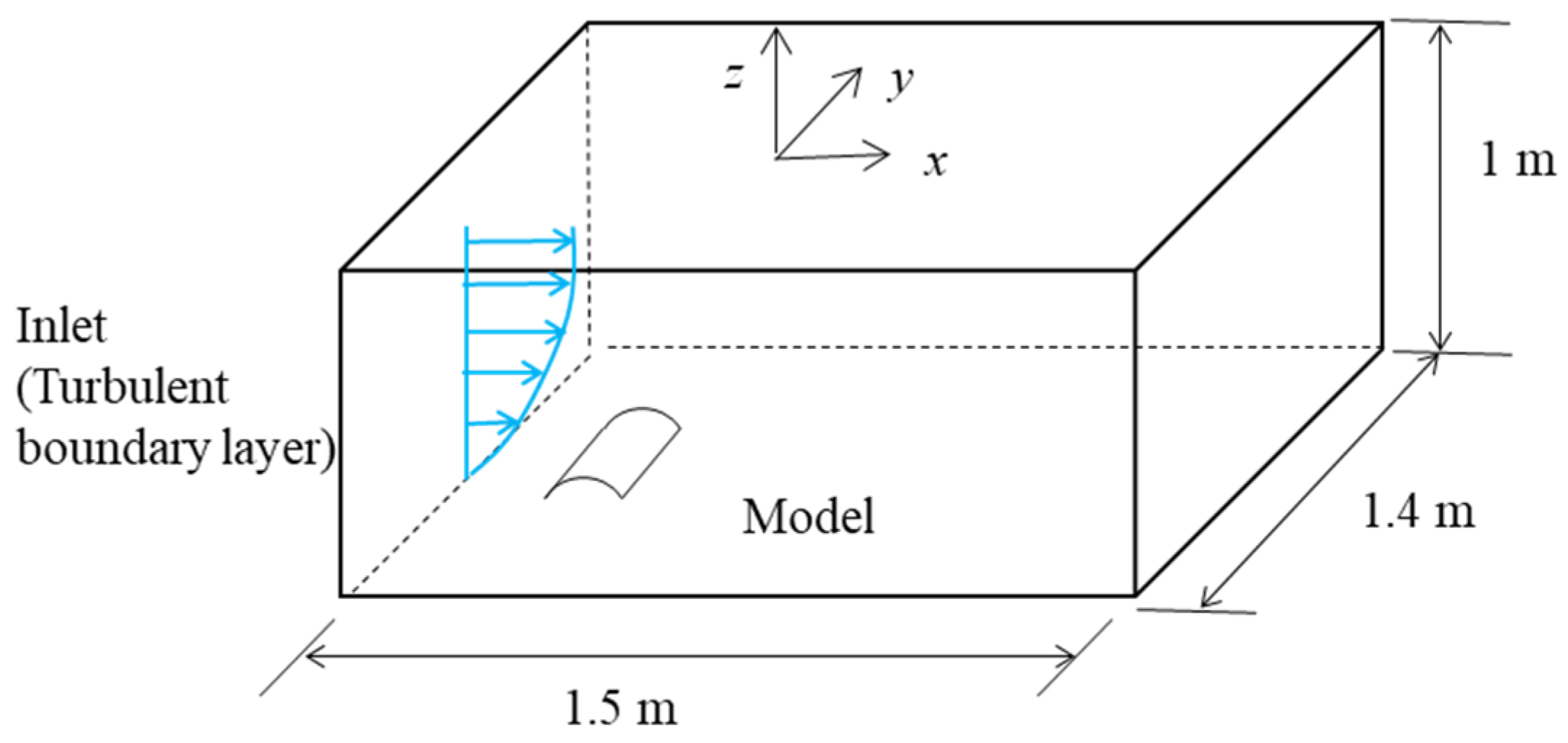
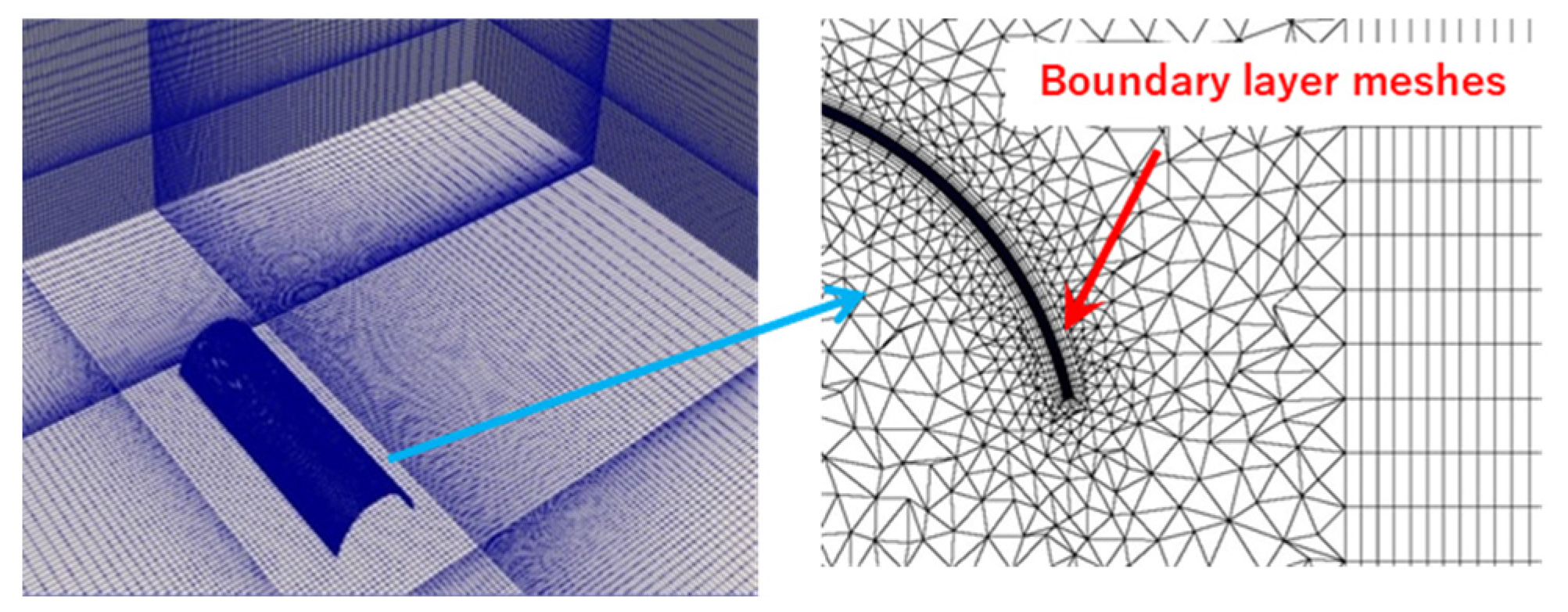
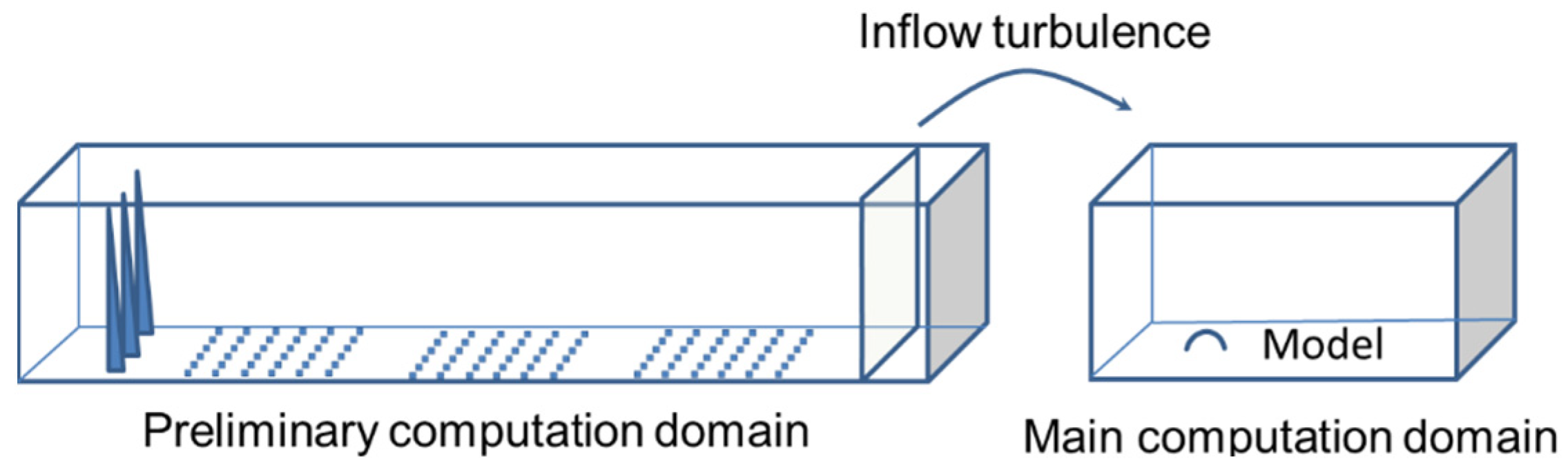
3.1. Computational Model and Domain
3.2. Computational and Boundary Conditions
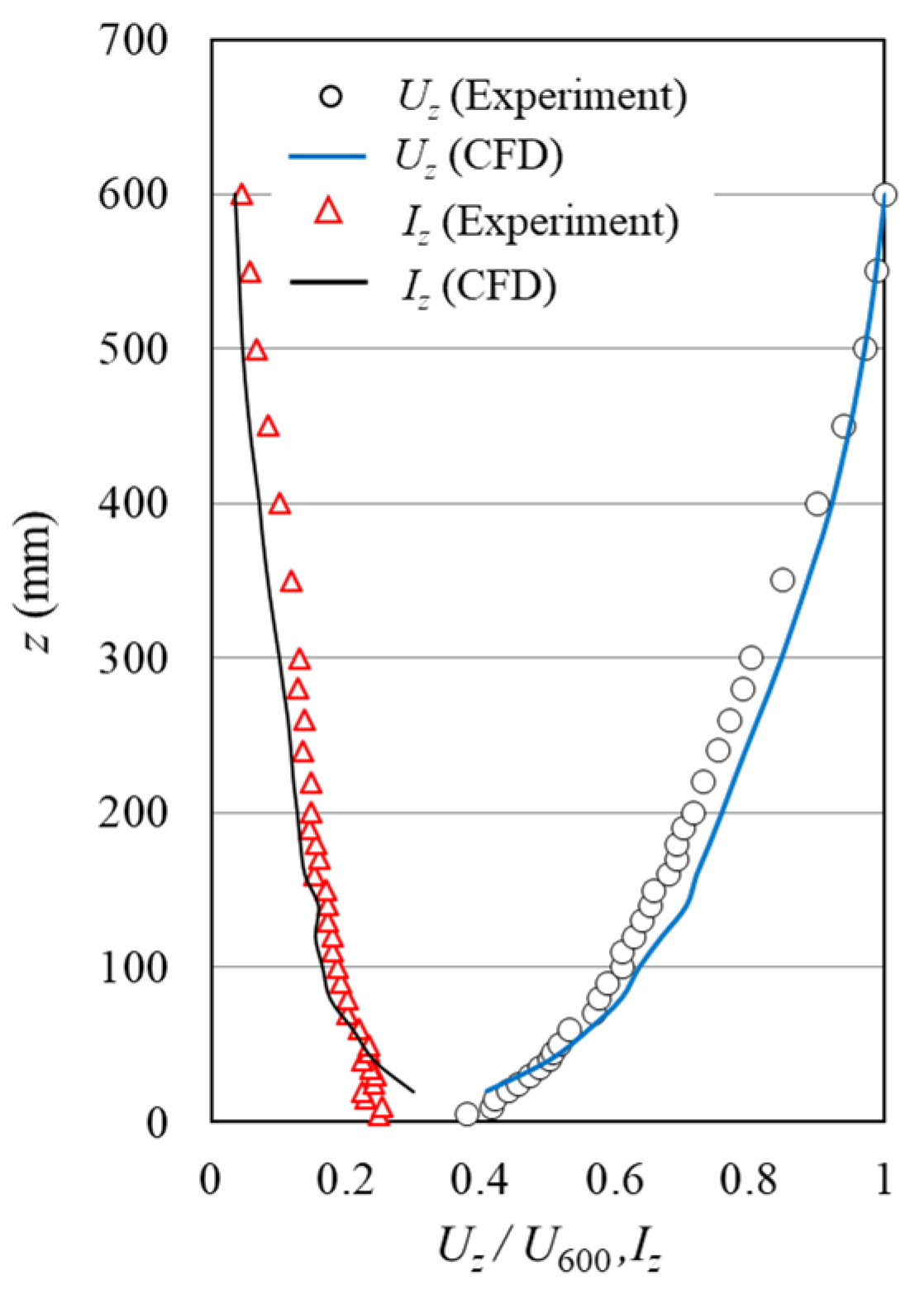
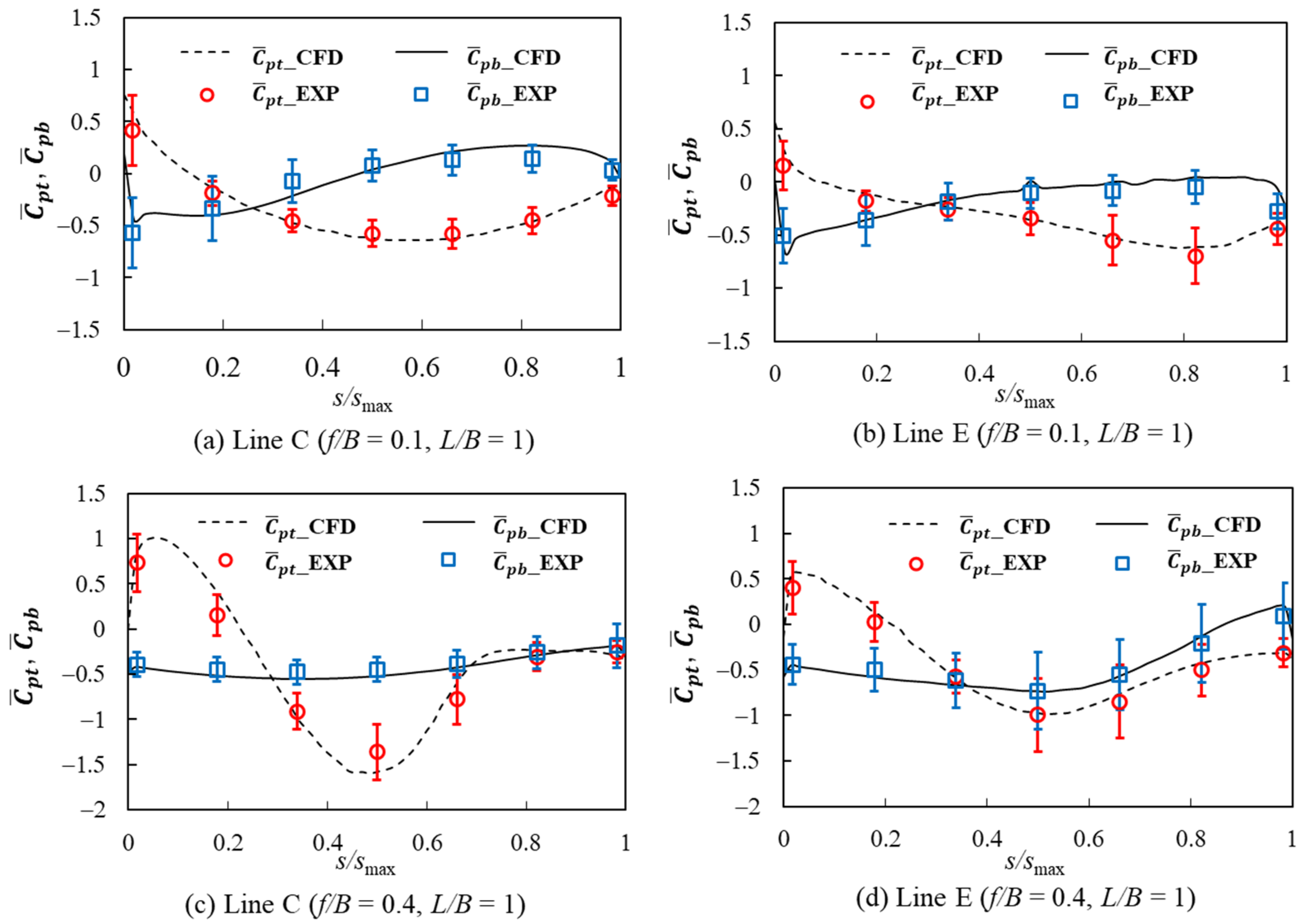
3.3. Validation of the CFD Analysis
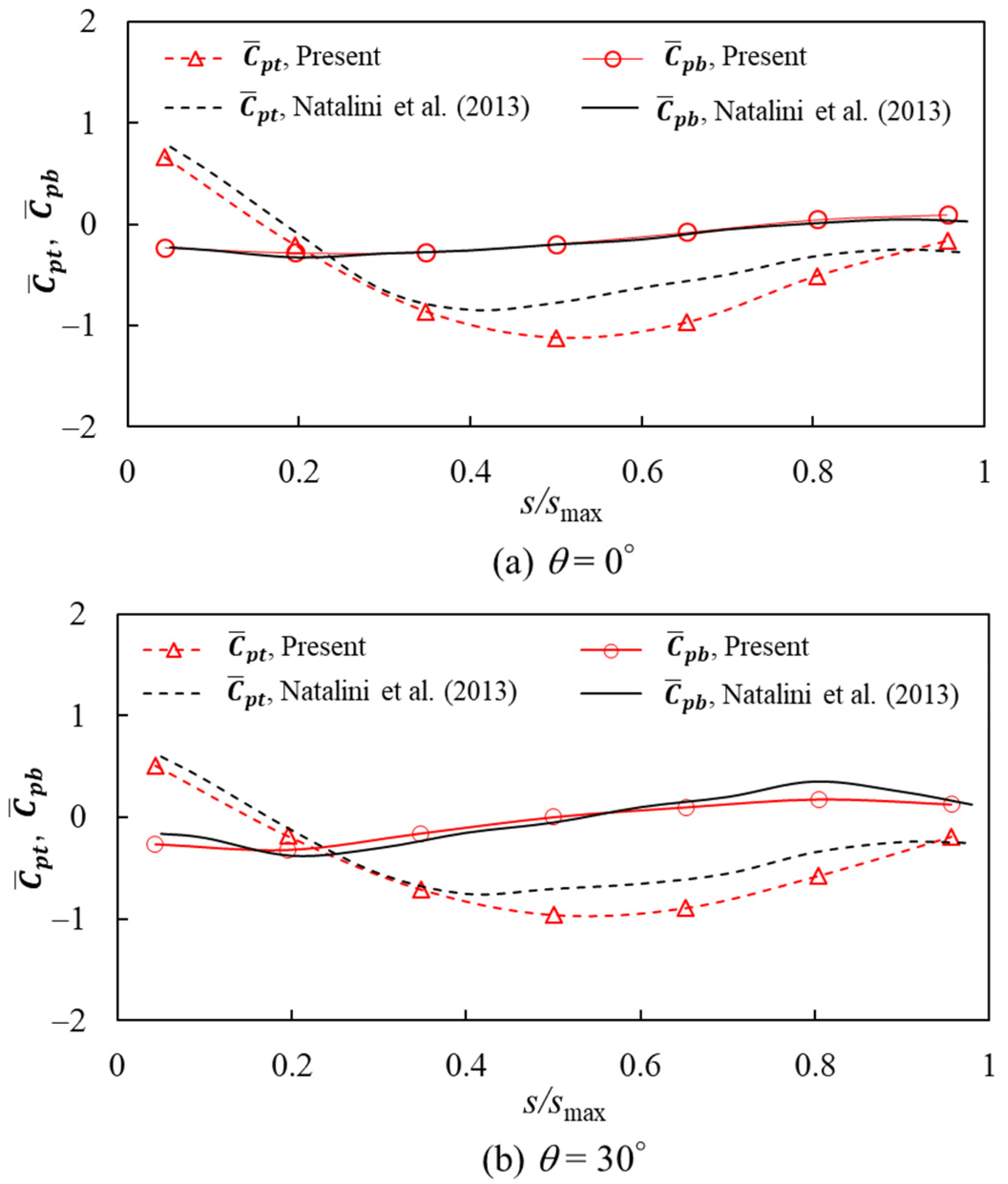
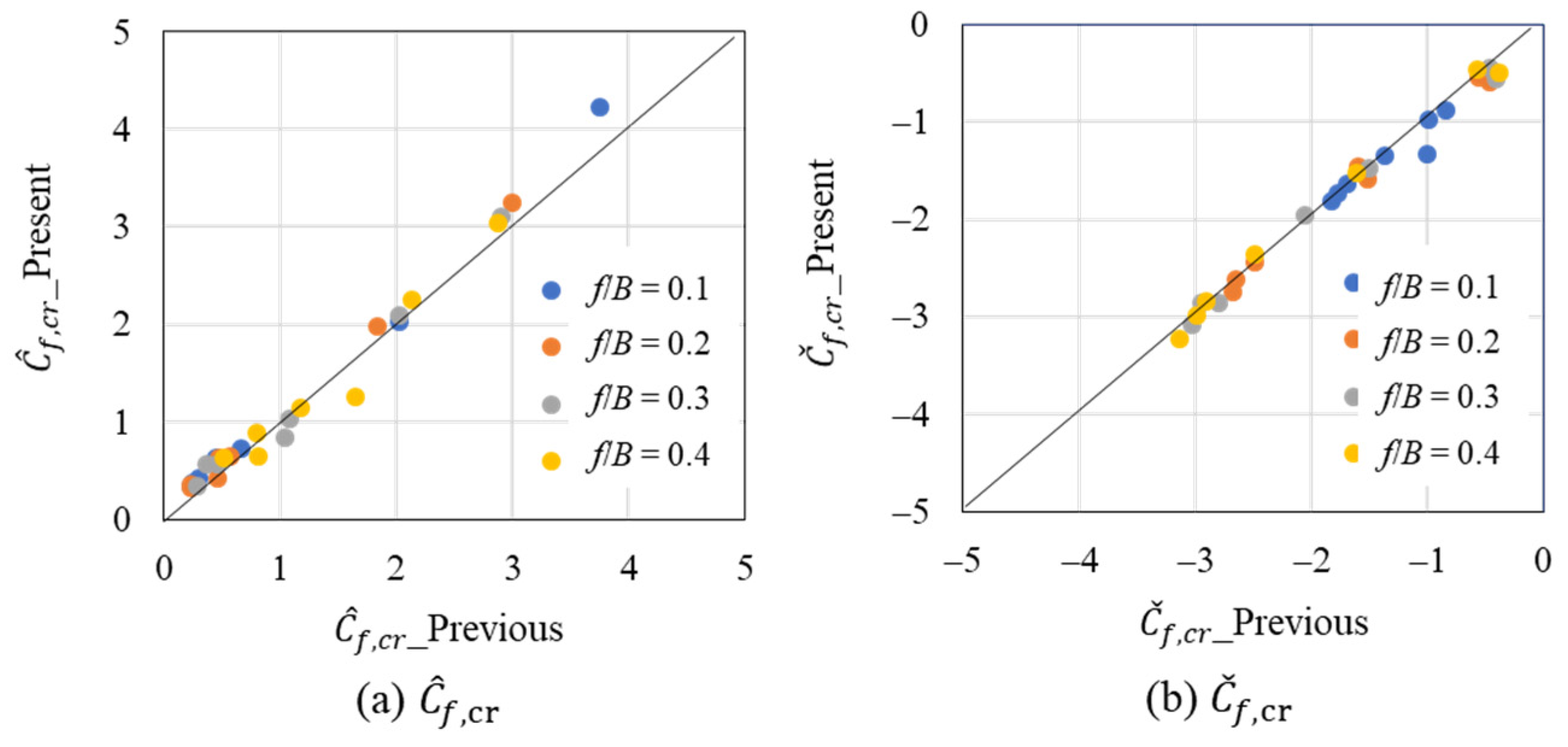
4. Comparison with Previous Results
5. Results and Discussion
5.1. Mean Wind Pressure and Force Coefficients
5.1.1. General Features
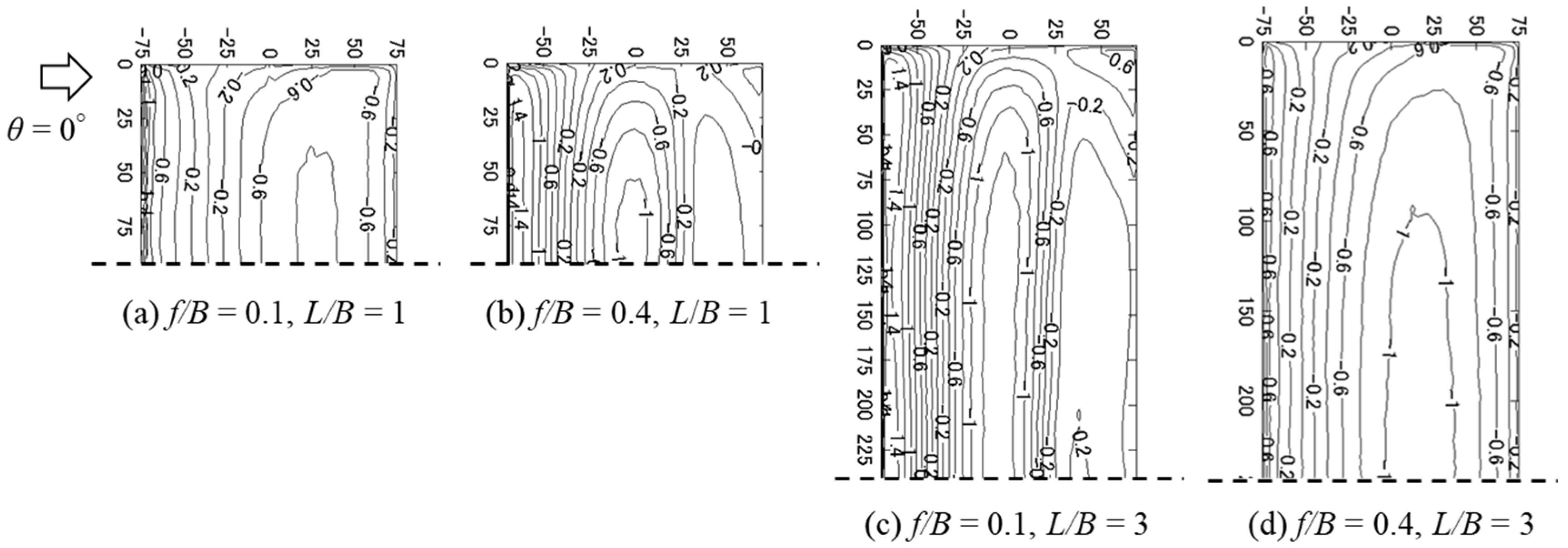
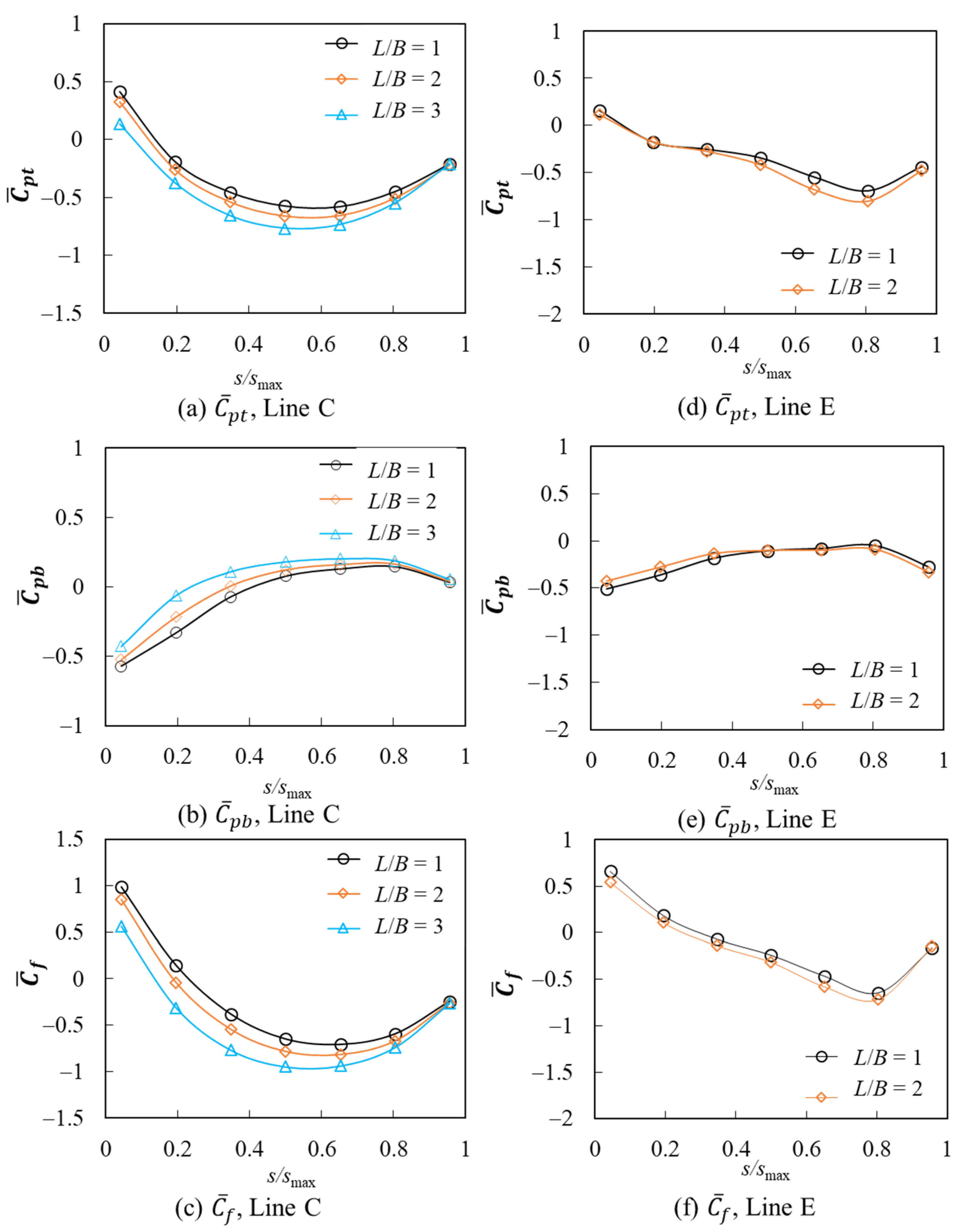
5.1.2. Effects of f/B and L/B on the Mean Wind Pressure and Force Coefficients
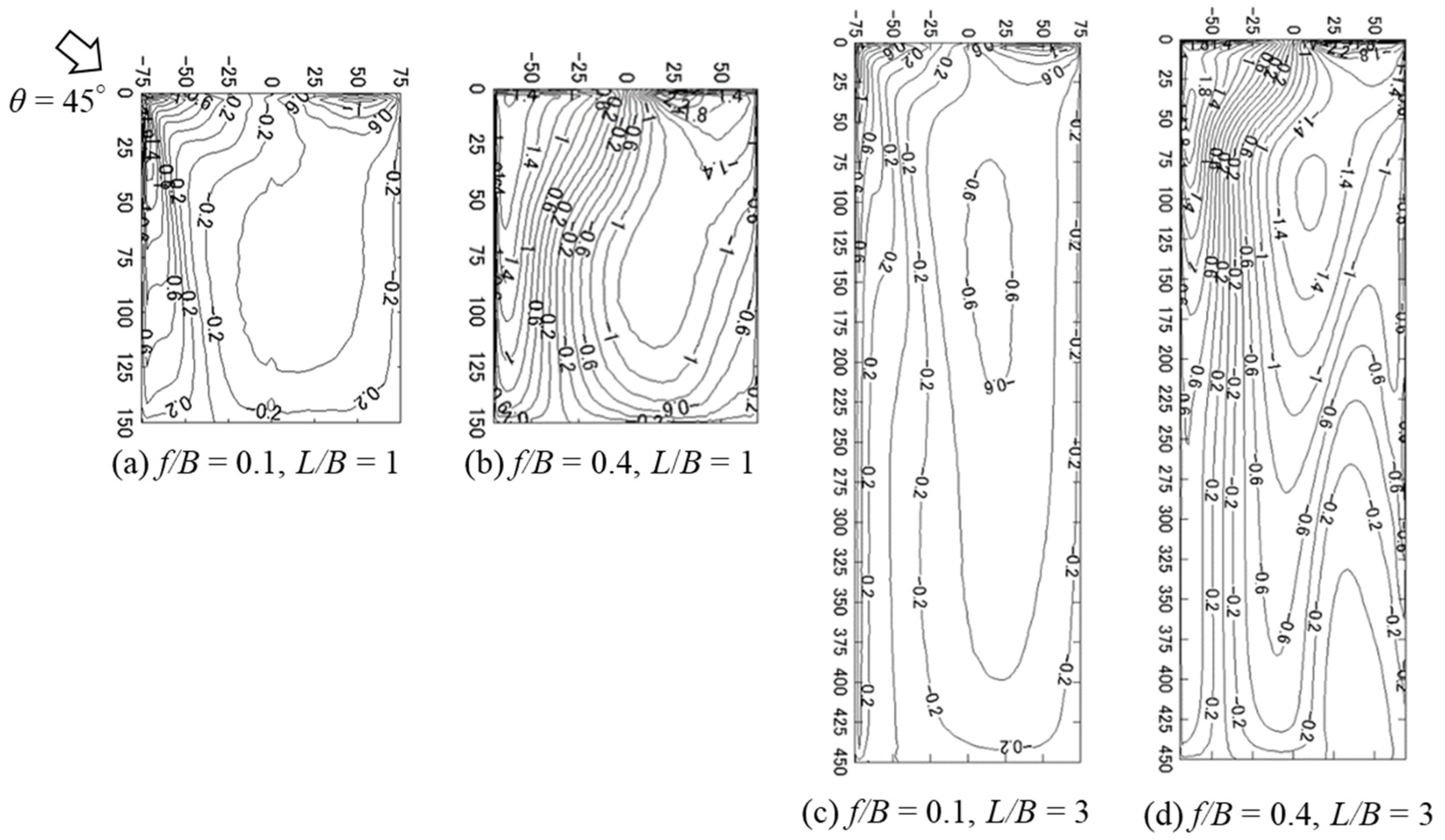
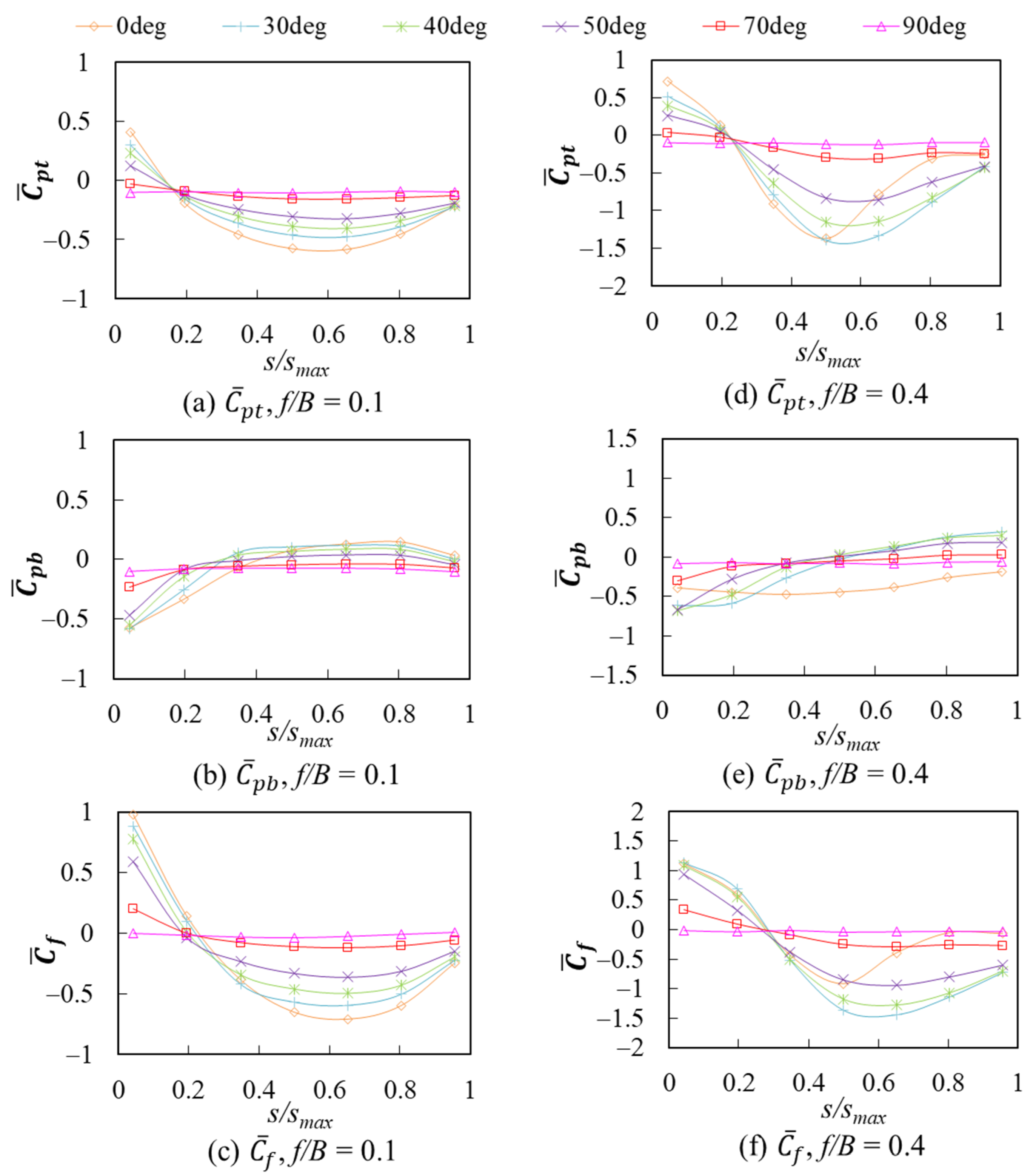
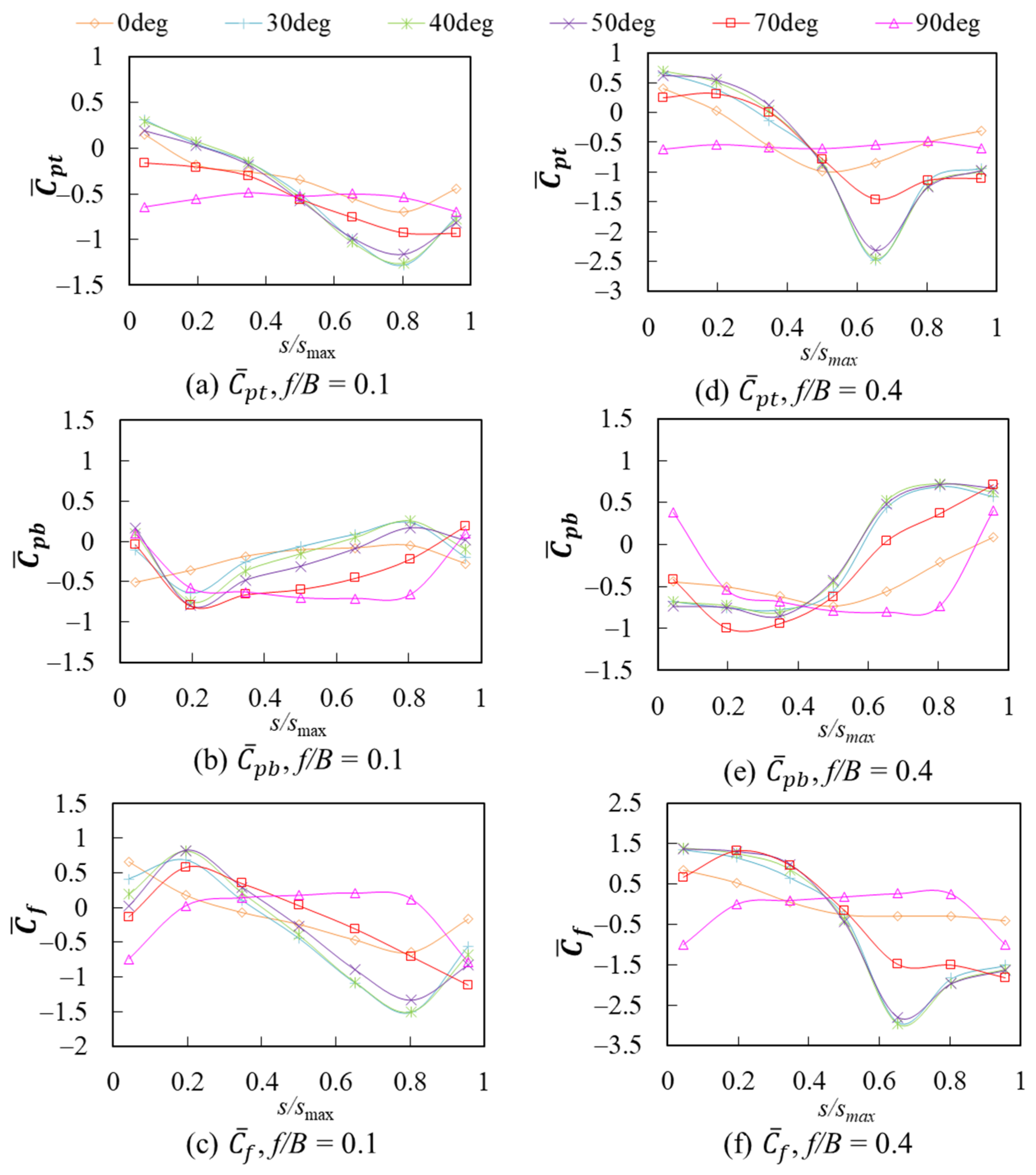
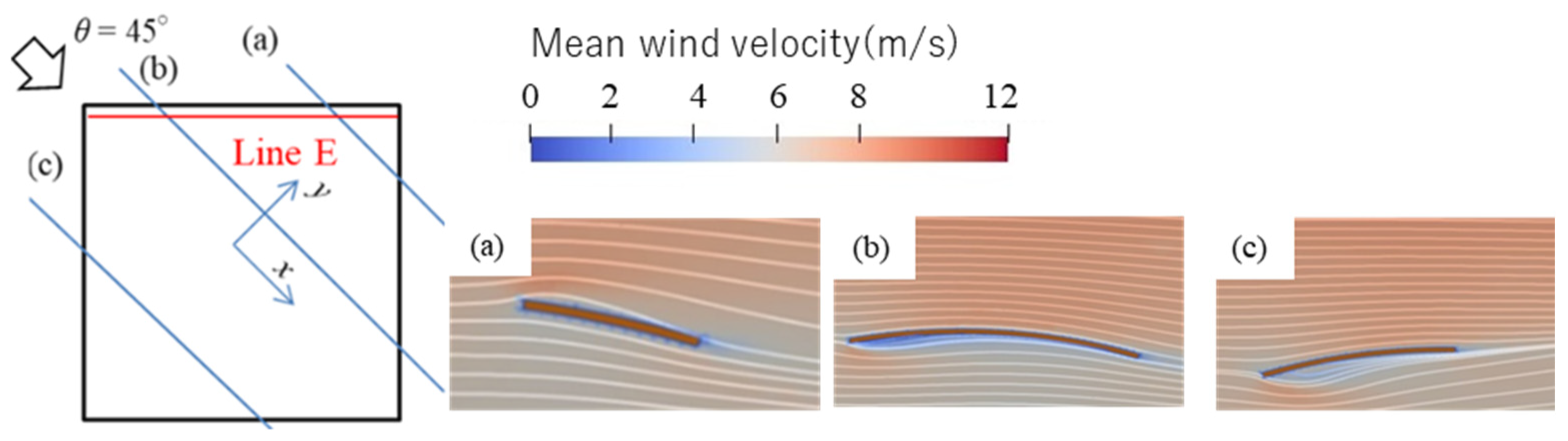
5.1.3. Effect of Wind Direction θ on the Mean Wind Pressure and Wind Force Coefficients
5.1.4. Empirical Formulas for the Mean Wind Force Coefficient Distributions
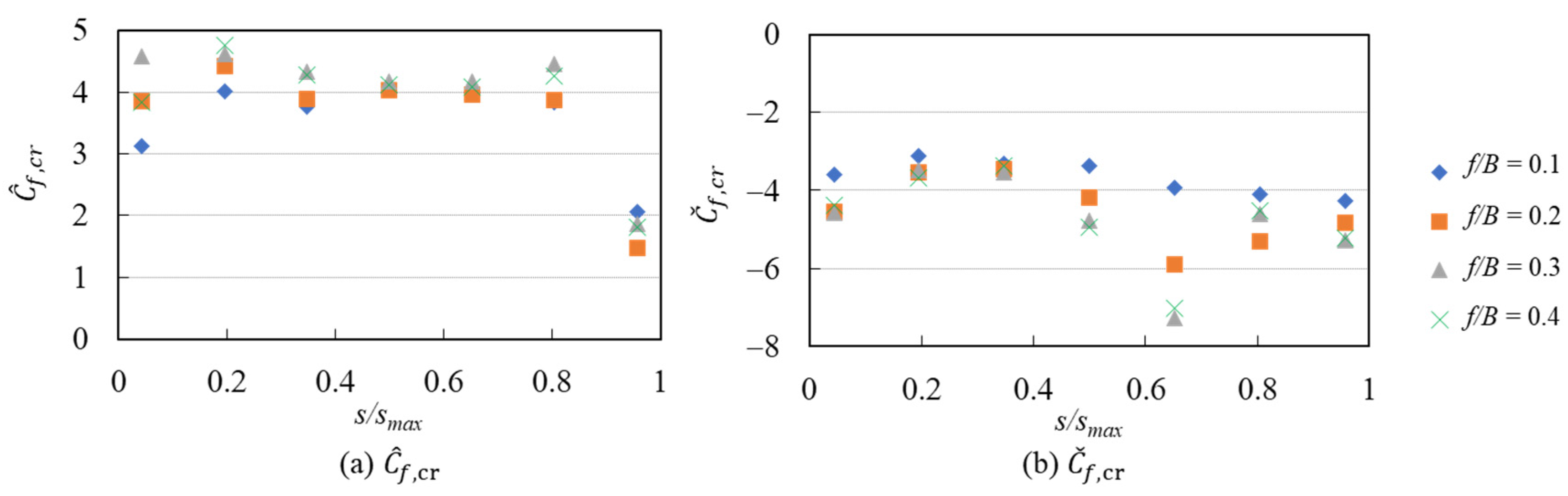
5.2. Maximum and Minimum Peak Wind Force Coefficients
5.2.1. General Features
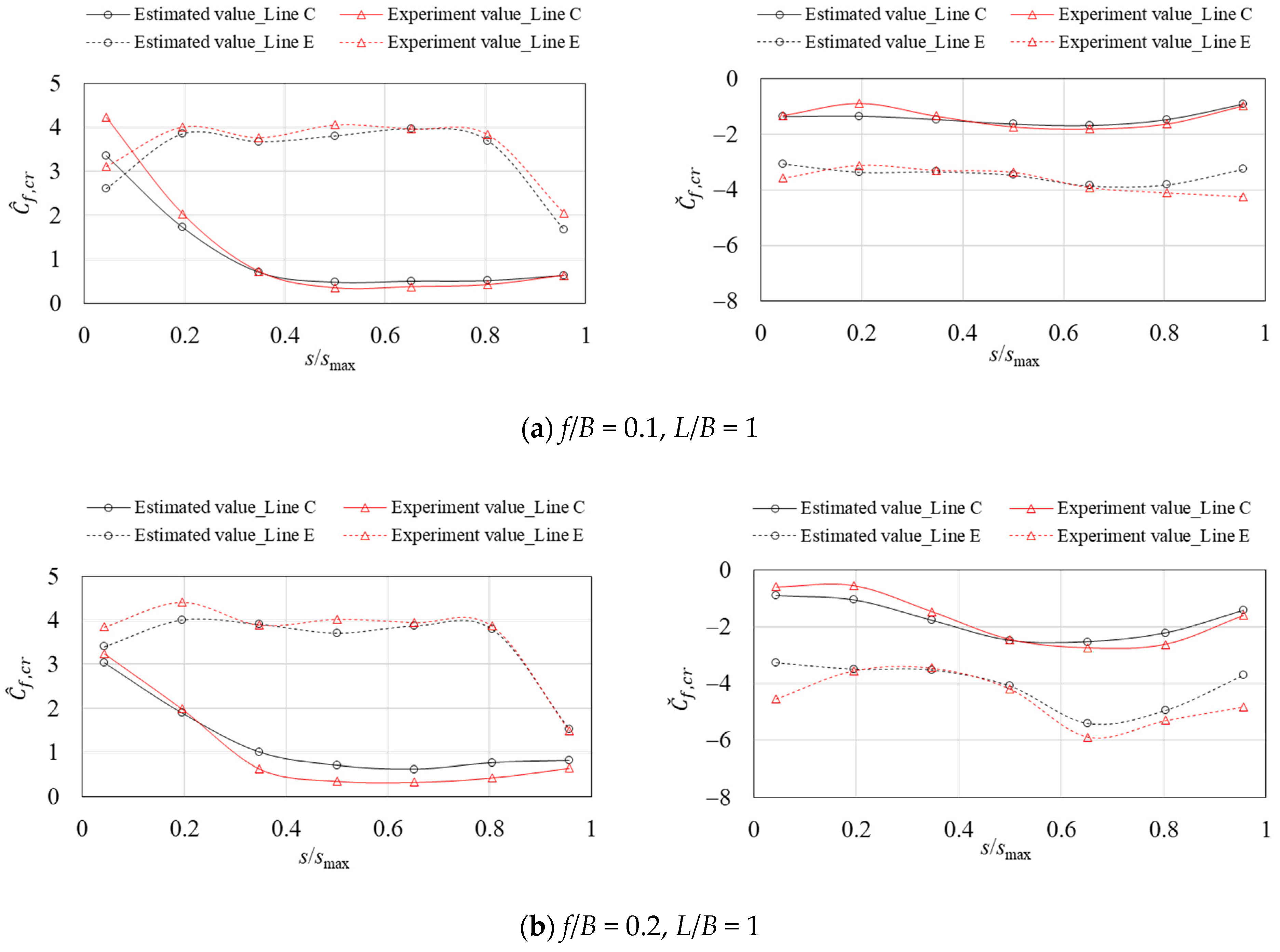
5.2.2. Estimation of the Maximum and Minimum Peak Wind Force Coefficients Based on a Peak Factor Approach
6. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Qiu, Y.; Sun, Y.; Wu, Y.; Tamura, Y. Modeling the mean wind loads on cylindrical roofs with consideration of the Reynolds number effect in uniform flow with low turbulence. J. Wind. Eng. Ind. Aerodyn. 2014, 129, 11–21. [Google Scholar] [CrossRef]
- Natalini, B.; Natalini, M.B. Wind loads on buildings with vaulted roofs and side walls—A review. J. Wind Eng. Ind. Aerodyn. 2017, 161, 9–16. [Google Scholar] [CrossRef]
- Xu, Y.; Lyu, X.; Song, H.; Lin, B.; Wei, M.; Yin, Y.; Wang, S. Large-span M-shaped greenhouse with superior wind resistance and ventilation performance. J. Wind Eng. Ind. Aerodyn. 2023, 238, 105410. [Google Scholar] [CrossRef]
- ASCE/SEI 7-22; Minimum Design Loads and Associated Criteria for Buildings and Other Structures. American Society of Civil Engineers: Reston, VA, USA, 2022.
- AS/NZS 1170.2; Standards New Zealand, Standards Australia. Australia/New Zealand Standard. 2021. Available online: https://www.standards.govt.nz/shop/asnzs-1170-22021/ (accessed on 30 May 2023).
- Recommendations of Loads on Buildings; Architectural Institute of Japan: Tokyo, Japan, 2015; Available online: https://www.aij.or.jp/jpn/ppv/pdf/aij_recommendations_for_loads_on_buildings_2015.pdf (accessed on 30 May 2023).
- Tian, Y.; Guan, H.; Shao, S.; Yang, Q. Provisions and comparison of Chinese wind load standard for roof components and cladding. Structures 2021, 33, 2587–2598. [Google Scholar] [CrossRef]
- Natalini, B.; Marighetti, J.O.; Natalini, M.B. Wind tunnel modeling of mean pressures on planar canopy roof. J. Wind Eng. Ind. Aerodyn. 2002, 90, 427–439. [Google Scholar] [CrossRef]
- Colliers, J.; Bollaert, M.; Degroote, J.; Laet, L.D. Prototyping of thin shell wind tunnel models to facilitate experimental wind load analysis on curved canopy structures. J. Wind Eng. Ind. Aerodyn. 2019, 188, 308–322. [Google Scholar] [CrossRef]
- Sun, X.; Yu, R.; Wu, Y. Investigation on wind tunnel experiments of ridge-valley tensile membrane structures. Eng. Struct. 2019, 187, 104371. [Google Scholar] [CrossRef]
- Sun, X.; Arjun, K.; Wu, Y. Investigation on wind tunnel experiment of oval-shaped arch-supported membrane structures. J. Wind Eng. Ind. Aerodyn. 2020, 206, 104371. [Google Scholar] [CrossRef]
- Kandel, A.; Sun, X.; Wu, Y. Wind-induced responses and equivalent static design method of oval-shaped arch-supported membrane structure. J. Wind Eng. Ind. Aerodyn. 2021, 213, 104620. [Google Scholar] [CrossRef]
- Ding, W.; Wen, L.; Uematsu, Y. Fundamental study of wind loads on domed free roofs. J. Membr. Struct. 2022, 2, 11–19. (In Japanese) [Google Scholar]
- Su, N.; Peng, S.; Hong, N.; Hu, T. Wind tunnel investigation on the wind load of large-span coal sheds with porous gables: Influence of gable ventilation. J. Wind Eng. Ind. Aerodyn. 2020, 204, 104242. [Google Scholar] [CrossRef]
- Natalini, M.B.; Morel, C.; Natalini, B. Mean loads on vaulted canopy roofs. J. Wind Eng. Ind. Aerodyn. 2013, 119, 102–113. [Google Scholar] [CrossRef]
- Uematsu, Y.; Yamamura, R. Wind loads for designing the main wind force resisting systems of cylindrical free-standing canopy roofs, Technical Transactions. Civ. Eng. 2019, 116, 125–143. [Google Scholar]
- Pagnini, L.; Torre, S.; Freda, A.; Piccardo, G. Wind pressure measurements on a vaulted canopy roof. J. Wind Eng. Ind. Aerodyn. 2022, 223, 104934. [Google Scholar] [CrossRef]
- Ding, W.; Uematsu, Y. Discussion of design wind loads on a vaulted free roof. Wind 2022, 2, 479–494. [Google Scholar] [CrossRef]
- Kasperski, M. Extreme wind load distributions for linear and non-linear design. Eng. Struct. 1992, 14, 27–34. [Google Scholar] [CrossRef]
- Tieleman, H.W.; Reinhold, T.A.; Marshall, R.D. On the wind-tunnel simulation of the atmospheric surface layer for the study of wind loads on low-rise buildings. J. Wind Eng. Ind. Aerodyn. 1978, 3, 21–38. [Google Scholar] [CrossRef]
- Tieleman, H.W. Pressures on surface-mounted prisms: The effects of incident turbulence. J. Wind Eng. Ind. Aerodyn. 1993, 49, 289–299. [Google Scholar] [CrossRef]
- Tieleman, H.W.; Hajj, M.R.; Reinhold, T.A. Wind tunnel simulation requirements to assess wind loads on low-rise buildings. J. Wind Eng. Ind. Aerodyn. 1998, 74–76, 675–686. [Google Scholar] [CrossRef]
- ASCE/SEI 49-12; Wind Tunnel Testing for Buildings and Other Structures. American Society of Civil Engineers: Reston, VA, USA, 2012.
- Macdonald, P.A.; Kwok, K.C.S.; Holmes, J.D. Wind loads on circular storage bins, silos and tanks: I. Point pressure measurements on isolated structures. J. Wind Eng. Ind. Aerodyn. 1988, 31, 165–188. [Google Scholar] [CrossRef]
- Liu, L.; Sun, Y.; Su, N.; Wu, Y.; Peng, S. Three-dimensional Reynolds number effects and wind load models for cylindrical storage tanks with low aspect ratios. J. Wind Eng. Ind. Aerodyn. 2022, 227, 105080. [Google Scholar] [CrossRef]
- Smagorinsky, J. General circulation experiments with the primitive equations: I. The basic experiment. Mon. Weather Rev. 1963, 91, 99–164. [Google Scholar] [CrossRef]
- Yang, Q.S.; Wang, T.F.; Yan, B.W.; Li, T.; Liu, M. Nonlinear motion-induced aerodynamic forces on large hyperbolic paraboloid roofs using LES. J. Wind Eng. Ind. Aerodyn. 2021, 216, 104703. [Google Scholar] [CrossRef]
- Yang, Q.S.; Chen, F.X.; Yan, B.W.; Li, T.; Yan, J.H. Effects of free-stream turbulence on motion-induced aerodynamic forces of a long-span flexible flat roof using LES simulations. J. Wind Eng. Ind. Aerodyn. 2022, 231, 105236. [Google Scholar] [CrossRef]
- Takadate, Y.; Uematsu, Y. Steady and unsteady aerodynamic forces on a long-span membrane structure. J. Wind Eng. Ind. Aerodyn. 2019, 193, 103946. [Google Scholar] [CrossRef]




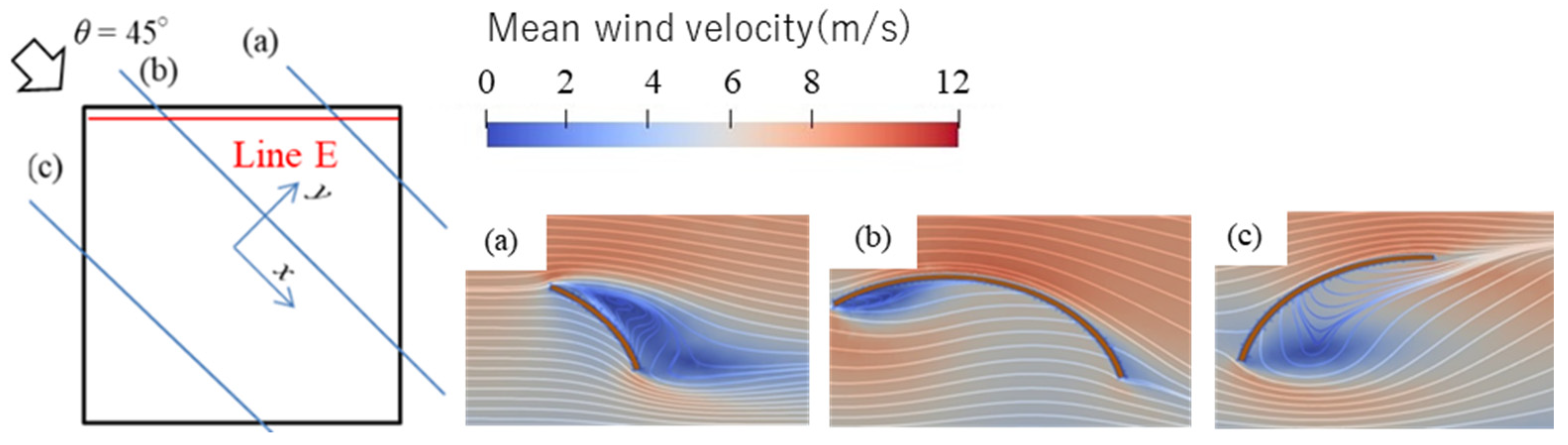

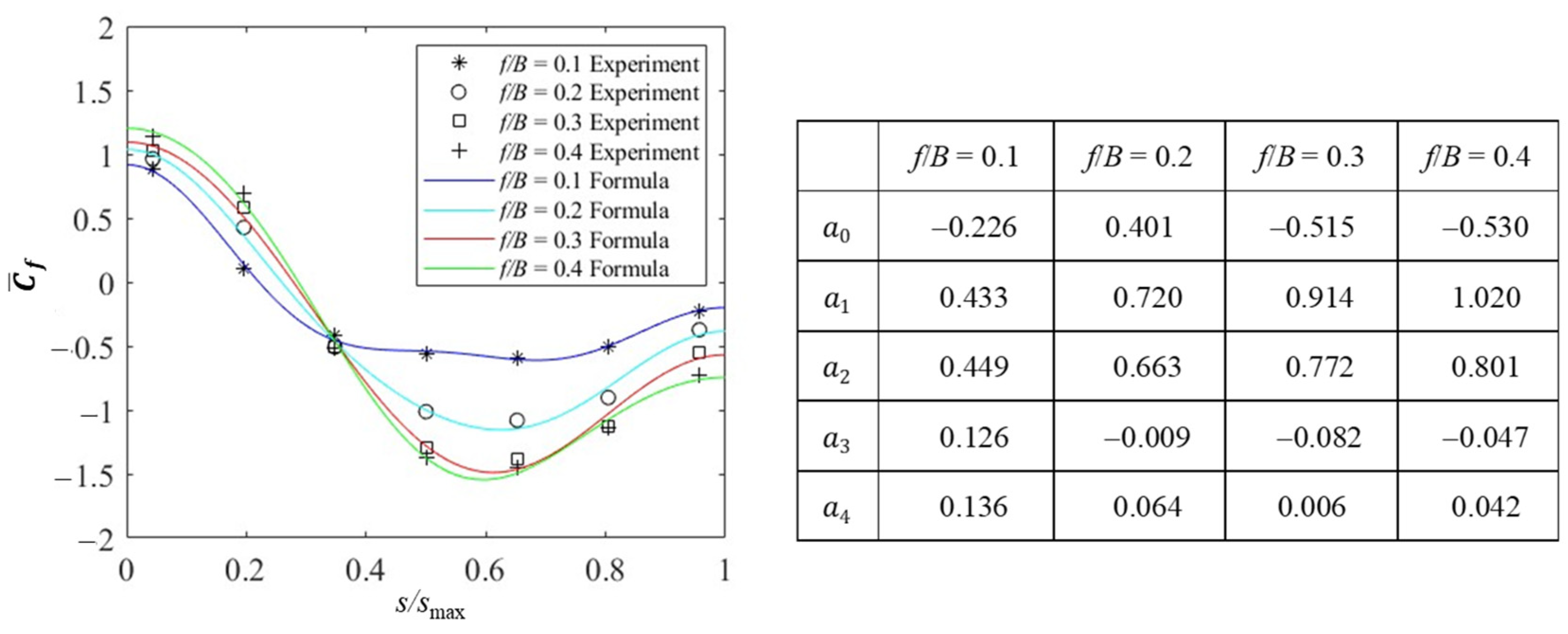
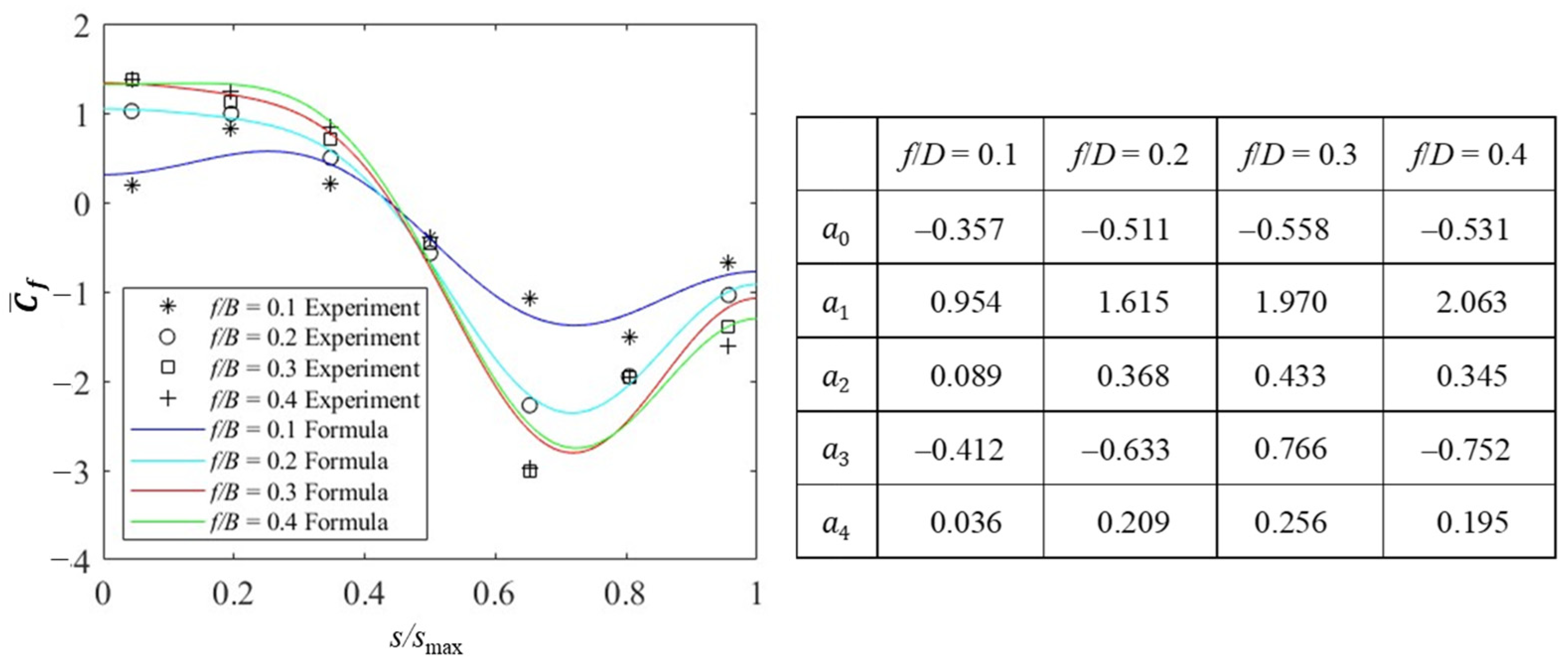
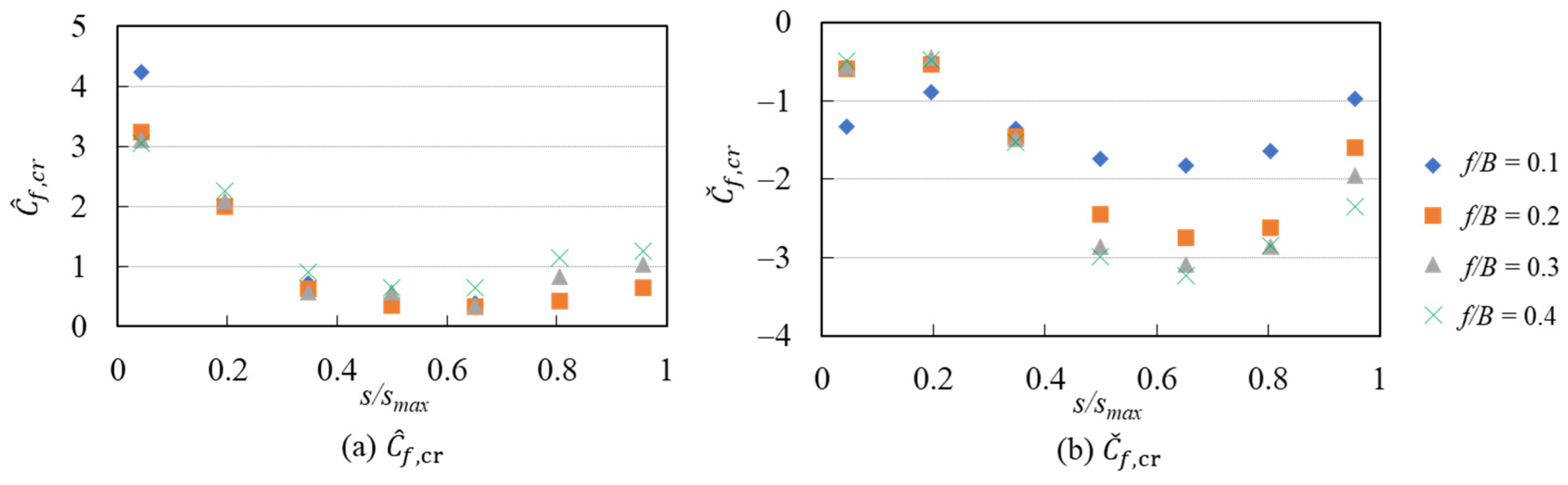


| f/B | L/B | f (m) | B (m) | L (m) | htop (m) |
|---|---|---|---|---|---|
| 0.1 | 1, 2, 3 | 1.5 | 15 | 15, 30, 45 | 8.8 |
| 0.2 | 1, 2, 3 | 3.0 | 15 | 15, 30, 45 | 9.5 |
| 0.3 | 1, 2, 3 | 4.5 | 15 | 15, 30, 45 | 10.3 |
| 0.4 | 1, 2, 3 | 6.0 | 15 | 15, 30, 45 | 11.0 |
| Inlet boundary | Inflow turbulence (Sampling data from preliminary computation) |
| Outlet boundary | Advective outflow condition |
| Side and top boundaries | Free-slip condition |
| Surfaces of ground and roof | No-slip condition |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, W.; Uematsu, Y.; Wen, L. Fundamental Characteristics of Wind Loading on Vaulted-Free Roofs. Wind 2023, 3, 394-417. https://doi.org/10.3390/wind3040023
Ding W, Uematsu Y, Wen L. Fundamental Characteristics of Wind Loading on Vaulted-Free Roofs. Wind. 2023; 3(4):394-417. https://doi.org/10.3390/wind3040023
Chicago/Turabian StyleDing, Wei, Yasushi Uematsu, and Lizhi Wen. 2023. "Fundamental Characteristics of Wind Loading on Vaulted-Free Roofs" Wind 3, no. 4: 394-417. https://doi.org/10.3390/wind3040023
APA StyleDing, W., Uematsu, Y., & Wen, L. (2023). Fundamental Characteristics of Wind Loading on Vaulted-Free Roofs. Wind, 3(4), 394-417. https://doi.org/10.3390/wind3040023








