On Cosmological Inflation in Palatini F(R,ϕ) Gravity †
Abstract
1. Introduction
2. Overview of Gravity within Palatini Formalism
3. Single-Field Inflation
3.1. Various Limitations of Action 9
- Limit I:This limit corresponds to the slow-roll inflationary paradigm with a canonical scalar field . The action represents the model in this limit is given by:where is re-scaled field defined as:The slow-roll parameters and , in addition to the observables r and , are summarized in Table 1.
- Limit II:Considering this limit, the model appears as a K-inflationary scenario. the action representing this situation is given by:Now the re-scaled field is given as:Table 1 summarizes the model’s main characteristics and observable formulas of this limit.
- Limit III:Under the above limit, the model has the following action:where the re-scaled is given by the Formula (12). Additionally, we treat the model at this limit according to the constant-roll scenario.Again, the primary model’s characteristics are summarized in Table 1.
3.2. Case Study: Cosine and Exponential Potentials
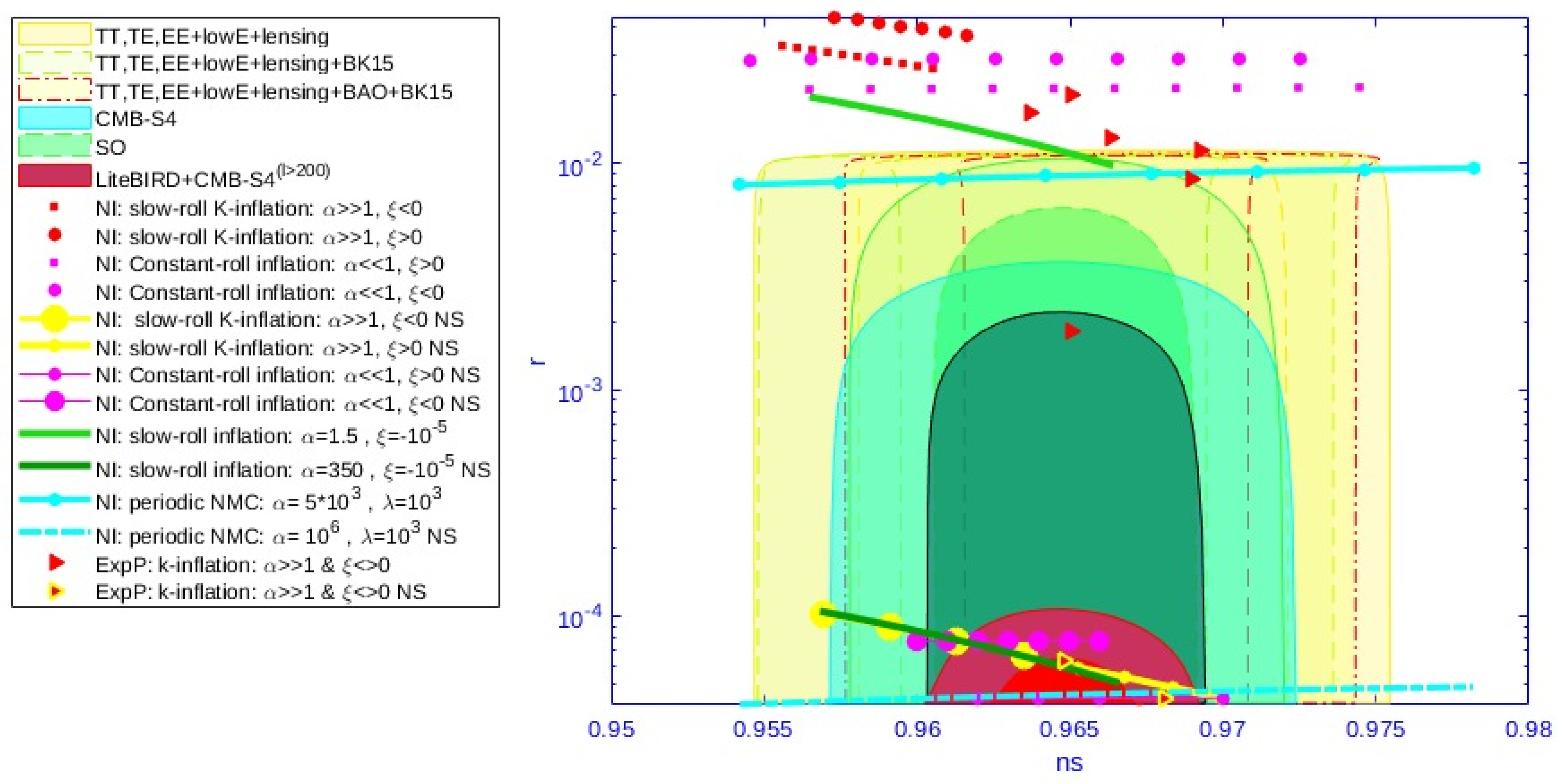
4. Results
5. Discussion and Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Guth, A.H. The Inflationary Universe: A Possible Solution to the Horizon and Flatness Problems. Phys. Rev. D 1981, 23, 347–356. [Google Scholar] [CrossRef]
- Sotiriou, T.P.; Faraoni, V. Unified cosmic history in modified gravity: From F (R) theory to Lorentz non-invariant models. Rev. Mod. Phys. 2010, 82, 451–497. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D. Unified cosmic history in modified gravity: From F(R) theory to Lorentz non-invariant models. Phys. Rept. 2011, 505, 59–144. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D.; Oikonomou, V.K. Modified Gravity Theories on a Nutshell: Inflation, Bounce and Late-time Evolution. Phys. Rept. 2017, 692, 1–104. [Google Scholar] [CrossRef]
- Myrzakulov, R.; Sebastiani, L.; Vagnozzi, S. Inflation in f(R,ϕ) -theories and mimetic gravity scenario. Eur. Phys. J. C 2015, 75, 444. [Google Scholar] [CrossRef]
- Enckell, V.M.; Enqvist, K.; Rasanen, S.; Wahlman, L.P. Inflation with R2 term in the Palatini formalism. JCAP 2019, 2, 022. [Google Scholar] [CrossRef]
- Ade, P.A.; Ahmed, Z.; Amiri, M.; Barkats, D.; Thakur, R.B.; Bischoff, C.A.; Beck, D.; Bock, J.J.; Boenish, H.; Bullock, E.; et al. Improved Constraints on Primordial Gravitational Waves using Planck, WMAP, and BICEP/Keck Observations through the 2018 Observing Season. Phys. Rev. Lett. 2021, 127, 151301. [Google Scholar] [CrossRef] [PubMed]
- AlHallak, M.; AlRakik, A.; Chamoun, N.; El-Daher, M.S. Palatini f(R) Gravity and Variants of k-/Constant Roll/Warm Inflation within Variation of Strong Coupling Scenario. Universe 2022, 8, 126. [Google Scholar] [CrossRef]
- AlHallak, M.; Chamoun, N.; Eldaher, M.S. Natural Inflation with non minimal coupling to gravity in R2 gravity under the Palatini formalism. JCAP 2022, 10, 001. [Google Scholar] [CrossRef]
- Freese, K.; Frieman, J.A.; Olinto, A.V. Natural inflation with pseudo—Nambu-Goldstone bosons. Phys. Rev. Lett. 1990, 65, 3233–3236. [Google Scholar] [CrossRef] [PubMed]
- Chernikov, N.A.; Tagirov, E.A. Quantum theory of scalar field in de Sitter space-time. In Annales de l’IHP Physique Théorique; Joint Inst. for Nuclear Research: Dubna, Russia, 1968; pp. 109–141, Section A, Tome 9. [Google Scholar]
- Reyimuaji, Y.; Zhang, X. Natural inflation with a nonminimal coupling to gravity. JCAP 2021, 3, 059. [Google Scholar] [CrossRef]
- Ferreira, R.Z.; Notari, A.; Simeon, G. Natural Inflation with a periodic non-minimal coupling. JCAP 2018, 11, 021. [Google Scholar] [CrossRef]
- AlHallak, M.; Chamoun, N. Realization of Power-Law Inflation & Variants via Variation of the Strong Coupling Constant. JCAP 2016, 9, 006. [Google Scholar] [CrossRef]
- Chamoun, N.; Landau, S.J.; Vucetich, H. On Inflation and Variation of the Strong Coupling Constant. Int. J. Mod. Phys. D 2007, 16, 1043–1052. [Google Scholar] [CrossRef]
- AlHallak, M.; Rakik, A.A.; Bitar, S.; Chamoun, N.; Eldaher, M.S. Inflation by variation of the strong coupling constant: Update for Planck 2018. Int. J. Mod. Phys. A 2021, 36, 2150226. [Google Scholar] [CrossRef]
- Bahr-Kalus, B.; Parkinson, D.; Easther, R. Constraining Cosmic Inflation with Observations: Prospects for 2030. arXiv 2022, arXiv:2212.04115. [Google Scholar] [CrossRef]
| Inflationary Scenario | Slow-Roll Canonical Inflation | Slow-Roll K-Inflation | Constant-Roll K-Inflation |
|---|---|---|---|
| Model Characteristics | Limit 1 | Limit 2 | Limit 3 |
| r |
| Case I | Case II | Case III | |
|---|---|---|---|
| Potential: | |||
| NMC term: |
| Case | ||||||
|---|---|---|---|---|---|---|
| Limit I, Case I | ||||||
| Slow-roll NI | 350 | |||||
| Limit I, Case II | 1000 | 1000 | 1000 | |||
| Slow-roll NI | 1000 | 1000 | 1000 | |||
| Limit II, Case I | 250 | |||||
| K-inflation NI | 21,000 | |||||
| Limit II, Case III | 500 | |||||
| K-inflation ExP | ||||||
| Limit III, Case I | 10 | |||||
| Constant-roll NI | 10 | |||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
AlHallak, M. On Cosmological Inflation in Palatini F(R,ϕ) Gravity. Phys. Sci. Forum 2023, 7, 35. https://doi.org/10.3390/ECU2023-14048
AlHallak M. On Cosmological Inflation in Palatini F(R,ϕ) Gravity. Physical Sciences Forum. 2023; 7(1):35. https://doi.org/10.3390/ECU2023-14048
Chicago/Turabian StyleAlHallak, Mahmoud. 2023. "On Cosmological Inflation in Palatini F(R,ϕ) Gravity" Physical Sciences Forum 7, no. 1: 35. https://doi.org/10.3390/ECU2023-14048
APA StyleAlHallak, M. (2023). On Cosmological Inflation in Palatini F(R,ϕ) Gravity. Physical Sciences Forum, 7(1), 35. https://doi.org/10.3390/ECU2023-14048






