Simulation-Based Inference of Bayesian Hierarchical Models While Checking for Model Misspecification †
Abstract
1. Introduction
2. Method
2.1. Bayesian Hierarchical Models with a Latent Function
2.2. Latent Function Inference with SELFI
2.3. Check for Model Misspecification
2.4. Score Compression and Simulation-Based Inference
3. Lotka–Volterra BHM
3.1. Lotka–Volterra Solver
3.2. Lotka–Volterra Observer
3.2.1. Full Data Model
- Demographic Gaussian noise with zero mean and variance proportional to the true underlying population, i.e., and . The parameter r gives the strength of demographic noise.
- Observational Gaussian noise that accounts for observer efficiency, coupling prey and predators such thatThe parameter s gives the overall amplitude of observational noise, and the parameter t controls the strength of the non-diagonal component (it should be chosen such that the covariance matrix appearing in Equation (18) is positive semi-definite).
3.2.2. Simplified Data Model
4. Results
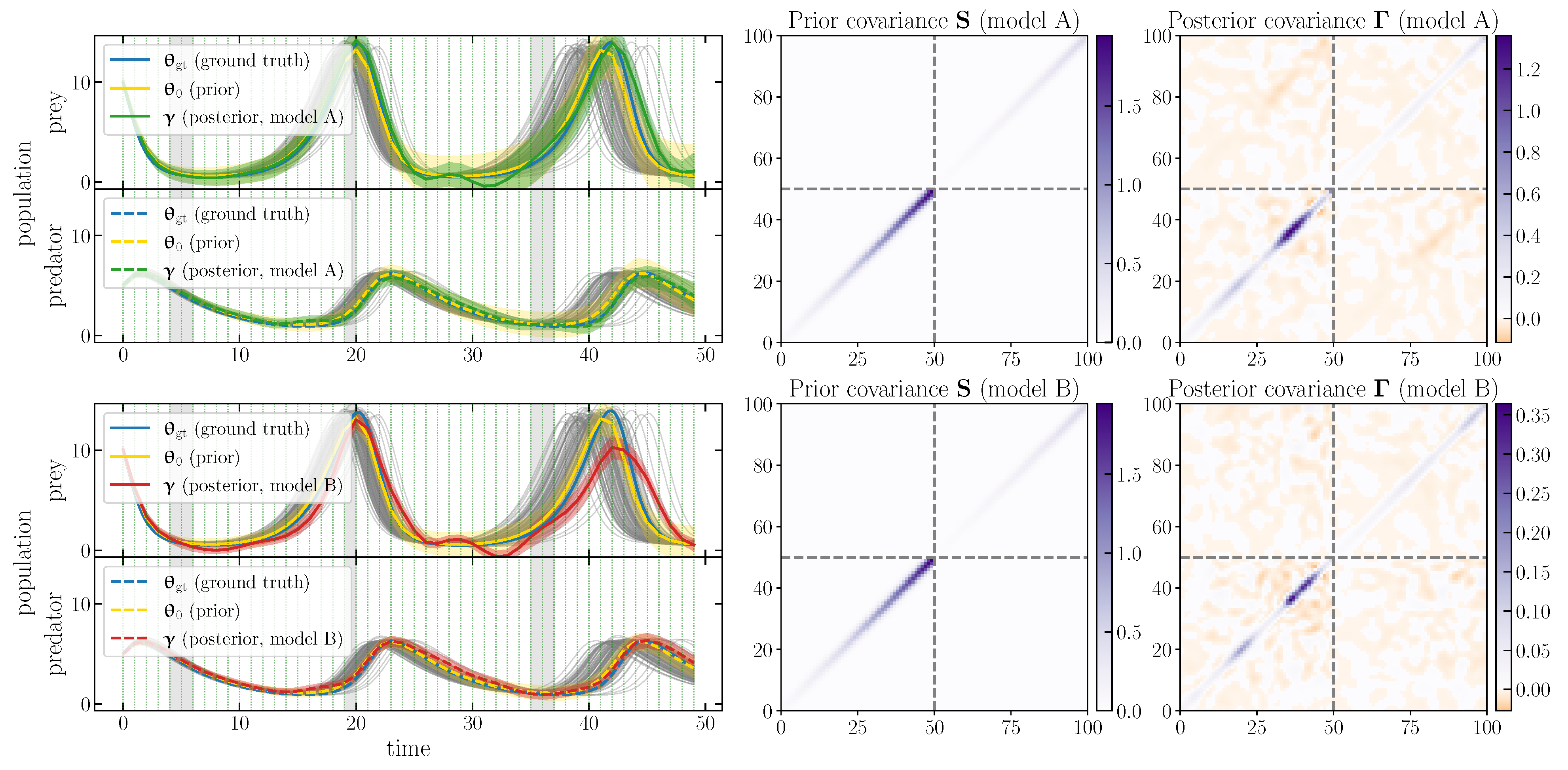
4.1. Inference of Population Functions with SELFI
4.2. Check for Model Misspecification
4.3. Score Compression
4.4. Inference of Parameters Using Likelihood-Free Rejection Sampling
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Leclercq, F.; Enzi, W.; Jasche, J.; Heavens, A. Primordial power spectrum and cosmology from black-box galaxy surveys. Mon. Not. R. Astron. Soc. 2019, 490, 4237–4253. [Google Scholar] [CrossRef]
- Rousset, F. Inferences from Spatial Population Genetics. In Handbook of Statistical Genetics; John Wiley & Sons, Ltd.: London, UK, 2007; Chapter 28; pp. 945–979. [Google Scholar] [CrossRef]
- Alsing, J.; Wandelt, B. Generalized massive optimal data compression. Mon. Not. R. Astron. Soc. Lett. 2018, 476, L60–L64. [Google Scholar] [CrossRef]
- Papamakarios, G.; Murray, I. Fast ϵ-free Inference of Simulation Models with Bayesian Conditional Density Estimation. In Advances in Neural Information Processing Systems 29: Proceedings of the 30th International Conference on Neural Information Processing Systems, 5–10 December 2016, Barcelona, Spain; Curran Associates Inc.: Red Hook, NY, USA, 2016; pp. 1036–1044. [Google Scholar]
- Alsing, J.; Wandelt, B.; Feeney, S. Massive optimal data compression and density estimation for scalable, likelihood-free inference in cosmology. Mon. Not. R. Astron. Soc. 2018, 477, 2874–2885. [Google Scholar] [CrossRef]
- Gutmann, M.U.; Corander, J. Bayesian Optimization for Likelihood-Free Inference of Simulator-Based Statistical Models. J. Mach. Learn. Res. 2016, 17, 1–47. [Google Scholar]
- Leclercq, F. Bayesian optimization for likelihood-free cosmological inference. Phys. Rev. D 2018, 98, 063511. [Google Scholar] [CrossRef]
- Thomas, O.; Pesonen, H.; Sá-Leão, R.; de Lencastre, H.; Kaski, S.; Corander, J. Split-BOLFI for misspecification-robust likelihood free inference in high dimensions. arXiv, 2020; arXiv:2002.09377v1. [Google Scholar]
- Beaumont, M.A. Approximate Bayesian Computation. Annu. Rev. Stat. Its Appl. 2019, 6, 379–403. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leclercq, F. Simulation-Based Inference of Bayesian Hierarchical Models While Checking for Model Misspecification. Phys. Sci. Forum 2022, 5, 4. https://doi.org/10.3390/psf2022005004
Leclercq F. Simulation-Based Inference of Bayesian Hierarchical Models While Checking for Model Misspecification. Physical Sciences Forum. 2022; 5(1):4. https://doi.org/10.3390/psf2022005004
Chicago/Turabian StyleLeclercq, Florent. 2022. "Simulation-Based Inference of Bayesian Hierarchical Models While Checking for Model Misspecification" Physical Sciences Forum 5, no. 1: 4. https://doi.org/10.3390/psf2022005004
APA StyleLeclercq, F. (2022). Simulation-Based Inference of Bayesian Hierarchical Models While Checking for Model Misspecification. Physical Sciences Forum, 5(1), 4. https://doi.org/10.3390/psf2022005004





