Residual Stress Characterization and Part Distortion in Extruded Heat-Treated Aluminum Alloy Used in the Fabrication of Second Rib Structure of an Aircraft Wing †
Abstract
:1. Introduction
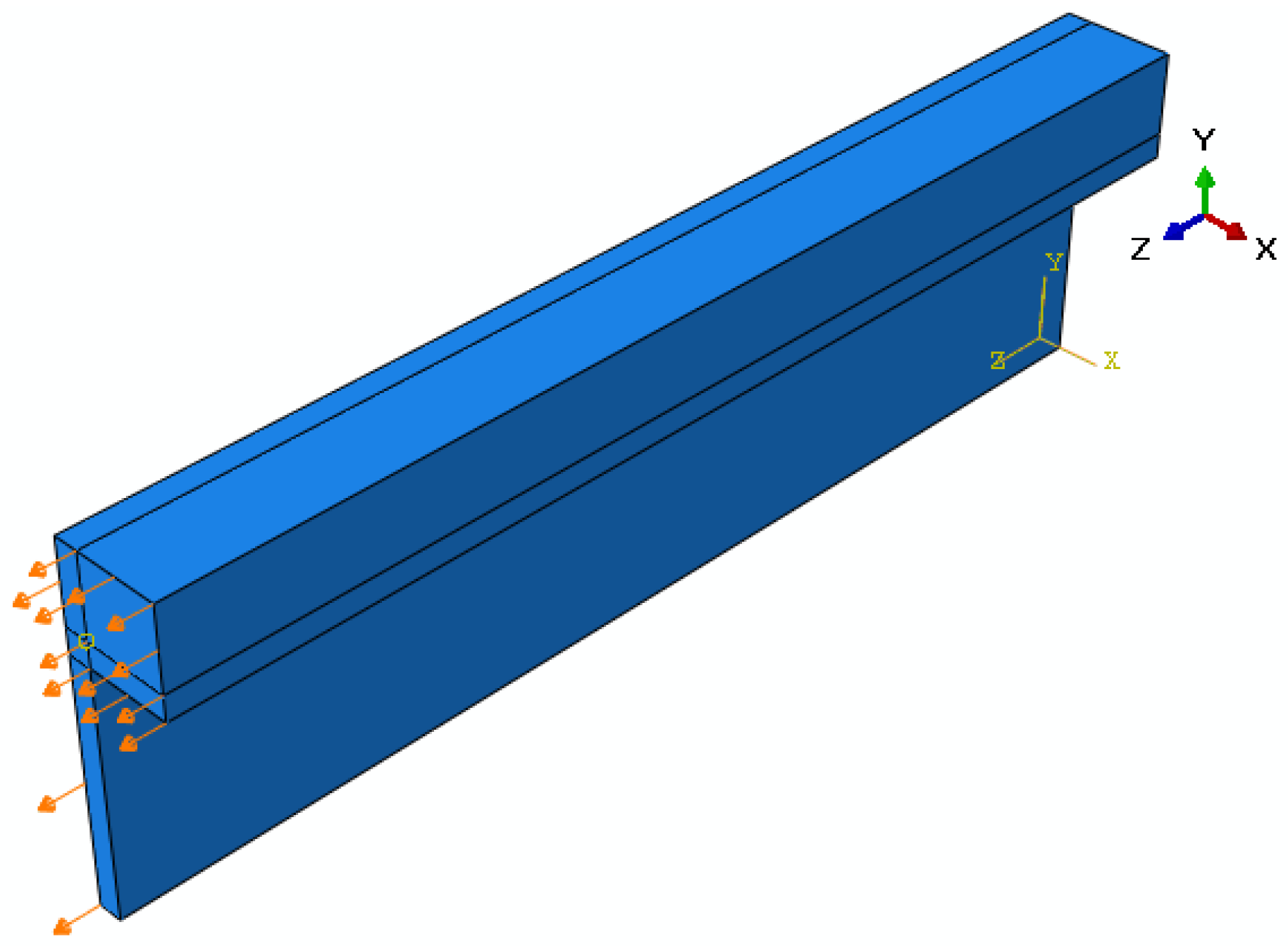
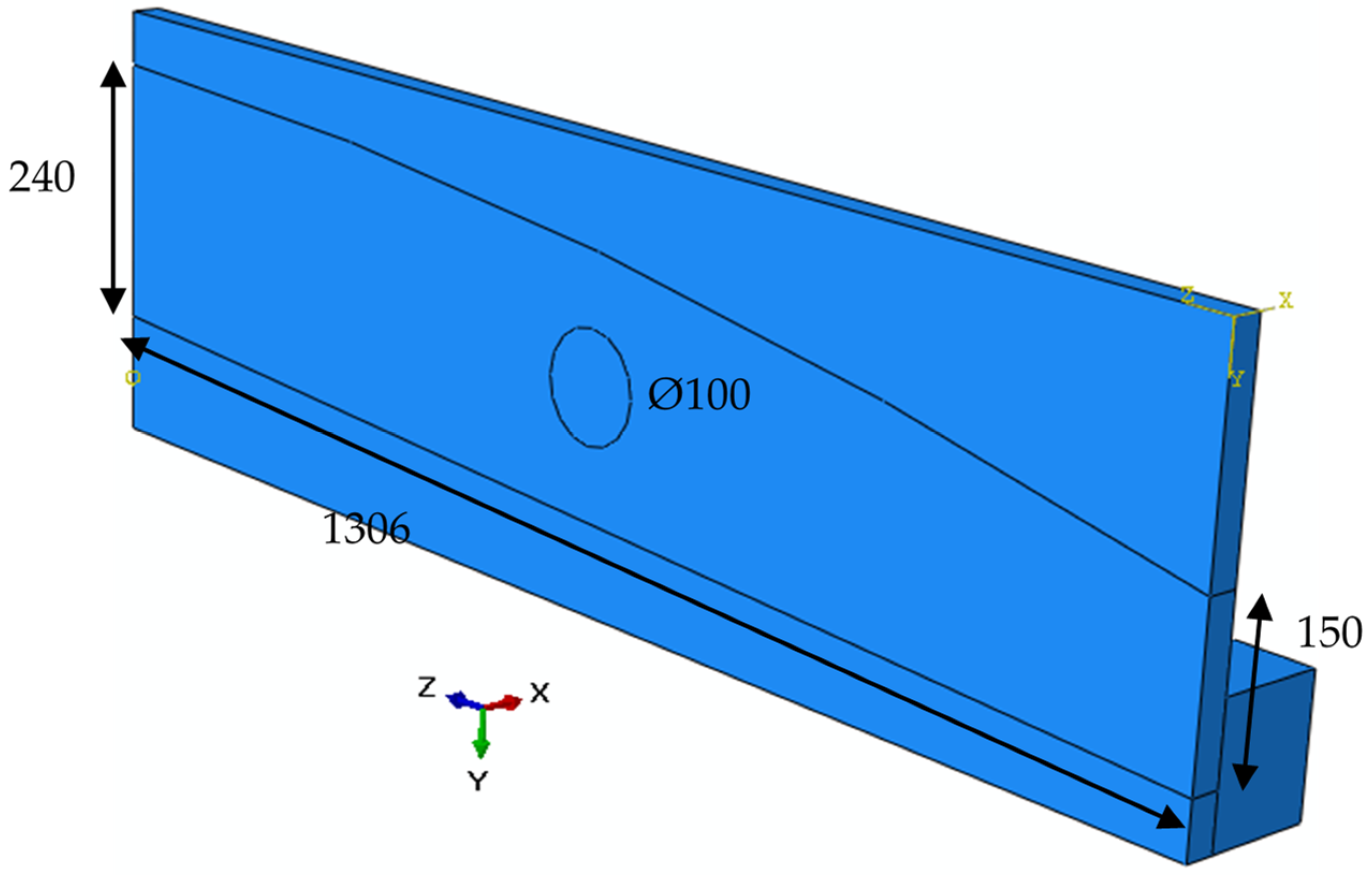
2. Specimen and Material Description
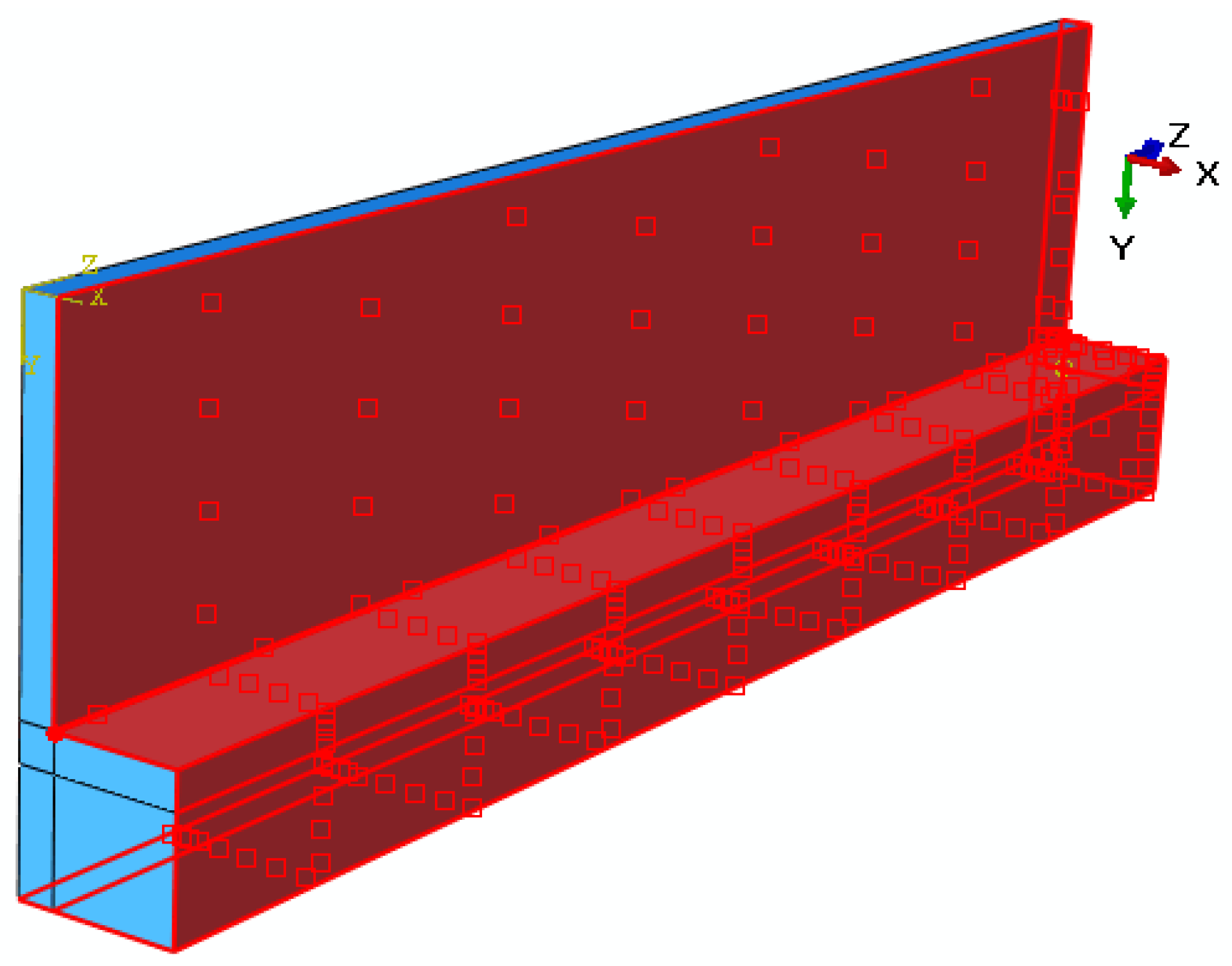
3. Finite Element Analysis
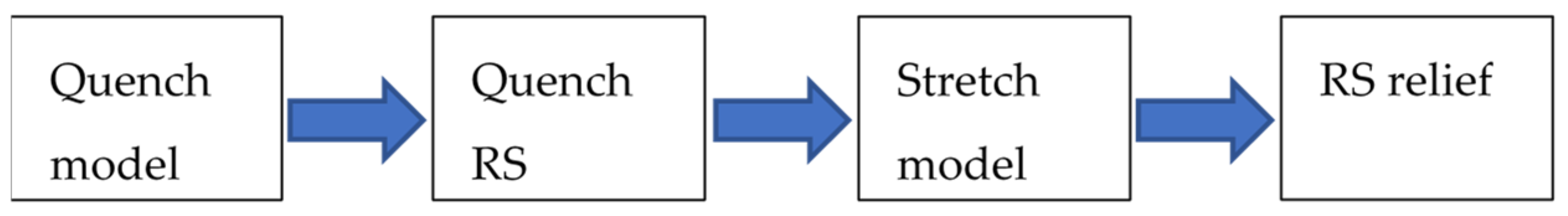
3.1. Finite Element Analysis of Quenching Process
3.2. Finite Element Analysis of Stretching
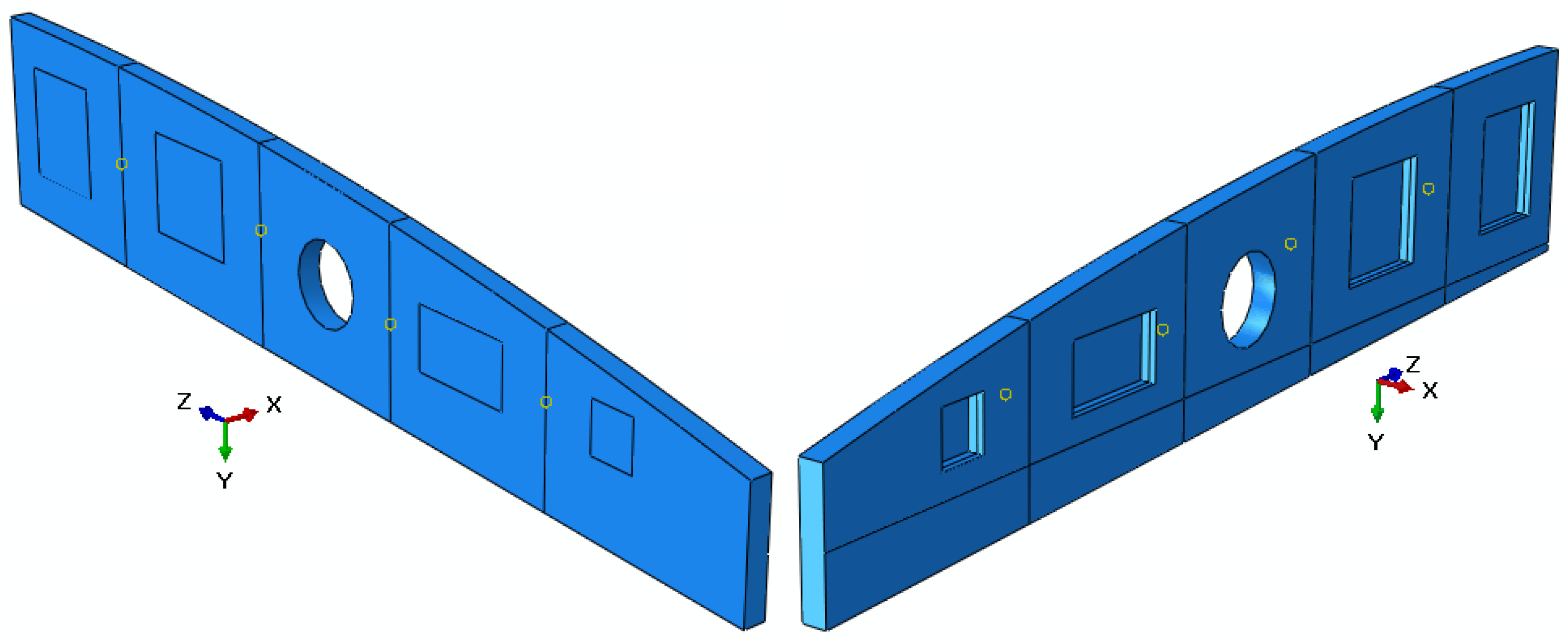
3.3. Finite Element Analysis of Rib Fabrication
4. Experimental Study
4.1. Process Condition
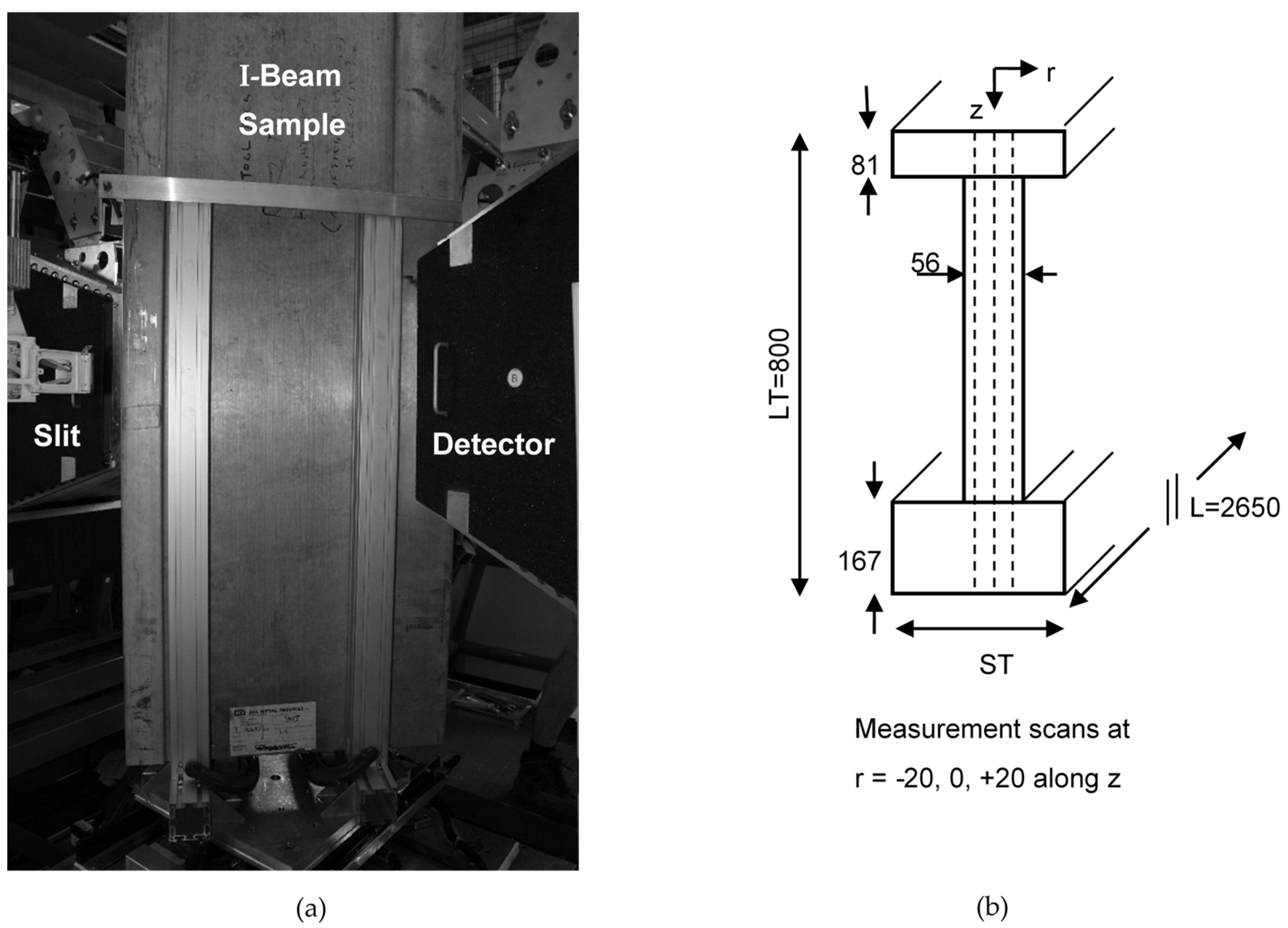
4.2. Residual Stress Measurement
5. Results and Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lequeu, P.; Lassince, P.; Warner, T.; Raynaud, G.M. Engineering for the future: Weight saving and cost reduction initiatives. Aircr. Eng. Aerosp. Technol. 2001, 73, 147–159. [Google Scholar] [CrossRef]
- Mouritz, A.P. Introduction to Aerospace Materials, Ch. 24 Disposal and Recycling of Aerospace Materials; Woodhead Publishing: Sawston, UK, 2012; pp. 558–568. ISBN 9781855739468. [Google Scholar]
- Sim, W.M. Challenges of residual stress and part distortion in the civil airframe industry. Int. J. Microstruct. Mater. Prop. 2010, 5, 446–455. [Google Scholar] [CrossRef]
- Werke, M.; Hossain, M.; Semere, D.; Wretland, A. Machining Distortion Analysis of Aerospace Components using the Contour Method. In Proceedings of the 10th Aerospace Technology Congress, Stockholm, Sweden, 8–9 October 2019. [Google Scholar] [CrossRef] [Green Version]
- The Aluminum Association. Aluminum Standards and Data; Aluminum Association Inc.: Arlington, VA, USA, 2013. [Google Scholar]
- Yu, X.; Robinson, J. Measurement of the Heat Transfer Coefficient during Quenching of the Aluminium Alloy 7449; COMPACT Project: Łódź, Poland, 2007. [Google Scholar]
- Simulia. ABAQUS Analysis User’s Manual, Version 6.12; Dassault Systemes Simulia, Inc.: Johnston, RI, USA, 2012. [Google Scholar]
- Fitzpatrick, M.E.; Lodini, A. Analysis of Residual Stress by Diffraction using Neutron and Synchrotron Radiation; Taylor and Francis: London, UK, 2003. [Google Scholar]
- Daymond, M.R.; Bourke, M.A.M.; Von Dreele, R.B. Use of Rietveld refinement to fit a hexagonal crystal structure in the presence of elastic and plastic anisotropy. J. Appl. Phys. 1999, 85, 739–747. [Google Scholar] [CrossRef]
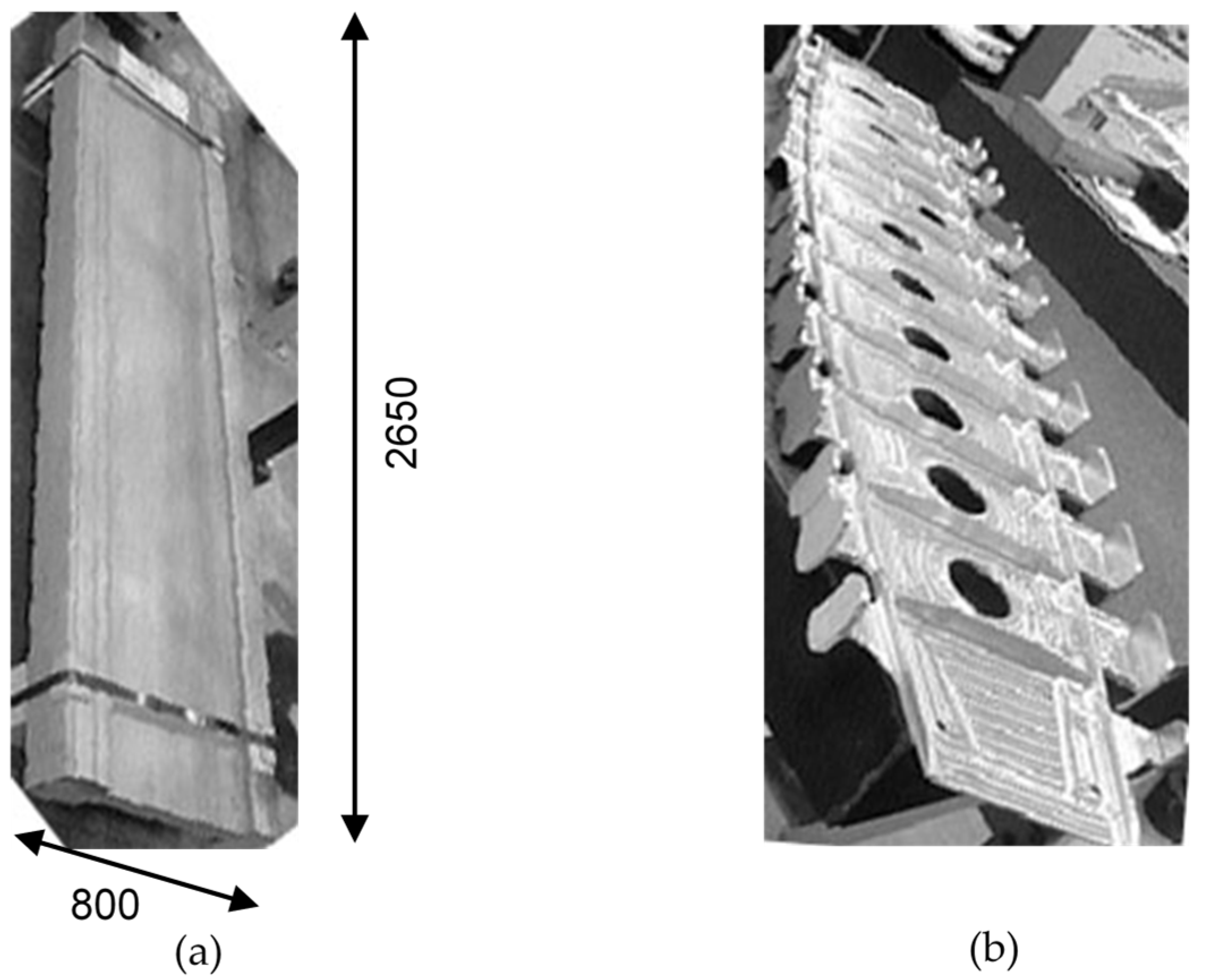

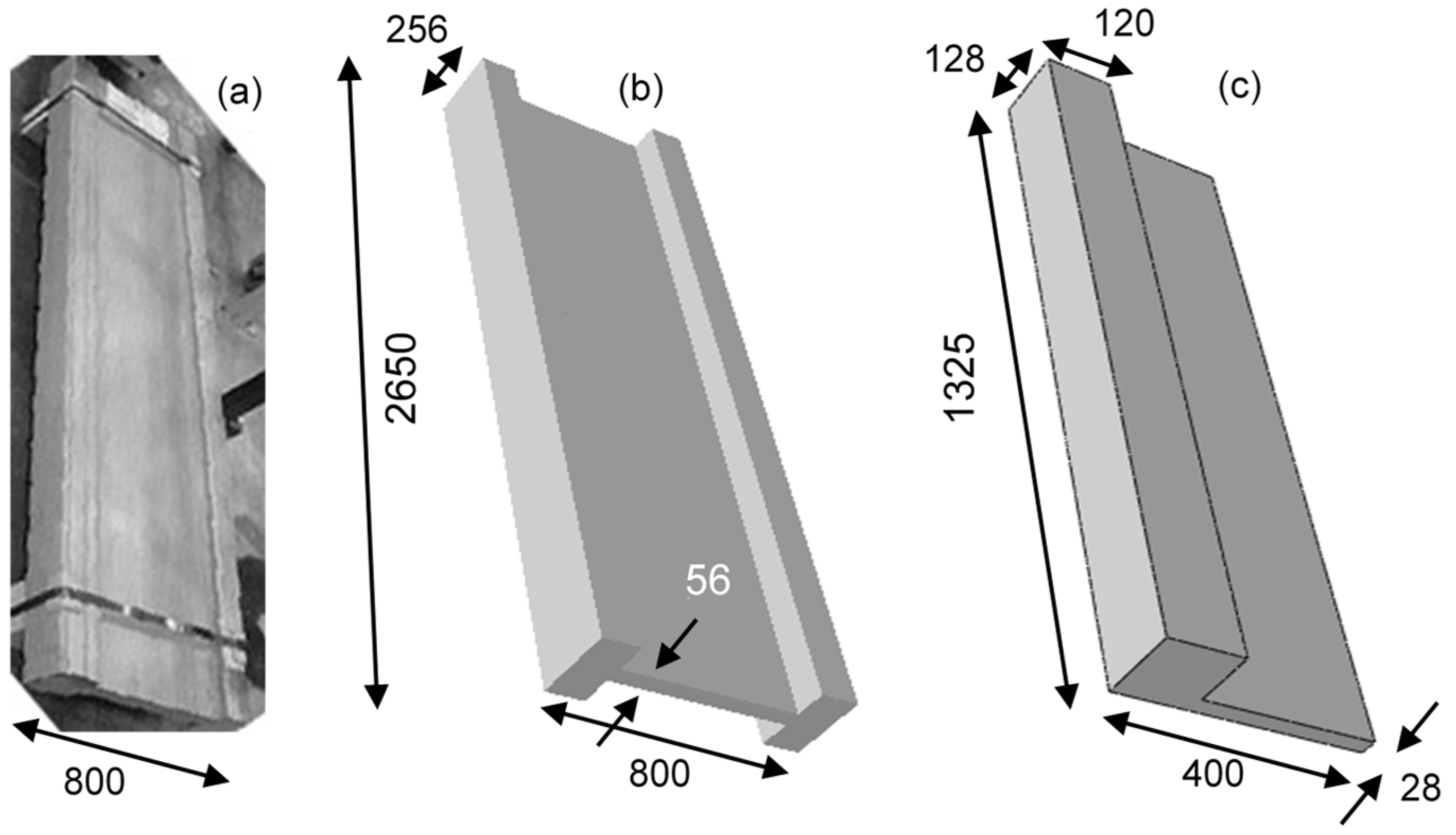


| Element | Al | Zn | Cu | Mg | Zr | Fe | Si | Mn | Ti | Cr |
|---|---|---|---|---|---|---|---|---|---|---|
| Percentage, % | 87.3–92.1 | 5.7–6.7 | 2.0–2.6 | 1.9–2.6 | 0.08–0.15 | 0–0.15 | 0–0.12 | 0–0.1 | 0–0.06 | 0–0.04 |
| Temperature, °C | Conductivity, Wm−2 K−1 | Specific Heat, Jkg−1 K−1 | Density, kgm−3 |
|---|---|---|---|
| 20 | 166 | 842 | 2796 |
| 93 | 175 | 900 | 2781 |
| 205 | 180 | 963 | 2759 |
| 316 | 175 | 1055 | 2737 |
| 427 | 163 | 1172 | 2715 |
| 475 | 156 | 1230 | 2705 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hossain, M.S.; Al-Hinai, S.S.; Miah, M.S. Residual Stress Characterization and Part Distortion in Extruded Heat-Treated Aluminum Alloy Used in the Fabrication of Second Rib Structure of an Aircraft Wing. Phys. Sci. Forum 2022, 4, 19. https://doi.org/10.3390/psf2022004019
Hossain MS, Al-Hinai SS, Miah MS. Residual Stress Characterization and Part Distortion in Extruded Heat-Treated Aluminum Alloy Used in the Fabrication of Second Rib Structure of an Aircraft Wing. Physical Sciences Forum. 2022; 4(1):19. https://doi.org/10.3390/psf2022004019
Chicago/Turabian StyleHossain, Mohammad Sayeed, Sami Salim Al-Hinai, and Md Salim Miah. 2022. "Residual Stress Characterization and Part Distortion in Extruded Heat-Treated Aluminum Alloy Used in the Fabrication of Second Rib Structure of an Aircraft Wing" Physical Sciences Forum 4, no. 1: 19. https://doi.org/10.3390/psf2022004019
APA StyleHossain, M. S., Al-Hinai, S. S., & Miah, M. S. (2022). Residual Stress Characterization and Part Distortion in Extruded Heat-Treated Aluminum Alloy Used in the Fabrication of Second Rib Structure of an Aircraft Wing. Physical Sciences Forum, 4(1), 19. https://doi.org/10.3390/psf2022004019






