Measuring Concentrated Solar Radiation Flux in a Linear Fresnel-Type Solar Collector †
Abstract
:1. Introduction
2. Materials and Methods
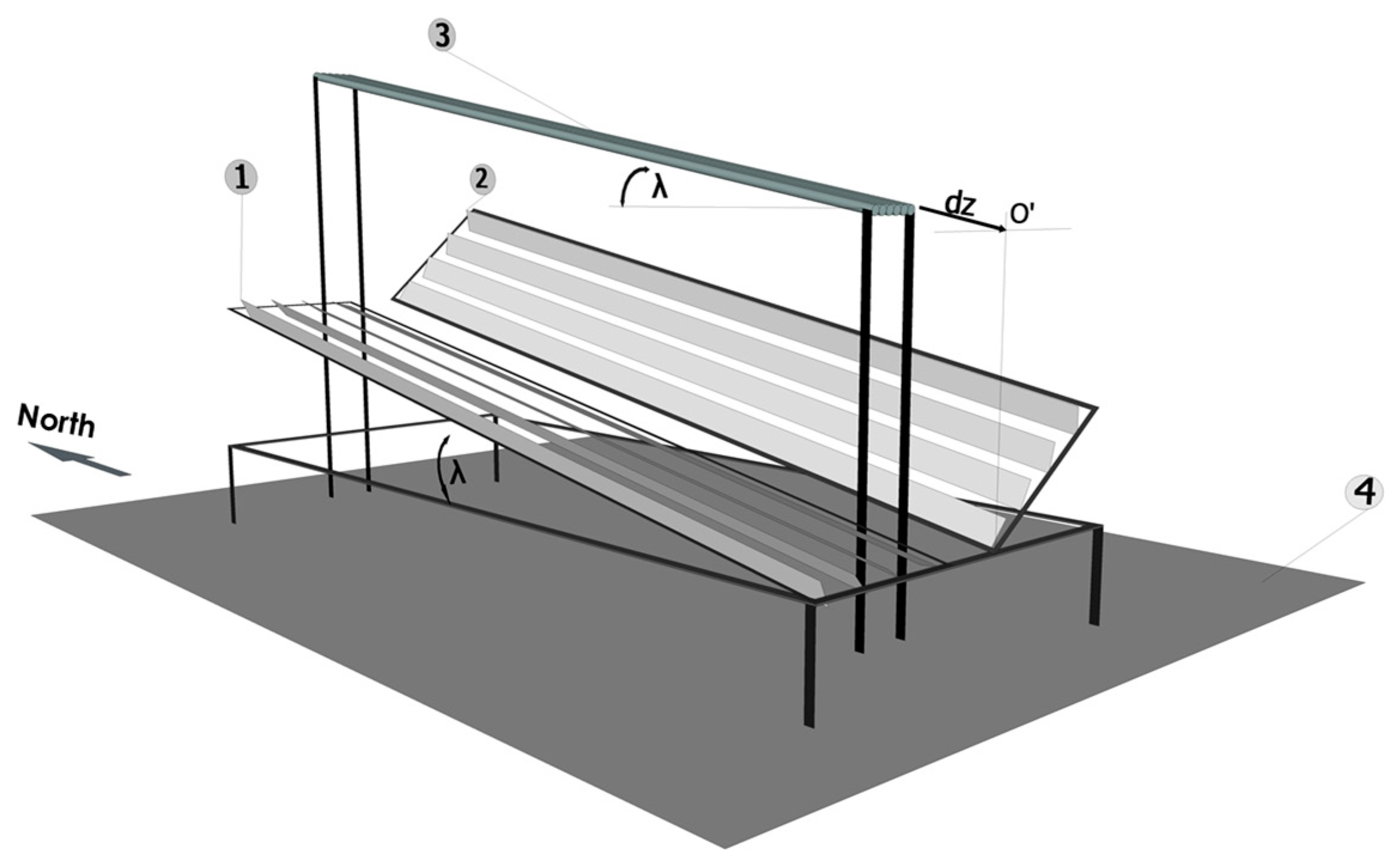
2.1. Description of the Linear Fresnel Collector
2.1.1. Geometry of the Collector System
2.1.2. Optical Efficiency of the Novel Design
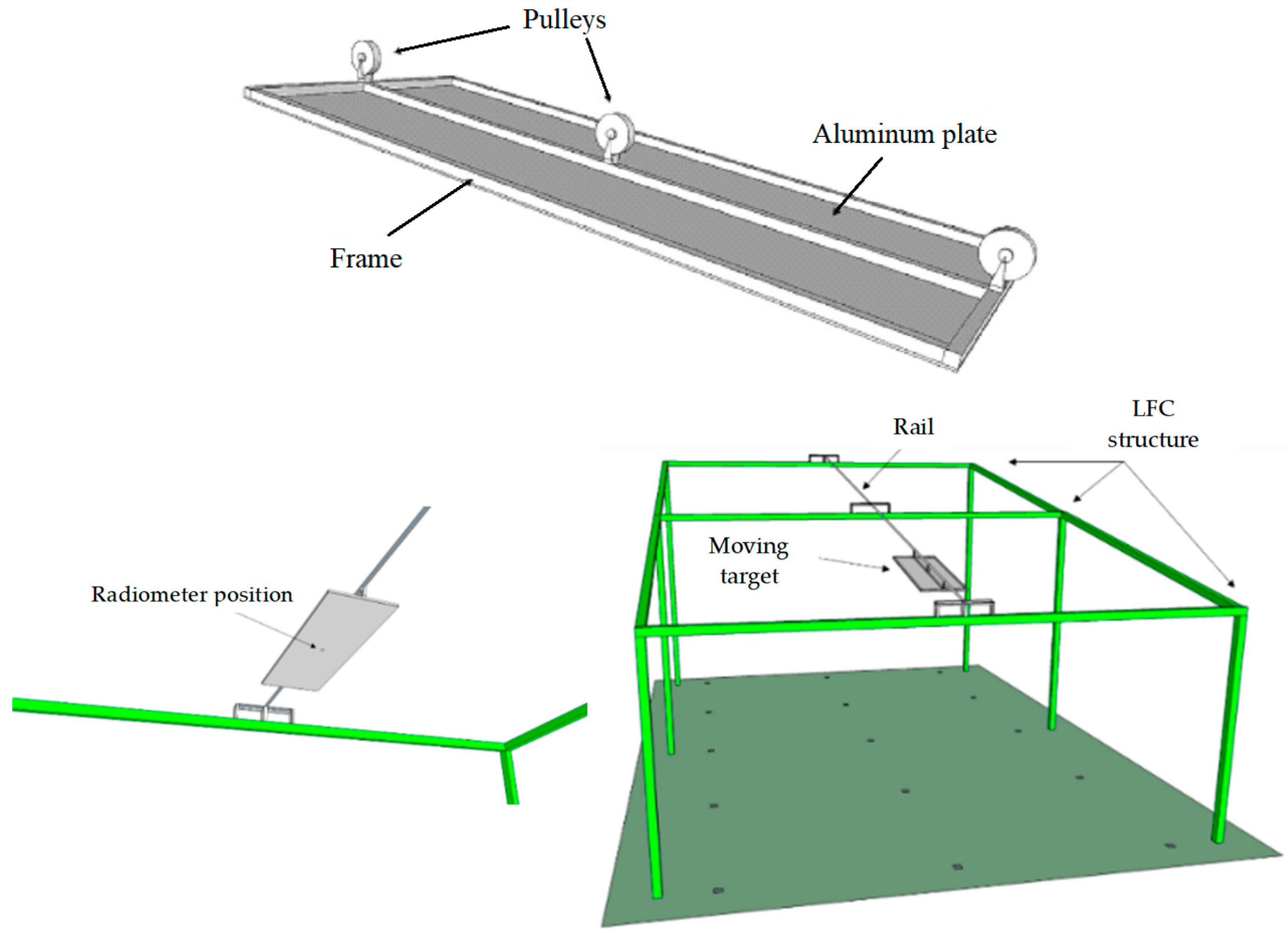
2.2. Concentrated Solar Radiation Flux Measurement Methodology
2.2.1. Description of the Measuring Device
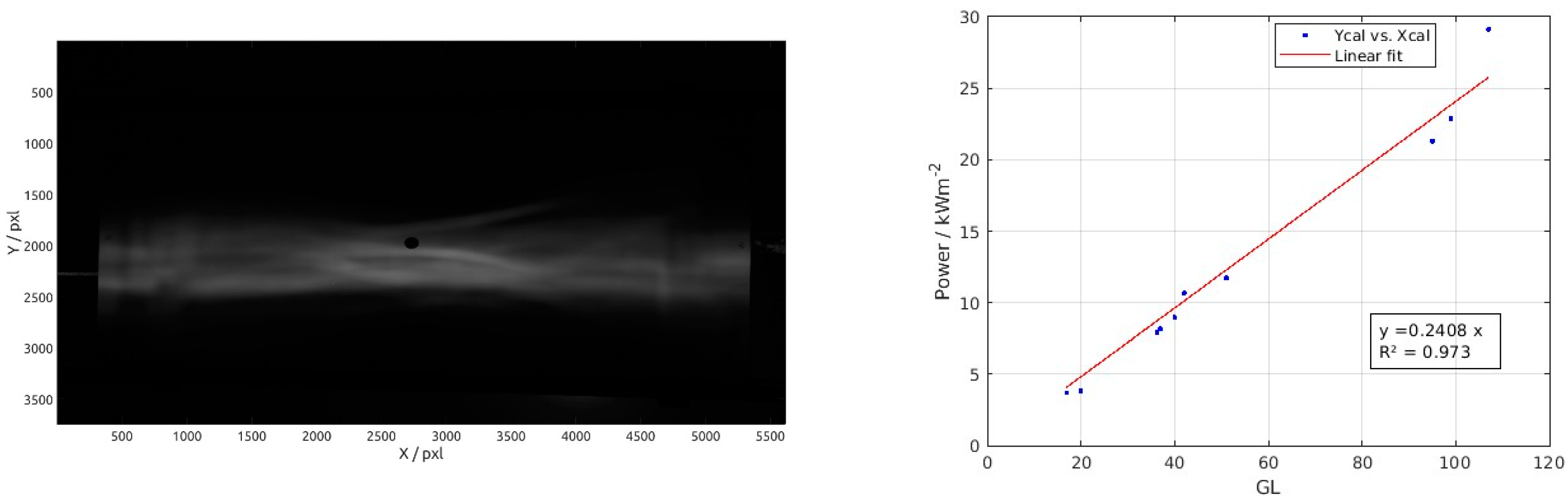
2.2.2. Calibration Procedure of the Flux Measurement System
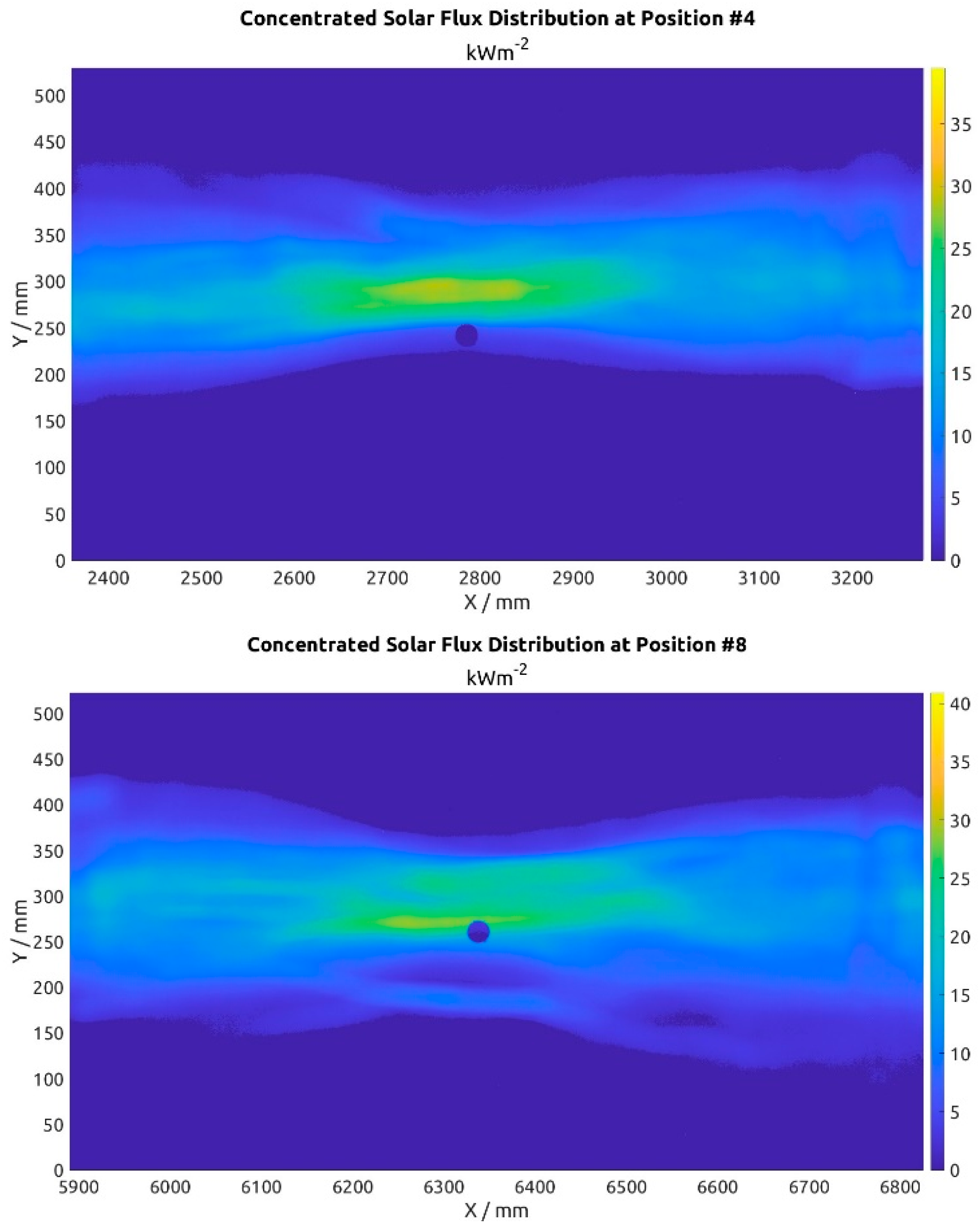
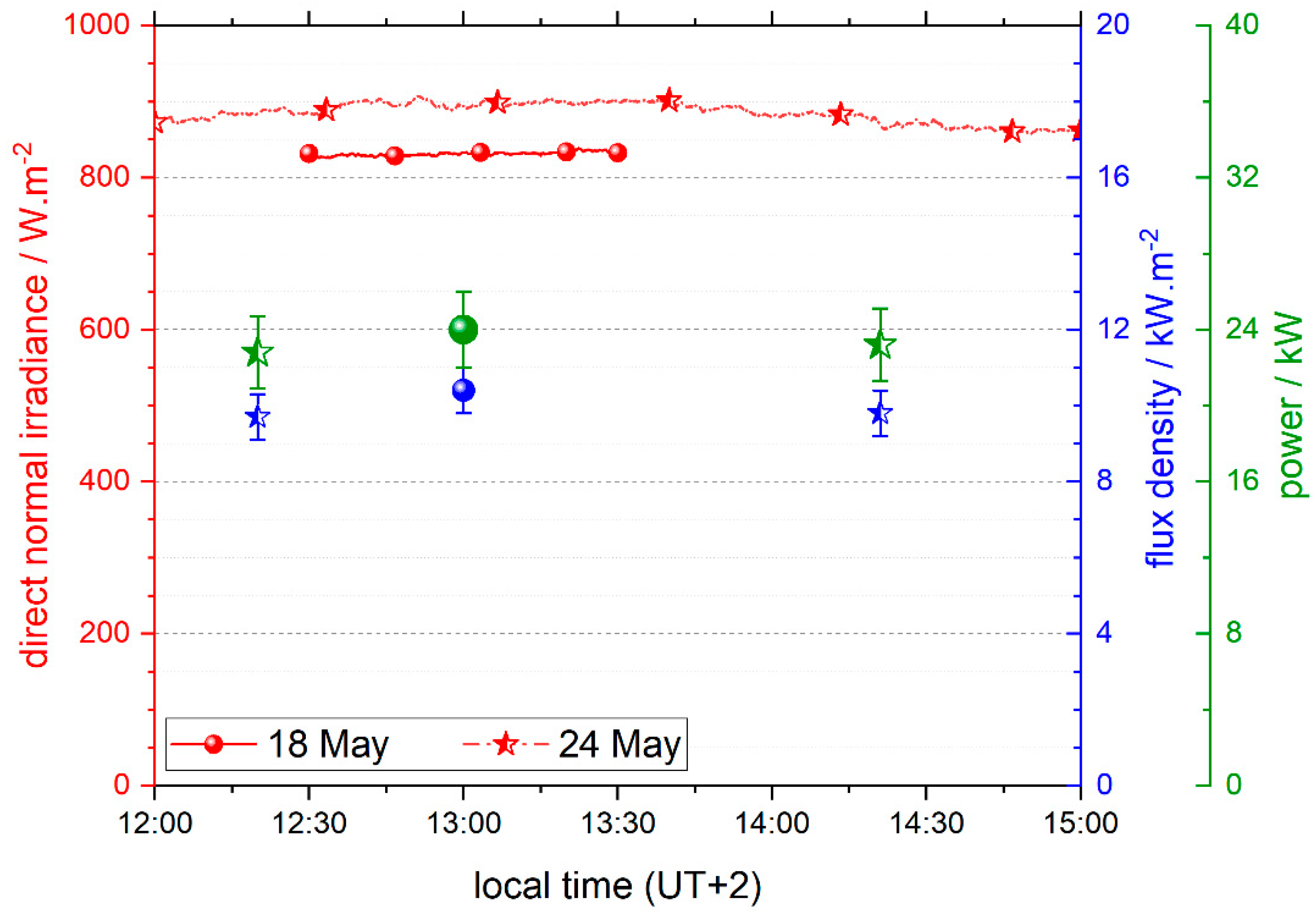
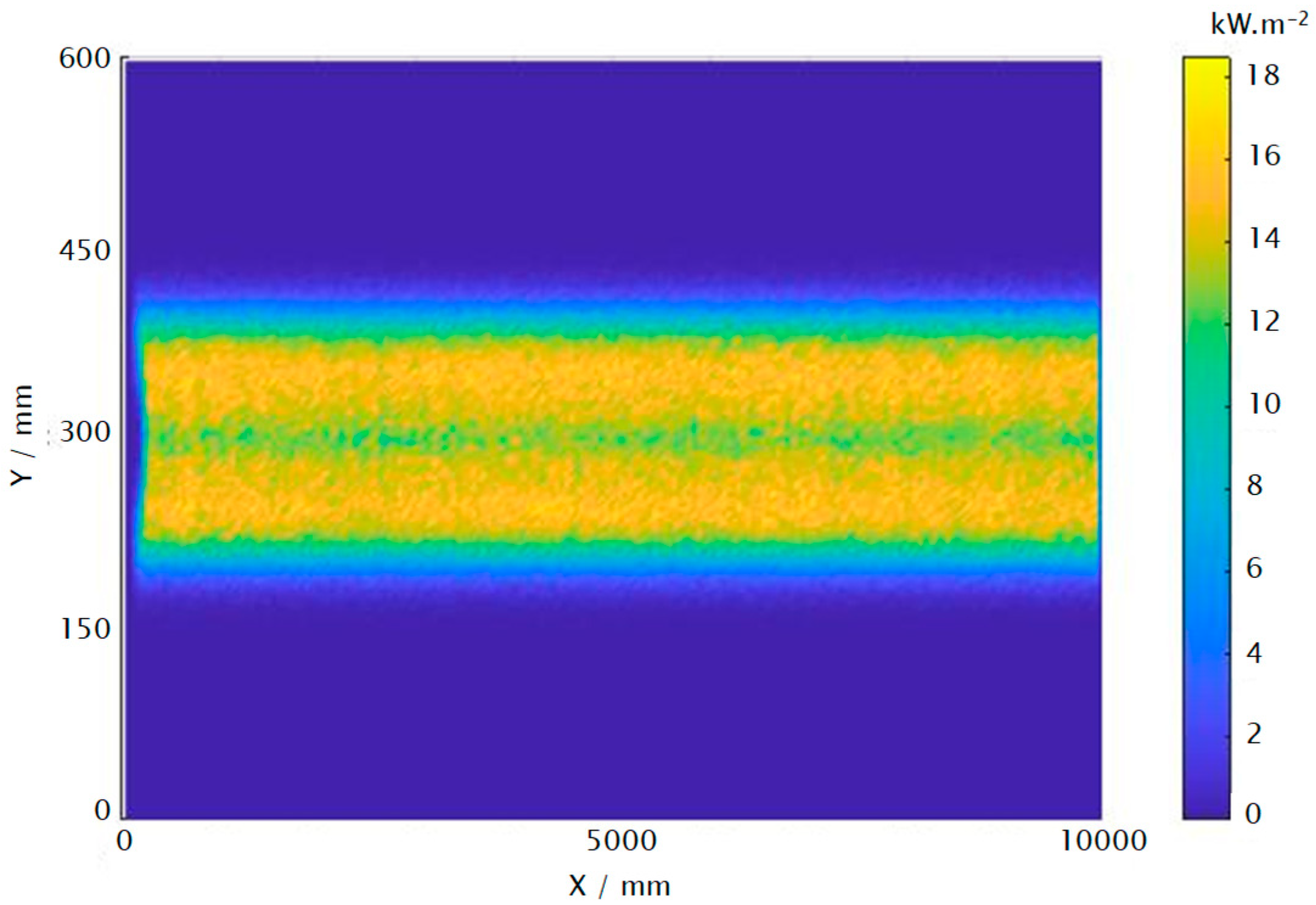
3. Results
4. Discussion
4.1. Flux Density, Power, and Optical Efficiency from Experiment
4.2. Comparison of Experimental to Simulation Results
5. Conclusions
6. Patents
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CMOS | complementary metal-oxide semiconductor |
| DN | diameter nominal |
| DNI | direct normal irradiance (W/m2) |
| IAM | incidence angle modifier |
| ISO | International Standards Organization |
| LFC | linear Fresnel collector |
| PSA | Plataforma Solar de Almería |
| UT | universal time |
References
- Platzer, W.J.; Mills, D.; Gardner, W. Linear Fresnel collector (LFC) solar thermal technology. In Concentrating Solar Power Technology, 1st ed.; Lovegrove, K., Stein, W., Eds.; Woodhead Publishing: Cambridge, UK, 2021; Chapter 6; pp. 165–217. [Google Scholar] [CrossRef]
- Pulido-Iparraguirre, D.; Valenzuela, L.; Serrano-Aguilera, J.J. Optimized design of a Linear Fresnel reflector for solar process heat applications. Renew. Energy 2019, 131, 1089–1106. [Google Scholar] [CrossRef]
- Zhu, J.; Chen, Z. Optical design of compact linear fresnel reflector systems. Sol. Energy Mater. Sol. Cells 2018, 176, 239–250. [Google Scholar] [CrossRef]
- Hongn, M.; Flores Larsen, S. Hydrothermal model for small-scale linear Fresnel absorbers with non-uniform stepwise solar distribution. Appl. Energy 2018, 223, 329–346. [Google Scholar] [CrossRef]
- Yang, M.; Zhu, Y.; Taylor, R.A. End losses minimization of linear Fresnel reflectors with a simple, two-axis mechanical tracking system. Energy Convers. Manag. 2018, 161, 284–293. [Google Scholar] [CrossRef]
- Ma, J.; Chang, Z. Understanding the effects of end-loss on linear Fresnel collectors. IOP Conf. Ser. Earth Environ. Sci. 2018, 121, 052052. [Google Scholar] [CrossRef]
- Manikumar, R.; Valan Arasu, A. Heat loss characteristics study of a trapezoidal cavity absorber with and without plate for a linear Fresnel reflector solar concentrator system. Renew. Energy 2014, 63, 98–108. [Google Scholar] [CrossRef]
- Zhu, Y.; Shi, J.; Li, Y.; Wang, L.; Huang, Q.; Xu, G. Design and experimental investigation of a stretched parabolic linear Fresnel reflector collecting system. Energy Convers. Manag. 2016, 126, 89–98. [Google Scholar] [CrossRef]
- Zhu, J.; Huang, H. Design and thermal performances of Semi-Parabolic Linear Fresnel Reflector solar concentration collector. Energy Convers. Manag. 2014, 77, 733–737. [Google Scholar] [CrossRef]
- Zhao, X.; Yan, S.; Zhang, N.; Zhao, N.; Gao, H. Solar flux measuring and optical efficiency forecasting of the linear Fresnel reflector concentrator after dust accumulation. J. Therm. Sci. 2022, 31, 663–677. [Google Scholar] [CrossRef]
- Fernández-Reche, J.; Pulido-Iparraguirre, D.; Valenzuela, L. Medida de flujo de radiación solar concentrada en un captador solar lineal Fresnel. In Proceedings of the XVIII Congreso Ibérico y XIV Congreso Iberoamericano de Energía Solar, Palma, Spain, 20–22 June 2022; pp. 89–96. [Google Scholar]
- AGC Solar Mirror Thin, Datasheet. Available online: https://www.agc-solar.com/product-solutions/ (accessed on 25 May 2021).
- Pulido-Iparraguirre, D.; Valenzuela, L.; Fernández-Reche, J.; Galindo, J.; Rodríguez, J. Design, Manufacturing and Characterization of Linear Fresnel Reflector’s Facets. Energies 2019, 12, 2795. [Google Scholar] [CrossRef]
- Abbas, R.; Muñoz-Antón, J.; Valdés, M.; Martínez-Val, J.M. High concentration linear Fresnel reflectors. Energy Convers. Manag. 2013, 72, 60–68. [Google Scholar] [CrossRef]
- Blanco, M.J.; Amieva, J.M.; Mancillas, A. The Tonatiuh Software Development Project: An open source approach to the simulation of solar concentrating systems. In ASME 2005 International Mechanical Engineering Congress and Exposition (IMECE); American Society of Mechanical Engineers: Orlando, FL, USA, 2005; pp. 157–164. [Google Scholar] [CrossRef]
- Valenzuela, L.; Pulido-Iparraguirre, D.; Fernández-Reche, J. Mejora de la eficiencia óptica de un captador linear Fresnel innovador. In Proceedings of the XVIII Congreso Ibérico y XIV Congreso Iberoamericano de Energía Solar, Palma, Spain, 20–22 June 2022. [Google Scholar]
- Lüpfert, E.; Heller, P.; Ulmer, S.; Monterreal, R.; Fernández-Reche, J. Concentrated solar radiation measurement with video image processing and online fluxgage calibration. In Proceedings of the Solar Thermal 2000 International Conference, Sydney, Australia, 8–10 March 2000. [Google Scholar]
- Ballestrin, J.; Ulmer, S.; Morales, A.; Barnes, A.; Langley, L.W.; Rodríguez, M. Systematic error in the measurement of very high solar irradiance. Sol. Energy Mater. Sol. Cells 2003, 80, 375–381. [Google Scholar] [CrossRef]
- Pulido-Iparraguirre, D. Desarrollo de un Prototipo de Captador Solar Fresnel Lineal de Media Temperatura. Ph.D. Thesis, Universidad de Almería, Almería, Spain, February 2020. [Google Scholar]
- Chekh, B.A.; Fernández-Reche, J.; Valenzuela, L.; Pulido-Iparraguirre, D.; Villasante, C. Compact system for fast on-line geometry characterization of facets for solar concentrators. SolarPACES 2020. AIP Conf. Proc. 2022, 2445, 070001. [Google Scholar] [CrossRef]
- García, G.; Egea, A.; Valenzuela, L.; Pulido-Iparraguirre, D.; Liria, J. Advanced sun-tracking control of an innovative linear Fresnel collector. In Proceedings of the 27th SolarPACES Conference, Online Event, 27 September–1 October 2021. [Google Scholar]



| Equipment | Characteristics |
|---|---|
| Image acquisition | CANON EOS 5d MarkII Camera |
| Lens focal length: 80 mm | |
| Aperture: f/22 | |
| Exposure time: 1/1250 s | |
| ISO: 6400 | |
| Filter: neutral optical density 2.0 (log Ii/It = 2) | |
| Radiometer | Vatell water cooled heat flux sensor |
| Coating: zynolite | |
| Calibration constant: 5.32 kW·m−2·mV−2 (±3%) | |
| Pyrheliometer | Kipp & Zonen CH1 |
| Installed on a robotic solar tracker | |
| Uncertainty < 1% | |
| Data acquisition | Midi LOGGER GL200. Graphtec Corp. |
| Position | Average Flux Density (kW/m2) | Maximum Flux Density (kW/m2) | Total Incident Power (kW) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| 18 May | 24 May Bef. Noon | 24 May Aft. Noon | 18 May | 24 May Bef. Noon | 24 May Aft. Noon | 18 May | 24 May Bef. Noon | 24 May Aft. Noon | |
| #1 | 10.2 ± 0.6 | 9.5 ± 0.6 | 9.7 ± 0.5 | 38 ± 2 | 36 ± 2 | 36.3 ± 1.8 | 1.96 ± 0.13 | 1.83 ± 0.11 | 1.86 ± 0.12 |
| #2 | 10.6 ± 0.6 | 9.9 ± 0.6 | 10.0 ± 0.5 | 31.4 ± 1.9 | 29.3 ± 1.8 | 29.9 ± 1.5 | 2.51 ± 0.17 | 2.35 ± 0.14 | 2.39 ± 0.16 |
| #3 | 10.5 ± 0.6 | 9.8 ± 0.6 | 9.9 ± 0.5 | 35 ± 2 | 33 ± 2 | 33.5 ± 1.7 | 2.95 ± 0.20 | 2.75 ± 0.16 | 2.80 ± 0.18 |
| #4 | 10.8 ± 0.6 | 10.1 ± 0.6 | 10.3 ± 0.5 | 42 ± 3 | 39 ± 2 | 40 ± 2 | 2.10 ± 0.14 | 1.96 ± 0.12 | 1.99 ± 0.13 |
| #5 | 10.9 ± 0.6 | 10.1 ± 0.6 | 10.3 ± 0.5 | 42 ± 3 | 39 ± 2 | 40 ± 2 | 2.53 ± 0.17 | 2.36 ± 0.14 | 2.40 ± 0.16 |
| #6 | 9.2 ± 0.6 | 8.6 ± 0.5 | 8.8 ± 0.5 | 38 ± 2 | 36 ± 2 | 36.3 ± 1.8 | 2.74 ± 0.18 | 2.56 ± 0.15 | 2.61 ± 0.17 |
| #7 | 10.1 ± 0.6 | 9.5 ± 0.6 | 9.6 ± 0.5 | 49 ± 3 | 45 ± 3 | 46 ± 2 | 2.12 ± 0.14 | 1.98 ± 0.12 | 2.02 ± 0.13 |
| #8 | 10.4 ± 0.6 | 9.7 ± 0.6 | 9.9 ± 0.5 | 43 ± 3 | 40 ± 2 | 41 ± 2 | 2.28 ± 0.15 | 2.13 ± 0.13 | 2.17 ± 0.14 |
| #9 | 10.7 ± 0.6 | 10.0 ± 0.6 | 10.2 ± 0.5 | 41 ± 2 | 38 ± 2 | 38.5 ± 1.9 | 2.53 ± 0.17 | 2.36 ± 0.14 | 2.41 ± 0.16 |
| #10 | 10.2 ± 0.6 | 9.5 ± 0.6 | 9.7 ± 0.5 | 41 ± 2 | 38 ± 2 | 38.8 ± 1.9 | 2.68 ± 0.18 | 2.50 ± 0.15 | 2.55 ± 0.17 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fernández-Reche, J.; Valenzuela, L.; Pulido-Iparraguirre, D. Measuring Concentrated Solar Radiation Flux in a Linear Fresnel-Type Solar Collector. Solar 2022, 2, 401-413. https://doi.org/10.3390/solar2040024
Fernández-Reche J, Valenzuela L, Pulido-Iparraguirre D. Measuring Concentrated Solar Radiation Flux in a Linear Fresnel-Type Solar Collector. Solar. 2022; 2(4):401-413. https://doi.org/10.3390/solar2040024
Chicago/Turabian StyleFernández-Reche, Jesús, Loreto Valenzuela, and Diego Pulido-Iparraguirre. 2022. "Measuring Concentrated Solar Radiation Flux in a Linear Fresnel-Type Solar Collector" Solar 2, no. 4: 401-413. https://doi.org/10.3390/solar2040024
APA StyleFernández-Reche, J., Valenzuela, L., & Pulido-Iparraguirre, D. (2022). Measuring Concentrated Solar Radiation Flux in a Linear Fresnel-Type Solar Collector. Solar, 2(4), 401-413. https://doi.org/10.3390/solar2040024








