Modelling Systems with a Finite-Capacity Queue: A Theoretical Investigation into the Design and Operation of Real Systems
Abstract
:1. Introduction
2. Assessment of Physical Performance
2.1. System Characteristics
2.2. Probability of Customer Rejection
2.3. System Capacity with Waiting and Rejection
2.4. Average Waiting Time
- The probability of losing customers while the system is busy.
- The average waiting time compared to a specified threshold accepted for waiting time or total transit time.
3. Optimisation of the System Structure
3.1. Cost Function Related to System Operation
- Pr—the probability of rejection given by Equation (4).
- v—the profit obtained for customer service.
- h—the cost associated with a place for waiting.
- c—the cost associated with a server
3.2. Number of Servers
- The arrival rate is 320 vehicles/day (λ = 320 vehicles/day).
- The service rate of a refuelling server is 160 vehicles/day (μ = 160 vehicles/day).
- There are six places for waiting for fuelling (m = 6).
- The fixed costs associated with a server comprise 600 monetary units/day (c = 600 m.u./day).
- The profit resulting from a vehicle fuelling comprises 10 monetary units/vehicle (v = 10 m.u./vehicle).
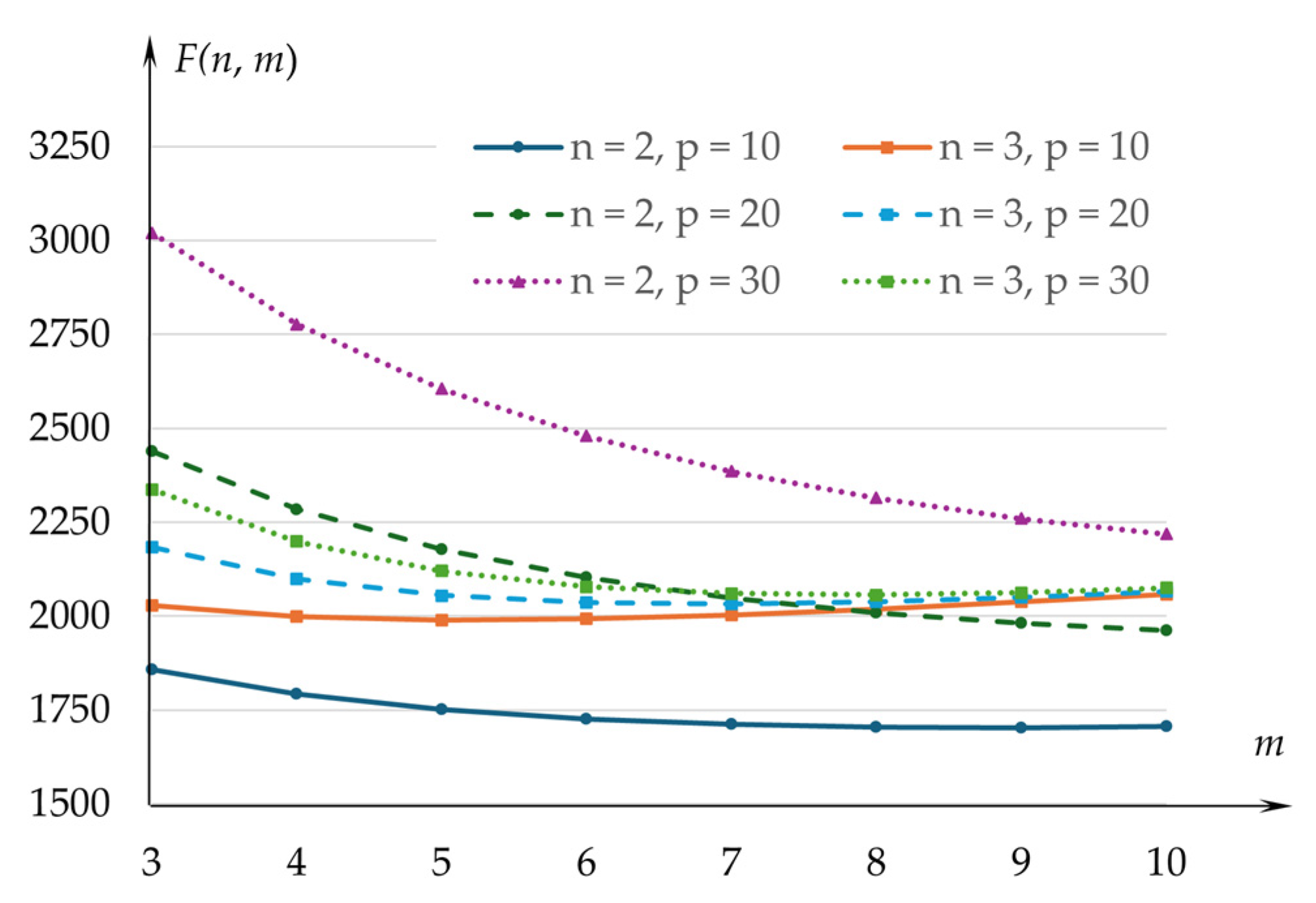
3.3. Number of Servers and Number of Waiting Places
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Asanjarani, A.; Nazarathy, Y.; Taylor, P. A survey of parameter and state estimation in queues. Queueing Syst. 2021, 97, 39–80. [Google Scholar] [CrossRef]
- Gross, D.; Shortie, J.F.; Thompson, J.M.; Harris, C.M. Fundamentals of Queueing Theory; Wiley Series in Probability and Statistics; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar] [CrossRef]
- Hall, R.W. Queueing Methods for Services and Manufacturing; Prentice Hall: Hoboken, NJ, USA, 1991. [Google Scholar]
- Barndorff-Nielsen, O.E.; Cox, D.R.; Kluppelberg, C. (Eds.) Complex Stochastic Systems, 1st ed.; CRC Press. Taylor & Francis Group: Boca Raton, FL, USA, 2000. [Google Scholar] [CrossRef]
- Kempa, W.M.; Kobielnik, M. Transient solution for the queue-size distribution in a finite-buffer model with general independent input stream and single working vacation policy. Appl. Math. Mod. 2018, 59, 614–628. [Google Scholar] [CrossRef]
- Sanga, S.S. Nidhi. Cost optimisation and ANFIS computing for M/M/(R+c)/N queue under admission control policy and server breakdown. Simul. Model. Pr. Theory 2025, 138, 103037. [Google Scholar] [CrossRef]
- Jain, M.; Sanga, S.S. State dependent queueing models under admission control F-policy: A survey. J. Ambient. Intell. Humaniz. Comput. 2020, 11, 3873–3891. [Google Scholar] [CrossRef]
- Yang, D.Y.; Chiang, Y.C.; Tsou, C.S. Cost analysis of a finite capacity queue with server breakdowns and threshold-based recovery policy. J. Manuf. Syst. 2013, 32, 174–179. [Google Scholar] [CrossRef]
- Raicu, S.; Costescu, D.; Popa, M. Effects of the queue discipline on system performance. AppliedMath 2023, 3, 37–48. [Google Scholar] [CrossRef]
- Gupta, S.M. Interrelationship between controlling arrival and service in queueing systems. Comput. Oper. Res. 1995, 22, 1005–1014. [Google Scholar] [CrossRef]
- Tian, N.; Zhang, Z.G. Vacation Queueing Models. Theory and Applications; Springer: New York, NY, USA, 2006. [Google Scholar] [CrossRef]
- Yang, D.Y.; Wang, K.H.; Wu, C.H. Optimization and sensitivity analysis of controlling arrivals in the queueing system with single working vacation. J. Comput. Appl. Math. 2010, 234, 545–556. [Google Scholar] [CrossRef]
- Huang, H.I.; Hsu, P.C.; Ke, J.C. Controlling arrival and service of a two-removable-server system using genetic algorithm. Expert Syst. Appl. 2011, 38, 10054–10059. [Google Scholar] [CrossRef]
- Yang, D.Y.; Yang, N.C. Performance and cost analysis of a finite capacity queue with two heterogeneous servers under F-policy. Int. J. Serv. Oper. Inform. 2018, 9, 101–115. [Google Scholar] [CrossRef]
- Jain, M.; Kumari, S.; Qureshi, R.; Shankaran, R. Markovian multi-server queue with reneging and provision of additional removable servers. In Performance Prediction and Analytics of Fuzzy, Reliability and Queuing Models. Asset Analytics; Deep, K., Jain, M., Salhi, S., Eds.; Springer: Singapore, 2019; pp. 203–217. [Google Scholar] [CrossRef]
- Abdul Rasheed, K.V.; Manoharan, M. Markovian queueing system with discouraged arrivals and self-regulatory servers. Adv. Oper. Res. 2016, 2016, 2456135. [Google Scholar] [CrossRef]
- Jain, M.; Sanga, S.S. F-Policy for M/M/1/K retrial queueing model with state-dependent rates. In Performance Prediction and Analytics of Fuzzy, Reliability and Queuing Models. Asset Analytics; Deep, K., Jain, M., Salhi, S., Eds.; Springer: Singapore, 2019; pp. 127–138. [Google Scholar] [CrossRef]
- Kim, J.; Kim, B. A survey of retrial queueing systems. Ann. Oper. Res. 2016, 247, 3–36. [Google Scholar] [CrossRef]
- Artalejo, J.R.; Gómez-Corral, A. Retrial Queueing Systems. A Computational Approach; Springer: Berlin, Germany, 2008. [Google Scholar] [CrossRef]
- MacGregor Smith, J. System capacity and performance modelling of finite buffer queueing networks. Int. J. Prod. Res. 2014, 52, 3125–3163. [Google Scholar] [CrossRef]
- Pourvaziri, H.; Sarhadi, H.; Azad, N.; Afshari, H.; Taghavi, M. Planning of electric vehicle charging stations: An integrated deep learning and queueing theory approach. Transp. Res. Part E Logist. Transp. Rev. 2024, 186, 103568. [Google Scholar] [CrossRef]
- Cox, D.R.; Smith, W. Queues, 1st ed.; Chapman & Hall/CRC: New York, NY, USA, 1991. [Google Scholar] [CrossRef]
- Natvig, B. On the transient state probabilities for a queueing model where potential customers are discouraged by queue length. J. Appl. Probab. 1974, 11, 345–354. [Google Scholar] [CrossRef]



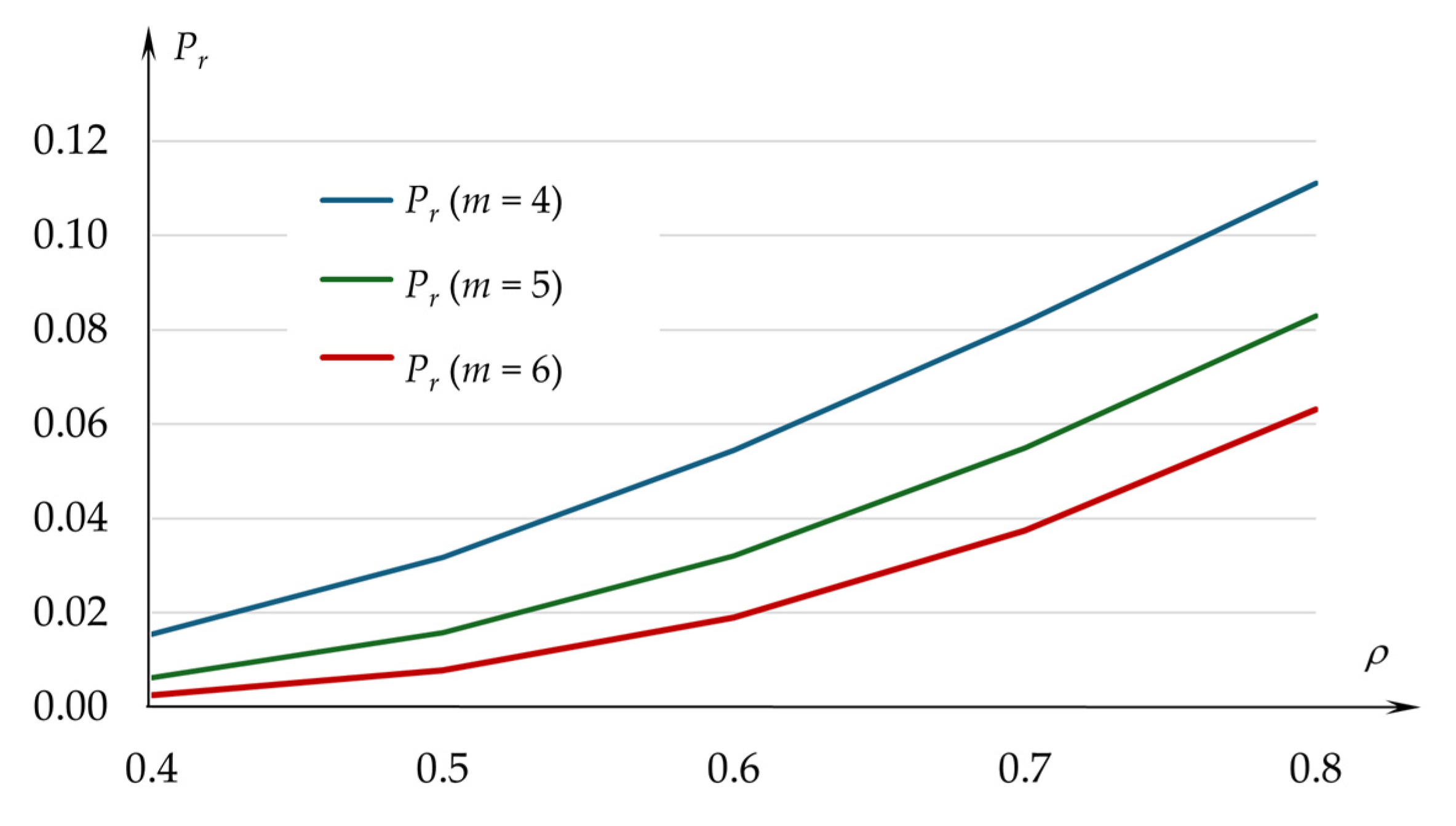
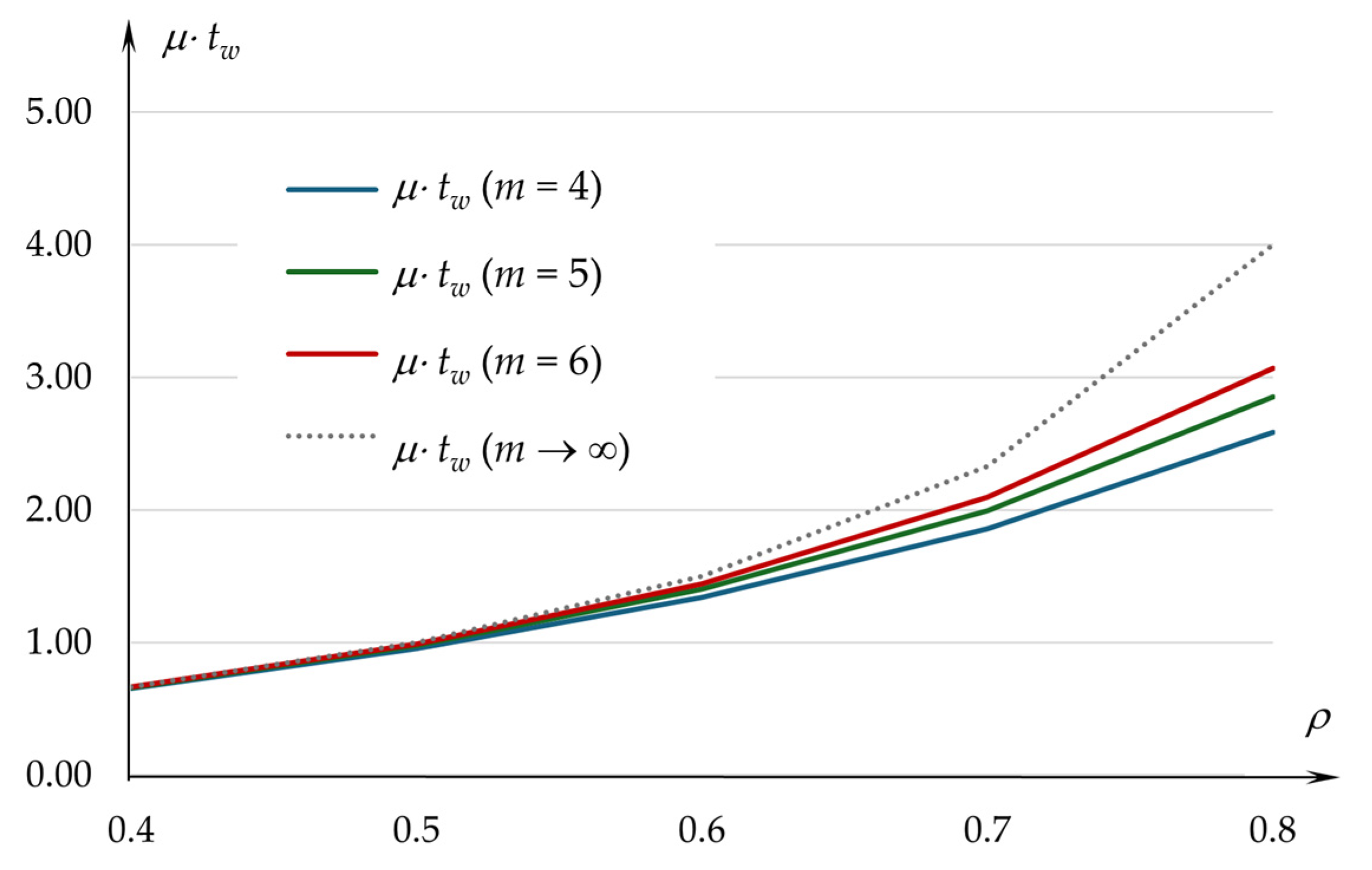
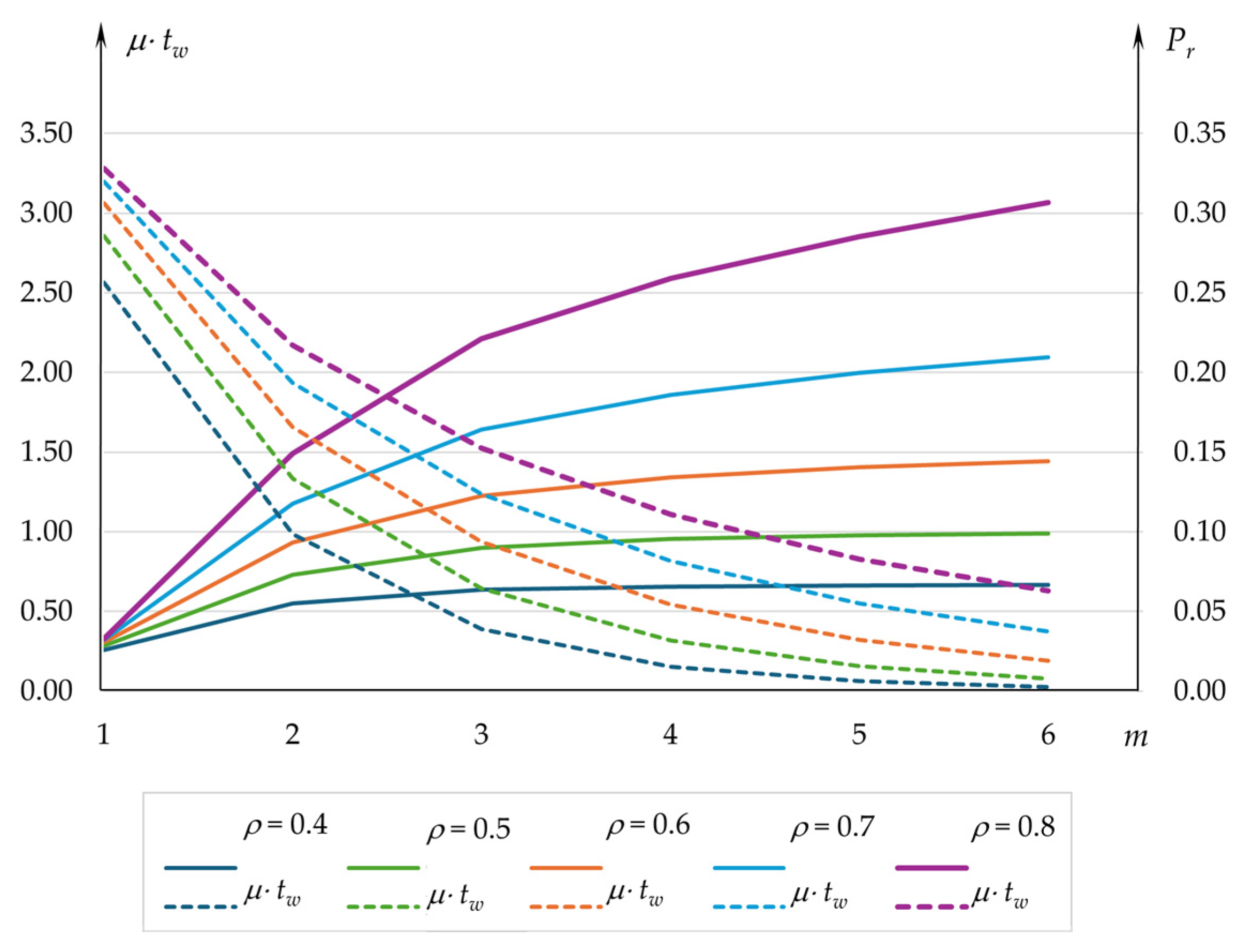

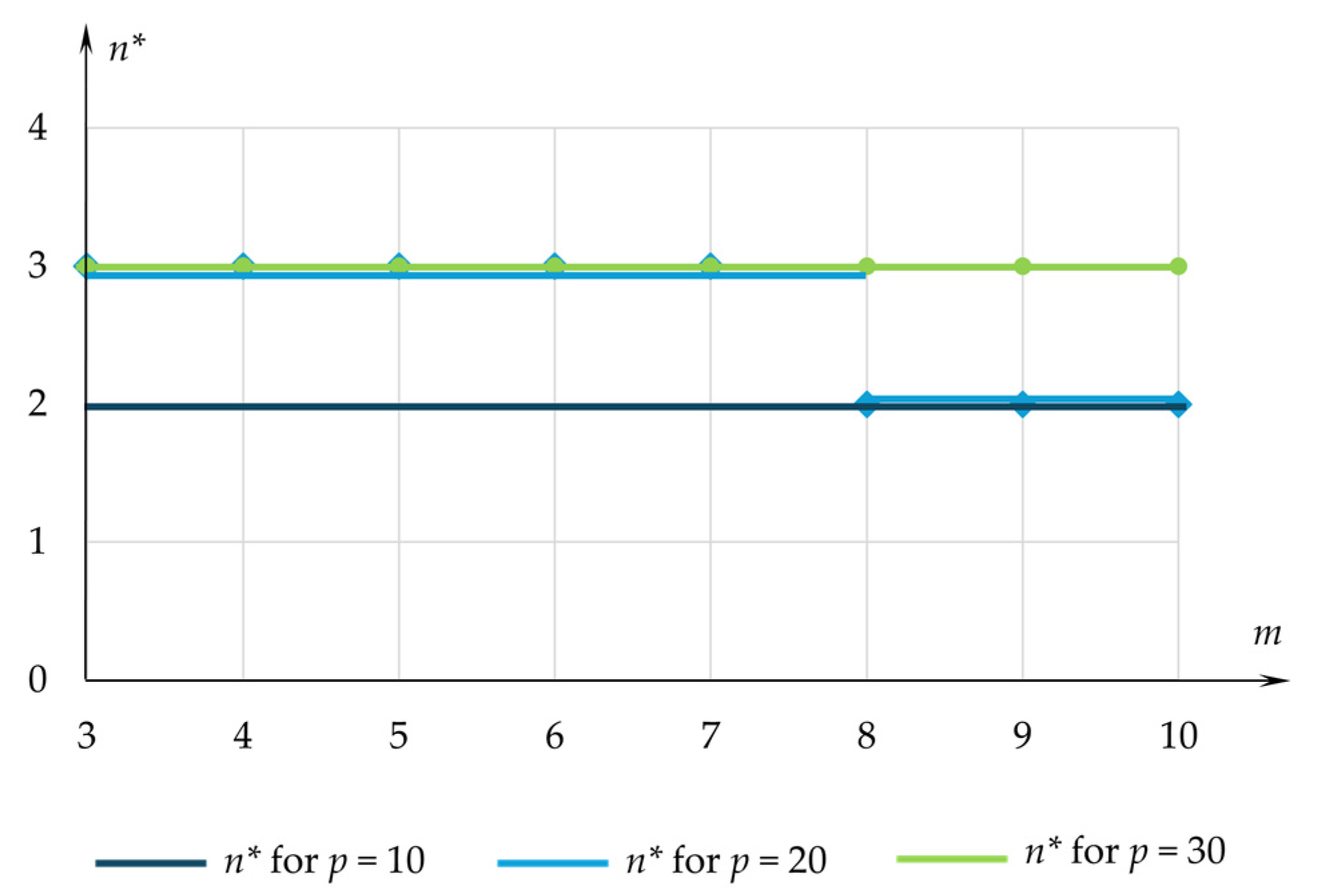
| n | Pr(n) | Pr(n) − Pr(n + 1) | Ratio (c/pλ) | Pr(n − 1) − Pr(n) |
|---|---|---|---|---|
| 1 | 0.5020 | - | - | - |
| 2 | 0.1177 | 0.1043 | 0.1875 | 0.3843 |
| 3 | 0.0134 | 0.0120 | 0.1875 | 0.1043 |
| 4 | 0.0014 | 0.0012 | 0.1875 | 0.0120 |
| 5 | 0.0001 | 0.0001 | 0.1875 | 0.0012 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Raicu, S.; Costescu, D.; Popa, M. Modelling Systems with a Finite-Capacity Queue: A Theoretical Investigation into the Design and Operation of Real Systems. AppliedMath 2025, 5, 17. https://doi.org/10.3390/appliedmath5010017
Raicu S, Costescu D, Popa M. Modelling Systems with a Finite-Capacity Queue: A Theoretical Investigation into the Design and Operation of Real Systems. AppliedMath. 2025; 5(1):17. https://doi.org/10.3390/appliedmath5010017
Chicago/Turabian StyleRaicu, Serban, Dorinela Costescu, and Mihaela Popa. 2025. "Modelling Systems with a Finite-Capacity Queue: A Theoretical Investigation into the Design and Operation of Real Systems" AppliedMath 5, no. 1: 17. https://doi.org/10.3390/appliedmath5010017
APA StyleRaicu, S., Costescu, D., & Popa, M. (2025). Modelling Systems with a Finite-Capacity Queue: A Theoretical Investigation into the Design and Operation of Real Systems. AppliedMath, 5(1), 17. https://doi.org/10.3390/appliedmath5010017









