On the Topological Structure of Nonlocal Continuum Field Theories
Abstract
1. Introduction
2. Preliminary Considerations
2.1. What Is Nonlocality?
2.2. Key Contributions and Motivations in the Present Work
- Infinitesimal interactions: this characterizes local field theories, e.g., local electromagnetism, where all operators are differential operators.
- Non-infinitesimal but local interactions: here, nonlocal operators, such as integral operators, may be present. In this type of theory, interactions are extended into small topological neighborhoods around the source/observation point.
2.3. An Outline of the Present Work
3. The Nonlocal Continuum Response Model
3.1. A Generic Nonlocal Response Model in Inhomogeneous Continua
On the other hand, if the medium is local, then the material response function can be written asIn nonlocal continuum field theories, knowledge of the field response at a specific point requires knowledge of the cause (excitation field) on an entire topological neighborhood set .
3.2. Spatial Dispersion in Homogeneous Nonlocal Material Domains
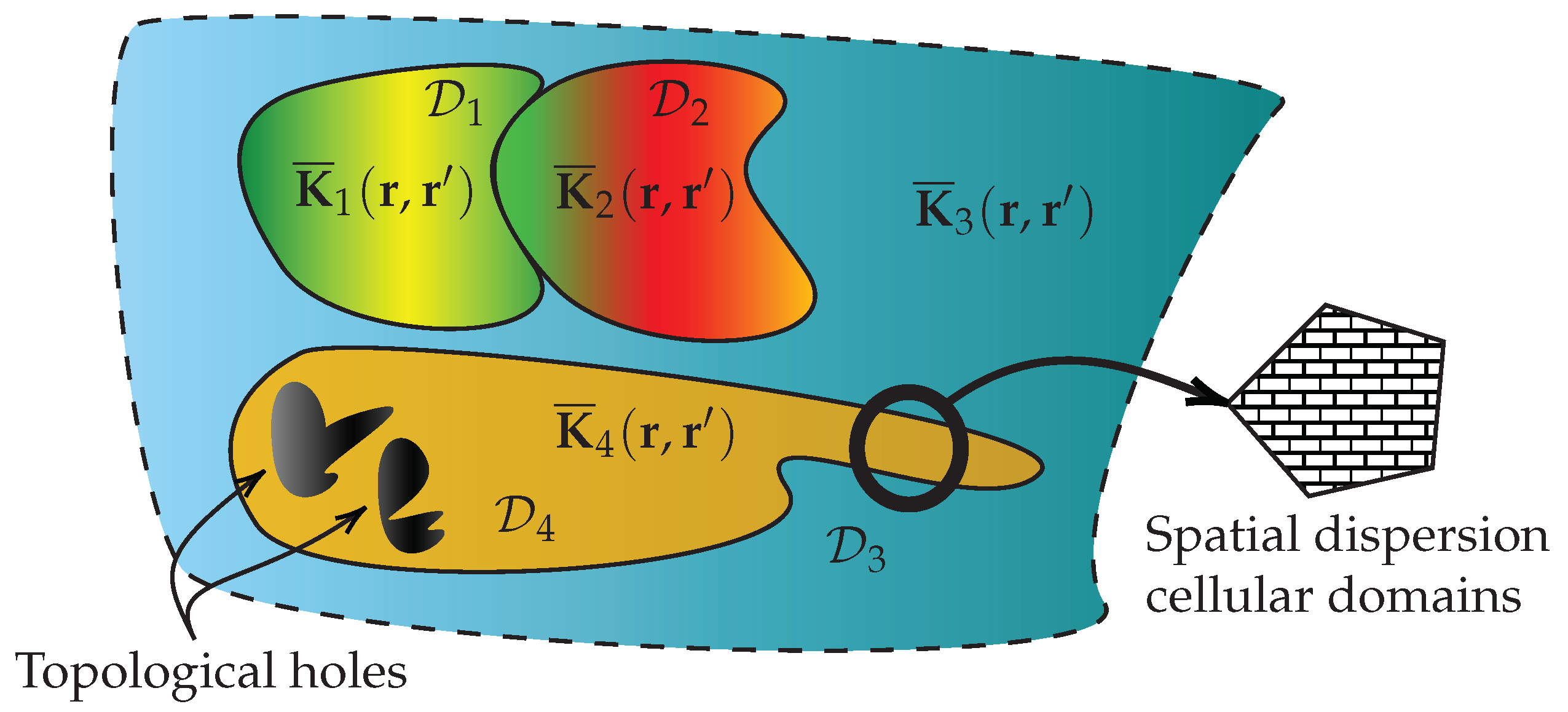
3.3. Preliminary Remarks on the Existence of Multiple Topological Scales in Nonlocal Continuum Field-Theoretic Structures
- The first is the geometrical separation between different nonlocal domains, such as and discussed in Section 3.2 and illustrated by Figure 1.
- The second is the case captured by the inset in the right hand side in Figure 1. Fine “microscopic” cells, each homogeneous and, hence, describable by a response function of the form , can be combined to build up a complex effective nonlocal response tensor over its topologically global domain . Such juxtaposition at the microscopically local level that effectively leads to the emergence of a global behavior is a classic example of multiscale physics. However, note that it even acquires a higher importance in the present context due to the fact that both of the constituent cell level (rectangular “bricks” in the inset of Figure 1) and the global domain level already belong to the physically, e.g., electromagnetically, nonlocal dimension of the relevant nonlocal continuum field theory.
- Finally, the third directly observable topological scale is that connected to what we termed “topological holes” in Figure 1. These are arbitrarily-shaped gaps, such as holes, vias, etchings, etc., which are intentionally introduced in order to influence the electromagnetic response by modifying the topology of the three-dimensional material manifolds .
- Physics-based local/non-local distinction: this is where basically physical considerations are at stakes. We distinguish between:
- (a)
- Physics-based non-local level: this includes how the response of the material continuum depends on locations not infinitesimally close to the point where the excitation field is applied. That is, is nonzero but it is also not a differential. (On infinitesimal domains, see Remark 1.)
- (b)
- Physics-based local level: this is the physical regime whose essence is captured by local constitutive relations of the form (15).
- Topology-based local/non-local distinction: mathematical considerations dominate at this level. We have:
- (a)
- Topology-based non-local level: this is the topologically global level, e.g., the entire topological manifold in contrast to the local description applicable only to a coordinate patch [57], and so on. At this level, the non-local-as-global is an emerging structure based on gluing together “smaller pieces” of the total manifold. We will see examples of processes occurring basically at this level when we use partition of unity methods.
- (b)
4. The Microscopic Topological Structure of Physics-Based Nonlocal Domains
4.1. Introduction
- Initially, in the present Section, we introduce the rudiments of the main physics-based microtopological structure associated with nonlocality in continuum field theories, but without delving into considerable mathematical details. The aim is to familiarize ourselves with the minimal necessary physical setting and how it naturally gives rise to a more refined picture of the nonlocal material domain compared with the traditional (and much simpler) topological structure of local electromagnetism based on spacetime points.
- In the second stage, treated in Section 5, a more careful mathematical picture is developed using the theory of topological fiber bundles. We eventually show (Section 5.3) that the physics-based (in this case the electromagnetic) nonlocal operator (20) can be reformulated as a Banach bundle map (homomorphism) over the three-dimensional space of the material domain under consideration. Some computational examples and applications are provided in the later Sections, e.g., see Section 7.
4.2. The Concept of Topological Microdomains in Nonlocal Continuum Field Theories
4.3. Construction of Excitation Field Function Spaces on the Topological Microdomains of Nonlocal Media
4.4. The Global Topological Structure of Nonlocal Electromagnetic Material Domains: First Look
- Each open domain in will by assigned a distribution of open sets , i.e., the physics-based nonlocality microdomains topology defined in Section 4.2, see in particular Definition 1 and Remark 5. Physically, it expresses the fine microtopological structure of nonlocal continua, e.g., electromagnetic material nonlocality.
- The structure is solely determined by the physics of field–matter interaction. A concrete example explicitly illustrating how the detailed physical content of the underlying process contributes to the construction of will be given in Section 7.
- We further emphasize that the various sets constitute an open cover of D, that is, we haveIn this way, the model can accommodate excitation fields applied at every point in .
- The decomposition of the material domain D into smaller building blocks exemplified by (32) is fundamental for computational topological models of nonlocal MTMs. For example, in Section 7 we will exploit this expansion in order to construct a topological coarse-grained model for inhomogeneous nonlocal semiconductor metamaterials.
- Finally, the topology induces the “function superspace” (30) defined as a class of function spaces , where each vector field acts on one microdomain element chosen from the topology .
4.5. A Reformulation of the Nonlocal Continuum Response Function
5. The Fiber Bundle Superspace Formalism in the Field Theory of Generic Nonlocal Continua
5.1. Preparatory Step: Promoting the Material Domain D to a Manifold
- It provides a natural and obvious generalization of the basic structure (31) from the mathematical perspective.
- Engineers often need to insert metamaterials into specific device settings, hence the shape of the material becomes highly restricted. It is therefore important to develop efficient tools to deal with variations of geometric and topological degrees of freedom and how they could possibly impact the design process.
- Applied scientists and engineers are often interested in deriving fundamental limitation on metamaterials, e.g., what are the ultimate allowable response–excitation relations or constitutive response functions possible given this material domain topology?
- Sophisticated full-wave electromagnetic numerical solvers prefer working with local coordinates in order to handle complicated shapes, even if a global coordinate system is sometimes available, making the deployment of the three-manifold structures for describing the material domain D useful.
- In topological photonics and materials [11], most applications seem to focus on lower-dimensional states of matter like those associated with quantum Hall effects and edge states (surface waves).14 There, new phenomena appear at material structures where the base space (material domain D) is a two-surface, which is best described mathematically as a differential two-manifold.
5.2. Attaching Fibers to Generic Points in the Nonlocal Material Manifold D
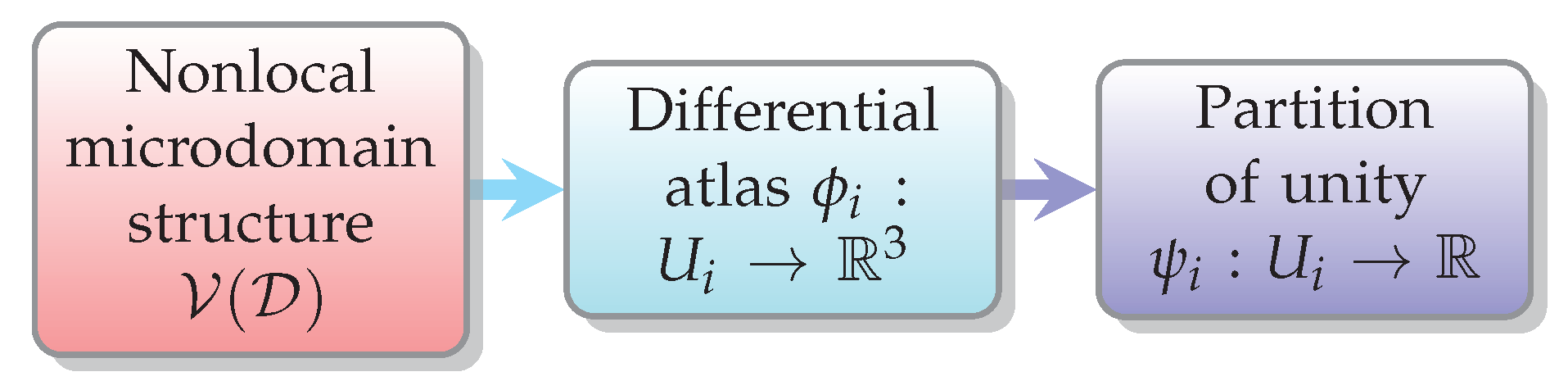
The above technical problem will be solved in Section 5.3 by using the technique of partition of unity borrowed from differential topology [26,57,62]. It will allow us to split up each full microdomain into several suitable sub-microdomains (details below), which can be later joined up together in order to give back the original EM nonlocality microdomain .Since the differential structure associated with chartscan be fixed by essentially mathematical considerations alone, while the collection of microdomainsis solely determined by the physics of electromagnetic nonlocality (See Remark 3 and Section 4), there is no direct and simple way to determine and express the vector transformationbecause several different coordinate patches other than and , belonging to the differential three-manifold atlas, might be involved in geometrically building up the microdomain .
- and each function is .16
- Since the open cover is locally finite, at each point , only a finite number of will intersect .
- Let the set of indices of those intersecting be . Then we require thatwhere the sum is always convergent because the set is finite.
- Initially, the physics-based collection of setsfor example, the EM nonlocal microdomain structure based on each point in the nonlocal metamaterial , is obtained using a suitable physical microscopic theory or some other procedure.17
- Introduce a differential atlason the smooth manifold subordinated to ) and representing the nonlocal material domain under consideration.
- Finally, the same atlas is linked to a set of functions (partition of unity) that can be recruited as “topological bases” in order to expand any differentiable field excitation function into sum of individual sub-fields defined on open subsets of the material domain (see Section 5.3).
- Step I: Construct a tailored fiber bundle based on the partition of unity charts introduced above.
- Step II: the original physical structure (31) is recovered by gluing together various sub-microdomain of each EM nonlocal microdomain .
5.3. Direct Construction of Bundle Homomorphism as Generalization of Linear Operators in Electromagnetic Theory
5.3.1. The Basic Definition of the Nonlocal Material, (or Continuum, Metamaterial (MTM), etc.), Banach (Fiber) Bundle Superspace
5.3.2. The Nonlocal Material Continuum Fiber Bundle Homomorphism
- The continuum itself is mathematically modeled as a Banach bundle superspace instead of its conventional differential manifold representation . The response of the medium is to be sought at some point .
- The bundle superspace encompasses an additional structure compared to , namely a distinct copy of a linear function space attached at each point . This is nothing but the fiber , which is a Banach space of functions defined on the region . This function space can be intuitively understood as a rigorous and exact model of the excitation field when the latter is restricted to (topologically localized at) the physics-based nonlocal domain .
- It should be noted that in local continuum field theory, e.g., conventional electromagnetism in normal temporally dispersive media, each one of the subdomains is essentially one point . Therefore, in the case of local continua, the excitation field F is there found to be preferably defined as acting on the conventional space instead of being a section of a Banach bundle superspace .
- A vector bundle homomorphism (to be formalized in Definition 3) will map one element of this fiber function space, namely, the particular excitation field to its value in the range vector bundle . For the case of electromagnetic field theory, the latter may be taken as a vector space fiber isomorphic to with a copy of this fiber attached to each .
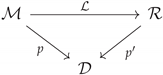
5.3.3. Computing Global Data Starting from Local Data
As mentioned before, it is the partition of unity , what will make this expansion of the topological formulation technically feasible.How can we extend the description of the nonlocal continuum’s response operators starting from excitation fields defined locally to excitation fields applied on the entire physical cluster of nonlocal microdomains ?
- Region ;
- Vector field acting on .
6. Interlude: The Nonlocal Continuum Fiber Bundle Superspace Algorithm—Summary and Transition to Applications
| Algorithm 1 The nonlocal continuum fiber bundle algorit |
|
7. Applications to Advanced Materials: Nonlocal Inhomogeneous Semiconductors
7.1. Introduction
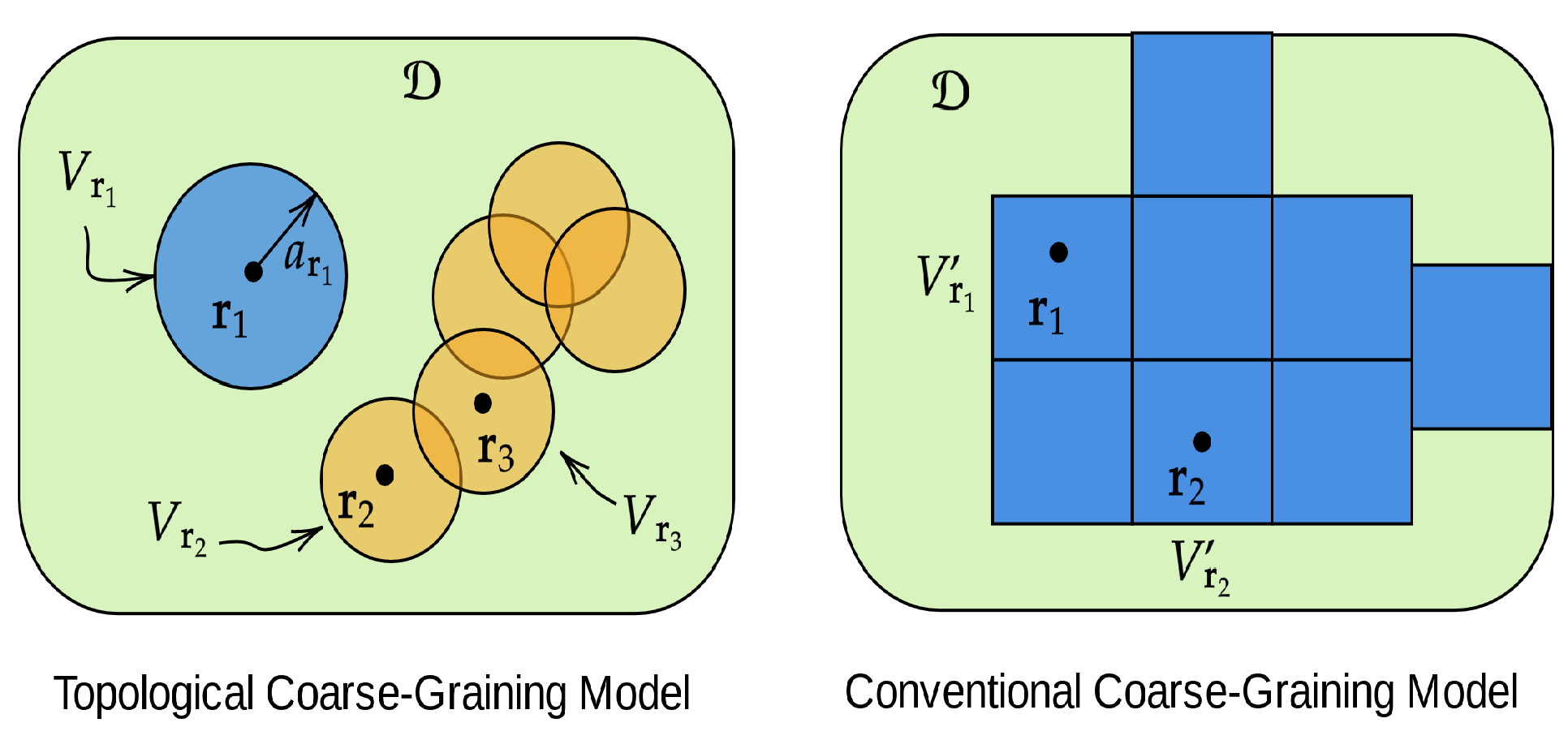
7.2. A Topological Coarse-Grained Model for Inhomogeneous Nonlocal Material Domains
- The proposed topological coarse-grained model utilizing the set of balls (left).
- The conventional paradigm where the unit cells are non-overlapping (right).
7.3. Resonant Nonlocal Semiconductor Domains and the Nonlocal Exciton-Polariton Model
7.4. Quantitative Estimation of the Electromagnetic Nonlocality Microdomain Structure in the Exciton-Polariton Dielectric Model
- Second, the presence of a spatially-decaying exponential factor of the form makes the Green function highly attenuating in spite of the fact that this attenuation is not mainly due to thermodynamic losses.
7.5. The Locally-Homogeneous Model of Nonlocal Semiconducting Domains
8. Application to Fundamental Theory: Electromagnetic Boundary Conditions in the Fiber Bundle Superspace Formalism
- The existence of extra or additional structures in the fiber bundle superspace approach to nonlocality in complex continua forces on us the need for introducing additional boundary conditions or information coming from the microscopic topological structure of the corresponding material superspaces.
- The fiber bundle superspace formalism of nonlocal metamaterials appears to be able to capture the intricate processes taking place inside and across various nonlocal material domains joined together through interfaces.
- This is achieved by providing an efficient apparatus to topologically encode some of rich and complex physics of field–matter interactions via the construction of appropriate infinite-dimensional function spaces (Banach space fibers) attached at each point of the materials’ base manifold.
- It is suggested that the relations between those additional fiber spaces are in fact what should be mainly taken into account while formulating boundary conditions for nonlocal continuum field theories, hence not merely the conventional relations involving only spatial interfaces between the material base manifolds as has been usually the practice in local field theories.
9. Conclusions
Funding
Conflicts of Interest
Appendix A
Appendix A.1. Survey of the Literature on Nonlocal Metamaterials
Appendix A.1.1. Introduction
Appendix A.1.2. Historically Important Examples
Appendix A.1.3. General Theories of Nonlocal Continua
Appendix A.1.4. Semiconductors, Metals, Plasma, Periodic Structures
Appendix A.1.5. Boundary Conditions in Nonlocal Metamaterials
Appendix A.1.6. Computational Techniques
Appendix A.1.7. Novel Systems and Devices with New Electromagnetic Behavior
Appendix A.1.8. Homogenization
Appendix A.1.9. Topological Materials and Photonics
Appendix A.2. On the History of Spatial Dispersion in Crystal and Plasma Physics
Appendix A.3. Some Further Engineering Applications of Nonlocal Metamaterials
Appendix A.3.1. Communications Systems and Information Transmission
Appendix A.3.2. Electromagnetic Metamaterials
Appendix A.3.3. Near-Field Engineering, Nonlocal Antennas, and Energy Applications
Appendix A.4. On the Concept of Superspace
Appendix A.5. Guide to the Mathematical Background
- Differential manifolds,
- Banach and Sobolev spaces,
- Vector bundles, and
- Partition of unity
Appendix A.5.1. Topology on Smooth Manifolds
Appendix A.5.2. Banach and Hilbert Spaces
Appendix A.5.3. Banach and Hilbert Manifolds
Appendix A.5.4. Sobolev Spaces
Appendix A.5.5. Vector Bundles
Appendix A.5.6. Additional Remarks on the Use of Sobolev Spaces in the Fiber Bundle Superspace Formalism
Appendix A.5.7. Partition of Unity Techniques
Appendix A.6. The General Electromagnetic Model of Nonlocal (Spatially-Dispersive) Isotropic Domains
- First, fundamental theory is deployed to derive analytical expressions for and .
- Afterwards, depending on the concrete values of the various physical parameters that enter into these expressions, e.g., frequency, temperature, molecular charge/mass/spin, density, etc., the obtained analytical expressions are expanded in power series with the proper number of terms.
- The expression of the dielectric tensor function is then put in the form of either a polynomial or rational polynomial in .
Appendix A.7. Origin of Electromagnetic Nonlocality in Excitonic Semiconductors
Appendix A.7.1. Review of the Semiconductor Physics of Excitons
- The electron mass must be replaced by the reduced exciton masswhere and are the electron and hole masses, respectively.
- Due to the screening of Coulomb attraction by the dielectric medium, the effective electron charge should be replaced by , where is the static dielectric constant.
Appendix A.7.2. Simple Explanation of How Nonlocality Emerges in the Excitonic Semiconductor
Appendix A.8. An Alternative Intuitive Derivation of the Dielectric Model (90) and the Quantum Origin of Nonlocality in Excitonic Semiconductors
Appendix A.9. Computation of the Inverse Fourier Transform (114)
Appendix A.10. On Extending Definition 1 to Noncompact Regions
Appendix A.11. Possible Applications of the Superspace Formalism to Fundamental Methods in Metamaterials Research
Appendix A.11.1. Estimating Fundamental Limitations on Nonlocal Metamaterials
Appendix A.11.2. Numerical Methods
- The ability to resolve the issue of generalized boundary condition (already discussed in Section 8).
- Since every point belonging to a fiber superspace is in itself a smooth function defined on an entire material sub-microdomain, by building a new system of discretized recursive equations approximating the behavior of electromagnetic solutions living in the enlarged superspaces and one may anticipate arriving at a deeper understanding of the physics of nonlocality. The reason is that the topology of the nonlocal interaction regime is explicitly encoded into the geometry of the new expanded solution superspace itself. Characterizing this geometry is then possible through a suitable discrete approximation of the interior microtopological content of the superspace (fiber bundle) structure itself; i.e., not just at the “exterior” parts often found in the boundary conditions of classical local field continuum theories, but also “going inside” the problem space as such.
- It is also possible that such numerical methods may emerge as more computationally efficient and broader in applicability than the conventional methods rooted in local electromagnetism. One reason for this is that the Banach vector bundle formulation introduced in this paper is quite natural and appears to reflect the underlying physics of nonlocal metamaterials in a direct manner. From our general experience in numerical methods, “natural operations” tend to translate into numerical methods with better convergence, sensitivity, and robustness.
Appendix A.11.3. Topological Photonics
| 1 | The author would like to thank one of the anonymous reviewers for suggesting this connection. |
| 2 | |
| 3 | See Appendix A.1 and Appendix A.2 for the literature review. |
| 4 | For a brief discussion of some possible engineering applications of metamaterials, see Section A.3. |
| 5 | Cf. Section 3.3. |
| 6 | If D asymptotically grows into an unbounded region, then the problem reduces to that of homogeneous unbounded domain (bulk media), well treated in the basic literature on spatial dispersion. Clearly, in this paper, we are not interested in such a topologically trivial problem. |
| 7 | Cf. Remark 3. |
| 8 | |
| 9 | This compactness of the response kernel’s support cannot be proved in general, but is very plausible on physical grounds (causality considerations). Therefore, we posit such compactness as an axiomatic feature of all physically-realizable causal nonlocal continuum field theories. However, see Appendix A.10. |
| 10 | |
| 11 | In this section and the one to follow, we do not worry much about the details of the electromagnetic model and for simplicity assume that only one vector field acts as excitation and one response field is induced. More complex media like bi-anisotropic domains and others [67] may also be treated within this formulation. For example, if two response fields are needed, the codomain in (33) can be simply changed to . |
| 12 | Cf. Remark 3. |
| 13 | See the discussion of nonlocal and topological metamaterials applications in Appendix A.3. |
| 14 | Cf. Appendix A.1. |
| 15 | See [59,68] for the full technical definition of subordinated cover. A collection of subsets of a topological space is said to be locally finite, if each point in the space has a neighborhood that intersects only finitely many of the sets in the collection. What we need here is that there exists some i and such that is inside , i.e., where . |
| 16 | |
| 17 | See Section 7 for one possible method and examples. |
| 18 | Cf. Remark 3. |
| 19 | The discretization of the nonlocal MTM bundle homomorphism itself is outside the scope of the present work and will be addressed elsewhere. |
| 20 | |
| 21 | For instance, by introducing holes into a simply-connected domain in order to make the latter disconnected. |
| 22 | The numerical value of may be different for transverse and longitudinal excitation fields. |
| 23 | In the effective-mass approximation, a simple way to estimate the exciton mass is via the relation , i.e., the sum of the effective electron and hole masses introduced in Appendix A.7. However, it must be noted that this relation is far from being universal, e.g., it should be modified when there are strong interactions [81,82] |
| 24 | |
| 25 | It should be noted that there is no loss of generality here. The computational model to be presented shortly allows the estimation of the nonlocal microdomain topology based on a generic model of the form (90). If and are not identical, then the same procedure can be applied to each one of them separately. |
| 26 | There is a nice parallelism here with temporal dispersion where the latter is known to arise from the inertial effects of electrons in interaction with radiation fields [37]. |
| 27 | This is more obvious in FEM or FDTD than MoM. |
| 28 | Cf. Section 5.2. |
| 29 | Cf. Section 8. |
| 30 | |
| 31 | Cf. Section 8 and Appendix A.1. |
References
- Schwarz, A.S. Topology for Physicists; Springer: Berlin, Germany; New York, NY, USA,, 1994. [Google Scholar]
- Monastyrskiĭ, M.I. Riemann, Topology and Physics; Birkháuser: Boston, MA, USA, 1999. [Google Scholar]
- Penrose, R. Techniques of Differential Topology in Relativity; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 1972. [Google Scholar]
- Mikki, S. Topological circuit theory: A Lie group perspective. Prog. Electromagn. Res. B 2020, 89, 133–156. [Google Scholar] [CrossRef]
- Weyl, H. The Theory of Groups and Quantum Mechanics; Martino Publishing: Mansfield Centre, CT, USA, 2014. [Google Scholar]
- Poincaré, H. New Methods of Celestial Mechanics; American Institute of Physics: Woodbury, NY, USA, 1993. [Google Scholar]
- Poincare, H. Mémoire sur les courbes définies par une équation différentielle I-IV. J. Mathématiques Pures Appliqués 1881, 7, 375–422. [Google Scholar]
- Eringen, A. Nonlocal Continuum Field Theories; Springer: New York, NY, USA, 2002. [Google Scholar]
- Fabrizio, M.; Morro, A. Electromagnetism of Continuous Media: Mathematical Modelling and Applications; Oxford University Press: Oxford, UK, 2003. [Google Scholar]
- Hasan, M.Z.; Kane, C.L. Colloquium: Topological insulators. Rev. Mod. Phys. 2010, 82, 3045–3067. [Google Scholar] [CrossRef]
- Ozawa, T.; Price, H.M.; Amo, A.; Goldman, N.; Hafezi, M.; Lu, L.; Rechtsman, M.C.; Schuster, D.; Simon, J.; Zilberberg, O.; et al. Topological photonics. Rev. Mod. Phys. 2019, 91, 015006. [Google Scholar] [CrossRef]
- Schwinger, J.; Deraad, L.L.; Milton, K.A.; Tsai, W.Y. Classical Electrodynamics; Perseus Books: New York, NY, USA, 1998. [Google Scholar]
- Landau, L.D.; Lifshitz, E. Electrodynamics of Continuous Media; Butterworth-Heinemann: Oxford, UK, 1984. [Google Scholar]
- Ginzburg, V.L. The Propagation of Electromagnetic Waves in Plasmas; Pergamon Press: Oxford, UK; New York, NY, USA, 1970. [Google Scholar]
- Ginzburg, V.L. Theoretical Physics and Astrophysics; Pergamon Press: Oxford, UK, 1979. [Google Scholar]
- Agranovich, V.; Ginzburg, V. Crystal Optics with Spatial Dispersion, and Excitons; Springer: Berlin/Heidelberg, Germany, 1984. [Google Scholar]
- Deppman, A.; Megías, E.; P. Menezes, D. Fractal Structures of Yang–Mills Fields and Non-Extensive Statistics: Applications to High Energy Physics. Physics 2020, 2, 455–480. [Google Scholar] [CrossRef]
- Auyang, S. How Is Quantum Field Theory Possible; Oxford University Press: New York, NY, USA, 1995. [Google Scholar]
- Coleman, S. Quantum Field Theory: Lectures of Sidney Coleman; World Scientific: Singapore, 2019. [Google Scholar]
- Espagnat, B. On Physics and philosophy; Princeton University Press: Princeton, NJ, USA, 2013. [Google Scholar]
- Frisch, M. Non-Locality in Classical Electrodynamics. Br. J. Philos. Sci. 2002, 53, 1–19. [Google Scholar] [CrossRef]
- Bernabeu, J.; Navarro-Salas, J. A Non-Local Action for Electrodynamics: Duality Symmetry and the Aharonov-Bohm Effect, Revisited. Symmetry 2019, 11, 1191. [Google Scholar] [CrossRef]
- Mashhoon, B. Toward Nonlocal Electrodynamics of Accelerated Systems. Universe 2020, 6, 229. [Google Scholar] [CrossRef]
- Cvijanovich, G.B. Classical electrodynamics with nonlocal constitutive equations. Found. Phys. 1977, 7, 785–799. [Google Scholar] [CrossRef]
- Mikki, S. Proca Metamaterials, Massive Electromagnetism, and Spatial Dispersion. Ann. Der Phys. 2021, 533, 2000625. [Google Scholar] [CrossRef]
- Lang, S. Introduction to Differentiable Manifolds; Interscience: New York, NY, USA, 1962. [Google Scholar]
- Colton, D.; Kress, R. Inverse Acoustic and Electromagnetic Scattering Theory; Springer: Cham, Switzerland, 2019. [Google Scholar]
- Chew, W.C. Waves and Fields in Inhomogenous Media; Wiley-IEEE: Piscataway, NJ, USA, 1999. [Google Scholar]
- Nedelec, J.C. Acoustic and Electromagnetic Equations: Integral Representations for Harmonic Problems; Springer: New York, NY, USA, 2001. [Google Scholar]
- Zeidler, E. Quantum Field Theory I: Basics in Mathematics and Physics; Springer: New York, NY, USA, 2009. [Google Scholar]
- Hassani, S. Mathematical Physics: A Modern Introduction to Its Foundations; Springer: Cham, Switzerland, 2013. [Google Scholar]
- Mikki, S.; Antar, Y. New Foundations for Applied Electromagnetics: The Spatial Structure of Fields; Artech House: London, UK, 2016. [Google Scholar]
- Mikki, S.; Antar, Y. The antenna current Green’s function formalism–Part I. IEEE Trans. Antennas Propagat 2013, 9, 4493–4504. [Google Scholar] [CrossRef]
- Mikki, S.; Antar, Y. The antenna current Green’s function formalism—Part II. IEEE Trans. Antennas Propagat 2013, 9, 4505–4519. [Google Scholar] [CrossRef]
- Mikki, S. The Antenna Spacetime System Theory of Wireless Communications. Proc. R. Soc. Math. Phys. Eng. Sci. 2019, 475, 2224. [Google Scholar] [CrossRef]
- Mikki, S.; Antar, Y. A rigorous approach to mutual coupling in general antenna systems through perturbation theory. IEEE Antennas Wirel. Commun. Lett. 2015, 14, 115–118. [Google Scholar] [CrossRef]
- Ilinskii, Y.A.; Keldysh, L. Electromagnetic Response of Material Media; Springer Science Business Media: New York, NY, USA, 1994. [Google Scholar]
- Keller, O. Quantum Theory of Near-Field Electrodynamics; Springer: Berlin, Germany, 2011. [Google Scholar]
- Melrose, D.B.; McPhedran, R.C. Electromagnetic Processes in Dispersive Media: A Treatment Based on the Dielectric Tensor; Cambridge University Press: Cambridge, UK; New York, NY, USA, 1991. [Google Scholar]
- Felsen, L. Radiation and Scattering of Waves; IEEE Press: Piscataway, NJ, USA, 1994. [Google Scholar]
- Geyi, W. Foundations of Applied Electrodynamics; Wiley: Hoboken, NJ, USA, 2010. [Google Scholar]
- Melrose, D.B. Instabilities in Space and Laboratory Plasmas; Cambridge University Press: Cambridge, UK; New York, NY, USA, 1986. [Google Scholar]
- Jackson, J. Classical Electrodynamics; Wiley: New York, NY, USA, 1999. [Google Scholar]
- Jin, J. The Finite Element Method in Electromagnetics; John Wiley & Sons Inc: Hoboken, NJ, USA, 2014. [Google Scholar]
- Taflove, A. Computational Electrodynamics: The Finite-Difference Time-Domain Method; Artech House: Boston, MA, USA, 2005. [Google Scholar]
- Gibson, W.C. The Method of Moments in Electromagnetics; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Mikki, S.; Kishk, A. Nonlocal Electromagnetic Media: A Paradigm for Material Engineering. In Passive Microwave Components and Antennas; InTech: Nappanee, IN, USA, 2010. [Google Scholar]
- Agranovich, V.M.; Shen, Y.R.; Baughman, R.H.; Zakhidov, A.A. Linear and nonlinear wave propagation in negative refraction metamaterials. Phys. Rev. B 2004, 69, 165112. [Google Scholar] [CrossRef]
- Agranovich, V.M.; Gartstein, Y.N.; Zakhidov, A.A. Negative refraction in gyrotropic media. Phys. Rev. B 2006, 73, 045114. [Google Scholar] [CrossRef]
- Cho, K. Optical Response of Nanostructures: Microscopic Nonlocal Theory; Springer: Berlin, Germany; New York, NY, USA, 2003. [Google Scholar]
- Cho, K. A single susceptibility scheme of macroscopic Maxwell equations: Beyond the ‘E,D,B,H’ approach. J. Phys. Condens. Matter 2008, 20, 175202. [Google Scholar] [CrossRef]
- Mikki, S.; Kishk, A. Electromagnetic Wave Propagation in Nonlocal Media: Negative Group Velocity and Beyond. Prog. Electromagn. Res. B 2009, 14, 149–174. [Google Scholar] [CrossRef]
- Cho, K. Reconstruction of Macroscopic Maxwell Equations: A Single Susceptibility Theory; Springer: Berlin, Germany, 2018. [Google Scholar]
- Toyozawa, Y. Optical Processes in Solids; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2003. [Google Scholar]
- Pekar, S.I. Crystal Optics and Additional Light Waves; Benjamin/Cummings Pub. Co: Menlo Park, CA, USA, 1983. [Google Scholar]
- Churchill, R.J.; Philbin, T.G. Electromagnetic reflection, transmission, and energy density at boundaries of nonlocal media. Phys. Rev. B 2016, 94, 235422. [Google Scholar] [CrossRef]
- Lee, J. Introduction to Smooth Manifolds; Springer: New York, NY, USA; London, UK, 2012. [Google Scholar]
- Hausdorff, F. Set Theory; American Mathematical Society: Providence, RI, USA, 2005. [Google Scholar]
- Kelley, J. General Topology; Dover Publications, Inc.: New York, NY, USA, 2017. [Google Scholar]
- Baker, A. Matrix Groups: An Introduction to Lie Group THEORY; Springer: London, UK; New York, NY, USA, 2002. [Google Scholar]
- Horn, R.; Johnson, C.R. Matrix Analysis; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2012. [Google Scholar]
- Hirsch, M. Differential Topology; Springer: New York, NY, USA, 1976. [Google Scholar]
- Dundas, B.I. A Short Course in Differential Topology; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2018. [Google Scholar]
- Showalter, R.E. Hilbert space methods in partial differential equations; Dover Publications: Mineola, NY, USA, 2010. [Google Scholar]
- Zeidler, E. Applied Functional Analysis: Applications to Mathematical Physics; Springer: Berlin/Heidelberg, Germany, 1995. [Google Scholar]
- Hebey, E. Nonlinear Analysis on Manifolds: Sobolev Spaces and Inequalities; Courant Institute of Mathematical Sciences American Mathematical Society: New York, NY, USA, 2000. [Google Scholar]
- Mackay, T.; Lakhtakia, A. Electromagnetic Anisotropy and Bianisotropy: A Field Guide; World Scientific Publishing Co. Pte. Ltd: Singapore; Hackensack, NJ, USA, 2019. [Google Scholar]
- Mukherjee, A. Differential Topology; Birkháuser: Cham, Switzerland, 2015. [Google Scholar]
- Appel, W. Mathematics for Physics and Physicists; Princeton University Press: Princeton, NJ, USA, 2007. [Google Scholar]
- Kahn, D. Introduction to Global Analysis; Dover Publications: Mineola, NY, USA, 2007. [Google Scholar]
- Nestruev, J. Smooth Manifolds and Observables; Springer: Cham, Switzerland, 2020. [Google Scholar]
- Edelsbrunner, H. Computational Topology: An Introduction; American Mathematical Society: Providence, RI, USA, 2010. [Google Scholar]
- Guillemin, V.; Pollack, A. Differential Topology; AMS Chelsea Pub.: Providence, RI, USA, 2010. [Google Scholar]
- Majthay, A. Foundations of Catastrophe Theory; Pitman Advanced Pub. Program: Boston, MA, USA, 1985. [Google Scholar]
- Strang, G.; Fix, G. An Analysis of the Finite Element Method; Wellesley-Cambridge Press: Wellesley, MA, USA, 2008. [Google Scholar]
- Kushnir, O.S. Spatial dispersion in incommensurately modulated insulators. J. Physics: Condens. Matter 2004, 16, 1245–1267. [Google Scholar] [CrossRef]
- Ginzburg, V. Electromagnetic Waves in Isotropic and Crystalline Media Characterized by Dielectric Permittivity with Spatial Dispersion. JETP 1958, 7, 1096. [Google Scholar]
- Hopfield, J.J. Theory of the Contribution of Excitons to the Complex Dielectric Constant of Crystals. Phys. Rev. 1958, 112, 1555–1567. [Google Scholar] [CrossRef]
- Hopfield, J.J.; Thomas, D.G. Theoretical and Experimental Effects of Spatial Dispersion on the Optical Properties of Crystals. Phys. Rev. 1963, 132, 563–572. [Google Scholar] [CrossRef]
- Forstmann, F. Optics in spatially dispersive media: The energy theorem and the boundary conditions. Z. Phys. B Condens. Matter Quanta 1979, 32, 385–393. [Google Scholar] [CrossRef]
- Mattis, D.C.; Gallinar, J.P. What is the Mass of an Exciton? Phys. Rev. Lett. 1984, 53, 1391–1393. [Google Scholar] [CrossRef]
- Davydov, A.; Enolskii, V. Effective mass of the Pekar polaron. JETP 1988, 67, 313. [Google Scholar]
- Halevi, P. Spatial Dispersion in Solids and Plasmas; North-Holland: Amsterdam, The Netherlands; New York, NY, USA, 1992. [Google Scholar]
- Frenkel, J. On the Transformation of light into Heat in Solids—Part I. Phys. Rev. 1931, 37, 17–44. [Google Scholar] [CrossRef]
- Frenkel, J. On the Transformation of Light into Heat in Solids—Part II. Phys. Rev. 1931, 37, 1276–1294. [Google Scholar] [CrossRef]
- Davydov, A.S. Theory of Molecular Excitons; Plenum Press: New York, NY, USA, 1971. [Google Scholar]
- Balakin, A.A.; Gospodchikov, E.D. Operator formalism for permittivity tensor in smoothly inhomogeneous media with spatial dispersion. J. Phys. B At. Mol. Opt. Phys. 2015, 48, 215701. [Google Scholar] [CrossRef]
- Balakin, A.A.; Balakina, M.A.; Permitin, G.V.; Smirnov, A.I. Wave beams in inhomogeneous anisotropic and gyrotropic media. Radiophys. Quantum Electron. 2007, 50, 955–972. [Google Scholar] [CrossRef]
- Mikki, S.; Kishk, A. A Symmetry-Based Formalism for the Electrodynamics of Nanotubes. Prog. Electromagn. Res. 2008, 86, 111–134. [Google Scholar] [CrossRef]
- Mikki, S.M.; Kishk, A.A. Exact derivation of the Dyadic Green’s functions of carbon nanotubes using microscopic theory. In Proceedings of the 2007 IEEE Antennas and Propagation Society International Symposium, Honolulu, HI, USA, 9–15 June 2007; pp. 4332–4335. [Google Scholar] [CrossRef]
- Mikki, S.M.; Kishk, A.A. Electrodynamics of low-dimensional structures: Lattice dynamics formalism for carbon nanotubes. In Proceedings of the 2008 IEEE Antennas and Propagation Society International Symposium, San Diego, CA, USA, 5–11 July 2008; pp. 1–4. [Google Scholar] [CrossRef]
- Mikki, S.; Kishk, A. Derivation of the Carbon Nanotube Susceptibility Tensor Using Lattice Dynamics Formalism. Prog. Electromagn. Res. B 2008, 9, 1–26. [Google Scholar] [CrossRef]
- Kushnir, O.S.; Lokot, L.O. Features of the optical response of dielectric crystals with incommensurate phases. Phys. Solid State 2001, 43, 818–822. [Google Scholar] [CrossRef]
- Agranovich, V.M.; Gartstein, Y. Spatial dispersion and negative refraction of light. Physics-Uspekhi 2006, 49, 1029. [Google Scholar] [CrossRef]
- Qi, G. Optical Beams in Media with Spatial Dispersion. Chin. Phys. Lett. 2002, 20, 64–67. [Google Scholar] [CrossRef]
- Altland, A.; Simmons, B. Condensed Matter Field Theory; Cambridge University Press: Leiden, The Netherlands, 2010. [Google Scholar]
- Morro, A. Optical activity and spatial dispersion. Phys. Rev. E 1997, 56, 1124–1128. [Google Scholar] [CrossRef]
- Agranovich, V.; Yudson, V. On phenomenological electrodynamics of gyrotropic media. Opt. Commun. 1973, 9, 58–60. [Google Scholar] [CrossRef]
- Trigger, S.A. Equilibrium radiation in a plasma medium with spatial and frequency dispersion. Phys. Scr. 2020, 95, 075504. [Google Scholar] [CrossRef]
- Esquivel-Sirvent, R.; Villarreal, C.; Mochán, W.L.; Contreras-Reyes, A.M.; Svetovoy, V.B. Spatial dispersion in Casimir forces: A brief review. J. Phys. A Math. Gen. 2006, 39, 6323–6331. [Google Scholar] [CrossRef]
- Leung, P.T.; Chang, R. Reciprocity in nonlocal nano-optics. J. Opt. A Pure Appl. Opt. 2008, 10, 075201. [Google Scholar] [CrossRef][Green Version]
- Xie, H.Y.; Leung, P.T.; Tsai, D.P. General proof of optical reciprocity for nonlocal electrodynamics. J. Phys. A Math. Theor. 2008, 42, 045402. [Google Scholar] [CrossRef]
- Xie, H.Y.; Leung, P.T.; Tsai, D.P. Reciprocity theorem for nonlocal optics: Completion of proof and application to spectroscopic analysis. J. Opt. 2010, 12, 035006. [Google Scholar] [CrossRef]
- Bareev, D.D.; Gavrilenko, V.G. On the Lorentz lemma for media with spatial dispersion and its application to calculation of the radiation fields of given sources. Radiophys. Quantum Electron. 2009, 52, 735–739. [Google Scholar] [CrossRef]
- Tokman, M.; Westerhof, E.; Gavrilova, M. Wave power balance in resonant dissipative media with spatial and temporal dispersion. Nucl. Fusion 2003, 43, 1295–1304. [Google Scholar] [CrossRef]
- Amooshahi, M.; Kheirandish, F. Electromagnetic field quantization in an anisotropic magnetodielectric medium with spatial–temporal dispersion. J. Phys. A Math. Theor. 2008, 41, 275402. [Google Scholar] [CrossRef]
- Buhmann, S.Y.; Butcher, D.T.; Scheel, S. Macroscopic quantum electrodynamics in nonlocal and nonreciprocal media. New J. Phys. 2012, 14, 083034. [Google Scholar] [CrossRef]
- Horsley, S.A.R.; Philbin, T.G. Canonical quantization of electromagnetism in spatially dispersive media. New J. Phys. 2014, 16, 013030. [Google Scholar] [CrossRef][Green Version]
- Nastyshyn, S.Y.; Bolesta, I.M.; Tsybulia, S.A.; Lychkovskyy, E.; Fedorovych, Z.Y.; Khaustov, D.Y.; Ryzhov, Y.; Vankevych, P.I.; Nastishin, Y.A. Optical spatial dispersion in terms of Jones calculus. Phys. Rev. A 2019, 100, 013806. [Google Scholar] [CrossRef]
- Raza, S.; Bozhevolnyi, S.I.; Wubs, M.; Mortensen, N.A. Nonlocal optical response in metallic nanostructures. J. Phys. Condens. Matter 2015, 27, 183204. [Google Scholar] [CrossRef]
- Adolph, B.; Gavrilenko, V.I.; Tenelsen, K.; Bechstedt, F.; Del Sole, R. Nonlocality and many-body effects in the optical properties of semiconductors. Phys. Rev. B 1996, 53, 9797–9808. [Google Scholar] [CrossRef]
- Dommermuth, M.; Schopohl, N. On the theory of light propagation in crystalline dielectrics. J. Phys. Commun. 2018, 2, 075012. [Google Scholar] [CrossRef]
- Brillouin, L. Wave Propagation in Periodic Structures, Electric Filters and Crystal Lattices; Dover Publications: New York, NY, USA, 1953. [Google Scholar]
- Mikki, S.; Kishk, A. An Efficient Algorithm for the Analysis and Design of Carbon Nanotube Photonic Crystals. Prog. Electromagn. Res. C 2018, 83, 83–96. [Google Scholar] [CrossRef][Green Version]
- Mechelen, T.V.; Jacob, Z. Nonlocal topological electromagnetic phases of matter. Phys. Rev. B 2019, 99, 205146. [Google Scholar] [CrossRef]
- Born, M.; Huang, K. Dynamical theory of crystal lattices; Clarendon Press Oxford University Press: Oxford, UK; New York, NY, USA, 1988. [Google Scholar]
- Silveirinha, M.G. Additional boundary conditions for nonconnected wire media. New J. Phys. 2009, 11, 113016. [Google Scholar] [CrossRef]
- Agarwal, G.S.; Pattanayak, D.N.; Wolf, E. Electromagnetic fields in spatially dispersive media. Phys. Rev. B 1974, 10, 1447–1475. [Google Scholar] [CrossRef]
- Maradudin, A.A.; Mills, D.L. Effect of Spatial Dispersion on the Properties of a Semi-Infinite Dielectric. Phys. Rev. B 1973, 7, 2787–2810. [Google Scholar] [CrossRef]
- Fang, M.; Huang, Z.X.; Sha, W.E.I.; Xiong, X.Y.Z.; Wu, X.L. Full hydrodynamic model of nonlinear electromagnetic response in metallic metamaterials (Invited Paper). Prog. Electromagn. Res. 2016, 157, 63–78. [Google Scholar] [CrossRef]
- Galynsky, V.M.; Furs, A.N.; Barkovsky, L.M. Integral formalism for surface electromagnetic waves in bianisotropic media. J. Phys. Math. Gen. 2004, 37, 5083–5096. [Google Scholar] [CrossRef]
- Forestiere, C.; Capretti, A.; Miano, G. Surface integral method for second harmonic generation in metal nanoparticles including both local-surface and nonlocal-bulk sources. J. Opt. Soc. Am. B 2013, 30, 2355–2364. [Google Scholar] [CrossRef]
- Mnasri, K.; Khrabustovskyi, A.; Plum, M.; Rockstuhl, C. Retrieving effective material parameters of metamaterials characterized by nonlocal constitutive relations. Phys. Rev. B 2019, 99, 035442. [Google Scholar] [CrossRef]
- Vallejos, P.; Johnson, T.; Hellsten, T. An iterative method to include spatial dispersion for waves in nonuniform plasmas using wavelet decomposition. J. Phys. Conf. Ser. 2016, 775, 012016. [Google Scholar] [CrossRef]
- Vallejos, P.; Hellsten, T.; Jonsson, T. A numerical tool based on FEM and wavelets to account for spatial dispersion in ICRH simulations. J. Phys. Conf. Ser. 2018, 1125, 012020. [Google Scholar] [CrossRef]
- JiSheng, C.; JiaRong, L. The Boundary Value Problem and the Nonlinear Spatial Dispersion in Collisionless Quark-Gluon Plasma. Commun. Theor. Phys. 2000, 34, 251–260. [Google Scholar] [CrossRef]
- Musienko, T.; Rudakov, V.; Solov’ev, L. On the application of Kramers-Kronig relations to media with spatial dispersion. J. Phys. Condens. Matter 1989, 1, 6745–6753. [Google Scholar] [CrossRef]
- Goffi, F.Z.; Mnasri, K.; Plum, M.; Rockstuhl, C.; Khrabustovskyi, A. Towards more general constitutive relations for metamaterials: A checklist for consistent formulations. Phys. Rev. B 2020, 101, 195411. [Google Scholar] [CrossRef]
- Mikki, S.; Antar, Y. On electromagnetic radiation in nonlocal environments: Steps toward a theory of near field engineering. In Proceedings of the 2015 9th European Conference on Antennas and Propagation (EuCAP), Lisbon, Portugal, 13–17 April 2015; pp. 1–5. [Google Scholar]
- Konovalenko, A.; Reyes-Avendaño, J.A.; Méndez-Blas, A.; Cervera, F.; Myslivets, E.; Radic, S.; Sánchez-Dehesa, J.; Pérez-Rodríguez, F. Nonlocal electrodynamics of homogenized metal-dielectric photonic crystals. J. Opt. 2019, 21, 085102. [Google Scholar] [CrossRef]
- Zheng, L.; Qi-Huang, G. Surface Polaritons in a Wire Medium with Spatial Dispersion. Chin. Phys. Lett. 2008, 25, 298–301. [Google Scholar] [CrossRef]
- Belov, P.A.; Marqués, R.; Maslovski, S.I.; Nefedov, I.S.; Silveirinha, M.; Simovski, C.R.; Tretyakov, S.A. Strong spatial dispersion in wire media in the very large wavelength limit. Phys. Rev. B 2003, 67, 113103. [Google Scholar] [CrossRef]
- Silveirinha, M.G. Nonlocal homogenization model for a periodic array of ϵ-negative rods. Phys. Rev. E 2006, 73, 046612. [Google Scholar] [CrossRef] [PubMed]
- Simovski, C.R.; Belov, P.A.; Atrashchenko, A.V.; Kivshar, Y.S. Wire Metamaterials: Physics and Applications. Adv. Mater. 2012, 24, 4229–4248. [Google Scholar] [CrossRef]
- Moradi, A. Spatial nonlocality in the infrared absorption spectra of polar semiconductor nanospheres. Semicond. Sci. Technol. 2015, 30, 115003. [Google Scholar] [CrossRef]
- Maack, J.R.; Mortensen, N.A.; Wubs, M. Size-dependent nonlocal effects in plasmonic semiconductor particles. EPL (Europhys. Lett.) 2017, 119, 17003. [Google Scholar] [CrossRef]
- Dong, T.; Ma, X. Electromagnetic scattering by gyrotropic semiconductor spheres when considering spatial dispersion. J. Phys. D Appl. Phys. 2018, 51, 285302. [Google Scholar] [CrossRef]
- Eremin, Y.A.; Mädler, L.; Wriedt, T. Influence of the Nonlocal Effect on the Optical Properties of Nonspherical Plasmonic Semiconductor Nanoparticles. Comput. Math. Model. 2020, 31, 58–74. [Google Scholar] [CrossRef]
- Souza, R.F.; Alencar, M.A.R.C.; Meneghetti, M.R.; Dupont, J.; Hickmann, J.M. Nonlocal optical nonlinearity of ionic liquids. J. Phys. Condens. Matter 2008, 20, 155102. [Google Scholar] [CrossRef]
- Poddubny, A.; Iorsh, I.; Belov, P.; Kivshar, Y. Hyperbolic metamaterials. Nat. Photonics 2013, 7, 948–957. [Google Scholar] [CrossRef]
- Orlov, A.; Voroshilov, P.M.; Belov, P.A.; Kivshar, Y.S. Engineered optical nonlocality in nanostructured metamaterials. Phys. Rev. B 2011, 84, 045424. [Google Scholar] [CrossRef]
- Becchi, M.; Oldano, C.; Ponti, S. Spatial dispersion and optics of crystals. J. Opt. Pure Appl. Opt. 1999, 1, 713–718. [Google Scholar] [CrossRef]
- Shvartsburg, A.B.; Agranat, M.B.; Chefonov, O.V. Nanooptics of gradient dielectric films. Quantum Electron. 2009, 39, 948–952. [Google Scholar] [CrossRef]
- Weissglas, P. Spatial dispersion and absorption of waves in bounded low-density plasma. J. Nucl. Energy Part C Plasma Phys. Accel. Thermonucl. Res. 1964, 6, 251–265. [Google Scholar] [CrossRef]
- Brodin, G.; Marklund, M. On the possibility of metamaterial properties in spin plasmas. New J. Phys. 2008, 10, 115031. [Google Scholar] [CrossRef]
- Sakai, O.; Tachibana, K. Plasmas as metamaterials: A review. Plasma Sources Sci. Technol. 2012, 21, 013001. [Google Scholar] [CrossRef]
- Wang, C.Y.; Wang, G.H. Spatially nonlocal effects on optical absorption properties in coupled quantum wells with an applied electric field. Chin. Phys. B 2014, 23, 127103. [Google Scholar] [CrossRef]
- Ikeda, K.; Akimoto, O. Steady propagation of a coherent light pulse in a dielectric medium – Part II. The effect of spatial dispersion. J. Phys. A Math. Gen. 1979, 12, 1105–1120. [Google Scholar] [CrossRef]
- Alfassi, B.; Rotschild, C.; Manela, O.; Segev, M.; Christodoulides, D.N. Nonlocal Surface-Wave Solitons. Phys. Rev. Lett. 2007, 98, 213901. [Google Scholar] [CrossRef]
- Zhong, W.P.; Yi, L.; Xie, R.H.; Belić, M.; Chen, G. Robust three-dimensional spatial soliton clusters in strongly nonlocal media. J. Phys. B At. Mol. Opt. Phys. 2008, 41, 025402. [Google Scholar] [CrossRef]
- Zhang, H.; Xu, D.; Li, L. An approximate solution for describing a fundamental soliton in nonlocal nonlinear media. J. Opt. A Pure Appl. Opt. 2009, 11, 125203. [Google Scholar] [CrossRef]
- Pu, S.; Hou, C.; Zhan, K.; Du, Y. Beam splitters in inhomogeneous nonlocal media. Phys. Scr. 2012, 86, 025404. [Google Scholar] [CrossRef]
- Alberucci, A.; Peccianti, M.; Assanto, G.; Dyadyusha, A.; Kaczmarek, M. Two-Color Vector Solitons In Nonlocal Media. Phys. Rev. Lett. 2006, 97, 153903. [Google Scholar] [CrossRef]
- Vestgården, J.I.; Mikheenko, P.; Galperin, Y.M.; Johansen, T.H. Nonlocal electrodynamics of normal and superconducting films. New J. Phys. 2013, 15, 093001. [Google Scholar] [CrossRef]
- Abdumalikov, A.; Alfimov, G.L.; Malishevskii, A.S. Nonlocal electrodynamics of Josephson vortices in superconducting circuits. Supercond. Sci. Technol. 2009, 22, 023001. [Google Scholar]
- Binkowski, F.; Zschiedrich, L.; Hammerschmidt, M.; Burger, S. Modal analysis for nanoplasmonics with nonlocal material properties. Phys. Rev. B 2019, 100, 155406. [Google Scholar] [CrossRef]
- Luo, Y.; Fernandez-Dominguez, A.I.; Wiener, A.; Maier, S.A.; Pendry, J.B. Surface Plasmons and Nonlocality: A Simple Model. Phys. Rev. Lett. 2013, 111, 093901. [Google Scholar] [CrossRef] [PubMed]
- Moaied, M.; Palomba, S.; Ostrikov, K. Quantum plasmonics: Longitudinal quantum plasmons in copper, gold, and silver. J. Opt. 2017, 19, 105402. [Google Scholar] [CrossRef]
- Ramírez, E.V.G.; Hernández, S.A.S.; Rodríguez, J.J.M.; Díaz, G.; Carrasco, M.L.A.; Esqueda, J.A.R. Nonlocality in Ag nanocubes: Dependence on the concentration and incident wavelength. Mater. Res. Express 2020, 6, 1250c6. [Google Scholar] [CrossRef]
- Gratus, J.; Kinsler, P.; McCall, M.W.; Thompson, R.T. On spacetime transformation optics: Temporal and spatial dispersion. New J. Phys. 2016, 18, 123010. [Google Scholar] [CrossRef]
- Silveirinha, M.G. Chern invariants for continuous media. Phys. Rev. B 2015, 92, 125153. [Google Scholar] [CrossRef]
- Banerjee, N.; Dutta, S.; Roychowdhury, D. Chern–Simons superconductor. Class. Quantum Gravity 2014, 31, 245005. [Google Scholar] [CrossRef][Green Version]
- Mikki, S.; Kishk, A. Electromagnetic wave propagation in dispersive negative group velocity media. In Proceedings of the 2008 IEEE MTT-S International Microwave Symposium Digest, Atlanta, GA, USA, 15–20 June 2008; pp. 205–208. [Google Scholar]
- Hapuarachchi, H.; Gunapala, S.D.; Premaratne, M. Plasmonic metaresonances: Harnessing nonlocal effects for prospective biomedical applications. J. Phys. Condens. Matter 2019, 31, 325301. [Google Scholar] [CrossRef] [PubMed]
- Mikki, S. Theory of Electromagnetic Radiation in Nonlocal Metamaterials—Part I: Foundations. Prog. Electromagn. Res. B 2020, 89, 63–86. [Google Scholar] [CrossRef]
- Mikki, S. Theory of Electromagnetic Radiation in Nonlocal Metamaterials—Part II: Applications. Prog. Electromagn. Res. B 2020, 89, 87–109. [Google Scholar] [CrossRef]
- Gorlach, M.A.; Belov, P.A. Nonlocality in uniaxially polarizable media. Phys. Rev. B 2015, 92, 085107. [Google Scholar] [CrossRef]
- Skettrup, T. Effective optical constants in the treatment of spatial dispersion. J. Phys. D Appl. Phys. 1981, 14, 1343–1354. [Google Scholar] [CrossRef]
- Pérez-Huerta, J.S.; Ortiz, G.P.; Mendoza, B.S.; Mochán, W.L. Macroscopic optical response and photonic bands. New J. Phys. 2013, 15, 043037. [Google Scholar] [CrossRef]
- Ciattoni, A.; Rizza, C. Nonlocal homogenization theory in metamaterials: Effective electromagnetic spatial dispersion and artificial chirality. Phys. Rev. B 2015, 91, 184207. [Google Scholar] [CrossRef]
- Mnasri, K.; Khrabustovskyi, A.; Stohrer, C.; Plum, M.; Rockstuhl, C. Beyond local effective material properties for metamaterials. Phys. Rev. B 2018, 97, 075439. [Google Scholar] [CrossRef]
- Raghu, S.; Haldane, F.D.M. Analogs of quantum-Hall-effect edge states in photonic crystals. Phys. Rev. A 2008, 78, 033834. [Google Scholar] [CrossRef]
- Lu, L.; Joannopoulos, J.D.; Soljačić, M. Topological photonics. Nat. Photonics 2014, 8, 821–829. [Google Scholar] [CrossRef]
- Chen, W.J.; Hou, B.; Zhang, Z.Q.; Pendry, J.B.; Chan, C.T. Metamaterials with index ellipsoids at arbitrary k-points. Nat. Commun. 2018, 9, 2086. [Google Scholar] [CrossRef]
- Gorlach, M.; Belov, P.A. Effect of spatial dispersion on the topological transition in metamaterials. Phys. Rev. B 2014, 90, 115136. [Google Scholar] [CrossRef]
- Bliokh, K.Y.; Leykam, D.; Lein, M.; Nori, F. Topological non-Hermitian origin of surface Maxwell waves. Nat. Commun. 2019, 10, 1–7. [Google Scholar] [CrossRef]
- Silveirinha, M.G. Topological theory of non-Hermitian photonic systems. Phys. Rev. B 2019, 99, 125155. [Google Scholar] [CrossRef]
- Pekar, S. The Theory of Electromagnetic Waves in a Crystal in which Excitons Are Produced. JETP 1957, 6, 785. [Google Scholar]
- Agranovich, V.M.; Ginzburg, V.L. Crystal optics with allowance for spatial dispersion: Exciton theory—Part I. Sov. Phys. Uspekhi 1962, 5, 323–346. [Google Scholar] [CrossRef]
- Agranovich, V.M.; Ginzburg, V.L. Crystal optics with allowance for spatial dispersion: Exciton theory—Part II. Sov. Phys. Uspekhi 1963, 5, 675–710. [Google Scholar] [CrossRef]
- Ginzburg, V.L. The Development of Crystal Optics with Allowance for Spatial Dispersion. Sov. Phys. Uspekhi 1973, 15, 839–841. [Google Scholar] [CrossRef]
- Belov, P.A.; Simovski, C.R. On the low-frequency spatial dispersion in wire media. In Proceedings of the IEEE International Workshop on Antenna Technology: Small Antennas and Novel Metamaterials, Singapore, 7–9 March 2005; pp. 363–366. [Google Scholar] [CrossRef]
- Wells, B.M.; Zayats, A.V.; Podolskiy, V.A. Nonlocal optics of plasmonic nanowire metamaterials. Phys. Rev. B 2014, 89, 035111. [Google Scholar] [CrossRef]
- Mikki, S.; Kishk, A. Mean-Field Electrodynamic Theory of Aligned Carbon Nanotube Composites. IEEE Trans. Antennas Propag. 2009, 57, 1412–1419. [Google Scholar] [CrossRef]
- Chebykin, A.V.; Gorlach, M.A.; Gorlach, A.; Belov, P.A. Spatial dispersion in metamaterials based on three-dimensional arrays of spheres and disks. In Proceedings of the 2015 Days on Diffraction (DD), St. Petersburg, Russia, 25–29 May 2015; pp. 1–5. [Google Scholar] [CrossRef]
- Guan, Y.; Zhong, L.X.; Chew, K.H.; Chen, H.; Wu, Q.; Chen, R.P. Evolution of cos-gaussian beams in a strongly nonlocal nonlinear medium. Prog. Electromagn. Res. 2013, 141, 403–414. [Google Scholar] [CrossRef]
- McMahon, J.M.; Gray, S.K.; Schatz, G.C. Nonlocal Optical Response of Metal Nanostructures with Arbitrary Shape. Phys. Rev. Lett. 2009, 103, 097403. [Google Scholar] [CrossRef]
- Ciecierska, E.; Boczkowska, A.; Kurzydlowski, K.J. Quantitative description of the spatial dispersion of carbon nanotubes in polymeric matrix. J. Mater. Sci. 2010, 45, 2305–2310. [Google Scholar] [CrossRef]
- Gomez-Diaz, J.S.; Mosig, J.R.; Perruisseau-Carrier, J. Effect of Spatial Dispersion on Surface Waves Propagating Along Graphene Sheets. IEEE Trans. Antennas Propag. 2013, 61, 3589–3596. [Google Scholar] [CrossRef]
- Rodriguez-Lopez, P.; Kort-Kamp, W.J.M.; Dalvit, D.A.R.; Woods, L.M. Nonlocal optical response in topological phase transitions in the graphene family. Phys. Rev. Mater. 2018, 2, 014003. [Google Scholar] [CrossRef]
- Hanson, G.W. Drift-Diffusion: A Model for Teaching Spatial-Dispersion Concepts and the Importance of Screening in Nanoscale Structures. IEEE Antennas Propag. Mag. 2010, 52, 198–207. [Google Scholar] [CrossRef]
- Mikki, S. Exact Derivation of the Radiation Law of Antennas Embedded into Generic Nonlocal Metamaterials: A Momentum-Space Approach. In Proceedings of the 14th European Conference on Antennas and Propagation (EuCAP), Copenhagen, Denmark, 15–20 March 2020; pp. 1–5. [Google Scholar]
- Wang, J.; Qu, S.; Zhang, J.; Ma, H. Spatial-temporal dispersion engineering of longitudinally coupled spoof surface plasmon polaritons for free-space EM wave modulation. In Proceedings of the 2016 Progress in Electromagnetic Research Symposium (PIERS), Shanghai, China, 8–11 August 2016; pp. 1571–1572. [Google Scholar] [CrossRef]
- Lathi, B.P.; Ding, Z. Modern Digital and Analog Communication Systems; Oxford University Press: New York, NY, USA, 2019. [Google Scholar]
- Mikki, S. Theory of Nonsinusoidal Antennas for Near-Field Communication System Analysis. Prog. Electromagn. Res. 2020, 86, 177–193. [Google Scholar] [CrossRef]
- Mikki, S.; Kishk, A. Effective Medium Theory for Carbon Nanotube Composites and their Potential Applications as Metamaterials. In Proceedings of the 2007 IEEE/MTT-S International Microwave Symposium, Honolulu, HI, USA, 3–8 June 2007; pp. 1137–1140. [Google Scholar] [CrossRef]
- Mikki, S.; Antar, Y. Reactive, localized, and stored energies: The fundamental differences and proposals for new experiments. In Proceedings of the 2015 IEEE AP-S Symposium on Antennas and Propagation and URSI CNC/USNC Joint Meeting, Vancouver, BC, Canada, 19–24 July 2015; p. 366. [Google Scholar]
- Mikki, S.; Sarkar, D.; Antar, Y. On Localized Antenna Energy in Electromagnetic Radiation. Prog. Electromagn. Res. M 2019, 79, 1–10. [Google Scholar] [CrossRef]
- Sarkar, D.; Mikki, S.; Srivastava, K.V.; Antar, Y. Dynamics of Antenna Reactive Energy Using Time-Domain IDM Method. IEEE Trans. Antennas Propag. 2019, 67, 1084–1093. [Google Scholar] [CrossRef]
- Sarkar, D.; Mikki, S.M.; Antar, Y.M.M. Poynting Localized Energy: Method and Applications to Gain Enhancement in Coupled Antenna Systems. IEEE Trans. Antennas Propag. 2020, 68, 3978–3988. [Google Scholar] [CrossRef]
- Shvarts, A.S. On the definition of superspace. Theor. Math. Phys. 1984, 60, 657–660. [Google Scholar] [CrossRef]
- Barbour, J. The End of Time: The Next Revolution in Physics; Oxford University Press: Oxford, UK; New York, NY, USA, 2000. [Google Scholar]
- Anderson, E. The Problem of Time: Quantum Mechanics Versus General Relativity; Springer: Cham, Switzerland, 2017. [Google Scholar]
- Penrose, R. The Road to Reality: A Complete Guide to the Laws of the Universe; Vintage Books: New York, NY, USA, 2007. [Google Scholar]
- McClain, B.; Niemi, A.; Taylor, C.; Wijewardhana, L.C.R. Superspace, Negative Dimensions, and Quantum Field Theories. Phys. Rev. Lett. 1982, 49, 252–255. [Google Scholar] [CrossRef]
- Castagnino, M. Mathematical structure of quantum superspace as a consequence of time asymmetry. Phys. Rev. D 1998, 57, 750–767. [Google Scholar] [CrossRef]
- Zeidler, E. Quantum Field Theory II: Quantum Electrodynamics; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Zeidler, E. Quantum Field Theory III: Gauge Theory; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Russell, B. The Analysis of Matter; Martino Fine Books: Mansfield Centre, CT, USA, 2014. [Google Scholar]
- Janner, A.; Janssen, T. Symmetry of periodically distorted crystals. Phys. Rev. B 1977, 15, 643–658. [Google Scholar] [CrossRef]
- Tsakalakos, T. Modulated Structure Materials; M. Nijhoff: Dordrecht, The Netherlands; Ner Boston, MA, USA, 1984. [Google Scholar]
- Elcoro, L.; Perez-Mato, J.M. Superspace description of quasiperiodic structures and the nonuniqueness of superspace embedding. Phys. Rev. B 1996, 54, 12115–12124. [Google Scholar] [CrossRef]
- Andreev, A.F. Mesoscopic superconductivity in superspace. J. Exp. Theor. Phys. Lett. 1998, 68, 673–678. [Google Scholar] [CrossRef]
- Geroch, R. Infinite-Dimensional Manifolds; Minkowski Institute Press: Montreal, QC, USA, 2013. [Google Scholar]
- Weyl, H. Space, Time, Matter; Dover Publications: New York, NY, USA, 1952. [Google Scholar]
- Agricola, I. Global Analysis: Differential Forms in Analysis, Geometry, and Physics; American Mathematical Society: Providence, RI, USA, 2002. [Google Scholar]
- Wannier, G.H. The Structure of Electronic Excitation Levels in Insulating Crystals. Phys. Rev. 1937, 52, 191–197. [Google Scholar] [CrossRef]
- Quattropani, A.; Czaja, W. Quantum Theory of Exciton-Polaritons with Spatial Dispersion. Phys. Scr. 1989, T29, 162–166. [Google Scholar] [CrossRef]
- Bamba, M.; Ishihara, H. QED of excitons with nonlocal susceptibility in arbitrarily structured dielectrics. Phys. Rev. B 2008, 78, 085109. [Google Scholar] [CrossRef]
- Cho, K. (Ed.) Excitons; Springer: Berlin/Heidelberg, Germany, 1979. [Google Scholar]
- Lagoudakis, K. The Physics of Exciton-Polariton Condensates; CRC Press: Lausanne, Switzerland; Boca Raton, FL, USA, 2013. [Google Scholar]
- Kira, M.; Koch, S.W. Semiconductor Quantum Optics; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2012. [Google Scholar]
- Bohm, D.; Pines, D. A Collective Description of Electron Interactions–Part I: Magnetic Interactions. Phys. Rev. 1951, 82, 625–634. [Google Scholar] [CrossRef]
- Pines, D.; Bohm, D. A Collective Description of Electron Interactions–Part II: Collective vs Individual Particle Aspects of the Interactions. Phys. Rev. 1952, 85, 338–353. [Google Scholar] [CrossRef]
- Mattuck, R. A Guide to Feynman Diagrams in the Many-Body Problem; Dover Publications: New York, NY, USA, 1992. [Google Scholar]
- Pines, D. The Many-Body Problem; Addison-Wesley: Reading, MA, USA, 1997. [Google Scholar]
- Pines, D. Elementary Excitations in Solids: Lectures on Protons, Electrons, and Plasmons; Advanced Book Program, Perseus Books: Reading, MA, USA, 1999. [Google Scholar]
- Dresselhaus, G. Effective mass approximation for excitons. J. Phys. Chem. Solids 1956, 1, 14–22. [Google Scholar] [CrossRef]
- Prigogine, I. The End of Certainty: Time, Chaos, and the New Laws of Nature; Free Press: New York, NY, USA, 1997. [Google Scholar]
- Zomorodian, A. Topology for Computing; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar]



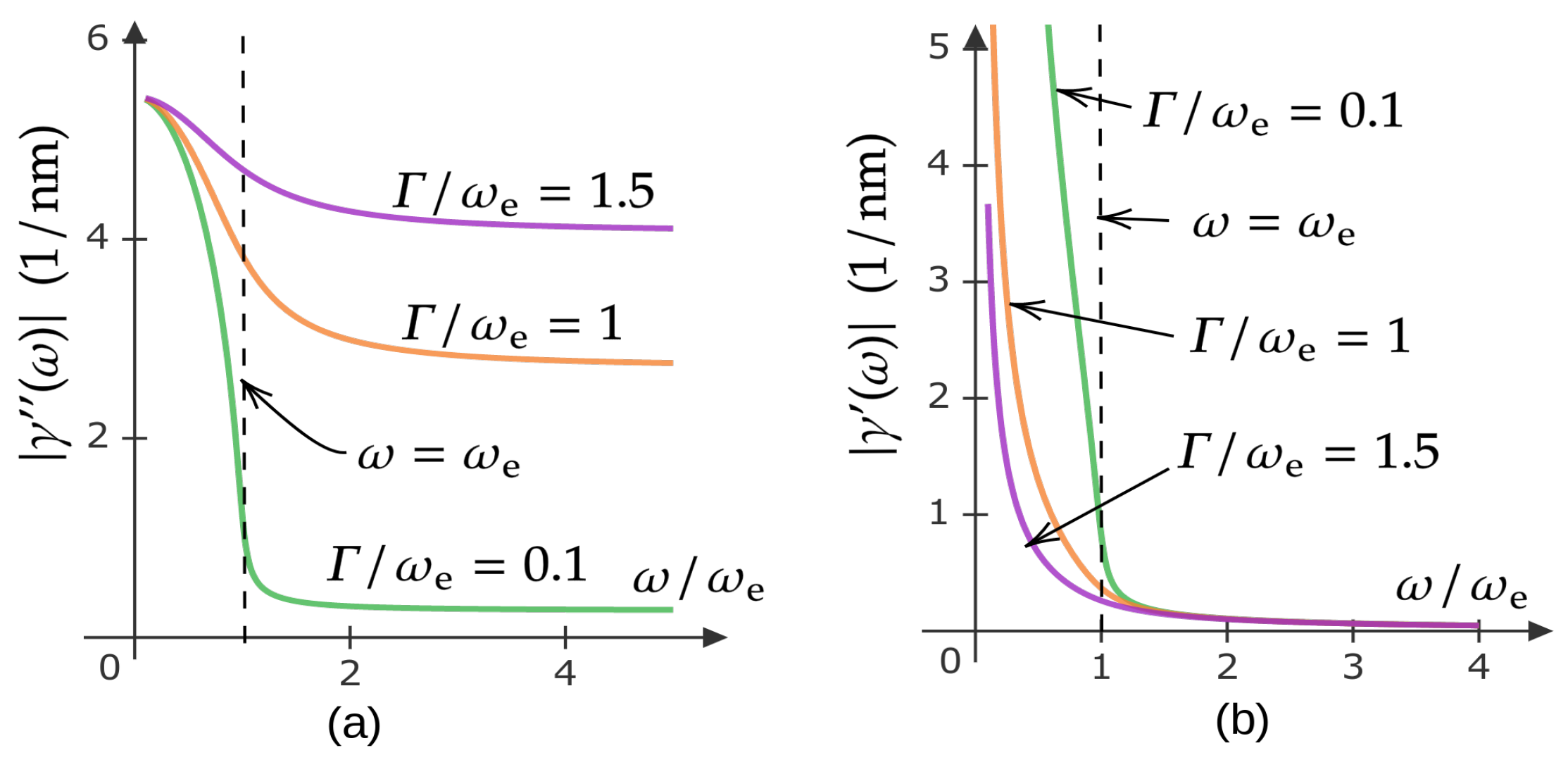
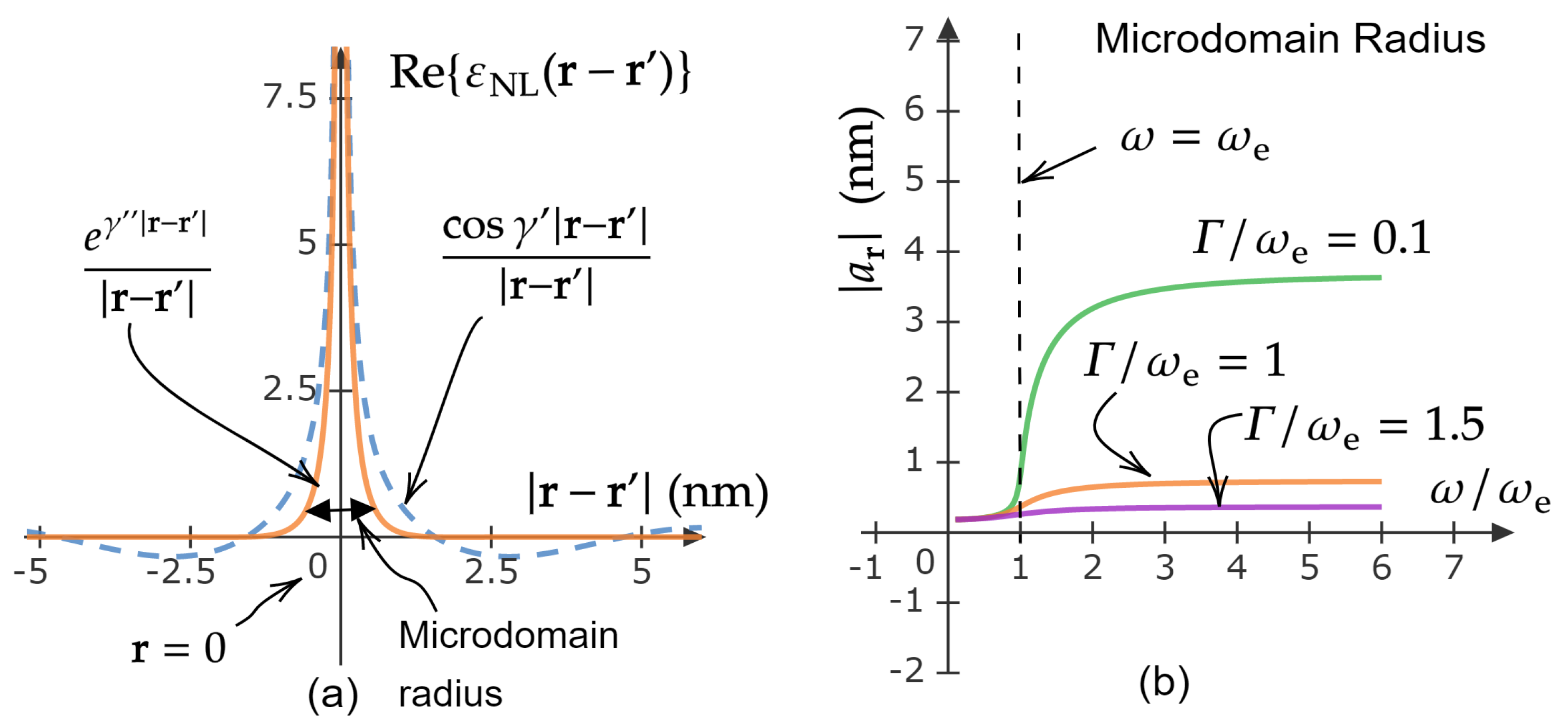

| Scale | Type | Meaning | Formula |
|---|---|---|---|
| spatial | excitation field wavelength | ||
| spatial | exciton wavelength | ||
| spatial | microdomain radius | ||
| temporal | excitation field period | ||
| temporal | exciton lifetime | ||
| temporal | exciton period |
| (THz) | (m) | m) | |||
|---|---|---|---|---|---|
| 19,090 | 0.8 | 0.0003 | 1.01 | 2.5834 | |
| 21,476 | 0.9 | 0.0004 | 1.01 | 0.2583 | |
| 23,862 | 1.0 | 0.0582 | 1.01 | 0.0259 | |
| 26,248 | 1.1 | 7.6670 | 1.01 | 0.0028 | |
| 35,793 | 1.5 | 13.7174 | 1.01 | 0.0028 | |
| 47,724 | 2.0 | 15.9382 | 2.0000 | 1.01 | 0.0002 |
| 59,655 | 2.5 | 16.8674 | 20.000 | 1.01 | 0.0001 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mikki, S. On the Topological Structure of Nonlocal Continuum Field Theories. Foundations 2022, 2, 20-84. https://doi.org/10.3390/foundations2010003
Mikki S. On the Topological Structure of Nonlocal Continuum Field Theories. Foundations. 2022; 2(1):20-84. https://doi.org/10.3390/foundations2010003
Chicago/Turabian StyleMikki, Said. 2022. "On the Topological Structure of Nonlocal Continuum Field Theories" Foundations 2, no. 1: 20-84. https://doi.org/10.3390/foundations2010003
APA StyleMikki, S. (2022). On the Topological Structure of Nonlocal Continuum Field Theories. Foundations, 2(1), 20-84. https://doi.org/10.3390/foundations2010003





