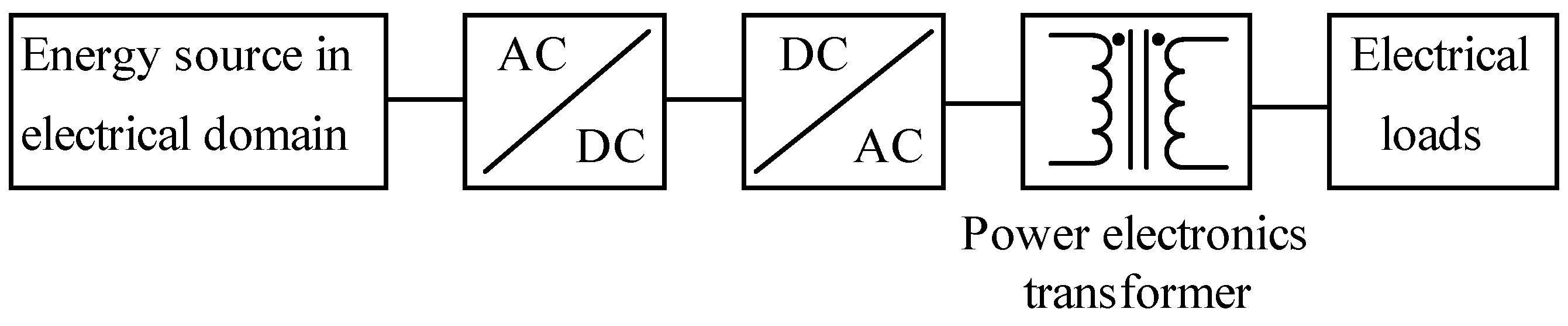
1. Introduction
Power electronics converters [
1] are used to ensure efficient handling of electrical energy where, as shown in
Figure 1, power electronic transformer (PET) is an integral part [
2,
3,
4,
5,
6,
7]. Though it is a passive component, its role in power converters is immense. Apart from mandatory safety isolation, a PET could be used either for voltage [
8] or current multiplication [
4] to match the load characteristics of applications. Depending upon the topology of power converter used, additionally, it is often used to perform certain assisting roles in different soft-switched inverter operations [
9,
10,
11]. The PET is used for instantaneous power transfer, and it should be efficient and compact. Due to the availability of a wide range of soft magnetic materials [
12,
13] in different geometries as well as of copper conductors (litz wires and copper foils) [
14], the process of design optimization of a PET is now elaborate. For optimal design of a PET, the following aspects need to be looked into:
The power loss characteristics and its distribution in core and copper [
15,
16,
17,
18,
19,
20,
21].
The distribution of steady state temperature in different parts of the core and copper windings [
3,
21].
The influence of soft magnetic materials of suitable geometry [
13] as well as that of copper conductors.
The leakage inductance [
11,
22].
The prospect of static and dynamic DC bias in the PET [
8,
17], etc.
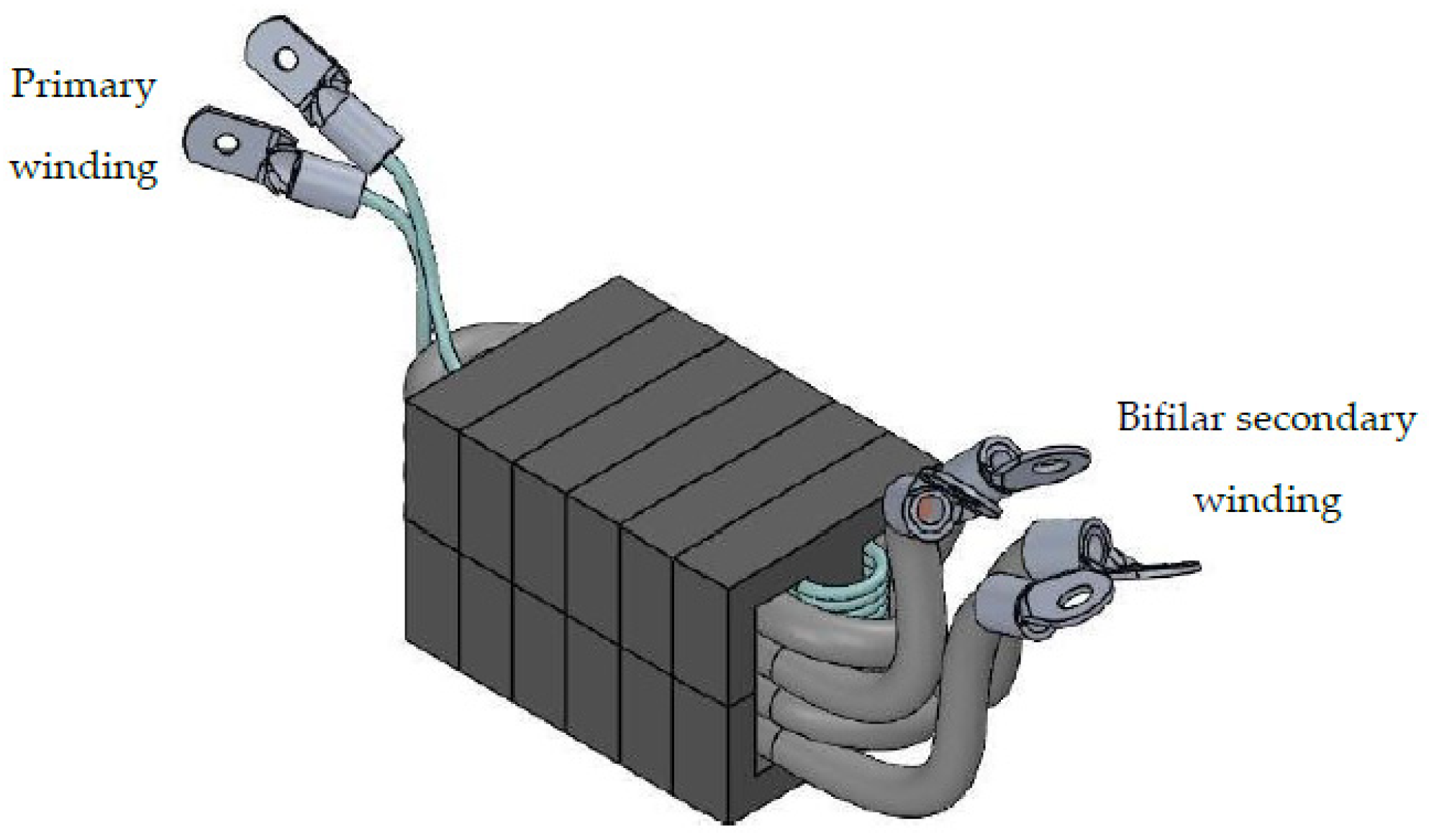
One typical 2-winding PET is shown in
Figure 2. Its design optimization involves optimal use of core and copper; it is possible if both the core and copper losses are minimized. The copper loss
Pcu depends on the current density of conductors, the impact of proximity effects on the resistance values of primary and secondary conductors, winding configuration and the construction of conductors [
14,
23]. On the other hand, the core loss
Pcore depends on the properties of the core material, peak operating flux density
Bm, the excitation frequency
fs, the waveform pattern and the core temperature [
18,
19,
24,
25]. Thirdly, the design also involves devising a thermal circuit so that the temperature rise in core, copper and insulation are not only within the respective safe operating limit, but there should also be increased uniformity of maximum temperature rise in different parts of windings as well as in the core. Ensuring near-uniform temperature rise is complex because the distribution of power loss in the core is not uniform and so is the case for windings. Moreover, the thermal behavior of each circuit is also different. Ideally, for the design of the thermal circuit for heat removal, the average flux density per cycle is considered to be zero where the core loss is decided by the values of
Bm and
fs; it is true when the DC bias in core is absent [
17]. The presence of DC bias could adversely affect the performance of the PET in several ways. Primarily, the core loss increases significantly under DC bias; it could work as a hindrance to draw any comparative statement on performance among different PETs. Secondly, depending upon the DC bias capacity of the magnetic circuit, there could be core saturation that affects the performance of the power controller. The DC bias capacity of the magnetic circuit is poor for zero-gap magnetic circuit using high permeability materials (e.g., toroidal core using nanocrystalline materials). The DC bias could be static [
26] or dynamic [
27,
28].
Except in a few applications [
8] where certain leakage inductance is desired, the design of PET necessitates that the two windings are overlaid on a small segment of the core volume [
29]. Therefore, the bulk of its total power loss is concentrated around a small core volume (e.g., the central limb of
Figure 2). Removal of such concentrated heat loss is a complex task. It would decide the operating limits of the core and the windings [
21]. Large, concentrated power loss along with the constrained heat removal features of core and windings would result nonuniform temperature rise [
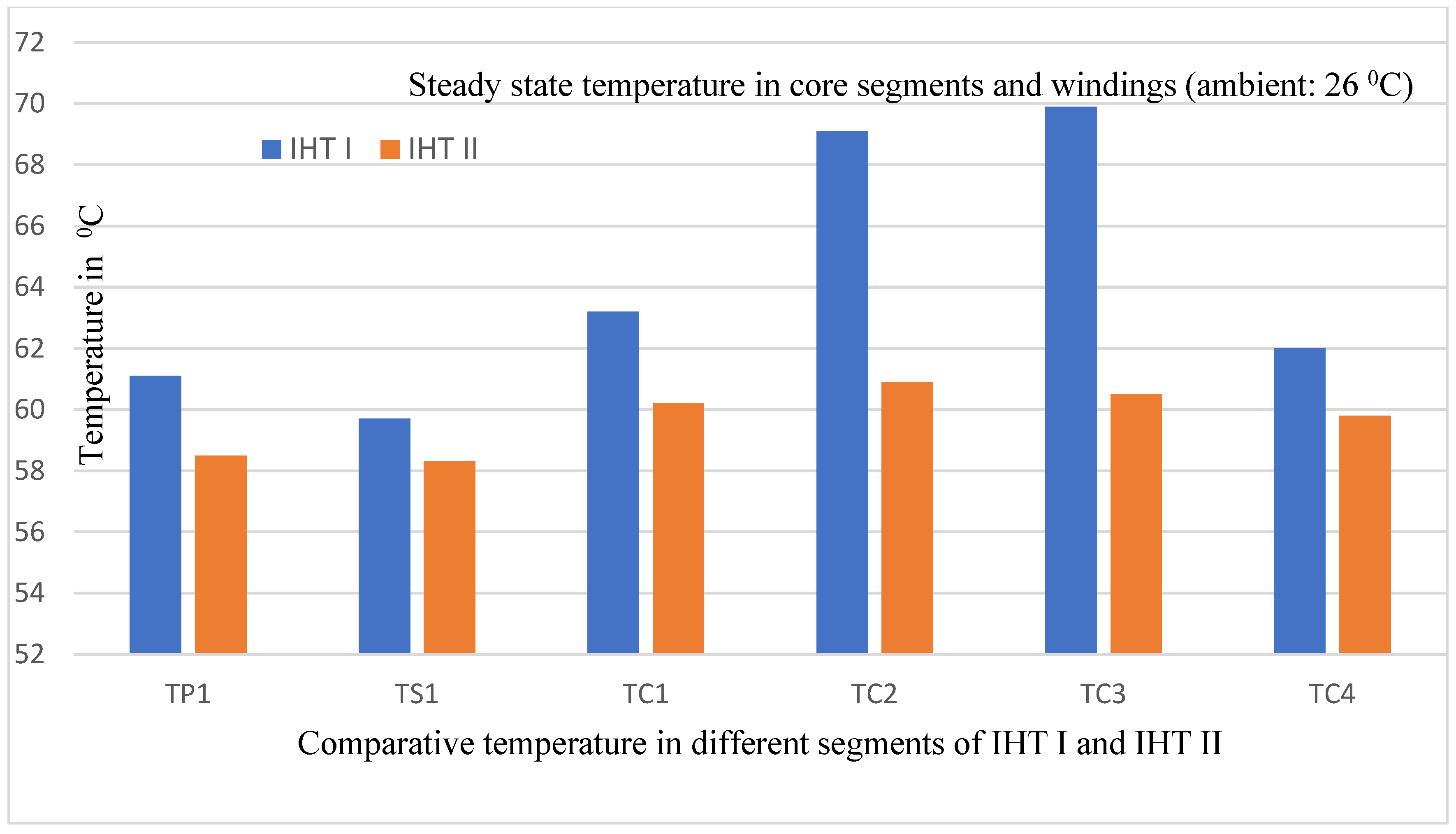
3]. The respective value of maximum temperature rise would decide the capacity of core and windings. It forces the core to operate at reduced flux density because the maximum permissible operating temperature of core is less than that of copper or its insulation. It would affect the power density of the PET. To get the desired flux level, several cores are integrated. Until recently, for medium- to high-frequency PETs, ferrites have been prominently used to build the magnetic circuit. As shown in
Table 1, for ferrites, the heat removal by thermal conduction is not attractive where the prospect of creation of hot spot is comparatively large [
20]. The safe operating temperature range of ferrites is also not large and several of its parameters are sensitive to temperature [
13]. Therefore, cores are usually integrated to achieve multiple goals, e.g., to meet the desired flux level, to have improved thermal conduction features to distribute the core loss over larger core surface area, to reduce the number of turns and layers in the windings, etc.
As detailed in [
3], the temperature rise in various segments of core and copper is different; the safe operating temperature limit of each component of a PET could as well be different. For any power electronic component, for example, the differential value between its limiting temperature and maximum (hot spot) operating temperature would decide its utility as well as the service life [
30]. The prospect of creation of hot spot temperature in copper is high on winding close to the core. The temperature of core is also maximum there [
3]. The value of thermal conductivity
K of core could play an important role in controlling the temperature distribution because, as shown in
Figure 2, a major part of core assembly remains exposed to the surrounding environment. The bulk of power loss in a PET is concentrated around a small part of the core where primary and secondary windings are overlaid. Traditionally, to share the magnetic burden (flux density, core loss) equally, several cores of a similar type have been used to integrate the flux in the magnetic circuit and reduce the core loss density. Laterally, it would reduce the thermal resistance of the magnetic circuit. Often, for parametric matching dynamically, the. same batch code of cores has been strongly recommended. Now, wide-range soft magnetic materials possessing different parametric values are available. To improve the utility of a PET, can different core materials [
12,
13,
25,
31,
32] be integrated in the magnetic circuit? What could be their characteristic features for integration in series or parallel configuration in a magnetic circuit to reduce either the power loss and/or to improve the thermal behavior of the PET?
The inspiration for this experiment-driven research has been the work reported in [
3]. Using finite element analysis (FEA) and validated by requisite practical demonstration, it could correctly estimate the temperature distribution in different segments of core and copper. With the aim to improve the performance of PET, this article proposes, with detailed practical demonstration, to integrate different soft magnetic materials into the magnetic circuit. Here, an improved thermal conductivity and/or reduced loss density of the new core placed in a zone of high power loss is aimed to aid the cooling of regions of maximum heat generation. The structure of the article is organized as follows:
Section 2 details the features of PETs for two different characteristics applications—one used for voltage ratio and the other for current multiplication. It also discusses their design issues.
Section 3 details the different perspectives of two types of mixed-core configuration suitable for two different applications. To take care of important issues such as the magnetic compatibility, etc., it also introduces application specific suitability factor for integrating different soft magnetic materials into the core. Finally,
Section 4 details the practical validation of mixed-core, air-cooled transformers for two different application domains.
2. Power Electronics Transformer for Divergent Load Characteristics
Any new idea needs to be validated in different application domains. A PET is used to make the load compatible to the source; it could be achieved in several ways. A PET could be part of power transfer in PWM controlled full-bridge DC−DC converters (FBDC) [
21,
33], resonant converters [
6,
34] or for feeding a resonant tank circuit [
4]. The nature of waveforms of current and voltage could change in applications. The PET could be used for voltage ratio where, at zero output power, the current in windings could be zero, or it could be used for current ratio where its primary voltage is decided by the load. In the first case, the magnetic circuit could remain loaded and the value of copper loss
Pcu could be zero at no load. In the second case, irrespective of the magnitude of the delivered power, the windings always draw the set current. The value of the core loss
Pcore is negligible at zero power.
Achieving optimum power density of a PET is a major design goal [
35]. It depends on the total power loss and the design of thermal circuit. The PET is said to be thermally loaded to its capacity when the rated power, say,
, is delivered to the load. Traditionally, the area product
AwAc in Equation (1) is used to define the extent of optimization of a PET. At a particular frequency
fs, it suggests a large value of
Bm in core as well as the current density
J in copper windings, as given below,
The expression of
Bm for a square wave input voltage
Vin is,
Aw is the window area, Ac is the core area and np is the number of primary turns.
For effective use of a PET, its core loss
Pcore [
18] and copper loss
Pcu [
23] need to be calculated accurately. For sinusoidal primary voltage, the Steinmetz equation [
16] is used to calculate the value of
Pcore; its parameters are mostly mentioned in core datasheet. However, in high-frequency applications, the primary voltage is rarely sinusoidal. Using the same Steinmetz parameters, the improved generalized Steinmetz equation (iGSE) is used to calculate
Pcore for any input voltage waveform [
17,
19]. Using the iGSE, the expression of
Pcore with square wave excitation is,
where
.
KS,
α and
β are Steinmetz parameters,
dpwm is duty cycle of square wave input and
Wc is the core weight. For pure square wave input (
dpwm: 1.0), Equation (3) may be modified to,
The expressions of
in primary (
Ppri) and secondary (
Psec) windings are,
F1 and
F2 are the ac resistance factors,
ip and
is are the primary and the secondary current, respectively, and
rdc1 and
rdc2 are their respective dc resistance values. Both
F1 and
F2 depend on several factors such as skin and proximity effects where proper choice of copper conductors (litz wire or thin foil) and layout of windings are important [
23].
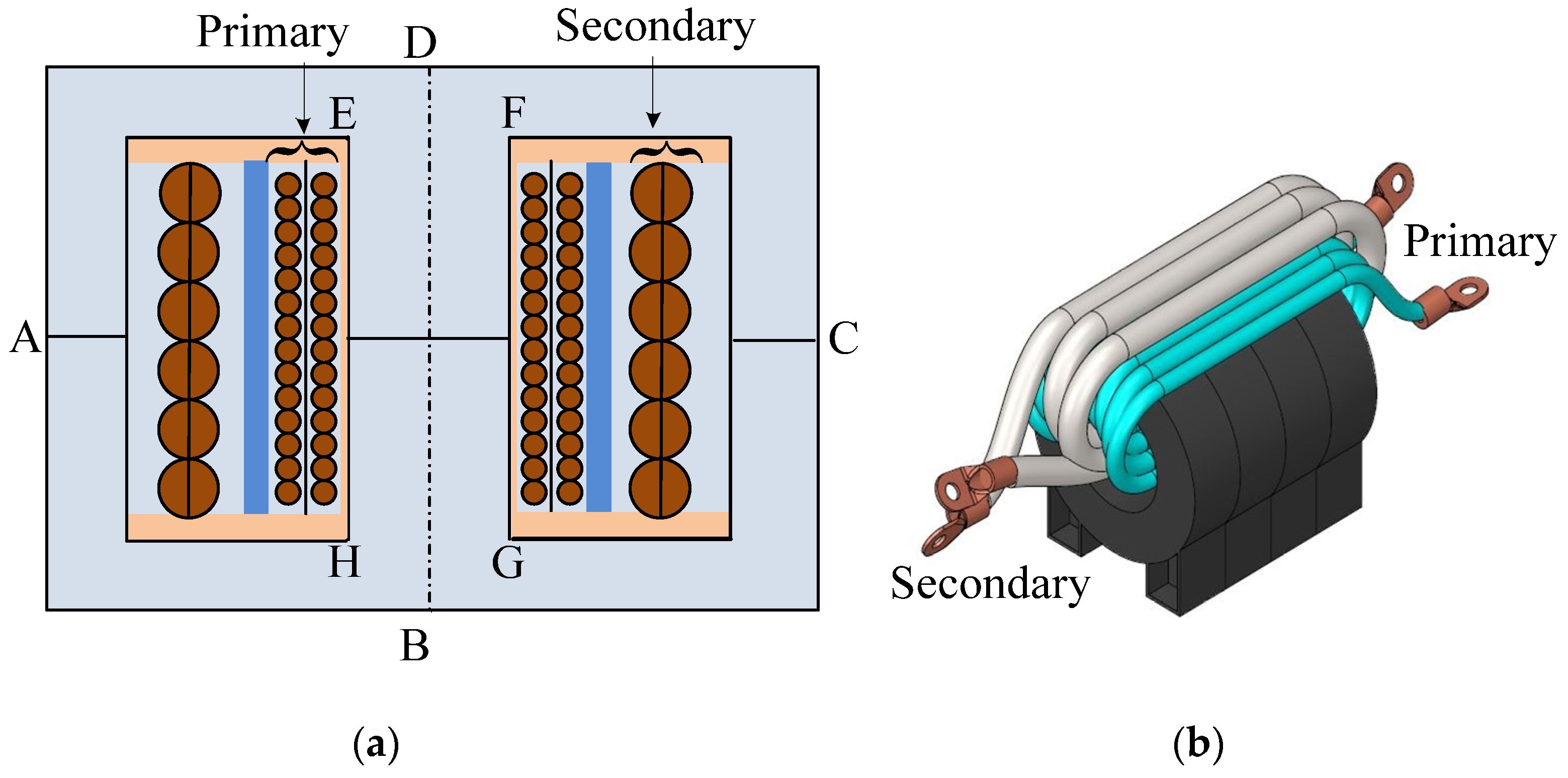
The popular geometry of the magnetic circuit could be based on any of EE, UU or CC as shown in
Figure 3a, or zero-gap toroidal shaped cores of
Figure 3b. The dynamic profile of the input voltage
Vin decides the value of
Bm in core; it depends on the power controller and the characteristics of the connected load. Depending upon the value of
Bm, the magnetic circuit could face nonlinearity as well as the magnetic saturation. Here, two application types are considered where the dynamics of
Vin or
Bm are completely different.
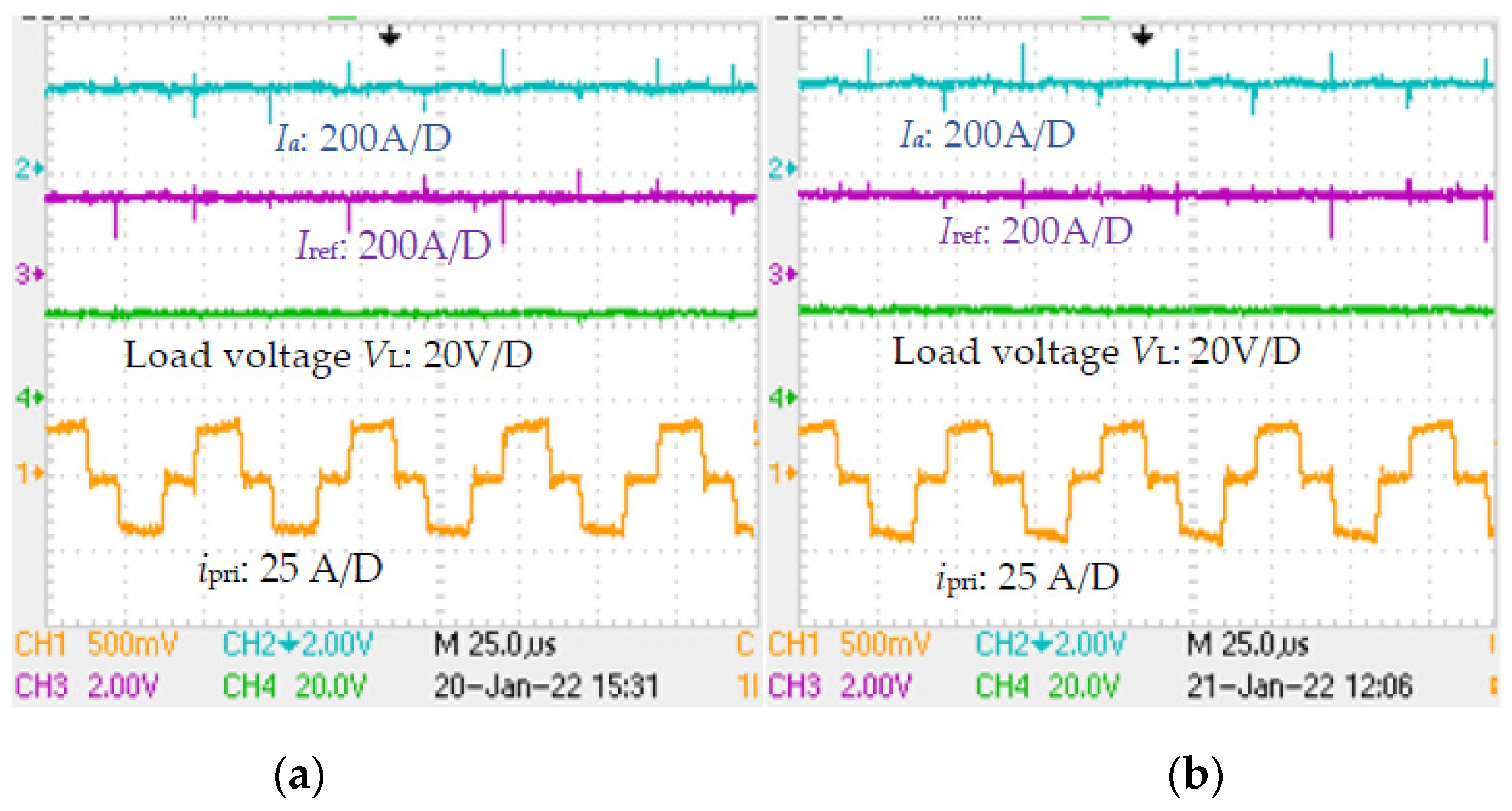
2.1. PET for Full-Bridge DC−DC Converter (FBDC)
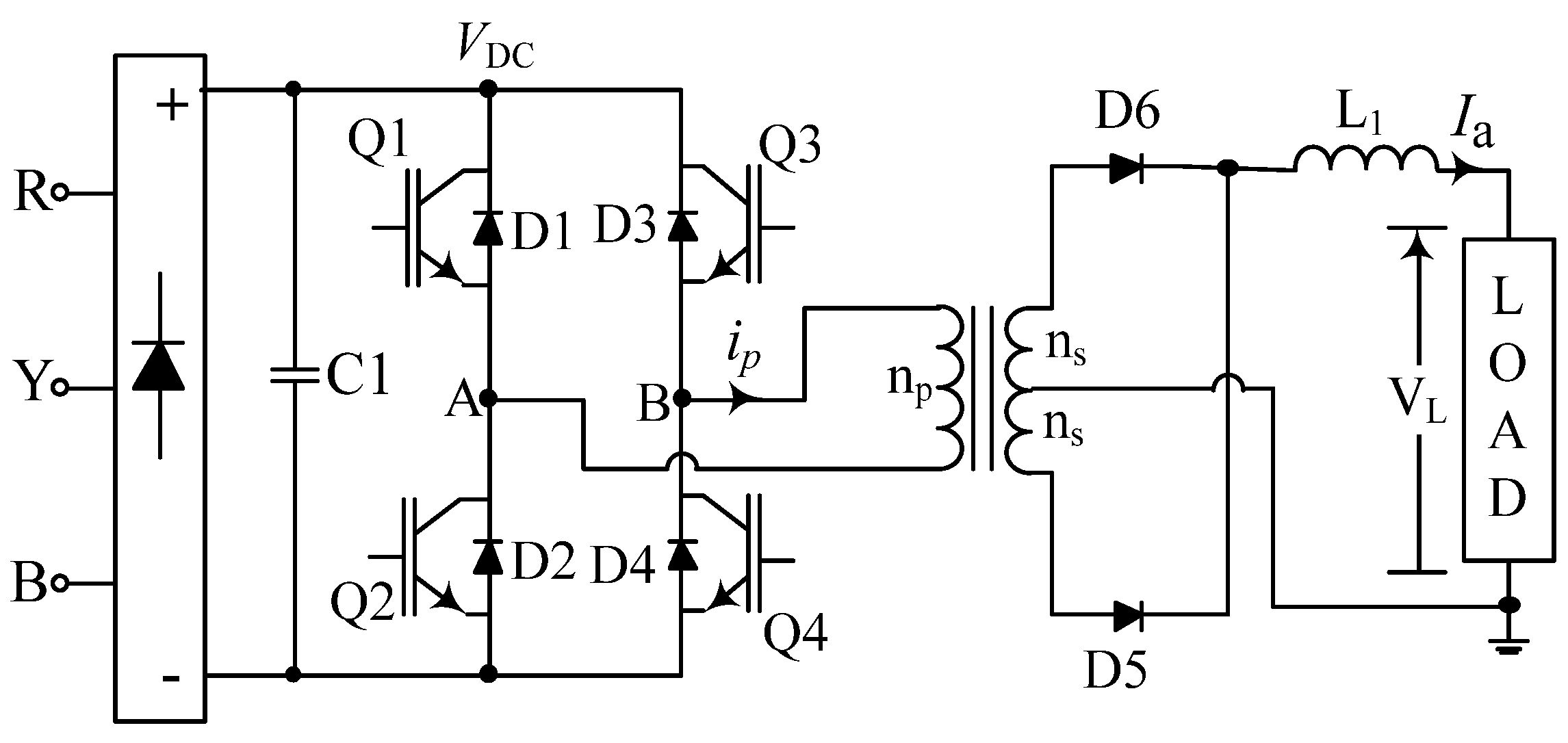
One typical circuit of FBDC is shown in
Figure 4. Here, the magnitude of output current depends on the power drawn by the applied load at voltage
VL and its effective resistance, e.g., battery charging [
11], arc welding [
33], etc. Even at zero load current the cores could be fully loaded. For a nonlinear load (e.g., welding arc), the dynamic control of DC current
Ia would decide the value of
Vin or
Bm through dynamic change in
dpwm, such as,
VDC is the supply voltage,
is the ratio of primary (
np) to secondary (
ns) turns,
k1 is constant and the control
is used to ensure zero current error. The transient disturbance in the arc welding process is large [
28].
The permissible value of
J in windings would be decided by several factors such as values of
F1,
F2 and
dpwm. For the arrangement of secondary side rectifier of
Figure 4, the primary current
ip and the current
is in each bifilar secondary could be expressed as,
If care is not taken, there could be error in
Pcore because the core in FBDC often faces both the static or dynamic DC bias. The static DC bias could be compensated by a simple approach [
26]. The dynamic DC bias [
27,
28] in core would depend on how the control
u (or
dpwm) reacts to ripple in steady state error as well as the load transients. The DC bias is more prominent when the loop gains are large where the ripple in
Ia becomes transparent in control input
u [
28]. Under DC bias conditions, the values of Steinmetz parameters drifts.
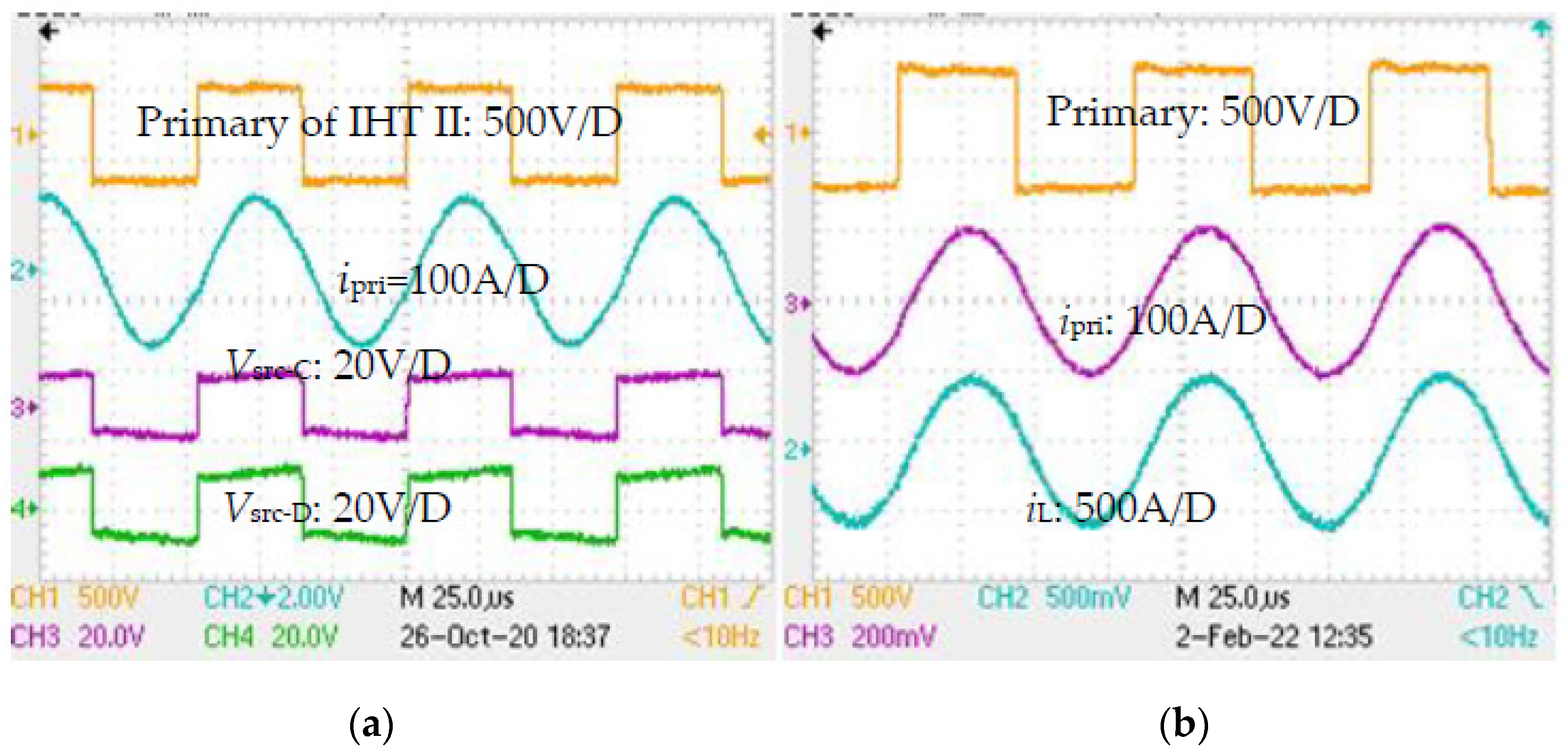
2.2. PET for Series Resonant Induction Heating Equipment
The characteristics of the connected load to PET as well as its associated dynamics is quite different in induction heating. Here, the coil head L4 is kept energized with rated current
iL at the frequency decided by the tank circuit parameters L4 and Cr. The coil facilitates the power transfer when a metallic object is taken close to the coil. For a noncontact mode of power transfer, normally, the coil current
iL is kept large. To reduce the stress on primary side components, as shown in
Figure 5, the induction heating transformer (IHT) is used to step up the inverter current. The value of
Pcu is always at its rated value. The loading of its magnetic circuit and hence the core loss
Pcore depends on the power
POUT drawn through L4; it depends on multiple parameters, such as,
L4 is the inductance value of coil, iL is the coil current, fs is frequency of iL and the parameter Kc depends on coupling between the coil and the load. Req represents the effective load resistance reflected in the tank circuit.
The input voltage to IHT is a square wave
, but the current in both the windings are pure sinusoidal. The DC blocking capacitor
Cdc is connected to eliminate any static DC bias present in the core. Zero voltage switching (ZVS) condition of switches Q5–Q8 is inherently achieved because the phase-locked loop (PLL) ensures
fs at slightly higher than the resonant frequency
. Plus, the buck converter controls the input voltage
Vin to achieve near-zero current switching of Q5–Q8. Under ZVZCS condition, the inverter input voltage
VCH or the primary voltage of IHT could be approximated as,
where
rac is the ac resistance of L4 and
n is the turns ratio of IHT. At no load, the value of
Bm is negligible because the value of
is small. The change in load of IHT, i.e., the change in
Req is never abrupt. Its value increases when a job is brought close to the coil (i.e., when more power is drawn through L4), and decreases gradually either near the Curie point or when the job is taken away from the coil head mechanically. They ensure that the dynamic change in
Bm is also not abrupt. Moreover, the response time of the buck chopper decides the dynamics of
Vin. Therefore, the prospect of dynamic DC bias in IHT would be small. Furthermore, the slow dynamic DC bias in the core, if any, could be effectively tackled in the PLL loop [
36].
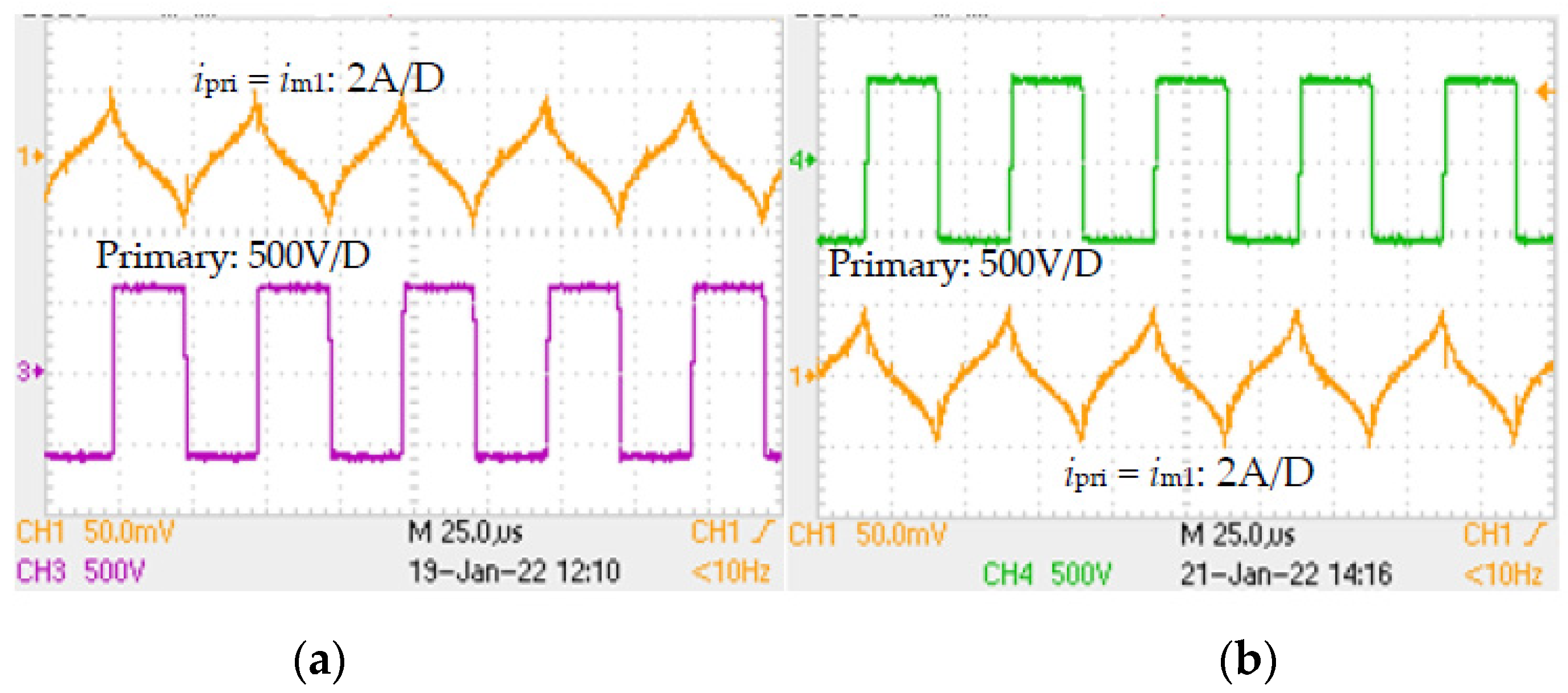
The design of IHT involves deciding on the values of
np and
ns; selecting a core material of suitable geometry to afford optimal values of
Bm and
J. In ZVZCS conditions, the value of
n would be maximum at
nmax because the load behaves as resistive,
At , the current is minimum in the primary winding and the length of conductor used in the secondary is also minimized, and together they help minimize Pcu. Large values of Bsat and small values of Pc of nanocrystalline cores would allow optimal choice of np as well.
Secondly, for efficient transfer of power
, tracking of
fr should be accurate. The value of
Req would be more at higher values of
fr [
37]. When L4 is loaded, its inductance value drifts down to, say,
Leq; then, the corresponding value of
fr is,
Llk is leakage inductance of IHT; its large value would be a hindrance to effective power transfer [
37]. The value of
Llk is small for high-permeability ungapped toroidal cores.
Thirdly, the primary current
ipri consists of triangular wave magnetizing current with peak at
Im plus the reflected sinusoidal coil current
iL. For minimum phase error between
Vpri and
ipri of IHT, the value of
Im should be small; it is expressed as,
where
lm is the mean core length. A large value of
µr is needed for small value of
Im and
np.
For IHT, the high-permeability nanocrystalline material-based ungapped toroidal cores (shown in
Figure 3b) would be preferred [
15,
38,
39,
40], particularly because the DC bias in the core is negligible. Laterally, these cores would ensure small values of
Im and
Llk and also the minimum of number of turns where the value of
Bm would be large.
3. Mixed-Core Transformer Configuration
It was clear in
Section 2.1 and
Section 2.2 that the type of application or load characteristics could influence the design of PET. The nature of loading of magnetic circuit, in particular, could vary in applications, e.g., the value of
Pcore could be fixed and that of
Pcu would be decided by the load. Along with reducing the core and the copper losses, the optimization process involves design of a thermal circuit to ensure near-uniform temperature rise in core and also in copper so that the PET is enabled to deliver more power. Due to multiple loss centers, the thermal circuit of the core and windings are coupled. For the magnetic circuit, the distribution of heat and its removal by thermal convection could be improved if the value of
K as well as that of the surface area of core are increased. To have requisite flux
AcBm, several cores are integrated. Often, for magnetic compatibility, cores of the same material with the same batch code are preferred. It is important to find whether such arrangement is best suitable for efficient heat removal, both from the core assembly as well as from the windings. On the other hand, can some other combinations, such as the hybrid core configuration, manage the heat loss or the thermal issues better?
3.1. Thermal Behavior of Power Electronic Transformer
As shown in Equations (3) and (5), the values of
Pcore and
Pcu, respectively, increase exponentially with
Bm and with
. The design optimization of a PET is complex because the layout of the windings and thermal behavior of the PET often contradict. As shown in
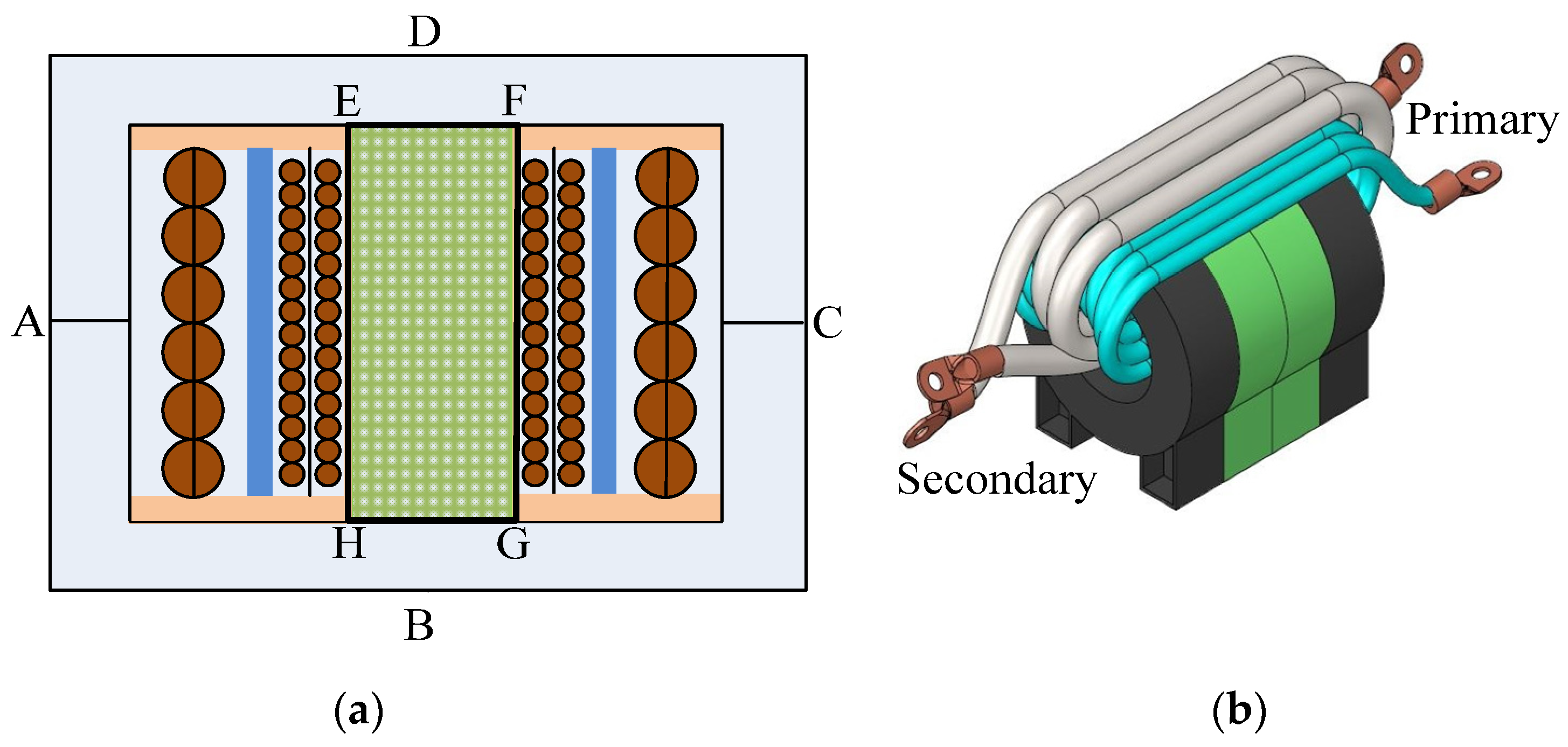
Figure 3a,b, two windings are overlaid for better magnetic coupling and also for reduced eddy current loss in core [
29]. Such arrangement needs good heat removal features because the bulk of total power loss
is concentrated around a small core segment where the secondary winding is laid above the primary. Removing the heat loss from the multilayered primary winding is difficult because there exists insulation on either side of each layer and also between the windings. The prospect of creation of hot spot is more in the primary [
3]. Furthermore, due to the increased impact of the proximity effect, the value of
Pcu would be more in multilayered winding. Though the thermal circuit of PET is coupled in a complex manner, the limiting values of
Bm and
J would be decided by the effectiveness of the thermal circuit [
3,
39,
40]. With given loss, the safe operating temperature for the core would depend on the soft magnetic materials and that for copper would be on the insulation of litz wire strands as well as that placed between the layers.
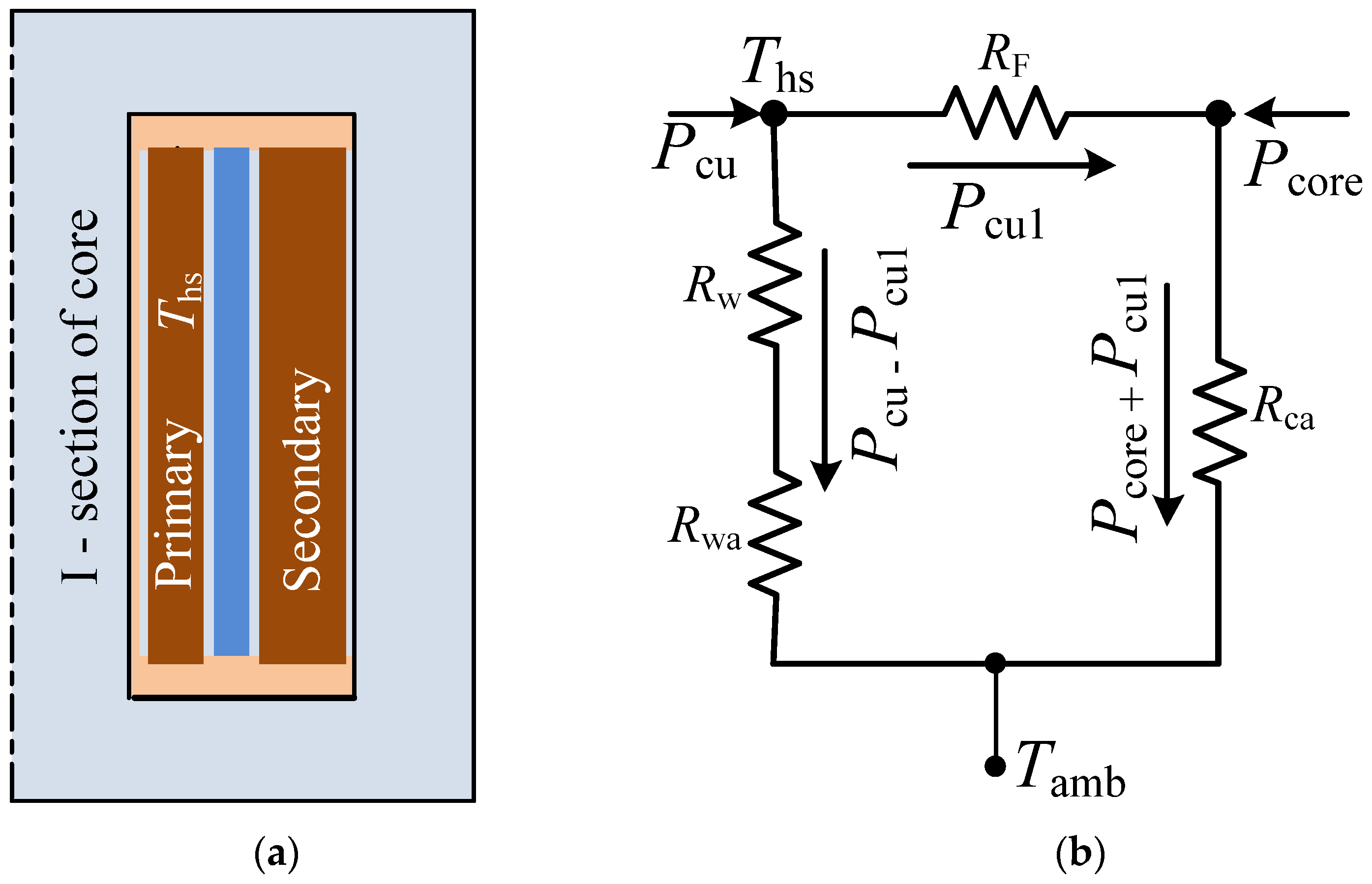
Due to the complex nature of the thermal circuit, there exist multiple heat conduction channels with different heat transfer coefficients, and they are mostly coupled [
3]. The directions of heat flow would be decided by the location of the hot spot temperature
Ths of the PET; it could be decided by the temperature differential
, such as,
is the effective thermal resistance of the PET and
Tamb is the ambient temperature.
RPET mostly consists of thermal conduction (
Rth) and thermal convection (
Rconv). Though it plays certain role, the heat transfer by radiation is ignored here. For compact design of PET, apart from reducing the total loss
Ptot, the value of
should be minimum. Large surface area of the core and the secondary winding are available for heat transfer. The major part of
Ptot is removed by thermal convection [
21,
41,
42] where the speed of the moving medium would play a significant role. The expressions of conductive and convective thermal resistances are,
Acond and
Aconv, respectively, are areas available for conduction and convection and
hcond and
hconv are corresponding heat transfer coefficients. The value of
hconv depends on thermal conductivity of the attached medium and also on its speed where fan cooling improves its value significantly. Backed by practical validation, the finite element method (FEM) was extensively used to establish reasons behind the formation of hot spots in core and copper in high-power transformers [
3]. It was realized that the temperature rise in copper was alarming on multilayer winding with constrained heat transfer features, e.g., the primary winding of
Figure 3a. In multilayer winding, a significant part of
Pcu is concentrated in the internal layer of primary winding closest to the core where the proximity effect is more prominent. The hot spot temperature
Ths is located here; its value needs to be reduced. It could be made possible if a part of
Ppri close to I-section, in particular, is channelized to the ambience through the core. Considering the heat conduction is symmetrical around the I-section of
Figure 3a, the overall heat conduction circuit of half of the PET (shown in
Figure 6a), is represented in
Figure 6b. The part
Pcu1 of total copper loss
Pcu that could be channelized through the core is expressed as [
41],
Rw and Rwa, respectively, are the conductive and the convective resistance of the winding, and RF and Rca, respectively, are the effective thermal resistance of the coil former and the core. It is clear that more heat loss (i.e., Pcu1) would be channelized through the core if either of Rca or Pcore or together could be reduced through design or selection of suitable soft magnetic material; reduction of RF would also play certain assisting role.
To achieve the abovementioned objective, it is proposed to use a mixed-core configuration for the magnetic circuit. It is known that a small fraction of core volume handles most of Ptot while the major part of its surface area is exposed to the ambience. Therefore, if that particular small section of core volume is replaced by a suitable soft magnetic material, then better heat removal feature by convection and radiation could be realized. The new core material is desired to possess following features:
Superior thermal conductivity.
Reduced core loss density.
Higher maximum operating temperature than the parent core.
The new material must be magnetically compatible.
Basic features of different soft magnetic materials are listed in
Table 1.
3.2. Magnetic Compatibility of Different Types of Magnetic Circuits
For a given magneto motive force (MMF)
Fm, the magnitude of magnetic flux
φ linking the windings is decided by the reluctance value
Rm of its magnetic circuit. The reluctance circuits of the core assemblies of commonly used high-power PETs of
Figure 3a,b could, respectively, be represented in
Figure 7a,b. Traditionally, the value of loss density
Pc is considered at the same value everywhere. It means, for one type of cores, the value of
Bm should be same everywhere. However, in the proposed idea of using the mixed core configuration, the value of loss density could be different. The expression of flux
φ linking the windings is,
For
Figure 7a, with MMF
Fm1, the total flux
in the central limb is,
It is desired to have , and it could be achieved when is met.
For the magnetic circuit of
Figure 7b, considering the same value of reluctance in each circuit, the total flux
in the core assembly could be expressed as,
where
im2 is the magnetizing current and
np2 is the number of turns at primary. Neglecting the dimensional tolerance of the cores, the dynamic value of
Rm of each parallel path plays an important role in flux distribution. When composite core segments are used, the dynamic behavior of
µr becomes critical for designing the magnetic circuit of
Figure 7b. The value of
µr could change differently with respect to operating value of
Bm,
fs, temperature, etc.
Characteristics of Different Soft Magnetic Materials
It is clear that for the magnetic compatibility, the role of relative permeability
µr is extremely important. For better thermal performance, the heat distribution (Equation (15)) should be proper, i.e., more heat needs to be transferred to the core surface area exposed to the environment. Here, both the core loss density
Pc and the thermal conductivity
K would play significant roles. The basic parameters of popular soft magnetic materials are listed in
Table 1 [
20]. For ferrite cores, the parametric variation with respect to temperature [
13] is large. Compared to others, its Curie temperature is much lower. The reduction in
Bsat value vs. temperature is also sharp. For integration of different core types into a magnetic circuit of PET, the value of
µr at different flux density and core temperature is important. The influence of temperature on
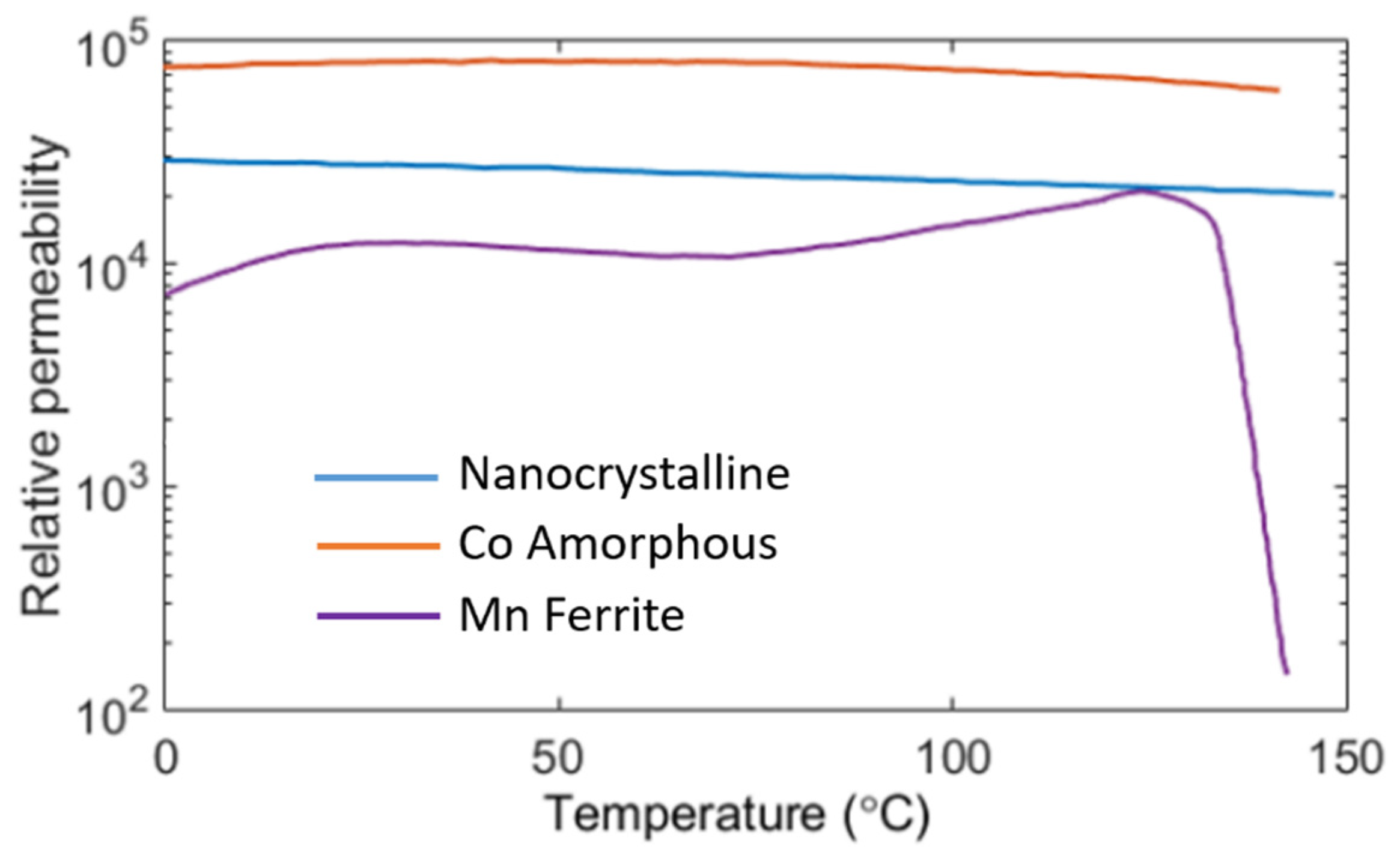
µr of different soft magnetic materials is shown in
Figure 8 [
43] where nanocrystalline cores appear to be parametrically robust. It is also known that, for ferrite cores, the value of
µr changes significantly with flux density [
4]. However, for nanocrystalline cores, as shown in
Figure 9, the value of
µr is mostly constant, and only at large values of
Bm is there gradual monotonic drooping in its value. Moreover, compared to other soft magnetic materials, the core loss of nanocrystalline cores at a particular frequency is comparatively less at any flux density (shown in
Figure 10). Therefore, due to the parametric robustness, small core loss density, superior thermal conductivity, higher saturation flux density and high Curie temperature, the nanocrystalline cores of proper ribbon thickness are superior candidates for mixed-core configuration. It would not disturb the behavior of the magnetic circuit. If geometry permits, Fe-based nanocrystalline cores could ideally be suitable as flux integrators for medium- to high-frequency PETs. The drooping characteristics of
µr, as shown in
Figure 9, make these cores suitable for integration into a magnetic circuit where the MMF feeds several parallel magnetic circuits (shown in
Figure 3b).
3.3. Figure of Merit of Mixed-Core Magnetic Circuit for Series Reluctance Model
It is known that for the PET of
Figure 3a, the bulk of total power loss
Ptot is concentrated around the central I-section EFGH. The hot spot temperature in the core and the primary winding, in particular, would reside in and around the I-section [
3]. In order to properly utilize the large surface area of core exposed to the ambient medium for thermal convection and radiation, there needs to be an improvement in spreading the heat loss in the core by thermal conduction. A higher value of thermal conductivity
K of core in the I-section EFGH would help remove the concentrated heat loss to the surface area of the magnetic circuit. As detailed in Equation (15), more power loss could be channelized if,
The value of Pc of the I-section is small.
The value of K of soft magnetic material used in the I-section is more.
The value of thermal resistance in coil former is reduced.
The first two features could simply be achieved if the central I-section is replaced by a geometrically compatible core material (shown in
Figure 11a) with superior features so that the value of
(see Equation (13)) in the I-section of core is reduced. The compatibility of the new material would be based on its dynamic magnetic parameters, core loss density
Pc and the value of
K. From the magnetic characteristics point of view, the role of I-section is not complicated. The
Figure of Merit of the new core material for the I-section could be gauged by a suitability parameter
Score(SR); it is introduced in simple form as,
When compared with parent material, a smaller value of
Pc and the same or larger value of
K would result in superior distribution of heat in core. The permeability
µrn of new I-section should be around the same value as
µrp, i.e., of parent material. The value of
K and other relevant parameters for different core materials are listed in
Table 1.
3.4. Figure of Merit of Mixed-Core for Parallelly Connected Magnetic Circuit
Multiple toroidal cores are stacked (shown in
Figure 3b) to support the desired flux level in the core of IHT. It is difficult to remove the heat loss from each core uniformly. The surface area of inner cores exposed to the ambient medium is relatively much less. It could lead to differential temperature rise across the core segments. In practice, the inner cores are found to be hotter. Like the PET of
Figure 11a, here as well, as shown in the arrangement of
Figure 11b, the problem could be reduced if the inner cores are replaced by a superior material. For a magnetic circuit where cores are used in parallel, it is difficult to establish the desired flux density everywhere. The dynamic value of relative permeability of each core being integrated would play an important role. To find the compatibility of the new core material for parallel reluctance model, and also to take care of sharing of flux, the suitability factor
Score of Equation (19) is modified to,
where
µr(
Bm) is the value of
µr of core at the operating value of
Bm. It takes care of the distribution of flux when the condition
is met. As shown in
Figure 9, nanocrystalline cores support such characteristics. Furthermore, as shown in
Figure 8, the value of
µr for this core is also stable against temperature.