Magnetic Vortex Core String Gyrotropic Oscillations in Thick Cylindrical Dots
Abstract
:1. Introduction
2. Theory
2.1. Stability of the Magnetic Vortex State in Cylindrical Geometry
2.2. The Generalized Thiele Equation of Motion of the Magnetic Soliton Center
3. Magnetic Vortex String Gyrotropic Dynamics
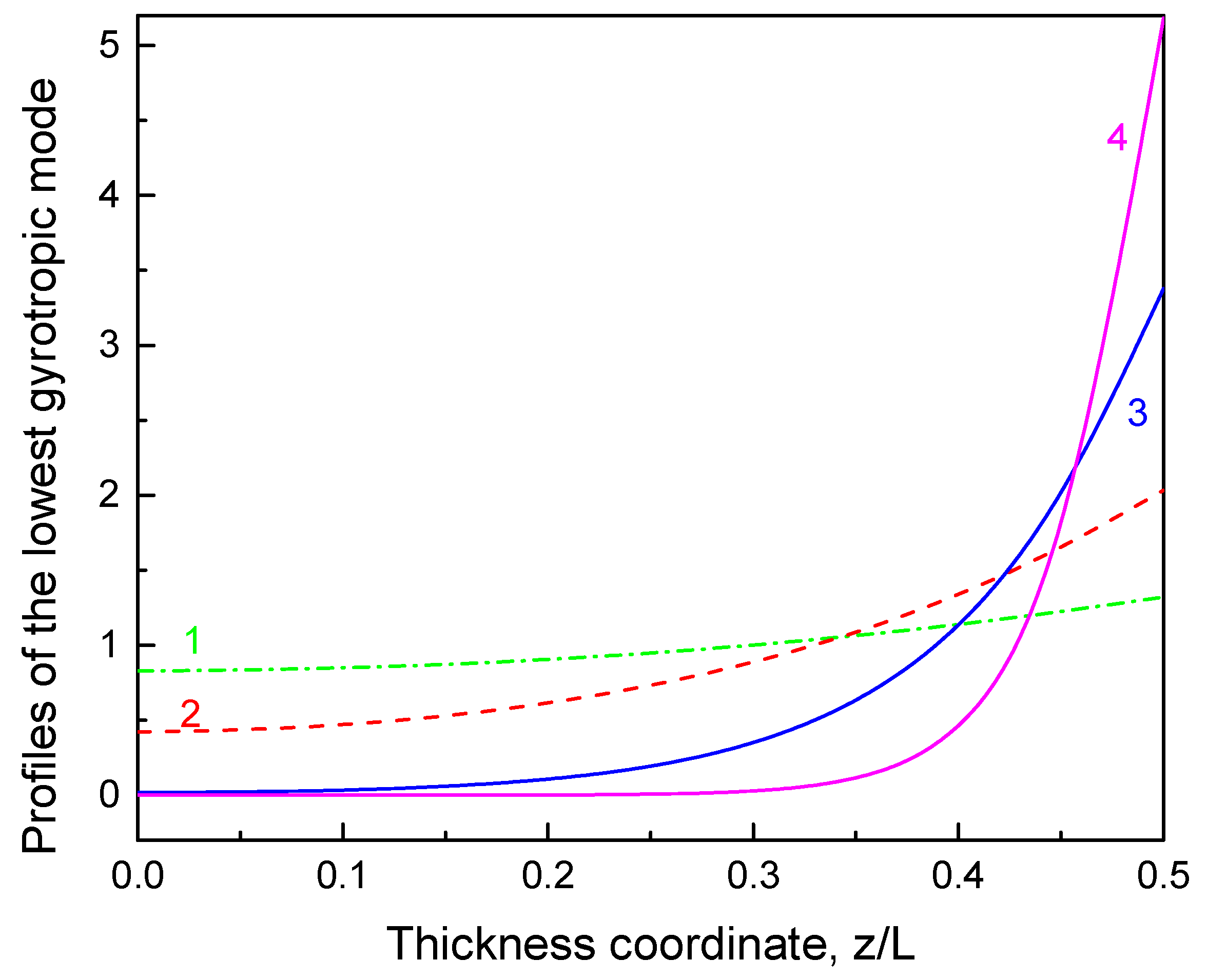
3.1. The Lowest Frequency Gyrotropic Mode
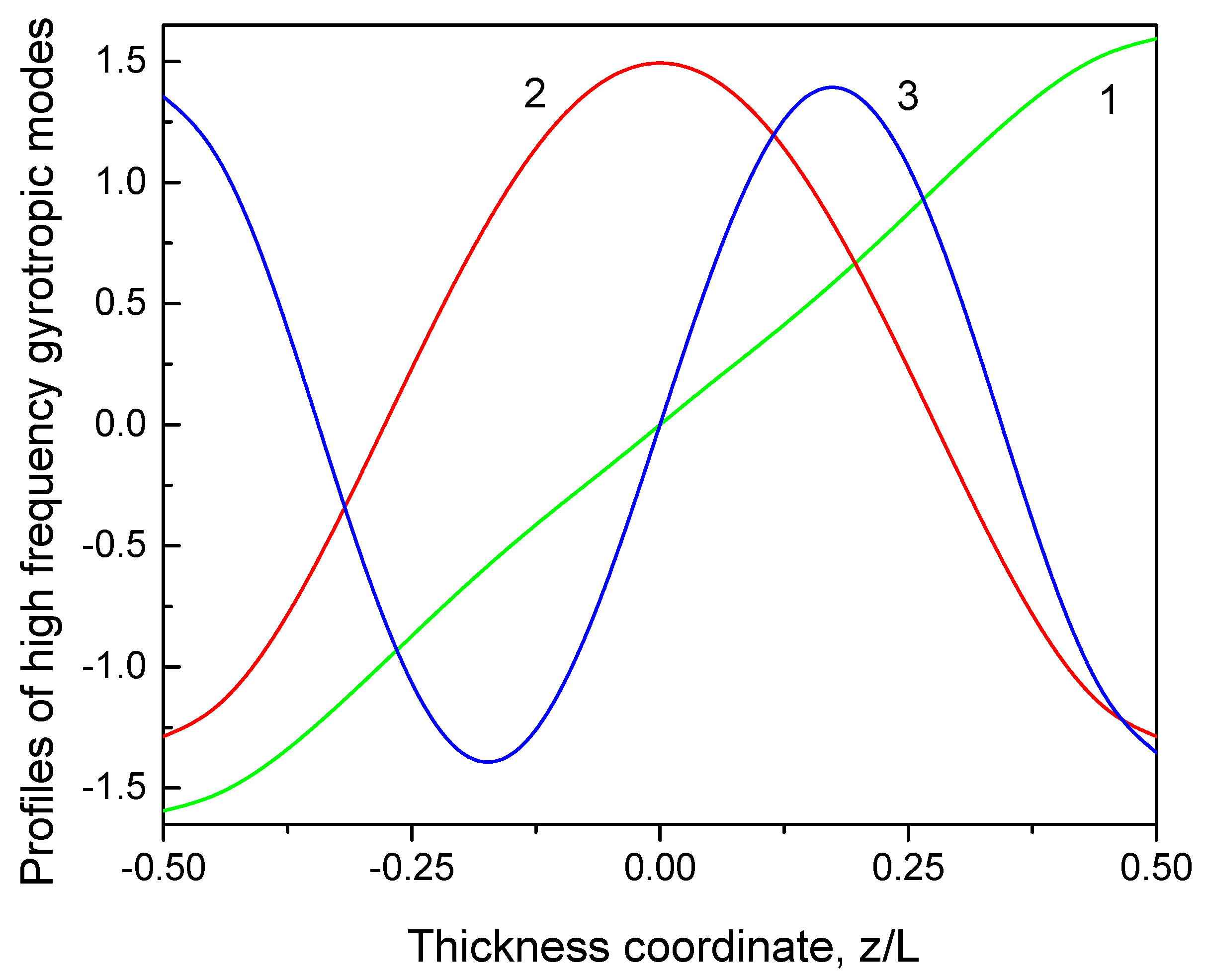
3.2. High-Frequency Vortex Gyrotropic Modes
3.3. The Role of the Vortex Mass in the Gyrotropic Dynamics
4. Discussion and Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shinjo, T.; Okuno, T.; Hassdorf, R.; Shigeto, K.; Ono, T. Magnetic vortex core observation in circular dots of permalloy. Science 2000, 289, 930–932. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Guslienko, K.Y. Magnetic vortex state stability, reversal and dynamics in restricted geometries. J. Nanosci. Nanotechn. 2008, 8, 2745–2761. [Google Scholar] [CrossRef]
- Sampaio, J.; Cros, V.; Rohart, S.; Thiaville, A.; Fert, A. Nucleation, stability and current-induced motion of isolated magnetic skyrmions in nanostructures. Nat. Nanotechn. 2013, 8, 839. [Google Scholar] [CrossRef] [PubMed]
- Fert, A.; Reyren, N.; Cros, V. Magnetic skyrmions: Advances in physics and potential applications. Nat. Rev. Mat. 2017, 2, 17031. [Google Scholar] [CrossRef]
- Guslienko, K.Y. Magnetic vortices and skyrmions. J. Magnetics 2019, 4, 549–567. [Google Scholar] [CrossRef]
- Kent, N.; Reynolds, N.; Raftrey, D.; Campbell, I.T.G.; Virasawmy, S.; Dhuey, S.; Chopdekar, R.V.; Hierro-Rodriguez, A.; Sorrentino, A.; Pereiro, E.; et al. Creation and observation of Hopfions in magnetic multilayer systems. Nat. Commun. 2021, 12, 1562. [Google Scholar] [CrossRef]
- Tejo, F.; Hernández, H.R.; Chubykalo-Fesenko, O.; Guslienko, K.Y. The Bloch point 3D topological charge induced by the magnetostatic interaction. Sci. Reports 2021, 11, 21714. [Google Scholar] [CrossRef]
- Guslienko, K.Y.; Ivanov, B.A.; Novosad, V.; Otani, Y.; Shima, H.; Fukamichi, K. Eigenfrequencies of vortex state excitations in magnetic submicron-size disks. J. Appl. Phys. 2002, 91, 8037–8039. [Google Scholar] [CrossRef]
- Park, J.P.; Eames, P.; Engebretson, D.M.; Berezovsky, J.; Crowell, P.A. Imaging of spin dynamics in closure domain and vortex structures. Phys. Rev. B 2003, 67, 020403. [Google Scholar] [CrossRef] [Green Version]
- Choe, S.-B.; Acremann, Y.; Scholl, A.; Bauer, A.; Doran, A.; Sthr, J.; Padmore, H.A. Vortex Core-Driven Magnetization Dynamics. Science 2004, 304, 420–422. [Google Scholar] [CrossRef] [Green Version]
- Novosad, V.; Fradin, F.Y.; Roy, P.E.; Buchanan, K.S.; Guslienko, K.Y.; Bader, S.D. Magnetic vortex resonance in patterned ferromagnetic dots. Phys. Rev. B 2005, 72, 024455. [Google Scholar] [CrossRef] [Green Version]
- Pribiag, V.S.; Krivorotov, I.N.; Fuchs, G.D.; Braganca, P.M.; Ozatay, O.; Sankey, J.C.; Buhrman, R.A. Magnetic vortex oscillator driven by d.c. spin-polarized current. Nat. Phys. 2007, 3, 498–503. [Google Scholar] [CrossRef]
- Mistral, Q.; Van Kampen, M.; Hrkac, G.; Kim, J.-V.; Devolder, T.; Crozat, P.; Chappert, C.; Lagae, L.; Schrefl, T. Current-driven vortex oscillations in metallic nanocontacts. Phys. Rev. Lett. 2008, 100, 257201. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dussaux, A.; Georges, B.; Grollier, J.; Cros, V.; Khvalkovskiy, A.V.; Fukushima, A.; Fert, A. Large microwave generation from current-driven magnetic vortex oscillators in magnetic tunnel junctions. Nat. Commun. 2010, 1, 8. [Google Scholar] [CrossRef] [Green Version]
- Guslienko, K.Y.; Sukhostavtes, O.V.; Berkov, D.V. Nonlinear magnetic vortex dynamics in a circular nanodot excited by spin-polarized current. Nanoscale Res. Lett. 2014, 9, 386. [Google Scholar] [CrossRef] [Green Version]
- Boust, F.; Vukadinovic, N. Micromagnetic simulations of vortex-state excitations in soft magnetic nanostructures. Phys. Rev. B 2004, 70, 172408. [Google Scholar] [CrossRef]
- Guslienko, K.Y.; Hoffmann, A. Field evolution of tilted vortex cores in exchange-biased ferromagnetic dots. Phys. Rev. Lett. 2006, 97, 107203. [Google Scholar] [CrossRef]
- Yan, M.; Hertel, R.; Schneider, C.M. Calculations of three-dimensional magnetic normal modes in mesoscopic Permalloy prisms with vortex structure. Phys. Rev. B 2007, 76, 094407. [Google Scholar] [CrossRef] [Green Version]
- Ding, J.; Kakazei, G.N.; Liu, X.M.; Guslienko, K.Y.; Adeyeye, A.O. Higher order vortex gyrotropic modes in circular ferromagnetic nanodots. Sci. Rep. 2014, 4, 4796. [Google Scholar] [CrossRef]
- Ding, J.; Kakazei, G.N.; Liu, X.M.; Guslienko, K.Y.; Adeyeye, A.O. Intensity inversion of vortex gyrotropic modes in thick ferromagnetic nanodots. Appl. Phys. Lett. 2014, 104, 192405. [Google Scholar] [CrossRef]
- Usov, N.A.; Peschany, S.E. Vortex magnetization distribution in a thin ferromagnetic cylinder. Phys. Met. Metallogr. 1994, 78, 591. [Google Scholar]
- Metlov, K.L.; Guslienko, K.Y. Stability of magnetic vortex in soft magnetic nano-sized circular cylinder. J. Magn. Magn. Mat. 2002, 242–245, 1015. [Google Scholar] [CrossRef] [Green Version]
- Thiele, A.A. Steady-state motion of magnetic domains. Phys. Rev. Lett. 1973, 30, 230. [Google Scholar] [CrossRef]
- McLachlan, N.W. Theory and Application of Mathieu Functions, 1st ed.; Dover: New York, NY, USA, 1964. [Google Scholar]
- Bateman, H. Higher Transcendental Functions; McGraw-Hill: New York, NY, USA, 1955; Volume 3. [Google Scholar]
- Olver, F.W.J. Introduction to Asymptotics and Special Functions; Academic Press: New York, NY, USA; London, UK, 1974. [Google Scholar]
- Guslienko, K.Y.; Kakazei, G.N.; Ding, J.; Liu, X.M.; Adeyeye, A.O. Giant moving vortex mass in thick magnetic nanodots. Sci. Rep. 2015, 5, 13881. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Guslienko, K.Y.; Aranda, G.R.; Gonzalez, J. Topological gauge field in nanomagnets: Spin-wave excitations over a slowly moving magnetization background. Phys. Rev. B Condens. Matter 2010, 81, 014414. [Google Scholar] [CrossRef] [Green Version]
- Park, J.P.; Crowell, P.A. Interactions of spin waves with a magnetic vortex. Phys. Rev. Lett. 2005, 95, 167201. [Google Scholar] [CrossRef] [Green Version]
- Büttner, F.; Moutafis, C.; Schneider, M.; Krüger, B.; Günther, C.M.; Geilhufe, J.; Eisebitt, S. Dynamics and inertia of skyrmionic spin structures. Nat. Phys. 2015, 11, 225. [Google Scholar] [CrossRef]
- Verba, R.V.; Hierro-Rodriguez, A.; Navas, D.; Ding, J.; Liu, X.M.; Adeyeye, A.O.; Guslienko, K.Y.; Kakazei, G.N. Spin-wave excitation modes in thick vortex-state circular ferromagnetic nanodots. Phys. Rev. B 2016, 93, 214437. [Google Scholar] [CrossRef]
- Bondarenko, A.V.; Bunyaev, S.A.; Shukla, A.K.; Apolinario, A.; Singh, N.; Navas, D.; Guslienko, K.Y.; Adeyeye, A.O.; Kakazei, G.N. Dominant higher-order vortex gyromodes in dome-shaped magnetic nanodots. 2022, the manuscript is in preparation. The corresponding author is Kakazei, G.N. Instituto de Física de Materiales Avanzados, Nanotecnología y Fotónica (IFIMUP) y Departamento de Física e Astronomía, Universidade de Porto, 4099-002 Porto, Portugal.


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guslienko, K. Magnetic Vortex Core String Gyrotropic Oscillations in Thick Cylindrical Dots. Magnetism 2022, 2, 239-250. https://doi.org/10.3390/magnetism2030018
Guslienko K. Magnetic Vortex Core String Gyrotropic Oscillations in Thick Cylindrical Dots. Magnetism. 2022; 2(3):239-250. https://doi.org/10.3390/magnetism2030018
Chicago/Turabian StyleGuslienko, Konstantin. 2022. "Magnetic Vortex Core String Gyrotropic Oscillations in Thick Cylindrical Dots" Magnetism 2, no. 3: 239-250. https://doi.org/10.3390/magnetism2030018
APA StyleGuslienko, K. (2022). Magnetic Vortex Core String Gyrotropic Oscillations in Thick Cylindrical Dots. Magnetism, 2(3), 239-250. https://doi.org/10.3390/magnetism2030018




