Spatial Patterns in Fibrous Materials: A Metrological Framework for Pores and Junctions
Abstract
1. Introduction
2. Materials and Methods
2.1. Methods
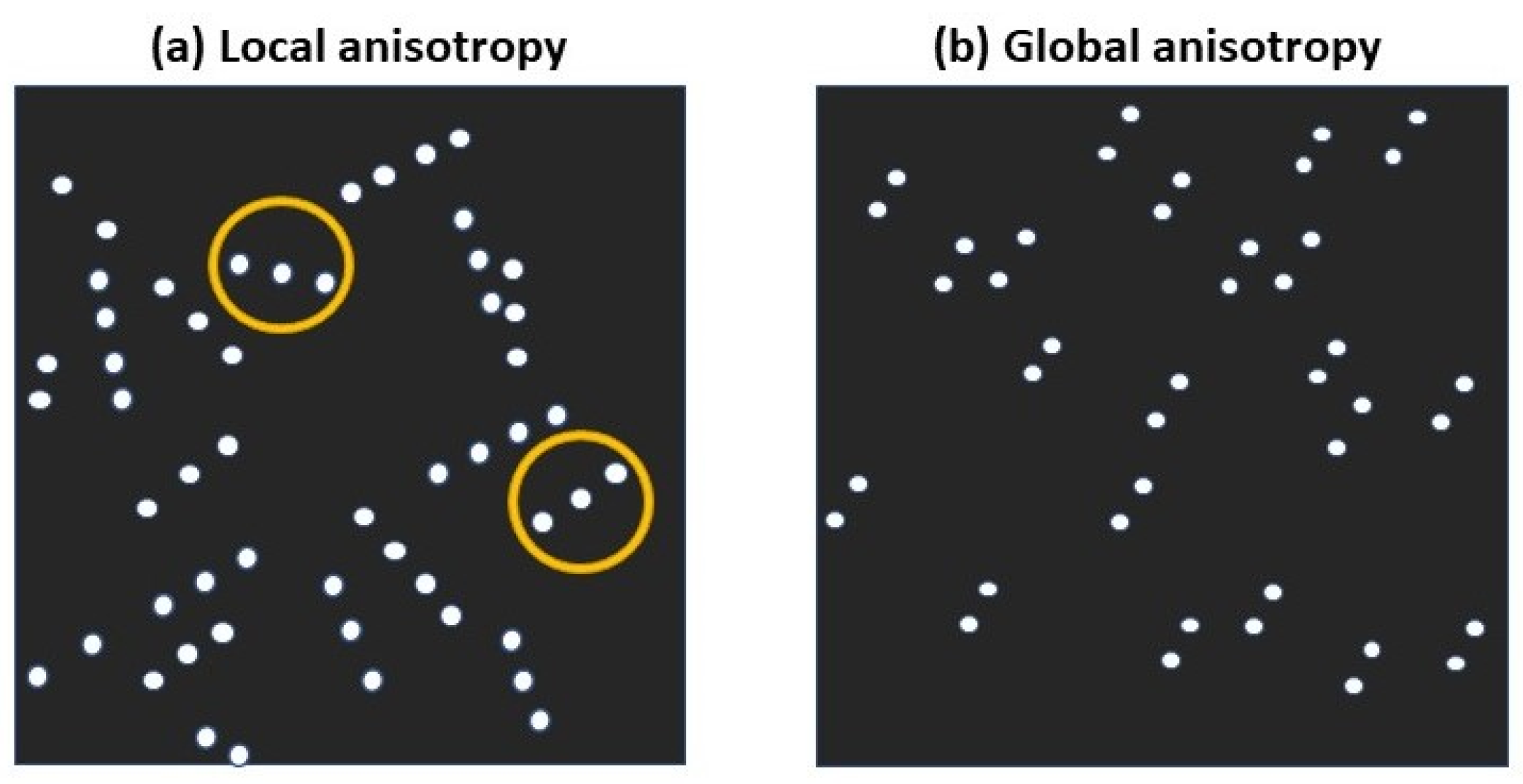
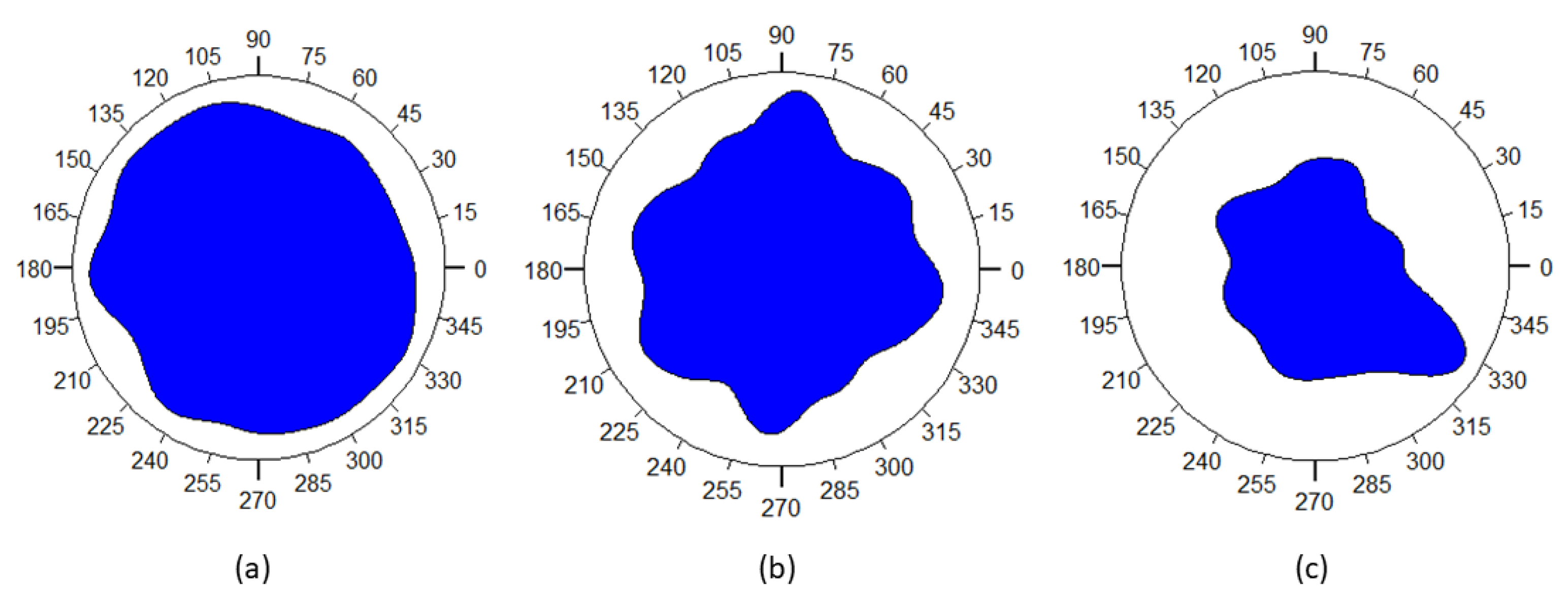
2.1.1. Global and Local Anisotropy
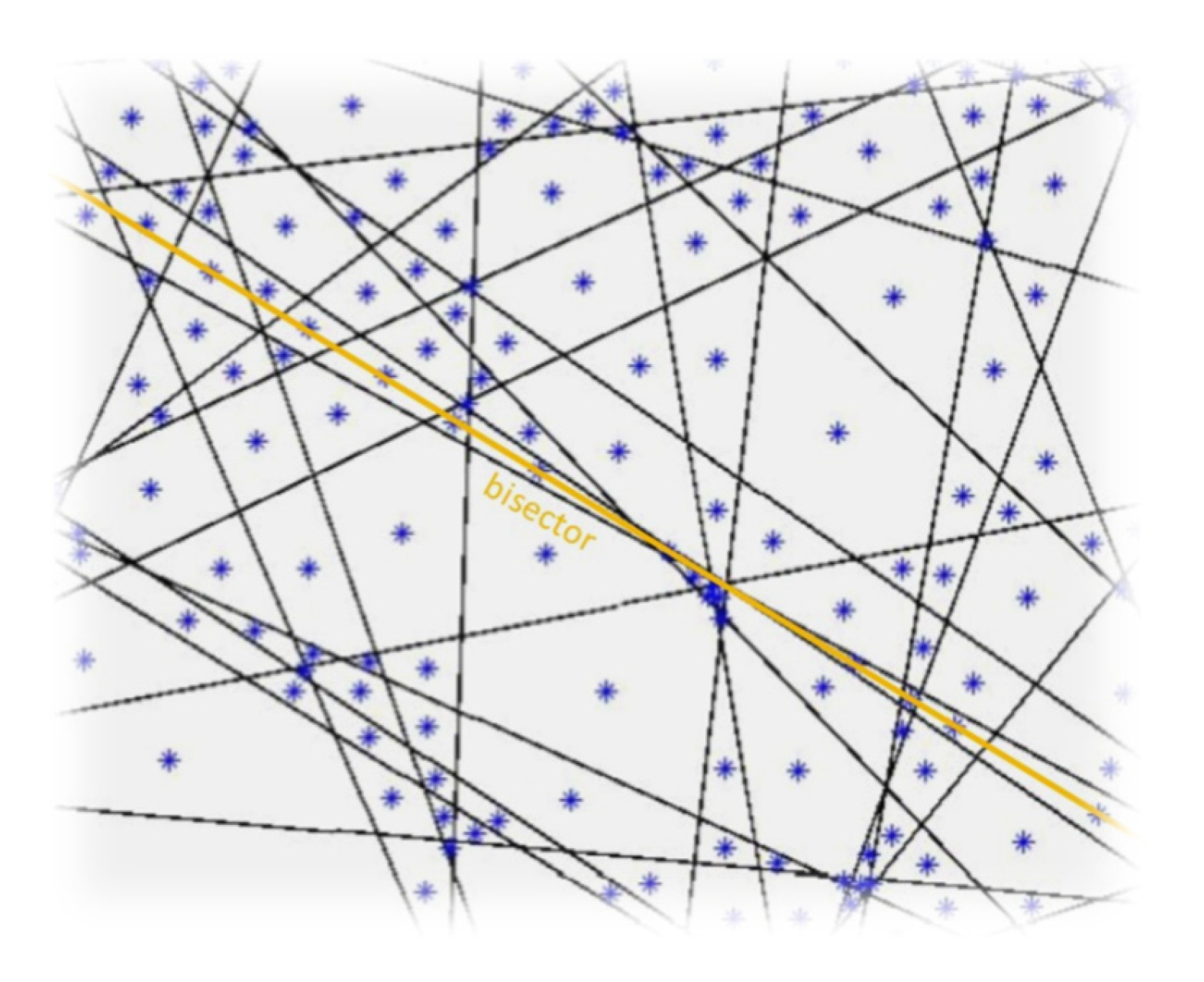
2.1.2. Nearest Neighbor Index (NNI)
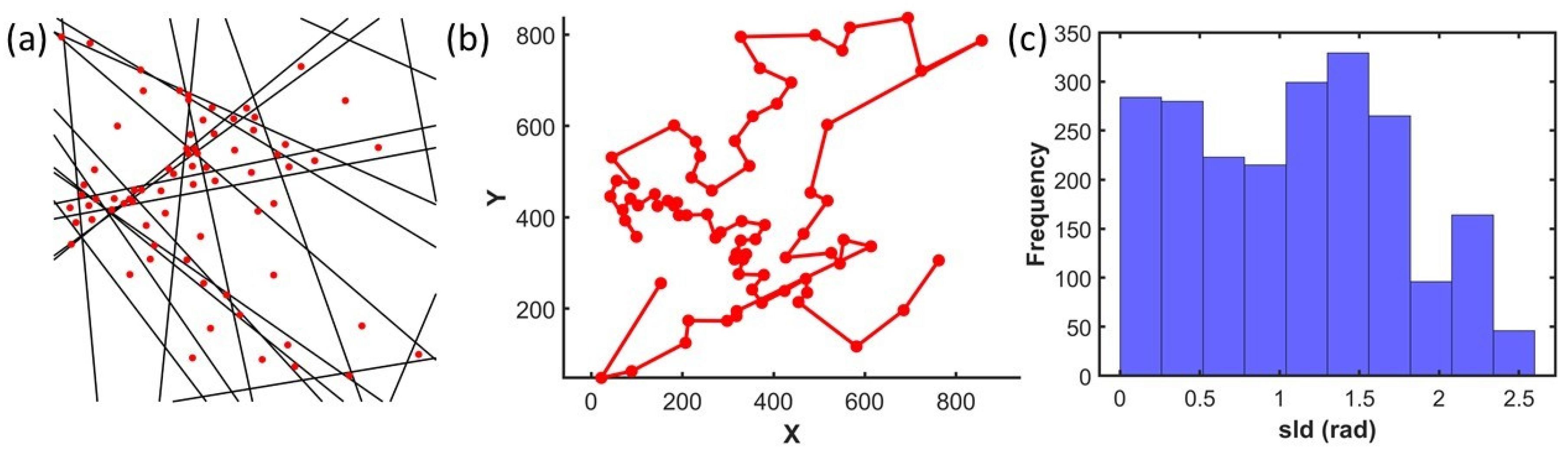
2.1.3. Nearest Neighbor Orientation Distribution
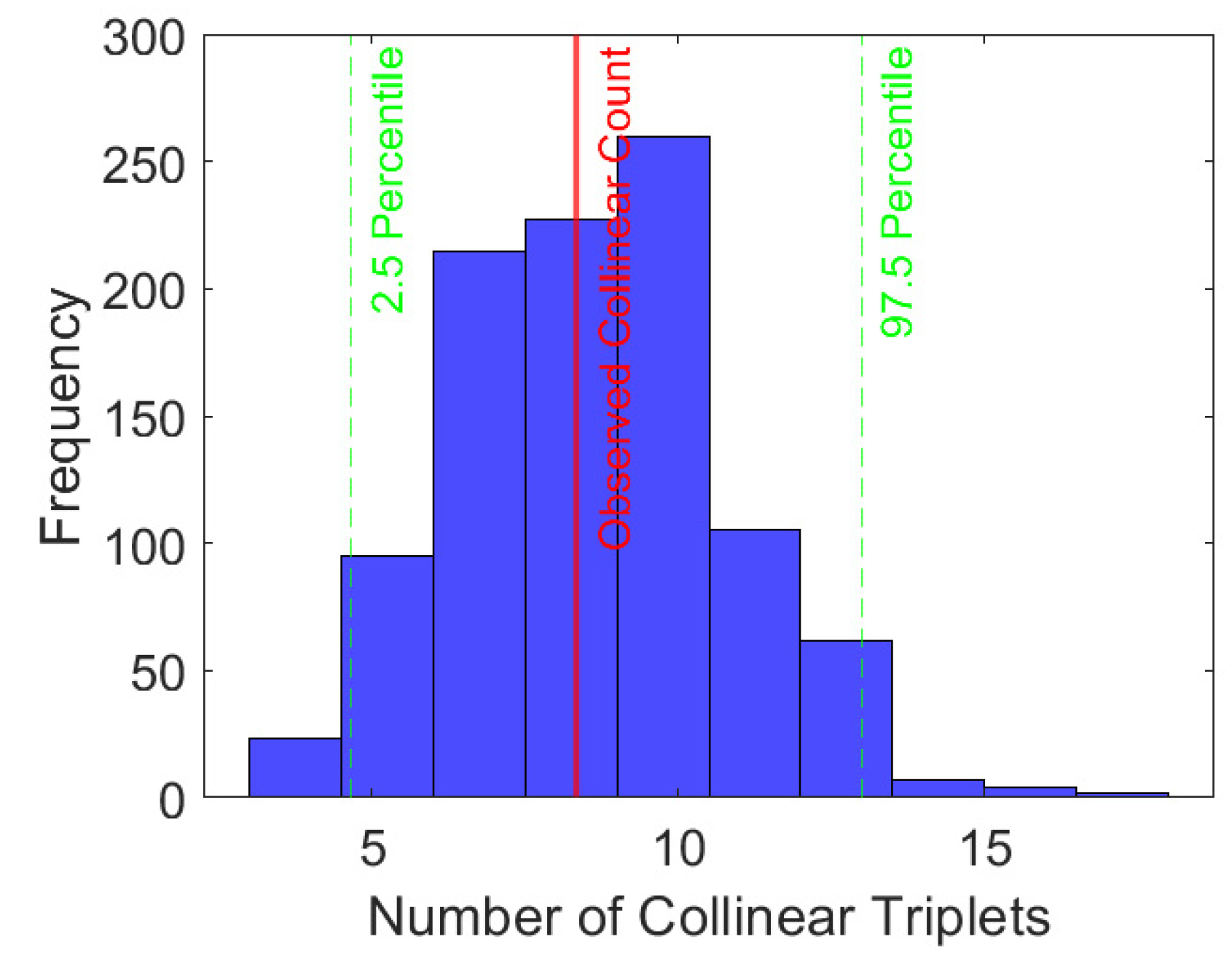
2.1.4. Local Collinearity Characterization (LCL)
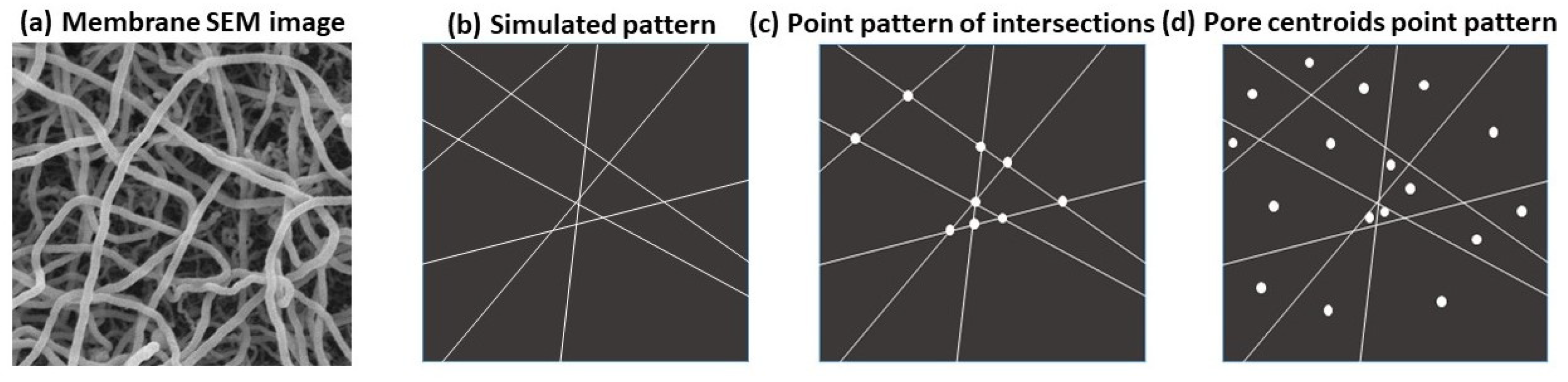
2.2. Materials
Analyzed SEM Images and Processing
3. Results
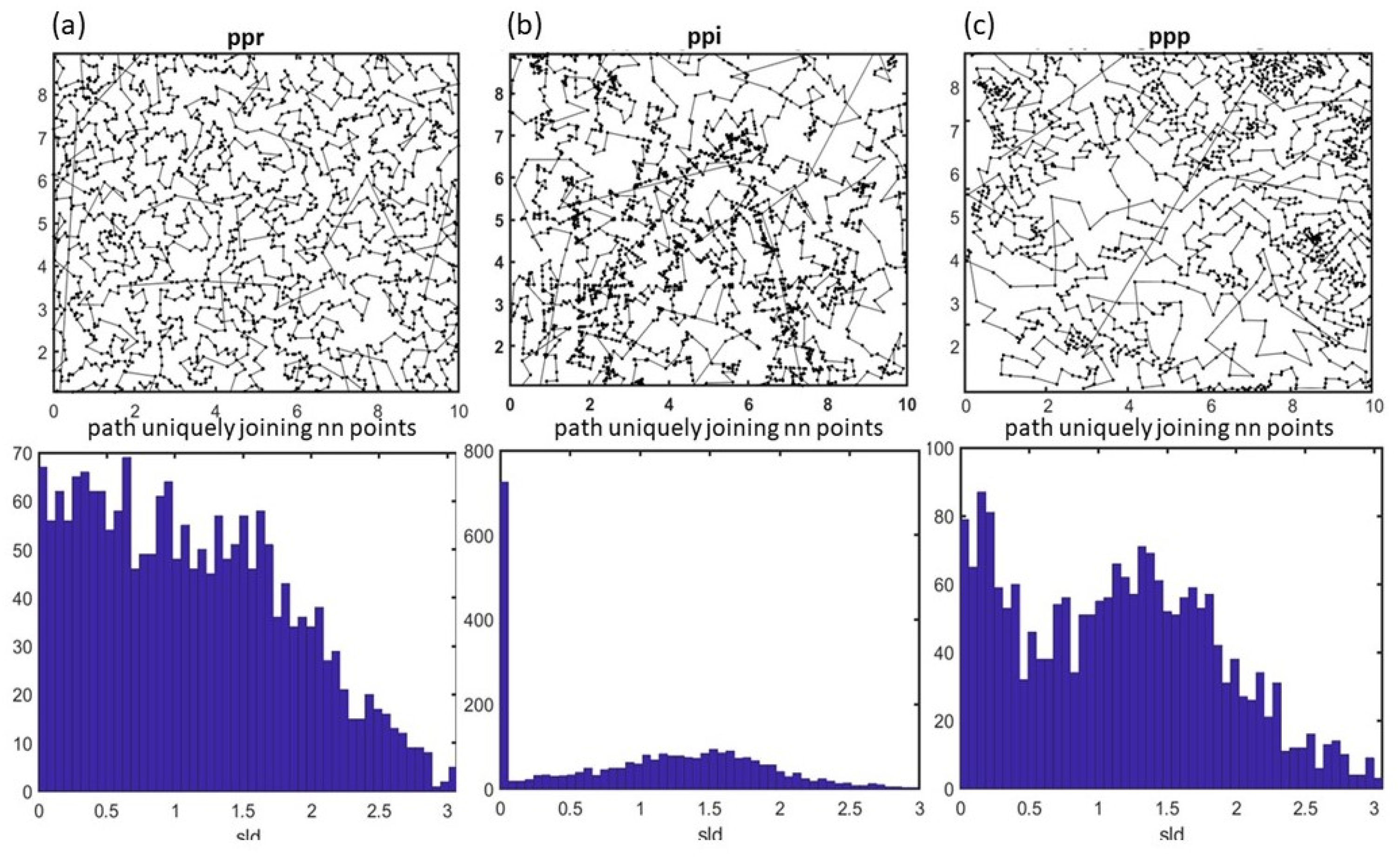
3.1. Characterizing Simulated Intersection and Pore Point Patterns
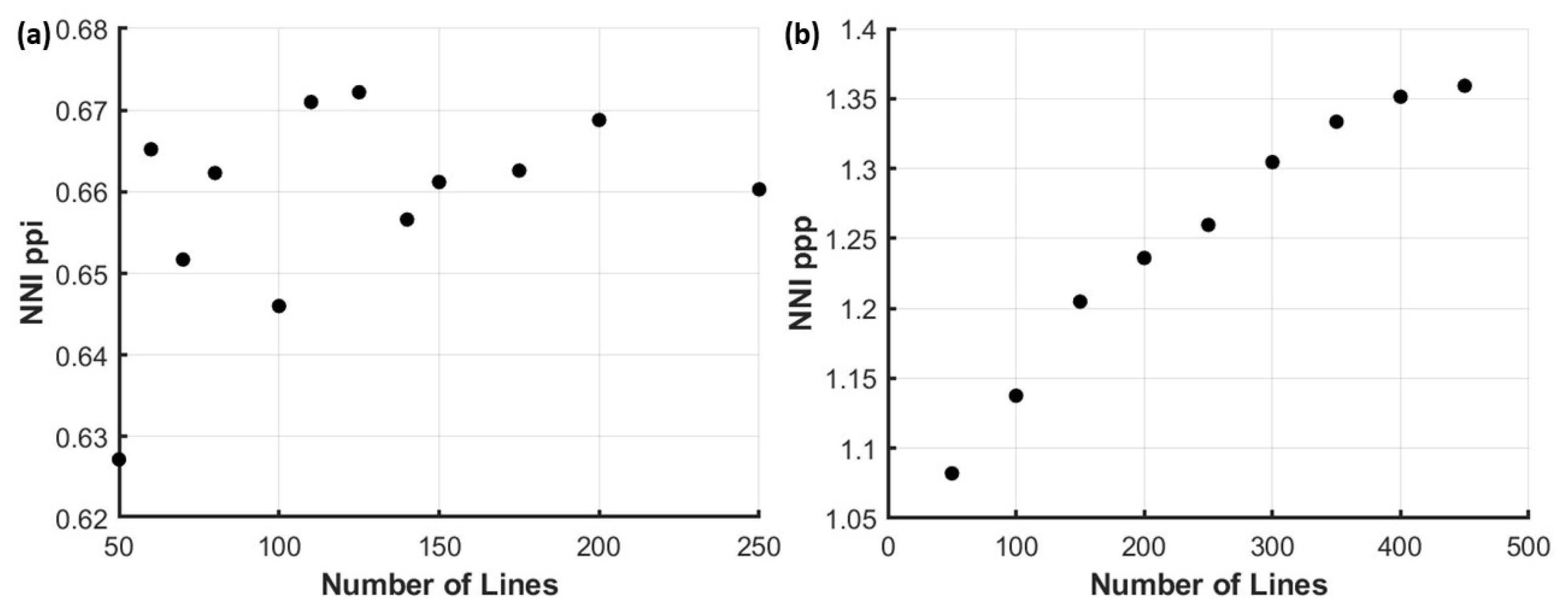
3.1.1. NNI
3.1.2. Nearest Neighbor Orientation Distribution
3.1.3. Local Collinearity
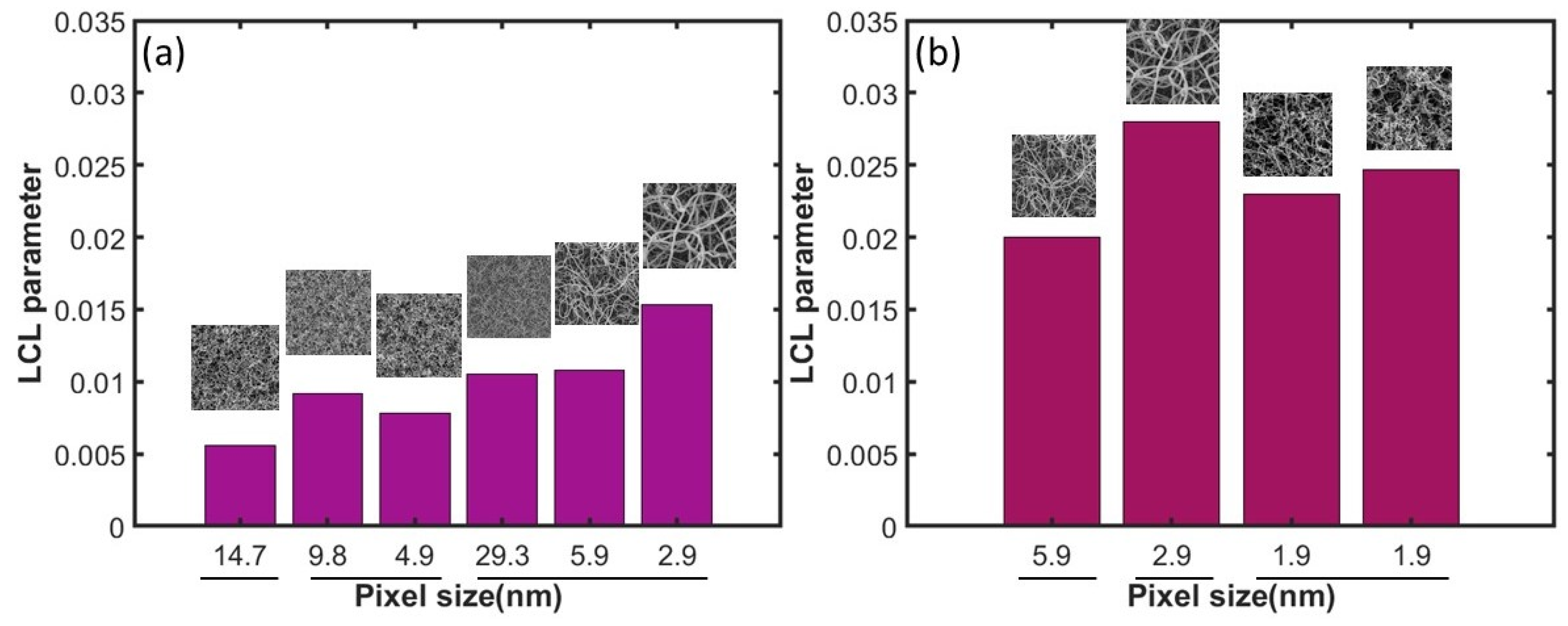
3.2. Characterizing Pore Point Patterns of Experimental Membrane Images
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Eichhorn, S.J.; Dufresne, A.; Aranguren, M.; Marcovich, N.E.; Capadona, J.R.; Rowan, S.J.; Weder, C.; Thielemans, W.; Roman, M.; Renneckar, S.; et al. Review: Current international research into cellulose nanofibres and nanocomposites. J. Mater. Sci. 2010, 45, 1–33. [Google Scholar] [CrossRef]
- Paul, D.R.; Robeson, L.M. Polymer nanotechnology: Nanocomposites. Polymer 2008, 49, 3187–3204. [Google Scholar] [CrossRef]
- Pham, Q.P.; Sharma, U.; Mikos, A.G. Electrospinning of polymeric nanofibers for tissue engineering applications: A review. Tissue Eng. 2006, 12, 11971211. [Google Scholar] [CrossRef]
- Fu, W.; Liu, Z.; Feng, B.; Hu, R.; He, X.; Wang, H.; Yin, M.; Huang, H.; Zhang, H.; Wang, W. Electrospun gelatin/PCL and collagen/PLCL scaffolds for vascular tissue engineering. Int. J. Nanomed. 2014, 9, 2335–2344. [Google Scholar] [CrossRef] [PubMed]
- Shen, H.; Zhou, Z.; Wang, H.; Zhang, M.; Han, M.; Durkin, D.P.; Shuai, D.; Shen, Y. Development of Electrospun Nanofibrous Filters for Controlling Coronavirus Aerosols. Environ. Sci. Technol. Lett. 2021, 8, 545–550. [Google Scholar] [CrossRef] [PubMed]
- Alcoutlabi, M.; Lee, H.; Watson, J.V.; Zhang, X. Preparation and properties of nanofiber-coated composite membranes as battery separators via electrospinning. J. Mater. Sci. 2013, 48, 2690–2700. [Google Scholar] [CrossRef]
- Cisneros, C.G.; Bloemen, V.; Mignon, A. Synthetic, Natural, and Semisynthetic Polymer Carriers for Controlled Nitric Oxide Release in Dermal Applications: A Review. Polymers 2021, 13, 760. [Google Scholar] [CrossRef]
- Wang, S.; Liu, C.; Wang, F.; Yin, X.; Yu, J.; Zhang, S.; Ding, B. Recent Advances in Ultrafine Fibrous Materials for Effective Warmth Retention. Adv. Fiber Mater. 2022, 5, 847–867. [Google Scholar] [CrossRef]
- Huang, X.L.; Dou, S.X.; Wang, Z.M. Fibrous cathode materials for advanced sodium-chalcogen batteries. Energy Storage Mater. 2022, 45, 265–280. [Google Scholar] [CrossRef]
- Tadrist, L.; Miscevic, M.; Rahli, O.; Topin, F. About the use of fibrous materials in compact heat exchangers. Exp. Therm. Fluid Sci. 2004, 28, 193–199. [Google Scholar] [CrossRef]
- Matsuo, T. Fibre materials for advanced technical textiles. Text. Prog. 2008, 40, 87–121. [Google Scholar] [CrossRef]
- Sampson, W.W. Modelling Stochastic Fibrous Materials with Mathematica; Springer: London, UK, 2009. [Google Scholar]
- Cho, Y.-S.; Roh, S.H. Sol–gel synthesis of porous titania fibers by electro-spinning for water purification. J. Dispers. Sci. Technol. 2018, 39, 33–44. [Google Scholar] [CrossRef]
- Kulkarni, A.; Bambole, V.A.; Mahanwar, P.A. Electrospinning of Polymers, Their Modeling and Applications. Polym. Plast. Technol. Eng. 2015, 49, 427–441. [Google Scholar] [CrossRef]
- Diaz-Alvarez, A.; Higuchi, R.; Sanz-Leon, P.; Marcus, I.; Shingaya, Y.; Stieg, A.Z.; Gimzewski, J.K.; Kuncic, Z.; Nakayama, T. Emergent dynamics of neuromorphic nanowire networks. Sci. Rep. 2019, 9, 14920. [Google Scholar] [CrossRef] [PubMed]
- Hochstetter, J.; Zhu, R.; Loeffler, A.; Diaz-Alvarez, A.; Nakayama, T.; Kuncic, Z. Avalanches and edge-of-chaos learning in neuromorphic nanowire networks. Nat. Commun. 2021, 12, 4008. [Google Scholar] [CrossRef]
- Jagota, M.; Tansu, N. Conductivity of Nanowire Arrays under Random and Ordered Orientation Configurations. Sci. Rep. 2015, 5, 10219. [Google Scholar] [CrossRef]
- Kuncic, Z.; Nakayama, T. Neuromorphic nanowire networks: Principles, progress and future prospects for neuro-inspired information processing. Adv. Phys. X 2021, 6, 1894234. [Google Scholar] [CrossRef]
- Lu, M.; Beguin, F.; Frackowiak, E. Supercapacitors: Materials. Systems and Applications; Wiley: Hoboken, NJ, USA, 2013. [Google Scholar]
- Leong, K.F.; Chua, C.K.; Sudarmadji, N.; Yeong, W.Y. Engineering functionally graded tissue engineering scaffolds. J. Mech. Behav. Biomed. Mater. 2008, 1, 140–152. [Google Scholar] [CrossRef] [PubMed]
- Dullien, F.A. Porous Media: Fluid Transport and Pore Structure; Academic Press: Cambridge, MA, USA, 2012. [Google Scholar]
- Liu, Q.; Lu, Z.; Hu, Z.; Li, J. Finite element analysis on tensile behaviour of 3D random fibrous materials: Model description and meso-level approach. Mater. Sci. Eng. A 2013, 587, 36–45. [Google Scholar] [CrossRef]
- Hull, R.; Keblinski, P.; Lewis, D.; Maniatty, A.; Meunier, V.; Oberai, A.A.; Picu, C.R.; Samuel, J.; Shephard, M.S.; Tomozawa, M.; et al. Stochasticity in materials structure, properties, and processing—A review. Appl. Phys. Rev. 2019, 5, 011302. [Google Scholar] [CrossRef]
- Sampson, W.W. Spatial variability of void structure in thin stochastic fibrous materials. Model. Simul. Mater. Sci. Eng. 2011, 20, 015008. [Google Scholar] [CrossRef]
- Eichhorn, S.J.; Sampson, W.W. Statistical geometry of pores and statistics of porous nanofibrous assemblies. J. R. Soc. Interface 2005, 2, 309–318. [Google Scholar] [CrossRef]
- Miles, R.E. Random polygons determined by random lines in a plane, II. Proc. Nat. Acad. Sci. USA 1964, 52, 1157–1160. [Google Scholar] [CrossRef]
- Abdel-Ghani, M.; Davies, G. Simulation of non-woven fibre mats and the application to coalescers. Chem. Eng. Sci. 1985, 40, 117–129. [Google Scholar] [CrossRef]
- Dodson, C.T.J.; Sampson, W.W. Planar Line Processes for Void and Density Statistics in Thin Stochastic Fibre Networks. J. Stat. Phys. 2007, 129, 311–322. [Google Scholar] [CrossRef]
- Chiu, S.N.; Stoyan, D.; Kendall, W.S.; Mecke, J. Stochastic Geometry and Its Applications; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Barthélemy, M. Spatial networks. Phys. Rep. 2011, 499, 1–101. [Google Scholar] [CrossRef]
- Illian, J.; Penttinen, A.; Stoyan, H.; Stoyan, D. Statistical Analysis and Modelling of Spatial Point Patterns; Wiley: Hoboken, NJ, USA, 2008. [Google Scholar]
- Shah, P.; Hou, Y.; Butt, H.-J.; Kappl, M. Nanofilament-Coated Superhydrophobic Membranes Show Enhanced Flux and Fouling Resistance in Membrane Distillation. ACS Appl. Mater. Interfaces 2023, 15, 55119–55128. [Google Scholar] [CrossRef]
- Dodson, C.T.J. Spatial variability and the theory of sampling in random fibrous networks. J. R. Stat. Soc. Ser. B 1971, 33, 88–94. [Google Scholar] [CrossRef]
- Rajala, T.; Redenbach, C.; Särkkä, A.; Sormani, M. A review on anisotropy analysis of spatial point patterns. Spat. Stat. 2018, 28, 141–168. [Google Scholar] [CrossRef]
- Pinder, D.A.; Witherick, M.E. The Principles, Practice and Pitfalls of Nearest-Neighbour Analysis. Geography 1972, 57, 277–288. [Google Scholar]
- Philo, C.; Philo, P. 2.15 or Not 2.15? An Historical-Analytical Inquiry into the Nearest-Neighbor Statistic. Geogr. Anal. 2021, 54, 333–356. [Google Scholar] [CrossRef]
- Mavrogonatos, A.; Papia, E.-M.; Constantoudis, V. Measuring the randomness of micro- and nanostructure spatial distributions: Effects of Scanning Electron Microscope image processing and analysis. J. Microsc. 2022, 289, 48–57. [Google Scholar] [CrossRef] [PubMed]
- Myllymäki, M.; Mrkvička, T.; Grabarnik, P.; Seijo, H.; Hahn, U. Global Envelope Tests for Spatial Processes. J. R. Stat. Soc. Ser. B Stat. Methodol. 2017, 79, 381–404. [Google Scholar] [CrossRef]
- Wiegand, T.; Grabarnik, P.; Stoyan, D. Envelope tests for spatial point patterns with and without simulation. Ecosphere 2016, 7, e01365. [Google Scholar] [CrossRef]
- Brownrigg, D.R.K. The weighted median filter. Commun. ACM 1984, 27, 807–818. [Google Scholar] [CrossRef]
- Ronse, C.; Heijmans, H.J.A.M. The algebraic basis of mathematical morphology: II. Openings and closings. CVGIP Image Underst. 1991, 54, 74–97. [Google Scholar] [CrossRef]
- Papia, E.-M.; Constantoudis, V.; Ioannou, D.; Zeniou, A.; Hou, Y.; Shah, P.; Kappl, M.; Gogolides, E. Quantifying pore spatial uniformity: Application on membranes before and after plasma etching. Micro Nano Eng. 2024, 24, 100278. [Google Scholar] [CrossRef]


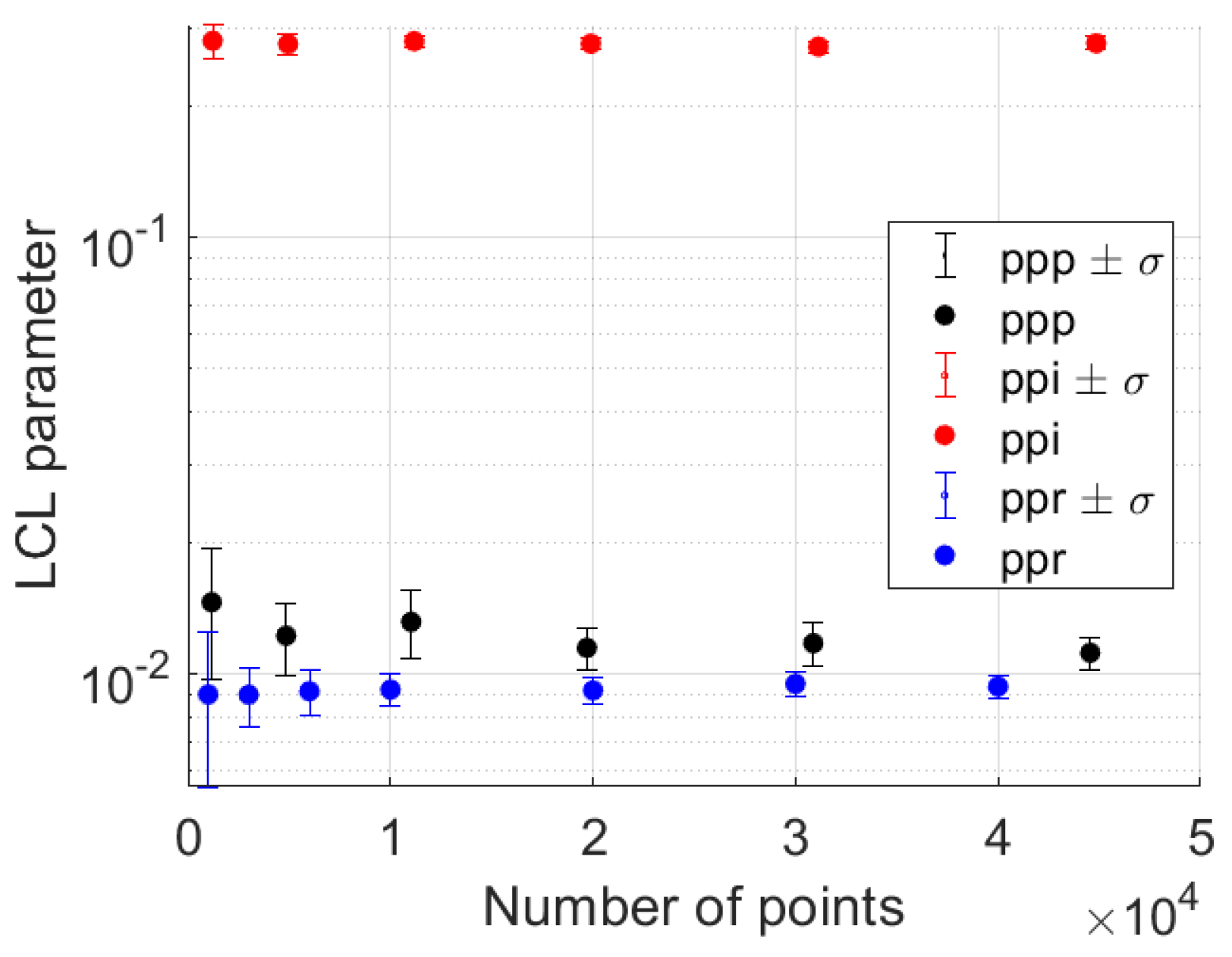
| ppp | ||
|---|---|---|
| Distance Threshold | Loss Percentage (%) | % of Original LCL Value |
| 1/5 L | 7.852 ± 1.12 | 90.3 ± 13.7 |
| 2/5 L | 2.47 ± 2.16 | 94.2 ± 12.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Papia, E.-M.; Constantoudis, V.; Hou, Y.; Shah, P.; Kappl, M.; Gogolides, E. Spatial Patterns in Fibrous Materials: A Metrological Framework for Pores and Junctions. Metrology 2025, 5, 26. https://doi.org/10.3390/metrology5020026
Papia E-M, Constantoudis V, Hou Y, Shah P, Kappl M, Gogolides E. Spatial Patterns in Fibrous Materials: A Metrological Framework for Pores and Junctions. Metrology. 2025; 5(2):26. https://doi.org/10.3390/metrology5020026
Chicago/Turabian StylePapia, Efi-Maria, Vassilios Constantoudis, Youmin Hou, Prexa Shah, Michael Kappl, and Evangelos Gogolides. 2025. "Spatial Patterns in Fibrous Materials: A Metrological Framework for Pores and Junctions" Metrology 5, no. 2: 26. https://doi.org/10.3390/metrology5020026
APA StylePapia, E.-M., Constantoudis, V., Hou, Y., Shah, P., Kappl, M., & Gogolides, E. (2025). Spatial Patterns in Fibrous Materials: A Metrological Framework for Pores and Junctions. Metrology, 5(2), 26. https://doi.org/10.3390/metrology5020026








