Functional and Metrological Issues in Arterial Simulators for Biomedical Testing Applications: A Review
Abstract
1. Introduction
2. Fields of Applications for Arterial Simulators
3. Framework of the Measurement Devices Commonly Employed in Experimental Set-Ups and Their General Critical Issues
4. Experimental Set-Ups in Artery Bifurcation Studies
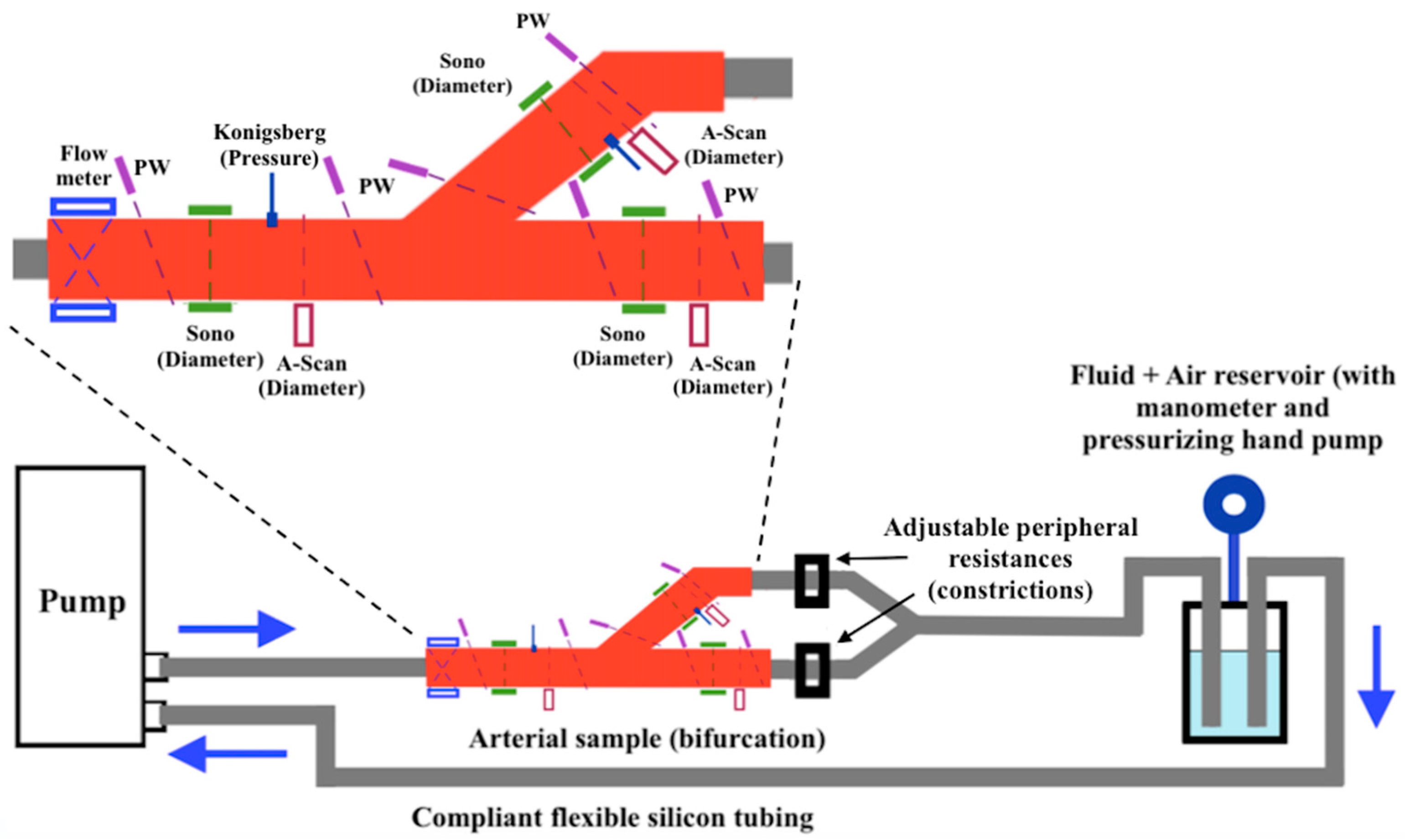
4.1. Suarez-Bagnasco’s Multiparameter Measurement System for Artery Bifurcation
4.2. Bale-Glickman’s Carotid Bifurcation Flow Phantoms
4.3. Botnar’s Study on In Vitro Carotid Artery Bifurcation and MRI Techniques
5. Experimental Set-Ups in Stenosis and Plaque Studies
5.1. Bertolotti’s Experimental Test Bench for Stenosed Coronary Bypasses
5.2. Brunette’s Study on a Stenosis Arterial Phantom
6. Experimental Set-Ups in Arterial Flow Studies
6.1. The Design of an Arterial Simulator for Backward Flows by Feng and Whir
6.2. The Investigation of Blood Flow in Experimental Set-Up of Micro-Vessels
6.3. Laser Doppler Anemometer (LDA) with an Aortic Flow Phantom Designed by Liepsch
6.4. The Employment of the PIV Method for Flow Behavior Analysis
7. Experimental Set-Ups in Clinical Practice and Device Testing
7.1. Miller’s Pulsatile Blood Vessel System for Clinician Practice
7.2. Gwak and the Improvement of Conventional Mock Circulatory Systems
7.3. Legendre and Vilchez-Monge’s Design of an Artificial Cardiovascular Simulator for Device Testing
7.4. The Design of a Reliable Implantable Circulatory Support Device by Yoshino
8. Experimental Set-Ups in Pulse Pressure Studies and Biomechanics
8.1. Fronek’s In Vitro Investigation on Pulse Pressure Waves
8.2. Lou’s Experimental Apparatus for the Determination of Pressure Wave Velocity
8.3. Horsten’s Investigation of Wave Propagation in Viscoelastic Tubes
8.4. The Introduction of Computer-Aided Simulators for the Study of Vessel Biomechanical Behavior
9. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Wilkins, E.; Wilson, L.; Wickramasinghe, K.; Bhatnagar, P.; Leal, J.; Luengo-Fernandez, R.; Burns, R.; Rayner, M.; Townsend, N. European Cardiovascular Disease Statistics 2017; European Heart Network: Bruselles, Belgium, 2017. [Google Scholar]
- Weaver, C.G.; Clement, F.M.; Campbell, N.R.; James, M.T.; Klarenbach, S.W.; Hemmelgarn, B.R.; Tonelli, M.; McBrien, K.A. Healthcare Costs Attributable to Hypertension: Canadian Population-Based Cohort Study. J. Hypertens. 2015, 66, 502–508. [Google Scholar] [CrossRef]
- Laurent, S.; Cockcroft, J.; Van Bortel, L.; Boutouyrie, P.; Giannattasio, C.; Hayoz, D.; Pannier, B.; Vlachopoulos, C.; Wilkinson, I.; Struijker-Boudier, H. Expert Consensus Document on Arterial Stiffness: Methodological Issues and Clinical Applications. Eur. Heart J. 2006, 27, 2588–2605. [Google Scholar] [CrossRef] [PubMed]
- Fiori, G.; Fuiano, F.; Scorza, A.; Conforto, S.; Sciuto, S.A. Non-Invasive Methods for PWV Measurement in Blood Vessel Stiffness Assessment. IEEE Rev. Biomed. Eng. 2022, 15, 169–183. [Google Scholar] [CrossRef] [PubMed]
- Younessi Heravi, M.A.; Khalilzadeh, M.A. Designing and Constructing an Optical System to Measure Continuous and Cuffless Blood Pressure Using Two Pulse Signals. Iran. J. Med. Phys. 2014, 11, 215–223. [Google Scholar] [CrossRef]
- Murakami, K.; Yoshioka, M.; Ozawa, J. Non-Contact Pulse Transit Time Measurement Using Imaging Camera, and Its Relation to Blood Pressure. In Proceedings of the 14th IAPR International Conference on Machine Vision Applications (MVA), Tokyo, Japan, 18–22 May 2015; pp. 414–417. [Google Scholar]
- Zaki, W.S.W.; Correia, R.; Korposh, S.; Hayes-Gill, B.R.; Morgan, S.P. Development of Tubular Cardiovascular Phantom System for Pulse Transit Time Simulation. Int. J. Recent. Technol. Eng. 2019, 8, 291–296. [Google Scholar] [CrossRef]
- Fuiano, F.; Fiori, G.; Vurchio, F.; Scorza, A.; Sciuto, S.A. Transit Time Measurement of a Pressure Wave through an Elastic Tube Based on LVDT Sensors. In Proceedings of the 24th IMEKO TC4 International Symposium 22nd International Workshop on ADC and DAC Modelling and Testing IMEKO TC-4 2020, Palermo, Italy, 14–16 September 2020. [Google Scholar]
- Klanchar, M.; Tarbell, J.M.; Wang, D.-M. In Vitro Study of the Influence of Radial Wall Motion on Wall Shear Stress in an Elastic Tube Model of the Aorta. Circ. Res. 1990, 66, 1624–1635. [Google Scholar] [CrossRef]
- Almeida, V.G.; Pereira, H.C.; Pereira, T.; Figueiras, E.; Borges, E.; Cardoso, J.M.R.; Correia, C. Piezoelectric Probe for Pressure Waveform Estimation in Flexible Tubes and Its Application to the Cardiovascular System. Sens. Actuator A 2011, 169, 217. [Google Scholar] [CrossRef]
- Dagdeviren, C.; Su, Y.; Joe, P.; Yona, R.; Liu, Y.; Kim, Y.-S.; Huang, Y.; Damadoran, A.R.; Xia, J.; Martin, L.W.; et al. Conformable Amplified Lead Zirconate Titanate Sensors with Enhanced Piezoelectric Response for Cutaneous Pressure Monitoring. Nat. Commun. 2014, 5, 4496. [Google Scholar] [CrossRef]
- Ma, Y.; Choi, J.; Hourlier-Fargette, A.; Xue, Y.; Chung, H.U.; Lee, J.Y.; Wang, X.; Xie, Z.; Kang, D.; Wang, H.; et al. Relation between Blood Pressure and Pulse Wave Velocity for Human Arteries. Proc. Natl. Acad. Sci. USA 2018, 115, 11144–11149. [Google Scholar] [CrossRef]
- Lu, N.; Lu, C.; Yang, S.; Rogers, J. Highly Sensitive Skin-Mountable Strain Gauges Based Entirely on Elastomers. Adv. Funct. Mater. 2012, 22, 4044–4050. [Google Scholar] [CrossRef]
- Navghare, S. Design and Simulation of MEMS Sensor for Detection of Arterial Pulse. Int. J. Comput. Appl. Technol. 2018, 17, 7247–7260. [Google Scholar] [CrossRef]
- Bae, J.-H.; Jeon, Y.J.; Kim, J.Y.; Kim, J.U. New Assessment Model of Pulse Depth Based on Sensor Displacement in Pulse Diagnostic Devices. Evid. Based Complement. Alternat. Med. 2013, 2013, 938641. [Google Scholar] [CrossRef] [PubMed]
- Laqua, D.; Pollnow, S.; Fischer, J.; Ley, S.; Husar, P. A Phantom with Pulsating Artificial Vessels for Non-Invasive Fetal Pulse Oximetry. Annu. Int. Conf. IEEE Eng. Med. Biol. Soc. 2014, 2014, 5631–5634. [Google Scholar] [CrossRef]
- Lantos, C.; Erd, B.; Nyers, T.; Pandula, Z.; Halász, G. The Examination of Deformation of a Silicone Elastomer Tube. In Proceedings of the 14th International Conference on Fluid Flow Technologies, Budapest, Hungary, 9–12 September 2009. [Google Scholar]
- Berrios, J.C.; Pedersen, P.C. Ultrasonic Measurement of Forced Diameter Variations in an Elastic Tube. Ultrason. Imaging 1994, 16, 124–142. [Google Scholar] [CrossRef]
- Vlachopoulos, C.; O’Rourke, M.; Nichols, W.W. McDonald’s Blood Flow in Arteries: Theoretical, Experimental and Clinical Principles, 6th ed.; CRC Press: London, UK, 2012; p. 88. [Google Scholar]
- Wilson, J.S. Sensor Technology Handbook, 1st ed.; Elsevier: London, UK, 2005; pp. 22–31. [Google Scholar]
- Webster, J.G. Measurement, Instrumentation, and Sensors Handbook: Spatial, Mechanical, Thermal, and Radiation Measurement, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2017; pp. 852–940. [Google Scholar]
- Bramwell, J.C.; Hill, A.V. The Velocity of Pulse Wave in Man. Proc. R. Soc. Lond. B Biol. Sci. 1922, 93, 298–306. [Google Scholar] [CrossRef]
- Suárez-Bagnasco, D.; Balay, G.; Cymberknop, L.; Armentano, R.L.; Negreira, C.A. Measurement System for an In-Vitro Characterization of the Biomechanics and Hemodynamics of Arterial Bifurcations. J. Phys. Conf. Ser. 2013, 421, 012018. [Google Scholar] [CrossRef]
- Bale-Glickman, J.; Selby, K.; Saloner, D.; Savaş, O. Experimental Flow Studies in Exact-Replica Phantoms of Atherosclerotic Carotid Bifurcations under Steady Input Conditions. J. Biomech. Eng. 2003, 125, 38–48. [Google Scholar] [CrossRef]
- Botnar, R.; Rappitsch, G.; Scheidegger, M.B.; Liepsch, D.; Perktold, K.; Boesiger, P. Hemodynamics in the Carotid Artery Bifurcation: A Comparison between Numerical Simulations and in Vitro MRI Measurements. J. Biomech. 2000, 33, 137–144. [Google Scholar] [CrossRef]
- Banks, J.; Bressloff, N.W. Turbulence Modeling in Three-Dimensional Stenosed Arterial Bifurcations. J. Biomech. Eng. 2007, 129, 40–50. [Google Scholar] [CrossRef]
- Ku, D.N.; Giddens, D.P. Laser Doppler Anemometer Measurements of Pulsatile Flow in a Model Carotid Bifurcation. J. Biomech. 1987, 20, 407–421. [Google Scholar] [CrossRef]
- Khir, A.W.; Parker, K.H. Measurements of Wave Speed and Reflected Waves in Elastic Tubes and Bifurcations. J. Biomech. 2002, 35, 775–783. [Google Scholar] [CrossRef]
- Bertolotti, C.; Deplano, V.; Fuseri, J.; Dupouy, P. Numerical and Experimental Models of Post-Operative Realistic Flows in Stenosed Coronary Bypasses. J. Biomech. 2001, 34, 1049–1064. [Google Scholar] [CrossRef]
- Deplano, V.; Siouffi, M. Experimental and Numerical Study of Pulsatile Flows through Stenosis: Wall Shear Stress Analysis. J. Biomech. 1999, 32, 1081–1090. [Google Scholar] [CrossRef]
- Tang, D.; Yang, C.; Walker, H.; Kobayashi, S.; Ku, D.N. Simulating Cyclic Artery Compression Using a 3D Unsteady Model with Fluid–Structure Interactions. Comput. Struct. 2002, 80, 1651–1665. [Google Scholar] [CrossRef]
- Brunette, J.; Mongrain, R.; Laurier, J.; Galaz, R.; Tardif, J.C. 3D Flow Study in a Mildly Stenotic Coronary Artery Phantom Using a Whole Volume PIV Method. Med. Eng. Phys. 2008, 30, 1193–1200. [Google Scholar] [CrossRef]
- Li, S.; Chin, C.; Thondapu, V.; Poon, E.K.W.; Monty, J.P.; Li, Y.; Ooi, A.S.H.; Tu, S.; Barlis, P. Numerical and Experimental Investigations of the Flow–Pressure Relation in Multiple Sequential Stenoses Coronary Artery. Int. J. Cardiovasc. Imaging 2017, 33, 1083–1088. [Google Scholar] [CrossRef]
- Filipovic, N.; Rosic, M.; Tanaskovic, I.; Parodi, O.; Fotiadis, D. Computer Simulation and Experimental Analysis of LDL Transport in the Arteries. Annu. Int. Conf. IEEE Eng. Med. Biol. Soc. 2011, 2011, 195–198. [Google Scholar] [CrossRef]
- Filipovic, N.; Zivic, M.; Obradovic, M.; Djukic, T.; Markovic, Z.; Rosic, M. Numerical and Experimental LDL Transport through Arterial Wall. Microfluid. Nanofluid. 2014, 16, 455–464. [Google Scholar] [CrossRef]
- Kenjereš, S. On Recent Progress in Modelling and Simulations of Multi-Scale Transfer of Mass, Momentum and Particles in Bio-Medical Applications. Flow Turbul. Combust 2016, 96, 837–860. [Google Scholar] [CrossRef]
- Brum, J.; Bia, D.; Benech, N.; Balay, G.; Armentano, R.; Negreira, C. Set up of a Cardiovascular Simulator: Application to the Evaluation of the Dynamical Behavior of Atheroma Plaques in Human Arteries. Phys. Procedia 2010, 3, 1095–1101. [Google Scholar] [CrossRef][Green Version]
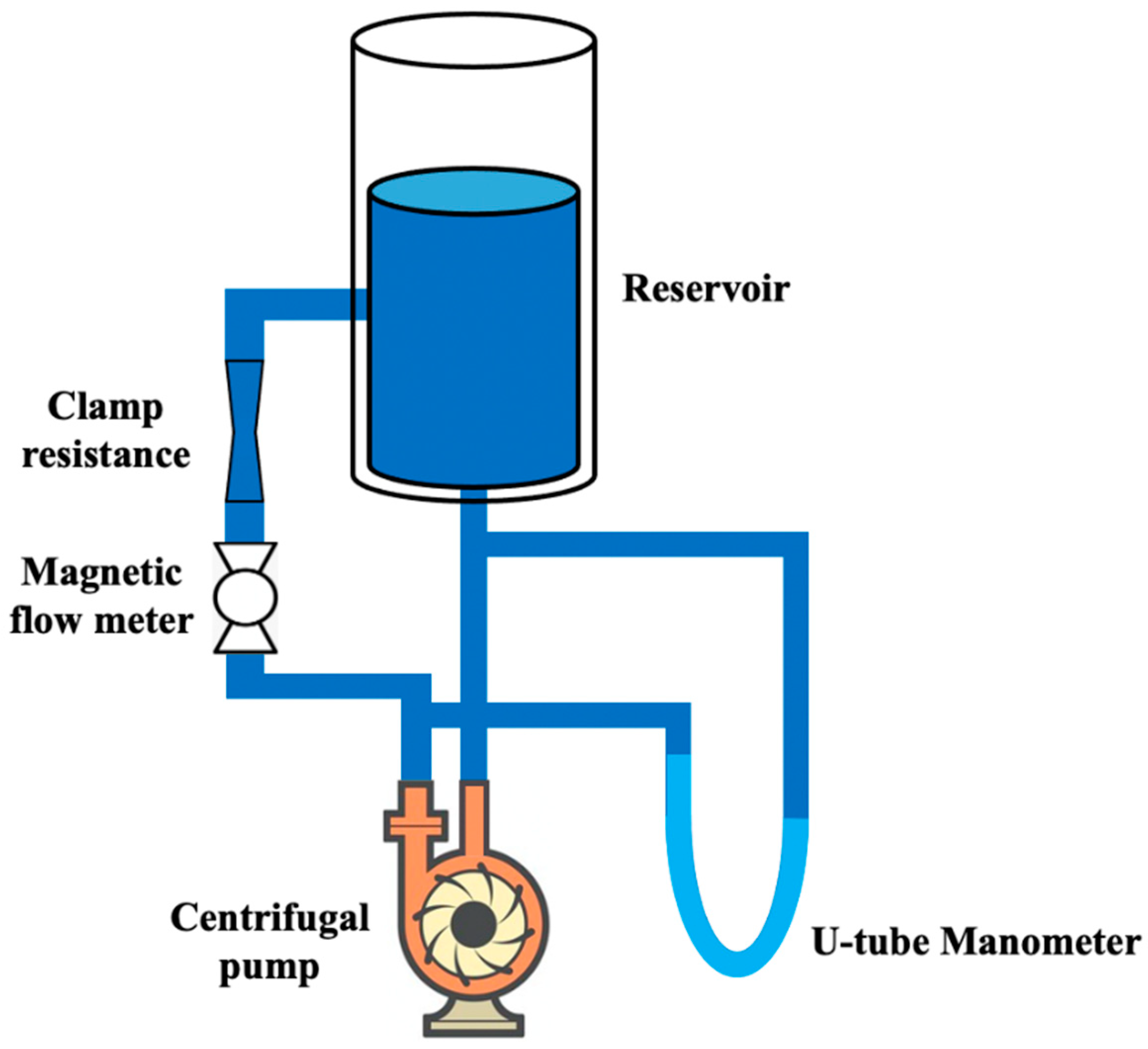
- Čanić, S.; Hartley, C.J.; Rosenstrauch, D.; Tambača, J.; Guidoboni, G.; Mikelić, A. Blood Flow in Compliant Arteries: An Effective Viscoelastic Reduced Model, Numerics, and Experimental Validation. Ann. Biomed. Eng. 2006, 34, 575–592. [Google Scholar] [CrossRef] [PubMed]
- Flaud, P.; Guesdon, P.; Fullana, J.-M. Experiments of Draining and Filling Processes in a Collapsible Tube at High External Pressure. Eur. Phys. J. Appl. Phys. 2012, 57, 31101. [Google Scholar] [CrossRef][Green Version]
- Bessems, D.; Giannopapa, C.G.; Rutten, M.C.M.; van de Vosse, F.N. Experimental Validation of a Time-Domain-Based Wave Propagation Model of Blood Flow in Viscoelastic Vessels. J. Biomech. 2008, 41, 284–291. [Google Scholar] [CrossRef] [PubMed]
- Wang, X. 1D Modeling of Blood Flow in Networks: Numerical Computing and Applications; Université Pierre et Marie Curie: Paris, France, 2014. [Google Scholar]
- Pontiga, F.; Gaytán, S.P. An Experimental Approach to the Fundamental Principles of Hemodynamics. Adv. Physiol. Educ. 2005, 29, 165–171. [Google Scholar] [CrossRef]
- Farsirotou, E.; Kasiteropoulou, D.; Stamatopoulou, D. Experimental Investigation of Fluid Flow in Horizontal Pipes System of Various Cross-Section Geometries. EPJ Web Conf. 2014, 67, 02026. [Google Scholar] [CrossRef]
- Kung, E.O. In-Vitro Experimental Validation of Finite Element Analysis of Blood Flow and Vessel Wall Dynamics. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 2010. [Google Scholar]
- Feng, J.; Khir, A.W. The Compression and Expansion Waves of the Forward and Backward Flows: An in-Vitro Arterial Model. Proc. Inst. Mech. Eng. Part H 2008, 222, 531–542. [Google Scholar] [CrossRef]
- Stergiou, Y.G.; Keramydas, A.T.; Anastasiou, A.D.; Mouza, A.A.; Paras, S.V. Experimental and Numerical Study of Blood Flow in μ-Vessels: Influence of the Fahraeus–Lindqvist Effect. Fluids 2019, 4, 143. [Google Scholar] [CrossRef]
- Holdsworth, D.W.; Rickey, D.W.; Drangova, M.; Miller, D.J.; Fenster, A. Computer-Controlled Positive Displacement Pump for Physiological Flow Simulation. Med. Biol. Eng. Comput. 1991, 29, 565–570. [Google Scholar] [CrossRef]
- Mouza, A.A.; Skordia, O.D.; Tzouganatos, I.D.; Paras, S.V. A Simplified Model for Predicting Friction Factors of Laminar Blood Flow in Small-Caliber Vessels. Fluids 2018, 3, 75. [Google Scholar] [CrossRef]
- Kung, E.O.; Les, A.S.; Figueroa, C.A.; Medina, F.; Arcaute, K.; Wicker, R.B.; McConnell, M.V.; Taylor, C.A. In Vitro Validation of Finite Element Analysis of Blood Flow in Deformable Models. Ann. Biomed. Eng. 2011, 39, 1947–1960. [Google Scholar] [CrossRef]
- Segers, P.; Verdonck, P. Role of Tapering in Aortic Wave Reflection: Hydraulic and Mathematical Model Study. J. Biomech. 2000, 33, 299–306. [Google Scholar] [CrossRef]
- Liepsch, D.; Moravec, S.; Baumgart, R. Some Flow Visualization and Laser-Doppler-Velocity Measurements in a True-to-Scale Elastic Model of a Human Aortic Arch—A New Model Technique. Biorheology 1992, 29, 563–580. [Google Scholar] [CrossRef] [PubMed]
- Shakeri, M.; Khodarahmi, I.; Sharp, M.K.; Amini, A.A. Optical Imaging of Steady Flow in a Phantom Model of Iliac Artery Stenosis: Comparison of CFD Simulations with PIV Measurements. In Proceedings of the Medical Imaging 2010: Biomedical Applications in Molecular, Structural, and Functional Imaging, San Diego, CA, USA, 14–16 February 2010; Volume 7626, p. 76260L. [Google Scholar] [CrossRef]
- Giurgea, C.; Bode, F.; Ioan Budiu, O.; Nascutiu, L.; Banyai, D.; Damian, M. Experimental Investigations of the Steady Flow through an Idealized Model of a Femoral Artery Bypass. EPJ Web Conf. 2014, 67, 02031. [Google Scholar] [CrossRef]
- Van’t Veer, M.; Geven, M.C.F.; Rutten, M.C.M.; van der Horst, A.; Aarnoudse, W.H.; Pijls, N.H.J.; van de Vosse, F.N. Continuous Infusion Thermodilution for Assessment of Coronary Flow: Theoretical Background and in Vitro Validation. Med. Eng. Phys. 2009, 31, 688–694. [Google Scholar] [CrossRef]
- Miller, S.F.; Sanz-Guerrero, J.; Dodde, R.E.; Johnson, D.D.; Bhawuk, A.; Gurm, H.S.; Shih, A.J. A Pulsatile Blood Vessel System for a Femoral Arterial Access Clinical Simulation Model. Med. Eng. Phys. 2013, 35, 1518–1524. [Google Scholar] [CrossRef] [PubMed]
- Huberts, W.; Van Canneyt, K.; Segers, P.; Eloot, S.; Tordoir, J.H.M.; Verdonck, P.; van de Vosse, F.N.; Bosboom, E.M.H. Experimental Validation of a Pulse Wave Propagation Model for Predicting Hemodynamics after Vascular Access Surgery. J. Biomech. 2012, 45, 1684–1691. [Google Scholar] [CrossRef]
- Westerhof, N.; Elzinga, G.; Sipkema, P. An Artificial Arterial System for Pumping Hearts. J. Appl. Physiol. 1971, 31, 776–781. [Google Scholar] [CrossRef]
- Timms, D.; Hayne, M.; McNeil, K.; Galbraith, A. A Complete Mock Circulation Loop for the Evaluation of Left, Right, and Biventricular Assist Devices. Artif. Organs 2005, 29, 564–572. [Google Scholar] [CrossRef]
- Bazan, O.; Ortiz, J.P. Experimental Validation of a Cardiac Simulator for in Vitro Evaluation of Prosthetic Heart Valves. Braz. J. Cardiovasc. Surg. 2016, 31, 151–157. [Google Scholar] [CrossRef][Green Version]
- Gwak, K.-W.; Paden, B.E.; Antaki, J.F.; Ahn, I.-S. Experimental Verification of the Feasibility of the Cardiovascular Impedance Simulator. IEEE Trans. Biomed. Eng. 2010, 57, 1176–1183. [Google Scholar] [CrossRef] [PubMed]
- Legendre, D.; Fonseca, J.; Andrade, A.; Biscegli, J.F.; Manrique, R.; Guerrino, D.; Prakasan, A.K.; Ortiz, J.P.; Lucchi, J.C. Mock Circulatory System for the Evaluation of Left Ventricular Assist Devices, Endoluminal Prostheses, and Vascular Diseases. Artif. Organs 2008, 32, 461–467. [Google Scholar] [CrossRef] [PubMed]
- Knierbein, B.; Reul, H.; Eilers, R.; Lange, M.; Kaufmann, R.; Rau, G. Compact Mock Loops of the Systemic and Pulmonary Circulation for Blood Pump Testing. Int. J. Artif. Organs 1992, 15, 40–48. [Google Scholar] [CrossRef]
- Van Canneyt, K.; Planken, R.N.; Eloot, S.; Segers, P.; Verdonck, P. Experimental Study of a New Method for Early Detection of Vascular Access Stenoses: Pulse Pressure Analysis at Hemodialysis Needle. Artif. Organs 2010, 34, 113–117. [Google Scholar] [CrossRef]
- Milo, S.; Rambod, E.; Gutfinger, C.; Gharib, M. Mitral Mechanical Heart Valves: In Vitro Studies of Their Closure, Vortex and Microbubble Formation with Possible Medical Implications. Eur. J. Cardiothorac. Surg. 2003, 24, 364–370. [Google Scholar] [CrossRef][Green Version]
- Pantalos, G.M.; Koenig, S.C.; Gillars, K.J.; Giridharan, G.A.; Ewert, D.L. Characterization of an Adult Mock Circulation for Testing Cardiac Support Devices. ASAIO J. 2004, 50, 37–46. [Google Scholar] [CrossRef]
- Patel, S.; Allaire, P.; Wood, G.; Adams, J. Design and Construction of a Mock Human Circulatory System. In Proceedings of the Summer Bioengineering Conference, Sonesta Beach Resort, Key Biscayne, FL, USA, 25–29 June 2003. [Google Scholar]
- Vilchez-Monge, M.; Truque-Barrantes, A.; Ortiz-Leon, G. Design and Construction of a Hydro-Pneumatic Mock Circulation Loop That Emulates the Systemic Circuit of the Circulatory System. In Proceedings of the IEEE 36th Central American and Panama Convention (CONCAPAN XXXVI), San Jose, Costa Rica, 9–11 November 2011. [Google Scholar] [CrossRef]
- Wu, Y.; Allaire, P.E.; Tao, G.; Adams, M.; Liu, Y.; Wood, H.; Olsen, D.B. A Bridge from Short-Term to Long-Term Left Ventricular Assist Device--Experimental Verification of a Physiological Controller. Artif. Organs 2004, 28, 927–932. [Google Scholar] [CrossRef]
- Yoshizawa, M.; Sato, T.; Tanaka, A.; Abe, K.; Takeda, H.; Yambe, T.; Nitta, S.; Nosé, Y. Sensorless Estimation of Pressure Head and Flow of a Continuous Flow Artificial Heart Based on Input Power and Rotational Speed. ASAIO J. 2002, 48, 443–448. [Google Scholar] [CrossRef]
- Timms, D.; Hayne, M.; Tan, A.; Pearcy, M. Evaluation of Left Ventricular Assist Device Performance and Hydraulic Force in a Complete Mock Circulation Loop. Artif. Organs 2005, 29, 573–580. [Google Scholar] [CrossRef]
- Yoshino, M.; Uemura, M.; Takahashi, K.; Watanabe, N.; Hoshi, H.; Ohuchi, K.; Nakamura, M.; Fujita, H.; Sakamoto, T.; Takatani, S. Design and Evaluation of a Single-Pivot Supported Centrifugal Blood Pump. Artif. Organs 2001, 25, 683–687. [Google Scholar] [CrossRef]
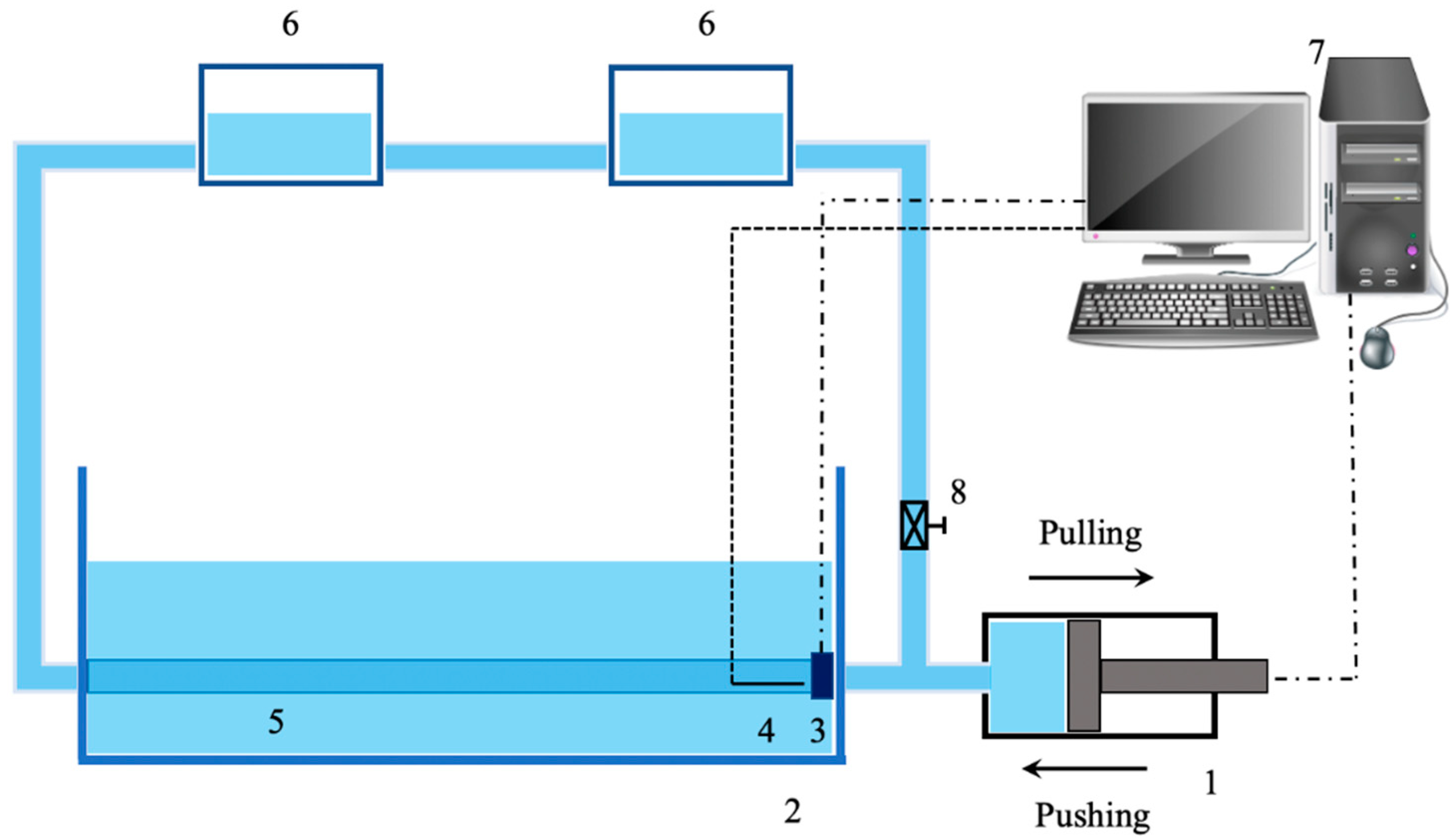
- Lee, J.-Y.; Jang, M.; Shin, S.-H. Mock Circulatory System with a Silicon Tube for the Study of Pulse Waves in an Arterial System. J. Korean Phys. Soc. 2014, 65, 1134–1141. [Google Scholar] [CrossRef]
- Fronek, K.; Schmid-Schoenbein, G.; Fung, Y.C. A Noncontact Method for Three-Dimensional Analysis of Vascular Elasticity in Vivo and in Vitro. J. Appl. Physiol. 1976, 40, 634–637. [Google Scholar] [CrossRef]
- Lou, Y.S. Experimental Study of Finite Amplitude Pulsatile Pressure Waves in Elastic Tubes. J. Biomech. 1976, 9, 747–753. [Google Scholar] [CrossRef]
- Horsten, J.B.A.M.; Van Steenhoven, A.A.; Van Dongen, M.E.H. Linear Propagation of Pulsatile Waves in Viscoelastic Tubes. J. Biomech. 1989, 22, 477–484. [Google Scholar] [CrossRef]
- Humphrey, J.D.; Kang, T.; Sakarda, P.; Anjanappa, M. Computer-Aided Vascular Experimentation: A New Electromechanical Test System. Ann. Biomed. Eng. 1993, 21, 33–43. [Google Scholar] [CrossRef]
- Zannoli, R.; Corazza, I.; Branzi, A. Mechanical Simulator of the Cardiovascular System. Phys. Med. 2009, 25, 94–100. [Google Scholar] [CrossRef]
- Feng, J.; Long, Q.; Khir, A.W. Wave Dissipation in Flexible Tubes in the Time Domain: In Vitro Model of Arterial Waves. J. Biomech. 2007, 40, 2130–2138. [Google Scholar] [CrossRef]
- Péry, E.; Blondel, W.C.P.M.; Didelon, J.; Leroux, A.; Guillemin, F. Simultaneous Characterization of Optical and Rheological Properties of Carotid Arteries via Bimodal Spectroscopy: Experimental and Simulation Results. IEEE Trans. Biomed. Eng. 2009, 56, 1267–1276. [Google Scholar] [CrossRef]
- Jang, M.; Lee, M.-W.; Kim, J.U.; Seo, S.-Y.; Shin, S.-H. Development of a Cardiovascular Simulator for Studying Pulse Diagnosis Mechanisms. J. Evid. Based Complement. Altern. Med. 2017, 2017, e6790292. [Google Scholar] [CrossRef]
- Yang, T.-H.; Kim, J.U.; Kim, Y.-M.; Koo, J.-H.; Woo, S.-Y. A New Blood Pulsation Simulator Platform Incorporating Cardiovascular Physiology for Evaluating Radial Pulse Waveform. J. Healthc. Eng. 2019, 2019, 4938063. [Google Scholar] [CrossRef]
- Alastruey, J.; Khir, A.W.; Matthys, K.S.; Segers, P.; Sherwin, S.J.; Verdonck, P.R.; Parker, K.H.; Peiró, J. Pulse Wave Propagation in a Model Human Arterial Network: Assessment of 1-D Visco-Elastic Simulations against in vitro Measurements. J. Biomech. 2011, 44, 2250–2258. [Google Scholar] [CrossRef]
- Anssari-Benam, A.; Korakianitis, T. An Experimental Model to Simulate Arterial Pulsatile Flow: In Vitro Pressure and Pressure Gradient Wave Study. Exp. Mech. 2013, 53, 649–660. [Google Scholar] [CrossRef]
- Balay, G.; Brum, J.; Bia, D.; Armentano, R.L.; Negreira, C.A. Improvement of Artery Radii Determination with Single Ultra Sound Channel Hardware & in Vitro Artificial Heart System. Annu. Int. Conf. IEEE Eng. Med. Biol. Soc. 2010, 2010, 2521–2524. [Google Scholar] [CrossRef]
- Blondel, W.C.; Didelon, J.; Maurice, G.; Carteaux, J.P.; Wang, X.; Stoltz, J.F. Investigation of 3-D Mechanical Properties of Blood Vessels Using a New in Vitro Tests System: Results on Sheep Common Carotid Arteries. IEEE Trans. Biomed. Eng. 2001, 48, 442–451. [Google Scholar] [CrossRef]
- Graf, S.; Gariepy, J.; Massonneau, M.; Armentano, R.L.; Mansour, S.; Barra, J.G.; Simon, A.; Levenson, J. Experimental and Clinical Validation of Arterial Diameter Waveform and Intimal Media Thickness Obtained from B-Mode Ultrasound Image Processing. Ultrasound Med. Biol. 1999, 25, 1353–1363. [Google Scholar] [CrossRef]
- Maryakhina, V.; Kostuganov, A. The Experimental Setup for Human Circulation Modeling. In Proceedings of the International Work-Conference on Bioinformatics and Biomedical Engineering, Granada, Spain, 1 April 2017. [Google Scholar]
- Saito, M.; Ikenaga, Y.; Matsukawa, M.; Watanabe, Y.; Asada, T.; Lagrée, P.-Y. One-Dimensional Model for Propagation of a Pressure Wave in a Model of the Human Arterial Network: Comparison of Theoretical and Experimental Results. J. Biomech. Eng. 2011, 133, 121005. [Google Scholar] [CrossRef]
- Shahmirzadi, D.; Li, R.X.; Konofagou, E.E. Pulse-Wave Propagation in Straight-Geometry Vessels for Stiffness Estimation: Theory, Simulations, Phantoms and In Vitro Findings. J. Biomech. Eng. 2012, 134, 114502–1145026. [Google Scholar] [CrossRef] [PubMed]
- Suarez-Bagnasco, D.; Cymberknop, L.J.; Ballarin, F.M.; Balay, G.; Negreira, C.A.; Abraham, G.; Armentano, R.L. An In Vitro Set Up for the Assessment of Electrospun Nanofibrous Vascular Grafts. IFMBE Proc. 2015, 49, 144–147. [Google Scholar] [CrossRef]
- Ursino, M.; Artioli, E.; Gallerani, M. An Experimental Comparison of Different Methods of Measuring Wave Propagation in Viscoelastic Tubes. J. Biomech. 1994, 27, 979–990. [Google Scholar] [CrossRef]
- Walker, R.D.; Smith, R.E.; Sherriff, S.B.; Wood, R.F. Latex Vessels with Customized Compliance for Use in Arterial Flow Models. Physiol. Meas. 1999, 20, 277–286. [Google Scholar] [CrossRef]
- Werneck, M.M.; Jones, N.B.; Morgon, J. Flexible Hydraulic Simulator for Cardiovascular Studies. Med. Biol. Eng. Comput. 1984, 22, 86–89. [Google Scholar] [CrossRef]
- Von Maltzahn, W.W.; Warriyar, R.G.; Keitzer, W.F. Experimental Measurements of Elastic Properties of Media and Adventitia of Bovine Carotid Arteries. J. Biomech. 1984, 17, 839–847. [Google Scholar] [CrossRef]
- Lee, J.-Y.; Jang, M.; Shin, S.-H. Study on the Depth, Rate, Shape, and Strength of Pulse with Cardiovascular Simulator. J. Evid. Based Complement. Altern. Med. 2017, 2017, e2867191. [Google Scholar] [CrossRef]
- Bia, D.; Armentano, R.L.; Zócalo, Y.; Barmak, W.; Migliaro, E.; Cabrera Fischer, E.I. In Vitro Model to Study Arterial Wall Dynamics through Pressure-Diameter Relationship Analysis. Lat. Am. Appl. Res. 2005, 35, 217–224. [Google Scholar]
- Brant, A.M.; Rodgers, G.J.; Borovetz, H.S. Measurement in Vitro of Pulsatile Arterial Diameter Using a Helium-Neon Laser. J. Appl. Physiol. 1987, 62, 679–683. [Google Scholar] [CrossRef]
- Bertram, C.D.; Pythoud, F.; Stergiopulos, N.; Meister, J.J. Pulse Wave Attenuation Measurement by Linear and Nonlinear Methods in Nonlinearly Elastic Tubes. Med. Eng. Phys. 1999, 21, 155–166. [Google Scholar] [CrossRef]
- Lee, J.-Y.; Jang, M.; Lee, S.; Kang, H.; Shin, S.-H. A Cardiovascular Simulator with Elastic Arterial Tree for Pulse Wave Studies. J. Mech. Med. Biol. 2015, 15, 1540045. [Google Scholar] [CrossRef]
- Vinall, P.E.; Simeone, F.A. Whole Mounted Pressurized In Vitro Model for the Study of Cerebral Arterial Mechanics. Blood Vessels 1987, 24, 51–62. [Google Scholar] [CrossRef]
- Jun, M.-H.; Jeon, Y.J.; Cho, J.-H.; Kim, Y.-M. Pulse Wave Response Characteristics for Thickness and Hardness of the Cover Layer in Pulse Sensors to Measure Radial Artery Pulse. BioMed Eng. OnLine 2018, 17, 118. [Google Scholar] [CrossRef]
- Gardner, R.M. Direct Arterial Pressure Monitoring. Curr. Anaesth. Crit. Care 1990, 1, 239–246. [Google Scholar] [CrossRef]
- Heimann, P.A. Assessment of Catheter-Manometer Systems Used for Invasive Blood Pressure Measurement. Master’s Thesis, University of Cape Town, Cape Town, South Africa, 1989. [Google Scholar]
- Using Manometers to Precisely Measure Pressure, Flow and Level, Meriam Instrument. 1997. Available online: Meriam.com/assets/eng/050-MHB-1.pdf (accessed on 10 June 2022).
- Liptak, B.G. Instrument Engineers’ Handbook, Volume One: Process Measurement and Analysis, 4th ed.; CRC Press: Boca Raton, FL, USA, 2003; pp. 259–374. [Google Scholar]
- Dracos, T.H. Three-Dimensional Velocity and Vorticity Measuring and Image Analysis Techniques; Springer Science & Business Media: Zürich, Switzerland, 2013; pp. 64–67. [Google Scholar]
- Pries, A.R.; Reglin, B.; Secomb, T.W. Remodeling of Blood Vessels: Responses of Diameter and Wall Thickness to Hemodynamic and Metabolic Stimuli. Hypertension 2005, 46, 725–731. [Google Scholar] [CrossRef] [PubMed]
- Fung, Y.C. Biodynamics: Circulation, 2nd ed.; Springer: New York, NY, USA, 1997; pp. 1–21. [Google Scholar]
- Fung, Y.C. Biomechanics: Motion, Flow, Stress, and Growth; Springer: New York, NY, USA, 1990; pp. 155–195. [Google Scholar]
- Fung, Y.C. Biomechanics: Mechanical Properties of Living Tissues; Springer Nature: New York, NY, USA, 1993; pp. 66–108. [Google Scholar]
| Physical Quantity | Measurement Device | Application Range | Accuracy | Functional and Metrological Issues | Reference |
|---|---|---|---|---|---|
| Pressure | Catheter manometer | −2.7–53.3 kPa | 1% | It allows continuous monitoring. Air bubbles in the physiologic solution could lead to a limited dynamic response. | [102,103] |
| Differential manometer | 0–250 Pa | 0.1% | Accuracy, sensitivity and measurement range can be altered according to the density of the chosen fluid. | [104] | |
| Flow | Venturi tube | 0.1–105 m3·h−1 | 2% | These devices are heavy and large. This leads to difficulty in the installation. | [21,105] |
| Orifice plate meter | 10−3–105 m3·h−1 | 2% | A disadvantage is given by the pressure loss, leading to deterioration of measurement accuracy and repeatability. It needs high maintenance. | ||
| Rotameter | 0.04 L·h−1–150 m3·h−1 | 0.4–4% | The accuracy is given with respect to the maximum flow. The measurement range varies according to the fluid state (e.g., gaseous or liquid). | [21] | |
| Flow velocity | Laser Doppler velocimeter (LDV) | It depends on the type of application | ≥0.1% | A high particle concentration is necessary for continuous velocity measurement. Very high accuracy can be reached with mean velocity measurements with a LDV system. | |
| Particle image velocimetry (PIV) | 0–600 m·s−1 | 1–2% | The accuracy is reported with respect to the mean flow velocity. The overall accuracy must be evaluated for each experiment and it is affected by many error sources such as systematic errors due to the optical techniques involved in the measurement, as well as random errors due to the evaluation process. | [106] | |
| Electromagnetic flowmeter | 0.3–10 m·s−1 | 0.25–5% | In general, an accuracy of 1% is reached in common applications, but it may be worsened to 5% because of fluid impurities. | [21] | |
| Ultrasonic transit-time flowmeter | 0.1–12 m·s−1 | 0.5–1% | Stability and temperature coefficients of the quartz oscillator are the factors affecting the accuracy of transit-time ultrasonic flowmeters. Transit time should be measured with a resolution of 10−10 s to have 1% accuracy on the velocity measurement. |
| Sensors/Devices | Measured Quantities | Accuracy/ Measurement Range | Functional and Metrological Issues | Reference |
|---|---|---|---|---|
| Transit-time flowmeter | Flow | 1% | Flow waveforms were perturbed by diameter variation due to pressure pulses, leading to a 10% error. | [23] |
| Pressure transducer | Pressure | 80–150 mmHg | Signals were acquired with a sampling rate of 3 kHz and a 12-bit A/D converter. The sensors employed had a frequency response of 1 kHz. Pressure measurements were influenced by air bubbles in the physiological solution, circuit mechanical vibrations and temperature variations. | |
| A-scan ultrasound | Wall thickness, length, diameter | Not specified | Ultrasound measurements were influenced by other radio frequency sources coming from other systems in the experimental set-up. The probes were fixed to a mechanical graduated positioning system with 1.5 mm resolution. | |
| Doppler | Cross-section flow velocity | 0–200 mm·s−1 | Doppler velocity profiles suffered from vessel motion artefacts. The measured velocities reached peaks of about 200 mm·s−1. | |
| PIV | Flow velocity | 14–260 cm·s−1 | The fluid had a density of 930 kg·m−3. For each run, 300 images were collected. Two consecutive images were separated at an interval from 5 to 30 μs. The camera frame rate was 30 Hz. The high velocity gradient caused a decrease in the accuracy of digital PIV. The velocity was measured with a flow rate gradually changing from 0 to 100 cm3·s−1. The measured peak flow velocities were 49 cm·s−1 and 14 cm·s−1 in systole and diastole, respectively, and they increased to 260 cm·s−1 and 87 cm·s−1 in correspondence to the stenosis. | [24] |
| MRI | Cross-section flow velocity | 15–20% 50–120 cm·s−1 | The spatial resolution was 0.5 × 0.5 mm2, whereas the time resolution was 25 ms. For secondary flow measurements, inaccuracies from 15 to 20% were obtained for vessel diameters from 5 to 6 mm due to image artefacts. Measured velocities went from 50 to 120 cm·s−1. | [25] |
| Electromagnetic flowmeter | Flow | Not specified | The waveform was provided by a computer-controlled step motor pump. The sampling frequency for flow waveform acquisition was 25 Hz. The mean flow rate was 9.4 mL·s−1. |
| Sensors/Devices | Measured Quantities | Accuracy/ Measurement Range | Functional and Metrological Issues | Reference |
|---|---|---|---|---|
| Flowmeter | Flow | 10% | The accuracy of the flowmeters was within 10%. Compliance was estimated from volume and pressure to be 6.53·10−4 mL·mmHg−1 for the silicone simulated artery. | [29] |
| Microtip catheter | Pressure | Not specified | ||
| Sonomicrometer | External diameter | Pulsatility was estimated from diameter variation and mean diameter to be 4.98% in the silicone simulated artery. | ||
| Doppler velocimeter | Cross-section flow velocity | ~1.5% | The sample volume provided by the probe was 0.12 mm3. The mean velocity was obtained from an average of 30 cardiac periods to guarantee the reproducibility. | |
| Flowmeter | Flow | 5–20 mL·s−1 | Experiments were carried out with two main pulsatile regimes or flow shapes: simple pulsatile flow rate (sinusoidal flow) and physiological flow rate (flow shape with hydrodynamic parameters set by the researchers). In the first case, the mean flow rate was 14 mL·s−1, in the second case, 17 mL·s−1. | [30] |
| Doppler velocimeter | Flow velocity | Not specified | The mean velocity was obtained from an average of 40 cardiac periods. Results have been obtained with a sample volume of 2 mm3. From velocity measurements the wall shear stress was derived through the equation −μ·∂w/∂r where μ is the viscosity and w the velocity axial component. The maximum retrieved value was ~125 dyn·cm−2 (~12.5 Pa). | |
| Flowmeter | Flow | 5% 5·10−3–20 L·min−1 | The flow rate was set to 2.27 L·min−1 to keep the flow characteristics (e.g., Re number) as constant as possible in the phantom. | [32] |
| PIV | Flow/velocity | 5–50 cm·s−1 | Fourteen pairs of images were used to assess velocity to stabilize the results. Two consecutive images were separated by a 200 μs interval. From velocity measurements, the mean wall shear stress was derived (between 2.8 and 9.2 Pa according to the proximity to the stenosis). The scanning spatial resolution in the z-direction was 1 mm. The maximum velocity at the stenosis entrance was 27.5 cm·s−1. | |
| Rheometer | Viscosity | 3% 5–107 cP | The fluid dynamic viscosity was estimated to be 3.5 cP (3.5·10−3 Pa·s), whereas the mixture viscosity was 14.5 cP. | |
| Flowmeter | Flow | 0.2% 0–2 L·s−1 | Both flow and pressure were acquired with a data acquisition device with a sampling frequency of 2 kHz. The overall flow and pressure measurement errors were estimated to be 2% and 1%, respectively. The fluid viscosity was 4 cP. | [33] |
| Pressure transducers | Pressure | 0.2% 7·10−2–700 bar | ||
| PIV | Flow velocity | 0–3 m·s−1 | Flow velocity measurements were compared with MRI techniques, showing a good agreement. The flow rate was set in the range 2–63 mL·s−1. The investigated particle diameters were in the range of 0.25–4 μm. The PIV resolution was 0.47 × 0.47 mm2. | [36] |
| MRI | Flow velocity | The MRI sequence lasted 45 min. The MRI resolution was 0.35 × 0.35 × 0.70 mm3. |
| Sensors/Devices | Measured Quantities | Accuracy/ Measurement Range | Functional and Metrological Issues | Reference |
|---|---|---|---|---|
| Pressure transducer | Pressure | 1% −50–300 mmHg | The pressure wave was recorded throughout 25 cardiac cycles. A pressure of 84–148 mmHg was measured. | [38] |
| N/P * | Radial displacement | Not specified | The tested elastic tube length was 34 cm. The measured tube diameter and wall thickness were 2.22 cm and 0.09 cm, respectively, at 84 mmHg. From the pressure, diameter and radial displacement measurements at 84 and 148 mmHg, the mean Young modulus of the vessel was estimated to be 1.3 MPa. | |
| Diameter | ||||
| Wall thickness | ||||
| Doppler velocimeter | Axial flow velocity | 0–1 m·s−1 | A difference of 3% was found between simulated and experimental axial velocities. | |
| Venturi tube | Flow | 5% | Flow velocity was retrieved through the mass conservation law. Minimum diameter = 16 mm, maximum diameter = 26 mm. Maximum measured flow rate was 0.25 L·s−1. | [43] |
| Orifice plate meter | Flow | Orifice diameter = 20 mm. Maximum measured flow rate was 0.36 L·s−1. | ||
| Rotameter | Flow | 1% 0.10–103 L·s−1 | Flow rate was measured with the equilibrium position of a float. Maximum measured flow rate was 0.10 L·s−1. | |
| Manometer | Pressure | Not specified | Measurements were collected from nine different cross-sections along the experimental set-up (pipe). | |
| Catheter pressure transducer | Pressure | 1% −50–300 mmHg | Every 100 mmHg input was converted to 0.5 V output. The average measured pressures were 128 mmHg and 159 mmHg under two testing conditions. | [44] |
| Ultrasonic transit-time flow sensor | Flow | 4% 0.5–10 L·min−1 | Flow rate and pressure data were acquired and averaged over 50 cycles for two flow conditions. The average measured values were 42 mL·s−1 and 88 mL·s−1. | |
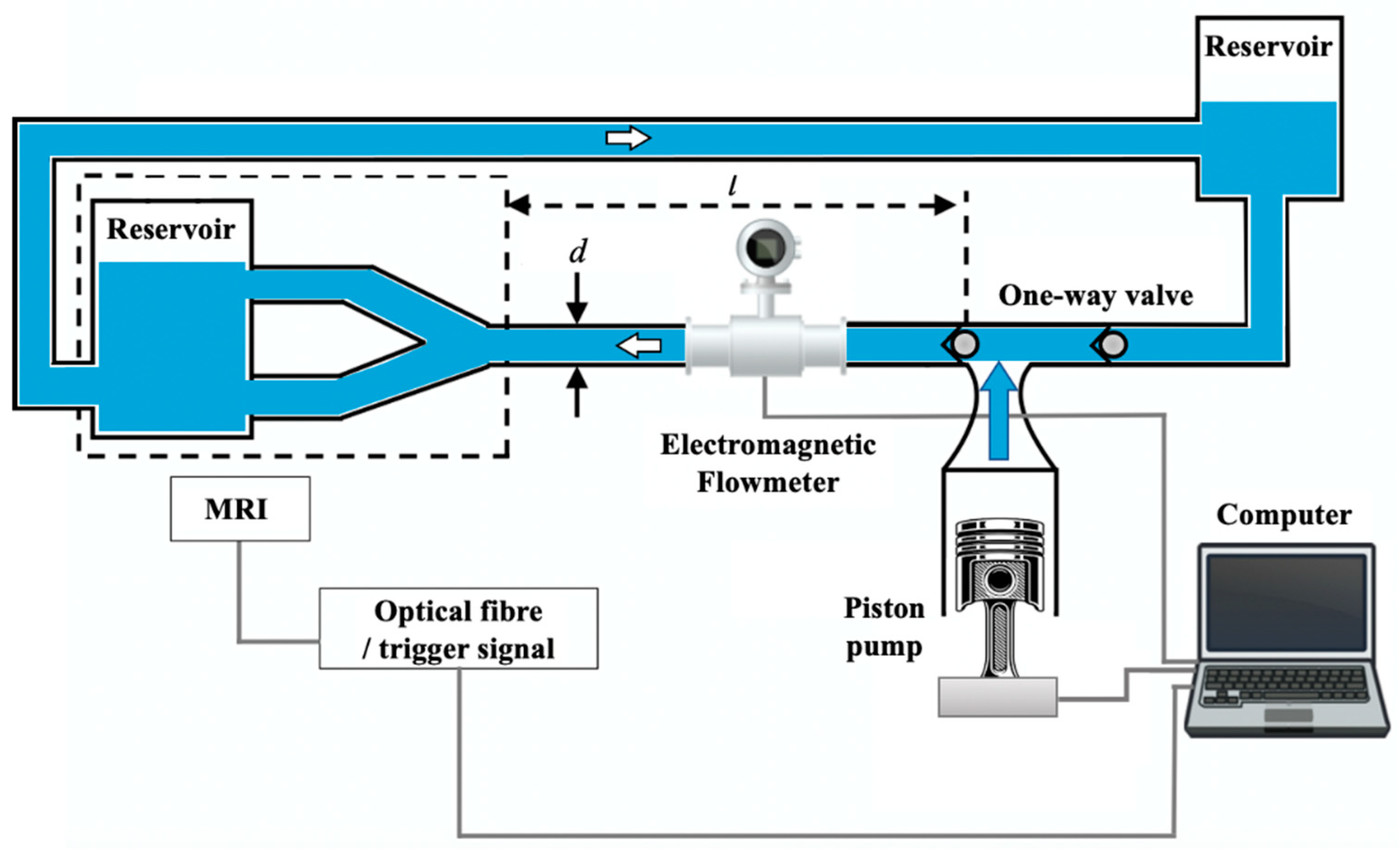
| MRI | Flow velocity | ≤10% 0–90 cm·s−1 | An MRI scanner with a 1.5 T magnetic field was used and the flow at different slice locations was acquired (5 mm slice thickness). A trigger-simulated signal was converted into an ECG signal for MRI system gating. The average measured values were 46 mL·s−1 and 96 mL·s−1, respectively. | |
| Ultrasonic flow probe | Flow | 4% | The flow waveform, generated by a piston pump, was half-sinusoidal. Displaced volume = 4·10−5 m3 (40 mL). | [45] |
| Catheter pressure transducer | Pressure | 1% −50–300 mmHg | The initial pressure was between 4 and 5 kPa. Through the PU-loop, the wave speed was estimated with about 22% global uncertainty. | |
| Sonomicrometer | Diameter | 2% 12–200 mm | The diameter, pressure and flow were measured at the same site and data were acquired with a sampling frequency of 500 Hz. | |
| μ-PIV | Axial flow velocity | Not specified | Flow was recorded through the CCD camera connected to an optical microscope with a numerical aperture of 0.45 and a depth of field of 2.8 μm. Velocity measurements were carried out for flow rates from 5 to 10 mL·h−1. The retrieved peak velocity was ~8 cm·s−1. | [46] |
| Plate rheometer | Fluid viscosity | Measurements were carried out at 24 °C, retrieving a viscosity value of ~1.2 cP (1.2·10−3 Pa·s). | ||
| Laser Doppler anemometer | Flow velocity | Not specified | Measurements were performed both in steady and pulsatile regimes, with a pulsatile flow frequency of 1 Hz. The maximum measured velocity was 50 cm·s−1. | [51] |
| Doppler flowmeter | Flow | 2% | It was used to measure the pulsatile flow. The mean recorded flow in the simulated system was 0.6 L·min−1. | |
| Inductive transducer | Pressure | Not specified | Mounted between the simulated ventricular and aortic arch. Pressure was kept constant at 100 ± 20 mmHg. | |
| Ultrasound flowmeter | Flow | 0.5% | It was used to monitor the flow rate through the graft. Measured inflow was 0.9 l·min−1. | [53] |
| Digital refractometer | Refractive index | 0.2% | The mean refractive index value was n = 1.38. | |
| PIV | Flow velocity | 2% | PIV images were acquired through a CCD camera with a 4 MP resolution and 16 Hz frame rate. Separation time Δt between consecutive images was set at 100 ms and 200 ms for the artery entrance and anastomosis heel, respectively. The maximum measured velocity was 0.55 m·s−1. | |
| Viscometer | Viscosity | 1% 0.3–104 cP | The measured mean viscosity value was 4.12 cP (4.12·10−3 Pa·s) at 19.6 °C. |
| Sensors/Devices | Measured Quantities | Accuracy/ Measurement Range | Functional and Metrological Issues | Reference |
|---|---|---|---|---|
| Flexible contact force sensor | Force | 3% 0–4.45 N | Measured force oscillated between 1.10 and 1.35 N. The maximum exerted force was 8.7% less than the numerically simulated one. | [55] |
| Pressure transducer | Pressure | 1% −50–300 mmHg | Measured pressure was between 0 and 0.85 mmHg. | |
| Ultrasound flowmeter | Flow | 4% | The flow rate has been measured with two pumps controlled with DC and AC motors. Pressure was kept at 100 mmHg. Resistance and compliance values were 1.2 mmHg·s·mL−1 and 1.1 mL·mmHg−1, respectively. | [60] |
| Differential pressure transducer | Pressure | 0.04% 53–262 mmHg | ||
| Pressure transducer | Pressure | 1% −50–300 mmHg | The mean measured pressure was 95 mmHg. | [61] |
| Ultrasonic flowmeter sensor | Flow | 4% | The mean measured flow was 4.86 L·min−1. | |
| Temperature sensor | Temperature | 0.05% −196–660 °C | A PT100 was employed as a temperature transducer. Temperature was stabilized at 36.5 ± 0.5 °C. | |
| Freescale absolute pressure sensor | Pressure | 2.5% | It provided a response after 1 ms. | [67] |
| Flowmeter | Flow | 3% 1–30 L·min−1 | The artificial mixture employed in the experimental set-up had a density of 1048.0 ± 4.2 kg·m−3 and a viscosity of 3.14 ± 0.02 cP. | |
| U-tube manometer | Pressure | Not specified | It measured the difference between pressures in correspondence with the pump input and output. | [71] |
| Electromagnetic flowmeter | Flow | Maximum flow was 9 L·min−1. |
| Sensors/Devices | Measured Quantities | Accuracy/ Measurement Range | Functional and Metrological Issues | Reference |
|---|---|---|---|---|
| Pressure transducer | Pressure | Not specified | Intraluminal pressure was recorded. The measured values were between 13 kPa and 17 kPa. | [73] |
| Force transducer | Force | The force related to the vessel elongation was measured. | ||
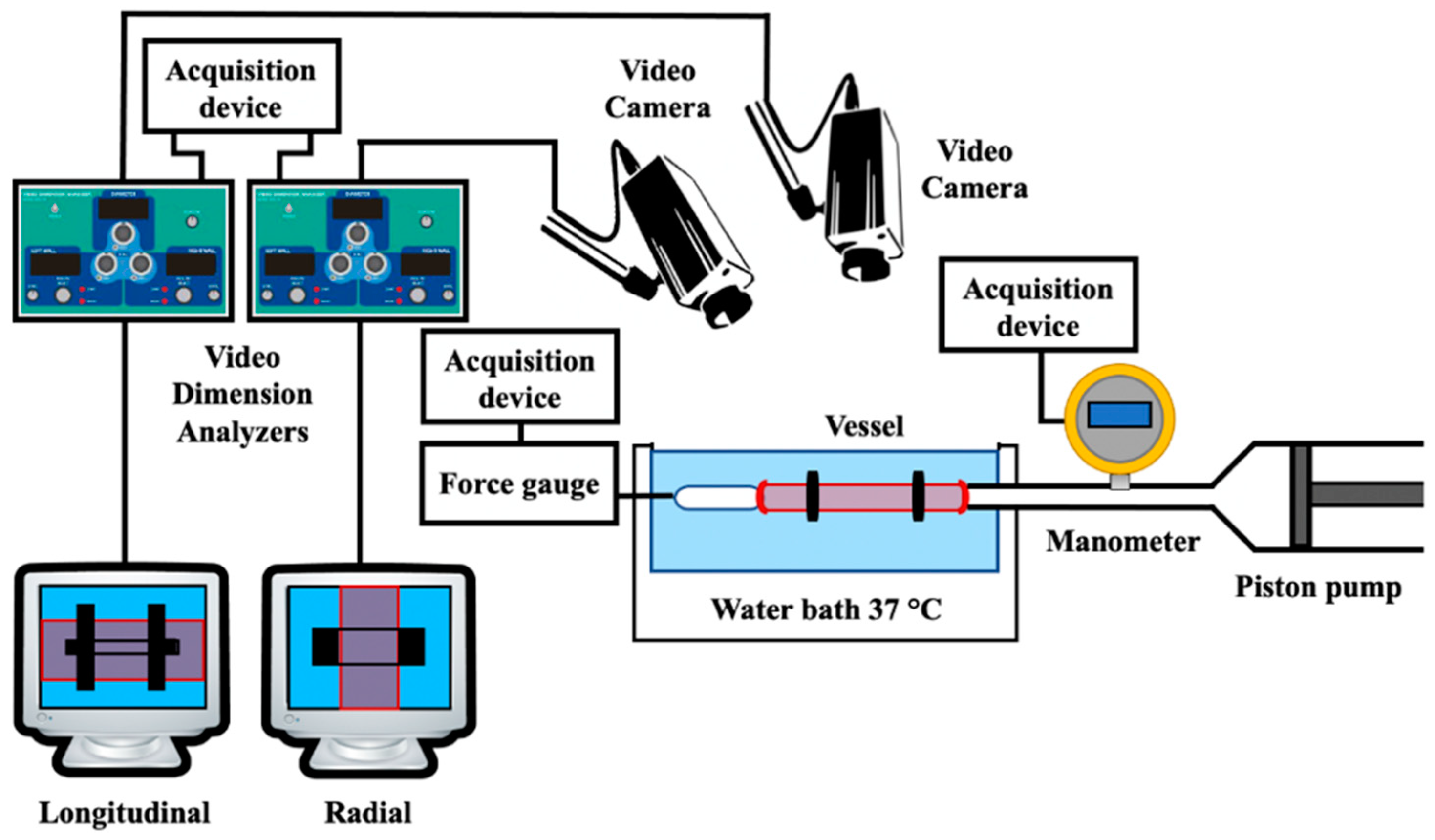
| Video dimension analyzer (VDA) | Vessel deformation | 0–90 mm | Both radial and longitudinal deformation of the vessel were monitored. Through the stress–strain relationship, the incremental elastic modulus was obtained for each specimen (1.13 ± 0.18 MPa and 1.02 ± 0.13 MPa, respectively). | |
| Microscope | Vessel wall thickness | Not specified | The average wall thickness was measured at eight sites on vessel slices under an optical microscope with ×400 magnification. | |
| Flowmeter | Flow | Not specified | A screw pump provided an almost pulseless water steady flow. The maximum flow value measured was 4.24 L·min−1. | [74] |
| Pressure transducer | Pressure | 0–1.86 N·cm−2 | The maximum pressure inside the circuit was 18.6 kPa. | |
| Capacitive transducer | Displacement | ~6% | Sensitivity was able to detect 5 μm-diameter changes. Transducers were located at a known distance along the tube and the measured transit time was 80 ± 1 ms. Pulse wave velocity (PWV) was also estimated for different wave frequencies. The mean velocity was 11.5 m·s−1. | |
| Catheter-tip manometer | Pressure | 1% −50–300 mmHg | The manometer influence on wave propagation was neglected by the authors. The transmural pressure was set at 2.4 kPa to avoid tube collapse. The overpressure was 50 kPa. Pulse pressure propagated at 3.2 m·s−1. | [75] |
| Photonic sensor | Diameter variation | Not specified | The tube diameter was 18 mm. The diameter variations were assessed to be less than 2%. To assure repeatability and accuracy, 10 experiments were carried out. From the mean values obtained, they determined pulse amplitude (accuracy of ±2%), pulse width (accuracy of ±4%) and wave velocity (accuracy of ±1%). All accuracies were estimated with a 95% confidence level. | |
| Micrometer | Tube diameter | 0.04% 0–25 mm | This parameter was measured in order to retrieve a relationship between the pressure and cross-section. From the pressure-area relationship, there was a compliance of (3.1 ± 0.1)·10−8 m2·Pa−1. | |
| Linear variable differential transformer (LVDT) | Length | 0.2% | The overall length variation of the specimen was measured with a resolution of 0.1 mm. | [76] |
| Pressure transducer | Pressure | 0–150 mmHg | Resolution of about 0.65 mmHg. | |
| Axial load cell | Force | 0.1% | Resolution of about 5·10−3 N (0.5 gf). | |
| Torque transducer | Torque | Not specified | Resolution of about 1.72·10−4 Nm (1.75 gf-cm). | |
| CCD cameras | Diameter variation | VDA provided noncontact information on the specimen deformation in the central region with a resolution of 0.02 mm. | ||
| Tipped catheter | Pressure | 0.4% | For repeatability, measurements were carried out ten times at two different distances from the tube inlet. The peak-measured pressure was 6217 ± 23 Pa and 6805 ± 18 Pa, respectively, with a repeatability standard deviation lower than 0.5%. The pressure data were acquired with a sampling rate of 500 Hz. | [78] |
| Ultrasound flow probes | Flow | 4% | Assuming a flat velocity profile, the authors used these quantities to estimate the mean velocity at each tube site. The flow sample volume was 40 mL. The flow data were acquired with a sampling rate of 500 Hz. | |
| Digital caliper | Wall thickness | Not specified | Experiments were carried out on four tubes with 4, 8, 12 and 16 mm diameters and a wall thickness in the range 0.1–0.2 mm. Wave speed was determined through the pressure-velocity (PU) loop method, with an overall accuracy of 12%, and compliance (per unit length) was obtained as a ratio between the cross-sectional area and pressure, at a hydrostatic pressure of 3.1 kPa. | |
| External diameter | ||||
| Invasive pressure sensor | Pressure | 0.25% | Data acquired at a sampling frequency of 1 kHz. The pressure range of the simulator was 71.0–113.0 mmHg in the simulated ascending aorta and 86.0–140.9 mmHg in the simulated radial artery. From pressure waveforms, the PWV was estimated as 5.9 m·s−1. | [80] |
| Ultrasound sensor | Flow | 10% | Data acquired at a sampling frequency of 100 Hz. The simulated cardiac output was set at 2.9 L min−1, which is 55% with respect to real cardiac output (5.3 L·min−1). | |
| Laser sensor | Distance | 0.1% 100–300 mm | The device was employed to measure the linear displacement of a piston used to generate pressure waves in the circuit. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fuiano, F.; Scorza, A.; Sciuto, S.A. Functional and Metrological Issues in Arterial Simulators for Biomedical Testing Applications: A Review. Metrology 2022, 2, 360-386. https://doi.org/10.3390/metrology2030022
Fuiano F, Scorza A, Sciuto SA. Functional and Metrological Issues in Arterial Simulators for Biomedical Testing Applications: A Review. Metrology. 2022; 2(3):360-386. https://doi.org/10.3390/metrology2030022
Chicago/Turabian StyleFuiano, Fabio, Andrea Scorza, and Salvatore Andrea Sciuto. 2022. "Functional and Metrological Issues in Arterial Simulators for Biomedical Testing Applications: A Review" Metrology 2, no. 3: 360-386. https://doi.org/10.3390/metrology2030022
APA StyleFuiano, F., Scorza, A., & Sciuto, S. A. (2022). Functional and Metrological Issues in Arterial Simulators for Biomedical Testing Applications: A Review. Metrology, 2(3), 360-386. https://doi.org/10.3390/metrology2030022








