Advancing Microscale Electromagnetic Simulations for Liquid Crystal Terahertz Phase Shifters: A Diagnostic Framework for Higher-Order Mode Analysis in Closed-Source Simulators
Abstract
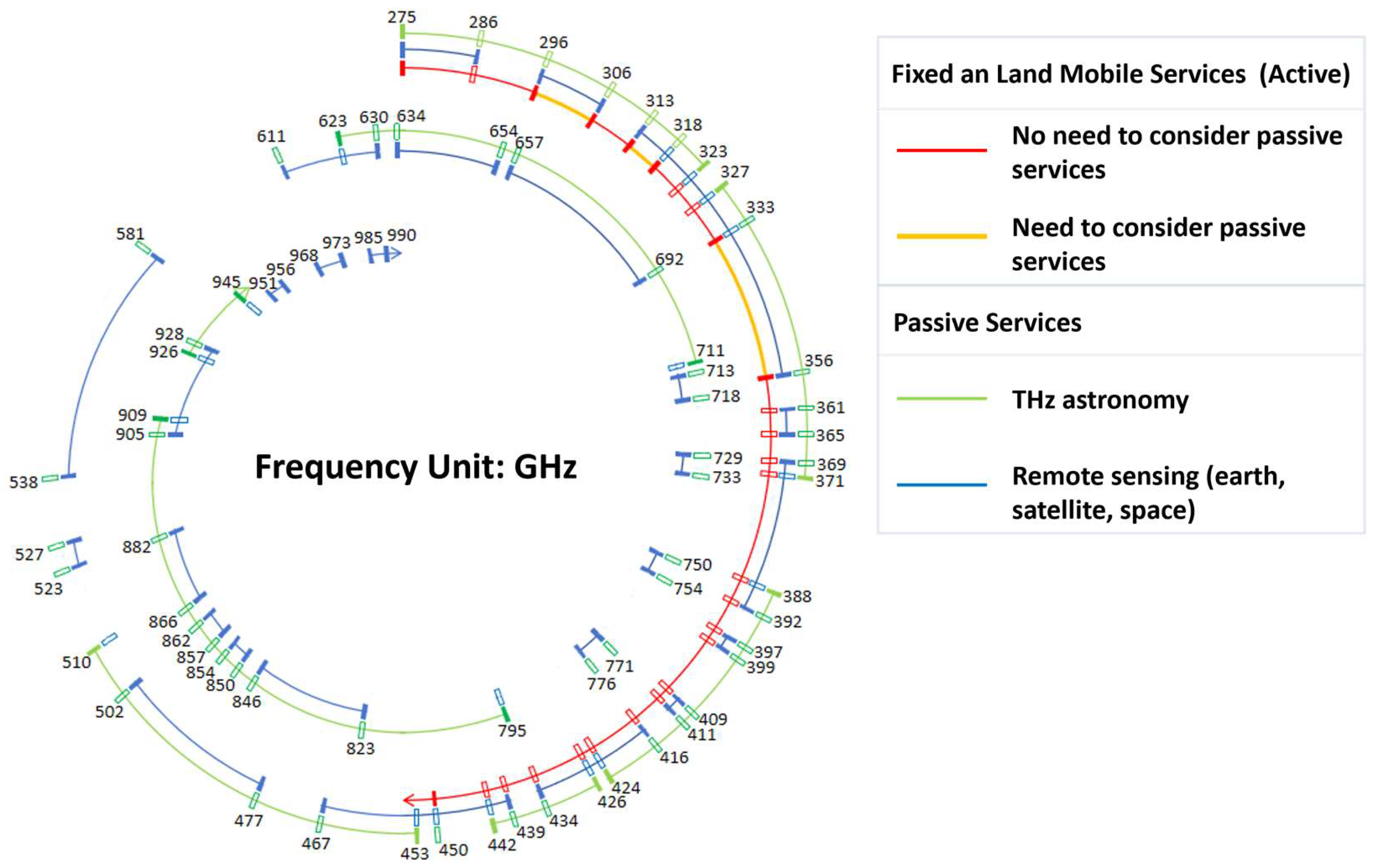
1. Introduction
2. Materials and Methods
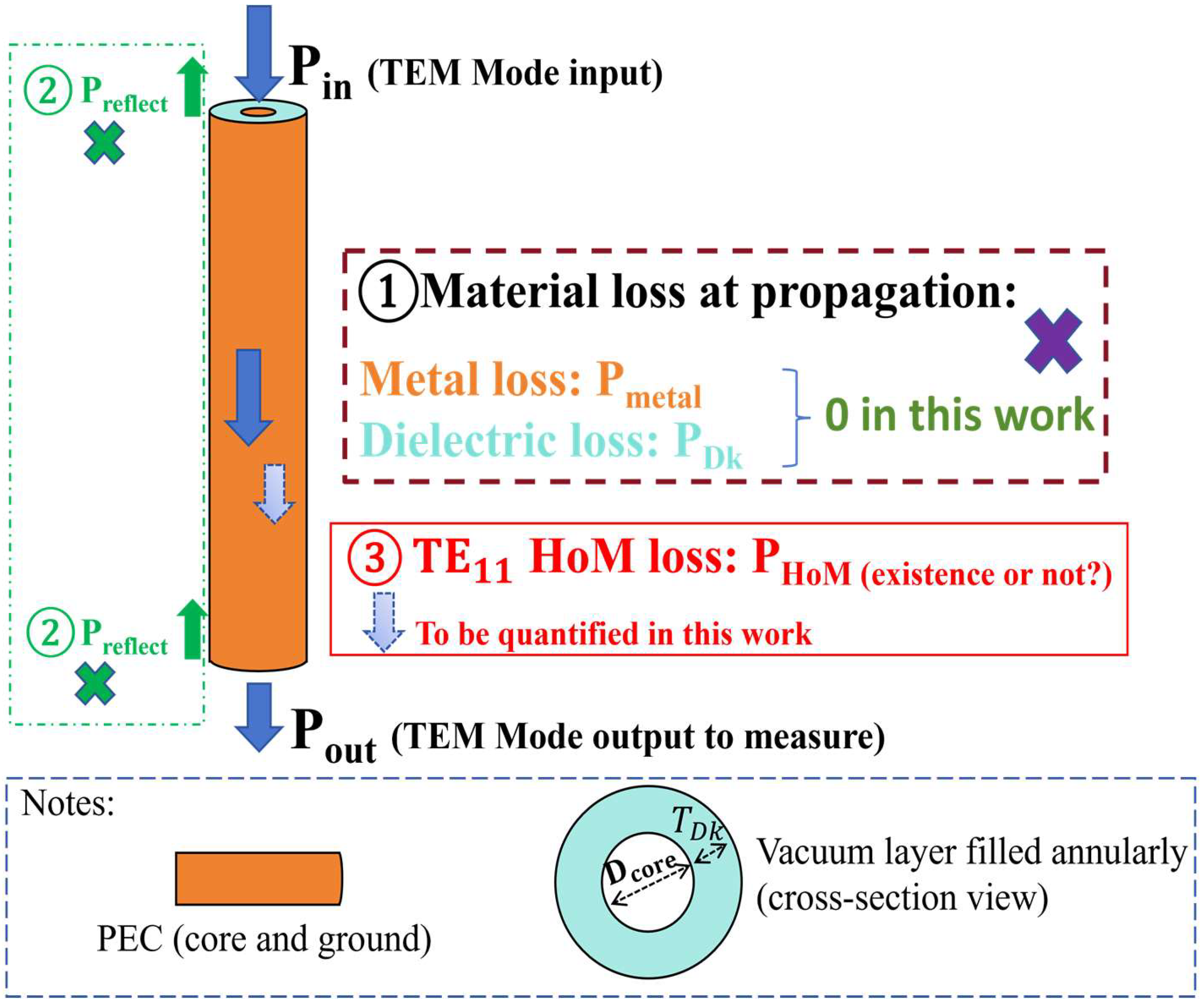
2.1. Eliminating Metal and Dielectric Losses Through PEC and Vacuum Approximations
2.2. Minimizing Reflection Losses Through Non-Renormalization Techniques
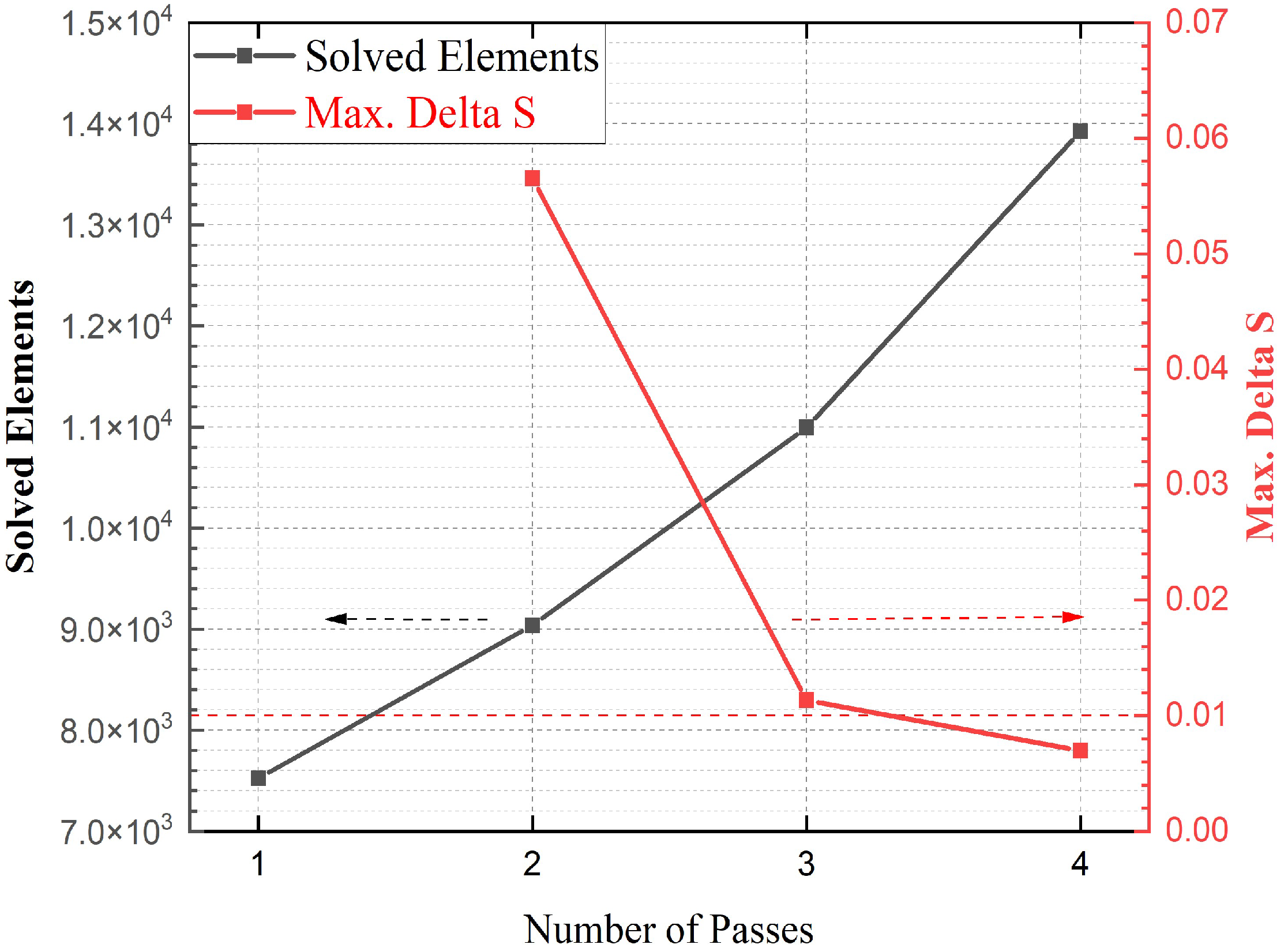
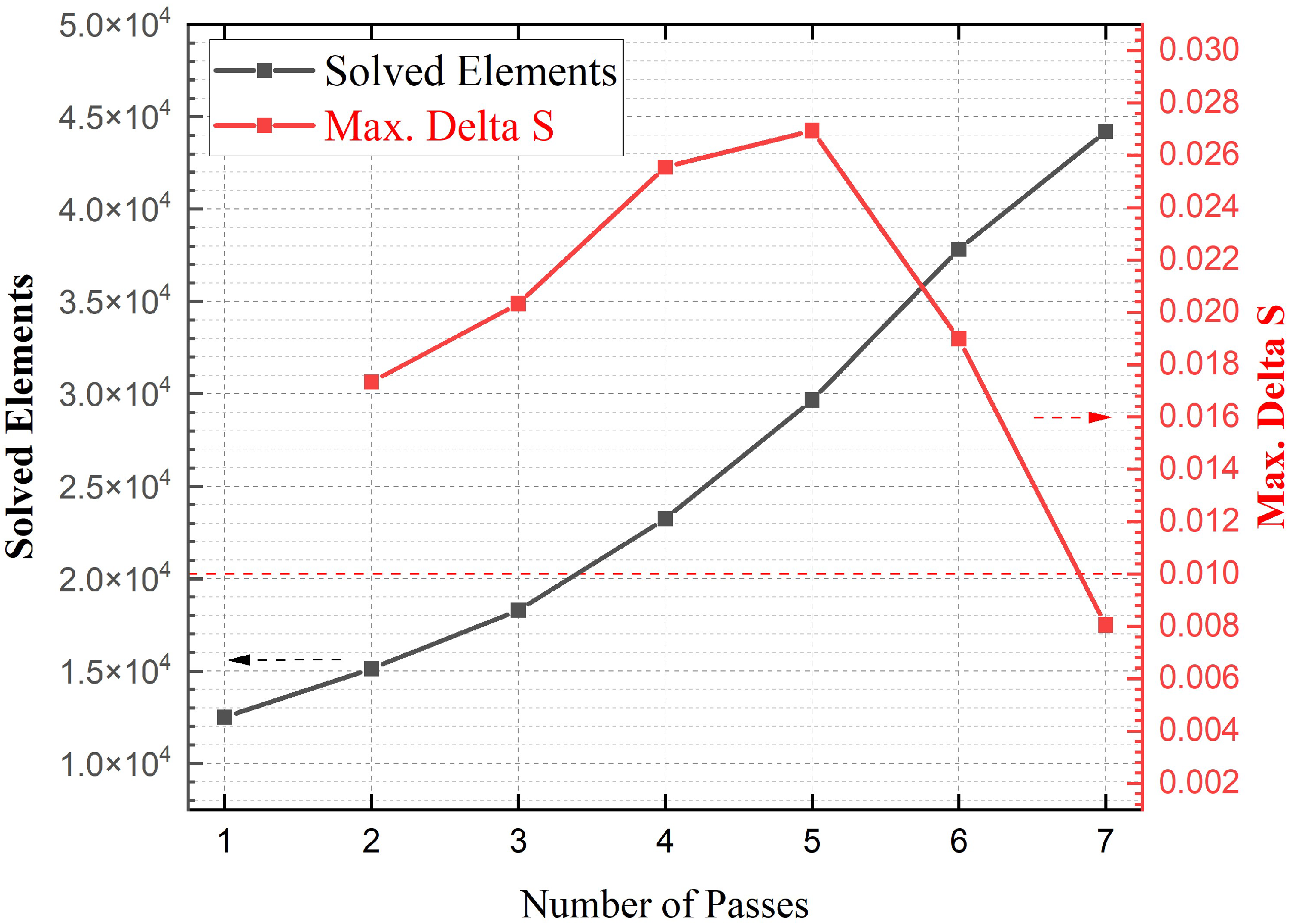
2.3. Benchmarking Uncertainties and Revealing Mode Purity to Improve Predictive Accuracy
3. Results
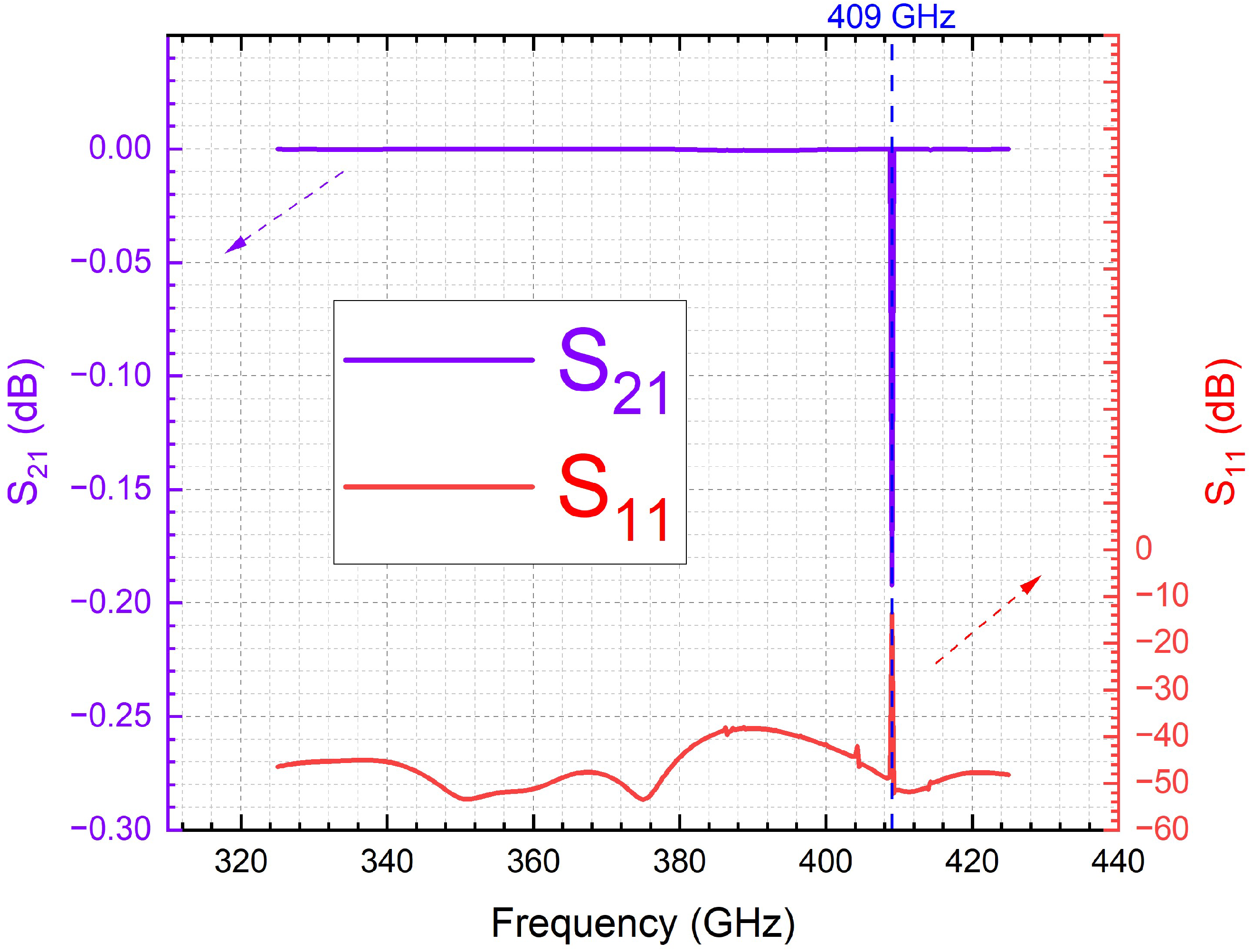
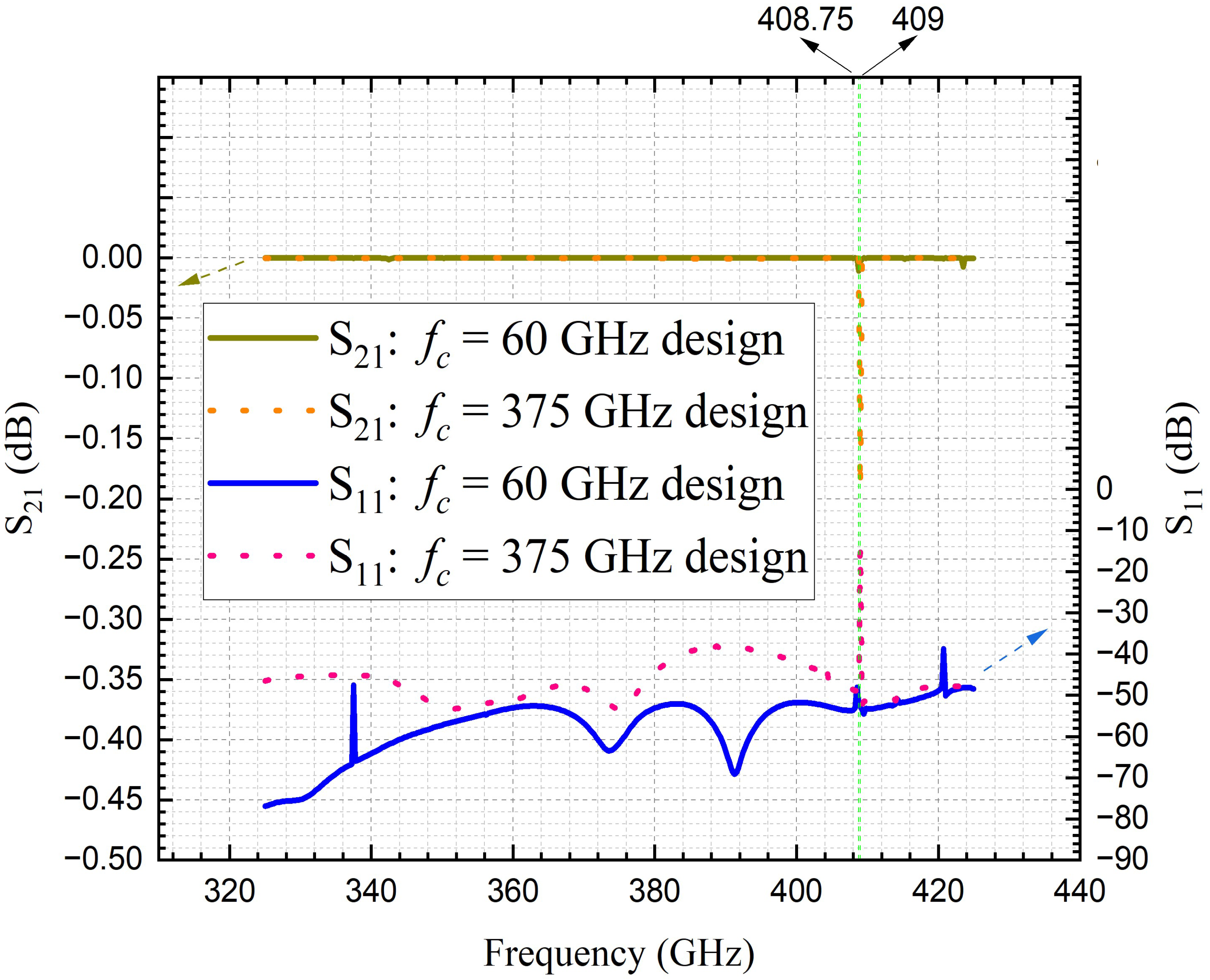
3.1. Design Analysis of a 375 GHz Cut-Off Frequency Component Operating Within the 325 GHz to 425 GHz Range
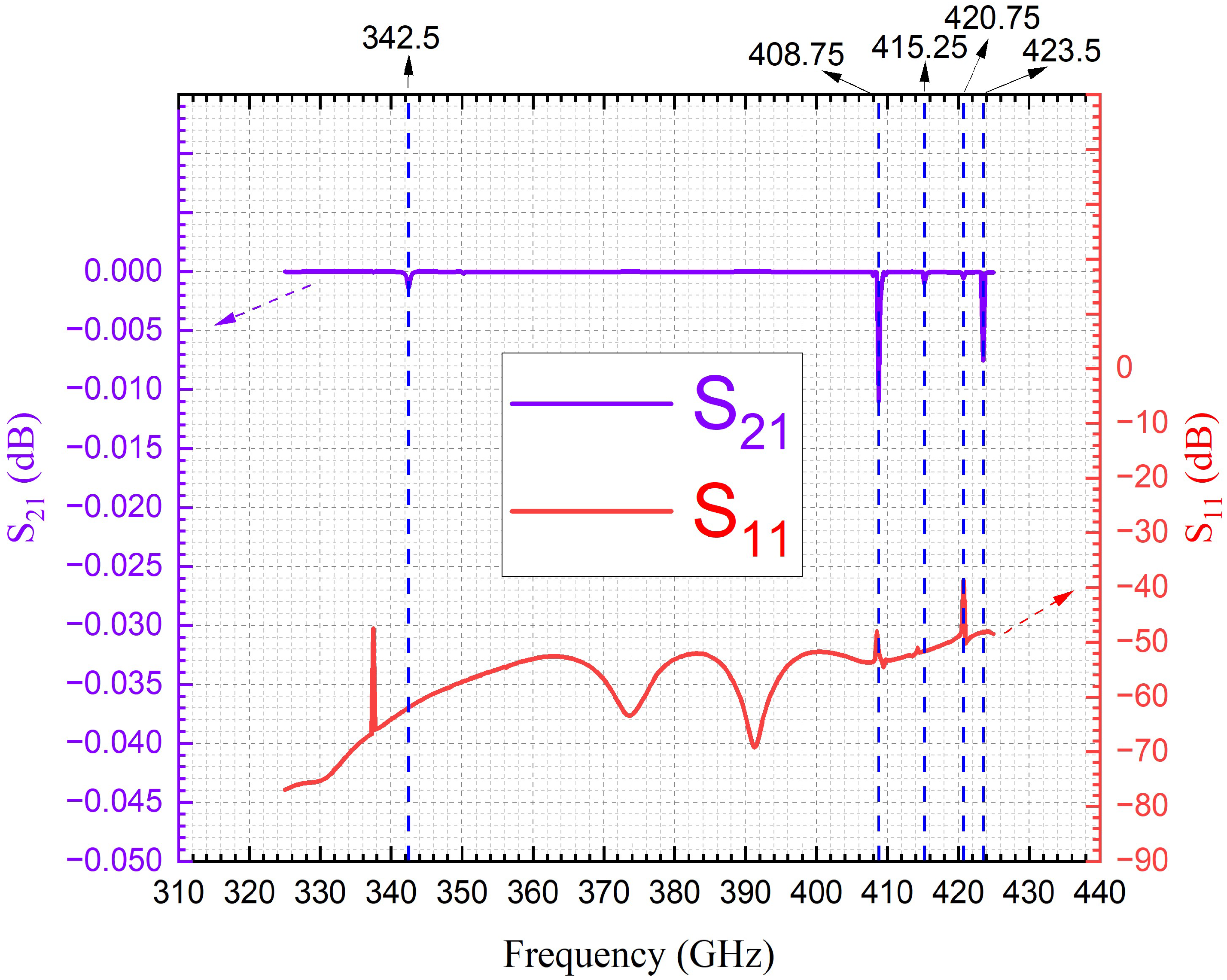
3.2. Analysis of a 60 GHz Cut-Off Frequency Design Operating Across 325 GHz to 425 GHz
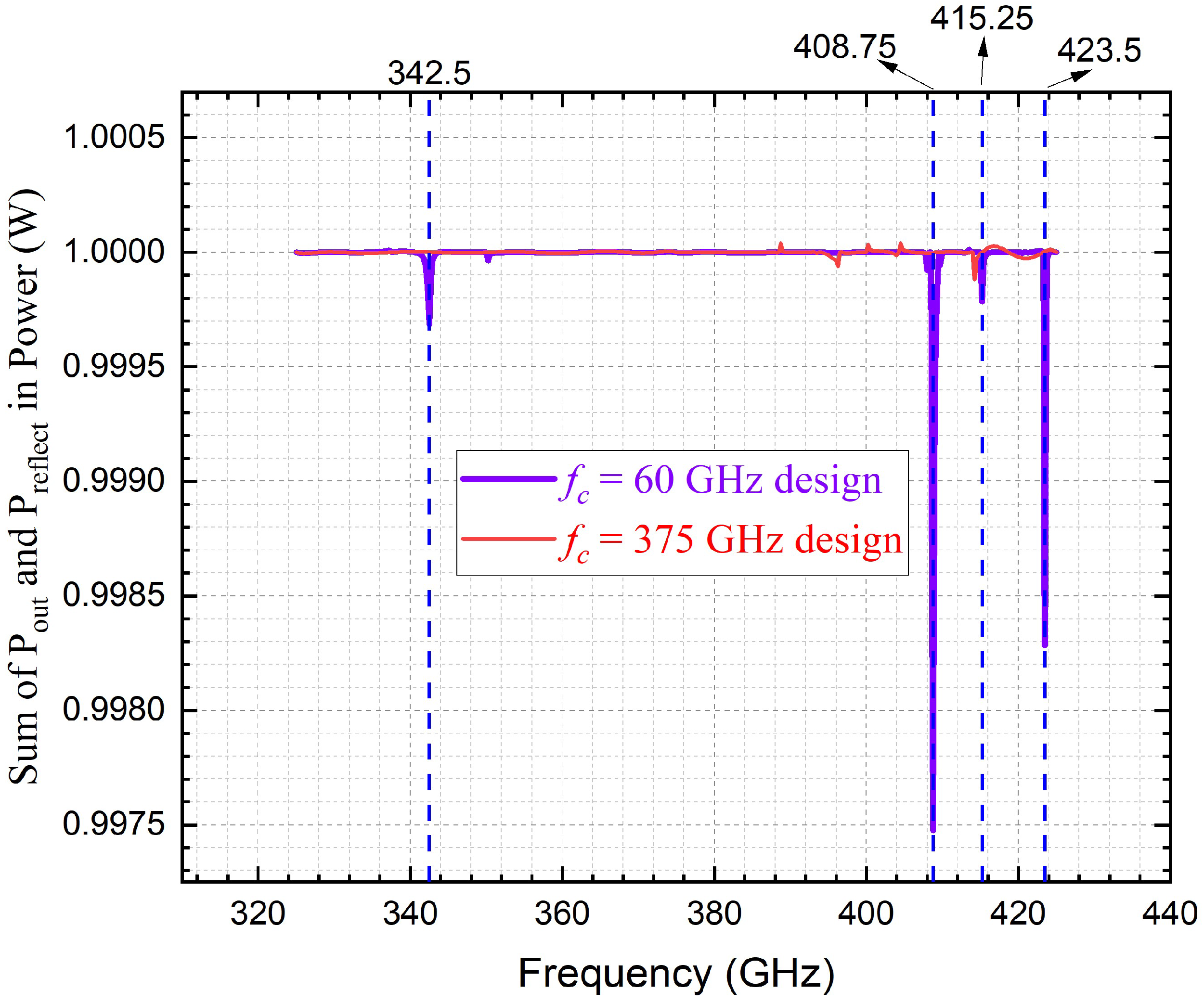
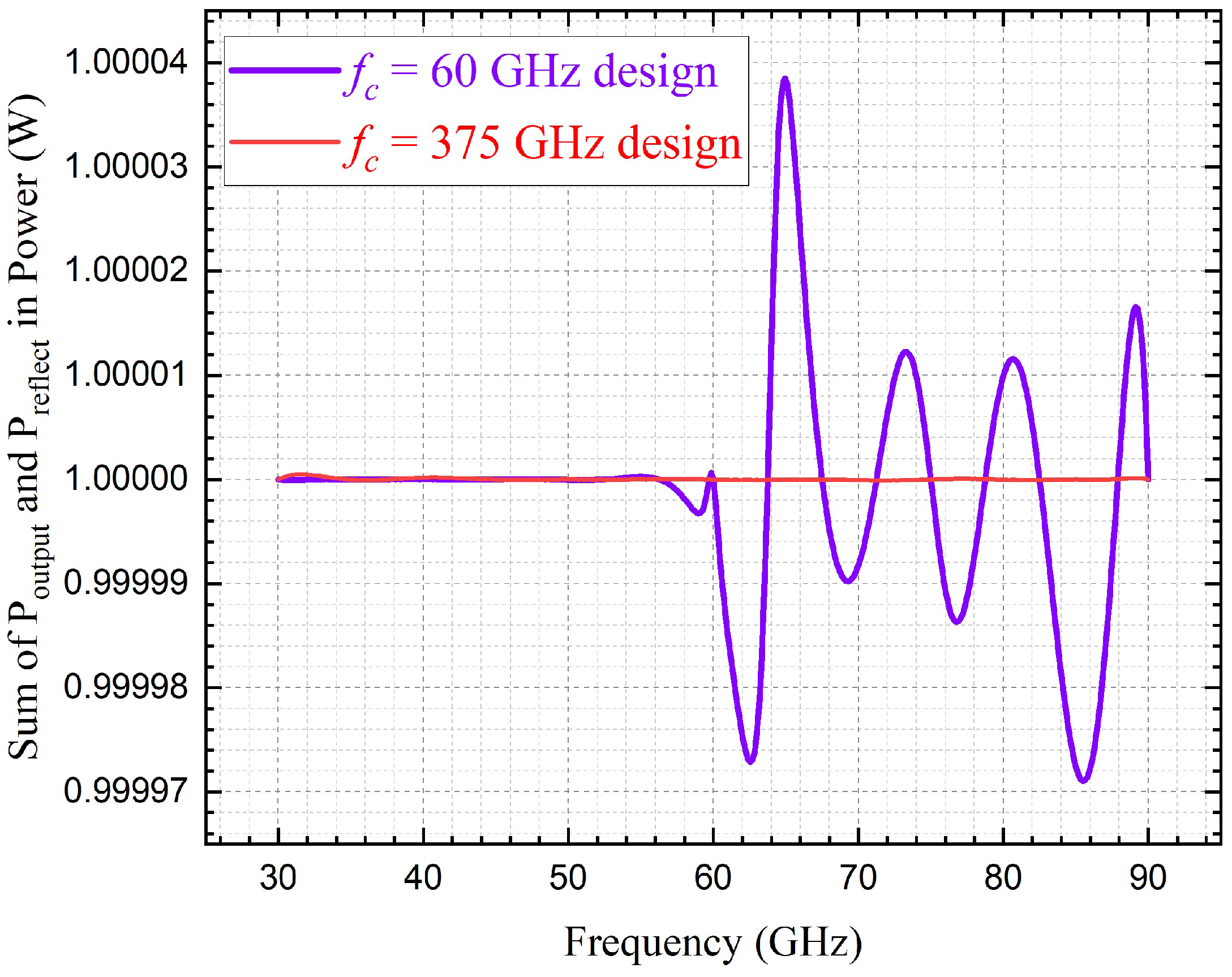
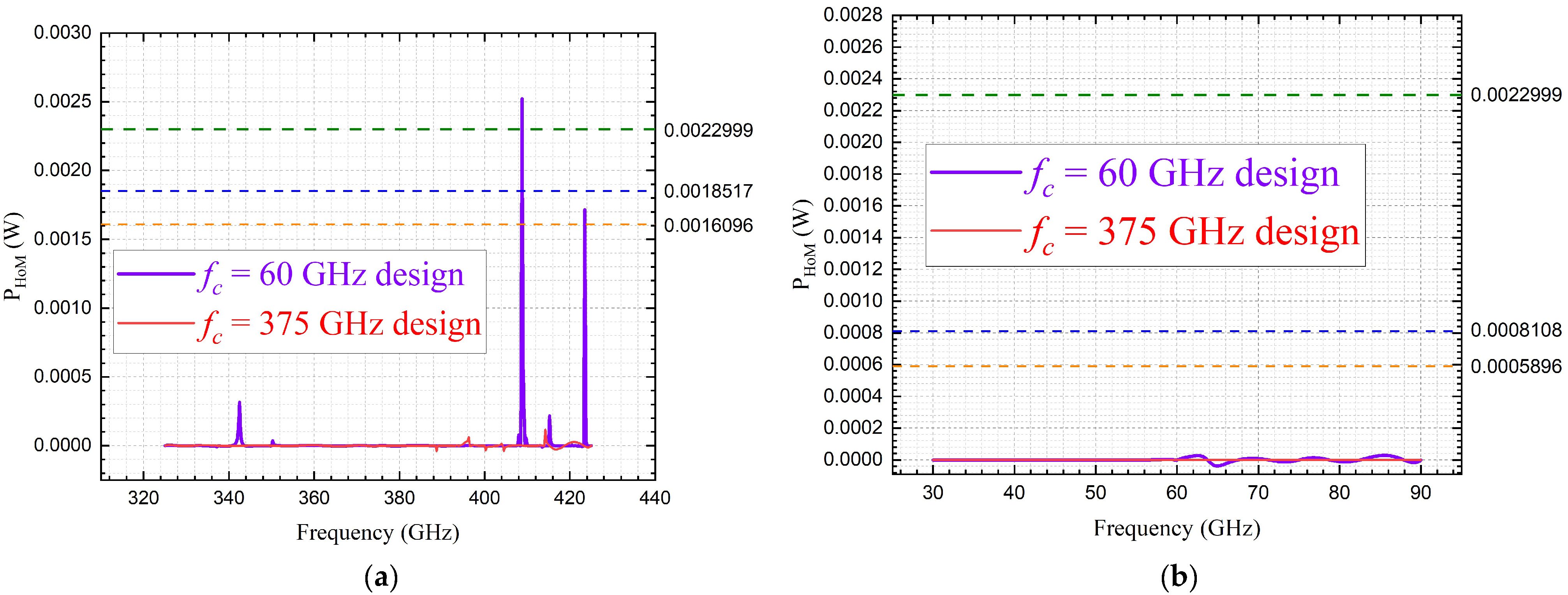
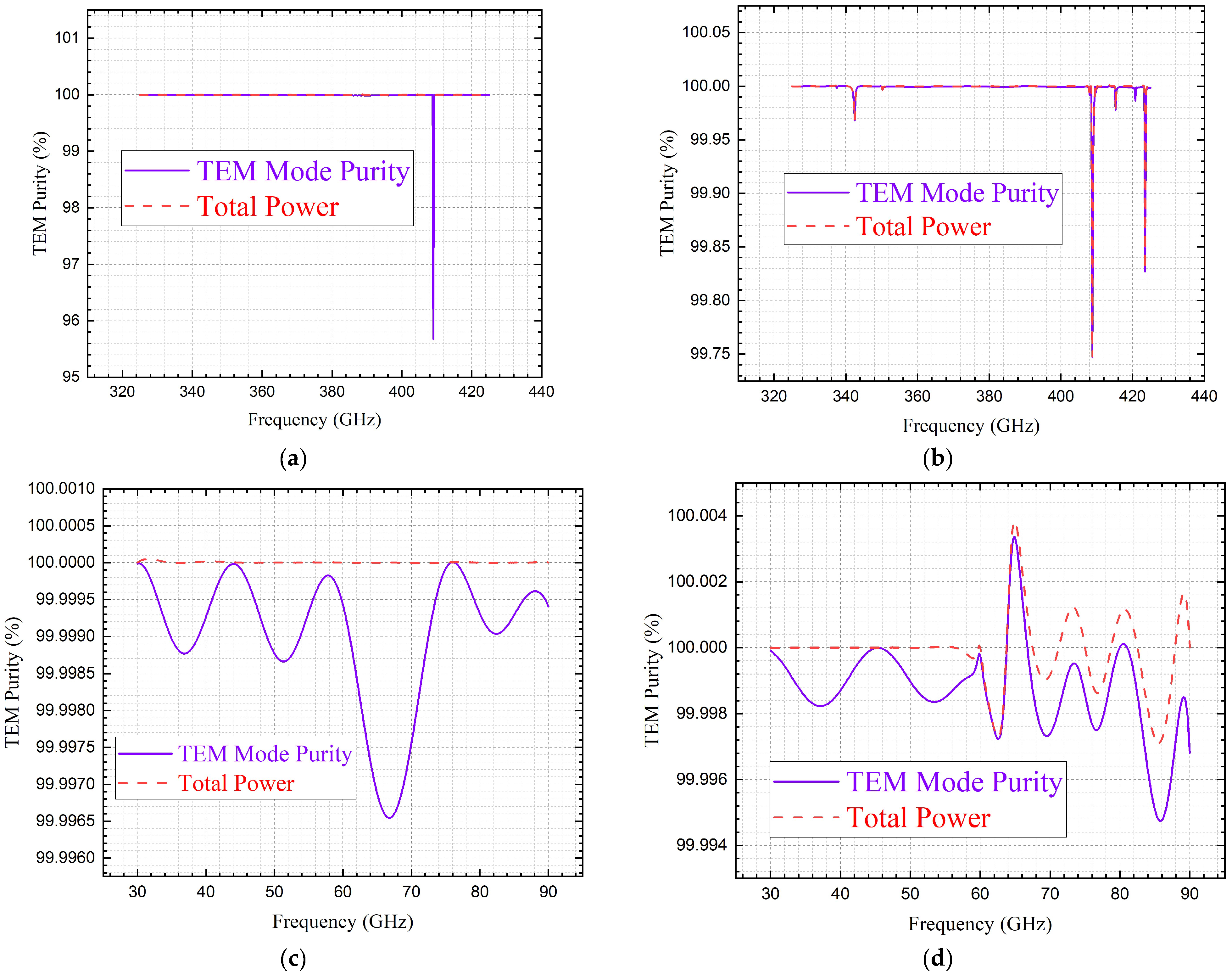
3.3. Quantification of HoM Power and TEM-Mode Purity
4. Discussion and Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| Nomenclatures | Abbreviations |
| Higher-order mode | HoM |
| Transverse electromagnetic | TEM |
| Transverse Electric | TE |
| Microwave | MW |
| Millimeter wave | mmW |
| Gigahertz | GHz |
| Terahertz | THz |
| Fifth-generation wireless | 5G |
| Sixth-generation wireless | 6G |
| Electronic design automation | EDA |
| High-frequency structure simulator | HFSS |
| Cut-off frequency | fc |
| Perfect electric conductor | PEC |
| Scattering parameters | S-parameters |
| Forward transmission coefficient | S21 |
| Forward reflection coefficient | S11 |
| Core line diameter | Dcore |
| Dielectric thickness | TDk |
| Dielectric constant | Dk |
| Dissipation factor | DF |
| Effective dielectric constant | εr |
| Three-dimensional | 3D |
| Finite-element method | FEM |
| Figure of merit | FoM |
| High-performance computing | HPC |
| Output power | Pout |
| Input power | Pin |
| Reflected power | Preflect |
| Higher-order mode power | PHoM |
| Characteristic impedance | Zc |
| Speed of light | c |
| Minimum | Min. |
| Maximum | Max. |
| Root-mean-square | RMS |
| Standard deviation | Std Dev. |
| Central processing unit | CPU |
| Computer simulation technology | CST |
| Vector network analyzer | VNA |
References
- Gopal, A.S. Ansys 2023 Annual Report; Ansys Inc.: Canonsburg, PA, USA, 2023; pp. 1–118. [Google Scholar]
- Cendes, Z. The development of HFSS. In Proceedings of the 2016 USNC-URSI Radio Science Meeting, Fajardo, PR, USA, 26 June–1 July 2016; pp. 39–40. [Google Scholar]
- Han, Z.; Manafu, A. Simulation Application of Finite Element Analysis Software HFSS in Antenna Engineering Design Under the Background of Big Data. In Proceedings of the 2022 International Conference on Inventive Computation Technologies, Lalitpur, Nepal, 20–22 July 2022; pp. 710–713. [Google Scholar]
- Migalin, M.M.; Koshkid’ko, V.G.; Demshevsky, V.V. Automated SIW Slotted Waveguide Antenna Design Using ANSYS HFSS Scripts. In Proceedings of the 2023 Radiation and Scattering of Electromagnetic Waves, Divnomorskoe, Russia, 26–30 June 2023; pp. 188–191. [Google Scholar]
- Linkous, L.; Karincic, E.; Lundquist, J.; Topsakal, E. Automated Antenna Calculation, Design and Tuning Tool for HFSS. In Proceedings of the 2023 United States National Committee of URSI National Radio Science Meeting, Boulder, CO, USA, 10–14 January 2023; pp. 229–230. [Google Scholar]
- Makarushkin, G.G.; Klimov, K.N. Using the Ansys HFSS Software Package for 3D Numerical Electromagnetic Modeling of a Four-Channel Antenna. In Proceedings of the 2024 Systems of Signal Synchronization, Generating and Processing in Telecommunications, Vyborg, Russia, 1–3 July 2024; pp. 1–4. [Google Scholar]
- Kovacs, P.; Raida, Z. Dispersion analysis of planar metallo-dielectric EBG structures in Ansoft HFSS. In Proceedings of the MIKON 2008—17th International Conference on Microwaves, Radar and Wireless Communications, Wroclaw, Poland, 19–21 May 2008; pp. 1–4. [Google Scholar]
- Ahme, S. HFSS Helps Early Prediction of Gaseous Discharges in RF and Microwave Devices. In Proceedings of the 2023 IEEE International Conference on Plasma Science, Santa Fe, NM, USA, 21–25 May 2023; p. 1. [Google Scholar]
- Li, J.; Li, H. Liquid Crystal-Filled 60 GHz Coaxially Structured Phase Shifter Design and Simulation with Enhanced Figure of Merit by Novel Permittivity-Dependent Impedance Matching. Electronics 2024, 13, 626. [Google Scholar] [CrossRef]
- Ozturk, Y.; Hossain, M.M.S.; Arage, A.; Nahar, N.K.; Sertel, K. Printed Circuit Board-Based Thin-Film Dielectric Characterization Fixture for mmW Bands. In Proceedings of the 2024 IEEE INC-USNC-URSI Radio Science Meeting (Joint with AP-S Symposium), Florence, Italy, 14–19 July 2024; p. 32. [Google Scholar]
- Cabbia, M.; Fregonese, S.; Deng, M.; Curutchet, A.; Yadav, C.; Céli, D.; Matos, M.; Zimmer, T. Meander-Type Lines: An Innovative Design for On-Wafer TRL Calibration for mmW and Sub-mmW Frequencies Measurements. IEEE Trans. Terahertz Sci. Technol. 2021, 11, 367–374. [Google Scholar] [CrossRef]
- Li, J.; Li, H. Modeling 0.3 THz Coaxial Single-Mode Phase Shifter Designs in Liquid Crystals with Constitutive Loss Quantifications. Crystals 2024, 14, 364. [Google Scholar] [CrossRef]
- Oates, D.E.; Tolpygo, S.K.; Bolkhovsky, V. Submicron Nb Microwave Transmission Lines and Components for Single-Flux-Quantum and Analog Large-Scale Superconducting Integrated Circuits. IEEE Trans. Appl. Supercond. 2017, 27, 1–5. [Google Scholar] [CrossRef]
- Wang, K.; Wu, K. Liquid crystal enabled substrate integrated waveguide variable phase shifter for millimeter-wave application at 60ghz and beyond. In Proceedings of the 2015 IEEE MTT-S International Microwave Symposium, Phoenix, AZ, USA, 17–22 May 2015; pp. 1–4. [Google Scholar]
- Zhu, K.; Jiang, D.; Bai, T.; Zhang, W.; Zhang, T.; Gao, J. Design of a terahertz phase shifter based on liquid crystal. In Proceedings of the 2021 14th UK-Europe-China Workshop on Millimetre-Waves and Terahertz Technologies, Lancaster, UK, 13–15 September 2021; pp. 1–3. [Google Scholar]
- Kowalski, E.J.; Shapiro, M.A.; Temkin, R.J. Simple Correctors for Elimination of High-Order Modes in Corrugated Waveguide Transmission Lines. IEEE Trans. Plasma Sci. 2014, 42, 29–37. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Li, J. Optically Steerable Phased Array Enabling Technology Based on Mesogenic Azobenzene Liquid Crystals for Starlink Towards 6G. In Proceedings of the 2020 IEEE Asia-Pacific Microwave Conference, Hong Kong, China, 8–11 December 2020; pp. 345–347. [Google Scholar]
- Neuder, R.; Späth, M.; Schüßler, M.; Sáez, A.J. Architecture for sub-100 ms liquid crystal reconfigurable intelligent surface based on defected delay lines. Commun. Eng. 2024, 3, 70. [Google Scholar] [CrossRef]
- Li, J.; Li, H. Computationally Sampling Surface and Volume Current Densities of Liquid Crystal Non-Planar Phase Shifters for Low-Loss 5G IoT and 6G AIoT. In Proceedings of the 2024 IEEE International Conference on Omni-Layer Intelligent Systems, London, UK, 29–31 July 2024; pp. 1–6. [Google Scholar]
- Chandramouli, D.; Maldonado, P.A.; Kolding, T. Evolution of Timing Services From 5G-A Toward 6G. IEEE Access 2023, 11, 35150–35157. [Google Scholar] [CrossRef]
- Guidotti, A.; Coralli, A.V.; Schena, V.; Chuberre, N.; Jaafari, M.; Puttonen, J.; Cioni, S. The path to 5G-Advanced and 6G Non-Terrestrial Network systems. In Proceedings of the 2022 11th Advanced Satellite Multimedia Systems Conference and the 17th Signal Processing for Space Communications Workshop, Graz, Austria, 6–8 September 2022; pp. 1–8. [Google Scholar]
- Li, J.; Li, H. Frequency Ripples Reduction in Differential Delay Time of Liquid Crystals Coaxial Delay Lines. In Proceedings of the 2024 IEEE INC-USNC-URSI Radio Science Meeting (Joint with AP-S Symposium), Florence, Italy, 14–19 July 2024; pp. 154–155. [Google Scholar]
- Green, H.E.; Cashman, J.D. Higher Order Mode Cutoff in Polygonal Transmission Lines. IEEE Trans. Microw. Theory Tech. 1985, 33, 67–69. [Google Scholar] [CrossRef]
- Liu, X.; Qi, W.; Mu, C. Analysis of higher-order modes in coaxial conical transmission line. In Proceedings of the 2017 International Workshop on Electromagnetics: Applications and Student Innovation Competition, London, UK, 30 May–1 June 2017; pp. 41–43. [Google Scholar]
- Kitabayashi, S.; Kuroki, F.; Kawahara, Y. Dielectric-tube-supported metal rod transmission line and its application to transition for 1mm coaxial connector at 80 GHz band. In Proceedings of the 2015 European Microwave Conference, Paris, France, 7–10 September 2015; pp. 355–358. [Google Scholar]
- Miller, C.M.; Sacks, R.C. Dielectric Loss in Coaxial Cables via Phase Delay Measurements. IEEE Trans. Instrum. Meas. 1974, 23, 447–454. [Google Scholar] [CrossRef]
- Walia, G.; Laforge, P.D.; Azam, S.; Paranthaman, R. Dielectric Characterization Using Reflected Group Delay of a Partially-Filled Coaxial Resonator. IEEE Access 2024, 12, 123581–123594. [Google Scholar] [CrossRef]
- Helszajn, J. Ridge, Coaxial, and Stripline Phase-shifters. In Microwave Polarizers, Power Dividers, Phase Shifters, Circulators, and Switches; IEEE: Piscataway, NJ, USA, 2019; pp. 81–93. [Google Scholar]
- Hensley, D.M.; Christodoulou, C.G.; Jackson, N. A Stretchable Liquid Metal Coaxial Phase Shifter. IEEE Open J. Antennas Propag. 2021, 2, 370–374. [Google Scholar] [CrossRef]
- Nose, T.; Ito, R.; Honma, M. Potential of Liquid-Crystal Materials for Millimeter-Wave Application. Appl. Sci. 2018, 8, 2544. [Google Scholar] [CrossRef]
- Garbvskiy, Y.; Zagoridnii, V.; Krivosik, P.; Lovejoy, J.; Camley, R.E.; Celinski, Z.; Glushchenko, A.; Dziaduszek, J.; Dabroeski, R. Liquid crystal phase shifter at millimeter wave frequencies. J. Appl. Phys. 2012, 111, 054504. [Google Scholar] [CrossRef]
- Nose, T.; Ito, R.; Honma, M. Characterization of Liquid Crystal Materials for Millimeter-Wave Wireless Communication. In Proceedings of the 2023 30th International Workshop on Active-Matrix Flatpanel Displays and Devices, Kyoto, Japan, 4–7 July 2023; pp. 20–23. [Google Scholar]
- Romanofsky, R.R.; Qureshi, A.H. A model for ferroelectric phase shifters. IEEE Trans. Magn. 2000, 36, 3491–3494. [Google Scholar] [CrossRef]
- Kremer, J.G.; Eltes, F.; Stark, P.; Stark, D.; Caimi, D.; Siegwart, H.; Offrein, B.J.; Fompeyrine, J.; Abel, S. A ferroelectric multilevel non-volatile photonic phase shifter. Nat. Photon. 2022, 16, 491–497. [Google Scholar] [CrossRef]
- Yasir, M.; Peinetti, F.; Savi, P. Enhanced Graphene Based Electronically Tunable Phase Shifter. Micromachines 2023, 14, 1877. [Google Scholar] [CrossRef] [PubMed]

| Dk | L | |||
|---|---|---|---|---|
| 1 | 375 GHz | 154.18 µm | 100.29 µm | 10 mm |
| 1 | 60 GHz | 626.83 µm | 963.62 µm | 10 mm |
| Wave Ports | Renormalizations to 50 Ω | ||
|---|---|---|---|
| 1 and 2 | Yes | ≥0 | |
| 1 and 2 | No | ≈0 |
| 13,926 Meshed Elements | Min. | Max. | RMS | Mean | Std Dev. |
|---|---|---|---|---|---|
| Edge length (µm) | 44.9616 | 264.766 | 129.899 | - | - |
| Element volume (µm3) | 739.734 | 177,813 | - | 56,104.5 | 27,025.5 |
| 44,194 Meshed Elements | Min. | Max. | RMS | Mean | Std Dev. |
|---|---|---|---|---|---|
| Edge length (µm) | 117.044 | 449.92 | 241.045 | - | - |
| Element volume (µm3) | 79,777.4 | 2,937,680 | - | 690,615 | 375,981 |
| Designs and Evaluation Cases | Average Memory/Process | Processes (Cores) | Time |
|---|---|---|---|
| fc = 375 GHz design (Dcore = 154.18 µm, TDk = 100.29 µm, L = 1 cm), evaluated from 325 GHz to 425 GHz | 184 MB | 8 (8) | 00:32:19 |
| fc = 60 GHz design (Dcore = 626.83 µm, TDk = 963.62 µm, L = 1 cm), evaluated from 325 GHz to 425 GHz | 1.01 GB | 8 (8) | 00:55:49 |
| fc = 375 GHz design (Dcore = 154.18 µm, TDk = 100.29 µm, L = 1 cm), evaluated from 30 GHz to 90 GHz | 148 MB | 8 (8) | 00:08:33 |
| fc = 60 GHz design (Dcore = 626.83 µm, TDk = 963.62 µm, L = 1 cm), evaluated from 30 GHz to 90 GHz | 86.8 MB | 8 (8) | 00:06:20 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, H.; Li, J. Advancing Microscale Electromagnetic Simulations for Liquid Crystal Terahertz Phase Shifters: A Diagnostic Framework for Higher-Order Mode Analysis in Closed-Source Simulators. Micro 2025, 5, 3. https://doi.org/10.3390/micro5010003
Li H, Li J. Advancing Microscale Electromagnetic Simulations for Liquid Crystal Terahertz Phase Shifters: A Diagnostic Framework for Higher-Order Mode Analysis in Closed-Source Simulators. Micro. 2025; 5(1):3. https://doi.org/10.3390/micro5010003
Chicago/Turabian StyleLi, Haorong, and Jinfeng Li. 2025. "Advancing Microscale Electromagnetic Simulations for Liquid Crystal Terahertz Phase Shifters: A Diagnostic Framework for Higher-Order Mode Analysis in Closed-Source Simulators" Micro 5, no. 1: 3. https://doi.org/10.3390/micro5010003
APA StyleLi, H., & Li, J. (2025). Advancing Microscale Electromagnetic Simulations for Liquid Crystal Terahertz Phase Shifters: A Diagnostic Framework for Higher-Order Mode Analysis in Closed-Source Simulators. Micro, 5(1), 3. https://doi.org/10.3390/micro5010003






