Translational Dynamics of Imidazolium-Based Ionic Liquids in Acetonitrile Solutions
Abstract
1. Introduction
2. Experimental Details
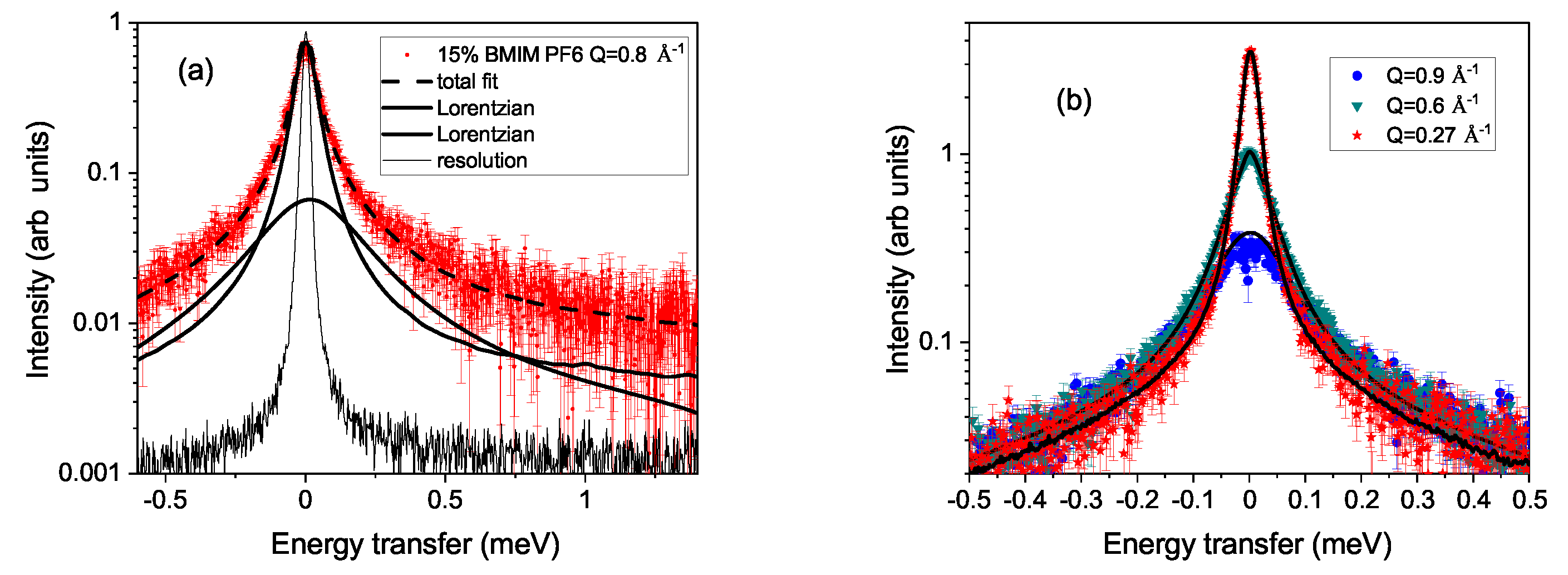
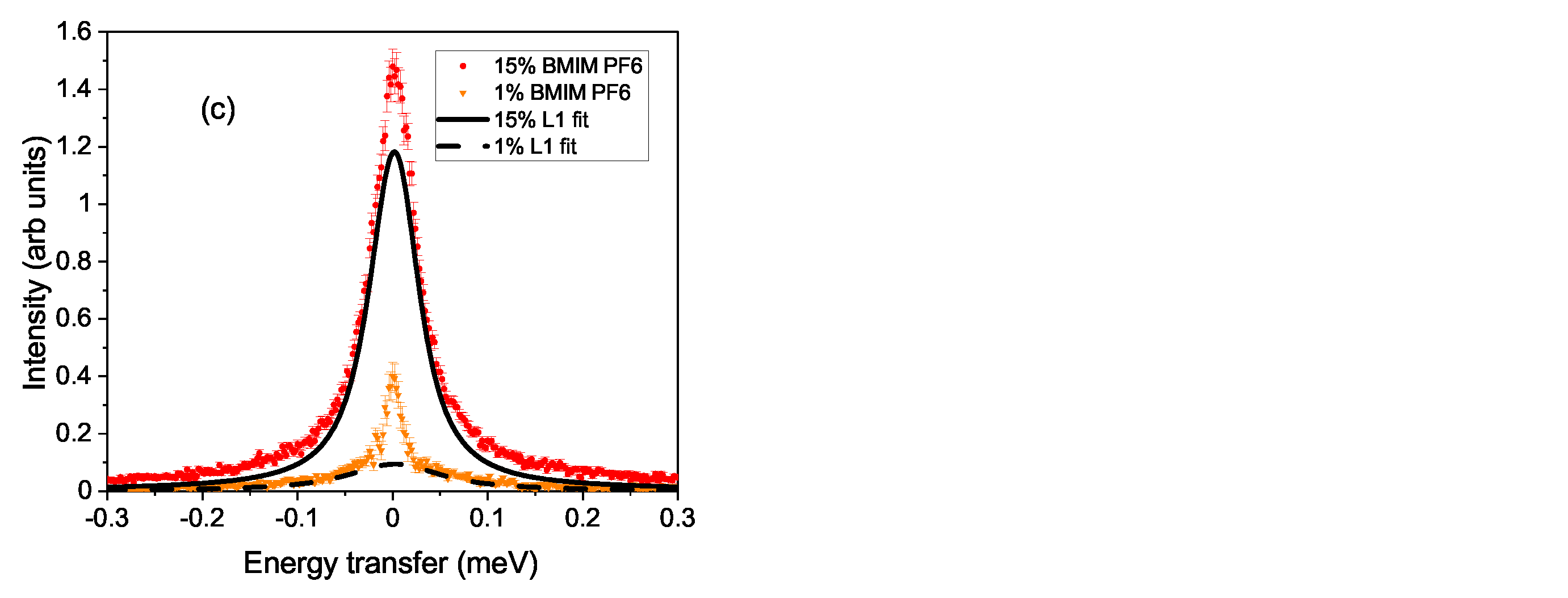
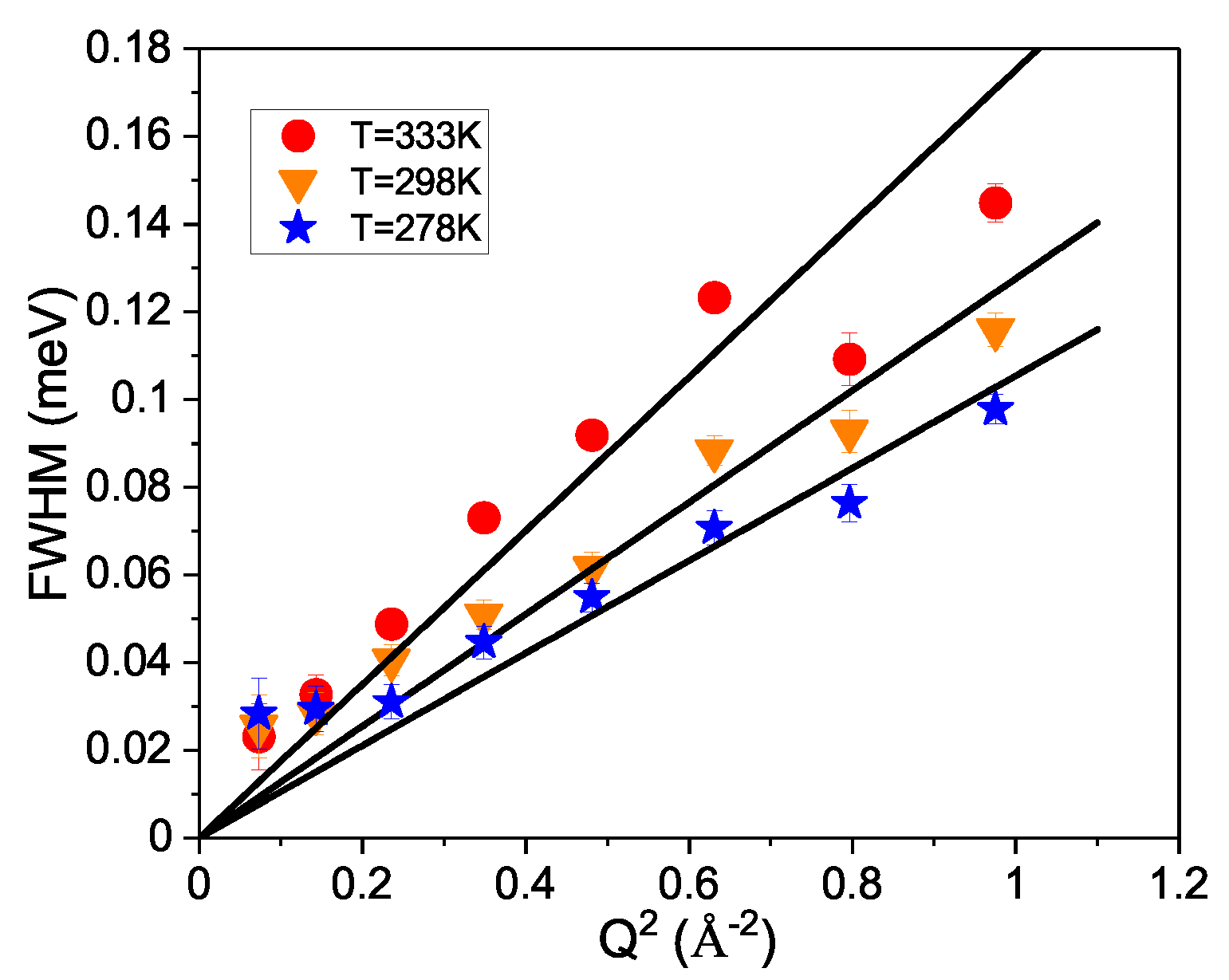
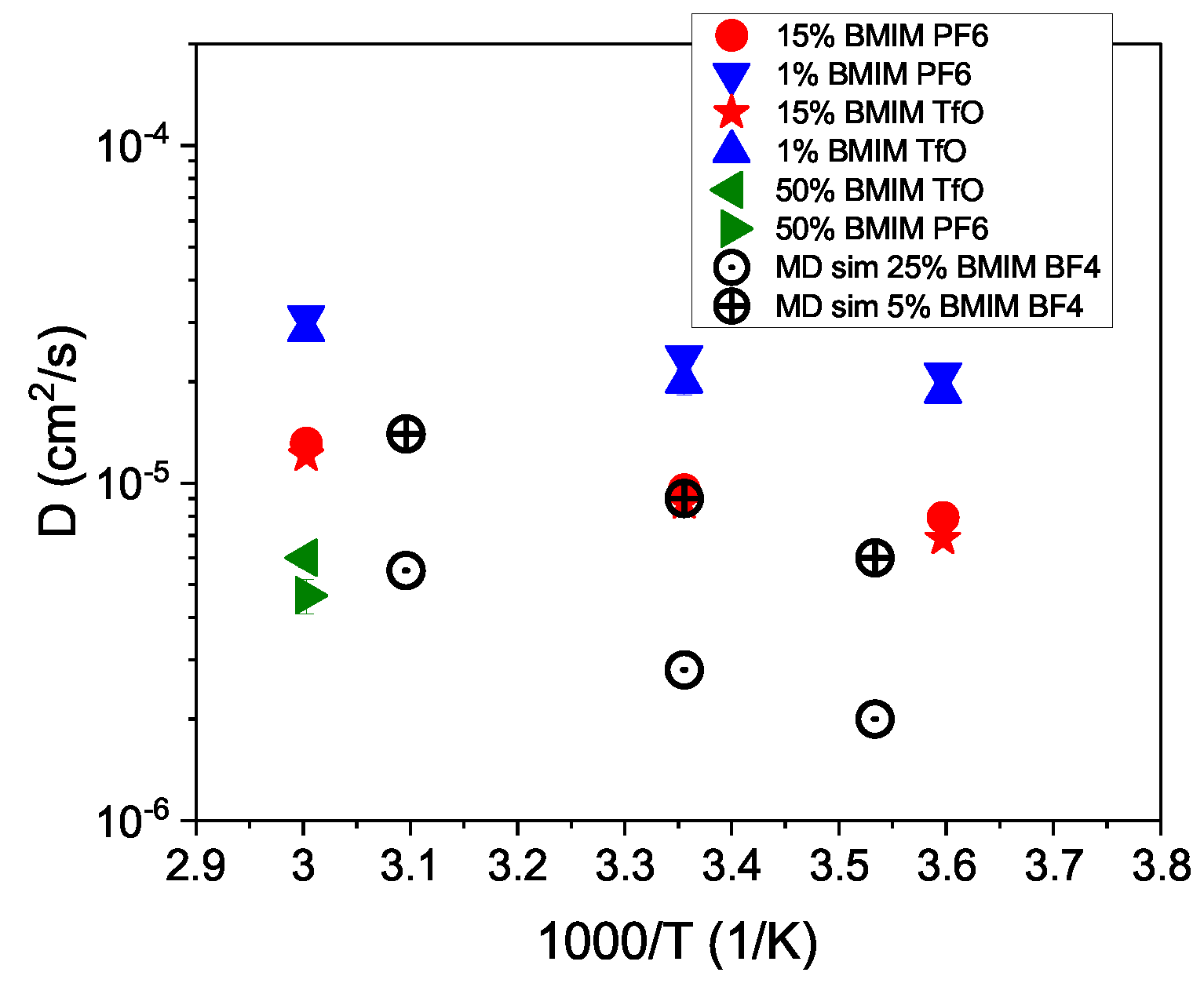
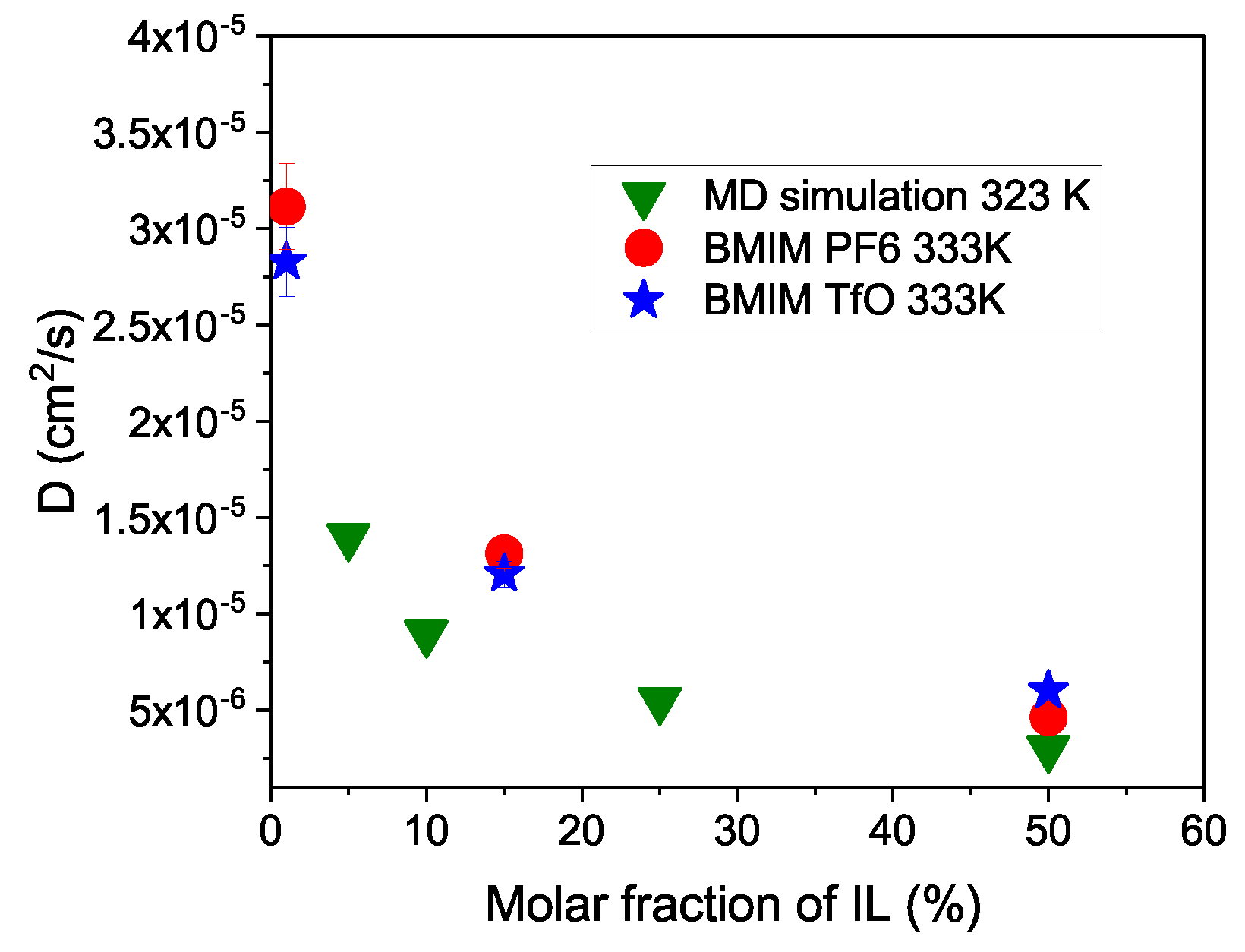
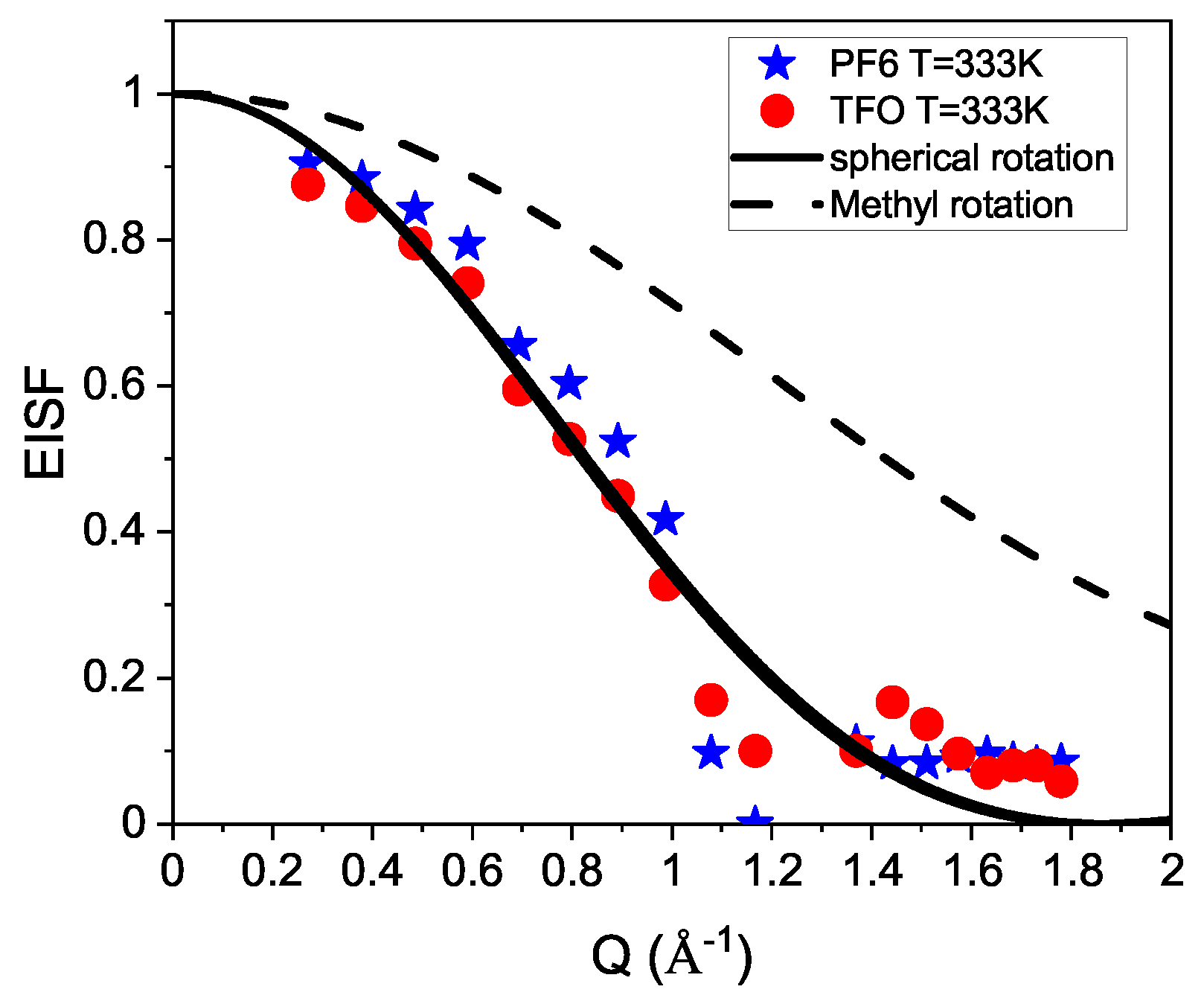
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Plechkova, N.V.; Seddon, K.R. Applications of ionic liquids in the chemical industry. Chem. Soc. Rev. 2008, 37, 123. [Google Scholar] [CrossRef]
- Welton, T. Room-Temperature Ionic Liquids. Solvents for Synthesis and Catalysis. Chem. Rev. 1999, 99, 2071. [Google Scholar] [CrossRef] [PubMed]
- Armand, M.; Endres, F.; MacFarlane, D.R.; Ohno, H.; Scrosati, B. ionic-liquid materials for the electrochemical challenges of the future. Nat. Mater. 2009, 8, 621. [Google Scholar] [CrossRef] [PubMed]
- Hardacre, C.; Holbrey, J.D.; McMath, S.E.J.; Bowron, D.T.; Soper, A.K. Structure of molten 1,3-dimethylimidazolium chloride using neutron diffraction. J. Chem. Phys. 2003, 118, 273. [Google Scholar] [CrossRef]
- Hardacre, C.; McMath, S.E.J.; Nieuwenhuyzen, M.; Bowron, D.T.; Soper, A.K. Liquid structure of 1,3-dimethylimidazolium salts. J. Phys. Condens. Matter 2003, 15, S159. [Google Scholar] [CrossRef]
- Deetlefs, M.; Hardacre, C.; Nieuwenhuyzen, M.; Padua, A.A.H.; Sheppard, O.; Soper, A.K. Liquid Structure of the Ionic Liquid 1,3-Dimethylimidazolium Bis(trifluoromethyl)sulfonylamide. J. Phys. Chem. B 2006, 110, 12055. [Google Scholar] [CrossRef]
- Hardacre, C.; Holbrey, J.D.; Nieuwenhuyzen, M.; Youngs, T.G.A. Structure and Solvation in Ionic Liquids. ACC Chem. Res. 2007, 40, 1146. [Google Scholar] [CrossRef]
- Bowron, D.T.; D’Agostino, C.; Gladden, L.F.; Hardacre, C.; Holbrey, J.D.; Lagunas, M.C.; McGregor, J.; Mantle, M.D.; Mullan, C.L.; Youngs, T.G.A. Structure and Dynamics of 1-Ethyl-3-methylimidazolium Acetate via Molecular Dynamics and Neutron Diffraction. J. Phys. Chem. B 2010, 114, 7760. [Google Scholar] [CrossRef]
- Aoun, B.; Goldbach, A.; Kohara, S.; Wax, J.F.; Gonzalez, M.A.; Saboungi, M.L. Structure of a Prototypic Ionic Liquid: Ethyl-methylimidazolium Bromide. J. Phys. Chem. B 2010, 114, 12623. [Google Scholar] [CrossRef]
- Macchiagodena, M.; Gontrani, L.; Ramondo, F.; Triolo, A.; Caminiti, R. Liquid structure of 1-alkyl-3-methylimidazolium-hexauorophosphates by wide angle X-ray and neutron scattering and molecular dynamics. J. Chem. Phys. 2011, 134, 114521. [Google Scholar] [CrossRef]
- Kastner, E.W.; Margulis, C.J.; Maroncelli, M.; Wishart, J.F. Ionic Liquids: Structure and Photochemical Reactions. Annu. Rev. Phys. Chem. 2011, 62, 85. [Google Scholar] [CrossRef] [PubMed]
- Bodo, E.; Gontrani, L.; Caminiti, R.; Plechkova, N.V.; Seddon, K.R.; Triolo, A. Structural Properties of 1-Alkyl-3-methylimidazolium Bis(trifluoromethyl)sulfonylamide Ionic Liquids: X-ray Diffraction Data and Molecular Dynamics Simulations. J. Phys. Chem. B 2010, 114, 16398. [Google Scholar] [CrossRef] [PubMed]
- Triolo, A.; Russina, O.; Bleif, H.J.; Di Cola, E. Nanoscale Segregation in Room Temperature Ionic Liquids. J. Phys. Chem. B 2007, 111, 4641. [Google Scholar] [CrossRef] [PubMed]
- Triolo, A.; Russina, O.; Fazio, B.; Triolo, R.; Di Cola, E. Morphology of 1-alkyl-3-methylimidazolium hexafluorophosphate room temperature ionic liquids. Chem. Phys. Lett. 2008, 457, 362. [Google Scholar]
- Triolo, A.; Russina, O.; Fazio, B.; Appetecchi, G.B.; Carewska, M.; Passerini, S. Nanoscale organization in piperidinium-based room temperature ionic liquids. J. Chem. Phys. 2009, 130, 164521. [Google Scholar] [CrossRef]
- Russina, O.; Triolo, A.; Gontrani, L.; Caminiti, R.; Xiao, D.; Hines, L.G., Jr.; Bartsch, R.A.; Quitevis, E.L.; Plechkova, N.; Seddon, K.R. Morphology and intermolecular dynamics of 1-alkyl-3-methylimidazolium bis(trifluoromethane)sulfonylamide ionic liquids: Structural and dynamic evidence of nanoscale segregation. J. Phys. Condens. Matter 2009, 21, 424121. [Google Scholar] [CrossRef]
- Hardacre, C.; Holbrey, J.D.; Mullan, C.L.; Youngs, T.G.A.; Bowron, D.T. Small angle neutron scattering from 1-alkyl-3-methylimidazolium hexafluorophosphate ionic liquids Cnmim PF6, n=4, 6, and 8. J. Chem. Phys. 2010, 133, 074510. [Google Scholar] [CrossRef]
- Aoun, B.; Goldbach, A.; Gonzalez, M.A.; Kohara, S.; Price, D.L.; Saboungi, M.L. Nanoscale heterogeneity in alkyl- methylimidazolium bromide ionic liquids. J. Chem. Phys. 2011, 134, 104509. [Google Scholar] [CrossRef]
- Alcaraz, O.; Demmel, F.; Trullas, J. Single ion dynamics in molten sodium bromide. J. Chem. Phys. 2014, 141, 244508. [Google Scholar] [CrossRef]
- Demmel, F.; Mukhopadhyay, S. Quasielastic neutron scattering measurements and ab initio MD-simulations on single ion motions in molten NaF. J. Chem. Phys. 2016, 144, 014503. [Google Scholar] [CrossRef]
- Demmel, F. Sodium ion self-diffusion in molten NaBr probed over different length scale. Phys. Rev. E 2020, 101, 062603. [Google Scholar] [CrossRef] [PubMed]
- Triolo, A.; Russina, O.; Arrighi, V.; Juranyi, F.; Janssen, S.; Gordon, C.M. Quasielastic neutron scattering characterization of the relaxation processes in a room temperature ionic liquid. J. Chem. Phys. 2003, 119, 8549. [Google Scholar] [CrossRef]
- Triolo, A.; Russina, O.; Hardacre, C.; Nieuwenhuyzen, M.; Gonzalez, M.A.; Grimm, H. Relaxation Processes in Room Temperature Ionic Liquids: The Case of 1-Butyl-3-Methyl Imidazolium Hexafluorophosphate. J. Phys. Chem. B 2005, 109, 22061. [Google Scholar] [CrossRef] [PubMed]
- Triolo, A.; Mandanici, A.; Russina, O.; Rodriguez-Mora, V.; Cutroni, M.; Hardacre, C.; Nieuwenhuyzen, M.; Bleif, H.J.; Keller, L.; Ramos, M.A. Thermodynamics, Structure, and Dynamics in Room Temperature Ionic Liquids: The Case of 1-Butyl-3-methyl Imidazolium Hexafluorophosphate ([bmim][PF6]). J. Phys. Chem. B 2006, 110, 21357. [Google Scholar] [CrossRef]
- Mamontov, E.; Luo, H.; Dai, S. Proton Dynamics in N,N,N’,N’-Tetramethylguanidinium Bis(perfluoroethylsulfonyl)imide Protic Ionic Liquid Probed by Quasielastic Neutron Scattering. J. Phys. Chem. B 2009, 113, 159. [Google Scholar] [CrossRef]
- Russina, O.; Beiner, M.; Pappas, C.; Russina, M.; Arrighi, V.; Unruh, T.; Mullan, C.L.; Hardacre, C.; Triolo, A. Temperature Dependence of the Primary Relaxation in 1-Hexyl-3-methylimidazolium bis(trifluoromethyl)sulfonylimide. J. Phys. Chem. B 2009, 113, 8469. [Google Scholar] [CrossRef] [PubMed]
- Aoun, B.; Gonzalez, M.A.; Olivier, J.; Russina, M.; Izaola, Z.; Price, D.L.; Saboungi, M.L. Translational and Reorientational Dynamics of an Imidazolium-Based Ionic Liquid. J. Phys. Chem. Lett. 2010, 1, 2503. [Google Scholar] [CrossRef]
- Kofu, M.; Someya, T.; Tatsumi, S.; Ueno, K.; Ueki, T.; Watanabe, M.; Matsunaga, T.; Shibayama, M.; Garcia Sakai, V.; Tyagi, M.; et al. Microscopic insights into ion gel dynamics using neutron spectroscopy. Soft Matter 2012, 8, 7888. [Google Scholar] [CrossRef]
- Embs, J.P.; Burankova, T.; Reichert, E.; Fossog, V.; Hempelmann, R. QENS Study of Diffusive and Localized Cation Motions of Pyridinium-based Ionic Liquids. J. Phys. Soc. Jpn. 2013, 82, SA003. [Google Scholar] [CrossRef]
- Aoun, B.; Gonzlez, M.A.; Russina, M.; Price, D.L.; Saboungi, M.-L. Dynamics of butyl- and hexyl-methylimidazolium bromide ionic liquids. J. Phys. Soc. Jpn. 2013, 82, SA002. [Google Scholar] [CrossRef]
- Kofu, M.; Tyagi, M.; Inamura, Y.; Miyazaki, K.; Yamamuro, O. Quasielastic neutron scattering studies on glass-forming ionic liquids with imidazolium cations. J. Chem. Phys. 2015, 143, 234502. [Google Scholar] [CrossRef]
- Berrod, Q.; Ferdeghini, F.; Zanotti, J.M.; Judeinstein, J.; Lairez, D.; Garca Sakai, V.; Czakkel, O.; Fouquet, P.; Constantin, D. Ionic Liquids: Evidence of the viscosity scale-dependence. Sci. Rep. 2017, 7, 2241. [Google Scholar] [CrossRef]
- Lundin, F.; Aguilera, L.; Hansen, H.W.; Lages, S.; Labrador, A.; Niss, K.; Frick, B.; Matic, A. Structure and dynamics of highly concentrated LiTFSI/acetonitrile electrolytes. Phys. Chem. Chem. Phys. 2021, 23, 13819. [Google Scholar] [CrossRef] [PubMed]
- De Roche, J.; Gordon, C.M.; Imrie, C.T.; Ingram, M.D.; Kennedy, A.R.; Lo Celso, F.; Triolo, A. Application of Complementary Experimental Techniques to Characterization of the Phase Behavior of [C16mim][PF6] and [C14mim][PF6]. Chem. Mater. 2003, 15, 3089. [Google Scholar] [CrossRef]
- Rivera, A.; Brodin, A.; Pugachev, A.; Rössler, E.A. Orientational and translational dynamics in room temperature ionic liquids. J. Chem. Phys. 2007, 126, 114503. [Google Scholar] [CrossRef] [PubMed]
- Keaveney, S.T.; Schaffarczyk McHale, K.S.; Stranger, J.W.; Ganbold, B.; Price, W.S.; Harper, J.B. NMR Diffusion Measurements as a Simple Method to Examine SolventSolvent and SolventSolute Interactions in Mixtures of the Ionic Liquid [Bmim][N(SO2CF3)2] and Acetonitrile. ChemPhysChem 2016, 17, 3853. [Google Scholar] [CrossRef]
- Hoarfrost, M.L.; Tyagi, M.; Segalman, R.A.; Reimer, J.A. Proton Hopping and Long-Range Transport in the Protic Ionic Liquid [Im][TFSI], Probed by Pulsed-Field Gradient NMR and Quasi-Elastic Neutron Scattering. J. Phys. Chem. B 2012, 116, 8201. [Google Scholar] [CrossRef] [PubMed]
- Lengvinaite, D.; Klimavicius, V.; Balevicius, V.; Aidas, K. Computational NMR Study of Ion Pairing of 1-Decyl-3-methyl-imidazolium Chloride in Molecular Solvents. J. Phys. Chem. B 2020, 124, 10776. [Google Scholar] [CrossRef] [PubMed]
- Urahata, S.M.; Ribeiro, M.C.C. Structure of ionic liquids of 1-alkyl-3-methylimidazolium cations: A systematic computer simulation study. J. Chem. Phys. 2004, 124, 1855. [Google Scholar] [CrossRef]
- Urahata, S.M.; Ribeiro, M.C.C. Single particle dynamics in ionic liquids of 1- alkyl-3-methylimidazolium cations. J. Chem. Phys. 2005, 122, 024511. [Google Scholar] [CrossRef]
- Ribeiro, M.C.C. Correlation between Quasielastic Raman Scattering and Configurational Entropy in an Ionic Liquid. J. Phys. Chem. B 2007, 111, 5008. [Google Scholar] [CrossRef] [PubMed]
- Schröder, C.; Wakai, C.; Weingärtner, H.; Steinhauser, O. Collective rotational dynamics in ionic liquids: A computational and experimental study of 1-butyl-3-methyl-imidazolium tetra?uoroborate. J. Chem. Phys. 2007, 126, 084511. [Google Scholar] [CrossRef] [PubMed]
- Chaban, V.V.; Voroshylova, I.V.; Kalugin, O.N. A new force field model for the simulation of transport properties of imidazolium-based ionic liquids. Phys. Chem. Chem. Phys. 2011, 13, 7910. [Google Scholar] [CrossRef]
- Chaban, V.V.; Voroshylova, I.V.; Kalugin, O.N.; Prezhdo, O.V. Acetonitrile Boosts Conductivity of Imidazolium Ionic Liquids. J. Phys. Chem. B 2012, 116, 7719. [Google Scholar] [CrossRef] [PubMed]
- Paschoal, V.H.; Faria, L.F.O.; Ribeiro, M.C.C. Vibrational Spectroscopy of Ionic Liquids. Chem. Rev. 2017, 117, 7053. [Google Scholar] [CrossRef]
- Bodo, E. Perspectives in the Computational Modeling of New Generation, Biocompatible Ionic Liquids. J. Phys. Chem. B 2022, 126, 3. [Google Scholar] [CrossRef]
- Ruiz-Martin, M.D.; Quereshi, N.; Gonzales, M.A.; Olivier, J.; Frick, B.; Farago, B. Influence of water on the microscopic dynamics of 1-butyl-3-methylimidazolium tetrafluoroborate studied by means of quasielastic neutron scattering. J. Chem. Phys. 2022, 156, 084505. [Google Scholar] [CrossRef] [PubMed]
- Hurle, R.L.; Woolfe, L.A. Self-diffusion in Liquid Acetonitrile under Pressure. J. Chem. Phys. Faraday Trans. 1982, 78, 2233. [Google Scholar] [CrossRef]
- Cohen, S.R.; Plazanet, M.; Rols, S.; Voneshen, D.J.; Fourkas, J.T.; Coasne, B. Structure and dynamics of acetonitrile: Molecular simulation and neutron scattering. J. Mol. Liqu. 2022, 348, 118423. [Google Scholar] [CrossRef]
- Tokuda, H.; Tsuzuki, S.; Hasan Susan, A.B.; Hayamizu, K.; Watanabe, M. How Ionic Are Room-Temperature Ionic Liquids? An Indicator of the Physicochemical Properties. J. Phys. Chem. B 2006, 110, 19593. [Google Scholar] [CrossRef]
- Stoppa, A.; Hunger, J.; Buchner, R. Conductivities of Binary Mixtures of Ionic Liquids with Polar Solvents. J. Chem. Eng. Data 2009, 54, 472. [Google Scholar] [CrossRef]
- Sears, V.F. Neutron scattering lengths and cross sections. Neutron News 1992, 3, 26–37. [Google Scholar] [CrossRef]
- Bee, M. Quasielastic Neutron Scattering; Adam Hilger: Bristol, UK, 1988. [Google Scholar]
- Telling, M.T.F.; Andersen, K.H. Spectroscopic characteristics of the OSIRIS near-backscattering crystal analyser spectrometer on the ISIS pulsed neutron source. Phys. Chem. Chem. Phys. 2005, 7, 1255. [Google Scholar] [CrossRef] [PubMed]
- Demmel, F.; Pokhilchuk, K. The resolution of the tof-backscattering spectrometer OSIRIS: Monte Carlo simulations and analytical calculations. Nucl. Instr. Meth. A 2014, 767, 426. [Google Scholar] [CrossRef]
- Demmel, F.; Pasqualini, D.; Morkel, C. Inelastic collective dynamics of liquid rubidium with increasing temperature by neutron scattering studies. Phys. Rev. B 2006, 74, 184207. [Google Scholar] [CrossRef]
- Sivia, D.S.; Carlile, C.J.; Howells, W.S.; König, S. Bayesian analysis of quasielastic neutron scattering data. Phys. B 1992, 182, 341. [Google Scholar] [CrossRef]
- Arnold, O.; Bilheux, J.C.; Borreguero, J.M.; Buts, A.; Campbell, J.I.; Chapon, L.; Doucet, M.; Draper, N.; Leal, R.F.; Gigg, M.A.; et al. Mantid-Data analysis and visualization package for neutron scattering and mu SR experiments. Nucl. Instr. Meth. A 2014, 764, 156. [Google Scholar] [CrossRef]
- Pinkert, A.; Ang, K.L.; Marsh, K.N.; Pang, S. Density, viscosity and electrical conductivity of protic alkanolammonium ionic liquids. Phys. Chem. Chem. Phys. 2011, 13, 5136. [Google Scholar] [CrossRef]
- Zheng, Y.Z.; Wang, N.N.; Luo, J.J.; Zhoua, Y.; Yu, Z.W. Hydrogen-bonding interactions between [BMIM][BF4] and acetonitrile. Phys. Chem. Chem. Phys. 2013, 15, 18055. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Demmel, F.; Howells, W.S. Translational Dynamics of Imidazolium-Based Ionic Liquids in Acetonitrile Solutions. Liquids 2023, 3, 203-213. https://doi.org/10.3390/liquids3020015
Demmel F, Howells WS. Translational Dynamics of Imidazolium-Based Ionic Liquids in Acetonitrile Solutions. Liquids. 2023; 3(2):203-213. https://doi.org/10.3390/liquids3020015
Chicago/Turabian StyleDemmel, Franz, and William S. Howells. 2023. "Translational Dynamics of Imidazolium-Based Ionic Liquids in Acetonitrile Solutions" Liquids 3, no. 2: 203-213. https://doi.org/10.3390/liquids3020015
APA StyleDemmel, F., & Howells, W. S. (2023). Translational Dynamics of Imidazolium-Based Ionic Liquids in Acetonitrile Solutions. Liquids, 3(2), 203-213. https://doi.org/10.3390/liquids3020015






