Evaporation/Decomposition Behavior of 1-Butyl-3-Methylimidazolium Chloride (BMImCL) Investigated through Effusion and Thermal Analysis Techniques
Abstract
1. Introduction
2. Materials and Methods
2.1. Theoretical Background of the Evaporation/Decomposition Competition Study with Thermogravimetry and Knudsen Effusion Techniques
2.2. Material
2.3. Thermal Analysis and Knudsen Effusion Experimental Details
3. Results and Discussion
3.1. Thermogravimetric Analysis
3.2. Knudsen Effusion Mass Loss (KEML)
- ln[(K · Δm/Δt)/Pa g0.5 mol−0.5 K−0.5] = (38.785 ± 0.636) − (18727 ± 270)/T(K) for the 3 mm orifice
- ln[(K · Δm/Δt)/Pa g0.5 mol−0.5 K−0.5] = (43.836 ± 1.216) − (20524 ± 522)/T(K) for the 1 mm orifice
- ln[(K · Δm/Δt)/Pa g0.5 mol−0.5 K−0.5] = (38.855 ± 2.065) − (17844 ± 897)/T(K) for the 0.3 mm orifice.
3.3. Knudsen Effusion Mass Spectrometry (KEMS)
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Maton, C.; De Vos, N.; Stevens, C.V. Ionic liquid thermal stabilities: Decomposition mechanisms and analysis tools. Chem. Soc. Rev. 2013, 42, 5963–5977. [Google Scholar] [CrossRef]
- Xu, C.; Cheng, Z. Thermal Stability of Ionic Liquids: Current Status and Prospects for Future Development. Processes 2021, 9, 337. [Google Scholar] [CrossRef]
- Paolone, A.; Haddad, B.; Villemin, D.; Boumediene, M.; Fetouhi, B.; Assenine, M.A. Thermal Decomposition, Low Temperature Phase Transitions and Vapor Pressure of Less Common Ionic Liquids Based on the Bis(trifuoromethanesulfonyl)imide Anion. Materials 2022, 15, 5255. [Google Scholar] [CrossRef] [PubMed]
- Earle, M.J.; Esperança, J.M.S.S.; Gilea, M.; Canongia Lopes, J.N.A. The distillation and volatility of ionic liquids. Nature 2006, 439, 831–834. [Google Scholar] [CrossRef]
- Brunetti, B.; Ciccioli, A.; Gigli, G.; Lapi, A.; Misceo, N.; Tanzi, L.; Vecchio Ciprioti, S. Vaporization of the prototypical ionic liquid BMImNTf2 under equilibrium conditions: A multitechnique study. Phys. Chem. Chem. Phys. 2014, 16, 15653–15661. [Google Scholar] [CrossRef]
- Barulli, L.; Mezzetta, A.; Brunetti, B.; Guazzelli, L.; Vecchio Ciprioti, S.; Ciccioli, A. Evaporation thermodynamics of the tetraoctylphosphonium bis(trifluoromethansulfonyl)imide([P8888]NTf2) and tetraoctylphosphonium nonafluorobutane-1-sulfonate ([P8888]NFBS) ionic liquids. J. Mol. Liq. 2021, 333, 115892. [Google Scholar] [CrossRef]
- Cao, Y.; Mu, T. Comprehensive Investigation on the Thermal Stability of 66 Ionic Liquids by Thermogravimetric Analysis. Ind. Eng. Chem. Res. 2014, 53, 8651–8664. [Google Scholar] [CrossRef]
- Verevkin, S.P.; Zaitsau, D.H.; Schick, C.; Heym, F. Development of Direct and Indirect Methods for the Determination of Vaporization Enthalpies of Extremely Low-Volatile Compounds. In Handbook of Thermal Analysis and Calorimetry; Vyazovkin, S., Koga, N., Schick, C., Eds.; Elsevier Science B.V.: Amsterdam, The Netherlands, 2018; Chapter 1; Volume 6, pp. 1–46. [Google Scholar] [CrossRef]
- Heym, F.; Korth, W.; Etzold, B.J.M.; Kern, C.; Jess, A. Determination of vapor pressure and thermal decomposition using thermogravimetrical analysis. Thermochim. Acta 2015, 622, 9–17. [Google Scholar] [CrossRef]
- Heym, F.; Etzold, B.J.M.; Kern, C.; Jess, A. Analysis of evaporation and thermal decomposition of ionic liquids by thermogravimetrical analysis at ambient pressure and high vacuum. Green Chem. 2011, 13, 1453–1466. [Google Scholar] [CrossRef]
- Federghini, C.; Guazzelli, L.; Pomelli, C.S.; Ciccioli, A.; Brunetti, B.; Mezzetta, A.; Vecchio Ciprioti, S. Synthesis, thermal behavior and kinetic study of N-morpholinium dicationic ionic liquids by thermogravimetry. J. Mol. Liq. 2021, 332, 115662. [Google Scholar] [CrossRef]
- Brunetti, B.; Ciccioli, A.; Lapi, A.; Buzyurov, A.V.; Nagrimanov, R.N.; Varfolomeev, M.A.; Vecchio Ciprioti, S. Sublimation Study of Six 5-Substituted-1,10-Phenanthrolines by Knudsen Effusion Mass Loss and Solution Calorimetry. Entropy 2022, 24, 192. [Google Scholar] [CrossRef] [PubMed]
- Deyko, A.; Lovelock, K.R.J.; Licence, P.; Jones, R.G. The vapour of imidazolium-based ionic liquids: A mass spectrometry study. Phys. Chem. Chem. Phys. 2011, 13, 16841–16850. [Google Scholar] [CrossRef] [PubMed]
- Armstrong, J.P.; Hurst, C.; Jones, R.G.; Licence, P.; Lovelock, K.R.J.; Satterley, C.J.; Villar-Garcia, I.J. Vapourisation of ionic liquids. Phys. Chem. Chem. Phys. 2007, 9, 982–990. [Google Scholar] [CrossRef] [PubMed]
- Verevkin, S.P.; Zaitsau, D.H.; Emelyanenko, V.N.; Heintz, A. A New Method for the Determination of Vaporization Enthalpies of Ionic Liquids at Low Temperatures. J. Phys. Chem. B 2011, 115, 12889–12895. [Google Scholar] [CrossRef] [PubMed]
- Zaitsau, D.H.; Yermalayeu, A.V.; Emel’yanenko, V.N.; Butler, S.; Schubert, T.; Verevkin, S.P. Thermodynamics of Imidazolium-Based Ionic Liquids Containing PF6 Anions. J. Phys. Chem. B 2016, 120, 7949–7957. [Google Scholar] [CrossRef]
- Volpe, V.; Brunetti, B.; Gigli, G.; Lapi, A.; Vecchio Ciprioti, S.; Ciccioli, A. Toward the Elucidation of the Competing Role of Evaporation and Thermal Decomposition in Ionic Liquids: A Multitechnique Study of the Vaporization Behavior of 1-Butyl-3-methylimidazolium Hexafluorophosphate under Effusion Conditions. J. Phys. Chem. B 2017, 121, 10382–10393. [Google Scholar] [CrossRef]
- Clarke, C.J.; Puttick, S.; Sanderson, T.J.; Taylor, A.W.; Bourne, R.A.; Lovelock, K.R.J.; Licence, P. Thermal stability of dialkylimidazolium tetrafluoroborate and hexafluorophosphate ionic liquids: Ex situ bulk heating to complement in situ mass spectrometry. Phys. Chem. Chem. Phys. 2018, 20, 16786–16800. [Google Scholar] [CrossRef]
- Dunaev, A.M.; Motalov, V.B.; Kudin, L.S. The Composition of Saturated Vapor over 1-Butyl-3-methylimidazolium Tetrafluoroborate Ionic Liquid: A Multi-Technique Study of the Vaporization Process. Entropy 2021, 23, 1478. [Google Scholar] [CrossRef]
- Kamavaram, V.; Reddy, R.G. Thermal stabilities of di-alkylimidazolium chloride ionic liquids. Int. J. Thermal Sci. 2008, 47, 773–777. [Google Scholar] [CrossRef]
- Chambreau, S.D.; Boatz, J.A.; Vaghjiani, G.L.; Koh, C.J.; Kostko, O.; Golan, A.; Leone, S.R. Thermal Decomposition Mechanisms of 1-Ethyl-3-methylimidazolium Bromide Ionic Liquid. J. Phys. Chem. A 2012, 116, 5867–5876. [Google Scholar] [CrossRef]
- Lovelock, K.R.J.; Armostrong, J.P.; Licence, P.; Jones, R.G. Vaporisation and thermal decomposition of dialkylimidazolium halide ion ionic liquids. Phys. Chem. Chem. Phys. 2014, 16, 1339–1353. [Google Scholar] [CrossRef] [PubMed]
- Zaitsau, D.H.; Siewert, R.; Pimerzin, A.A.; Bülow, M.; Held, C.; Loor, M.; Schulz; Verevkin, S.P. From volatility to solubility: Thermodynamics of imidazolium-based ionic liquids containing chloride and bromide anions. J. Mol. Liq. 2017, 323, 114998. [Google Scholar] [CrossRef]
- Dunaev, A.M.; Motalov, V.B.; Kudin, L.S.; Butman, M.F. Thermodynamic properties of the ionic vapor species over EMImNTf2 ionic liquid studied by Knudsen effusion mass spectrometry. J. Mol. Liq. 2016, 223, 407–411. [Google Scholar] [CrossRef]
- Dunaev, A.M.; Motalov, V.B.; Kudin, L.S.; Butman, M.F. Molecular and ionic composition of saturated vapor over EMImNTf2. J. Mol. Liq. 2016, 219, 599–601. [Google Scholar] [CrossRef]
- Matsagar, B.M.; Dhepe, P.L. Effects of cations, anions and H+ concentration of acidic ionic liquids on the valorization of polysaccharides into furfural. New J. Chem. 2017, 41, 6137–6144. [Google Scholar] [CrossRef]
- Gilbert, A.; Haines, R.S.; Harper, J.B. Controlling the reactions of 1-bromogalactose acetate in methanol using ionic liquids as co-solvents. Org. Biomol. Chem. 2020, 18, 5442–5452. [Google Scholar] [CrossRef]
- Karaiskakis, G.; Gavril, D. Determination of diffusion coefficients by gas chromatography. J. Chromat. A 2004, 1037, 147–189. [Google Scholar] [CrossRef]
- Ciccioli, A.; Gigli, G. The uncertain bond energy of the NaAu molecule: Experimental redetermination and coupled cluster calculations. J. Phys. Chem. A 2013, 117, 4956–4962. [Google Scholar] [CrossRef]
- Lucci, E.; Giarrusso, S.; Gigli, G.; Ciccioli, A. Determination of the bond energies by Knudsen effusion mass spectrometry experiments combined with ab initio calculations. J. Chem. Phys. 2022, 157, 084303. [Google Scholar] [CrossRef]
- Long, G.T.; Vyazovkin, S.; Brems, B.A.; Wight, C.A. Competitive Vaporization and Decomposition of Liquid RDX. J. Phys. Chem. B 2000, 104, 2570–2574. [Google Scholar] [CrossRef]
- NIST Mass Spectrometry Data Center; Wallace, W.E. Mass Spectra. In NIST Chemistry WebBook, NIST Standard Reference Database Number 69; Linstrom, P.J., Mallard, W.G., Eds.; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2023. [Google Scholar] [CrossRef]
- Lias, S.G.; Levin, R.D.; Kafafi, S.A. Ion Energetics Data. In NIST Chemistry WebBook, NIST Standard Reference Database Number 69; Linstrom, P.J., Mallard, W.G., Eds.; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2023. [Google Scholar] [CrossRef]
- Bull, J.N.; Harland, P.W.; Vallance, C. Absolute Total Electron Impact Ionization Cross-Sections for Many-Atom Organic and Halocarbon Species. J. Phys. Chem. A 2012, 116, 767–777. [Google Scholar] [CrossRef] [PubMed]
- Gupta, D.; Rahla Naghma, R.; Bobby Antony, B. Electron impact total ionisation cross sections for simple bio-molecules: A theoretical approach. Mol. Phys. 2014, 112, 1201–1209. [Google Scholar] [CrossRef]

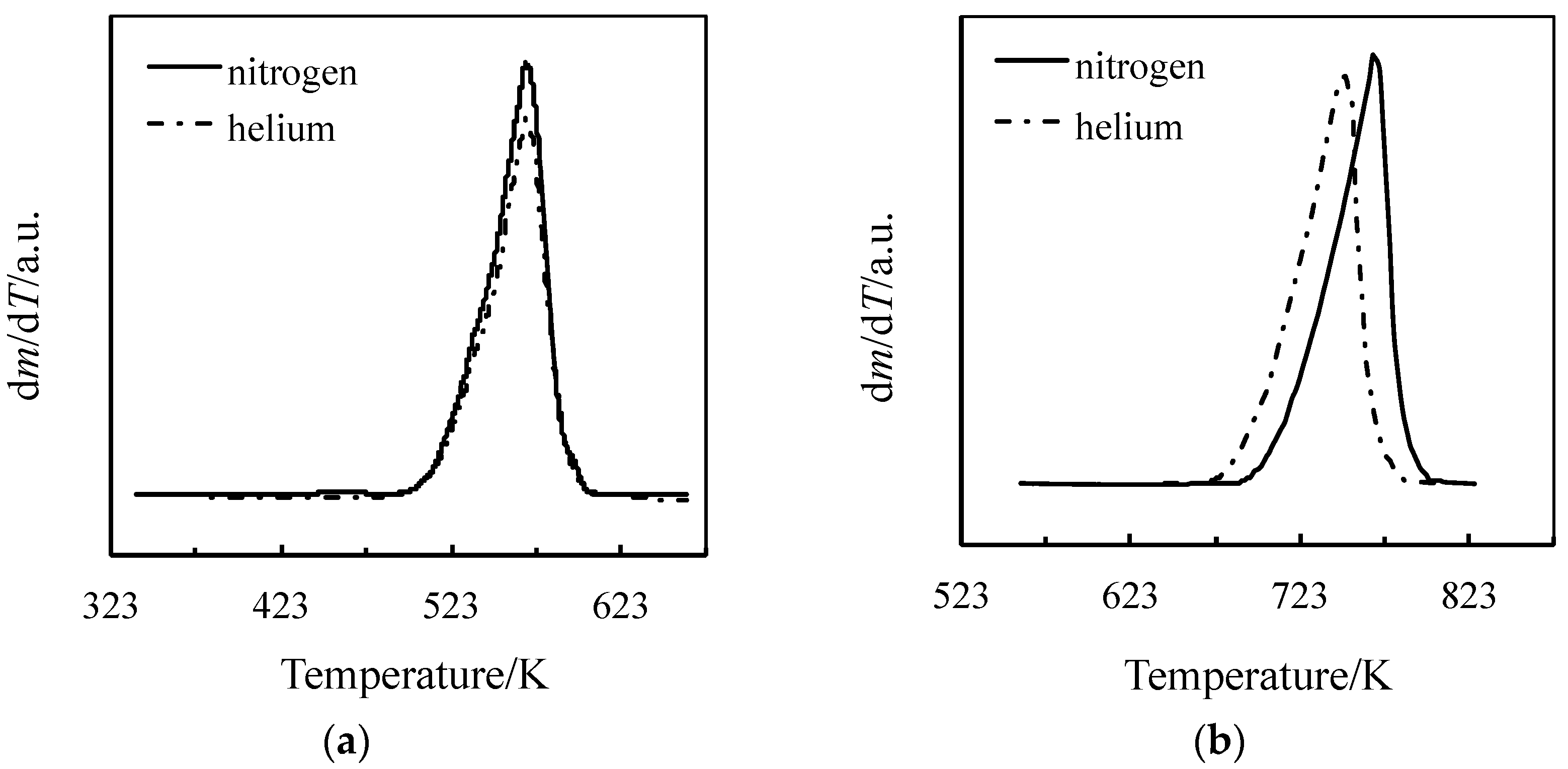

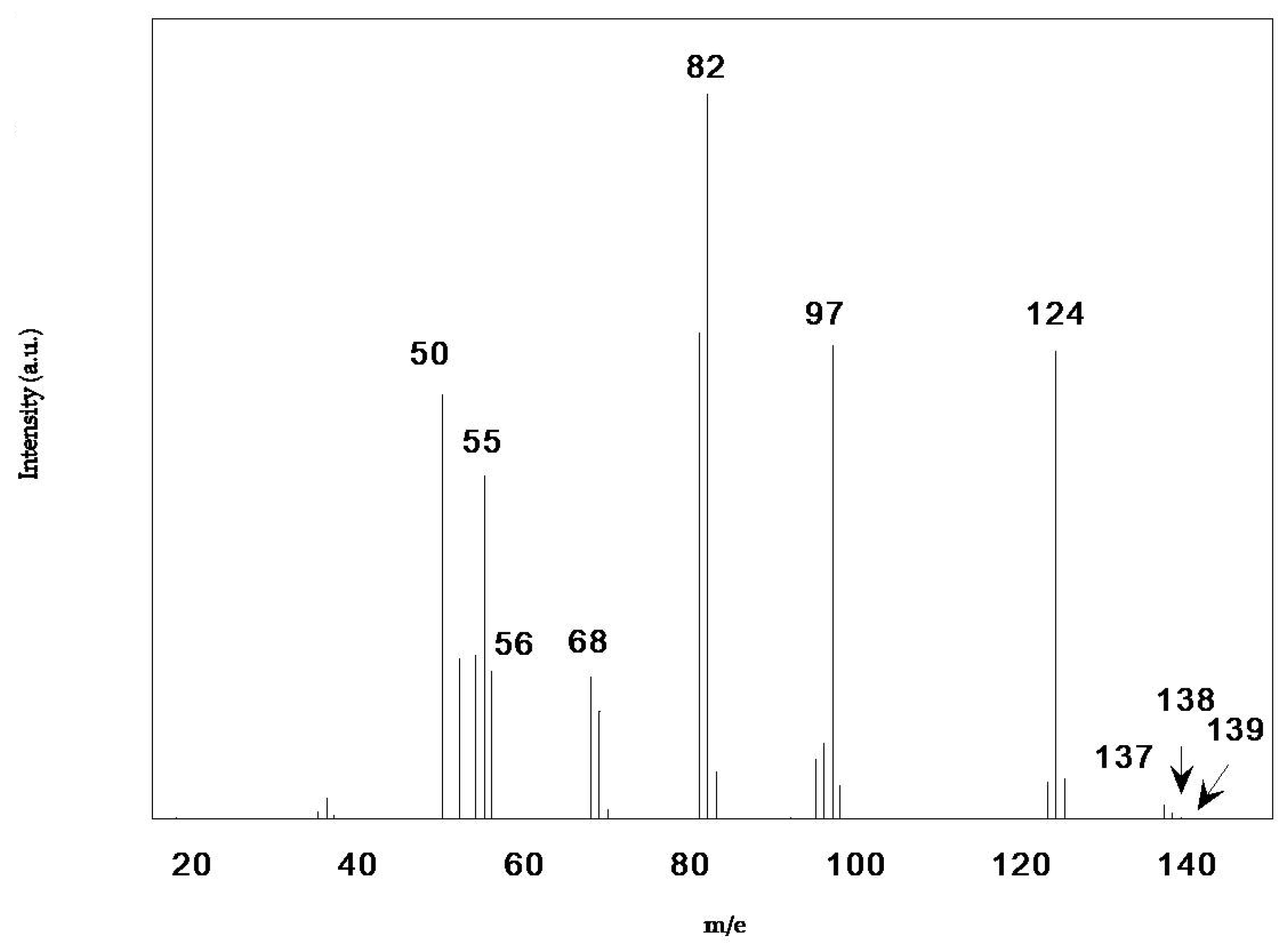
| Ionic Liquid | Molecular Formula | Molar Mass/ g mol−1 | CAS Number | Dig a/10−5 m2 s−1 | |
|---|---|---|---|---|---|
| Nitrogen | Helium | ||||
| BMImNTf2 | C10H15F6N3O4S2 | 419.4 | 174899-83-3 | 1.54 | 0.46 |
| BMImCl | C8H15ClN2 | 174.7 | 79917-90-1 | 2.03 | 0.60 |
| BMImBr | C8H15BrN2 | 219.1 | 85100-77-2 | 2.02 | 0.59 |
| BMImI | C8H15IN2 | 266.1 | 65039-05-6 | 1.97 | 0.57 |
| ϕ = 0.3 mm | ϕ = 1.0 mm | ϕ = 3.0 mm | |||
|---|---|---|---|---|---|
| T/K a | ln(K · ∆m/∆t) b | T/K a | ln(K · ∆m/∆t) b | T/K a | ln(K · ∆m/∆t) b |
| 457.5 | 0.17 | 480.7 | 1.24 | 452.7 | −2.56 |
| 448.0 | −0.81 | 468.2 | −0.032 | 443.0 | −3.41 |
| 438.1 | −1.66 | 457.8 | −1.01 | 433.3 | −4.37 |
| 428.3 | −2.50 | 448.0 | −2.09 | 423.1 | −5.72 |
| 418.5 | −3.33 | 438.3 | −3.07 | 412.7 | −6.56 |
| 452.7 | −0.60 | 473.0 | 0.29 | 402.8 | −7.63 |
| 443.0 | −1.47 | 463.4 | −0.31 | 447.6 | −3.09 |
| 433.2 | −2.35 | 453.1 | −1.25 | 437.9 | −3.97 |
| 423.0 | −3.29 | 442.7 | −2.29 | 428.2 | −4.95 |
| 413.2 | −4.16 | 467.4 | −0.06 | 418.4 | −5.98 |
| 455.7 | −0.48 | 457.7 | −1.23 | 408.2 | −7.07 |
| 445.9 | −1.40 | 448.0 | −2.03 | 398.4 | −8.23 |
| 436.2 | −2.34 | 438.1 | −3.03 | ||
| 427.5 | −3.15 | 433.2 | −3.56 | ||
| 416.5 | −4.14 | ||||
| 420.4 | −4.06 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brunetti, B.; Ciccioli, A.; Gigli, G.; Lapi, A.; Simonetti, G.; Toto, E.; Vecchio Ciprioti, S. Evaporation/Decomposition Behavior of 1-Butyl-3-Methylimidazolium Chloride (BMImCL) Investigated through Effusion and Thermal Analysis Techniques. Thermo 2023, 3, 248-259. https://doi.org/10.3390/thermo3020015
Brunetti B, Ciccioli A, Gigli G, Lapi A, Simonetti G, Toto E, Vecchio Ciprioti S. Evaporation/Decomposition Behavior of 1-Butyl-3-Methylimidazolium Chloride (BMImCL) Investigated through Effusion and Thermal Analysis Techniques. Thermo. 2023; 3(2):248-259. https://doi.org/10.3390/thermo3020015
Chicago/Turabian StyleBrunetti, Bruno, Andrea Ciccioli, Guido Gigli, Andrea Lapi, Giulia Simonetti, Elisa Toto, and Stefano Vecchio Ciprioti. 2023. "Evaporation/Decomposition Behavior of 1-Butyl-3-Methylimidazolium Chloride (BMImCL) Investigated through Effusion and Thermal Analysis Techniques" Thermo 3, no. 2: 248-259. https://doi.org/10.3390/thermo3020015
APA StyleBrunetti, B., Ciccioli, A., Gigli, G., Lapi, A., Simonetti, G., Toto, E., & Vecchio Ciprioti, S. (2023). Evaporation/Decomposition Behavior of 1-Butyl-3-Methylimidazolium Chloride (BMImCL) Investigated through Effusion and Thermal Analysis Techniques. Thermo, 3(2), 248-259. https://doi.org/10.3390/thermo3020015








