A 2D Moving Mesh Finite Element Analysis of Heat Transfer in Arctic Soils
Abstract
1. Introduction
Phase Change Modeling—Issues and Approaches to Latent Heat
2. Method
2.1. Meshing
2.2. Phase-Change Method
2.3. Boundary Conditions
2.4. Initial Conditions
3. Results
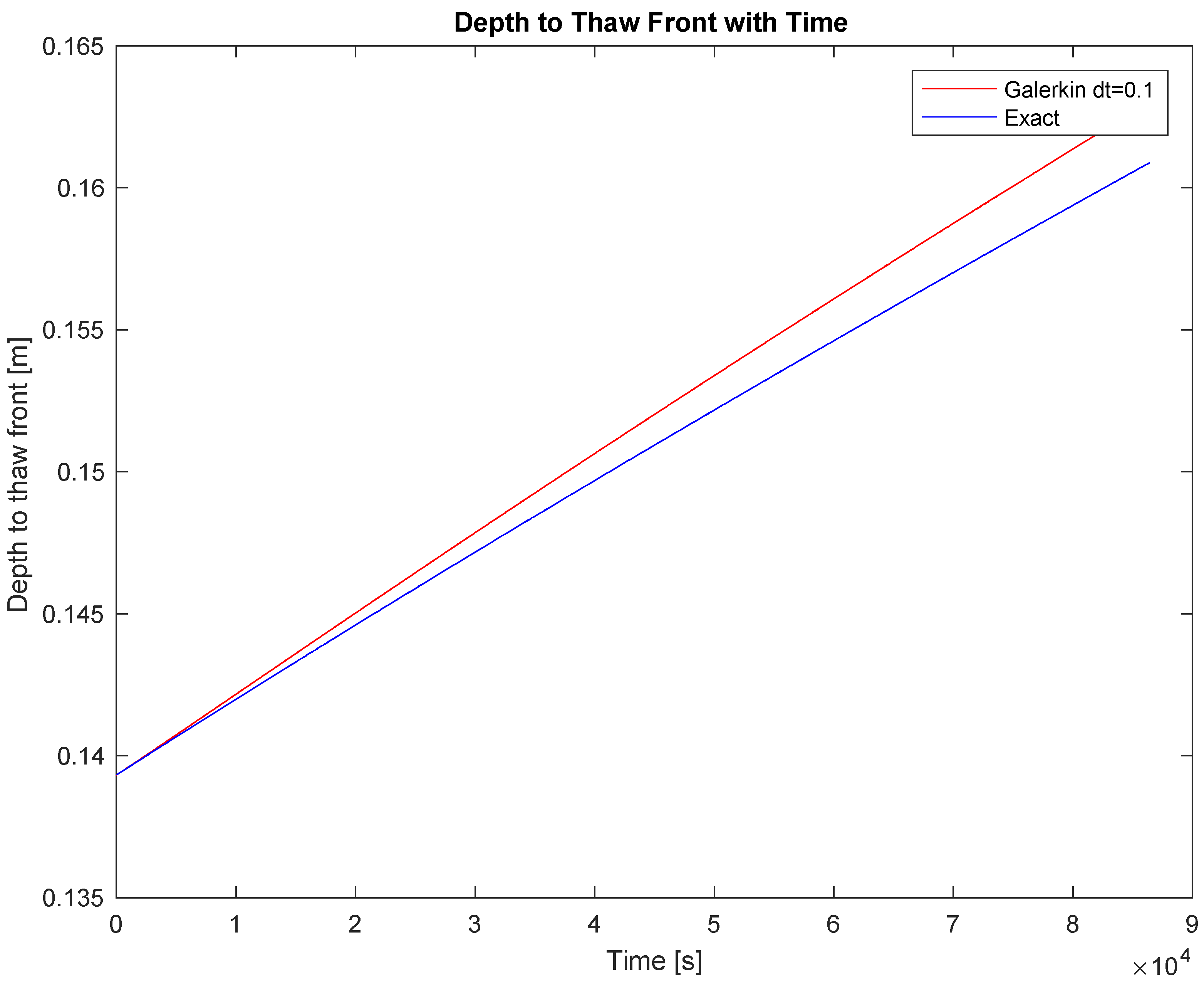
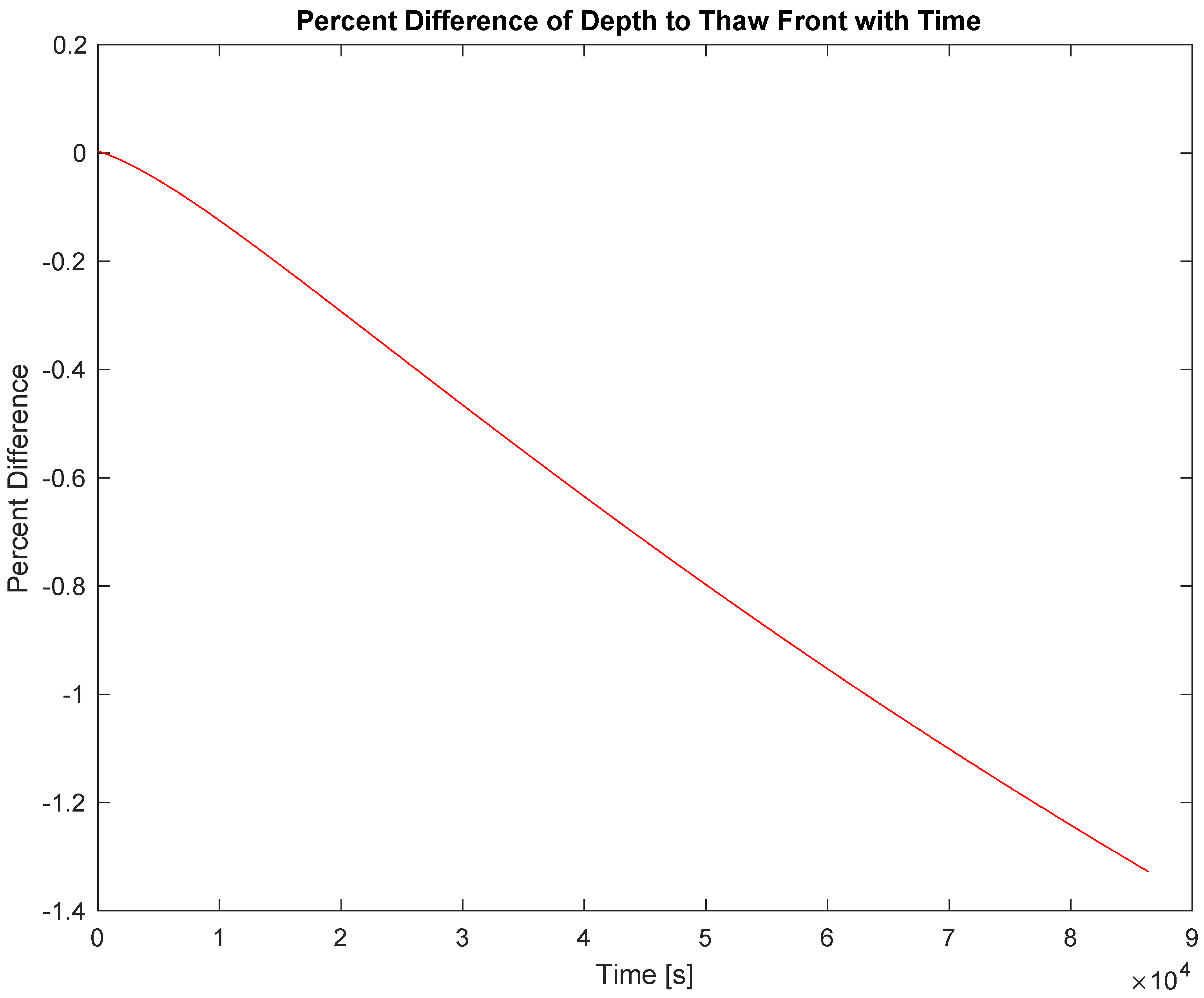
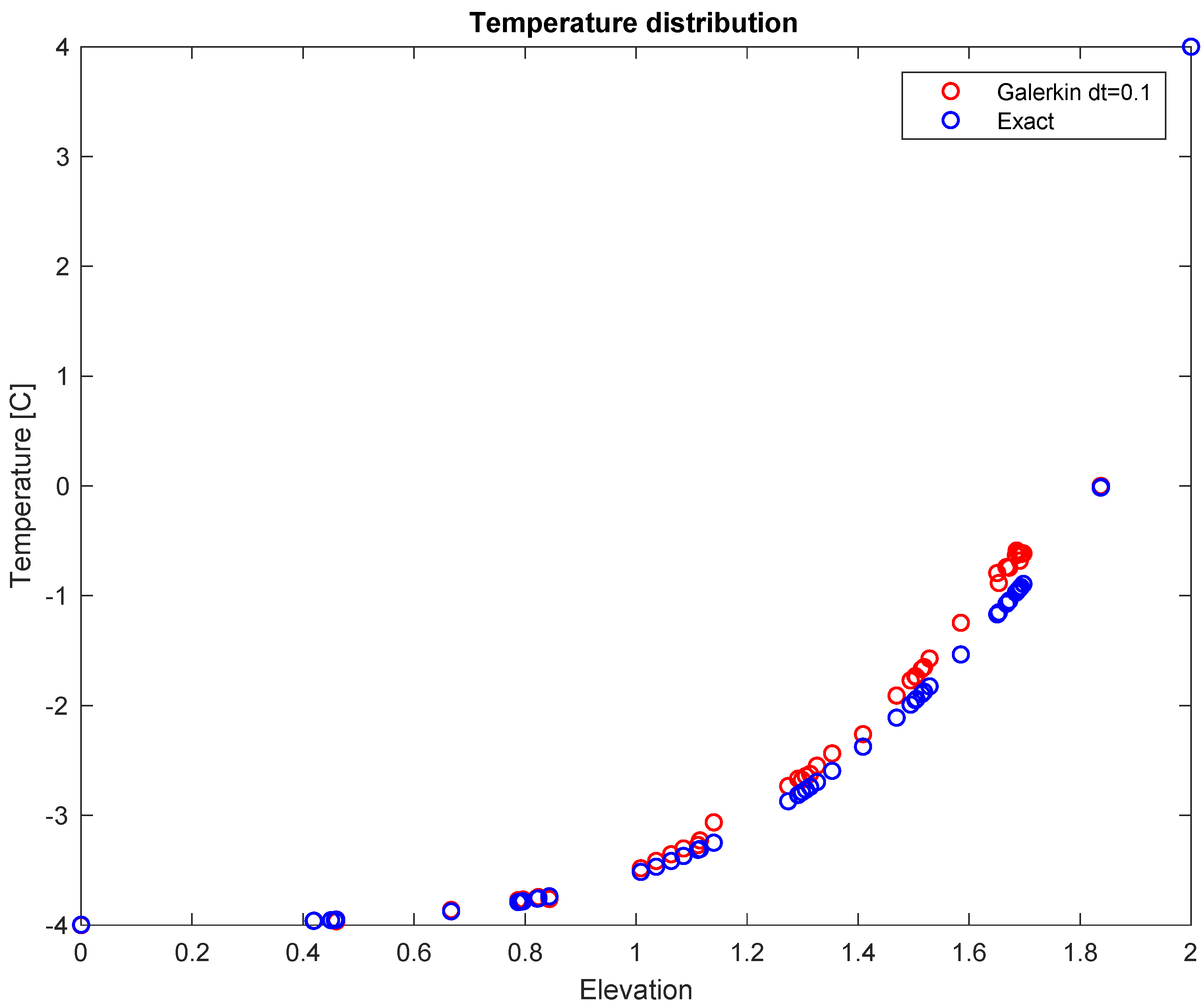
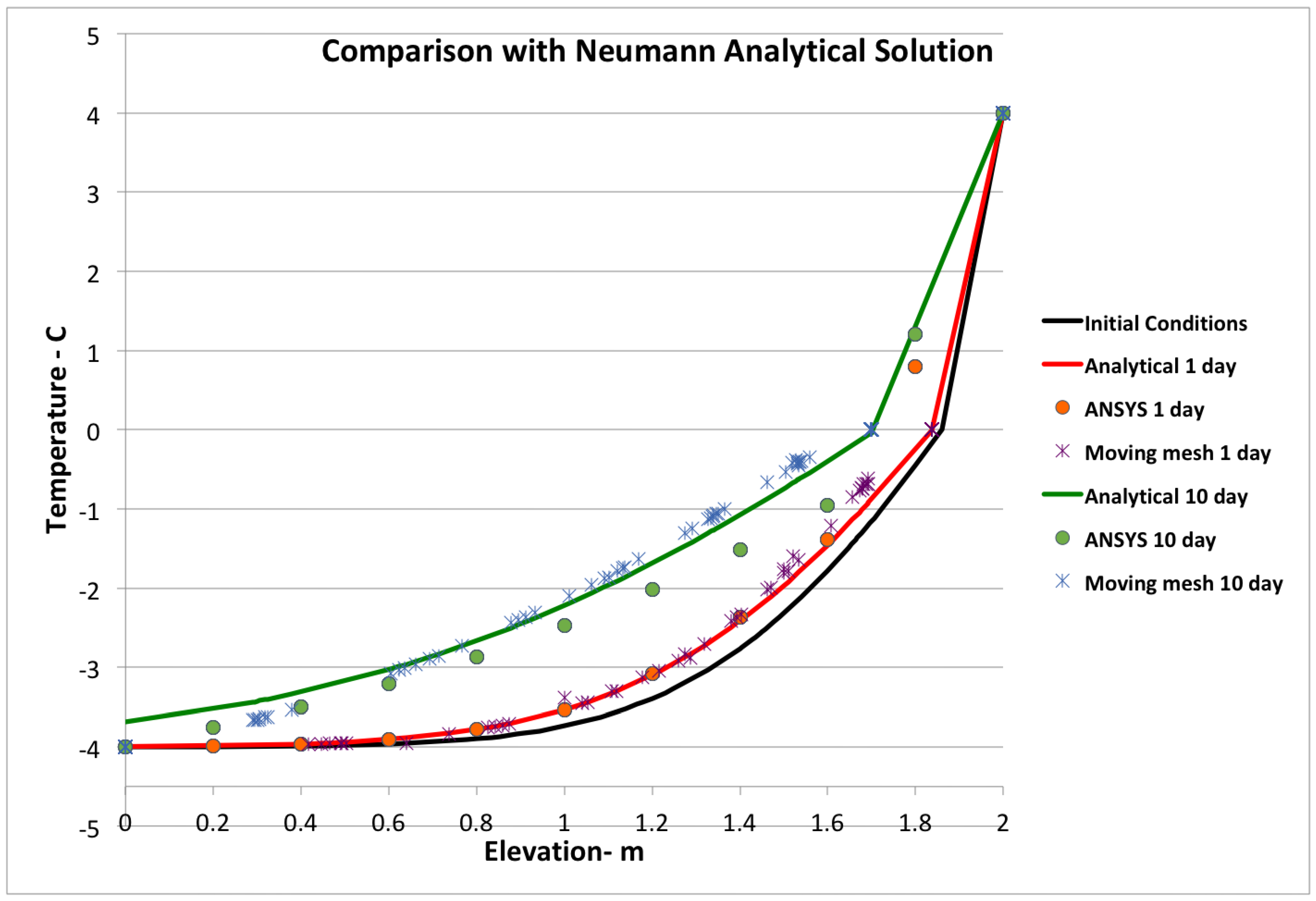
3.1. Analytical Check
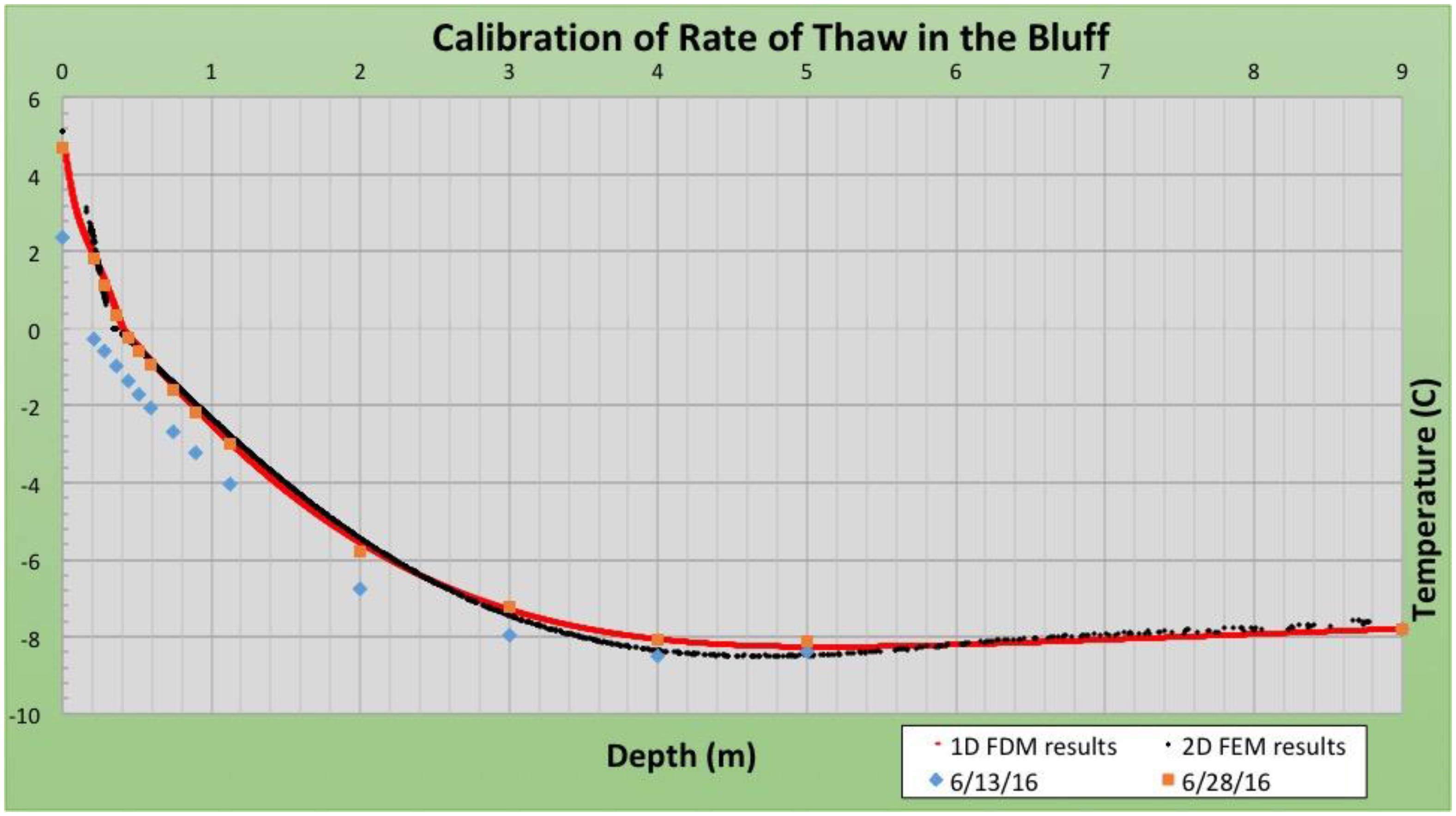
3.2. Check against 15 Days of Barrow Data and Finite Difference Model Results
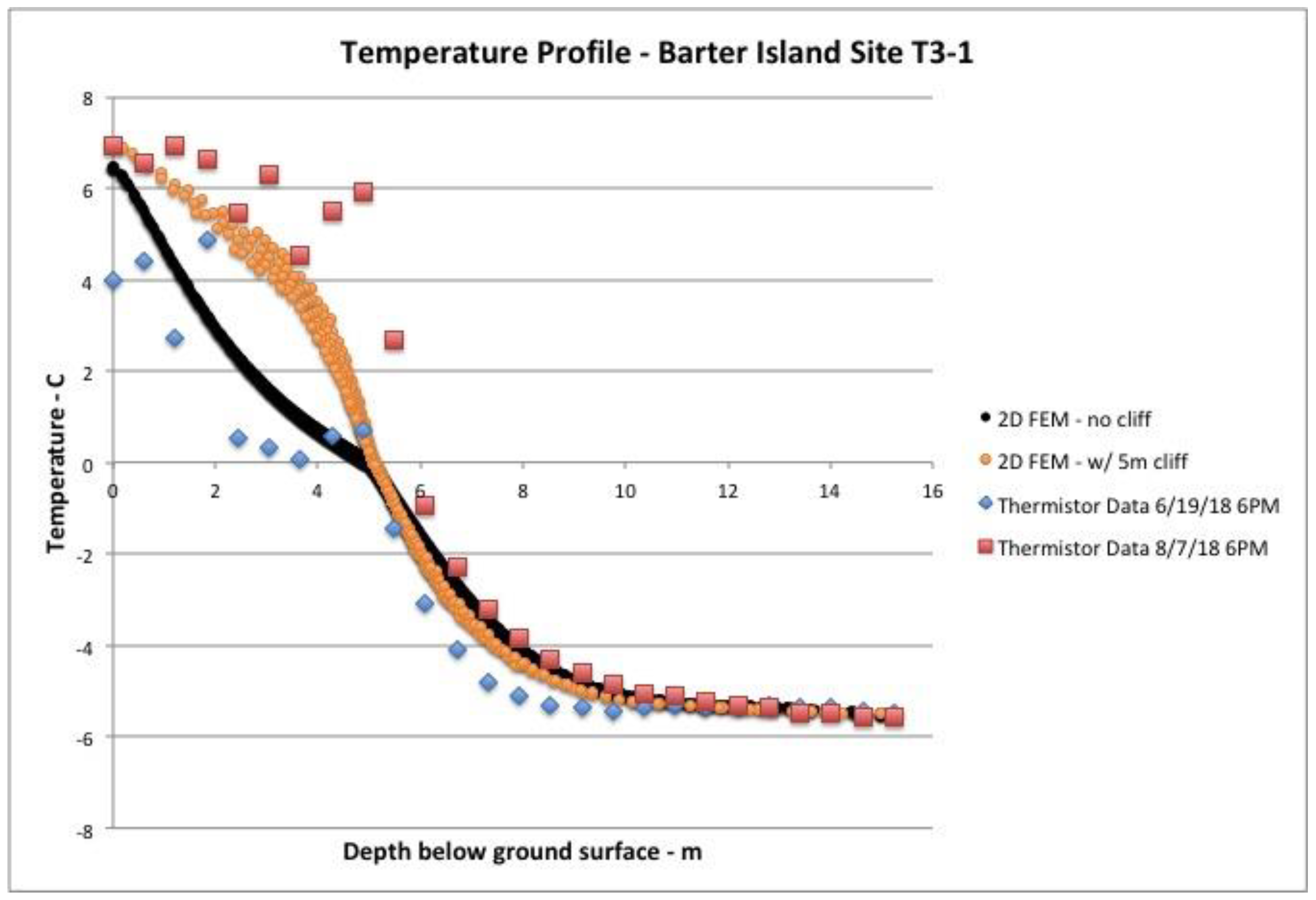
3.3. Check against 49 Days of Barter Island Data
| Ts = −6.33 − 13.5(2π((i Δt/86.4 × 103) + 170 − 26)/365), for model start at Julian day 170 |
3.4. ANSYS Comparison
4. Conclusions
- One-dimensional formulation of the models were checked and compared well with those presented in the reference used for the algorithm [16].
- One-dimensional and the two-dimensional version of the model were verified, with constant temperature boundary conditions, against the Neumann solution to the Stefan problem (a classic analytical solution to a simple two-phase phase change problem).
- Temperatures with depth agreed reasonably well, and thaw depths agreed to within a few percent at all times in simulations up to 60 days in length, or within 0.02 m, often an order of magnitude better.
- Thaw predictions of the moving mesh model agreed well with two borehole thermistor data sets collected for 15 days of from Barrow, and for 49 days from Barter Island.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- IPCC. Climate Change 2013: The Physical Science Basis. Contribution of Working Group I to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change; Stocker, T.F., Qin, D., Plattner, G.-K., Tignor, M., Allen, S., Boschung, J., Nauels, A., Xia, Y., Bex, V., Midgley, P., Eds.; Cambridge University Press: Cambridge, UK, 2013; 1535p. [Google Scholar] [CrossRef]
- Barnhart, K.R.; Anderson, R.S.; Overeem, I.; Wobus, C.; Clow, G.D.; Urban, F.E. Modeling erosion of ice-rich permafrost bluffs along the Alaskan Beaufort Seacoast. J. Geophys. Res. Earth Surf. 2014, 119, 1155–1179. [Google Scholar] [CrossRef]
- Zottola, J. Feasibility of Thermosyphons to Impede the Progress of Coastal Permafrost Erosion Along the Northern Coastline of Alaska. Master’s Thesis, University of Alaska, Anchorage, AK, USA, 2016. [Google Scholar]
- Reimnitz, E.; Kempema, E.; Barnes, P.W. Anchor ice, seabed freezing, and sediment dynamics in shallow Arctic Seas. J. Geophys. Res. Ocean. 1987, 92, 14671–14678. [Google Scholar] [CrossRef]
- Ravens, T.; Hailu, G.; Peng, J.; Ulmgren, M.; Wilber, M. Arctic-capable coastal geomorphic change modeling with application to Barter Island, Alaska. In Proceedings of the Oceans 17 Conference MTS/IEEE, Anchorage, AK, USA, 18–21 September 2017; Available online: http://www.oceans17mtsieeeanchorage.org (accessed on 28 September 2022).
- Reimnitz, E.; Graves, M.; Barnes, P.V. Beaufort Sea Coastal Erosion, Sediment Flux, Shoreline Evolution, and the Erosional Shelf Profile; Text to Accompany U.S. Geological Survey Series Map I-1182-G; Department of Tile Inferior, U.S. Geological Survey: Reston, VA, USA, 1988; 22p. Available online: https://pubs.usgs.gov/imap/1182g/report.pdf (accessed on 25 September 2022).
- Çengel, Y.A.; Ghajar, A.J. Heat and Mass Transfer, 5th ed.; McGraw Hill Education: New York, NY, USA, 2016. [Google Scholar]
- Moaveni, S. Finite Element Analysis: Theory and Application with ANSYS, 4th ed.; Pearson: London, UK, 2015. [Google Scholar]
- Voller, V.R. An Overview of Numerical Methods for Solving Phase Change Problems. In Advances in Numerical Heat Transfer; Minkowycz, W.J., Sparrow, E., Eds.; CRC Press: Boca Raton, FL, USA, 1996; Chapter 9; Volume 3. [Google Scholar]
- Huy, H.; Argyropoulos, S.A. Mathematical modeling of solidification and melting: A Review. Model. Simul. Mater. Sci. Eng. 1996, 4, 371–396. [Google Scholar] [CrossRef]
- Ling, F.; Zhang, T. Numerical simulation of permafrost thermal regime and talik development under shallow thaw lakes on the Alaskan Arctic Coastal Plain. J. Geophys. Res. 2003, 108, 4511. [Google Scholar] [CrossRef]
- Borisov, V. Mathematical Modeling of Underground Construction Temperature Influence on Permafrost Soils. Procedia Comput. Sci. 2015, 66, 112–121. [Google Scholar] [CrossRef]
- Liandi, F. Applications of the Finite-Element Method to the Problem of Heat Transfer in a Freezing Shaft Wall; CRREL Report 86-8; Cold Regions Research and Engineering Laboratory: Hanover, NH, USA, 1986; Available online: http://www.dtic.mil/dtic/tr/fulltext/u2/a172552.pdf (accessed on 25 September 2022).
- Darrow, M.M. Measurement of Temperature and Soil Properties for Finite Element Model Verification; Alaska Department of Transportation and Public Facilities and Alaska University Transportation Center: Juneau, AK, USA, 2010.
- Persson, P.; Strang, G. A simple mesh generator in MATLAB. SIAM Rev. 2004, 46, 329–345. [Google Scholar] [CrossRef]
- Mori, M. A finite element method for solving the two-phase Stefan problem. Publ. Res. Inst. Math. Sci. Ser. A 1977, 13, 723–753. [Google Scholar] [CrossRef]
- Beckett, G.; Mackenzie, J.; Robertson, M. A moving mesh finite element method for the solution of two-dimensional Stefan Problems. J. Comput. Phys. 2001, 168, 500–518. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Taylor, R.; Zhu, J. Chapter 6—Shape Functions, derivatives and integration. In The Finite Element Method: Its Basis and Fundamentals; Butterworth-Heinemann: Oxford, UK, 2013. [Google Scholar]

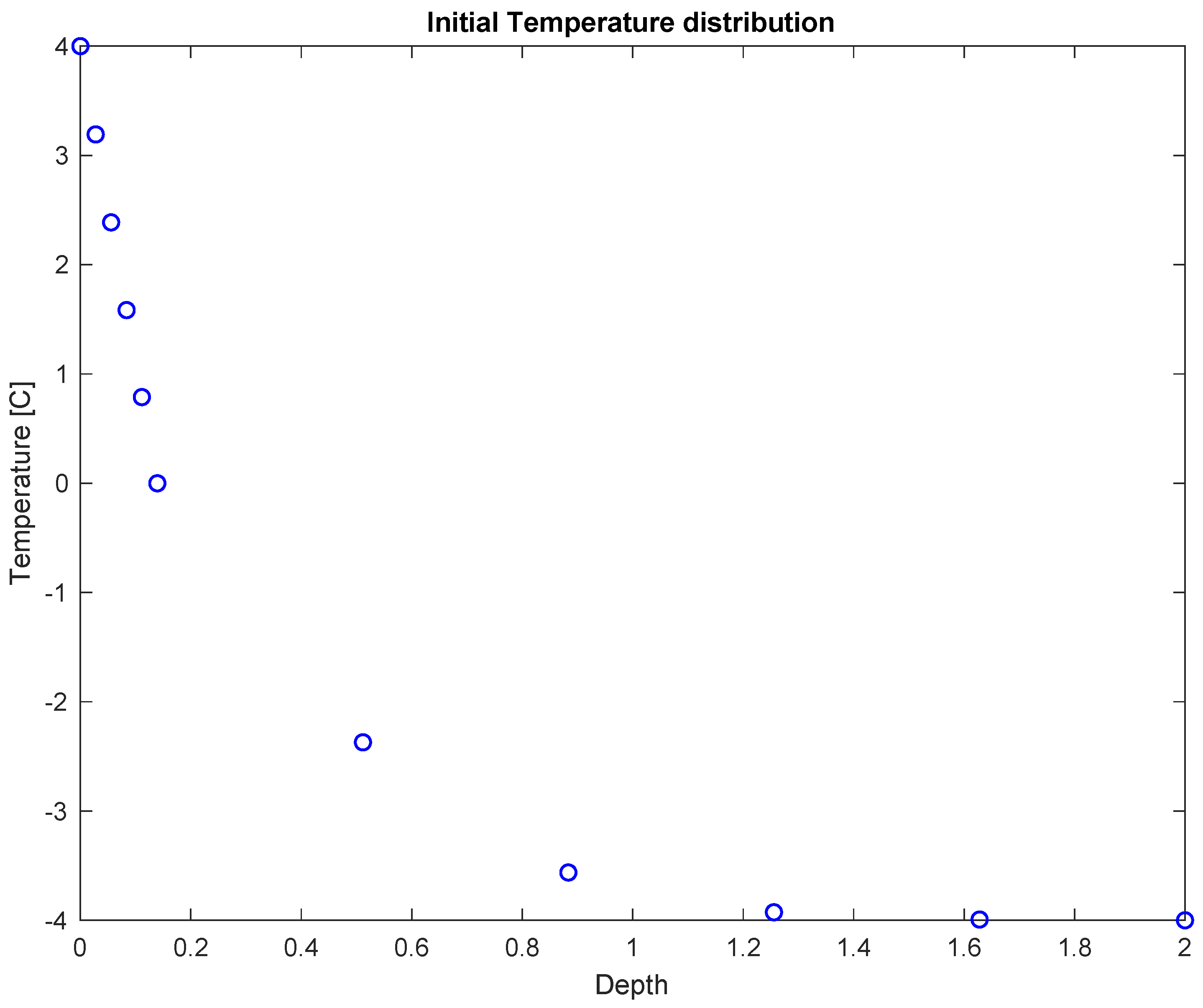




| Total length, | l = 2 m |
| Thermal conductivity of thawed soil, | k1 = 1.6 J/(s m C) |
| Thermal conductivity of frozen soil, | k2 = 1.2 J/(s m C) |
| Volumetric heat capacity of thawed soil, | c1 = 2.55 × 106 J/(m3C) |
| Volumetric heat capacity of frozen soil, | c2 = 2.35 × 106 J/(m3C) |
| Volumetric latent heat of water, | Lw = 3.34 × 108 J/m3 |
| Volumetric moisture content of soil, | W = 40% |
| Volumetric latent heat of soil, | L = W∗Lw |
| Phase change temperature, | Tm = 0 C |
| Boundary temperature at soil surface, | Ts = 4 C |
| Boundary temperature at bottom, | Tb = −4 C |
| Number of divisions in thawed and frozen regions, | n1 = n2 = 5 |
| Total time of numerical simulation, | t = 86,400 s |
| time step, | Δt = 0.1 s |
| Total horizontal dimension of model, | l = 4 m |
| Total depth of model, | d = 9 m |
| Node spacing at phase change boundary, | 0.05 m |
| Thermal conductivity of thawed soil, | k1 = 1.6 J/(s m C) |
| Thermal conductivity of frozen soil, | k2 = 1.2 J/(s m C) |
| Volumetric heat capacity of thawed soil, | c1 = 2.55 × 106 J/(m3C) |
| Volumetric heat capacity of frozen soil, | c2 = 2.35 × 106 J/(m3C) |
| Volumetric latent heat of water, | Lw = 3.34 × 108 J/m3 |
| Volumetric moisture content of soil, | W = 40% |
| Volumetric latent heat of soil, | L = W∗Lw |
| Phase change temperature, | Tm = 0 C |
| Boundary temperature at soil surface, | Ts = −6.8 − 13.5cos(2π((i Δt/86.4 × 103) + 165 − 26)/365) 1 |
| Boundary temperature at bottom, | Tb = −7.787 C |
| Total time of numerical simulation, | t = 15 days |
| time step, | Δt = 10 s |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wilber, M.; Hailu, G. A 2D Moving Mesh Finite Element Analysis of Heat Transfer in Arctic Soils. Thermo 2023, 3, 76-93. https://doi.org/10.3390/thermo3010005
Wilber M, Hailu G. A 2D Moving Mesh Finite Element Analysis of Heat Transfer in Arctic Soils. Thermo. 2023; 3(1):76-93. https://doi.org/10.3390/thermo3010005
Chicago/Turabian StyleWilber, Michelle, and Getu Hailu. 2023. "A 2D Moving Mesh Finite Element Analysis of Heat Transfer in Arctic Soils" Thermo 3, no. 1: 76-93. https://doi.org/10.3390/thermo3010005
APA StyleWilber, M., & Hailu, G. (2023). A 2D Moving Mesh Finite Element Analysis of Heat Transfer in Arctic Soils. Thermo, 3(1), 76-93. https://doi.org/10.3390/thermo3010005






