Study of Thermodynamic Modeling of Isothermal and Isobaric Binary Mixtures in Vapor-Liquid Equilibrium (VLE) of Tetrahydrofuran with Benzene (303.15 K) Cyclohexane (333.15 K), Methanol (103 kPa), and Ethanol (100 kPa)
Abstract
:1. Introduction
2. Methodology
2.1. Thermodynamic Databases
2.2. Theoretical Bases
2.2.1. Wilson Model
2.2.2. Non-Random Two Liquids (NRTL)
2.2.3. Universal Quasi-Chemical Model (UNIQUAC)
2.2.4. UNIQUAC Functional-Group Activity Coefficients (UNIFAC)
2.3. Thermodynamic Consistency of the Experimental Data
2.4. Correlation of VLE Data
3. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Isikgor, F.H.; Becer, C.R. Lignocellulosic Biomass: A Sustainable Platform for the Production of Bio-Based Chemicals and Polymers. Polym. Chem. 2015, 6, 4497–4559. [Google Scholar] [CrossRef] [Green Version]
- Fowles, J.; Boatman, R.; Bootman, J.; Lewis, C.; Morgott, D.; Rushton, E.; Van Rooij, J.; Banton, M. A Review of the Toxicological and Environmental Hazards and Risks of Tetrahydrofuran. Crit. Rev. Toxicol. 2013, 43, 811–828. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Jia, P.; Huang, D.; Lv, M.; Du, Y.; Li, W. Vapor−Liquid Equilibrium for Ternary and Binary Mixtures of Tetrahydrofuran, Cyclohexane, and 1,2-Propanediol at 101.3 kPa. J. Chem. Eng. Data 2013, 58, 3054–3060. [Google Scholar] [CrossRef]
- Parveen, S.; Singh, S.; Shukla, D.; Singh, K.P.; Gupta, M.; Shukla, J.P. Molecular Interaction Study of Binary Mixtures of THF with Methanol and o-Cresol—An Optical and Ultrasonic Study. Acta Phys. Polonica A 2009, 116, 1011–1017. [Google Scholar] [CrossRef]
- Scharzec, B.; Merschhoff, D.; Henrichs, J.; Kappert, E.J.; Skiborowski, M. Evaluation of membrane-assisted hybrid processes for the separation of a tetrahydrofuran-methanol-water mixture. Chem. Eng. Process. 2021, 167, 108545. [Google Scholar] [CrossRef]
- Nain, A.K. Densities and Volumetric Properties of Binary Mixtures of Tetrahydrofuran with Some Aromatic Hydrocarbons at Temperatures from 278.15 to 318.15 K. J. Solution Chem. 2006, 35, 1417–1439. [Google Scholar] [CrossRef]
- Ohmura, S.; Ueno, M.; Miyoshi, N. Strontium-mediated selective protonation of unsaturated linkage of aromatic hydrocarbons and these derivatives. Tetrahedron Lett. 2018, 59, 2268–2271. [Google Scholar] [CrossRef]
- Sharifian, H.; Park, S. Studies on electro-reduction mechanisms of selected aromatic hydrocarbons in tetrahydrofuran. J. Electroanal. Chem. 1983, 143, 337–351. [Google Scholar] [CrossRef]
- Global Tetrahydrofuran Market Size, Share|Industry Report 2027. Available online: https://marketresearch.biz/report/tetrahydrofuran-market/ (accessed on 19 May 2021).
- Available online: https://www.veritradecorp.com/en/COLOMBIA/imports-and-exports/tetrahydrofuran/293211 (accessed on 16 September 2021).
- Jaber, Y.; Shojaeian, A. Vapor-Liquid (Azeotropic Systems) and Liquid-Liquid Equilibrium Calculations Using UNIFAC and NRTL-SAC Activity Coefficient Models. Fluid Phase Equilibria 2019, 494, 33–44. [Google Scholar]
- Vapor-Liquid Equilibrium Data of Benzene + Tetrahydrofuran from Dortmund Data Bank. Available online: http://www.ddbst.com/en/EED/VLE/VLE%20Benzene%3BTetrahydrofuran.php (accessed on 19 May 2021).
- Binary Vapor-Liquid Equil. Data. Available online: https://www.cheric.org/research/kdb/hcvle/showvle.php?vleid=3848 (accessed on 27 August 2021).
- Li, Q.; Liu, P.; Cao, L.; Wen, F.; Zhang, S.; Wang, B. Vapor-Liquid Equilibrium for Tetrahydrofuran+methanol+tetrafluoroborate-Based Ionic Liquids at 101.3kPa. Fluid Phase Equilibria 2013, 360, 439–444. [Google Scholar] [CrossRef]
- Binary Vapor-Liquid Equil. Available online: https://www.cheric.org/research/kdb/hcvle/showvle.php?vleid=2376 (accessed on 19 September 2021).
- Villa, S.; Garriga, R.; Pérez, P.; Gracia, M.; González, J.A.; de la Fuente, I.G.; Cobos, J.C. Thermodynamics of mixtures with strongly negative deviations from Raoult’s law Part 9. Vapor–liquid equilibria for the system 1-propanol + di-n-propylamine at six temperatures between 293.15 and 318.15 K. Fluid Phase Equilibria 2006, 231, 211–220. [Google Scholar] [CrossRef]
- Wilson, G.M. Vapor-Liquid Equilibrium. XI. A New Expression for the Excess Free Energy of Mixing. J. Am. Chem. Soc. 1964, 86, 127–130. [Google Scholar] [CrossRef]
- Renon, H.; Prausnitz, J.M. Local Compositions in Thermodynamic Excess Functions for Liquid Mixtures. AICHE J. 1968, 14, 135–144. [Google Scholar] [CrossRef]
- Abrams, D.S.; Prausnitz, J.M. Statistical Thermodynamics of Liquid Mixtures: A New Expression for the Excess Gibbs Energy of Partly or Completely Miscible Systems. AIChE J. 1975, 21, 116–128. [Google Scholar] [CrossRef]
- Poling, B.E.; Prausnitz, J.M.; O’Connell, J.P. The Properties of Gases and Liquids, 5th ed.; McGrawHill: New York, NY, USA, 2001. [Google Scholar]
- Fredenslund, A.; Jones, R.L.; Prausnitz, J.M. Group-contribution Estimation of Activity Coefficients in Nonideal Liquid Mixtures. AIChE J. 1975, 21, 1086–1099. [Google Scholar] [CrossRef]
- Jackson, P.; Wilsak, R. Thermodynamic consistency tests based on the Gibbs-Duhem equation applied to isothermal, binary vapor-liquid equilibrium data: Data evaluation and model testing. Fluid Phase Equilibria 1995, 103, 155–197. [Google Scholar] [CrossRef]
- Herington, E.F. Test for the Consistency of Experimental Isobaric Vapor-liquid Equilibrium Data. J. Znst. Pet. 1961, 37, 457–470. [Google Scholar]
- Available online: https://trc.nist.gov/TDE/Help/TDE103b/ExptAndPredictedData-Binary.htm (accessed on 16 September 2021).
- Wisniak, J.; Ortega, J.; Fernández, L. A Fresh Look at the Thermodynamic Consistency of Vapour-Liquid Equilibria Data. J. Chem. Thermodyn. 2017, 105, 385–395. [Google Scholar] [CrossRef]
- Revellin, R.; Lips, S.; Neveu, P.; Bonjour, J. A comprehensive non-equilibrium thermodynamic analysis applied to a vapor–liquid two-phase flow of a pure fluid. Int. J. Multiph. Flow 2012, 42, 184–193. [Google Scholar] [CrossRef]
- Versaci, M.; Palumbo, A. Magnetorheological Fluids: Qualitative comparison between a mixture model in the Extended Irreversible Thermodynamics framework and an Herschel–Bulkley experimental elastoviscoplastic model. Int. J. Non-Linear Mech. 2020, 118, 103288. [Google Scholar] [CrossRef]
- Chen, K.C.; Yeh, C.S. A mixture model for magneto-rheological materials. Contin. Mech. Thermodyn. 2002, 15, 495–510. [Google Scholar] [CrossRef]
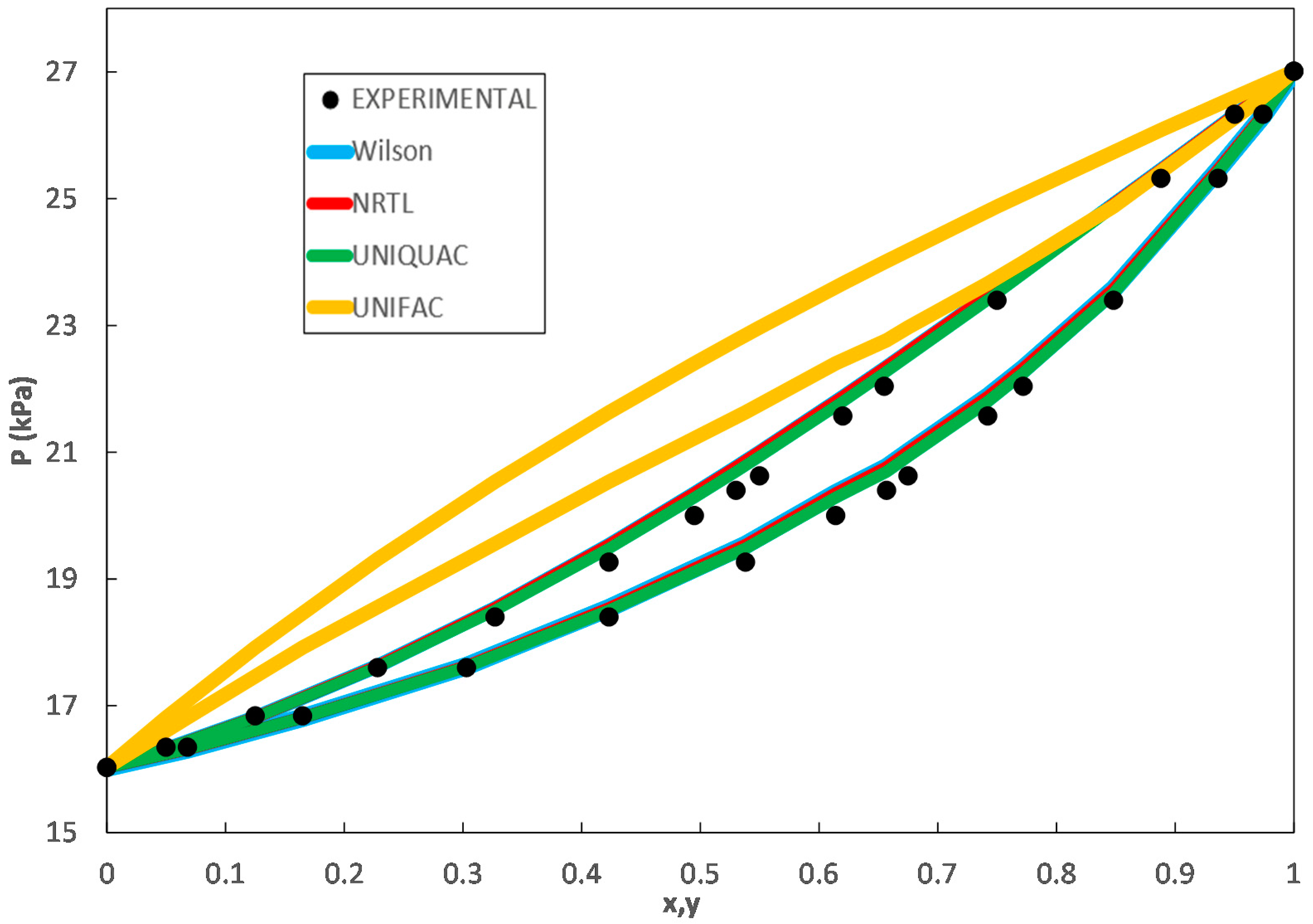

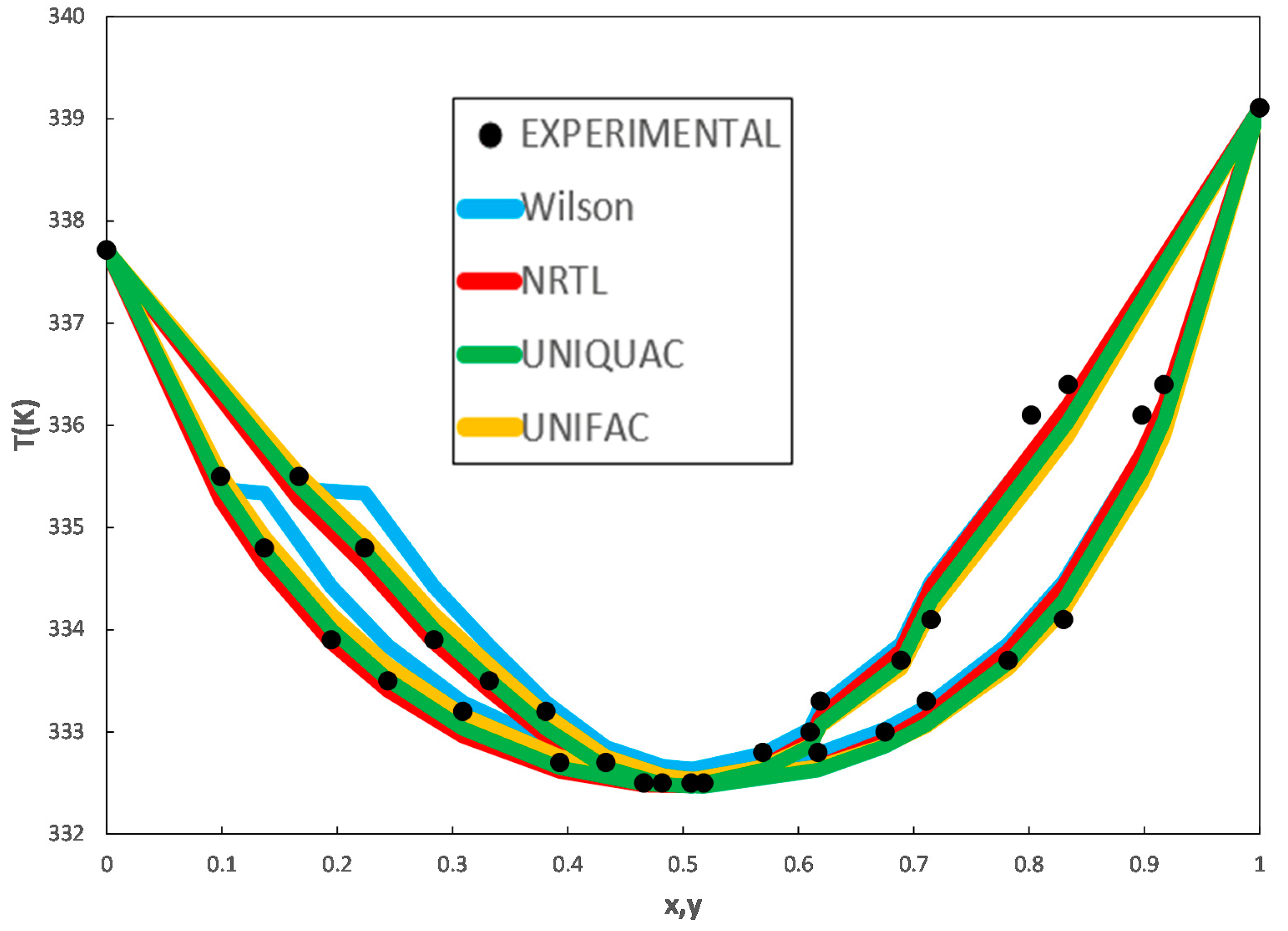
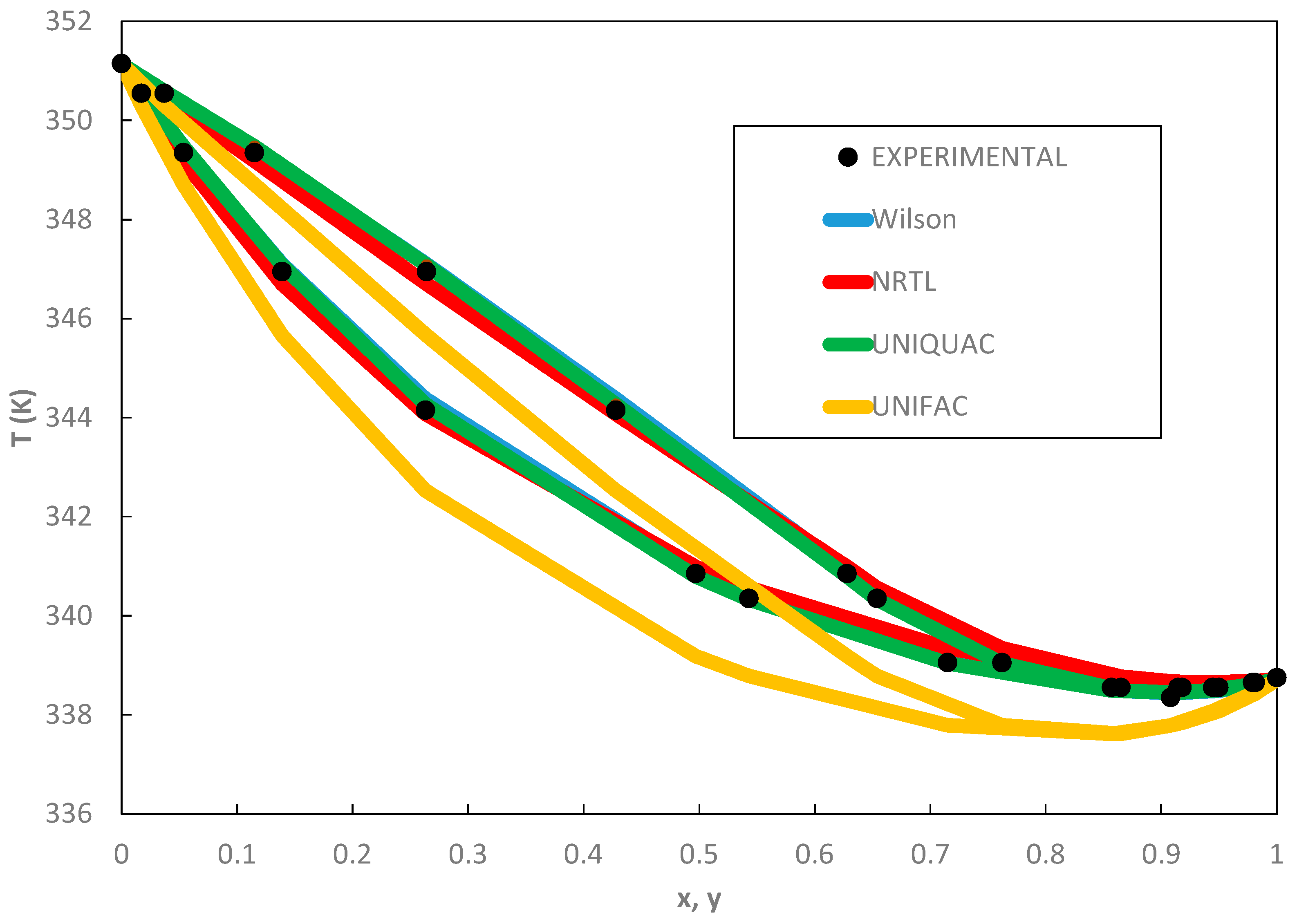

| Binary System | Type | Reference |
|---|---|---|
| THF(1) + Benzene(2) | isothermal | [12] |
| THF(1) + Cyclohexane(2) | Isothermal | [13] |
| THF(1) + Methanol(2) | Isobaric | [14] |
| THF(1) + Ethanol(2) | Isobaric | [15] |
| Component | ri | qi | Reference |
|---|---|---|---|
| Tetrahydrofuran | 2.9415 | 2.72 | [20] |
| Cyclohexane | 4.0464 | 3.24 | [20] |
| Benzene | 3.1878 | 2.4 | [20] |
| Methanol | 1.43 | 0.96 | [20] |
| Ethanol | 2.588 | 0.92 | [20] |
| Component | r | q | Reference |
|---|---|---|---|
| Tetrahydrofuran | 2.9415 | 2.72 | [18] |
| Cyclohexane | 4.0464 | 3.24 | [18] |
| Benzene | 3.1878 | 2.4 | [18] |
| Methanol | 1.43 | 1.432 | [18] |
| Ethanol | 2.588 | 2.588 | [18] |
| System | D | Consistency |
|---|---|---|
| THF(1) + Benzene(2) | 20.62 | (−) |
| THF(1) + cyclohexane(2) | 3.32 | (+) |
| System | Consistency | |
|---|---|---|
| THF(1) + Methanol(2) | 3.440 1.6806 | (+) |
| THF(1) + Ethanol(2) | 7.6011 2.1925 | (+) |
| Model | Aij (J/mol) | Aji (J/mol) | αij |
|---|---|---|---|
| THF(1) + Benzene(2) | |||
| Wilson | 0.9699 | 1.2618 | |
| NRTL | −938.057 | 386.177 | 0.3 |
| UNIQUAC | −8.2597 | −50.7358 | |
| THF(1) + Cyclohexane(2) | |||
| Wilson | 0.7778 | 0.7197 | |
| NRTL | 954.65 | 628.29 | 0.3 |
| UNIQUAC | −99.129 | 174.79 | |
| THF(1) + Methanol(2) | |||
| Wilson | 0.6960 | 0.5356 | |
| NRTLUNIQUAC | 1154.3955340 | 1423.46–135.25 | 0.3 |
| THF(1) + Ethanol(2) | 0.3 | ||
| Wilson | 1.1022 | 0.4420 | |
| NRTL | 767.15 | 933.81 | |
| UNIQUAC | 579.22 | −232.99 | |
| Model | ||
|---|---|---|
| THF(1) + Benzene(2) | ||
| Wilson | 0.2335 | 0.0112 |
| NRTL | 0.2334 | 0.012 |
| UNIQUAC | 0.1854 | 0.0121 |
| UNIFAC | 1.807 | 0.0633 |
| THF(1) + Cyclohexane(2) | ||
| Wilson | 0.5227 | 0.0076 |
| NRTL | 0.5257 | 0.0077 |
| UNIQUAC | 0.2048 | 0.0076 |
| UNIFAC | 1.4562 | 0.0363 |
| Model | ||
|---|---|---|
| THF(1) + Methanol(2) | ||
| Wilson | 0.2739 | 0.0088 |
| NRTL | 0.1823 | 0.0077 |
| UNIQUAC | 0.2482 | 0.0089 |
| UNIFAC | 0.1998 | 0.0109 |
| THF(1) + Ethanol(2) | ||
| Wilson | 0.1011 | 0.005 |
| NRTL | 0.1894 | 0.0069 |
| UNIQUAC | 0.081 | 0.0053 |
| UNIFAC | 1.0637 | 0.0302 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fernández, L.S.R.; Amado-Gonzaléz, E.; Hurtado, E.G.Y. Study of Thermodynamic Modeling of Isothermal and Isobaric Binary Mixtures in Vapor-Liquid Equilibrium (VLE) of Tetrahydrofuran with Benzene (303.15 K) Cyclohexane (333.15 K), Methanol (103 kPa), and Ethanol (100 kPa). Thermo 2021, 1, 286-296. https://doi.org/10.3390/thermo1030019
Fernández LSR, Amado-Gonzaléz E, Hurtado EGY. Study of Thermodynamic Modeling of Isothermal and Isobaric Binary Mixtures in Vapor-Liquid Equilibrium (VLE) of Tetrahydrofuran with Benzene (303.15 K) Cyclohexane (333.15 K), Methanol (103 kPa), and Ethanol (100 kPa). Thermo. 2021; 1(3):286-296. https://doi.org/10.3390/thermo1030019
Chicago/Turabian StyleFernández, Leonardo Steyman Reyes, Eliseo Amado-Gonzaléz, and Erik Germán Yanza Hurtado. 2021. "Study of Thermodynamic Modeling of Isothermal and Isobaric Binary Mixtures in Vapor-Liquid Equilibrium (VLE) of Tetrahydrofuran with Benzene (303.15 K) Cyclohexane (333.15 K), Methanol (103 kPa), and Ethanol (100 kPa)" Thermo 1, no. 3: 286-296. https://doi.org/10.3390/thermo1030019
APA StyleFernández, L. S. R., Amado-Gonzaléz, E., & Hurtado, E. G. Y. (2021). Study of Thermodynamic Modeling of Isothermal and Isobaric Binary Mixtures in Vapor-Liquid Equilibrium (VLE) of Tetrahydrofuran with Benzene (303.15 K) Cyclohexane (333.15 K), Methanol (103 kPa), and Ethanol (100 kPa). Thermo, 1(3), 286-296. https://doi.org/10.3390/thermo1030019






