Characterizing the Material Properties of the Kidney and Liver in Unconfined Compression and Probing Protocols with Special Reference to Varying Strain Rate
Abstract
1. Introduction
2. Materials and Methods
2.1. Specimens
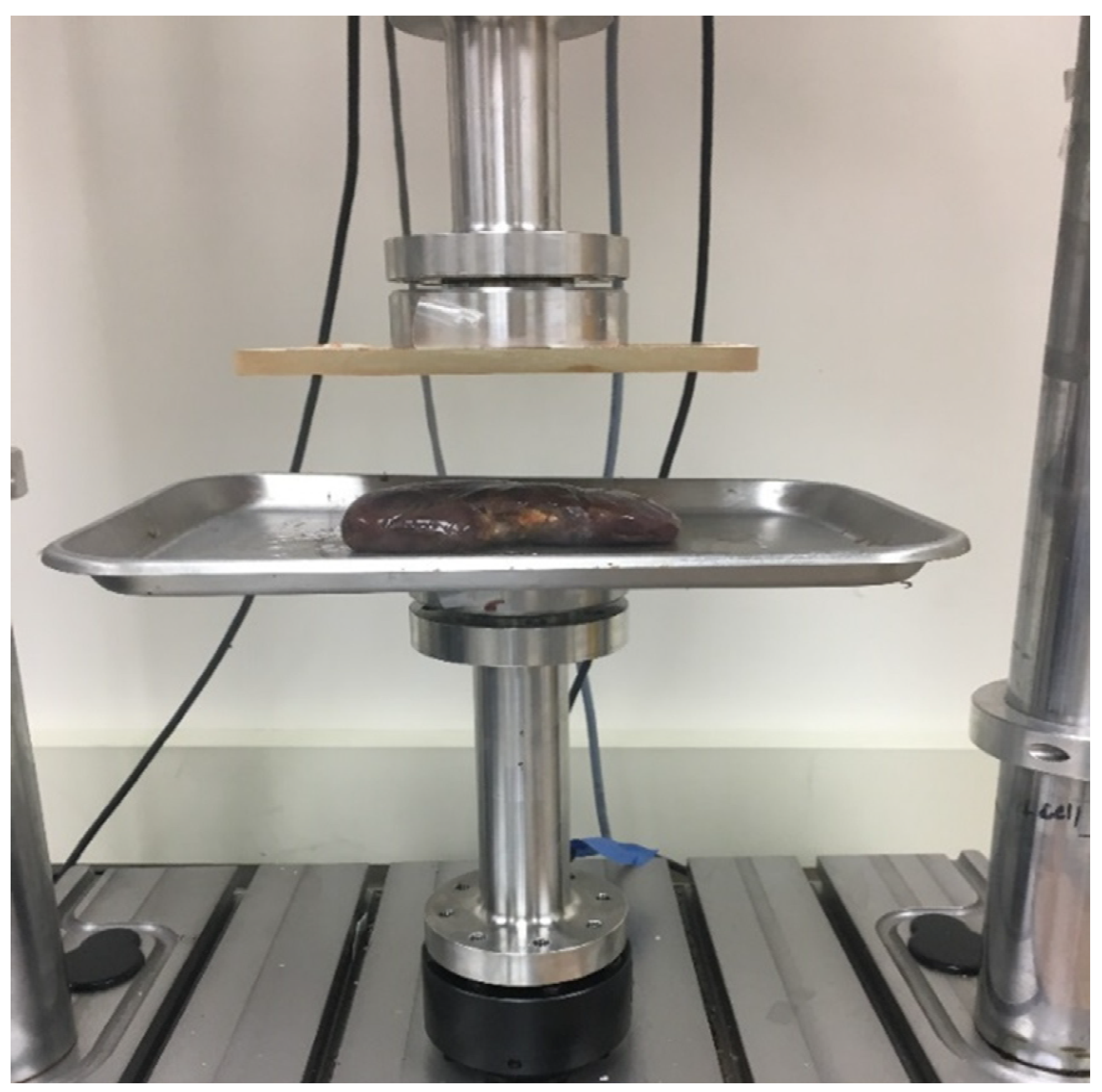
2.2. Material Testing Devices
2.3. Experimental Protocol
2.4. Data Analysis
3. Results
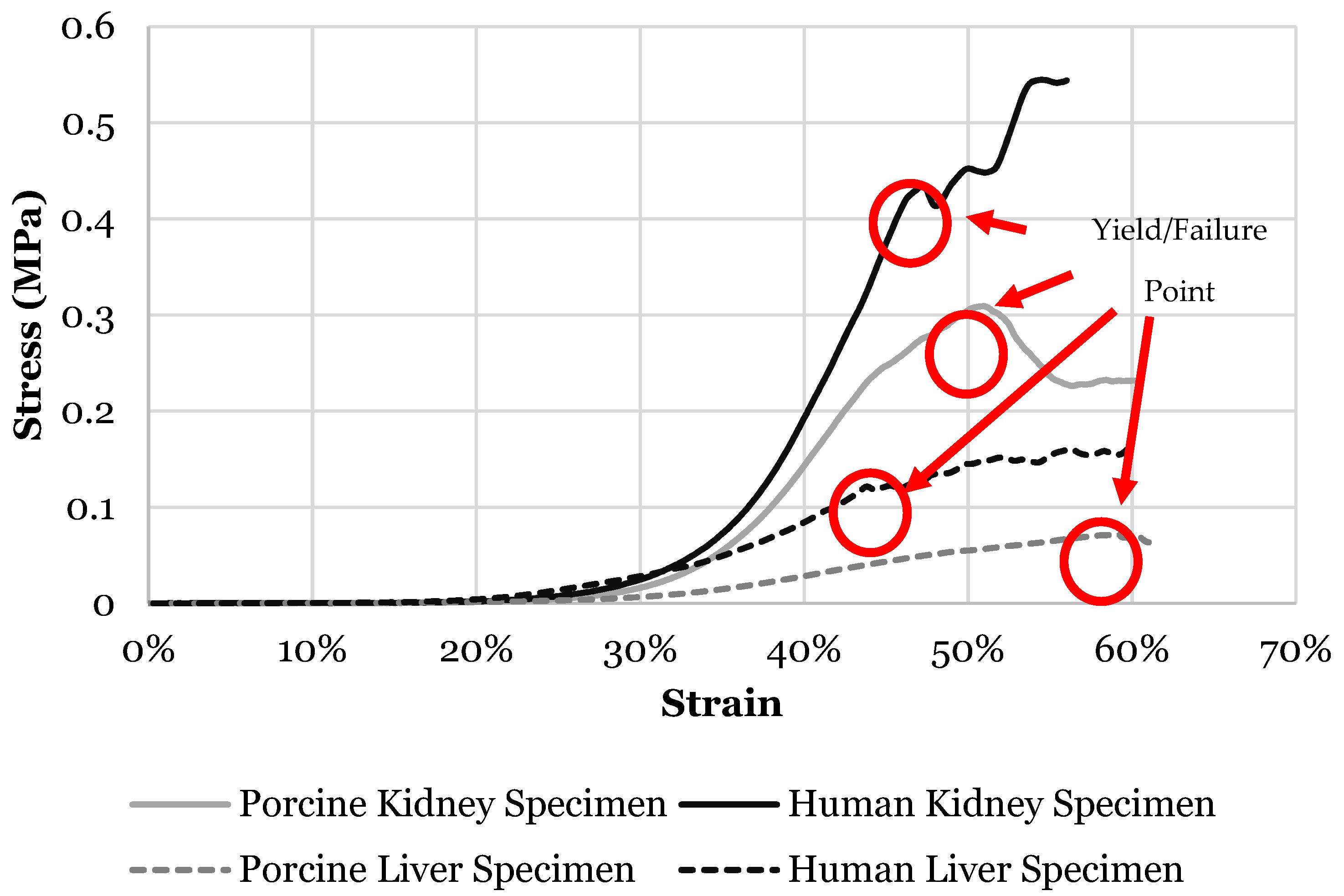
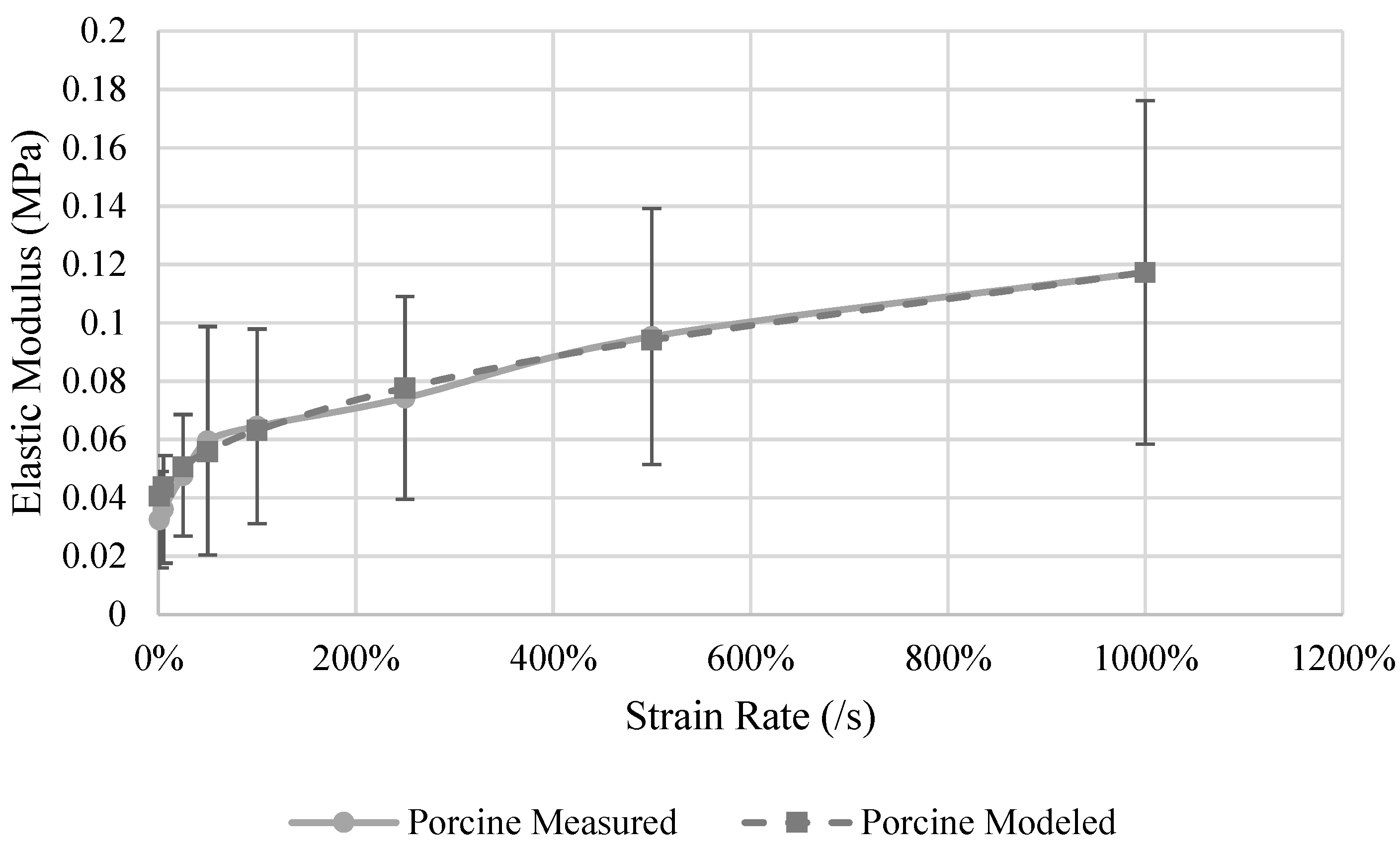
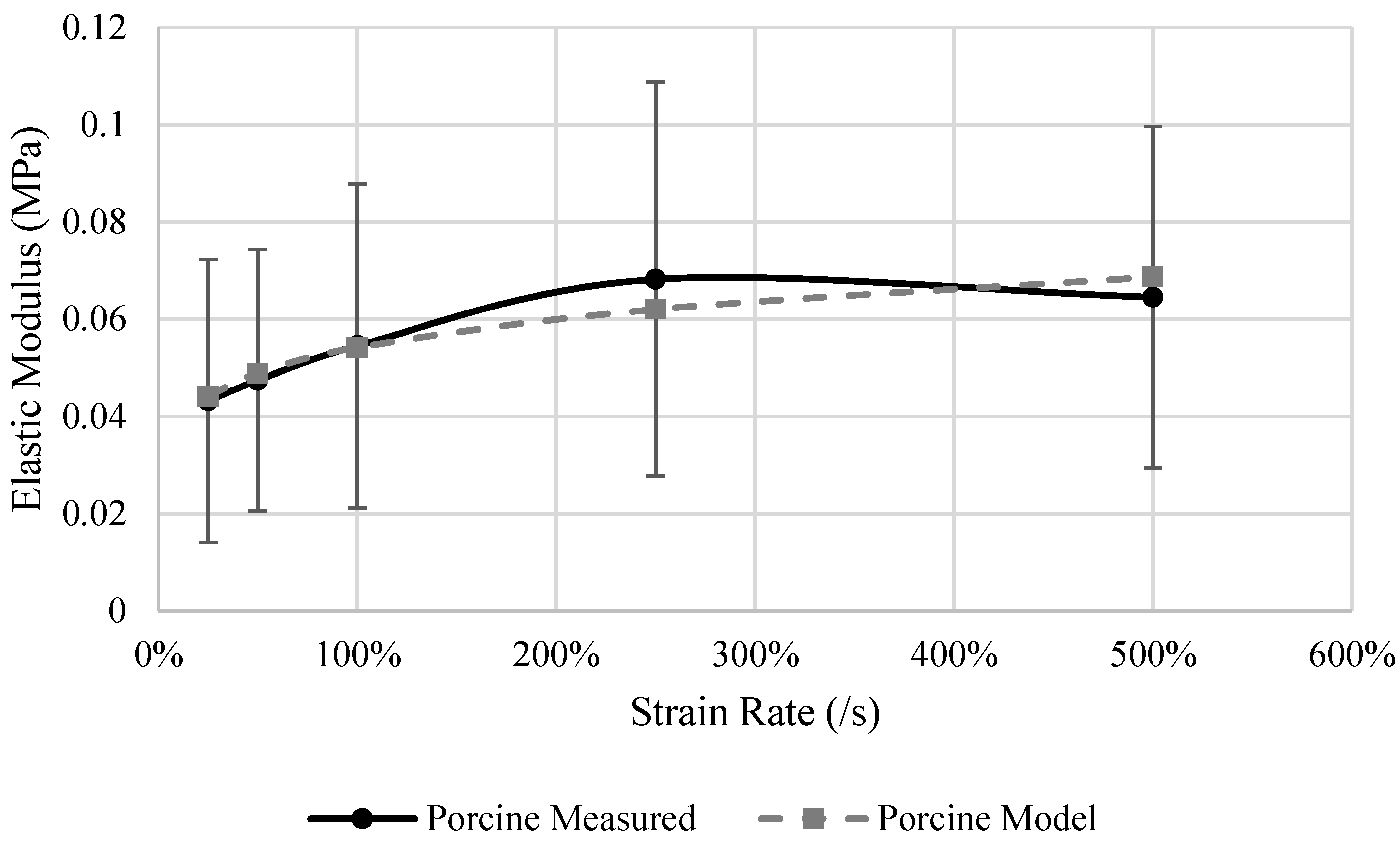
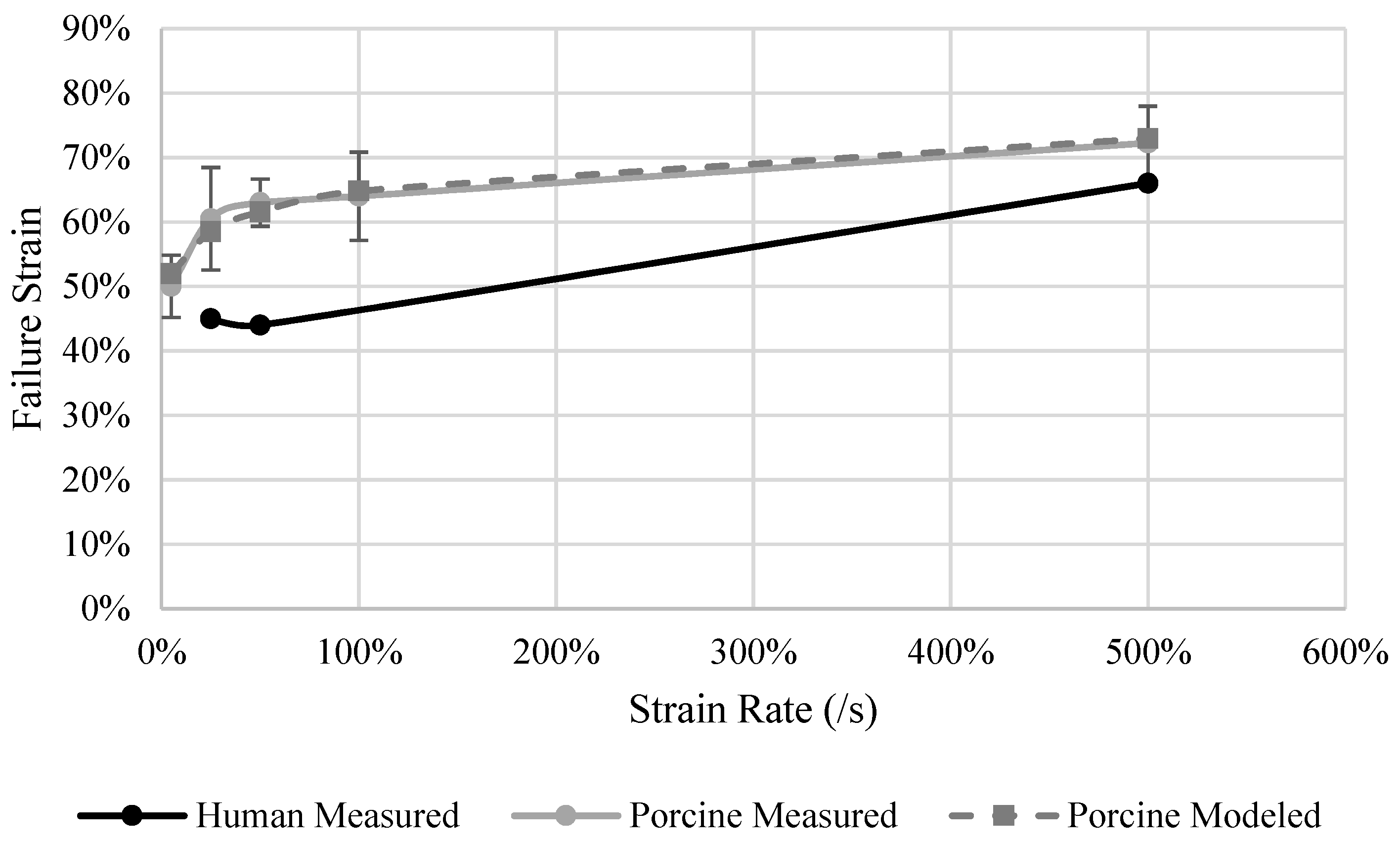
3.1. Kidney
3.2. Liver
4. Discussion
4.1. Kidney
4.2. Liver
5. Conclusions
- Kidney modulus and failure stress are dependent on the strain rate while failure strain was largely independent of strain rate. The strain rate dependence saturated at rates greater than 100%/s.
- Kidney modulus measured using whole organ testing was approximately twice as stiff as previously reported for kidney parenchyma specimens. Caution must be exercised when using material parameters derived from partial kidney specimens.
- Kidney modulus measured using the probing protocol was larger than under unconfined compression for both hosts. Therefore the results from two testing methods are not interchangeable.
- Porcine kidney was found to be four times stiffer than human kidney tissue and therefore the elastic modulus of porcine kidney cannot be used for human tissue modeling. Nevertheless, the failure stress and failure strain of the kidney from both hosts was found to be similar.
- Liver modulus, failure stress, and failure strain are dependent on the strain rate. Failure strain dependence on rate saturated at rates greater than 50%/s.
- Liver modulus measured using whole organ testing was much larger than previously reported for liver parenchyma specimens. Caution must be exercised when using material parameters derived from partial liver specimens.
- Liver modulus measured using the probing protocol was four times larger than under unconfined compression for the human specimens and twice as large for porcine livers. Therefore the results from two testing methods are not interchangeable.
- Porcine liver mechanical properties were found to be an adequate substitute for human kidney properties for elastic modulus and failure strain, but not for failure stress.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Notations and Abbreviations
| E | Elastic modulus |
| Eterm | Elastic modulus of terminal region |
| Etoe | Elastic modulus of toe region |
| ε | Engineering strain |
| εc | Center strain of inflection point |
| εf | Failure strain |
| Strain rate | |
| σ | Stress |
| σf | Failure stress |
| σND | Stress for non-destructive methodology |
| σP | Stress for probing methodology |
| ψ | Parameter describing the curvature of inflection region |
References
- Klinich, K.; Flannagan, C.; Nicholson, K.; Schneider, L.; Rupp, J. Abdominal Injury in Motor Vehicle Crashes; UMTRI-2008-40; University of Michigan: Ann Arbor, MI, USA, 2008. [Google Scholar]
- Asensio, J.; Trunkey, D. Current Therapy of Trauma and Surgical Critical Care E-Book; Elsevier Health Sciences: Amsterdam, The Netherlands, 2008. [Google Scholar]
- Chauhan, N.; Badgurjar, M.K.; Saxena, P. A Prospective Study of Assessment of Solid Organs in Cases of Blunt Abdominal Trauma. Int. J. Sci. Res. 2019, 8, 48–50. [Google Scholar]
- Loftis, K.; Edward, L.; Mazuchowski, L.; Clouser, M.; Gillich, P. Prominent Injury Types in Vehicle Underbody Blast. Mil. Med. 2019, 184, 261–264. [Google Scholar] [CrossRef]
- Umale, S.; Deck, C.; Bourdet, N.; Dhumane, P.; Soler, L.; Marescaux, J.; Willinger, R. Experimental Mechanical Characterization of Abdominal Organs: Liver, Kidney & Spleen. J. Mech. Behav. Biomed. Mater. 2013, 17, 22–33. [Google Scholar]
- Lizee, E.; Robin, S.; Song, E.; Bertholon, N.; Le Coz, J.-Y.; Besnault, B.; Lavaste, F. Development of a 3D Finite Element Model of the Human Body. SAE Trans. 1998, 107, 2760–2782. [Google Scholar]
- Bass, C.; Davis, M.; Rafaels, K.; Rountree, M.; Harris, R.; Sanderson, E.; Andrefsky, W.; DiMarco, G.; Zielinski, M. A Methodology for Assessing Blast Protection in Explosive Ordnance Disposal Bomb Suits. Int. J. Occup. Saf. Ergon. 2005, 11, 347–361. [Google Scholar] [CrossRef]
- Roberts, J.; Merkle, A.; Biermann, P.; Ward, E.; Carkhuff, B.; Cain, R.; O’Connor, J. Computational and Experimental Models of the Human Torso for Non-Penetrating Ballistic Impact. J. Biomech. 2007, 40, 125–136. [Google Scholar] [CrossRef] [PubMed]
- Ward, E.; Merkle, A.; Harrigan, T.; Roberts, J. Comparing Blast Effects on Human Torso Finite Element Model Against Existing Lethality Curves. In Proceedings of the Department of Defense Explosive Safety Board Seminar, Portland, OR, USA, 13–15 July 2010; Volume 34. [Google Scholar]
- Chen, Z.; Joli, P.; Feng, Z.-Q. Finite Element Modeling of Interactions Between Pelvic Organs Due to Pressure. In Proceedings of the 10e Colloque National En Calcul Des Structures, Giens, France, 9–13 May 2011. [Google Scholar]
- Gayzik, F.; Moreno, D.; Vavalle, N.; Rhyne, A.; Stitzel, J. Development of the Global Human Body Models Consortium Mid-Sized Male Full Body Model. In International Workshop on Human Subjects for Biomechanical Research; National Highway Traffic Safety Administration: Washington, DC, USA, 2011; Volume 39. [Google Scholar]
- Beillas, P.; Berthet, F. Performance of a 50th Percentile Abdominal Model for Impact: Effects of Size and Mass. J. Biomech. 2012, 45, S83. [Google Scholar] [CrossRef]
- Golman, A.; Danelson, K.; Miller, L.; Stitzel, J. Injury Prediction in a Side Impact Crash Using Human Body Model Simulation. Accid. Anal. Prev. 2014, 64, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Schwartz, D.; Guleyupoglu, B.; Koya, B.; Stitzel, J.; Gayzik, F. Development of a Computationally Efficient Full Human Body Finite Element Model. Traffic Inj. Prev. 2015, 16, S49–S56. [Google Scholar] [CrossRef]
- Tamura, A.; Omori, K.; Miki, K.; Lee, J.; Yang, K.; King, A. Mechanical Characterization of Porcine Abdominal Organs; SAE Technical Paper, No. 2002-22-0003; SAE: Warrendale, PA, USA, 2002. [Google Scholar]
- Kemper, A.; Santago, A.; Stitzel, J.; Sparks, J.; Duma, M. Biomechanical Response of Human Spleen in Tensile Loading. J. Biomech. 2012, 45, 348–355. [Google Scholar] [CrossRef] [PubMed]
- Johnson, B.; Campbell, S.; Campbell-Kyureghyan, N. The Differences in Measured Prostate Material Properties Between Probing and Unconfined Compression Testing Methods. Med Eng. Phys. 2020, 80, 44–51. [Google Scholar] [CrossRef] [PubMed]
- Johnson, B.; Campbell, S.; Campbell-Kyureghyan, N. Biomechanical Properties of Abdominal Organs Under Tension with Special Reference to Increasing Strain Rate. J. Biomech. 2020, 109, 109914. [Google Scholar] [CrossRef]
- Roberts, J.; O’Connor, J.; Ward, E. Modeling the Effect of Nonpenetrating Ballistic Impact as a Means of Detecting Behind-Armor Blunt Trauma. J. Trauma Acute Care Surg. 2005, 58, 1241–1251. [Google Scholar] [CrossRef]
- Hollenstein, M.; Nava, A.; Valtorta, D.; Snedeker, J.; Mazza, E. Mechanical Characterization of the Liver Capsule and Parenchyma. In International Symposium on Biomedical Simulation; Springer: Berlin/Heidelberg, Germany, 2006; pp. 150–158. [Google Scholar]
- Chui, C.; Kobayashi, E.; Chen, X.; Hisada, T.; Sakuma, I. Transversely Isotropic Properties of Porcine Liver Tissue: Experiments and Constitutive Modelling. Med Biol. Eng. Comput. 2007, 45, 99–106. [Google Scholar] [CrossRef] [PubMed]
- Karimi, A.; Shojaei, A. Measurement of the Mechanical Properties of the Human Kidney. IRBM 2017, 38, 292–297. [Google Scholar] [CrossRef]
- Rosen, J.; Brown, J.; De, S.; Sinanan, M.; Hannaford, B. Biomechanical Properties of Abdominal Organs in Vivo and Postmortem Under Compression Loads. J. Biomech. Eng. 2008, 130, 021020. [Google Scholar] [CrossRef] [PubMed]
- Santago, A.; Kemper, A.; McNally, C.; Sparks, J.; Duma, S. The Effect of Temperature on the Mechanical Properties of Bovine Liver. Biomed. Sci. Instrum. 2009, 45, 376–381. [Google Scholar]
- Brunon, A.; Bruyere-Garnier, K.; Coret, M. Mechanical Characterization of Liver Capsule Through Uniaxial Quasi-Static Tensile Tests Until Failure. J. Biomech. 2010, 43, 2221–2227. [Google Scholar] [CrossRef]
- Gao, Z.; Lister, K.; Desai, J. Constitutive Modeling of Liver Tissue: Experiment and Theory. Ann. Biomed. Eng. 2010, 38, 505–516. [Google Scholar] [CrossRef]
- Kemper, A.; Santago, A.; Stitzel, J.; Sparks, J.; Duma, S. Biomechanical Response of Human Liver in Tensile Loading. In Annals of Advances in Automotive Medicine/Annual Scientific Conference; Association for the Advancement of Automotive Medicine: Chicago, IL, USA, 2010; Volume 54, p. 15. [Google Scholar]
- Chatelin, S.; Oudry, J.; Perichon, N.; Sandrin, L.; Allemann, P.; Soler, L.; Willinger, R. In Vivo Liver Tissue Mechanical Properties by Transient Elastography: Comparison with Dynamic Mechanical Analysis. Biorheology 2011, 48, 75–88. [Google Scholar] [CrossRef]
- Lu, Y.-C.; Untaroiu, C. Effect of Storage Methods on Indentation-Based Material Properties of Abdominal Organs. Proc. Inst. Mech. Eng. Part H 2013, 227, 293–301. [Google Scholar] [CrossRef]
- Lu, Y.-C.; Kemper, A.; Untaroiu, C. Effect of Storage on Tensile Material Properties of Bovine Liver. J. Mech. Behav. Biomed. Mater. 2014, 29, 339–349. [Google Scholar] [CrossRef]
- Miller, K. Constitutive Modelling of Abdominal Organs. J. Biomech. 2000, 33, 367–373. [Google Scholar] [CrossRef]
- Carter, F.J.; Frank, T.G.; Davies, P.J.; McLean, D.; Cuschieri, A. Measurements and Modelling of the Compliance of Human and Porcine Organs. Medical Image Anal. 2001, 5, 231–236. [Google Scholar] [CrossRef]
- Dương, M.; Huynh Nguyễn, N.; Ngọc Trần, T.; Tolba, R.H.; Staat, M. Influence of Refrigerated Storage on Tensile Mechanical Properties of Porcine Liver and Spleen. Int. Biomech. 2015, 2, 79–88. [Google Scholar] [CrossRef][Green Version]
- Snedeker, J.; Niederer, P.; Schmidlin, F.R.; Farshad, M.; Demetropoulos, C.K.; Lee, J.B.; Yang, K.H. Strain-Rate Dependent Material Properties of the Porcine and Human Kidney Capsule. J. Biomech. 2005, 38, 1011–1021. [Google Scholar] [CrossRef] [PubMed]
- Roan, E.; Vemaganti, K. The Nonlinear Material Properties of Liver Tissue Determined From No-Slip Uniaxial Compression Experiments. J. Biomech. Eng. 2007, 129, 450–456. [Google Scholar] [CrossRef] [PubMed]
- Saraf, H.; Ramesh, K.; Lennon, A.; Merkle, A.; Roberts, J. Mechanical Properties of Soft Human Tissues Under Dynamic Loading. J. Biomech. 2007, 40, 1960–1967. [Google Scholar] [CrossRef] [PubMed]
- Tay, B.K.; Kim, J.; Srinivasan, M. In Vivo Mechanical Behavior of Intra-Abdominal Organs. IEEE Trans. Biomed. Eng. 2006, 53, 2129–2138. [Google Scholar] [CrossRef]
- Kemper, A.; Santago, A.; Stitzel, J.; Sparks, J.; Duma, S. Effect of Strain Rate on the Material Properties of Human Liver Parenchyma In Unconfined Compression. J. Biomech. Eng. 2013, 135, 104503. [Google Scholar] [CrossRef]
- Campbell-Kyureghyan, N.; Marras, W. Combined Experimental and Analytical Model of the Lumbar Spine Subjected to Large Displacement Cyclic Loads Part II–Model Validation. Int. J. Comput. Vis. Biomech. 2009, 2, 95–104. [Google Scholar]
- Giraud, S.; Favreau, F.; Chatauret, N.; Thuillier, R.; Maiga, S.; Hauet, T. Contribution of Large Pig For Renal Ischemia-Reperfusion and Transplantation Studies: The Preclinical Model. BioMed Res. Int. 2011, 2011, 532127. [Google Scholar] [CrossRef] [PubMed]
- Sherratt, M.J. Tissue Elasticity and The Ageing Elastic Fibre. Age 2009, 31, 305–325. [Google Scholar] [CrossRef] [PubMed]
- Nykonenko, A.; Petr, V.; Zonča, P. Anatomic Peculiarities of Pig and Human Liver. Exp. Clin. Transplant. 2017, 15, 21–26. [Google Scholar]
- Neuman, R.E.; Logan, M. The Determination of Collagen and Elastin in Tissues. J. Biol. Chem. 1950, 186, 549–556. [Google Scholar] [CrossRef]
- Rojkind, M.; Marie-Adele, G.; Biempica, L. Collagen Types in Normal and Cirrhotic Liver. Gastroenterology 1979, 76, 710–719. [Google Scholar] [CrossRef]


| Strain Rate | Human Kidney | Porcine Kidney | ||
|---|---|---|---|---|
| Compression | Probing | Compression | Probing | |
| 1%/s | √ | √ | √ | √ |
| 5%/s | √ | - | √ | - |
| 25%/s | √ | √ | √ | √ |
| 50%/s | √ | - | √ | - |
| 100%/s | √ | - | √ | - |
| 250%/s | √ | - | √ | - |
| 500%/s | √ | - | √ | - |
| 1000%/s | - | - | √ | - |
| Strain Rate | Human Liver | Porcine Liver | ||
|---|---|---|---|---|
| Compression | Probing | Compression | Probing | |
| 1%/s | √ | √ | √ | √ |
| 5%/s | √ | - | √ | - |
| 25%/s | √ | √ | √ | √ |
| 50%/s | √ | - | √ | - |
| 100%/s | - | - | √ | - |
| 500%/s | √ | - | √ | - |
| Strain Rate | |||
|---|---|---|---|
| Human | 1%/s | 5%/s | 25%/s |
| Etoe (MPa) | 0.0007 (±0.0007) | 0.0016 (±0.0006) | 0.0019 (±0.001) |
| Eterm (MPa) | 0.0056 (±0.0015) | 0.0069 (±0.0024) | 0.01 (±0.0033) |
| εc | 0.084 (±0.032) | 0.093(±0.025) | 0.11 (±0.043) |
| Ψ | 69.75 (±41.14) | 232.71 (±140.63) | 68.35 (±32.93) |
| R2 | 0.919 (±0.08) | 0.9339 (±0.06) | 0.917 (±0.046) |
| Porcine | |||
| Etoe (MPa) | 0.0003 (±0.0003) | 0.0002 (±0.0003) | 0.0002 (±0.0002) |
| Eterm (MPa) | 0.033 (±0.016) | 0.036 (±0.019) | 0.044 (±0.021) |
| εc | 0.191 (±0.012) | 0.19 (±0.015) | 0.195 (±0.014) |
| Ψ | 22.3 (±3.00) | 22.9 (±2.90) | 23.0 (±2.86) |
| R2 | 0.992 (±0.005) | 0.994 (±0.005) | 0.993 (±0.004) |
| Strain Rate | Host | Elastic Modulus (MPa) | p-Value |
|---|---|---|---|
| 1%/s | Human | 0.0056 (±0.0015) | * 0.001 |
| Porcine | 0.0325 (±0.0166) | ||
| 5%/s | Human | 0.0069 (±0.0024) | * 0.001 |
| Porcine | 0.0361 (±0.0185) | ||
| 25%/s | Human | 0.01 (±0.0033) | * 0.001 |
| Porcine | 0.0438 (±0.0208) |
| Variable | a | b | c | d | |
|---|---|---|---|---|---|
| Value | 0.038 | 0.025 | 0.5 | 1,919,803 | Strain Rate |
| Host | Strain Rate | Elastic Modulus (MPa) | p-Value |
|---|---|---|---|
| Human | 1%/s | 0.0353 (±0.0069) | * 0.006 |
| 25%/s | 0.0689 (±0.0279) | ||
| Porcine | 1%/s | 0.0363 (±0.0175) | >0.050 |
| 25%/s | 0.0479 (±0.0166) |
| Variable | A | b | c | d | |
|---|---|---|---|---|---|
| Value | 0.196 | 0.081 | 0.74 | 25.92 | Strain Rate |
| Strain Rate | |||
|---|---|---|---|
| Human | 1%/s | 5%/s | 25%/s |
| Etoe (MPa) | 0.0008 (±0.001) | 0.0005 (±0.0003) | 0.0005 (±0.0005) |
| Eterm (MPa) | 0.026 (±0.011) | 0.027 (±0.012) | 0.042 (±0.018) |
| εc | 0.12 (±0.015) | 0.15 (±0.024) | 0.16 (±0.03) |
| Ψ | 38.99 (±18.58) | 27.23 (±9.62) | 20.82 (±0.26) |
| R2 | 0.96 (±0.02) | 0.99 (±0.01) | 0.96 (±0.03) |
| Porcine | |||
| Etoe (MPa) | 0.0002 (±0.0002) | 0.0002 (±0.0001) | 0.0003 (±0.0002) |
| Eterm (MPa) | 0.032 (±0.012) | 0.042 (±0.018) | 0.043 (±0.028) |
| εc | 0.22 (±0.02) | 0.22 (±0.02) | 0.214 (±0.01) |
| Ψ | 15.57 (±2.49) | 15.09 (±2.33) | 14.07 (±2.49) |
| R2 | 0.997 (±0.002) | 0.996 (±0.005) | 0.994 (±0.002) |
| Strain Rate | Host | Elastic Modulus (MPa) | p-Value |
|---|---|---|---|
| 1%/s | Human | 0.0263 (±0.011) | >0.05 |
| Porcine | 0.0318 (±0.0131) | ||
| 5%/s | Human | 0.0271 (±0.0124) | >0.05 |
| Porcine | 0.0421 (±0.0171) | ||
| 25%/s | Human | 0.0459 (±0.0243) | >0.05 |
| Porcine | 0.0432 (±0.0291) |
| Variable | a | b | c | d | |
|---|---|---|---|---|---|
| Value | 0 | 0.054 | 0.14 | 2,070,615 | Strain Rate |
| Host | Strain Rate | Elastic Modulus (MPa) | p-Value |
|---|---|---|---|
| Human | 1%/s | 0.162 (±0.0743) | >0.050 |
| 25%/s | 0.248 (±0.0712) | ||
| Porcine | 1%/s | 0.0175 (±0.009) | >0.050 |
| 25%/s | 0.0232 (±0.0122) |
| Variable | a | b | c | d | |
|---|---|---|---|---|---|
| Value | 0.011 | 0.05 | 0.46 | 2,075,789 | Strain Rate |
| Variable | a | b | c | d | |
|---|---|---|---|---|---|
| Value | 0 | 0.65 | 0.07 | 2,510,543 | Strain Rate |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Johnson, B.; Campbell, S.; Campbell-Kyureghyan, N. Characterizing the Material Properties of the Kidney and Liver in Unconfined Compression and Probing Protocols with Special Reference to Varying Strain Rate. Biomechanics 2021, 1, 264-280. https://doi.org/10.3390/biomechanics1020022
Johnson B, Campbell S, Campbell-Kyureghyan N. Characterizing the Material Properties of the Kidney and Liver in Unconfined Compression and Probing Protocols with Special Reference to Varying Strain Rate. Biomechanics. 2021; 1(2):264-280. https://doi.org/10.3390/biomechanics1020022
Chicago/Turabian StyleJohnson, Blake, Scott Campbell, and Naira Campbell-Kyureghyan. 2021. "Characterizing the Material Properties of the Kidney and Liver in Unconfined Compression and Probing Protocols with Special Reference to Varying Strain Rate" Biomechanics 1, no. 2: 264-280. https://doi.org/10.3390/biomechanics1020022
APA StyleJohnson, B., Campbell, S., & Campbell-Kyureghyan, N. (2021). Characterizing the Material Properties of the Kidney and Liver in Unconfined Compression and Probing Protocols with Special Reference to Varying Strain Rate. Biomechanics, 1(2), 264-280. https://doi.org/10.3390/biomechanics1020022





