Critical Experiments and Thermodynamic Modeling of the Li2O-SiO2 System
Abstract
1. Introduction
2. Literature Review
3. Thermodynamic Models
3.1. Stoichiometric Compounds
3.2. Ionic Liquid
4. Methodology
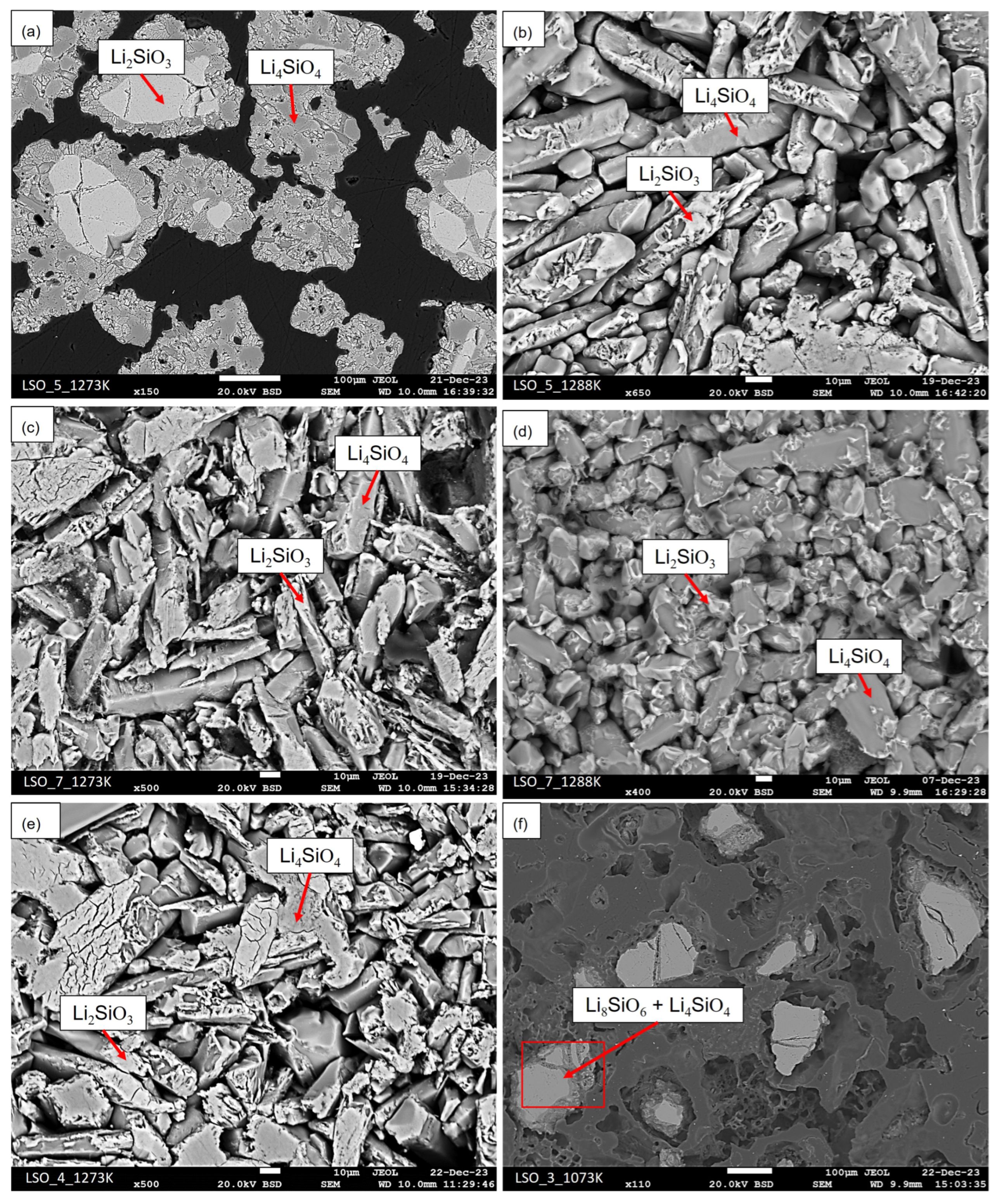
4.1. Sample Preparation and Microstructural Characterization
4.2. Thermal Analysis and Calorimetry
5. Results
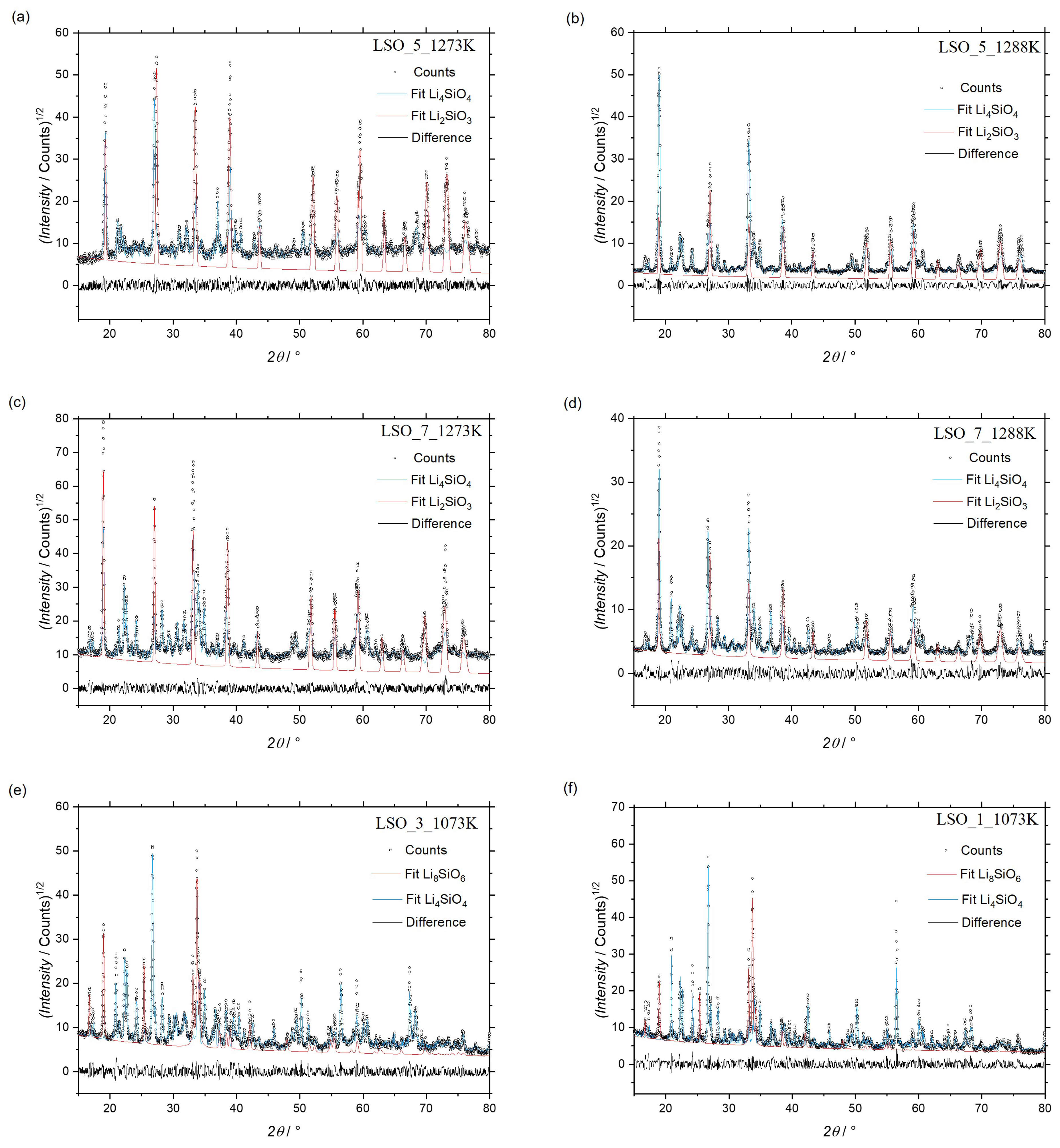
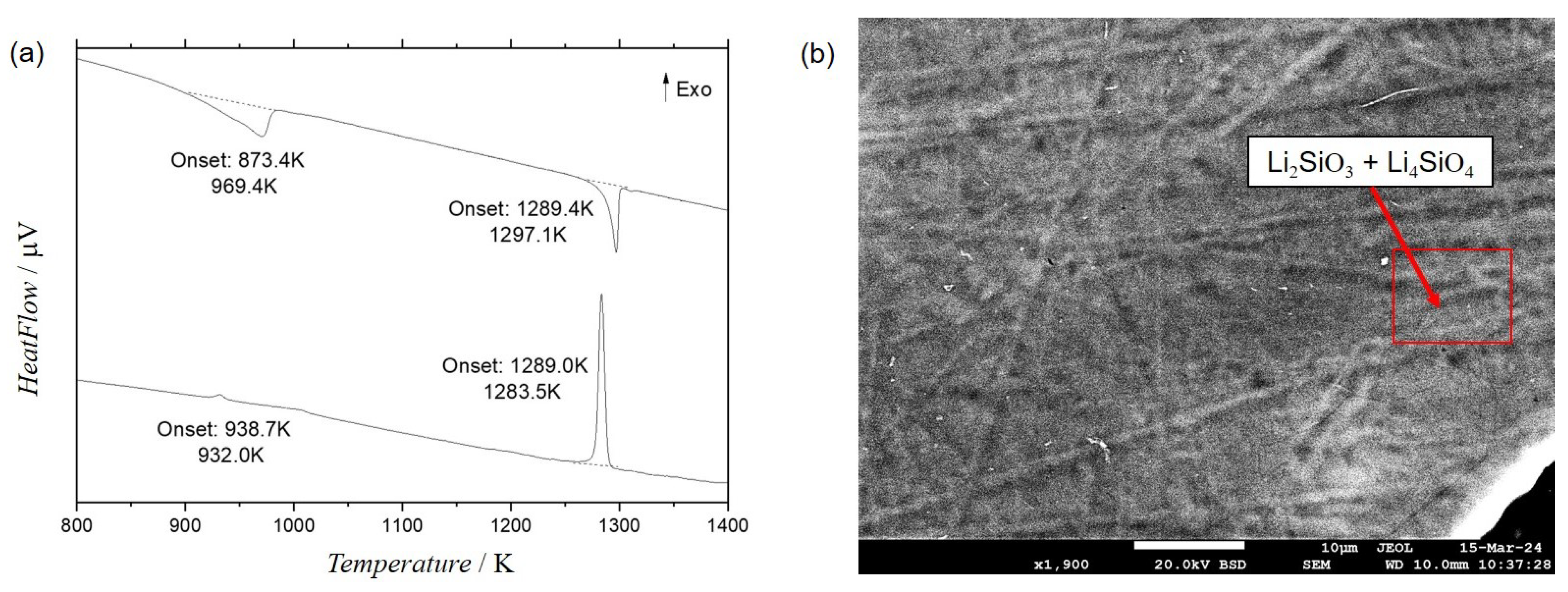
5.1. Experimental Results
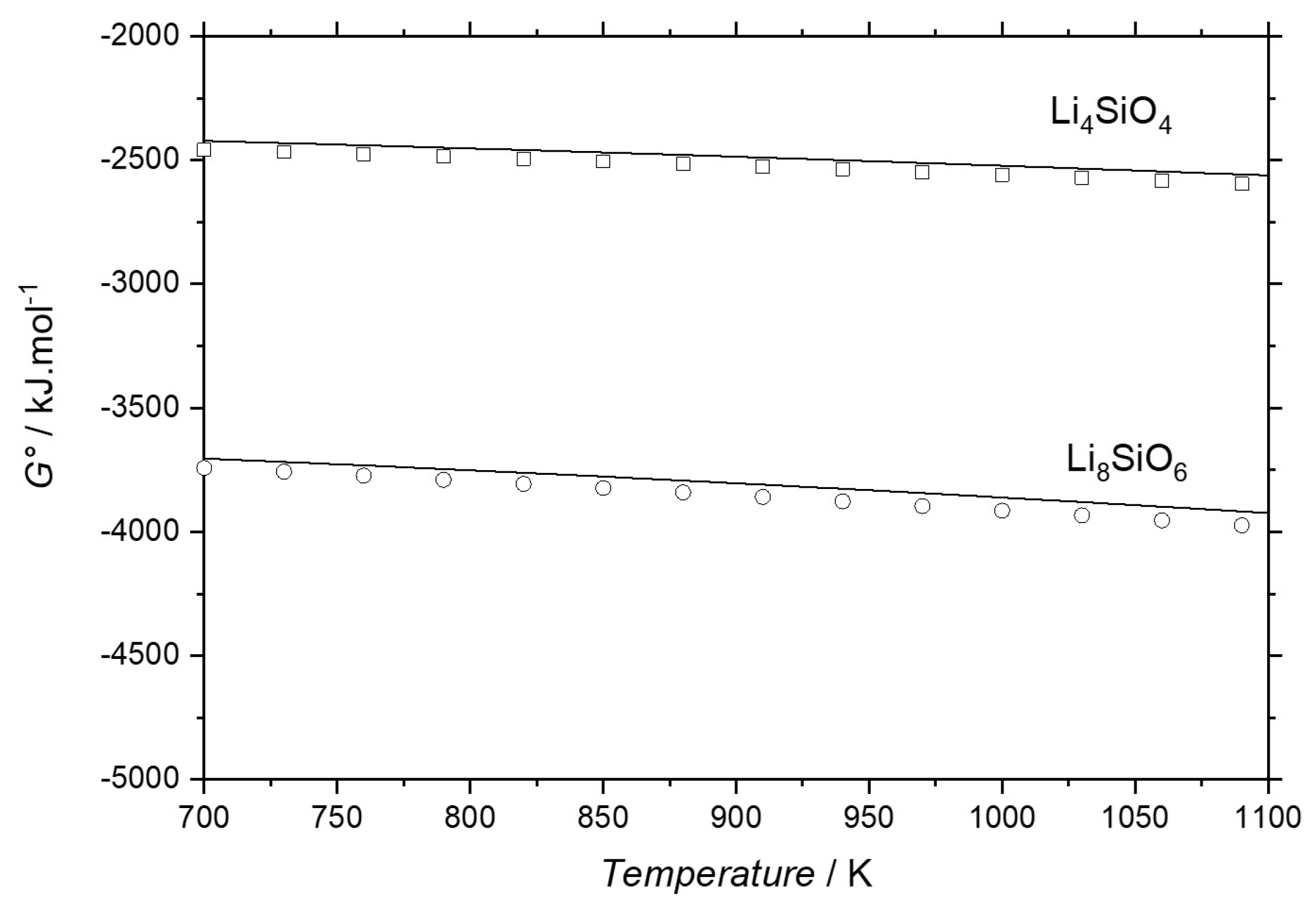
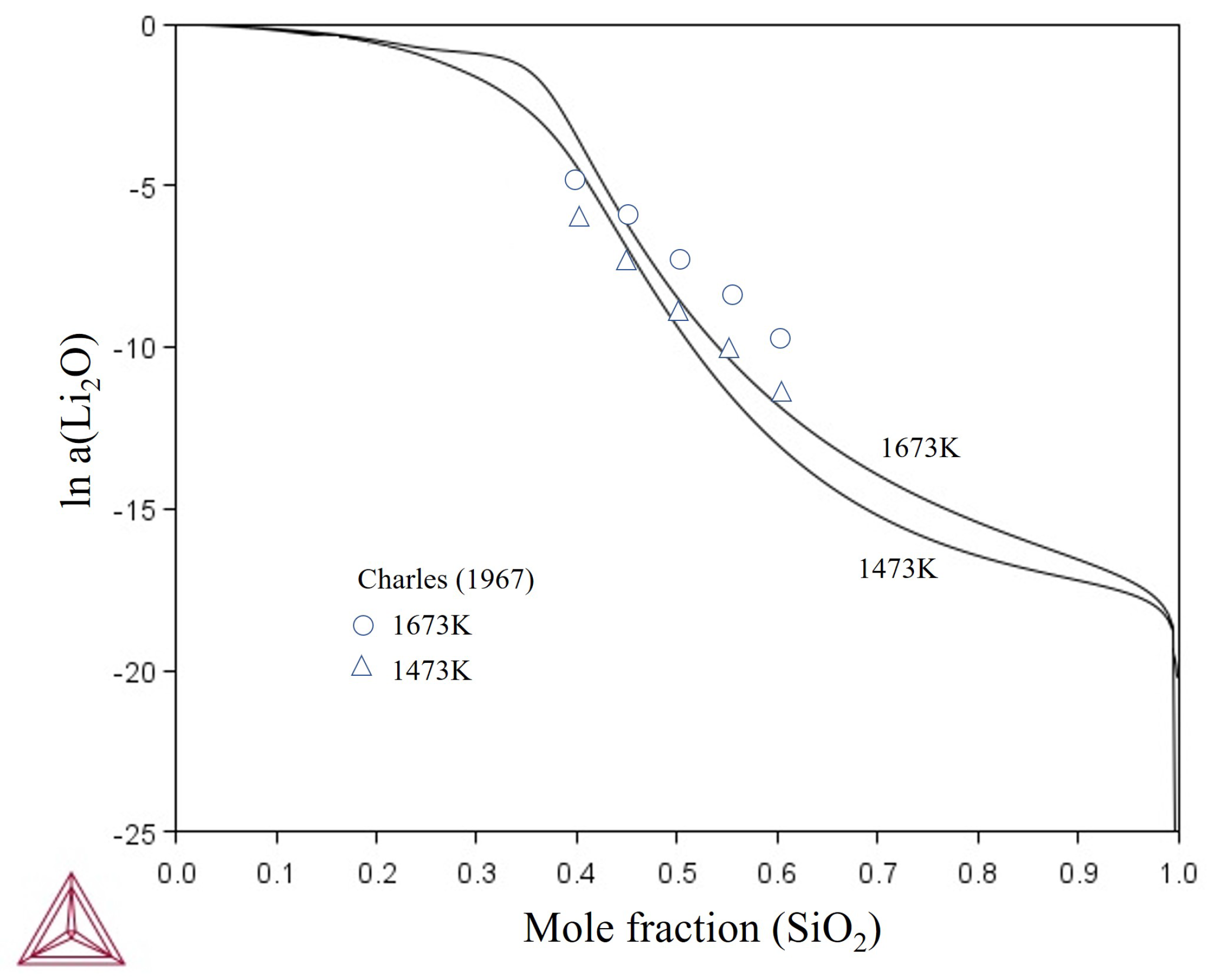
5.2. Thermodynamic Modeling
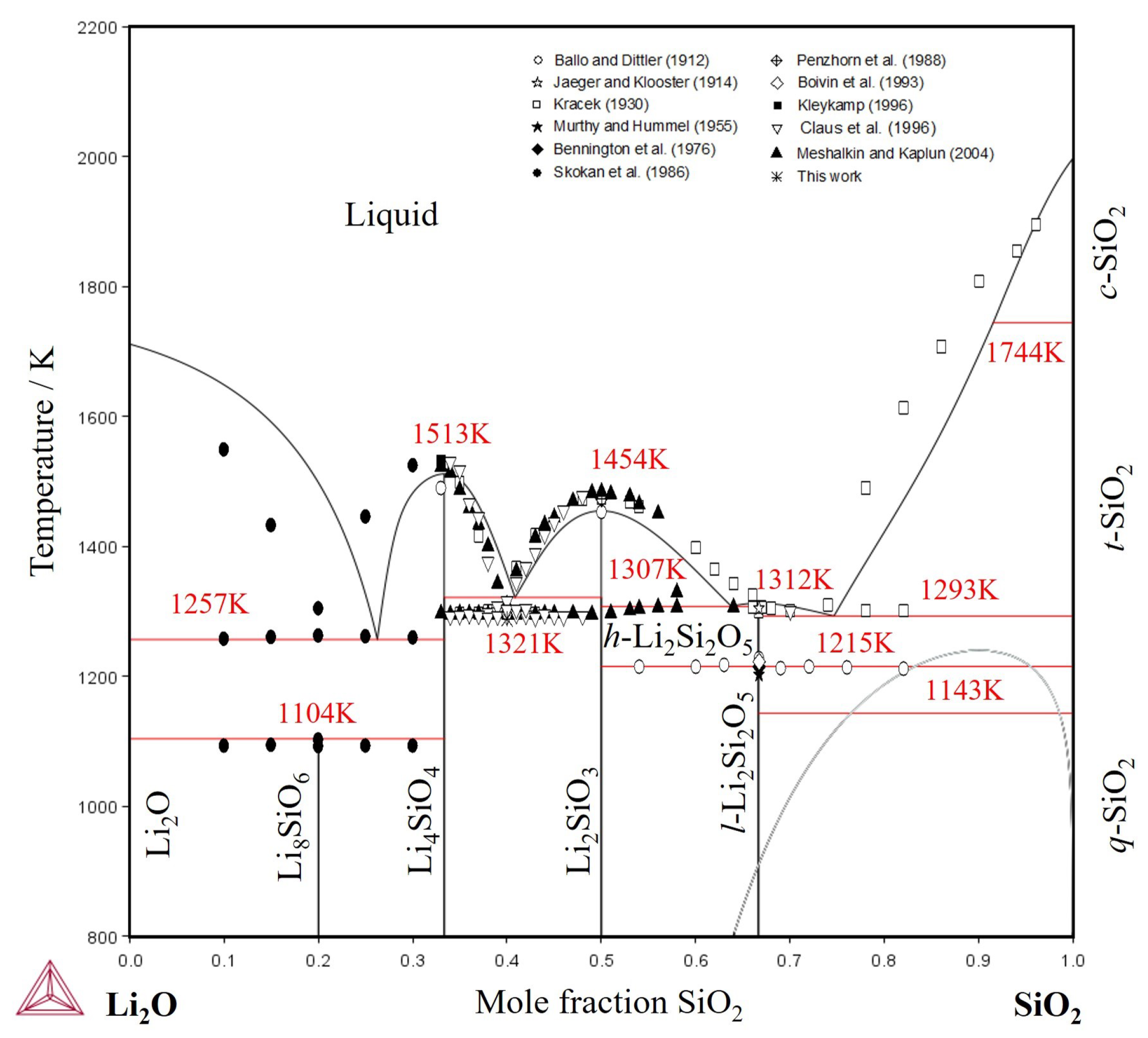
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Elwert, T.; Goldmann, D.; Strauss, K.; Schirmer, T. Phase composition of high lithium slags from the recycling of lithium ion batteries. World Metall.-Erzmetall. 2012, 65, 163–171. [Google Scholar]
- Schirmer, T.; Qiu, H.; Li, H.; Goldmann, D.; Fischlschweiger, M. Li-distribution in compounds of the Li2O-MgO-Al2O3-SiO2-CaO system — A first survey. Metals 2020, 10, 1633. [Google Scholar] [CrossRef]
- Cupid, D.; Reif, A.; Seifert, H. Enthalpy of formation of Li1+ xMn2-xO4 (0 < x < 0.1) spinel phases. Thermochim. Acta 2015, 599, 35–41. [Google Scholar]
- Schnickmann, A.; De Abreu, D.A.; Fabrichnaya, O.; Schirmer, T. Stabilization of Mn4+ in synthetic slags and identification of important slag forming phases. Minerals 2024, 14, 368. [Google Scholar] [CrossRef]
- Guoxing, R.; Songwen, X.; Meiqiu, X.; Bing, P.; Youqi, F.; Fenggang, W.; Xing, X. Recovery of valuable metals from spent lithium-ion batteries by smelting reduction process based on MnO-SiO2-Al2O3 slag system. In Advances in Molten Slags, Fluxes, and Salts, Proceedings of the 10th International Conference on Molten Slags, Fluxes, and Salts 2016, Seattle, WC, USA, 22–25 May 2016; Springer: Berlin/Heidelberg, Germany, 2016; pp. 211–218. [Google Scholar]
- Liu, H.; Zhang, W.; Guan, Z.; Li, N.; Wang, J.; Zhang, S.; Du, Y.; Wang, J.; Dang, F. Thermodynamic Perspective: Insights into the Capacity Increase Phenomenon and Regulation of the Capacity Tendency of MnO for Lithium-Ion Battery Anodes. ACS Appl. Energy Mater. 2021, 4, 12662–12670. [Google Scholar] [CrossRef]
- Li, N.; Li, D.; Zhang, W.; Chang, K.; Dang, F.; Du, Y.; Seifert, H. Development and application of phase diagram for Li-ion batteries using CALPHAD approach. Prog. Nat. Sci. Mater. Inter. 2019, 29, 265–276. [Google Scholar] [CrossRef]
- Haccuria, E.; Hayes, P.; Jak, E. Phase equilibria studies of the MnO-Al2O3-SiO2 system in equilibrium with metallic alloy. Part 1: Development of technique and determination of liquidus isotherms between 1423 and 1523 K. Int. J. Mat. Res. 2014, 105, 941–952. [Google Scholar] [CrossRef]
- Xiao, S.; Ren, G.; Xie, M.; Pan, B.; Fan, Y.; Wang, F.; Xia, X. Recovery of valuable metals from spent lithium-ion batteries by smelting reduction process based on MnO–SiO2–Al2O3 slag system. J. Sustain. Metall. 2017, 3, 703–710. [Google Scholar] [CrossRef]
- Kracek, F. The binary system Li2O–SiO2. J. Phys. Chem. 1930, 34, 2641–2650. [Google Scholar] [CrossRef]
- Skokan, A.; Wedemeyer, H.; Vollath, D.; Günther, E. Thermal properties and application of potential lithium silicate breeder materials. In Fusion Technology 1986. 2 v; International Atomic Energy Agency: Vienna, Austria, 1986. [Google Scholar]
- Claus, S.; Kleykamp, H.; Smykatz-Kloss, W. Phase equilibria in the Li4SiO2-Li2SiO2 region of the pseudobinary Li2O-SiO2 system. J. Nucl. Mater. 1996, 230, 8–11. [Google Scholar] [CrossRef]
- Kim, S.; Sanders, T., Jr. Thermodynamic modeling of phase diagrams in binary alkali silicate systems. J. Am. Ceram. Soc. 1991, 74, 1833–1840. [Google Scholar] [CrossRef]
- Moriya, Y. Phase Separation and Crystallization Process in Glasses. Osaka Kogyo Gijutsu Shikenjo Hokoku 1971, 339, 1–101. [Google Scholar] [CrossRef]
- Konar, B.; Van Ende, M.; Jung, I. Critical evaluation and thermodynamic optimization of the Li-O, and Li2O-SiO2 systems. J. Eur. Ceram. Soc. 2017, 37, 2189–2207. [Google Scholar] [CrossRef]
- Haller, W.; Blackburn, D.; Simmons, J. Miscibility Gaps in Alkali-Silicate Binaries—Data and Thermodynamic Interpretation. J. Am. Ceram. Soc. 1974, 57, 120–126. [Google Scholar] [CrossRef]
- Watanabe, A.; Kobayashi, G.; Matsui, N.; Yonemura, M.; Kubota, A.; Suzuki, K.; Hirayama, M.; Kanno, R. Ambient pressure synthesis and H- conductivity of LaSrLiH2O2. Electrochemistry 2017, 85, 88–92. [Google Scholar] [CrossRef]
- Hofmann, R.; Hoppe, R. Ein neues Oxogermanat: Li8GeO6= Li8O2 [GeO4].(Mit einer Bemerkung über Li8SiO6 und Li4GeO4). Z. Für Anorg. Und Allg. Chem. 1987, 555, 118–128. [Google Scholar] [CrossRef]
- Völlenkle, H.; Wittmann, A.; Nowotny, H. The crystal structure of Li 6[Si2O7]. Monatshefte Für Chem./Chem. Mon. 1969, 100, 295–303. [Google Scholar] [CrossRef]
- Grasso, M.L.; Blanco, M.V.; Cova, F.; Gonzalez, J.A.; Larochette, P.A.; Gennari, F.C. Evaluation of the formation and carbon dioxide capture by Li4SiO4 using in situ synchrotron powder X-ray diffraction studies. Phys. Chem. Chem. Phys. 2018, 20, 26570–26579. [Google Scholar] [CrossRef] [PubMed]
- Völlenkle, H. Verfeinerung der kristallstrukturen von Li2SiO3 und Li2GeO3. Z. Für Krist.-Cryst. Mater. 1981, 154, 77–82. [Google Scholar] [CrossRef]
- De Jong, B.; Slaats, P.; Super, H.; Veldman, N.; Spek, A. Extended structures in crystalline phyllosilicates: Silica ring systems in lithium, rubidium, cesium, and cesium/lithium phyllosilicate. J. Non-Cryst. Solids 1994, 176, 164–171. [Google Scholar] [CrossRef]
- Smith, R.; Howie, R.; West, A.; Aragon-Pina, A.; Villafuerte-Castrejon, M. The structure of metastable lithium disilicate, Li2Si2O5. Acta Crystallogr. Sect. 1990, 46, 363–365. [Google Scholar] [CrossRef]
- Lee, S.; Xu, H.; Xu, H.; Neuefeind, J. Crystal structure of moganite and its anisotropic atomic displacement parameters determined by synchrotron X-ray diffraction and X-ray/neutron pair distribution function analyses. Minerals 2021, 11, 272. [Google Scholar] [CrossRef]
- Tucker, M.; Keen, D.; Dove, M. A detailed structural characterization of quartz on heating through the α–β phase transition. Mineral. Mag. 2001, 65, 489–507. [Google Scholar] [CrossRef]
- Schmahl, W.; Swainson, I.; Dove, M.; Graeme-Barber, A. Landau free energy and order parameter behaviour of the α/β phase transition in cristobalite. Z. Für Krist. 1992, 201, 125–145. [Google Scholar] [CrossRef]
- Kihara, K.; Matsumoto, T.; Imamura, M. High-order thermal-motion tensor analyses of tridymite. Z. Für Krist.-Cryst. Mater. 1986, 177, 39–52. [Google Scholar] [CrossRef]
- Meshalkin, A.; Kaplun, A. Phase Equilibria in the Range 33.33–58 mol% SiO2 of the Li2O-SiO2 System. Russ. J. Inorg. Chem. 2004, 49, 772–774. [Google Scholar]
- de Abreu, D.A.; Löffler, M.; Kriegel, M.J.; Fabrichnaya, O. Experimental Investigation and Thermodynamic Modeling of the Li2O–Al2O3 System. J. Phase Equilibria Diffus. 2024, 45, 36–55. [Google Scholar] [CrossRef]
- Maier, C.; Kelley, K. An equation for the representation of high-temperature heat content data1. J. Am. Chem. Soc. 1932, 54, 3243–3246. [Google Scholar] [CrossRef]
- Hillert, M.; Jansson, B.; Sundman, B. Others A two-sublattice model for molten solutions with different tendency for ionization. Metall. Trans. 1985, 16, 261–266. [Google Scholar] [CrossRef]
- Sundman, B. Modification of the two-sublattice model for liquids. Calphad 1991, 15, 109–119. [Google Scholar] [CrossRef]
- Hillert, M. The compound energy formalism. J. Alloys Compd. 2001, 320, 161–176. [Google Scholar] [CrossRef]
- Belsky, A.; Hellenbrandt, M.; Karen, V.; Luksch, P. New developments in the Inorganic Crystal Structure Database (ICSD): Accessibility in support of materials research and design. Acta Crystallogr. Sect. Struct. Sci. 2002, 58, 364–369. [Google Scholar] [CrossRef] [PubMed]
- Kleykamp, H. Enthalpy, heat capacity, second-order transitions and enthalpyof fusion of Li4SiO4 by high-temperature calorimetry. Thermochim. Acta 1996, 287, 191–201. [Google Scholar] [CrossRef]
- Brandt, R.; Schulz, B. Specific heat of some Li-compounds. J. Nucl. Mater. 1988, 152, 178–181. [Google Scholar] [CrossRef]
- Asou, M.; Terai, T.; Takahashi, Y. High-temperature enthalpy-increment measurements and derived heat capacity of lithium orthosilicate (Li4SiO4) at temperatures from 300 K to 1000 K. J. Chem. Thermodyn. 1992, 24, 273–280. [Google Scholar] [CrossRef]
- Hollenberg, G.W. A transformation in lithium orthosilicate. J. Nucl. Mater. 1981, 103, 591–595. [Google Scholar] [CrossRef]
- Barin, I.; Platzki, G. Thermochemical Data of Pure Substances; Wiley Online Library: Hoboken, NJ, USA, 1989. [Google Scholar]
- Kleykamp, H. Enthalpies and heat capacities of Li2SiO3 and Li2ZrO3 between 298 and 1400 K by drop calorimetry. Thermochim. Acta 1994, 237, 1–12. [Google Scholar] [CrossRef]
- Bennington, K.; Ferrante, M.; Stuve, J. Thermodynamic Properties of two Lithium Silicates (Li2SiO3 and Li2Si2O5); US Department of the Interior, Bureau of Mines: Pittsburgh PA, USA, 1976.
- Stull, D.; Hildenbrand, D.; Oetting, F.; Sinke, G. Low-temperature heat capacities of 15 inorganic compounds. J. Chem. Eng. Data 1970, 15, 52–56. [Google Scholar] [CrossRef]
- Andersson, J.; Helander, T.; Höglund, L.; Shi, P.; Sundman, B. Thermo-Calc & DICTRA, computational tools for materials science. Calphad 2002, 26, 273–312. [Google Scholar]
- Sundman, B.; Lukas, H.; Fries, S. Computational Thermodynamics: The Calphad Method; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Chang, K.; Hallstedt, B. Thermodynamic assessment of the Li–O system. Calphad 2011, 35, 160–164. [Google Scholar] [CrossRef]
- Dumitrescu, L.; Sundman, B. A thermodynamic reassessment of the Si-Al-O-N system. J. Eur. Ceram. Soc. 1995, 15, 239–247. [Google Scholar] [CrossRef]
- Dinsdale, A. SGTE data for pure elements. Calphad 1991, 15, 317–425. [Google Scholar] [CrossRef]
- Wu, P.; Eriksson, G.; Pelton, A. Optimization of the thermodynamic properties and phase diagrams of the Na2O–SiO2 and K2O–SiO2 systems. J. Am. Ceram. Soc. 1993, 76, 2059–2064. [Google Scholar] [CrossRef]
- Ihle, H.; Penzhorn, R.; Schuster, P. The thermochemistry of lithium silicates in view of their use as breeder materials. Fusion Eng. Des. 1989, 8, 393–397. [Google Scholar] [CrossRef]
- Asano, M.; Kubo, K.; Nakagawa, H. Determination of heats of formation for solid and liquid lithium metasilicates by vaporization method. J. Nucl. Sci. Technol. 1983, 20, 1051–1053. [Google Scholar] [CrossRef][Green Version]
- Penzhorn, R.; Ihle, H.; Schuster, P.; Zmbov, K. The evaporation process of solid lithium metasilicate. J. Nucl. Mater. 1988, 155, 471–475. [Google Scholar] [CrossRef]
- Asano, M.; Nakagawa, H. Vaporization of lithium metasilicate in a graphite Knudsen cell. J. Nucl. Mater. 1989, 161, 190–196. [Google Scholar] [CrossRef]
- Migge, H. Estimation of free energies for Li8SiO6 and Li4SiO4 and calculation of the phase diagram of the Li-Si-O system. J. Nucl. Mater. 1988, 151, 101–107. [Google Scholar] [CrossRef]
- Ballo, R.; Dittler, E. Die binären Systeme: Li2SiO3: Al2(SiO3)3, Li4SiO4: Al4(SiO4)3, LiAlO2: SiO2 und Die Lithium-Aluminiumsilikatmineralien. Z. Für Anorg. Chem. 1912, 76, 39–69. [Google Scholar] [CrossRef]
- Jaeger, F.M.; Van Klooster, H.S. Studies in the field of silicate chemistry. I.Compounds of lithia with silica. Versl. Gewone Vergad. Afd. Natuurkd. K. Ned. Akad. Wet. 1914, 22, 900–923. [Google Scholar]
- Murthy, M.; Hummel, F. Phase equilibria in the system lithium metasilicate-forsterite–silica. J. Am. Ceram. Soc. 1955, 38, 55–59. [Google Scholar] [CrossRef]
- Boivin, P.; Berthelay, J.; Blanc, Y.; Coulet, A.; Castanet, R. Determination of temperature and enthalpy of melting of alkali disilicates by differential calorimetric analysis. J. Mater. Sci. 1993, 28, 1834–1838. [Google Scholar] [CrossRef]
- Charles, R. Activities in Li2O-, Na2O, and K2O-SiO2 Solutions. J. Am. Ceram. Soc. 1967, 50, 631–641. [Google Scholar] [CrossRef]

| Phase | Polymorph | Pearson Symbol | Space Group | Prototype | Reference |
|---|---|---|---|---|---|
| Li2O | - | cF12 | Fmm | CaF2 | [17] |
| Li8SiO6 | - | hP30 | P21m | Li8CoO6 | [18] |
| Li6Si2O7 | - | tP30 | P63cm | Li6Si2O7 | [19] |
| Li4SiO4 | - | mP126 | P21/m | Li15(SiO4)3PO4 | [20] |
| Li2SiO3 | - | oS24 | Cmc21 | Na2SiO3 | [21] |
| Li2Si2O5 | l | oS36 | Ccc2 | l-Li2Si2O5 | [22] |
| h | oP36 | Pbcn | h-Li2Si2O5 | [23] | |
| SiO2 | l-quartz | hP9 | P312 1 | quartz (l) | [24] |
| h-quartz | hP9 | P622 2 | quartz (h) | [25] | |
| cristobalite | cF24 | Fdm | cristobalite | [26] | |
| tridymite | hP12 | P63/mmc | tridymite | [27] |
| ID | Nominal Composition | Annealing T(K)/t(h) | Observed Phases (SEM/BSE and XRD) | Vol. Fraction (%) | Lattice Parameters (Å) | (°) | |
|---|---|---|---|---|---|---|---|
| x(SiO2) | x(Li2O) | ||||||
| LSO_1 | 0.20 | 0.80 | 1073 K/8 h | Li8SiO6 | 62.2 | a = 5.4179 | |
| c = 10.6507 | |||||||
| Li4SiO4 | 37.8 | a = 11.4244 | 98.5 | ||||
| b = 6.1667 | |||||||
| c = 16.6477 | |||||||
| 1173 K/4 h | Li4SiO4 | 100.0 | a = 11.4301 | 98.5 | |||
| b = 6.1535 | |||||||
| c = 16.7126 | |||||||
| LSO_2 | 0.17 | 0.83 | 1073 K/8 h | Li8SiO6 | 63.4 | a = 5.4211 | |
| c = 10.6454 | |||||||
| Li4SiO4 | 36.6 | a = 11.4291 | 98.5 | ||||
| b = 6.1676 | |||||||
| c = 16.6618 | |||||||
| 1173 K/4 h | Li4SiO4 | 100.0 | a = 11.4208 | 98.5 | |||
| b = 6.1642 | |||||||
| c = 16.7116 | |||||||
| LSO_3 | 0.13 | 0.87 | 1073 K/8 h | Li8SiO6 | 65.9 | a = 5.4216 | |
| c = 10.6472 | |||||||
| Li4SiO4 | 34.1 | a = 11.4252 | 98.5 | ||||
| b = 6.1664 | |||||||
| c = 16.6618 | |||||||
| LSO_4 | 0.40 | 0.60 | 1273 K/2 h | Li2SiO3 | 43.2 | a = 9.4042 | |
| b = 5.4010 | |||||||
| c = 4.6629 | |||||||
| Li4SiO4 | 56.8 | a = 11.4586 | 98.6 | ||||
| b = 6.1436 | |||||||
| c = 16.6299 | |||||||
| LSO_5 | 0.45 | 0.55 | 1273 K/2 h | Li2SiO3 | 47.5 | a = 9.3937 | |
| b = 5.3940 | |||||||
| c = 4.6614 | |||||||
| Li4SiO4 | 51.1 | a = 11.4293 | 98.2 | ||||
| b = 6.1348 | |||||||
| c = 16.7132 | |||||||
| 1288 K/2 h | Li2SiO3 | 43.57 | a = 9.3914 | ||||
| b = 5.3962 | |||||||
| c = 4.6624 | |||||||
| Li4SiO4 | 56.43 | a = 11.4261 | 98.4 | ||||
| b = 6.1504 | |||||||
| c = 16.6805 | |||||||
| melted - DTA | Li2SiO3 | - | - | ||||
| Li4SiO4 | - | - | |||||
| LSO_7 | 0.35 | 0.65 | 1273 K/2 h | Li2SiO3 | 32.0 | a = 9.3976 | |
| b = 5.3991 | |||||||
| c = 4.6655 | |||||||
| Li4SiO4 | 68.0 | a = 11.4266 | 98.4 | ||||
| b = 6.1680 | |||||||
| c = 16.6824 | |||||||
| 1288 K/2 h | Li2SiO3 | 31.9 | a = 9.3976 | ||||
| b = 5.3991 | |||||||
| c = 4.6655 | |||||||
| Li4SiO4 | 68.1 | a = 11.4266 | 98.4 | ||||
| b = 6.1680 | |||||||
| c = 16.6824 | |||||||
| Phase | Sublattice Model | Thermodynamic Parameter |
|---|---|---|
| Liquid | (Si+4, Li+1)P : (O−2, Va, , )Q | 0 = 2·GSIO2LIQ + 2,000,000 |
| 0 = 8·GSIO2LIQ + 2,000,000 | ||
| 0 = GLI2O + 58,585 − 34.24·T | ||
| 0 = GLIQSI | ||
| 0 = GLIQLI | ||
| 0 = GSIO2LIQ | ||
| 0 = GLI4SIO4 + 28,000 − 16·T | ||
| 0 = −1,482,600 + 14.4·T | ||
| 0 = 920 + 12.21·T | ||
| 0 = 120,000 + 55·T | ||
| 0 = L12 | ||
| 0 = L0 | ||
| 1 = L1 | ||
| 2 = L2 | ||
| 3 = L3 | ||
| 0 = 2·L0 | ||
| 1 = 2·L1 | ||
| 2 = 2·L2 | ||
| 3 = 2·L3 | ||
| Li2O | (Li+1)2 : (O−2)1 | 0 = GLI2O |
| Quartz | (Si+4)1 : (O−2)2 | 0 = GSIO2SOL |
| Cristobalite | (Si+4)1 : (O−2)2 | 0 = GCRISTOB |
| Tridymite | (Si+4)1 : (O−22 | 0 = GTRIDYM |
| Li2O | (Li+1)2 : (O−2)1 | 0 = GLI2O |
| Li8SiO6 | (Li+1)8 : (Si+4)1 : (O−2)6 | 0 = GLI8O6SI1 |
| Li4SiO4 | (Li+1)4 : (Si+4)1 : (O−2)4 | 0 = GLI4O4SI1 |
| Li2SiO3 | (Li+1)2 : (Si+4)1 : (O−2)3 | 0 = GLI2O3SI1 |
| l-Li2Si2O5 | (Li+1)2 : (Si+4)2 : (O−2)5 | 0 = GLI2O5SI2 |
| h-Li2Si2O5 | (Li+1)2 : (Si+4)2 : (O−2)5 | 0 = GLI2O5SI2 + 941.4 − 1·T |
| Name | Temp. Range (K) | Function |
|---|---|---|
| GHSERLI | (200–453.6) | −10,583.817 + 217.637482·T− 38.940488·T·ln(T) + 0.035466931·T2 − 1.9869816·10−5·T3 + 159,994·T−1 |
| (453.6–500) | −559,579.123 + 10,547.8799·T− 1702.88865·T·ln(T) + 2.25832944·T2 − 5.71066077·10−4·T3 + 33,885,874·T−1 | |
| (500–3000) | −9062.994 + 179.278285·T− 31.2283718·T·ln(T) + 0.002633221·T2 − 4.38058·10−7·T3 − 102,387·T−1 | |
| GLIQLI | (200–250) | 2700.205 − 5.795621·T + GHSERLI |
| (250–453.6) | 12,015.027 − 362.187078·T + 61.6104424·T·ln(T) − 0.182426463·T2 + 6.3955671·10−5·T3 − 559,968 ·T−1 | |
| (453.6–500) | −6057.31 + 172.652183·T− 31.2283718·T·ln(T) + 0.002633221·T2 − 4.38058·10−7·T3 − 102,387·T−1 | |
| (500–3000) | 3005.684 −6.626102·T + GHSERLI | |
| GHSERSI | (298.15–1687) | −8162.609 + 137.236859·T− 22.8317533·T·ln(T) − 0.001912904·T2 − 3.552·10−9·T3 + 176,667·T−1 |
| (1687–3600) | −9457.642 + 167.281367·T− 27.196·T·ln(T) − 4.20369·1030·T−9 | |
| GLIQSI | (298.15–1687) | 50,696.36 − 30.099439·T + GHSERSI 2.09307·10−21·T7 |
| (1687–3600) | 40,370.523 + 137.722298·T − 27.196·T·ln(T) | |
| GSIO2SOL | (298.15–540) | −900,936.64 − 360.892175·T + 61.1323·T·ln(T) − 0.189203605 ·T2 + 4.9509742·10−5·T3 − 854,401·T−1 |
| (540–770) | −1,091,466.54 + 2882.67275·T− 452.1367·T·ln(T) + 0.428883845·T2 − 9.0917706·10−5·T3 + 12,476,689·T−1 | |
| (770–848) | −1,563,481.44 + 9178.58655·T− 1404.5352·T·ln(T) + 1.28404426·T2 − 2.35047657· 10−4·T3 +56,402,304·T−1 | |
| (848–1800) | −928,732.923 + 356.218325·T− 58.4292·T·ln(T) − 0.00515995·T2 −2.47·10−10·T3 − 95,113·T−1 | |
| (1800–2960) | −924,076.574 + 281.229013·T− 47.451·T·ln(T) − 0.01200315·T2 − 6.7812·10−7·T3 + 665,385·T−1 | |
| (2960–4000) | −95,7997.4 + 544.992084·T− 82.709·T·ln(T) | |
| GSIO2LIQ | (298.15–2980) | −923,689.98 + 316.24766·T− 52.17·T·ln(T) − 0.012002·T2 +6.78·10−7·T3 + 665,550·T−1 |
| (2980–4000) | − 957,614.21 + 580.01419·T − 87.428·T·ln(T) | |
| GTRIDYM | (298.15–388) | −918,008.73 + 140.55925·T− 25.1574·T·ln(T) − 0.0148714·T2 − 2.2791833·10−5·T3 + 66,331·T−1 |
| (388–433) | −921,013.31 + 224.53808·T− 37.8701·T·ln(T) − 0.02368535·T2 − 1.6835·10−7·T3 | |
| (433–900) | −919,633.42 + 210.51651·T− 35.605·T·ln(T) − 0.03049985·T2 + 4.6255·10−6·T3 − 162,026·T−1 | |
| (900–1668) | −979,377.7 + 848.3098·T− 128.434·T·ln(T) + 0.03387055·T2 − 3.786883·10−6·T3 − 7,070,800·T−1 | |
| (1668–3300) | −943,685.26 + 493.58035·T− 77.5875·T·ln(T) + 0.003040245·T2 − 4.63118·10−7·T3 − 2,227,125·T−1 | |
| (3300–4000) | −974,449.74 + 587.37585·T−87.373·T·ln(T) | |
| GCRISTOB | (298.15–373) | −601,467.73 − 8140.2255·T + 1399.8908·T·ln(T) − 2.8579085·T2 + 0.0010408145·T3 − 13,144,016·T−1 |
| (373–453) | −1,498,711.3 + 13075.913·T− 2178.3561·T·ln(T) + 3.493609·T2 − 0.0010762132·T3 + 29,100,273·T−1 | |
| (453–543) | −3,224,538.7 + 47,854.938·T− 7860.2125·T·ln(T) + 11.817149·T2 − 0.0033651832·T3 + 127,502,720·T−1 | |
| (543–3300) | −943,127.51 + 493.26056·T− 77.5875·T·ln(T) + 0.003040245·T2 − 4.63118·10−7·T3 − 2,227,125·T−1 | |
| (3300–4000) | −973,891.99 + 587.05606·T− 87.373·T·ln(T) | |
| GLI2O | (298.15–3000) | −624800 + 446.6·T− 69.79·T·ln(T) − 0.00883·T2 + 925,000·T−1 |
| GLI4O4SI1 | (298.15–2200) | −2,332,887.25 + 566.24079·T− 95.6958·T·ln(T) − 0.094645·T2 −455,836.475·T−1 |
| GLI2O3SI1 | (298.15–2500) | −1,720,636.91 + 791.818565·T− 125.80476·T·ln(T) − 0.01716·T2 + 1,804,226.17·T−1 |
| GLI2O5SI2 | (298.15–1307) | −2,669,484.12 + 1279.33792·T− 202.0705·T·ln(T) − 0.01936355·T2 + 3,371,258·T−1 |
| (1307–2200) | −2,695,250.72 + 1627.09772·T− 251.04·T·ln(T) | |
| GLI8O6SI1 | (298.15–2200) | −3,623,067.542 + 1424.997383·T− 221.5594·T·ln(T) − 0.13585·T2 + 2,673,426.835·T−1 |
| L0 | (298.15–6000) | 26,902.7455 − 86.1824918·T |
| L1 | (298.15–6000) | 54,362.0415 − 34.9867572·T |
| L2 | (298.15–6000) | 128,176.217 − 69.9409477·T |
| L3 | (298.15–6000) | −757.7632 |
| L12 | (298.15–6000) | −67,793.1799 + 40·T |
| Phase | Δ (kJ mol−1) | Technique | Ref. |
|---|---|---|---|
| Li8SiO6 | −3515.1 | optimized | [15] |
| −3527.0 | optimized | This work | |
| Li4SiO4 | −2328.9 | KEM | [49] |
| −2314.4 | optimized | [15] | |
| −2299.0 | optimized | This work | |
| Li2SiO3 | −1646.1 | Solution calorimetry | [41] |
| −1655.0 | KEM | [50] | |
| −1629.0 | KEM | [51] | |
| −1674.5 | KEM | [52] | |
| −1647.8 | optimized | [15] | |
| −1669.5 | optimized | This work | |
| Li2Si2O5 | −2557.3 | Solution calorimetry | [41] |
| −2560.0 | optimized | [15] | |
| −2584.9 | optimized | This work | |
| Phase | (J mol−1 K−1) | Technique | Ref. |
| Li8SiO6 | 192.0 | optimized | [15] |
| 170.0 | optimized | This work | |
| Li4SiO4 | 117.0 | optimized | [15] |
| 126.0 | optimized | This work | |
| Li2SiO3 | 79.8 | AC | [42] |
| 79.8 | optimized | [15] | |
| 81.3 | optimized | This work | |
| Li2Si2O5 | 122.2 | AC | [41] |
| 122.6 | optimized | [15] | |
| 123.5 | optimized | This work |
| Reaction | Type | Temp. (K) | Liq. Composition x(SiO2) | Technique—Ref. |
|---|---|---|---|---|
| Liq. ⇌ Li2O + Li4SiO4 | Eutectic | 1263 | 0.20 | DTA—[11] |
| 1262 | 0.25 | optimized—[15] | ||
| 1257 | 0.26 | optimized—This work | ||
| Liq. ⇌ Li4SiO4 + Li2SiO3 | Eutectic | 1297 | 0.40 | DTA— [10] |
| 1297 | 0.39 | DTA—[28] | ||
| 1293 | 0.39 | optimized—[15] | ||
| 1289 | - | DTA—This work | ||
| 1321 | 0.41 | optimized—This work | ||
| Liq. ⇌ Li2SiO3 + h-Li2Si2O5 | Eutectic | 1307 | - | DTA—[28] |
| 1295 | 0.65 | optimized—[15] | ||
| 1307 | 0.64 | optimized—This work | ||
| Liq. ⇌ h-Li2Si2O5 + Trd-SiO2 | Eutectic | 1301 | 0.70 | quenching method—[12] |
| 1291 | 0.70 | optimized—[15] | ||
| 1293 | 0.75 | optimized—This work | ||
| Li2O + Li4SiO4 ⇌ Li8SiO6 | Peritectoid | 1103 | - | DTA—[11] |
| 1094 | - | optimized—[15] | ||
| 1104 | - | optimized—This work | ||
| Liq. ⇌ Li4SiO4 | Congruent | 1490 | - | Thermal analysis—[54] |
| 1528 | - | quenching method—[10] | ||
| 1531 | - | DTA—[12] | ||
| 1531 | - | DC—[35] | ||
| 1542 | - | optimized—[15] | ||
| 1513 | - | optimized—This work | ||
| Liq. ⇌ Li2SiO3 | Congruent | 1453 | - | Thermal analysis—[54] |
| 1474 | - | Thermal analysis—[10] | ||
| 1475 | - | quenching method—[10] | ||
| 1482 | - | DTA—[12] | ||
| 1474 | - | KEMS—[51] | ||
| 1484 | - | DTA—[28] | ||
| 1486 | - | optimized—[15] | ||
| 1454 | - | optimized—This work | ||
| h-Li2Si2O5 ⇌ l-Li2Si2O5 | Congruent | 1203 | - | DTA—[56] |
| 1215 | - | SC—[41] | ||
| 1223 | - | DSC—[57] | ||
| 1215 | - | optimized—[15] | ||
| 1215 | - | optimized—This work | ||
| Liq. ⇌ h-Li2Si2O5 | Congruent | 1228 | - | Thermal analysis—[54] |
| 1305 | - | Thermal analysis—[55] | ||
| 1300 | - | Quenching method—[10] | ||
| 1311 | - | DSC—[57] | ||
| 1296 | - | optimized—[15] | ||
| 1312 | - | optimized—This work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
de Abreu, D.A.; Fabrichnaya, O. Critical Experiments and Thermodynamic Modeling of the Li2O-SiO2 System. Solids 2024, 5, 303-320. https://doi.org/10.3390/solids5020020
de Abreu DA, Fabrichnaya O. Critical Experiments and Thermodynamic Modeling of the Li2O-SiO2 System. Solids. 2024; 5(2):303-320. https://doi.org/10.3390/solids5020020
Chicago/Turabian Stylede Abreu, Danilo Alencar, and Olga Fabrichnaya. 2024. "Critical Experiments and Thermodynamic Modeling of the Li2O-SiO2 System" Solids 5, no. 2: 303-320. https://doi.org/10.3390/solids5020020
APA Stylede Abreu, D. A., & Fabrichnaya, O. (2024). Critical Experiments and Thermodynamic Modeling of the Li2O-SiO2 System. Solids, 5(2), 303-320. https://doi.org/10.3390/solids5020020







