Phase Transition Field Effect Transistor Observed in an α-(BEDT-TTF)2I3 Single Crystal
Abstract
1. Introduction
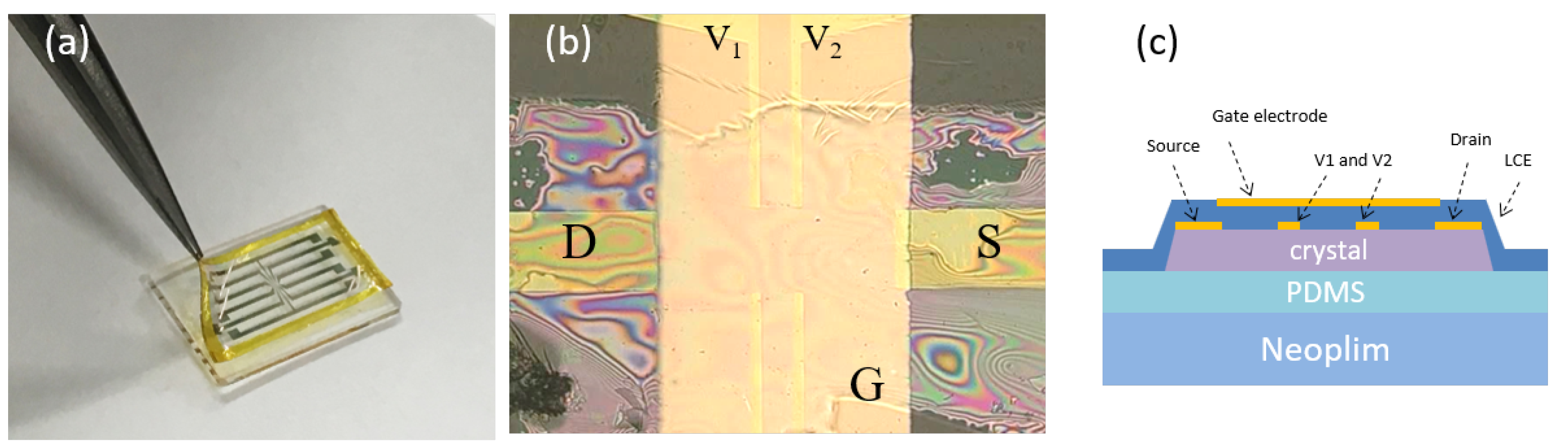
2. Experimental Details
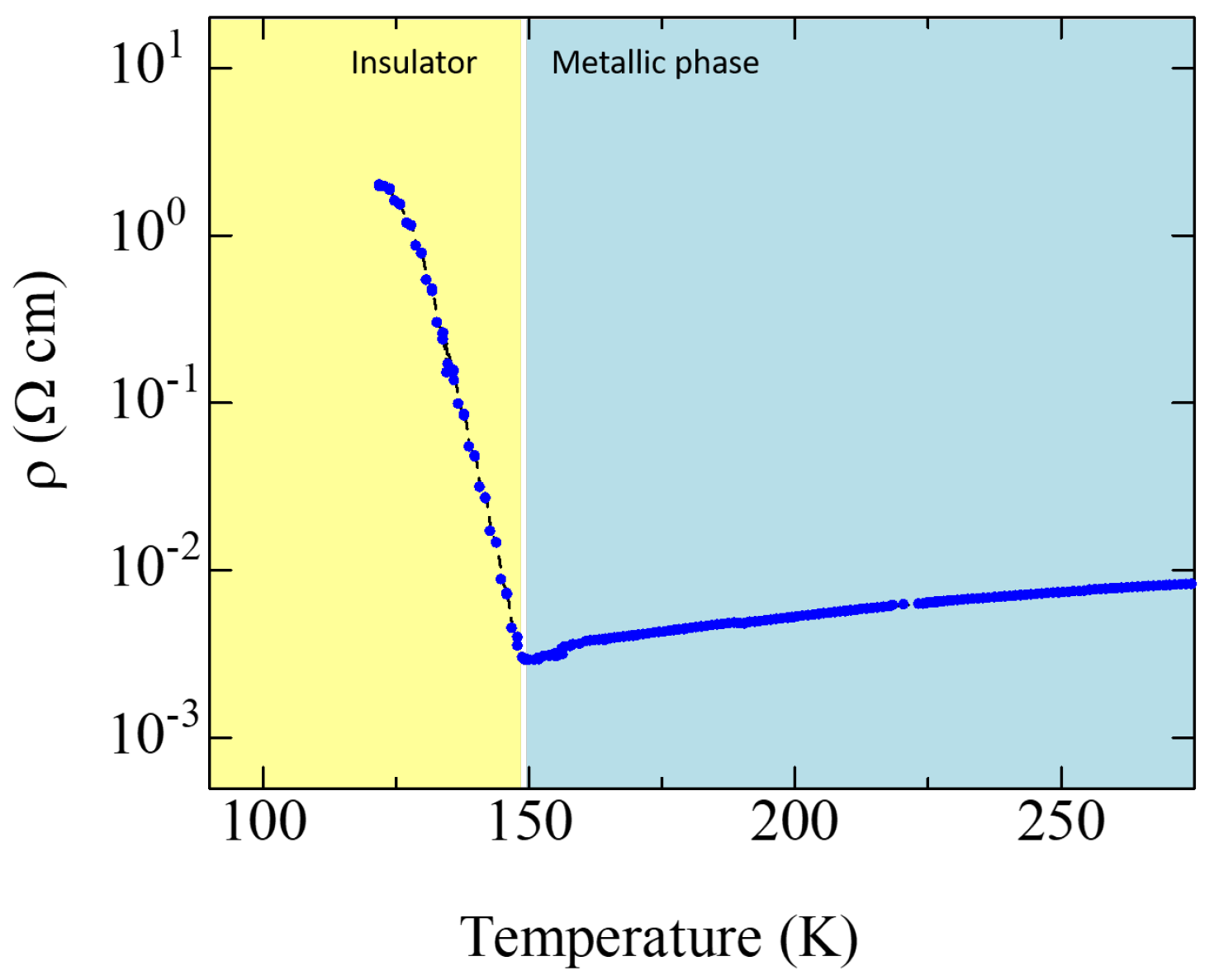
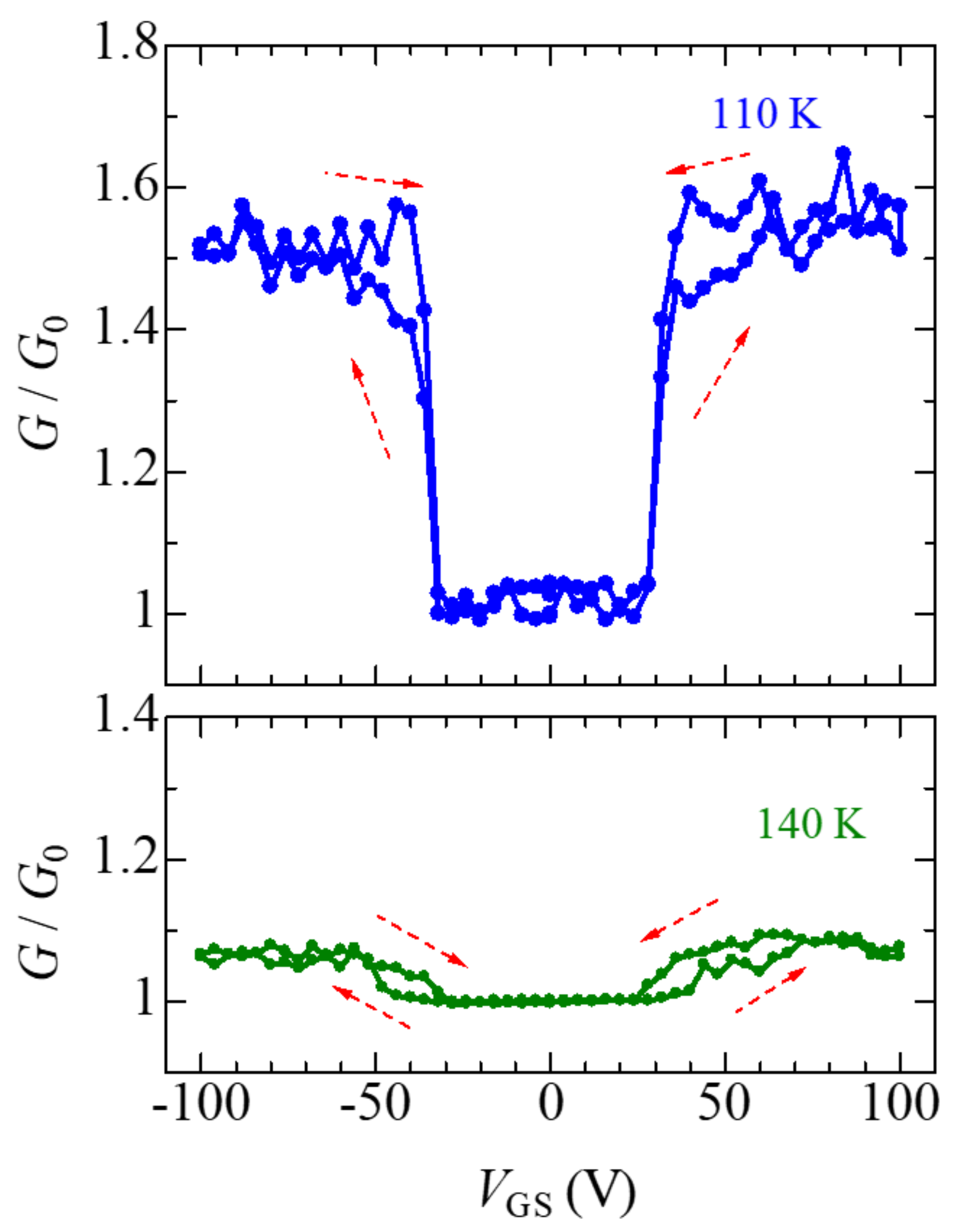
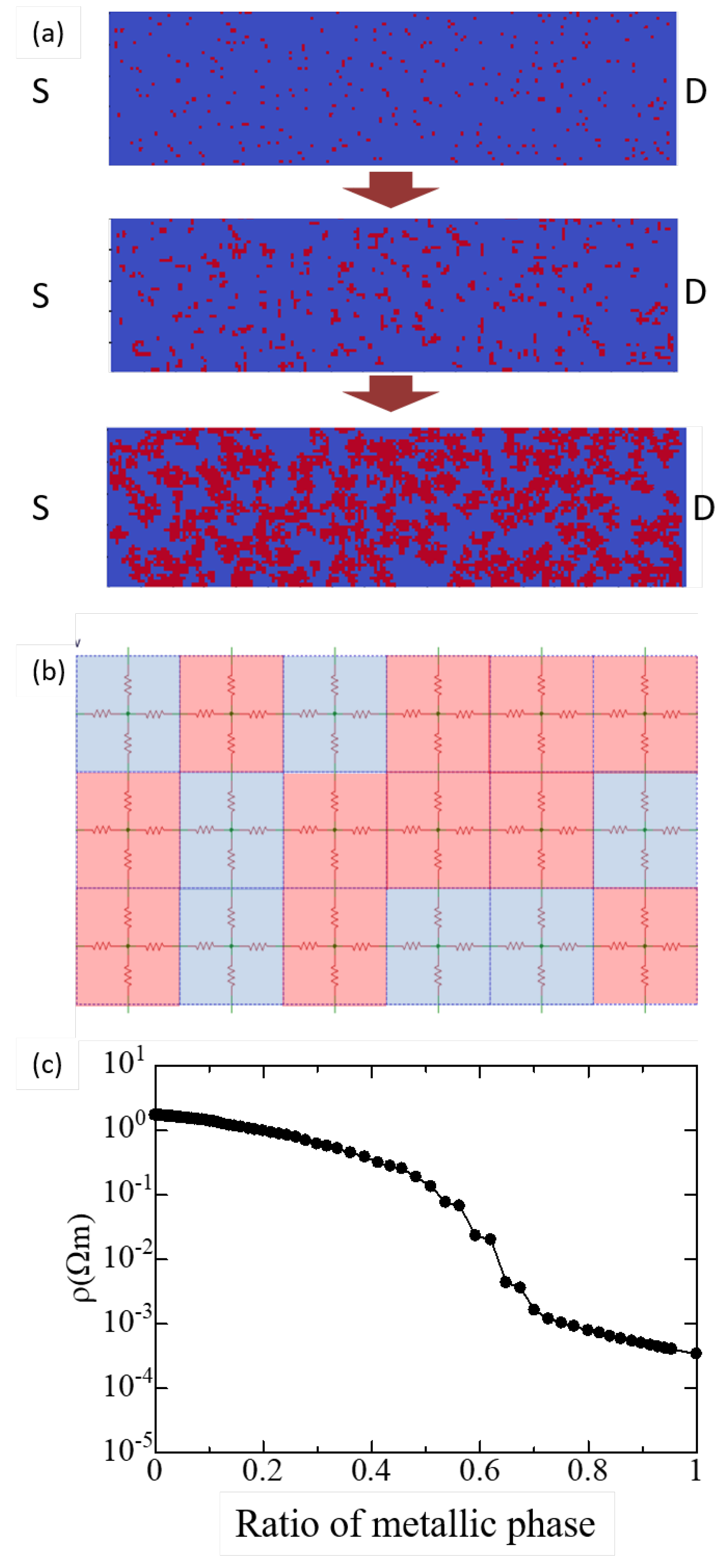
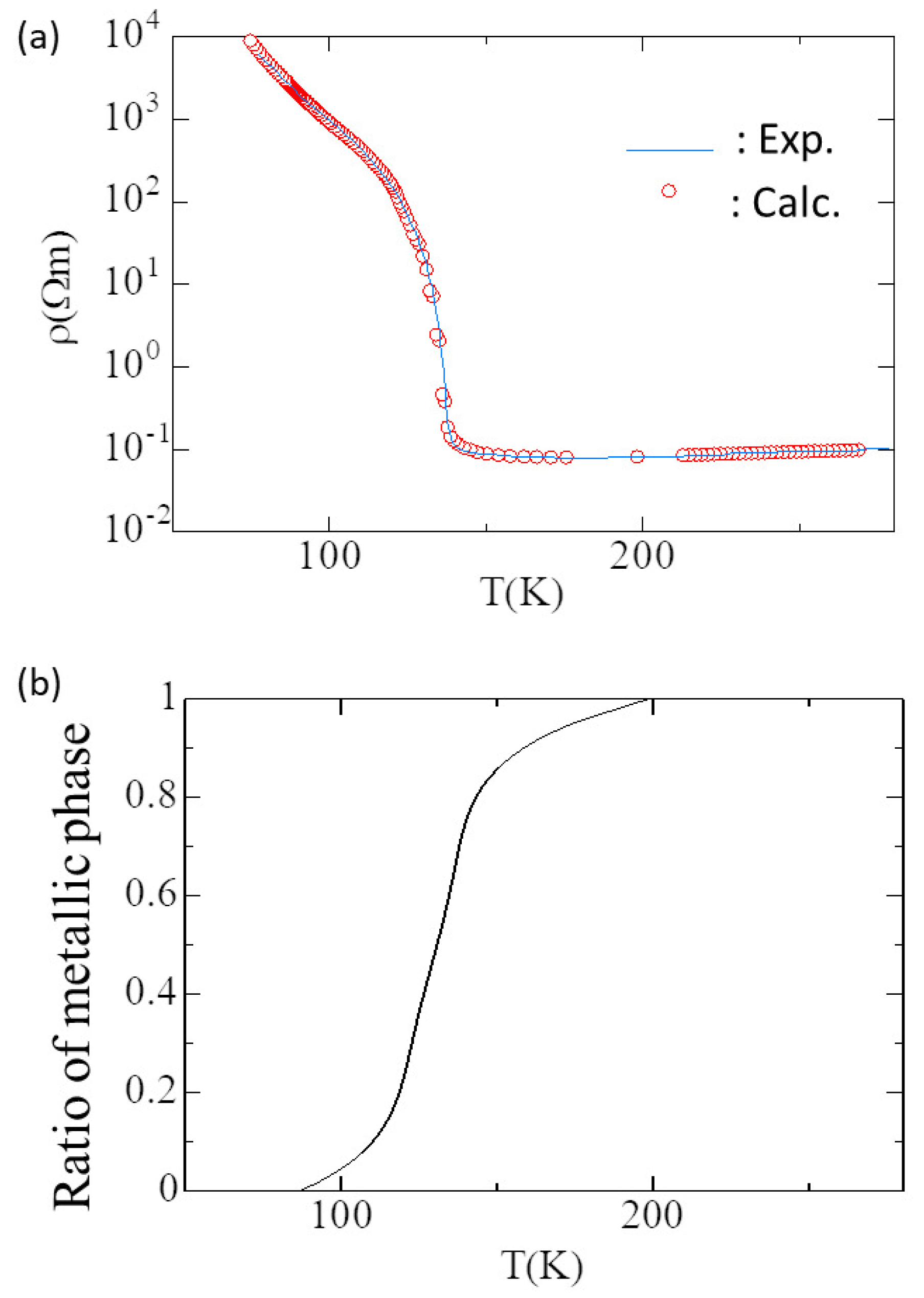
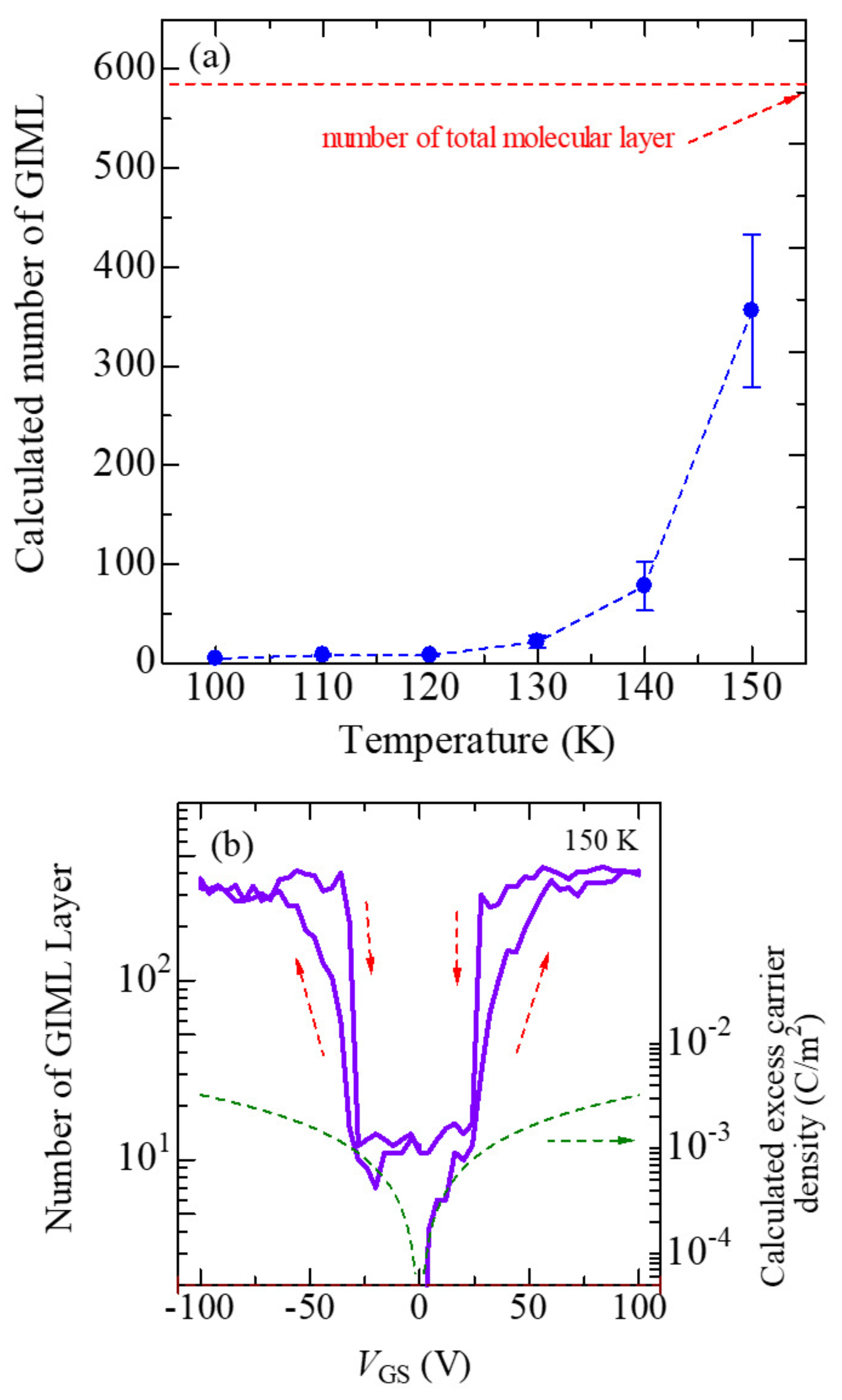
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhou, C.; Newns, D.M.; Misewich, J.A.; Pattnaik, P.C. A field effect transistor based on the Mott transition in a molecular layer. Appl. Phys. Lett. 1997, 70, 598–600. [Google Scholar] [CrossRef]
- Newns, D.M.; Misewich, J.A.; Tsuei, C.C.; Gupta, A.; Scott, B.A.; Schrott, A. Mott transition field effect transistor. Appl. Phys. Lett. 1998, 73, 780–782. [Google Scholar] [CrossRef]
- Hormoz, S.; Ramanathan, S. Limits on vanadium oxide Mott metal–insulator transition field-effect transistors. Solid-State Electron. 2010, 54, 654–659. [Google Scholar] [CrossRef]
- Zhou, Y.; Ramanathan, S. Correlated electron materials and field effect transistors for logic: A review. Crit. Rev. Solid State Mater. Sci. 2013, 38, 286–317. [Google Scholar] [CrossRef]
- Hasegawa, T.; Mattenberger, K.; Takeya, J.; Batlogg, B. Ambipolar field-effect carrier injections in organic Mott insulators. Phys. Rev. B 2004, 69, 245115. [Google Scholar] [CrossRef]
- Sakai, M.; Sakuma, H.; Ito, Y.; Saito, A.; Nakamura, M.; Kudo, K. Ambipolar field-effect transistor characteristics of (BEDT-TTF)(TCNQ) crystals and metal-like conduction induced by a gate electric field. Phys. Rev. B 2007, 76, 045111. [Google Scholar] [CrossRef]
- Yamamoto, H.M.; Hosoda, M.; Kawasugi, Y.; Tsukagoshi, K.; Kato, R. Field effect on organic charge-ordered/Mott insulators. Phys. B Condens. Matter 2009, 404, 413–415. [Google Scholar] [CrossRef]
- Sakai, M.; Ito, Y.; Takahara, T.; Ishiguro, M.; Nakamura, M.; Kudo, K. Ferroelectriclike dielectric response and metal-insulator transition in organic Mott insulator-gate insulator interface. J. Appl. Phys. 2010, 107, 043711. [Google Scholar] [CrossRef]
- Kawasugi, Y.; Yamamoto, H.M.; Hosoda, M.; Tajima, N.; Fukunaga, T.; Tsukagoshi, K.; Kato, R. Strain-induced superconductor/insulator transition and field effect in a thin single crystal of molecular conductor. Appl. Phys. Lett. 2008, 92, 243508. [Google Scholar] [CrossRef]
- Kawasugi, Y.; Yamamoto, H.M.; Tajima, N.; Fukunaga, T.; Tsukagoshi, K.; Kato, R. Field-Induced Carrier Delocalization in the Strain-Induced Mott Insulating State of an Organic Superconductor. Phys. Rev. Lett. 2009, 103, 116801. [Google Scholar] [CrossRef]
- Kawasugi, Y.; Yamamoto, H.M.; Tajima, N.; Fukunaga, T.; Tsukagoshi, K.; Kato, R. Electric-field-induced Mott transition in an organic molecular crystal. Phys. Rev. B 2011, 84, 125129. [Google Scholar] [CrossRef]
- Kimata, M.; Ishihara, T.; Ueda, A.; Mori, H.; Tajima, H. Fabrication of a field effect transistor structure using charge-ordered organic materials α-(BEDT-TTF)2I3 and α’-(BEDT-TTF)2IBr2. Synth. Met. 2013, 173, 43–45. [Google Scholar] [CrossRef]
- Kimata, M.; Ishihara, T.; Tajima, H. Electrostatic Charge Carrier Injection into the Charge-Ordered Organic Material α-(BEDT-TTF)2I3. J. Phys. Soc. Jpn. 2012, 81, 073704. [Google Scholar] [CrossRef]
- Hasegawa, H.; Noguchi, Y.; Ueda, R.; Kubota, T.; Mashiko, S. Organic Mott insulator-based nanowire formed by using the Nanoscale-electrocrystallization. Thin Solid Film. 2008, 516, 2491–2494. [Google Scholar] [CrossRef]
- Yamamoto, H.M.; Ueno, J.; Kato, R. Critical behavior of a filling-controlled Mott-transition observed at an organic field-effect-transistor interface. Eur. Phys. J. Spec. Top. 2013, 222, 1057–1063. [Google Scholar] [CrossRef]
- Yamamoto, H.M.; Suda, M.; Kawasugi, Y. Organic phase-transition transistor with strongly correlated electrons. Jpn. J. Appl. Phys. 2018, 57, 03EA02. [Google Scholar] [CrossRef]
- Suda, M.; Takashina, N.; Namuangruk, S.; Kungwan, N.; Sakurai, H.; Yamamoto, H.M. N-Type Superconductivity in an Organic Mott Insulator Induced by Light-Driven Electron-Doping. Adv. Mater. 2017, 29, 1606833. [Google Scholar] [CrossRef]
- Yang, F.; Suda, M.; Yamamoto, H.M. Fabrication and Operation of Monolayer Mott FET at Room Temperature. Bull. Chem. Soc. Jpn. 2017, 90, 1259–1266. [Google Scholar] [CrossRef]
- Kawasugi, Y.; Seki, K.; Edagawa, Y.; Sato, Y.; Pu, J.; Takenobu, T.; Yunoki, S.; Yamamoto, H.; Kato, R. Electron-hole doping asymmetry of Fermi surface reconstructed in a simple Mott insulator. Nat. Commun. 2016, 7, 12356. [Google Scholar] [CrossRef] [PubMed]
- Kawasugi, Y.; Seki, K.; Edagawa, Y.; Sato, Y.; Pu, J.; Takenobu, T.; Yunoki, S.; Yamamoto, H.M.; Kato, R. Simultaneous enhancement of conductivity and Seebeck coefficient in an organic Mott transistor. Appl. Phys. Lett. 2016, 109, 233301. [Google Scholar] [CrossRef]
- Suda, M.; Kawasugi, Y.; Minari, T.; Tsukagoshi, K.; Kato, R.; Yamamoto, H.M. Strain-Tunable Superconducting Field-Effect Transistor with an Organic Strongly-Correlated Electron System. Adv. Mater. 2014, 26, 3490–3495. [Google Scholar] [CrossRef]
- Yamamoto, H.M.; Kawasugi, Y.; Cui, H.; Nakano, M.; Iwasa, Y.; Kato, R. Asymmetric Phase Transitions Observed at the Interface of a Field-Effect Transistor Based on an Organic Mott Insulator. Eur. J. Inorg. Chem. 2014, 2014, 3841–3844. [Google Scholar] [CrossRef]
- Suda, M.; Yamamoto, H.M. Field-, strain- and light-induced superconductivity in organic strongly correlated electron systems. Phys. Chem. Chem. Phys. 2018, 20, 1321–1331. [Google Scholar] [CrossRef]
- Newns, D.; Doderer, T.; Tsuei, C.; Donath, W.; Misewich, J.; Gupta, A.; Grossman, B.; Schrott, A.; Scott, B.; Pattnaik, P.; et al. The Mott transition field effect transistor: A nanodevice? J. Electroceram. 2000, 4, 339–344. [Google Scholar] [CrossRef]
- Mori, T.; Terasaki, I.; Mori, H. New aspects of nonlinear conductivity in organic charge-transfer salts. J. Mater. Chem. 2007, 17, 4343–4347. [Google Scholar] [CrossRef]
- Ivek, T.; Kovačević, I.; Pinterić, M.; Korin-Hamzić, B.; Tomić, S.; Knoblauch, T.; Schweitzer, D.; Dressel, M. Cooperative dynamics in charge-ordered state of α-(BEDT-TTF) 2 I 3. Phys. Rev. B 2012, 86, 245125. [Google Scholar] [CrossRef]
- Itose, F.; Kawamoto, T.; Mori, T. Collective response to alternating current in the organic conductor α-(bis (ethylenedithio) tetrathiafulvalene) 2I3. J. Appl. Phys. 2013, 113, 213702. [Google Scholar] [CrossRef]
- Itose, F.; Kawamoto, T.; Mori, T. Rapid voltage oscillations and ac-dc interference phenomena in the two-dimensional charge-ordered organic conductor β”-(bis (ethylenedithio) tetrathiafulvalene) 3 (ClO4) 2. J. Appl. Phys. 2012, 112, 033714. [Google Scholar] [CrossRef]
- Tamura, K.; Ozawa, T.; Bando, Y.; Kawamoto, T.; Mori, T. Voltage oscillation associated with nonlinear conductivity in the organic conductor α-(BEDT-TTF) 2I3. J. Appl. Phys. 2010, 107, 103716. [Google Scholar] [CrossRef]
- Ito, A.; Nakamura, Y.; Nakamura, A.; Kishida, H. Measurement of the Nonlinear Conducting States of α-(BEDT- TTF) 2 I 3 Using Electronic Raman Scattering. Phys. Rev. Lett. 2013, 111, 197801. [Google Scholar] [CrossRef]
- Sabeth, F.; Iimori, T.; Ohta, N. Insulator–metal transitions induced by electric field and photoirradiation in organic mott insulator deuterated κ-(BEDT-TTF) 2Cu [N (CN) 2] Br. J. Am. Chem. Soc. 2012, 134, 6984–6986. [Google Scholar] [CrossRef]
- Inagaki, K.; Terasaki, I.; Mori, H.; Mori, T. Large dielectric constant and giant nonlinear conduction in the organic conductor θ-(BEDT-TTF) 2CsZn (SCN) 4. J. Phys. Soc. Jpn. 2004, 73, 3364–3369. [Google Scholar] [CrossRef]
- Mori, T.; Bando, Y.; Kawamoto, T.; Terasaki, I.; Takimiya, K.; Otsubo, T. Giant nonlinear conductivity and spontaneous current oscillation in an incommensurate organic superconductor. Phys. Rev. Lett. 2008, 100, 037001. [Google Scholar] [CrossRef] [PubMed]
- Mori, T.; Ozawa, T.; Bando, Y.; Kawamoto, T.; Niizeki, S.; Mori, H.; Terasaki, I. Nonlinear dynamics of conduction electrons in organic conductors. Phys. Rev. B 2009, 79, 115108. [Google Scholar] [CrossRef]
- Itoh, H.; Itoh, K.; Goto, K.; Yamamoto, K.; Yakushi, K.; Iwai, S. Efficient terahertz-wave generation and its ultrafast optical modulation in charge ordered organic ferroelectrics. Appl. Phys. Lett. 2014, 104, 173302. [Google Scholar] [CrossRef]
- Yamamoto, K.; Kowalska, A.A.; Yue, Y.; Yakushi, K. E–mv coupling of vibrational overtone in organic conductors: Relationship to optical nonlinearities and ferroelectricity. Phys. B Condens. Matter 2012, 407, 1775–1778. [Google Scholar] [CrossRef]
- Yamamoto, K.; Kowalska, A.A.; Yakushi, K. Second-harmonic generation microscopy of ferroelectric organic conductor using hydrostatic pressure apparatus with Ar as a heat sink. Phys. Status Solidi C 2012, 9, 1189–1192. [Google Scholar] [CrossRef]
- Yamamoto, K.; Kowalska, A.A.; Yakushi, K. Direct observation of ferroelectric domains created by Wigner crystallization of electrons in α-[bis (ethylenedithio) tetrathiafulvalene] 2I3. Appl. Phys. Lett. 2010, 96, 122901. [Google Scholar] [CrossRef]
- Monceau, P. Electronic crystals: An experimental overview. Adv. Phys. 2012, 61, 325–581. [Google Scholar] [CrossRef]
- Yamamoto, K.; Iwai, S.; Boyko, S.; Kashiwazaki, A.; Hiramatsu, F.; Okabe, C.; Nishi, N.; Yakushi, K. Strong optical nonlinearity and its ultrafast response associated with electron ferroelectricity in an organic conductor. J. Phys. Soc. Jpn. 2008, 77, 074709. [Google Scholar] [CrossRef]
- Yamamoto, K.; Kowalska, A.; Nakano, C.; Yakushi, K. Inhomogeneous ferroelectric polarization in α’-(BEDT-TTF) 2IBr2 revealed by second-harmonic generation microscopy. Phys. B Condens. Matter 2010, 405, S363–S364. [Google Scholar] [CrossRef]
- Nakaya, H.; Itoh, K.; Takahashi, Y.; Itoh, H.; Iwai, S.; Saito, S.; Yamamoto, K.; Yakushi, K. Terahertz responses of the high-temperature metallic phase and photoinduced metallic state in the ferroelectric charge-ordered organic salt α-(ET) 2 I 3. Phys. Rev. B 2010, 81, 155111. [Google Scholar] [CrossRef]
- Nad, F.; Monceau, P. Dielectric response of the charge ordered state in quasi-one-dimensional organic conductors. J. Phys. Soc. Jpn. 2006, 75, 051005. [Google Scholar] [CrossRef]
- Ishihara, S. Electronic ferroelectricity and frustration. J. Phys. Soc. Jpn. 2010, 79, 011010. [Google Scholar] [CrossRef]
- Sawano, F.; Terasaki, I.; Mori, H.; Mori, T.; Watanabe, M.; Ikeda, N.; Nogami, Y.; Noda, Y. An organic thyristor. Nature 2005, 437, 522–524. [Google Scholar] [CrossRef] [PubMed]
- Kawakami, Y.; Fukatsu, T.; Sakurai, Y.; Unno, H.; Itoh, H.; Iwai, S.; Sasaki, T.; Yamamoto, K.; Yakushi, K.; Yonemitsu, K. Early-stage dynamics of light-matter interaction leading to the insulator-to-metal transition in a charge ordered organic crystal. Phys. Rev. Lett. 2010, 105, 246402. [Google Scholar] [CrossRef]
- Seo, H. Broken symmetry states in quasi-one-dimensional molecular conductors–competitions, co-existences, and frustration–. Phys. B Condens. Matter 2010, 405, S126–S130. [Google Scholar] [CrossRef]
- Wojciechowski, R.; Yamamoto, K.; Yakushi, K.; Inokuchi, M.; Kawamoto, A. High-pressure Raman study of the charge ordering in α-(B E D T- T T F) 2 I 3. Phys. Rev. B 2003, 67, 224105. [Google Scholar] [CrossRef]
- Yue, Y.; Yamamoto, K.; Uruichi, M.; Nakano, C.; Yakushi, K.; Yamada, S.; Hiejima, T.; Kawamoto, A. Nonuniform site-charge distribution and fluctuations of charge order in the metallic state of α-(BEDT-TTF) 2 I 3. Phys. Rev. B 2010, 82, 075134. [Google Scholar] [CrossRef]
- Ivek, T.; Korin-Hamzic, B.; Milat, O.; Tomic, S.; Clauss, C.; Drichko, N.; Schweitzer, D.; Dressel, M. Electrodynamic response of the charge ordering phase: Dielectric and optical studies of α-(BEDT-TTF) 2I3. Phys. Rev. B 2011, 83, 10–1103. [Google Scholar] [CrossRef]
- Kodama, K.; Kimata, M.; Takahide, Y.; Kurita, N.; Harada, A.; Satsukawa, H.; Terashima, T.; Uji, S.; Yamamoto, K.; Yakushi, K. Charge Transport in Charge-Ordered States of Two-Dimensional Organic Conductors, α-(BEDT-TTF) 2I3 and α’-(BEDT-TTF) 2IBr2. J. Phys. Soc. Jpn. 2012, 81, 044703. [Google Scholar]
- Tajima, N.; Fujisawa, J.I.; Naka, N.; Ishihara, T.; Kato, R.; Nishio, Y.; Kajita, K. Photo-induced Insulator–Metal Transition in an Organic Conductor α-(BEDT-TTF) 2I3. J. Phys. Soc. Jpn. 2005, 74, 511–514. [Google Scholar]
- Tajima, N.; Sugawara, S.; Tamura, M.; Nishio, Y.; Kajita, K. Electronic phases in an organic conductor α-(BEDT-TTF) 2I3: Ultra narrow gap semiconductor, superconductor, metal, and charge-ordered insulator. J. Phys. Soc. Jpn. 2006, 75, 051010. [Google Scholar] [CrossRef]
- Mori, T.; Kobayashi, A.; Sasaki, Y.; Kobayashi, H.; Saito, G.; Inokuchi, H. Band structures of two types of (BEDT-TTF) 2I3. Chem. Lett. 1984, 13, 957–960. [Google Scholar]
- Bender, K.; Dietz, K.; Endres, H.; Helberg, H.W.; Hennig, I.; Keller, H.J.; Schäfer, H.; Schweitzer, D. (BEDT-TTF)+ 2J-3: A Two-Dimensional Organic Metal. Mol. Cryst. Liq. Cryst. 1984, 107, 45–53. [Google Scholar] [CrossRef]
- Ohki, D.; Yoshimi, K.; Kobayashi, A.; Misawa, T. Gap opening mechanism for correlated Dirac electrons in organic compounds α-(BEDT- TTF) 2 I 3 and α-(BEDT- TSeF) 2 I 3. Phys. Rev. B 2023, 107, L041108. [Google Scholar]
- Kakiuchi, T.; Wakabayashi, Y.; Sawa, H.; Takahashi, T.; Nakamura, T. Charge ordering in α-(BEDT-TTF) 2I3 by synchrotron X-ray diffraction. J. Phys. Soc. Jpn. 2007, 76, 113702. [Google Scholar] [CrossRef]
- Shimotani, H.; Asanuma, H.; Tsukazaki, A.; Ohtomo, A.; Kawasaki, M.; Iwasa, Y. Insulator-to-metal transition in ZnO by electric double layer gating. Appl. Phys. Lett. 2007, 91, 082106. [Google Scholar]
- Misra, R.; McCarthy, M.; Hebard, A.F. Electric field gating with ionic liquids. Appl. Phys. Lett. 2007, 90, 052905. [Google Scholar] [CrossRef]
- Yuan, H.; Shimotani, H.; Tsukazaki, A.; Ohtomo, A.; Kawasaki, M.; Iwasa, Y. High-density carrier accumulation in ZnO field-effect transistors gated by electric double layers of ionic liquids. Adv. Funct. Mater. 2009, 19, 1046–1053. [Google Scholar] [CrossRef]
- Asanuma, S.; Xiang, P.H.; Yamada, H.; Sato, H.; Inoue, I.; Akoh, H.; Sawa, A.; Ueno, K.; Shimotani, H.; Yuan, H.; et al. Tuning of the metal-insulator transition in electrolyte-gated NdNiO3 thin films. Appl. Phys. Lett. 2010, 97, 142110. [Google Scholar] [CrossRef]
- Scherwitzl, R.; Zubko, P.; Lezama, I.G.; Ono, S.; Morpurgo, A.F.; Catalan, G.; Triscone, J.M. Electric-Field Control of the Metal-Insulator Transition in Ultrathin NdNiO3 Films. Adv. Mater. 2010, 22, 5517–5520. [Google Scholar] [CrossRef] [PubMed]
- Ueno, K.; Nakamura, S.; Shimotani, H.; Ohtomo, A.; Kimura, N.; Nojima, T.; Aoki, H.; Iwasa, Y.; Kawasaki, M. Electric-field-induced superconductivity in an insulator. Nat. Mater. 2008, 7, 855–858. [Google Scholar] [CrossRef]
- Yuan, H.; Shimotani, H.; Ye, J.; Yoon, S.; Aliah, H.; Tsukazaki, A.; Kawasaki, M.; Iwasa, Y. Electrostatic and electrochemical nature of liquid-gated electric-double-layer transistors based on oxide semiconductors. J. Am. Chem. Soc. 2010, 132, 18402–18407. [Google Scholar] [CrossRef] [PubMed]
- Watanabe, H.; Ushikubo, S.; Sakai, M.; Kudo, K.; Masu, H. Effective Method for Multi-Probe Electrical Measurements of Organic Single Crystals: Four-Terminal Natural Adhesion Contact. Solids 2022, 3, 311–318. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ando, R.; Watanuki, R.; Kudo, K.; Masu, H.; Sakai, M. Phase Transition Field Effect Transistor Observed in an α-(BEDT-TTF)2I3 Single Crystal. Solids 2023, 4, 201-212. https://doi.org/10.3390/solids4030013
Ando R, Watanuki R, Kudo K, Masu H, Sakai M. Phase Transition Field Effect Transistor Observed in an α-(BEDT-TTF)2I3 Single Crystal. Solids. 2023; 4(3):201-212. https://doi.org/10.3390/solids4030013
Chicago/Turabian StyleAndo, Ryosuke, Ryo Watanuki, Kazuhiro Kudo, Hyuma Masu, and Masatoshi Sakai. 2023. "Phase Transition Field Effect Transistor Observed in an α-(BEDT-TTF)2I3 Single Crystal" Solids 4, no. 3: 201-212. https://doi.org/10.3390/solids4030013
APA StyleAndo, R., Watanuki, R., Kudo, K., Masu, H., & Sakai, M. (2023). Phase Transition Field Effect Transistor Observed in an α-(BEDT-TTF)2I3 Single Crystal. Solids, 4(3), 201-212. https://doi.org/10.3390/solids4030013




