Modeling Deformation and Fracture of Boron-Based Ceramics with Nonuniform Grain and Phase Boundaries and Thermal-Residual Stress
Abstract
:1. Introduction
2. Phase Field Mechanics
2.1. Coordinates and Order Parameters
2.2. Kinematics
2.3. Balance Laws and Thermodynamics
2.4. Twinning, Plastic Shear, and Dilatation
2.5. Isotropic Elastic Formulation
2.6. Initial Stress
3. Material Properties and Polycrystalline Microstructures
3.1. Boron Carbide Phase
3.2. Titanium Diboride Phase
| Parameter (Units) | BC | TiB | Boundary | Description (Refs.) |
|---|---|---|---|---|
| (g/cm) | 2.52 | 4.52 | - | mass density [34,58,59] |
| (GPa) | 205 | 240 | 211 | initial bulk modulus [14,59,60] |
| (GPa) | 187 | 255 | 200 | initial shear modulus [14,59,60] |
| (-) | 8.40 | 3.04 | 7.16 | bulk stiffening in (38) [14,61,62] |
| (J/m) | 3.27 | 4.14 | 3.47 | nominal fracture energy [34,48,63] |
| 10 | 100 | - | cleavage anisotropy [27,34,48,52] | |
| (J/m) | 0.54 | 0.12 | - | twin boundary or SF energy [12,34,48] |
| 0.31 | 0.015 | - | max twin shear or plastic slip [12,34,48] | |
| (MPa) | 188 | 11.4 | - | phase or dislocation energy [34,48,64] |
| (m) | 0.1 | 0.1 | 0.1 | regularization length [34,48,65] |
| (GPa) | −0.496 | 1.660 | - | nominal residual stress in (46) [4,6,34] |
| (MPa·m) | 1.54 | - | 1.25 | residual toughening in (47) [4] |
3.3. Grain and Phase Boundaries
3.4. Residual Stresses
4. Numerical Methods
4.1. Geometric Rendering and Investigated Parameters
- Composition: BC-23 vol. % TiB versus pure BC;
- Residual stress: nonzero enabled via (46) or suppressed ( = 0);
- Twinning and slip: enabled or suppressed ();
- Grain morphology: effects examined via different loading directions;
- Lattice orientation: randomized to activate different cleavage, habit, and slip planes.
4.2. Boundary Conditions and Homogenization
5. Model Results
- Plasticity, when it occurs, is much more prevalent in the TiB phase (basal slip) than the BC phase (twinning, shear bands).
- Plasticity reduces the tendency for transgranular fracture, especially in TiB grains of the composite.
- Average peak pressure is always slightly compressive, but average pressure is negligible compared to effective deviatoric stress , as expected for equi-biaxial loading.
- Thermal-residual stress enhances overall strength and ductility, primarily via toughening of the BC phase initially under residual compression.
- Heterogeneous grain and phase boundary energies from the Weibull strength statistics lead to more cracks and lower overall strength, in general, than constant boundary energies, which correspond to fewer very weak links in the microstructure.
- The composite, in which intergranular fractures dominate (with transgranular fractures arising sometimes, but less often) demonstrates greater overall strength and ductility than pure BC, in which transgranular fractures dominate.
- Peak effective stress for the BC-TiB composite, averaged over Sims 1, 2, and 3 from Table 2, is 1.58 GPa. Peak effective stress for pure BC, averaged over Sims 13, 14, and 15, is 1.20 GPa. The ratio of composite-to-monolithic material effective strength is 1.58/1.20 = 1.32.
- When plasticity is suppressed in constitutive models of both materials (Sims 4, 5, and 6 vs. 16, 17, and 18), the ratio of composite-to-monolithic material effective strength is 1.17.
Comparison with Experiments and Prior Modeling
- Elastic modulus increase of approximately 20%;
- Static flexure strength increase of approximately 20%;
- Dynamic flexure strength increase of approximately 30%;
- Static fracture toughness increase on the order of 100%;
- Increased dislocation mechanisms;
- Increased tendency for intergranular over transgranular fracture;
- Vickers hardness decrease of approximately 10%;
- Mass density increase of approximately 20%.
6. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ko, Y.; Tsurumi, T.; Fukunaga, O.; Yano, T. High pressure sintering of diamond-SiC composite. J. Mater. Sci. 2001, 36, 469–475. [Google Scholar] [CrossRef]
- Herrmann, M.; Matthey, B.; Hohn, S.; Kinski, I.; Rafaja, D.; Michaelis, A. Diamond-ceramics composites–new materials for a wide range of challenging applications. J. Eur. Ceram. Soc. 2012, 32, 1915–1923. [Google Scholar] [CrossRef]
- Clayton, J.; Guziewski, M.; Ligda, J.; Leavy, R.; Knap, J. A multi-scale approach for phase field modeling of ultra-hard ceramic composites. Materials 2021, 14, 1408. [Google Scholar] [CrossRef] [PubMed]
- Taya, M.; Hayashi, S.; Kobayashi, A.; Yoon, H. Toughening of a particulate-reinforced ceramic-matrix composite by thermal residual stress. J. Am. Ceram. Soc. 1990, 73, 1382–1391. [Google Scholar] [CrossRef]
- Sigl, L. Microcrack toughening in brittle materials containing weak and strong interfaces. Acta Mater. 1996, 44, 3599–3609. [Google Scholar] [CrossRef]
- Rubink, W.; Ageh, V.; Lide, H.; Ley, N.; Young, M.; Casem, D.; Faierson, E.; Scharf, T. Spark plasma sintering of B4C and B4C-TiB2 composites: Deformation and failure mechanisms under quasistatic and dynamic loading. J. Eur. Ceram. Soc. 2021, 41, 3321–3332. [Google Scholar] [CrossRef]
- Gao, Y.; Tang, T.; Yi, C.; Zhang, W.; Li, D.; Xie, W.; Huang, W.; Ye, N. Study of static and dynamic behavior of TiB2–B4C composite. Mater. Des. 2016, 92, 814–822. [Google Scholar] [CrossRef]
- Pittari, J., III; Subhash, G.; Zheng, J.; Halls, V.; Jannotti, P. The rate-dependent fracture toughness of silicon carbide-and boron carbide-based ceramics. J. Eur. Ceram. Soc. 2015, 35, 4411–4422. [Google Scholar] [CrossRef]
- Wenbo, H.; Jiaxing, G.; Jihong, Z.; Jiliang, Y. Microstructure and properties of B4C-ZrB2 ceramic composites. J. Eng. Innov. Technol. 2013, 3, 163–166. [Google Scholar]
- Sadowski, T.; Marsavina, L. Multiscale modelling of two-phase ceramic matrix composites. Comput. Mater. Sci. 2011, 50, 1336–1346. [Google Scholar] [CrossRef]
- Ortiz, M.; Suresh, S. Statistical properties of residual stresses and intergranular fracture in ceramic materials. J. Appl. Mech. 1993, 60, 77–84. [Google Scholar] [CrossRef]
- Vanderwalker, D.; Croft, W. Dislocations in shock-loaded titanium diboride. J. Mater. Res. 1988, 3, 761–763. [Google Scholar] [CrossRef]
- Vanderwalker, D. Fracture in titanium diboride. Phys. Status Solidi A 1989, 111, 119–126. [Google Scholar] [CrossRef]
- Clayton, J. Nonlinear thermodynamic phase field theory with application to fracture and dynamic inelastic phenomena in ceramic polycrystals. J. Mech. Phys. Solids 2021, 157, 104633. [Google Scholar] [CrossRef]
- Clayton, J.; Leavy, R.; Knap, J. Phase field mechanics of residually stressed ceramic composites. Philos. Mag. 2022, 102, 1891–1944. [Google Scholar] [CrossRef]
- Bryant, E.; Sun, W. A mixed-mode phase field fracture model in anisotropic rocks with consistent kinematics. Comput. Methods Appl. Mech. Eng. 2018, 342, 561–584. [Google Scholar] [CrossRef]
- Na, S.; Sun, W. Computational thermomechanics of crystalline rock, Part I: A combined multi-phase field/crystal plasticity approach for single crystal simulations. Comput. Methods Appl. Mech. Eng. 2018, 338, 657–691. [Google Scholar] [CrossRef]
- Nguyen, T.T.; Réthoré, J.; Yvonnet, J.; Baietto, M.C. Multi-phase field modeling of anisotropic crack propagation for polycrystalline materials. Comput. Mech. 2017, 60, 289–314. [Google Scholar] [CrossRef] [Green Version]
- Shahba, A.; Ghosh, S. Coupled phase field finite element model for crack propagation in elastic polycrystalline microstructures. Int. J. Fract. 2019, 219, 31–64. [Google Scholar] [CrossRef]
- Del Piero, G.; Lancioni, G.; March, R. A variational model for fracture mechanics: Numerical experiments. J. Mech. Phys. Solids 2007, 55, 2513–2537. [Google Scholar] [CrossRef]
- Agrawal, V.; Dayal, K. Dependence of equilibrium Griffith surface energy on crack speed in phase field models for fracture coupled to elastodynamics. Int. J. Fract. 2017, 207, 243–249. [Google Scholar] [CrossRef]
- Amirian, B.; Jafarzadeh, H.; Abali, B.; Reali, A.; Hogan, J. Thermodynamically-consistent derivation and computation of twinning and fracture in brittle materials by means of phase field approaches in the finite element method. Int. J. Solids Struct. 2022, 252, 111789. [Google Scholar] [CrossRef]
- Needleman, A. A continuum model for void nucleation by inclusion debonding. J. Appl. Mech. 1987, 54, 525–531. [Google Scholar] [CrossRef]
- Clayton, J. Dynamic plasticity and fracture in high density polycrystals: Constitutive modeling and numerical simulation. J. Mech. Phys. Solids 2005, 53, 261–301. [Google Scholar] [CrossRef]
- Foulk, J.; Vogler, T. A grain-scale study of spall in brittle materials. Int. J. Fract. 2010, 163, 225–242. [Google Scholar] [CrossRef]
- Clayton, J.; Knap, J. A phase field model of deformation twinning: Nonlinear theory and numerical simulations. Phys. D 2011, 240, 841–858. [Google Scholar] [CrossRef] [Green Version]
- Clayton, J.; Knap, J. A geometrically nonlinear phase field theory of brittle fracture. Int. J. Fract. 2014, 189, 139–148. [Google Scholar] [CrossRef]
- Clayton, J.; Williams, C. Modelling the anomalous shock response of titanium diboride. Proc. R. Soc. Lond. A 2022, 478, 20220253. [Google Scholar] [CrossRef]
- Clayton, J. Finsler differential geometry in continuum mechanics: Fundamental concepts, history, and renewed application to ferromagnetic solids. Math. Mech. Solids 2022, 27, 910–949. [Google Scholar] [CrossRef]
- Johnson, K. Contact Mechanics; Cambridge University Press: Cambridge, UK, 1985. [Google Scholar]
- Chen, X.; Hutchinson, J.; Evans, A. The mechanics of indentation induced lateral cracking. J. Am. Ceram. Soc. 2005, 88, 1233–1238. [Google Scholar] [CrossRef]
- Quinn, G.; Bradt, R. On the Vickers indentation fracture toughness test. J. Am. Ceram. Soc. 2007, 90, 673–680. [Google Scholar] [CrossRef]
- Kraft, R.; Molinari, J. A statistical investigation of the effects of grain boundary properties on transgranular fracture. Acta Mater. 2008, 56, 4739–4749. [Google Scholar] [CrossRef]
- Clayton, J.; Rubink, W.; Ageh, V.; Choudhuri, D.; Chen, R.; Du, J.; Scharf, T. Deformation and failure mechanics of boron carbide-titanium diboride composites at multiple scales. JOM 2019, 71, 2567–2575. [Google Scholar] [CrossRef]
- Zavattieri, P.; Raghuram, P.; Espinosa, H. A computational model of ceramic microstructures subjected to multi-axial dynamic loading. J. Mech. Phys. Solids 2001, 49, 27–68. [Google Scholar] [CrossRef]
- Wereszczak, A.; Kirkland, T.; Strong, K.; Jadaan, O.; Thompson, G. Size scaling of tensile failure stress in boron carbide. Adv. Appl. Ceram. 2010, 109, 487–492. [Google Scholar] [CrossRef]
- Giannakopoulos, A.; Larsson, P.L.; Vestergaard, R. Analysis of Vickers indentation. Int. J. Solids Struct. 1994, 31, 2679–2708. [Google Scholar] [CrossRef]
- Zeng, K.; Giannakopoulos, A.; Rowcliffe, D. Vickers indentations in glass II. Comparison of finite element analysis and experiments. Acta Metall. Et Mater. 1995, 43, 1945–1954. [Google Scholar] [CrossRef]
- Gurtin, M. Generalized Ginzburg-Landau and Cahn-Hilliard equations based on a microforce balance. Phys. D 1996, 92, 178–192. [Google Scholar] [CrossRef]
- Clayton, J.; Knap, J. Phase field modeling of coupled fracture and twinning in single crystals and polycrystals. Comput. Methods Appl. Mech. Eng. 2016, 312, 447–467. [Google Scholar] [CrossRef]
- Allen, S.; Cahn, J. A microscopic theory for antiphase boundary motion and its application to antiphase domain coarsening. Acta Metall. 1979, 27, 1085–1095. [Google Scholar] [CrossRef]
- Clayton, J. Nonlinear Mechanics of Crystals; Springer: Dordrecht, The Netherlands, 2011. [Google Scholar]
- Brace, W.; Paulding, B.; Scholz, C. Dilatancy in the fracture of crystalline rocks. J. Geophys. Res. 1966, 71, 3939–3953. [Google Scholar] [CrossRef]
- Curran, D.; Seaman, L.; Cooper, T.; Shockey, D. Micromechanical model for comminution and granular flow of brittle material under high strain rate application to penetration of ceramic targets. Int. J. Impact Eng. 1993, 13, 53–83. [Google Scholar] [CrossRef]
- Clayton, J.; Knap, J. Phase field modeling of directional fracture in anisotropic polycrystals. Comput. Mater. Sci. 2015, 98, 158–169. [Google Scholar] [CrossRef]
- Borden, M.; Verhoosel, C.; Scott, M.; Hughes, T.; Landis, C. A phase field description of dynamic brittle fracture. Comput. Methods Appl. Mech. Eng. 2012, 217, 77–95. [Google Scholar] [CrossRef]
- Amor, H.; Marigo, J.J.; Maurini, C. Regularized formulation of the variational brittle fracture with unilateral contact: Numerical experiments. J. Mech. Phys. Solids 2009, 57, 1209–1229. [Google Scholar] [CrossRef]
- Clayton, J.; Leavy, R.; Knap, J. Phase field modeling of heterogeneous microcrystalline ceramics. Int. J. Solids Struct. 2019, 166, 183–196. [Google Scholar] [CrossRef]
- An, Q.; Goddard, W.A.; Cheng, T. Atomistic explanation of shear-induced amorphous band formation in boron carbide. Phys. Rev. Lett. 2014, 113, 095501. [Google Scholar] [CrossRef] [Green Version]
- An, Q.; Goddard, W. Atomistic origin of brittle failure of boron carbide from large-scale reactive dynamics simulations: Suggestions toward improved ductility. Phys. Rev. Lett. 2015, 115, 105051. [Google Scholar] [CrossRef] [Green Version]
- Clayton, J.; Knap, J. Continuum modeling of twinning, amorphization, and fracture: Theory and numerical simulations. Contin. Mech. Thermodyn. 2018, 30, 421–455. [Google Scholar] [CrossRef]
- Clayton, J.; Zorn, J.; Leavy, R.; Guziewski, M.; Knap, J. Phase field modeling of diamond-silicon carbide ceramic composites with tertiary grain boundary phases. Int. J. Fract. 2022, 237, 101–138. [Google Scholar] [CrossRef]
- Patil, S.; Heider, Y.; Padilla, C.; Cruz-Chú, E.; Markert, B. A comparative molecular dynamics-phase field modeling approach to brittle fracture. Comput. Methods Appl. Mech. Eng. 2016, 312, 117–129. [Google Scholar] [CrossRef]
- Hu, S.; Henager, C.; Chen, L.Q. Simulations of stress-induced twinning and de-twinning: A phase field model. Acta Mater. 2010, 58, 6554–6564. [Google Scholar] [CrossRef]
- Jafarzadeh, H.; Levitas, V.; Farrahi, G.; Javanbakht, M. Phase field approach for nanoscale interactions between crack propagation and phase transformation. Nanoscale 2019, 11, 22243–22247. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Hahn, E.; Branicio, P.; Yao, X.; Germann, T.; Feng, B.; Zhang, X. Defect reversibility regulates dynamic tensile strength in silicon carbide at high strain rates. Scr. Mater. 2022, 213, 114593. [Google Scholar] [CrossRef]
- Clayton, J.; Knap, J. Phase field modeling of twinning in indentation of transparent single crystals. Model. Simul. Mater. Sci. Eng. 2011, 19, 085005. [Google Scholar] [CrossRef]
- Thevenot, F. A review on boron carbide. Key Eng. Mater. 1991, 56, 59–88. [Google Scholar] [CrossRef]
- Munro, R. Material properties of titanium diboride. J. Res. Natl. Inst. Stand. Technol. 2000, 105, 709–720. [Google Scholar] [CrossRef]
- Swab, J.; Meredith, C.; Casem, D.; Gamble, W. Static and dynamic compression strength of hot-pressed boron carbide using a dumbbell-shaped specimen. J. Mater. Sci. 2017, 52, 10073–10084. [Google Scholar] [CrossRef]
- Dandekar, D.; Benfanti, D. Strength of titanium diboride under shock wave loading. J. Appl. Phys. 1993, 73, 673–679. [Google Scholar] [CrossRef]
- Dodd, S.; Saunders, G.; James, B. Temperature and pressure dependences of the elastic properties of ceramic boron carbide (B4C). J. Mater. Sci. 2002, 37, 2731–2736. [Google Scholar] [CrossRef]
- Beaudet, T.; Smith, J.; Adams, J. Surface energy and relaxation in boron carbide (101) from first principles. Solid State Commun. 2015, 219, 43–47. [Google Scholar] [CrossRef]
- Fanchini, G.; McCauley, J.; Chhowalla, M. Behavior of disordered boron carbide under stress. Phys. Rev. Lett. 2006, 97, 035502. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Clayton, J. Computational modeling of dual-phase ceramics with Finsler-geometric phase field mechanics. Comput. Model. Eng. Sci. CMES 2019, 120, 333–350. [Google Scholar] [CrossRef] [Green Version]
- Miehe, C.; Schaenzel, L.M.; Ulmer, H. Phase field modeling of fracture in multi-physics problems. Part I. Balance of crack surface and failure criteria for brittle crack propagation in thermo-elastic solids. Comput. Methods Appl. Mech. Eng. 2015, 294, 449–485. [Google Scholar] [CrossRef]
- Clayton, J.; Knap, J. Geometric micromechanical modeling of structure changes, fracture, and grain boundary layers in polycrystals. J. Micromech. Mol. Phys. 2018, 3, 1840001. [Google Scholar] [CrossRef]
- Hwang, C.; Du, J.; Yang, Q.; Celik, A.; Christian, K.; An, Q.; Schaefer, M.; Xie, K.; LaSalvia, J.; Hemker, K.; et al. Addressing amorphization and transgranular fracture of B4C through Si doping and TiB2 microparticle reinforcing. J. Am. Ceram. Soc. 2022, 105, 2959–2977. [Google Scholar] [CrossRef]
- Yang, Q.; Celik, A.; Du, J.; LaSalvia, J.; Hwang, C.; Haber, R. Advancing the mechanical properties of Si/B co-doped boron carbide through TiB2 reinforcement. Mater. Lett. 2020, 266, 127480. [Google Scholar] [CrossRef]
- Hu, G.; Chen, C.; Ramesh, K.; McCauley, J. Mechanisms of dynamic deformation and dynamic failure in aluminum nitride. Acta Mater. 2012, 60, 3480–3490. [Google Scholar] [CrossRef]
- Shen, Y.; Li, G.; An, Q. Enhanced fracture toughness of boron carbide from microalloying and nanotwinning. Scr. Mater. 2019, 162, 306–310. [Google Scholar] [CrossRef]
- Ye, K.; Wang, Z. Twins enhanced mechanical properties of boron carbide. Ceram. Int. 2022, 48, 14499–14506. [Google Scholar] [CrossRef]
- Chen, M.; McCauley, J.; Hemker, K. Shock-induced localized amorphization in boron carbide. Science 2003, 299, 1563–1566. [Google Scholar] [CrossRef] [PubMed]

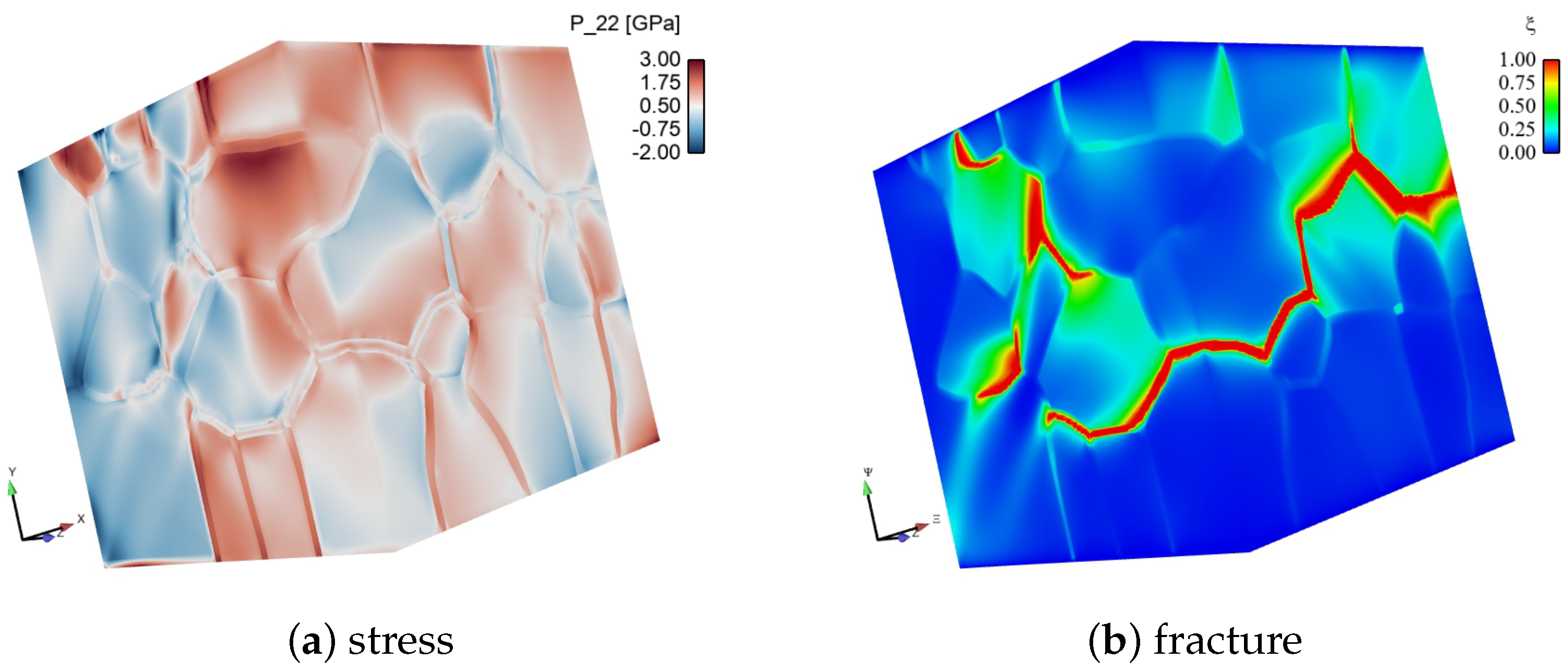
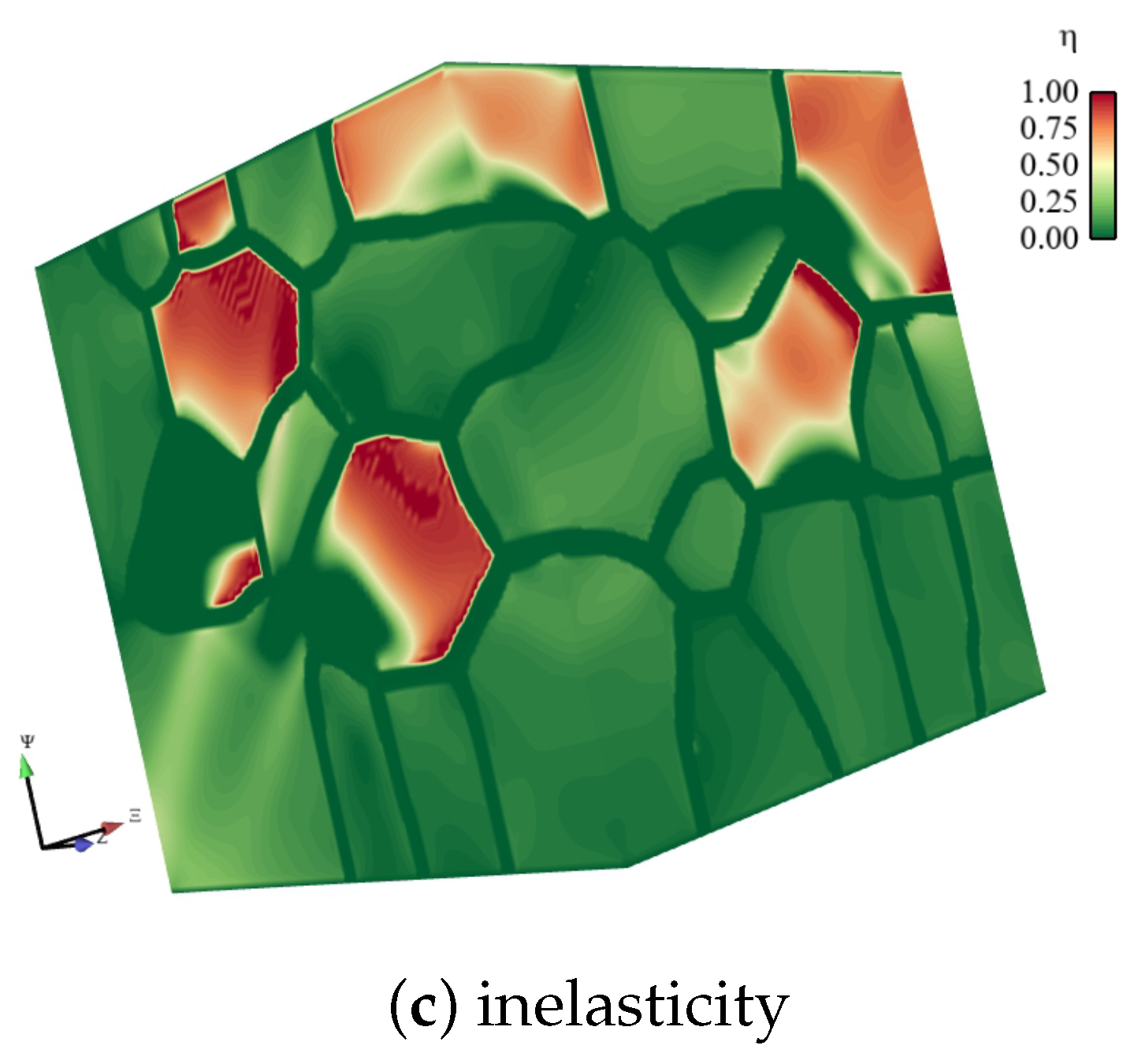

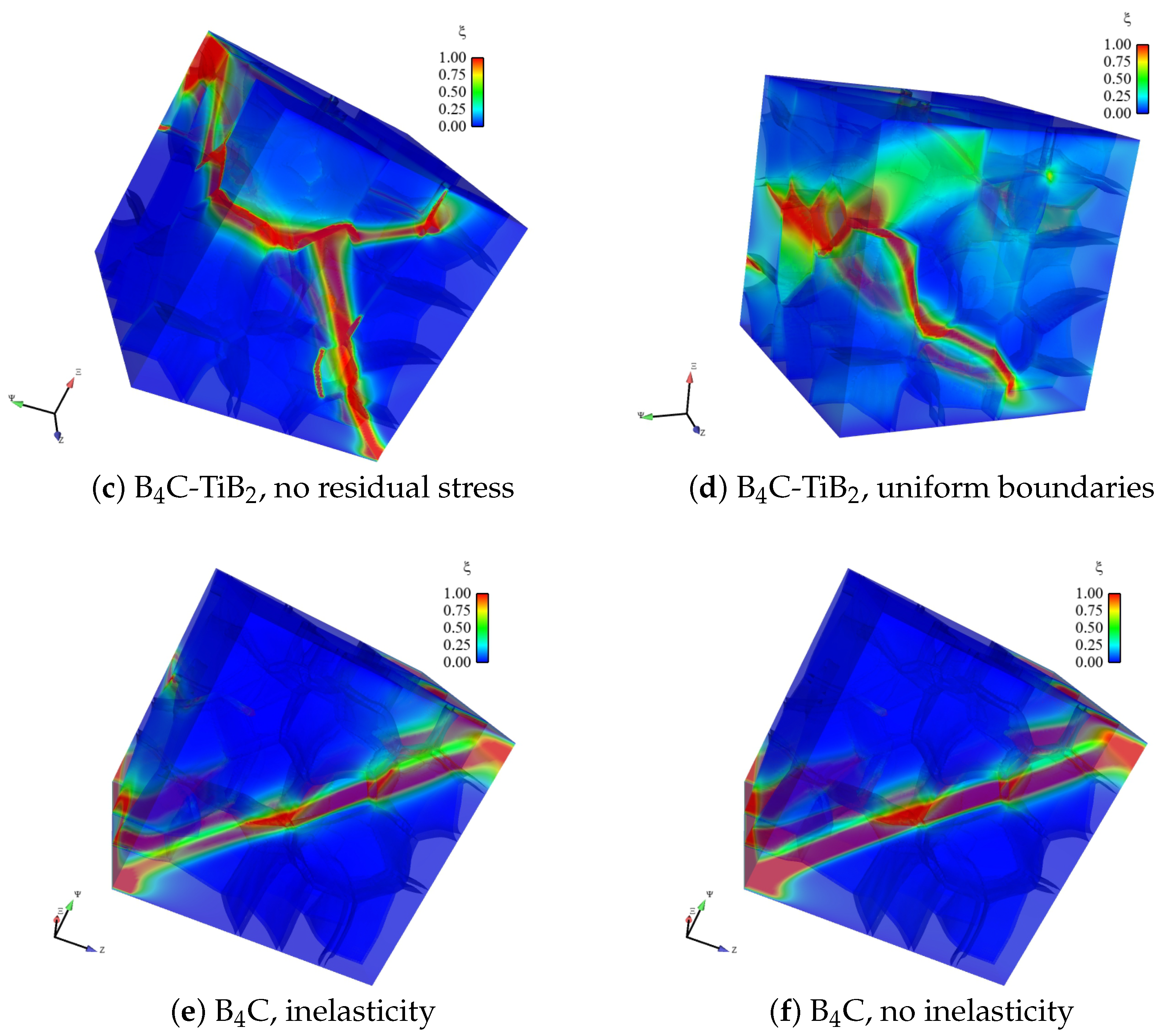


| Sim. | Material | Lattice | Bound. | Slip/Twin | Res. Stress | Weibull | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| Cond. | (GPa) | (GPa) | ||||||||
| 1 | BC-TiB | 1 | Y | Y | Y | 1.6349 | 0.006347 | 0.1451 | 0.1196 | |
| 2 | BC-TiB | 2 | Y | Y | Y | 1.4603 | 0.006776 | 0.1390 | 0.1261 | |
| 3 | BC-TiB | 3 | Y | Y | Y | 1.6419 | 0.007327 | 0.1420 | 0.1359 | |
| 4 | BC-TiB | 1 | N | Y | Y | 1.1341 | 0.005450 | 0.0000 | 0.1584 | |
| 5 | BC-TiB | 2 | N | Y | Y | 1.1302 | 0.003949 | 0.0000 | 0.1232 | |
| 6 | BC-TiB | 3 | N | Y | Y | 1.2422 | 0.004801 | 0.0000 | 0.1480 | |
| 7 | BC-TiB | 1 | Y | N | Y | 1.1776 | 0.005507 | 0.0758 | 0.1128 | |
| 8 | BC-TiB | 2 | Y | N | Y | 1.0666 | 0.005629 | 0.0766 | 0.1064 | |
| 9 | BC-TiB | 3 | Y | N | Y | 1.1479 | 0.007116 | 0.0784 | 0.1122 | |
| 10 | BC-TiB | 1 | Y | Y | N | 1.8663 | 0.009083 | 0.1782 | 0.1478 | |
| 11 | BC-TiB | 2 | Y | Y | N | 1.7105 | 0.008339 | 0.1618 | 0.1323 | |
| 12 | BC-TiB | 3 | Y | Y | N | 1.8670 | 0.010320 | 0.1813 | 0.1662 | |
| 13 | BC | 1 | Y | N | N | 1.2460 | 0.008489 | 0.0707 | 0.1485 | |
| 14 | BC | 2 | Y | N | N | 1.1580 | 0.007554 | 0.0749 | 0.1392 | |
| 15 | BC | 3 | Y | N | N | 1.1903 | 0.006258 | 0.0612 | 0.1217 | |
| 16 | BC | 1 | N | N | N | 1.0212 | 0.006541 | 0.0000 | 0.2383 | |
| 17 | BC | 2 | N | N | N | 1.0240 | 0.005984 | 0.0000 | 0.2374 | |
| 18 | BC | 3 | N | N | N | 1.0203 | 0.006182 | 0.0000 | 0.2383 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Clayton, J.D. Modeling Deformation and Fracture of Boron-Based Ceramics with Nonuniform Grain and Phase Boundaries and Thermal-Residual Stress. Solids 2022, 3, 643-664. https://doi.org/10.3390/solids3040040
Clayton JD. Modeling Deformation and Fracture of Boron-Based Ceramics with Nonuniform Grain and Phase Boundaries and Thermal-Residual Stress. Solids. 2022; 3(4):643-664. https://doi.org/10.3390/solids3040040
Chicago/Turabian StyleClayton, John D. 2022. "Modeling Deformation and Fracture of Boron-Based Ceramics with Nonuniform Grain and Phase Boundaries and Thermal-Residual Stress" Solids 3, no. 4: 643-664. https://doi.org/10.3390/solids3040040
APA StyleClayton, J. D. (2022). Modeling Deformation and Fracture of Boron-Based Ceramics with Nonuniform Grain and Phase Boundaries and Thermal-Residual Stress. Solids, 3(4), 643-664. https://doi.org/10.3390/solids3040040






