Stress Distribution in Modified Veneer Crowns: 3D Finite Element Analysis
Abstract
:1. Introduction
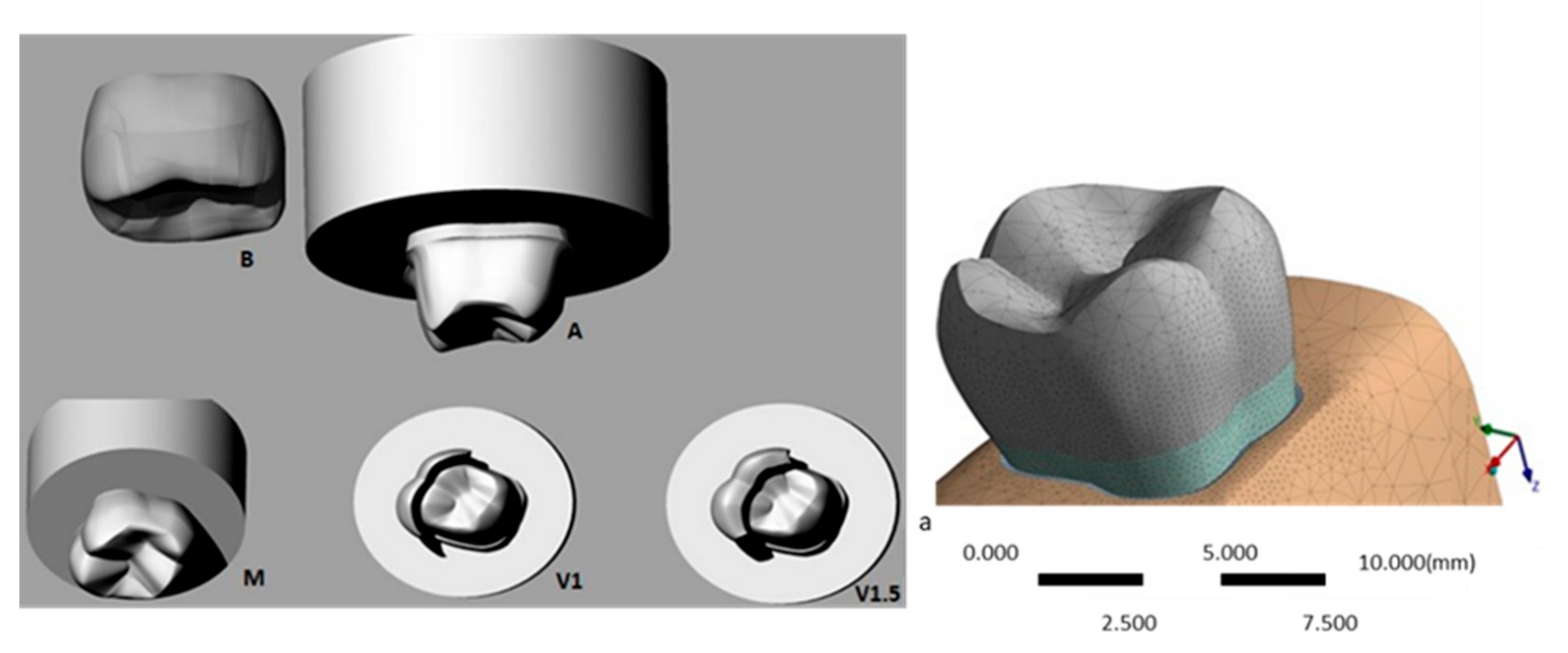
2. Materials and Methods
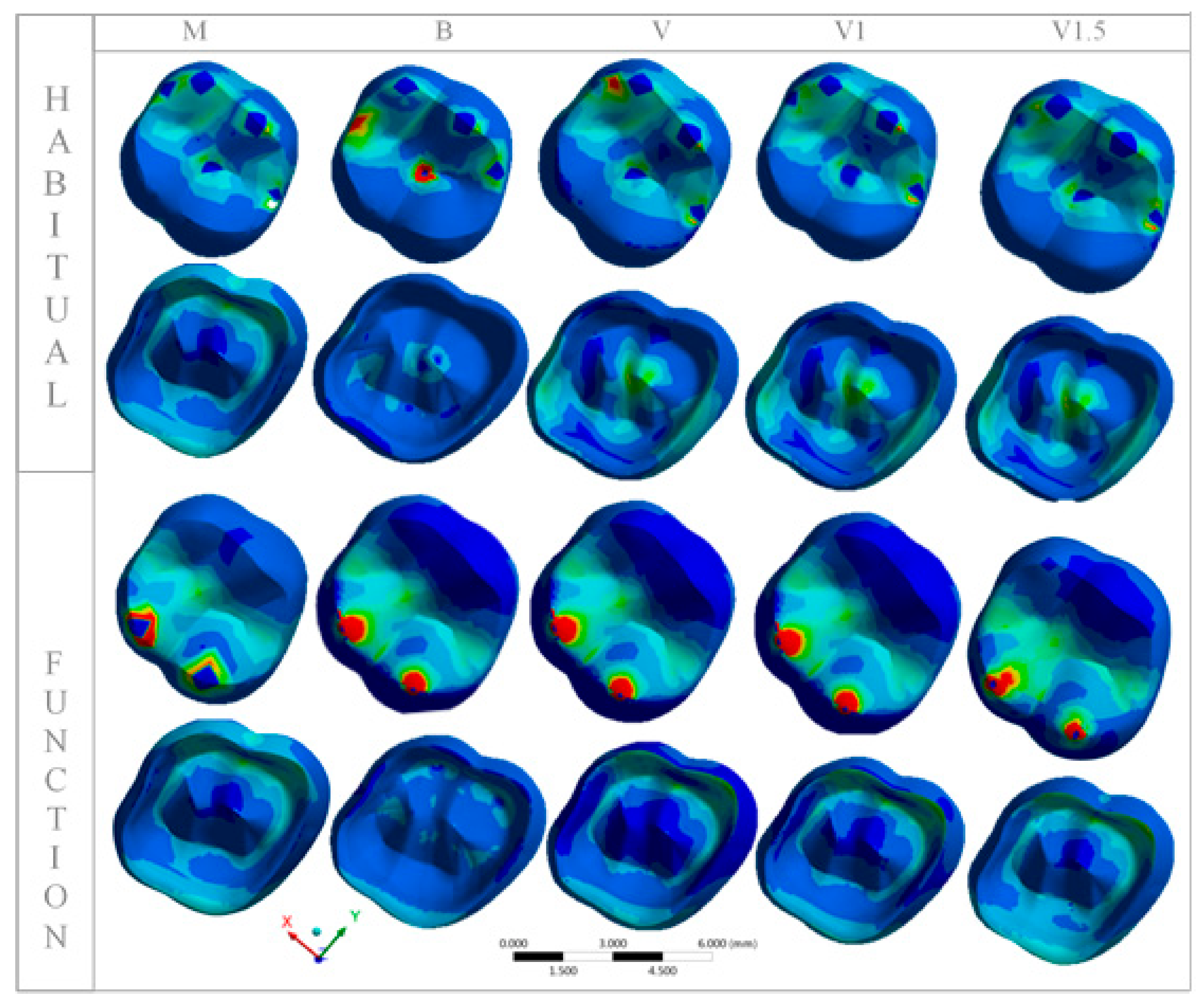
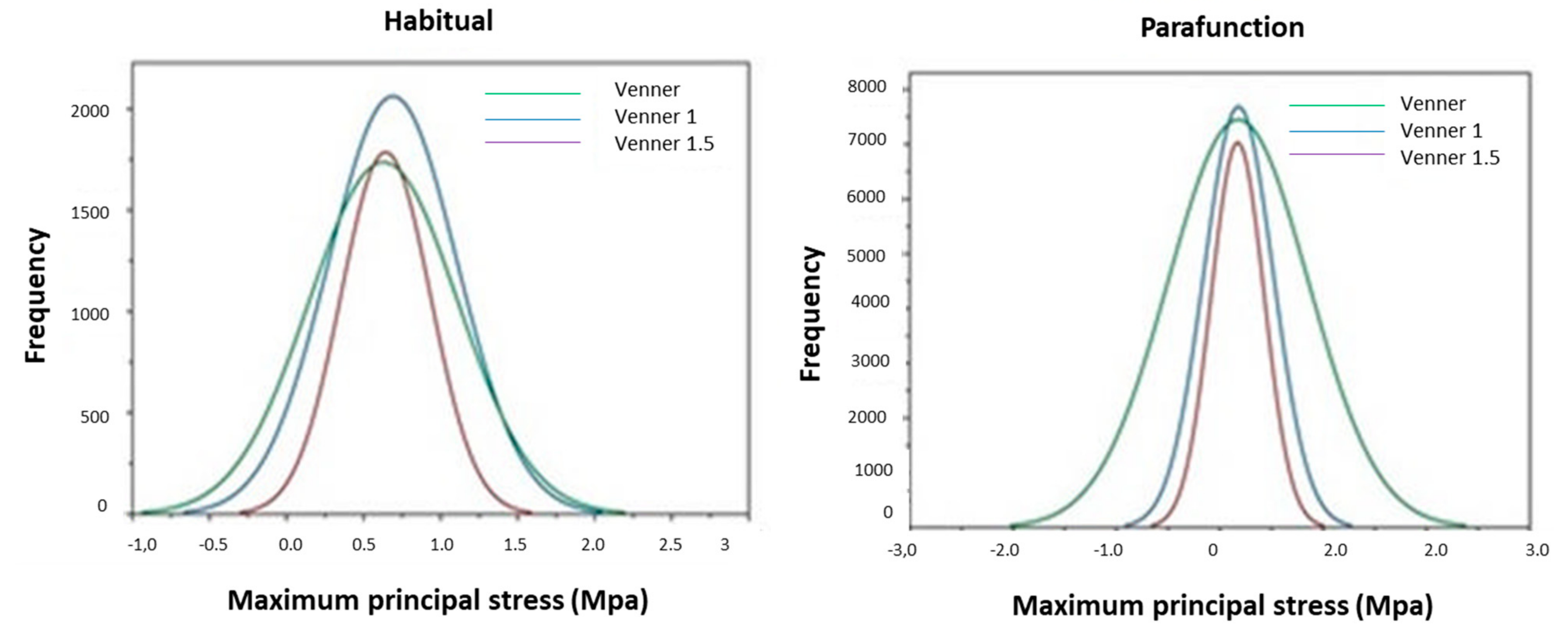
3. Results
Stress Concentration
4. Discussion
5. Conclusions
- (1)
- Crowns with veneer modifications exhibit similar biomechanical behavior to monolithic crowns and could be used in patients without parafunction.
- (2)
- The highly translucent zirconia ceramic only associated with buccal covering ceramic is a rigid system.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Braun, S.; Hnat, W.P.; Freudenthaler, J.W.; Marcotte, M.R.; Hönigle, K.; Johnson, B.E. A study of maximum bite force during growth and development. Angle Orthod. 1996, 66, 261–264. [Google Scholar]
- Tortopidis, D.; Lyons, M.F.; Baxendale, R.H.; Gilmour, W.H. The variability of bite force measurement between sessions, in different positions within the dental arch. J. Oral Rehabil. 1998, 25, 681–686. [Google Scholar] [CrossRef] [PubMed]
- Lawn, B.R.; Pajares, A.; Zhang, Y.; Deng, Y.; Polack, M.A.; Lloyd, I.K.; Rekow, E.D.; Thompson, V.P. Materials design in the performance of all-ceramiccrowns. Biomaterials 2004, 25, 2885–2892. [Google Scholar] [CrossRef] [PubMed]
- Raptis, N.V.; Michalakis, K.X.; Hirayama, H. Optical behavior of current ceramic systems. Int. J. Perio. Restor. Dent. 2006, 26, 31–41. [Google Scholar]
- Zhang, Y. Making yttria-stabilized tetragonal zirconiatranslucent. Dent. Mater. 2014, 30, 1195–1203. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Holand, W.; Apel, E.; Hoen, C.; Rheinberger, V. Studies of Crystal phase formations in high-strength lithium disilicate glass-ceramics. J. Non Cryst. Solids 2006, 352, 4041–4050. [Google Scholar] [CrossRef]
- Komine, F.; Blatz, M.B.; Matsumura, H. Current status of zirconia-based fixed restorations. J. Oral Sci. 2010, 52, 531–539. [Google Scholar] [CrossRef] [Green Version]
- Kang, S.H.; Chang, J.; Son, H.H. Flexural strength and microstructure of two lithium disilicate glass ceramics for CAD/CAM restoration in the dental clinic. Restor. Dent. Endod. 2013, 38, 134–140. [Google Scholar] [CrossRef]
- Sen, N. Mechanical and optical properties of monolithic CAD-CAM restorative materials. J. Prosthet. Dent. 2018, 119, 593–599. [Google Scholar] [CrossRef]
- Matsuzaki, F.; Sekine, H.; Honma, S.; Takanashi, T.; Furuya, K.; Yajimara, Y.; Youshinari, M. Translucency and flexural strength of monolithic translucent zirconia and porcelain-layered zirconia. Dent. Mater. 2015, 34, 910–917. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Chai, H.W.; Lee, J.J.; Lawn, B.R. Chipping Resistance of Graded Zirconia Ceramics for Dental Crowns. J. Dent. Res. 2012, 91, 311–315. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Benetti, P.; Kelly, J.R.; Sanchez, M.; Della Bona, A. Influence of thermal gradients on stress state of veneered restorations. Dent. Mater. 2014, 30, 554–563. [Google Scholar] [CrossRef]
- Lee, Y.K. Translucency of human teeth and dental restorative materials and its clinical relevance. J. Biomed. Opt. 2015, 20, 045002. [Google Scholar] [CrossRef]
- D’Arcangelo, C.; Vanini, L.; Rondoni, G.D.; De Angelis, F. Wear properties of dental ceramics and porcelains compared with human enamel. J. Prosthet. Dent. 2016, 115, 350–355. [Google Scholar] [CrossRef] [PubMed]
- Schwindling, F.S.; Rues, S.; Schmitter, M. Fracture resistance of glazed, full-contour ZLC incisor crowns. J. Prosthet. Dent. 2017, 61, 344–349. [Google Scholar]
- Harada, K.; Raigroski, A.J.; Chung, K.; Flinn, B.D.; Dogan, S.; Mancl, L.A. A comparative evaluation of the translucency of zirconias and lithium disilicate for monolithic restorations. J. Prosthet. Dent. 2016, 116, 257–263. [Google Scholar] [CrossRef] [PubMed]
- Ramos, G.F.; Monteiro, E.B.C.; Bottino, M.A.; Zhang, Y.; Melo, R.M.M. Failure probability of three designs of zirconia crowns. Int. J. Periodontics Restor. Dent. 2015, 35, 843–849. [Google Scholar] [CrossRef] [Green Version]
- Santos, G.C., Jr.; Boksman, L.L.; Santos, M.J. CAD/CAM technology and esthetic dentistry: A case report. Compend. Contin. Educ. Dent. 2013, 34, 764–768. [Google Scholar]
- Ender, A.; Mörmann, W.H.; Mehl, A. Efficiency of a mathematical model in generating CAD/CAM-partial crowns with natural tooth morphology. Clin. Oral Investig. 2011, 15, 283–289. [Google Scholar] [CrossRef] [Green Version]
- Arslan, Y.; Nemli, S.L.; Güngör, M.B.; Tamam, E.; Yilmaz, H. Evaluation of biogeneric design techniques with CEREC CAD/CAM system. J. Adv. Prosthodont. 2015, 7, 431–436. [Google Scholar] [CrossRef]
- Demkowicz, L. Computing with hp-adaptive finite elements. In One and Two Dimensional Elliptic and Maxwell Problems; Chapman & Hall/CRC: Boca Raton, FL, USA, 2007; Volume 1. [Google Scholar]
- Demkowicz, L.; Kurtz, J.; Pardo, D.; Paszyński, M.; Rachowicz, W.; Zdunek, A. Computing with hp adaptive finite elements. In Three-Dimensional Elliptic and Maxwell Problems with Applications; Chapman & Hall/CRC: Boca Raton, FL, USA, 2007; Volume 2. [Google Scholar]
- Zboiński, G. Adaptive hpq finite element methods for the analysis of 3D-based models of complex structures. Part 1. Hierarchical modeling and approximation. Comput. Methods Appl. Mech. Engrg. 2010, 199, 2913–2940. [Google Scholar] [CrossRef]
- Zboiński, G. Adaptive hpq finite element methods for the analysis of 3D-based models of complex structures. Part 2. A posteriori error estimation. Comput. Methods Appl. Mech. Eng. 2013, 267, 531–565. [Google Scholar] [CrossRef]
- Tribst, J.P.M.; Dal Piva, A.M.O.; Madruga, C.F.L.; Valera, M.C.; Borges, A.L.S.; Bresciani, E.; de Melo, R.M. Endocrown restorations: Influence of dental remnant and restorative material on stress distribution. Dent. Mater. 2018, 34, 1466–1473. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Versluis, A.; Tantbirojn, D.; Pintado, M.R.; DeLong, R.; Douglas, W.H. Residual shrinkage stress distributions in molars after composite restoration. Dent. Mater. 2004, 20, 554–564. [Google Scholar] [CrossRef] [PubMed]
- Dal Piva, A.M.O.; Tribst, J.P.M.; Souza, R.O.A.; Borges, A.L.S. Influence of Alveolar Bone Loss and Cement Layer Thickness on the Biomechanical Behavior of Endodontically Treated Maxillary Incisors: A 3-dimensional Finite Element Analysis. J. Endod. 2017, 43, 791–795. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- De Jager, N.; Pallav, P.; Feilzer, A.J. The influence of design parameters on the FEA-determined stress distribution in CAD-CAM produced all-ceramic dental crowns. Dent. Mater. 2005, 21, 242–251. [Google Scholar] [CrossRef]
- Sailer, I.; Feher, A.; Filser, F.; Gauckler, L.J.; Lüthy, H.; Hämmerle, C.H. Five-year clinical results of zirconia frameworks for posterior fixed partial dentures. Int. J. Prosthod. 2007, 20, 383–388. [Google Scholar]
- Silva, N.R.; Bonfante, E.A.; Zavanelli, R.A.; Thompson, V.P.; Ferencz, J.L.; Coelho, P.G. Reliability of metalloceramic and zirconia-based ceramic crowns. J. Dent. Res. 2010, 89, 1051–1056. [Google Scholar] [CrossRef] [PubMed]
- Christensen, R.P.; Ploeger, B.J. A clinical comparison of zirconia, metal and alumina fixed-prosthesis frameworks veneered with layered or pressed ceramic: A three-year report. J. Am. Dent Assoc. 2010, 141, 1317–1329. [Google Scholar] [CrossRef]
- Zhang, Y.; Sailer, I.; Lawn, B.R. Fatigue of dental ceramics. J. Dent. 2013, 41, 1135–1147. [Google Scholar] [CrossRef] [Green Version]
- Corazza, P.H.; Feitosa, A.S.; Borges, A.L.; Della Bona, A. Influence of convergence angle of tooth preparation on the fracture resistance of Y-TZP-based all-ceramic restorations. Dent. Mater. 2013, 29, 339–347. [Google Scholar] [CrossRef] [PubMed]
- Van Ejjden, T.M. Three-dimensional analyses of human bite-force magnitude and moment. Arch. Oral Biol. 1991, 36, 535–539. [Google Scholar] [CrossRef]
- Varga, S.; Spalj, S.; Varga, M.L.; Milosevic, A.S.; Mestrovic, S.; Slaj, M. Maximum voluntary molar bite force in subjects with normal occlusion. Eur. J. Orthod. 2011, 33, 427–433. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kollmuss, M.; Jakob, F.M.; Kirchner, H.G.; Ilie, N.; Hickel, R.; Huth, K.C. Comparison of biogenerically reconstructed and waxedup complete occlusal surfaces with respect to the original tooth morphology. Clin. Oral Investig. 2013, 17, 851–857. [Google Scholar] [CrossRef]
- Bonfante, E.A.; Rafferty, B.; Zavanelli, R.A.; Silva, N.R.F.A.; Rekow, E.D.; Thompson, V.P.; Coelho, P.G. Thermal/mechanical simulation and laboratory fatigue testing of an alternative yttria tetragonal zirconia polycrystal core-veneer all-ceramic layered crown design. Eur. J. Oral Sci. 2010, 118, 202–209. [Google Scholar] [CrossRef]
- Baldassarri, M.; Stappert, C.F.; Wolff, M.S.; Thompson, V.P.; Zhang, Y. Residual stresses in porcelain-veneered zirconia prostheses. Dent. Mater. 2012, 28, 873–879. [Google Scholar] [CrossRef] [Green Version]
- Lucas, T.J.; Lawsona, N.C.; Janowski, G.M.; Burgess, J.O. Effect of grain size on the monoclinic transformation, hardness, roughness, and modulus of aged partially stabilized zirconia. Dent. Mater. 2015, 31, 1487–1492. [Google Scholar] [CrossRef]
- Campo Silva, E.; Leonec, R.; Gremillarda, L.; Sorrentinoc, R.; Zaronec, F.; Ferrarid, M.; Chevaliera, J. Aging resistance, mechanical properties and translucency of different yttria-stabilized zirconia ceramics for monolithic dental crown applications. Dent. Mater. 2018, 34, 879–890. [Google Scholar] [CrossRef]

| Material | Elastic Modulus (GPa) | Poisson | References |
|---|---|---|---|
| Enamel | 84 | 0.30 | [25] |
| Dentin | 18.6 | 0.23 | [25] |
| Periodontal ligament | 0.069 | 0.45 | [26] |
| Polyurethane | 3.6 | 0.3 | [26] |
| IPS e.max Ceram (lithium disilicate ceramic) | 65 | 0.23 | Ivoclar Vivadent AG Schaan (Liechtenstein, German) |
| IPS e.max ZirCAD MT (zirconia ceramic) | 210 | 0.29 | Ivoclar Vivadent AG Schaan (Liechtenstein, German) |
| Groups | Habitual | Function |
|---|---|---|
| Group M—Occlusal | 43.68 | 113.04 |
| Group M—Internal | 25.01 | 19.84 |
| Group B—Occlusal | 87.66 | 370.03 |
| Group B—Internal | 6.67 | 7.40 |
| Group V—Occlusal | 54.84 | 2000.00 |
| Group V—Internal | 26.66 | 25.11 |
| Group V1—Occlusal | 51.14 | 1032.00 |
| Group V1—Internal | 26.01 | 25.84 |
| Group V1.5—Occlusal | 50.14 | 865 |
| Group V1.5—Internal | 27.02 | 26.14 |
| Groups | Habitual | Function |
|---|---|---|
| Group V—Occlusal | 4.19 | 747.00 |
| Group V—Internal | 3.80 | 530.00 |
| Group V1—Occlusal | 5.35 | 1031.00 |
| Group V1—Internal | 3.38 | 59.00 |
| Group V 1.5—Occlusal | 5.79 | 865.00 |
| Group V1.5—Internal | 3.40 | 26.47 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Madruga, C.F.L.; Ramos, G.F.; Borges, A.L.S.; Saavedra, G.d.S.F.A.; Souza, R.O.; Marinho, R.M.d.M.; Penteado, M.M. Stress Distribution in Modified Veneer Crowns: 3D Finite Element Analysis. Oral 2021, 1, 272-280. https://doi.org/10.3390/oral1030026
Madruga CFL, Ramos GF, Borges ALS, Saavedra GdSFA, Souza RO, Marinho RMdM, Penteado MM. Stress Distribution in Modified Veneer Crowns: 3D Finite Element Analysis. Oral. 2021; 1(3):272-280. https://doi.org/10.3390/oral1030026
Chicago/Turabian StyleMadruga, Camila Ferreira Leite, Gabriela Freitas Ramos, Alexandre Luiz Souto Borges, Guilherme de Siqueira Ferreira Anzaloni Saavedra, Rodrigo Othávio Souza, Renata Marques de Melo Marinho, and Marcela Moreira Penteado. 2021. "Stress Distribution in Modified Veneer Crowns: 3D Finite Element Analysis" Oral 1, no. 3: 272-280. https://doi.org/10.3390/oral1030026
APA StyleMadruga, C. F. L., Ramos, G. F., Borges, A. L. S., Saavedra, G. d. S. F. A., Souza, R. O., Marinho, R. M. d. M., & Penteado, M. M. (2021). Stress Distribution in Modified Veneer Crowns: 3D Finite Element Analysis. Oral, 1(3), 272-280. https://doi.org/10.3390/oral1030026





