Simulation of M2 Profiles in a Channel with Rigid Emergent Vegetation †
Abstract
1. Introduction
2. Theory
2.1. Overview and Basic Definition
2.2. Existing Predictors of Drag Coefficient
3. Experimental Data
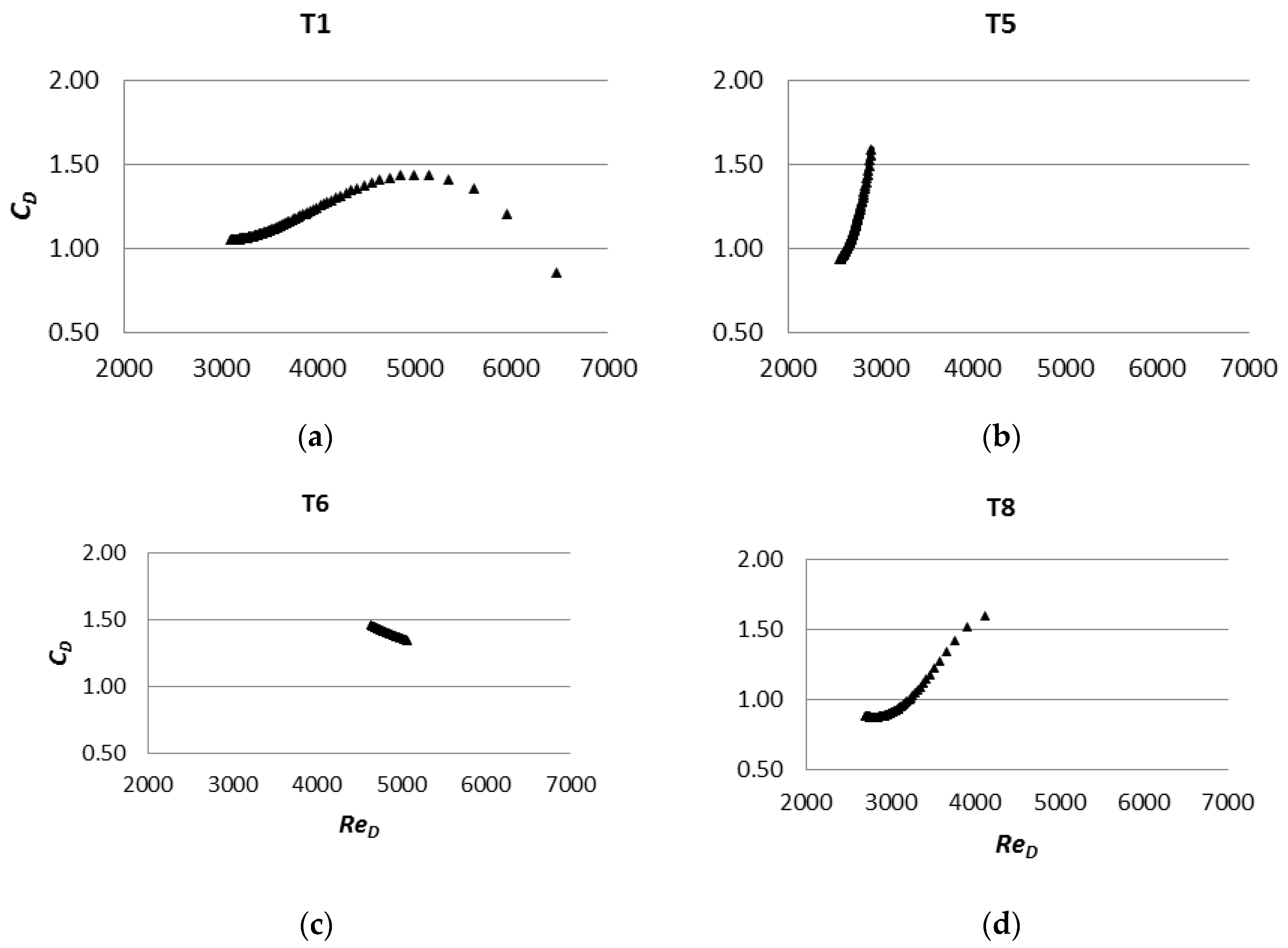
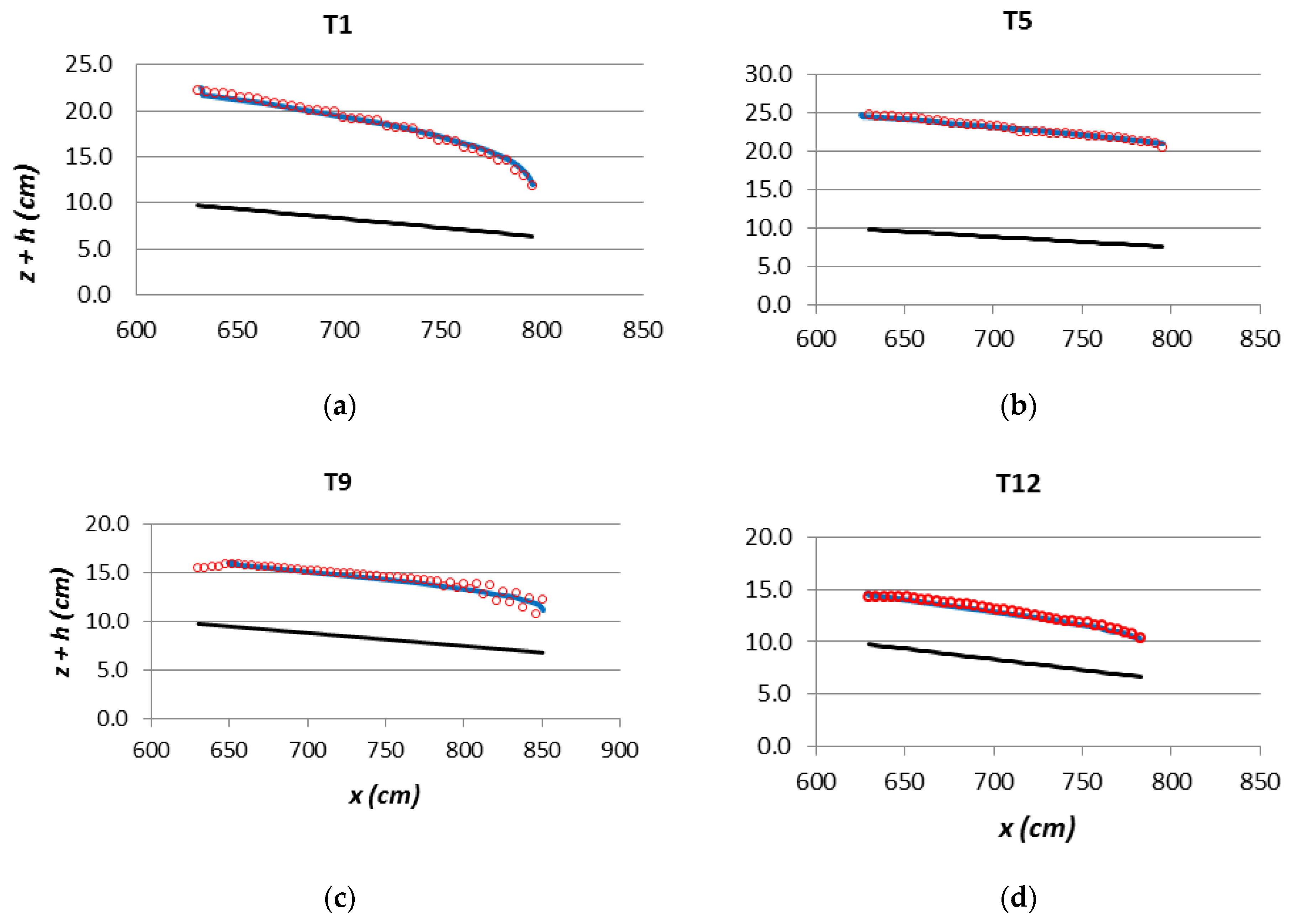
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, W.-J.; Huai, W.-X.; Thompson, S.; Katul, G.G. Steady nonuniform shallow flow within emergent vegetation. Water Resour. Res. 2015, 51, 10047–10064. [Google Scholar] [CrossRef]
- Liu, M.Y.; Huai, W.X.; Yang, Z.H.; Zeng, Y.H. A genetic programming-based model for drag coefficient of emergent vegetation in open channel flows. Adv. Water Resour. 2020, 140, 103582. [Google Scholar] [CrossRef]
- Bonilla-Porras, J.A.; Armanini, A.; Crosato, A. Extended Einstein’s parameters to include vegetation in existing bedload predictors. Adv. Water Resour. 2021, 152, 103928. [Google Scholar] [CrossRef]
- Nepf, H.M. Hydrodynamics of vegetated channels. J. Hydraul. Res. 2012, 50, 262–279. [Google Scholar] [CrossRef]
- Green, J.C. Comparison of blockage factors in modelling the resistance of channels containing submerged macrophytes. River Res. Applic. 2005, 21, 671–686. [Google Scholar] [CrossRef]
- Caroppi, G.; Västilä, K.; Järvelä, J.; Rowiński, P.M.; Giugni, M. Turbulence at water-vegetation interface in open channel flow: Experiments with natural-like plants. Adv. Water Resour. 2019, 127, 180–191. [Google Scholar] [CrossRef]
- Penna, N.; Coscarella, F.; D’Ippolito, A.; Gaudio, R. Bed roughness effects on the turbulence characteristics of flows through emergent rigid vegetation. Water 2020, 12, 2401. [Google Scholar] [CrossRef]
- Kazem, M.; Afzalimehr, H.; Sui, J. Characteristics of turbulence in the downstream region of a vegetation patch. Water 2021, 13, 3468. [Google Scholar] [CrossRef]
- Penna, N.; Coscarella, F.; D’Ippolito, A.; Gaudio, R. Effects of fluvial instability on the bed morphology in vegetated channels. Environ. Fluid Mech. 2022, 22, 619–644. [Google Scholar] [CrossRef]
- Vargas-Luna, A.; Crosato, A.; Calvani, G.; Uijttewaal, W.S.J. Representing plants as rigid cylinders in experiments and models. Adv. Water Resour. 2016, 93, 205–222. [Google Scholar] [CrossRef]
- Lama, G.F.C.; Crimaldi, M.; Pasquino, V.; Padulano, R.; Chirico, G.B. Bulk drag predictions of riparian arundo donax stands through UAV-acquired multispectral images. Water 2021, 13, 1333. [Google Scholar] [CrossRef]
- Caroppi, G.; Västilä, K.; Järvelä, J.; Lee, C.; Ji, U.; Kim, H.S.; Kim, S. Flow and wake characteristics associated with riparian vegetation patches: Result from field-scale experiments. Hydrol. Process. 2022, 36, e14506. [Google Scholar] [CrossRef]
- Sturm, T.W. Open Channel Hydraulics, 2nd ed.; McGraw-Hill: New York, NY, USA, 2010; p. 546. [Google Scholar]
- Rowinski, P.M.; Kubrak, J. A mixing-length model for predicting vertical velocity distribution in flows through emergent vegetation. Hydrol. Sci. J. 2002, 47, 893–904. [Google Scholar] [CrossRef]
- Cheng, N.S. Calculation of drag coefficient for array of emergent circular cylinder with pseudofluid model. J. Hydraul. Eng. 2013, 139, 602–611. [Google Scholar] [CrossRef]
- Sonnenwald, F.; Stovin, V.; Guymer, I. Estimating drag coefficient for arrays of rigid cylinders representing emergent vegetation. J. Hydraul. Res. 2019, 47, 591–597. [Google Scholar] [CrossRef]
- D’Ippolito, A.; Calomino, F.; Alfonsi, G.; Lauria, A. Flow Resistance in Open Channel Due to Vegetation at Reach Scale: A Review. Water 2021, 13, 116. [Google Scholar] [CrossRef]
- D’Ippolito, A.; Calomino, F.; Alfonsi, G.; Lauria, A. Drag Coefficient of in-line emergent vegetation in open channel flow. Int. J. River Basin Manag. 2021. [Google Scholar] [CrossRef]
- Cheng, N.S.; Nguyen, H.T. Hydraulic radius for evaluating resistance induced by simulated emergent vegetation in open-channel flow. J. Hydraul. Eng. 2011, 137, 995–1004. [Google Scholar] [CrossRef]
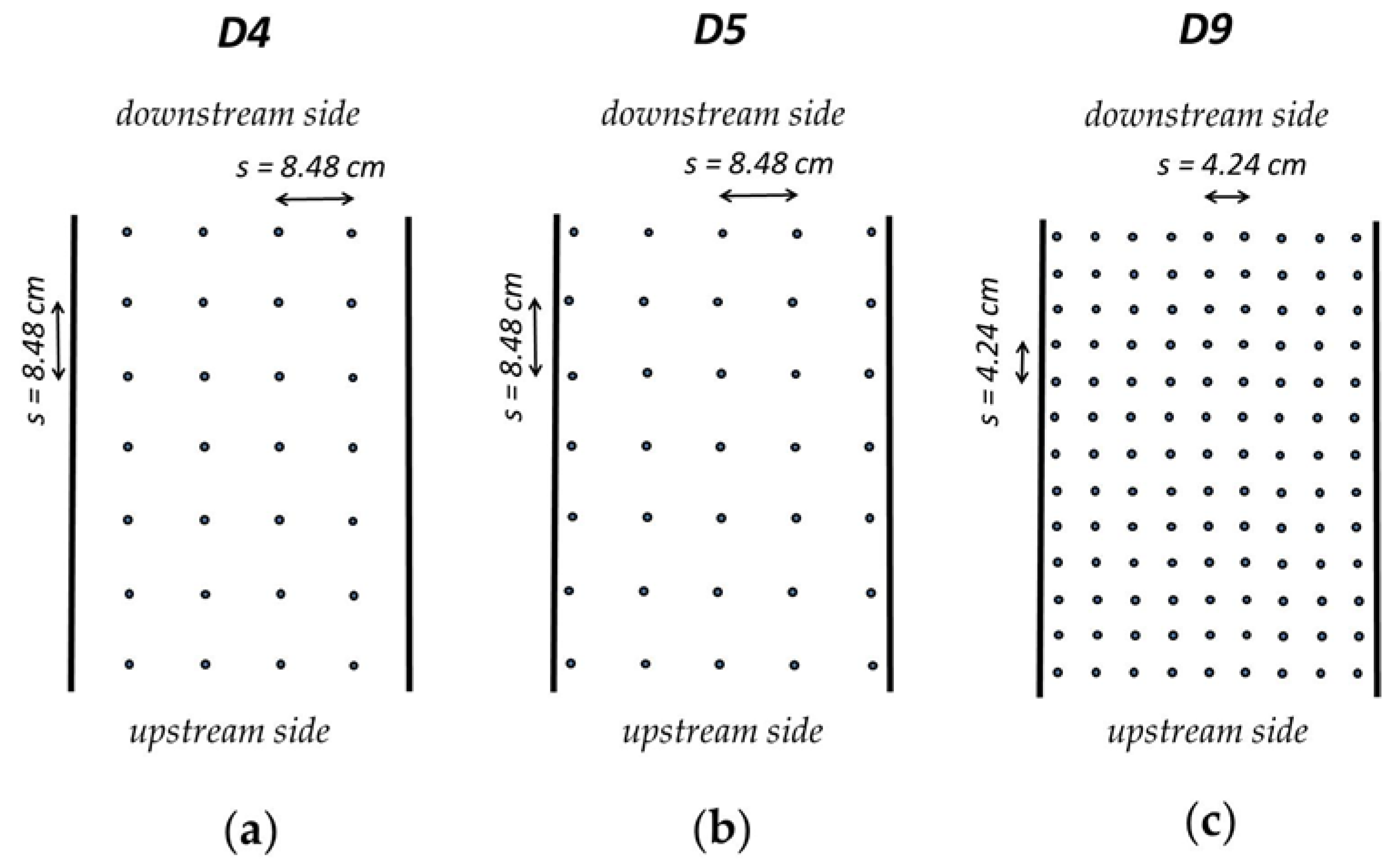

| Test n. | Arrangement | Q (L/s) | i % | d (mm) | Profile Type | |
|---|---|---|---|---|---|---|
| T1 | D9 | 13.55 | 2.02 | 10 | 4.36 | M2 |
| T2 | D9 | 16.39 | 2.02 | 10 | 4.36 | M2 |
| T3 | D9 | 7.90 | 1.35 | 10 | 4.36 | M2 |
| T4 | D9 | 10.96 | 1.35 | 10 | 4.36 | M2 |
| T5 1 | D9 | 13.77 | 1.35 | 10 | 4.36 | M2 |
| T6 | D5 | 16.31 | 2.02 | 10 | 1.09 | M3-M2 |
| T7 | D4 | 10.96 | 1.35 | 10 | 1.09 | M3-M2 |
| T8 | D9 | 8.60 | 2.02 | 8 | 2.79 | M2 |
| T9 | D5 | 10.92 | 1.35 | 10 | 1.09 | M2 |
| T10 | D9 | 5.29 | 1.35 | 10 | 4.36 | M2 |
| T11 1 | D9 | 14.62 | 0.48 | 8 | 2.79 | M2 |
| T12 | D9 | 5.77 | 2.02 | 8 | 2.79 | M2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
D’Ippolito, A.; Calomino, F. Simulation of M2 Profiles in a Channel with Rigid Emergent Vegetation. Environ. Sci. Proc. 2022, 21, 73. https://doi.org/10.3390/environsciproc2022021073
D’Ippolito A, Calomino F. Simulation of M2 Profiles in a Channel with Rigid Emergent Vegetation. Environmental Sciences Proceedings. 2022; 21(1):73. https://doi.org/10.3390/environsciproc2022021073
Chicago/Turabian StyleD’Ippolito, Antonino, and Francesco Calomino. 2022. "Simulation of M2 Profiles in a Channel with Rigid Emergent Vegetation" Environmental Sciences Proceedings 21, no. 1: 73. https://doi.org/10.3390/environsciproc2022021073
APA StyleD’Ippolito, A., & Calomino, F. (2022). Simulation of M2 Profiles in a Channel with Rigid Emergent Vegetation. Environmental Sciences Proceedings, 21(1), 73. https://doi.org/10.3390/environsciproc2022021073






