Innovative Approach for Selection of Pump as Turbine in Water Distribution Network †
Abstract
1. Introduction
2. Methodology
2.1. PAT Layouts
2.2. PAT Operation Model
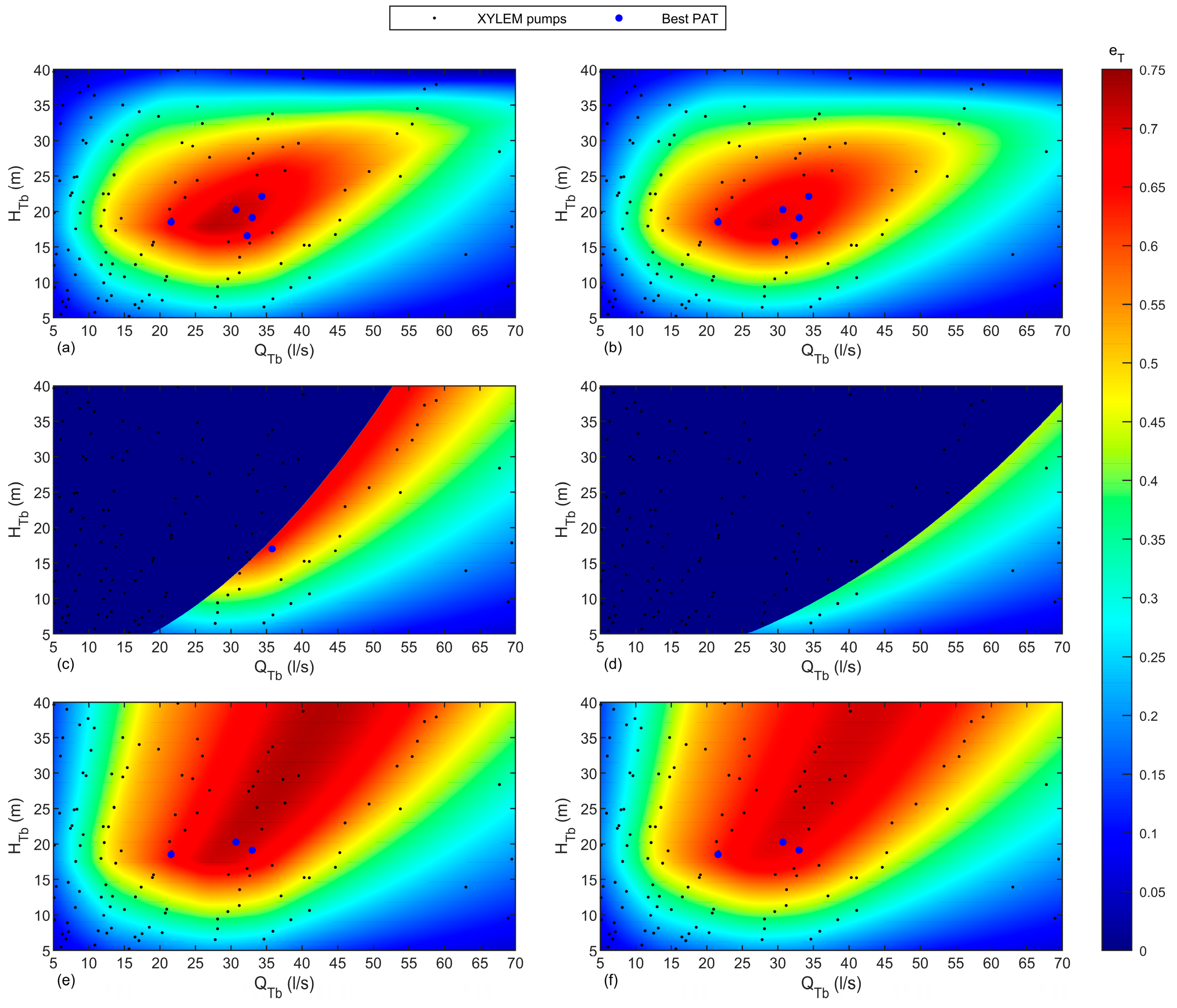
2.3. PAT Selection
2.4. Cost Model
3. Application
4. Results
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Farley, M.; Trow, S. Losses in Water Distribution Networks; IWA Publishing: London, UK, 2003; ISBN 1900222116. [Google Scholar]
- Xu, Q.; Chen, Q.; Ma, J.; Blanckaert, K.; Wan, Z. Water Saving and Energy Reduction through Pressure Management in Urban Water Distribution Networks. Water Resour. Manag. 2014, 28, 3715–3726. [Google Scholar] [CrossRef]
- Nazif, S.; Karamouz, M.; Tabesh, M.; Moridi, A. Pressure Management Model for Urban Water Distribution Networks. Water Resour. Manag. 2010, 24, 437–458. [Google Scholar] [CrossRef]
- de Paola, F.; Giugni, M. Leakages and Pressure Relation: An Experimental Research. Drink. Water Eng. Sci. 2012, 5, 59–65. [Google Scholar] [CrossRef]
- Fontana, N.; Giugni, M.; Glielmo, L.; Marini, G.; Zollo, R. Operation of a Prototype for Real Time Control of Pressure and Hydropower Generation in Water Distribution Networks. Water Resour. Manag. 2019, 33, 697–712. [Google Scholar] [CrossRef]
- Fontana, N.; Eng, C.; Asce, M.; Giugni, M.; Glielmo, L.; Marini, G. Real Time Control of a Prototype for Pressure Regulation and Energy Production in Water Distribution Networks. J. Water Resour. Plan. Manag. 2016, 142, 04016015. [Google Scholar] [CrossRef]
- Fontana, N.; Marini, G. A Methodology to Assess Optimal Operation of a Prototype for Pressure Regulation and Hydropower Generation. J. Water Resour. Plan. Manag. 2021, 147, 04021088. [Google Scholar] [CrossRef]
- Fontana, N.; Giugni, M.; Glielmo, L.; Marini, G.; Zollo, R. Use of Hydraulically Operated PRVs for Pressure Regulation and Power Generation in Water Distribution Networks. J. Water Resour. Plan. Manag. 2020, 146, 04020047. [Google Scholar] [CrossRef]
- Sharma, K. Small Hydroelectric Project-Use of Centrifugal Pumps as Turbines; Kirloskar Electric Co.: Bangalore, India, 1985. [Google Scholar]
- Fernández García, I.; Mc Nabola, A. Maximizing Hydropower Generation in Gravity Water Distribution Networks: Determining the Optimal Location and Number of Pumps as Turbines. J. Water Resour. Plan. Manag. 2020, 146, 04019066. [Google Scholar] [CrossRef]
- Carravetta, A.; del Giudice, G.; Fecarotta, O.; Ramos, H.M. Energy Production in Water Distribution Networks: A PAT Design Strategy. Water Resour. Manag. 2012, 26, 3947–3959. [Google Scholar] [CrossRef]
- García, I.F.; Novara, D.; Nabola, A.M. A Model for Selecting the Most Cost-Effective Pressure Control Device for More Sustainable Water Supply Networks. Water 2019, 11, 1297. [Google Scholar] [CrossRef]
- Williams, A.A. WREX 1996 Pumps as Turbines for Low Cost Micro I-Iydro Power; Nottingham Trent University: Nottingham, UK, 1996. [Google Scholar]
- Derakhshan, S.; Nourbakhsh, A. Experimental Study of Characteristic Curves of Centrifugal Pumps Working as Turbines in Different Specific Speeds. Exp. Therm. Fluid Sci. 2008, 32, 800–807. [Google Scholar] [CrossRef]
- Pugliese, F.; de Paola, F.; Fontana, N.; Giugni, M.; Marini, G. Experimental Characterization of Two Pumps As Turbines for Hydropower Generation. Renew. Energy 2016, 99, 180–187. [Google Scholar] [CrossRef]
- Nautiyal, H.; Varun; Kumar, A. Reverse Running Pumps Analytical, Experimental and Computational Study: A Review. Renew. Sustain. Energy Rev. 2010, 14, 2059–2067. [Google Scholar] [CrossRef]
- Fontana, N.; Asce, M.; Marini, G.; Creaco, E. Comparison of PAT Installation Layouts for Energy Recovery from Water Distribution Networks. J. Water Resour. Plan. Manag. 2021, 147, 04021083. [Google Scholar] [CrossRef]
- Lima, G.M.; Luvizotto, E.; Brentan, B.M. Selection and Location of Pumps as Turbines Substituting Pressure Reducing Valves. Renew. Energy 2017, 109, 392–405. [Google Scholar] [CrossRef]
- Carravetta, A.; del Giudice, G.; Fecarotta, O.; Ramos, H.M. PAT Design Strategy for Energy Recovery in Water Distribution Networks by Electrical Regulation. Energies 2013, 6, 411–424. [Google Scholar] [CrossRef]
- Alberizzi, J.C.; Renzi, M.; Nigro, A.; Rossi, M. Study of a Pump-as-Turbine (PaT) Speed Control for a Water Distribution Network (WDN) in South-Tyrol Subjected to High Variable Water Flow Rates. Energy Procedia 2018, 148, 226–233. [Google Scholar] [CrossRef]
- Stefanizzi, M.; Capurso, T.; Balacco, G.; Binetti, M.; Torresi, M.; Camporeale, S.M. Pump as Turbine for Throttling Energy Recovery in Water Distribution Networks. In Proceedings of the AIP Conference Proceedings; American Institute of Physics Inc.: College Park, MD, USA, 2019; Volume 2191. [Google Scholar]
- Novara, D.; Carravetta, A.; McNabola, A.; Ramos, H.M. Cost Model for Pumps as Turbines in Run-of-River and In-Pipe Microhydropower Applications. J. Water Resour. Plan. Manag. 2019, 145, 04019012. [Google Scholar] [CrossRef]
- de Marchis, M.; Fontanazz, C.M.; Freni, G.; Messineo, A.; Milici, B.; Napoli, E.; Notaro, V.; Puleo, V.; Scopa, A. Energy Recovery in Water Distribution Networks. Implementation of Pumps as Turbine in a Dynamic Numerical Model. In Proceedings of the Procedia Engineering; Elsevier Ltd.: Amsterdam, The Netherlands, 2014; Volume 70, pp. 439–448. [Google Scholar]
- Fontana, N.; Giugni, M.; Portolano, D. Losses Reduction and Energy Production in Water-Distribution Networks. ASCE 2012, 138, 237–244. [Google Scholar] [CrossRef]
- Fontana, N.; Giugni, M.; Glielmo, L.; Marini, G.; Zollo, R. Real-Time Control of Pressure for Leakage Reduction in Water Distribution Network: Field Experiments. J. Water Resour. Plan. Manag. 2018, 144, 04017096. [Google Scholar] [CrossRef]
- Fontana, N.; Giugni, M.; Glielmo, L.; Marini, G.; Zollo, R. Hydraulic and Electric Regulation of a Prototype for Real-Time Control of Pressure and Hydropower Generation in a Water Distribution Network. J. Water Resour. Plan. Manag. 2018, 144, 04018072. [Google Scholar] [CrossRef]
- Chapallaz, J.-M.; Eichenberger, P.; Fischer, G. Manual on Pumps Used as Turbines; Deutsche Gesellschaft fuer Technische Zusammenarbeit GmbH (GTZ): Eschborn, Germany, 1992. [Google Scholar]
- ABB (Asea Brown Boveri). ABB Industrial Drives Technical Catalogue; ABB: Zurich, Switzerland, 2007. [Google Scholar]
- DM 04.07.2019 Incentivazione Dell’energia Elettrica Prodotta Dagli Impianti Eolici on Shore, Solari Fotovoltaici, Idroelettrici e a Gas Residuati Dei Processi Di Depurazione. Italy. Available online: https://www.gazzettaufficiale.it/eli/id/2019/08/09/19A05099/sg (accessed on 12 May 2022).
- Saidur, R.; Mekhilef, S.; Ali, M.B.; Safari, A.; Mohammed, H.A. Applications of Variable Speed Drive (VSD) in Electrical Motors Energy Savings. Renew. Sustain. Energy Rev. 2012, 16, 543–550. [Google Scholar] [CrossRef]
- Xylem. 2019 E-NSC Series Catalogue, Cod. 191002950; Xylem Water Solutions Italia S.R.L.: Lainate, Italy, 2019. [Google Scholar]



| Layout | Pattern | Pump ID | (l/s) | (m) | (-) | (1/s) | (m) | (EUR) | (kWh/day) |
|---|---|---|---|---|---|---|---|---|---|
| LAY1 | Weekday | e-NSC 65-125/55 | 25.61 | 15.43 | 0.80 | 30.71 | 20.25 | 11,822.62 | 62.05 |
| long-period | e-NSC 65-200/40 | 18.05 | 14.17 | 0.80 | 21.57 | 18.52 | 11,043.93 | 55.53 | |
| LAY2 | Weekday | e-NSC 80-200/55 | 30.68 | 13.5 | 0.82 | 35.79 | 17.00 | 9896.61 | 56.28 |
| long-period | \ | \ | \ | \ | \ | \ | \ | \ | |
| LAY3 | Weekday | e-NSC 65-125/55 | 25.61 | 15.43 | 0.80 | 30.71 | 20.25 | 7592.04 | 62.48 |
| long-period | e-NSC 65-200/40 | 18.05 | 14.17 | 0.80 | 21.57 | 18.52 | 7051.24 | 55.08 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marini, G.; Maio, M.; Di Menna, F.; Pugliese, F.; De Paola, F.; Fontana, N. Innovative Approach for Selection of Pump as Turbine in Water Distribution Network. Environ. Sci. Proc. 2022, 21, 25. https://doi.org/10.3390/environsciproc2022021025
Marini G, Maio M, Di Menna F, Pugliese F, De Paola F, Fontana N. Innovative Approach for Selection of Pump as Turbine in Water Distribution Network. Environmental Sciences Proceedings. 2022; 21(1):25. https://doi.org/10.3390/environsciproc2022021025
Chicago/Turabian StyleMarini, Gustavo, Marco Maio, Francesco Di Menna, Francesco Pugliese, Francesco De Paola, and Nicola Fontana. 2022. "Innovative Approach for Selection of Pump as Turbine in Water Distribution Network" Environmental Sciences Proceedings 21, no. 1: 25. https://doi.org/10.3390/environsciproc2022021025
APA StyleMarini, G., Maio, M., Di Menna, F., Pugliese, F., De Paola, F., & Fontana, N. (2022). Innovative Approach for Selection of Pump as Turbine in Water Distribution Network. Environmental Sciences Proceedings, 21(1), 25. https://doi.org/10.3390/environsciproc2022021025










