Microgrid Protection through Adaptive Overcurrent Relay Coordination
Abstract
1. Introduction
- We propose a protection mechanism that handles the two types of disturbances caused by the fault current variations.
- We develop a new technique to identify the MG mode of operation using the DG measurements from the power flow analysis, without reliance on communication with the main feeder.
- We introduce a novel MG protection strategy based on the correlation of primary/backup relay pairs. The approach identifies the impacted area with the minimum number of relays to be readjusted.
- We mathematically formulate the overcurrent relay coordination problem; the optimization is accurately identifying the boundaries of a fault containment zone with the least number of relays involved in.
2. Related Work
2.1. Distance-Based Protection
2.2. Differential Protection
2.3. Overcurrent Protection
2.4. Adaptive and Pre-Planned Protection
3. System Model and Preliminaries
3.1. Microgrid System Model
3.1.1. Power Source
3.1.2. Transmission Lines
3.1.3. Load Model
3.2. Protection System Model
4. Microgrid Protection System
4.1. Approach Overview
4.2. Identifying MG Mode of Operation
4.3. Power Flow Optimization (Phase I)
4.4. Containment Zone Determination (Phase II)
4.5. Relay Coordination Optimization (Phase II)
4.5.1. DOCR Operating Time Formulation
Objective Function
Constraints
5. Approach Validation
5.1. Validation Setup
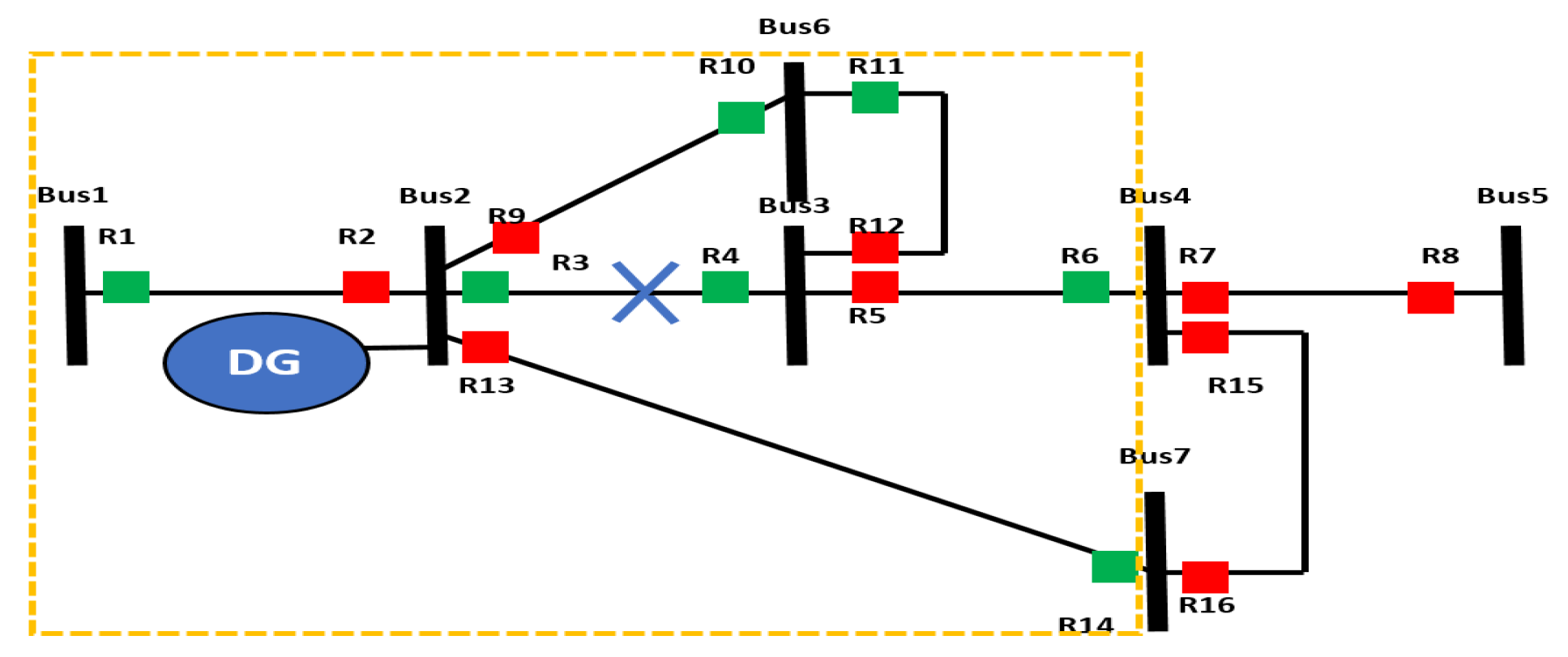
5.2. Handling Tolerable Disturbances
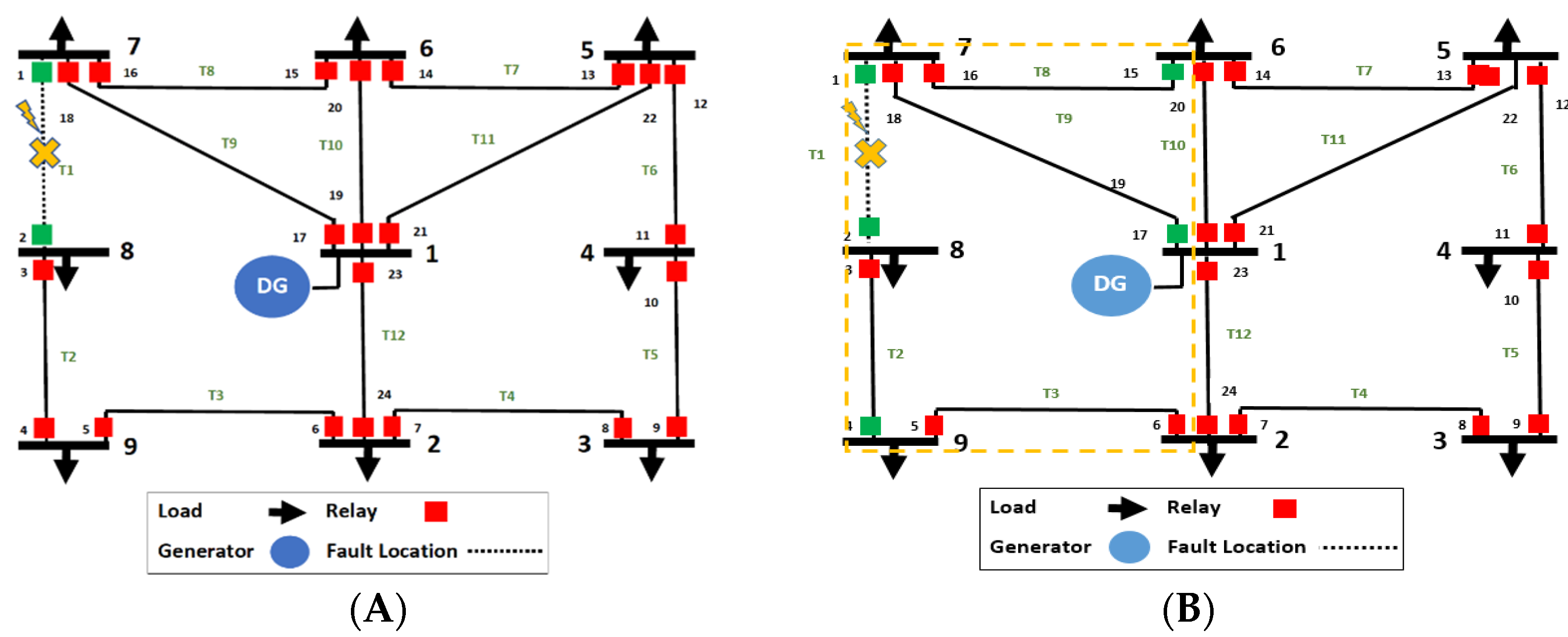
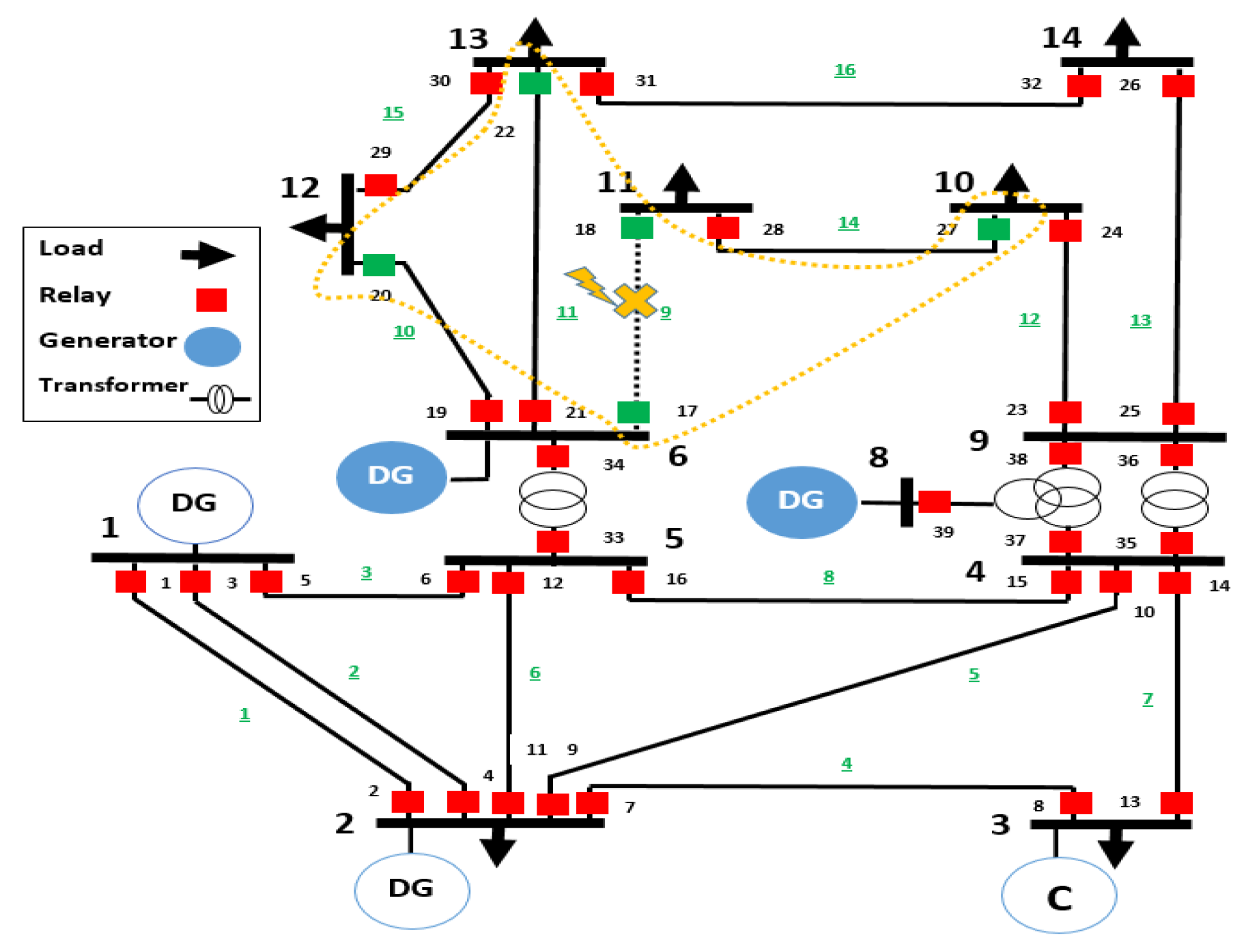
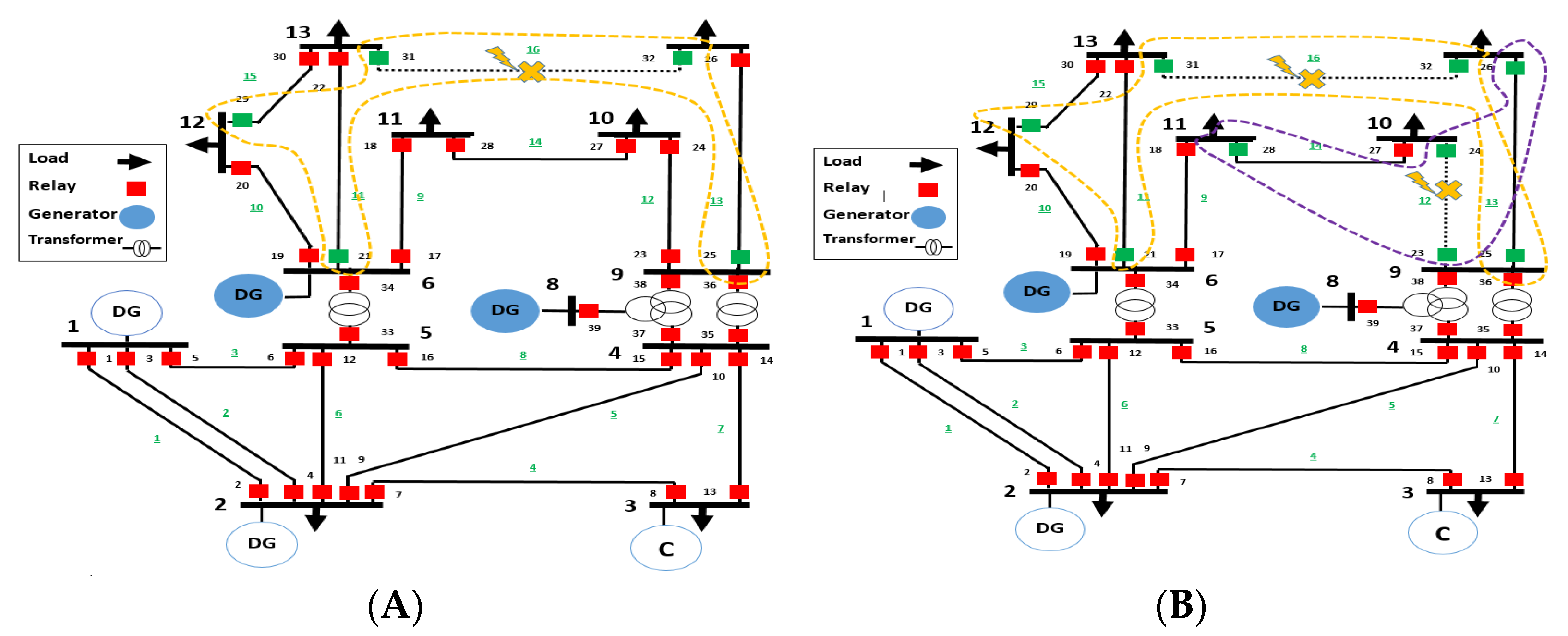
5.3. Major Disturbance Tests
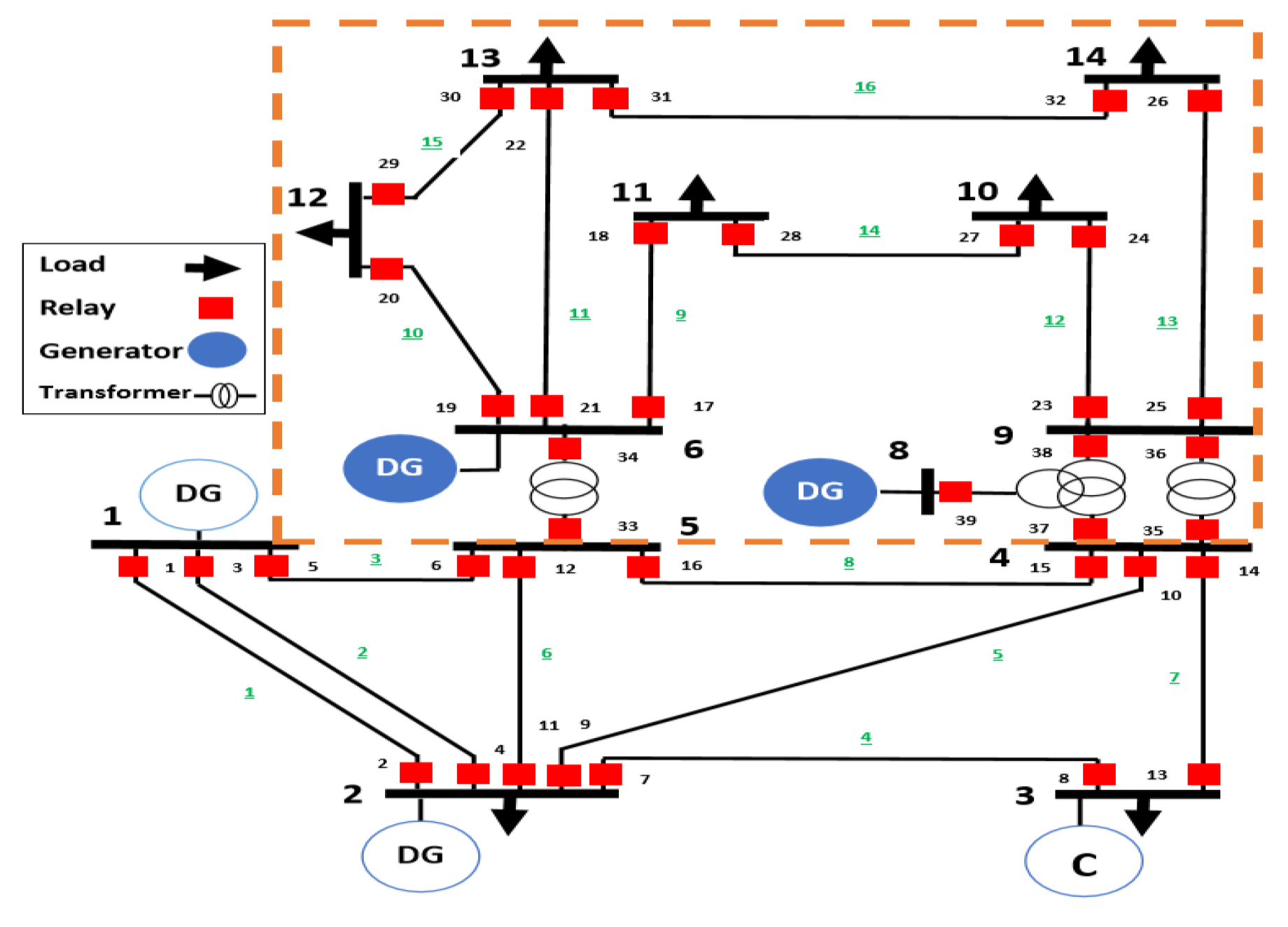
5.3.1. IEEE 9-Bus System
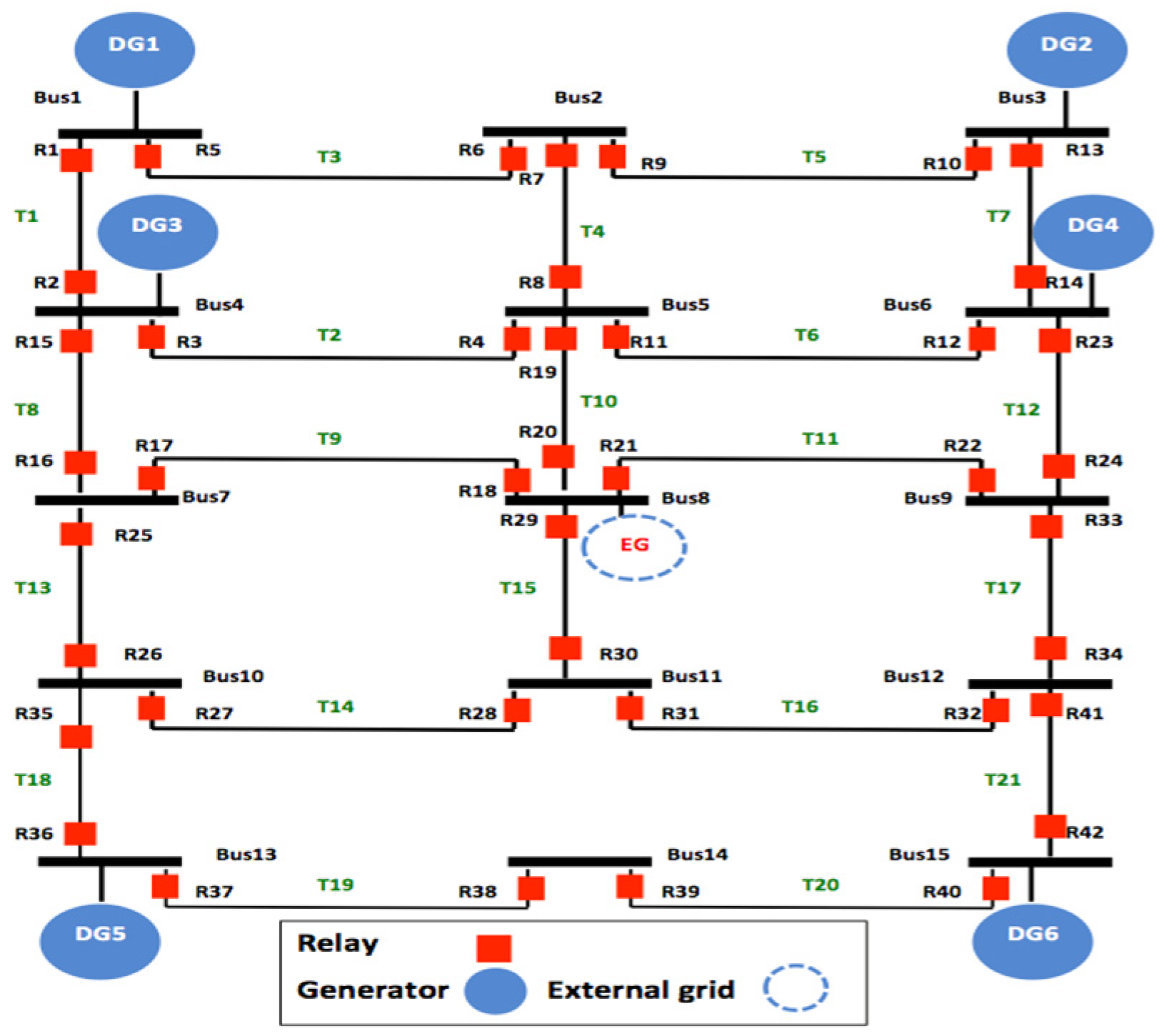
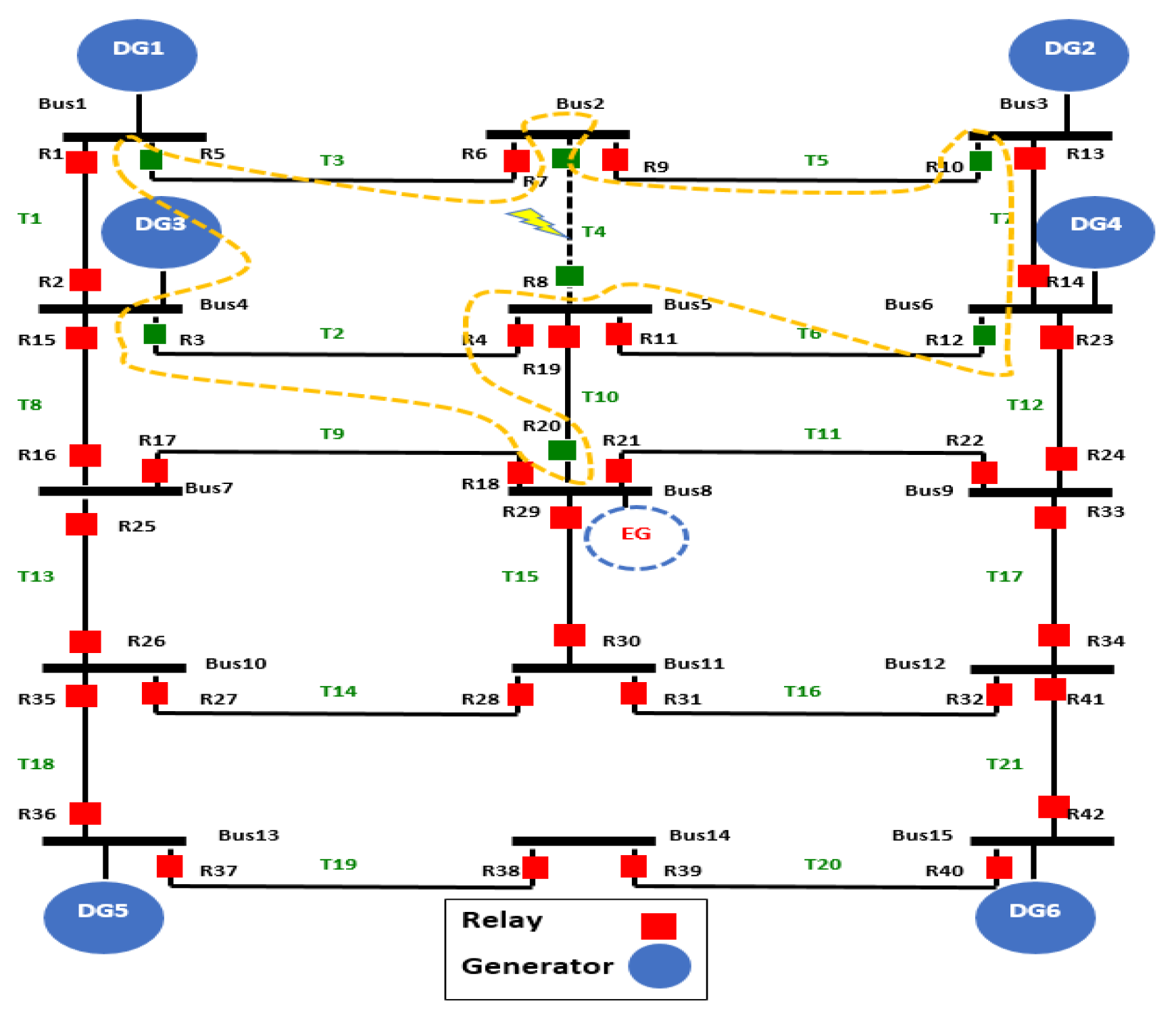
5.3.2. IEEE 14-Bus System
5.3.3. Comparison with Baseline Approach
5.3.4. Comparison on Large Disturbances
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Key Definitions
Appendix B. Validation Tests
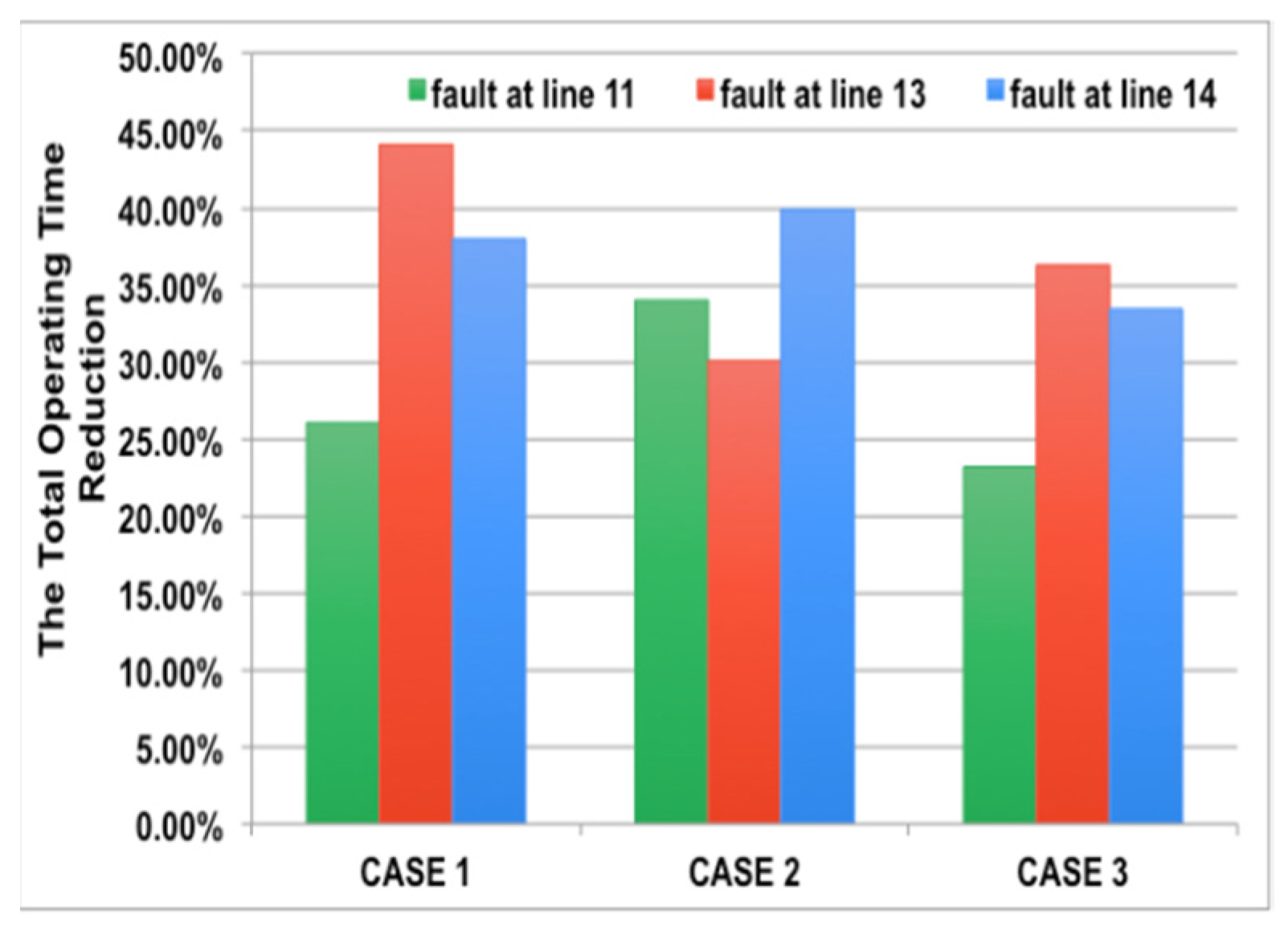
Appendix B.1. Effect of DGs and Fault Locations for IEEE14 Bus System
| DGs No. | DG1 | DG2 | DG1 &DG2 |
|---|---|---|---|
| Case 1 | ON | OFF | OFF |
| Case 2 | OFF | ON | OFF |
| Case 3 | OFF | OFF | ON |
| Fault Location | No. of Relays in Containment Zone | Total Operating Time | % of Adjusted Relays | |
|---|---|---|---|---|
| Case 1 | Line 11 | 10 | 7.0355 | 62.50% |
| Line 13 | 4 | 4.9721 | 25.00% | |
| Line 14 | 7 | 7.5094 | 43.75% | |
| Case 2 | Line 11 | 6 | 5.0644 | 37.50% |
| Line 13 | 7 | 9.9321 | 43.75% | |
| Line 14 | 7 | 9.0237 | 43.75% | |
| Case 3 | Line 11 | 10 | 7.0370 | 62.50% |
| Line 13 | 7 | 9.9515 | 43.75% | |
| Line 14 | 12 | 12.4028 | 75.00% |
| Fault at Line 11 | ||||||||
|---|---|---|---|---|---|---|---|---|
| Case 1 | Case 2 | Case 3 | ||||||
| Relay No | TDS | Operating Time in (s) | Relay No | TDS | Operating Time in (s) | Relay No | TDS | Operating Time in (s) |
| 18 | 0.1983 | 0.4903 | 18 | 0.1000 | 0.9253 | 18 | 0.1545 | 0.6827 |
| 20 | 0.1829 | 0.4951 | 20 | 0.2531 | 0.9920 | 20 | 0.3542 | 0.6726 |
| 21 | 0.2531 | 0.3547 | 21 | 0.1015 | 0.6862 | 21 | 0.1242 | 0.5936 |
| 22 | 0.1029 | 0.3279 | 22 | 0.1572 | 0.7679 | 22 | 0.1976 | 0.5119 |
| 23 | 0.1015 | 1.1964 | 29 | 0.1499 | 0.8570 | 23 | 0.1786 | 0.6615 |
| 24 | 0.1793 | 1.7721 | 32 | 0.1603 | 0.8358 | 24 | 0.1654 | 0.8177 |
| 25 | 0.1000 | 1.6531 | 25 | 0.1536 | 1.0805 | |||
| 27 | 0.1455 | 0.6981 | 27 | 0.1098 | 0.7510 | |||
| 29 | 0.1046 | 0.5381 | 29 | 0.1000 | 0.6323 | |||
| 32 | 0.1881 | 0.5101 | 32 | 0.1000 | 0.6330 | |||
| Fault at Line 13 | ||||||||
|---|---|---|---|---|---|---|---|---|
| Case 1 | Case 2 | Case 3 | ||||||
| Relay No | TDS | Operating Time in (s) | Relay No | TDS | Operating Time in (s) | Relay No | TDS | Operating Time in (s) |
| 24 | 0.1766 | 1.6531 | 21 | 0.1531 | 1.6898 | 21 | 0.1306 | 1.4589 |
| 25 | 0.1891 | 0.8279 | 24 | 0.1670 | 1.1687 | 24 | 0.1986 | 1.2762 |
| 26 | 0.1070 | 0.8209 | 25 | 0.1678 | 1.1241 | 25 | 0.1793 | 1.1800 |
| 31 | 0.1428 | 1.6702 | 26 | 0.1860 | 1.1179 | 26 | 0.1025 | 1.3342 |
| 28 | 0.1699 | 1.7798 | 28 | 0.1536 | 1.4711 | |||
| 29 | 0.1029 | 1.5611 | 29 | 0.1912 | 1.7980 | |||
| 31 | 0.1468 | 1.4905 | 31 | 0.1654 | 1.4323 | |||
| Fault at Line 14 | ||||||||
|---|---|---|---|---|---|---|---|---|
| Case 1 | Case 2 | Case 3 | ||||||
| Relay No | TDS | Operating Time in (s) | Relay No | TDS | Operating Time in (s) | Relay No | TDS | Operating Time in (s) |
| 17 | 0.1000 | 0.8563 | 17 | 0.1972 | 1.3927 | 17 | 0.1291 | 0.64215 |
| 20 | 0.1532 | 1.9854 | 20 | 0.1439 | 1.2794 | 20 | 0.1985 | 0.4225 |
| 22 | 0.1150 | 1.3562 | 22 | 0.1943 | 1.2894 | 21 | 0.1025 | 0.9854 |
| 23 | 0.1218 | 0.8721 | 23 | 0.1869 | 1.2314 | 22 | 0.1004 | 0.3453 |
| 26 | 0.1699 | 0.9526 | 26 | 0.1654 | 1.6425 | 23 | 0.1958 | 1.4013 |
| 27 | 0.1043 | 0.73875 | 27 | 0.1578 | 1.0636 | 25 | 0.1439 | 1.2125 |
| 28 | 0.1592 | 0.74805 | 28 | 0.1008 | 1.1246 | 26 | 0.1995 | 1.8773 |
| 27 | 0.1000 | 0.6291 | ||||||
| 28 | 0.1565 | 0.6043 | ||||||
| 29 | 0.1793 | 1.7293 | ||||||
| 31 | 0.1344 | 1.3521 | ||||||
| 32 | 0.1978 | 1.8437 | ||||||

Appendix B.2. Comparison on the Effect of DGs and Fault Locations for Baseline IEEE 14 Bus System
| Cases | Fault Location | The Total Operating Time in Second | |
|---|---|---|---|
| Proposed Objective Function | The Objective Function of [23] | ||
| Case #1 | Line 11 | 7.2206 | 9.764 |
| Line 13 | 4.9528 | 8.8631 | |
| Line 14 | 8.0486 | 12.977 | |
| Case #2 | Line 11 | 5.1861 | 7.864 |
| Line 13 | 10.7304 | 15.3445 | |
| Line 14 | 12.0135 | 19.986 | |
| Case #3 | Line 11 | 7.6653 | 9.9786 |
| Line 13 | 8.2684 | 12.975 | |
| Line 14 | 12.6324 | 18.9753 | |
Appendix B.3. High DG-Penetrated Microgrid Test for IEEE15-Bus System
IEEE 15-Bus System
| Containment Zone Relays | TDS | PS | Operating Time (s) |
|---|---|---|---|
| Relay 3 | 1.08 | 1.88 | 0.48 |
| Relay 5 | 0.92 | 2.41 | 0.52 |
| Relay 7 | 0.97 | 1.56 | 0.91 |
| Relay 8 | 0.99 | 1.02 | 0.25 |
| Relay10 | 0.43 | 0.54 | 0.81 |
| Relay12 | 0.47 | 2.39 | 0.37 |
| Relay20 | 0.42 | 2.22 | 0.51 |
References
- Parhizi, S.; Lotfi, H.; Khodaei, A.; Bahramirad, S. State of the art in research on microgrids: A review. IEEE Access 2015, 3, 890–925. [Google Scholar] [CrossRef]
- Usama, M.; Moghavvemi, M.; Mokhlis, H.; Mansor, N.N.; Farooq, H.; Pourdaryaei, A. Optimal Protection Coordination Scheme for Radial Distribution Network Considering ON/OFF-Grid. IEEE Access 2021, 9, 34921–34937. [Google Scholar] [CrossRef]
- Kumar, G.V.N.; Kumar, B.S.; Rao, B.V.; Sobhan, P.V.S.; Naidu, K.A. Linear programming technique based optimal relay coordination in a radial distribution system. Int. J. Eng. Technol. 2018, 7, 51–55. [Google Scholar] [CrossRef][Green Version]
- Tsimtsios, A.M.; Safigianni, A.S.; Nikolaidis, V.C. Generalized distance-based protection design for DG integrated MV radial distribution networks—Part II: Application to an actual distribution line. Electr. Power Syst. Res. 2019, 176. [Google Scholar] [CrossRef]
- Alam, M.N. Adaptive Protection Coordination Scheme Using Numerical Directional Overcurrent Relays. IEEE Trans. Ind. Inform. 2019, 15, 64–73. [Google Scholar] [CrossRef]
- Hussain, N.; Nasir, M.; Vasquez, J.C.; Guerrero, J.M. Recent Developments and Challenges on AC Microgrids Fault Detection and Protection Systems—A Review. Energies 2020, 13, 2149. [Google Scholar] [CrossRef]
- Tailor, J.K.; Osman, A. Restoration of fuse-recloser coordination in distribution system with high DG penetration. In Proceedings of the 2008 IEEE Power and Energy Society General Meeting-Conversion and Delivery of Electrical Energy in the 21st Century, Pittsburgh, PA, USA, 20–24 July 2008. [Google Scholar] [CrossRef]
- North American Electric Reliability Corporation. Performance of Distributed Energy Resources During and After System Disturbance Voltage and Frequency Ride-Through Requirements. 2013. Available online: https://www.nerc.com/pa/RAPA/ra/Reliability Assessments DL/IVGTF17_PC_FinalDraft_December_clean.pdf (accessed on 24 November 2020).
- Tsimtsios, A.M.; Korres, G.N.; Nikolaidis, V.C. A pilot-based distance protection scheme for meshed distribution systems with distributed generation. Int. J. Electr. Power Energy Syst. 2018, 105, 454–469. [Google Scholar] [CrossRef]
- Lin, H.; Liu, C.; Guerrero, J.; Vasquez, J.C. Distance protection for microgrids in distribution system. In IECON 2015—41st Annual Conference of the IEEE Industrial Electronics Society; IEEE: Piscataway, NJ, USA, 2015; pp. 731–736. [Google Scholar] [CrossRef]
- Rao, H.V.G.; Prabhu, N.; Mala, R.C. Adaptive Distance Protection for Transmission Lines Incorporating SSSC with Energy Storage Device. IEEE Access 2020, 8, 156017–156026. [Google Scholar] [CrossRef]
- Biller, M.; Jaeger, J.; Mladenovic, I.; Schacherer, C.; Wolter, D.; Stoetzel, M. Protection systems in distribution grids with variable short-circuit conditions. In Proceedings of the 13th International Conference on Development in Power System Protection, Edinburgh, UK, 7–10 March 2016; Volume 2016. [Google Scholar] [CrossRef]
- Ustun, T.S.; Ozansoy, C.; Zayegh, A. Differential protection of microgrids with central protection unit support. In IEEE 2013 Tencon—Spring, TENCONSpring 2013—Conference Proceedings; IEEE: Piscataway, NJ, USA, 2013; pp. 15–19. [Google Scholar] [CrossRef]
- Conti, S.; Raffa, L.; Vagliasindi, U. Innovative solutions for protection schemes in autonomous MV micro-grids. In Proceedings of the 2009 International Conference on Clean Electrical Power, ICCEP 2009, Capri, Italy, 9–11 June 2009; pp. 647–654. [Google Scholar] [CrossRef]
- He, J.; Wang, Z.; Zhang, Q.; Liu, L.; Zhang, D.; Crossley, P.A. Distributed protection for smart substations based on multiple overlapping units. CSEE J. Power Energy Syst. 2016, 2, 44–50. [Google Scholar] [CrossRef]
- Habib, H.F.; Youssef, T.; Cintuglu, M.H.; Mohammed, O.A. Multi-Agent-Based Technique for Fault Location, Isolation, and Service Restoration. IEEE Trans. Ind. Appl. 2017, 53, 1841–1851. [Google Scholar] [CrossRef]
- Choudhary, N.K.; Mohanty, S.R.; Singh, R.K. Protection coordination of over current relays in distribution system with DG and superconducting fault current limiter. In Proceedings of the 2014 Eighteenth National Power Systems Conference, Guwahati, India, 18–20 December 2014. [Google Scholar] [CrossRef]
- Jones, D.; Kumm, J.J. Future distribution feeder protection using directional overcurrent elements. IEEE Trans. Ind. Appl. 2014, 50, 1385–1390. [Google Scholar] [CrossRef]
- Yazdaninejadi, A.; Golshannavaz, S.; Nazarpour, D.; Teimourzadeh, S.; Aminifar, F. Dual-Setting Directional Overcurrent Relays for Protecting Automated Distribution Networks. IEEE Trans. Ind. Inform. 2018, 15, 730–740. [Google Scholar] [CrossRef]
- Darabi, A.; Bagheri, M.; Gharehpetian, G. Dual feasible direction-finding nonlinear programming combined with metaheuristic approaches for exact overcurrent relay coordination. Int. J. Electr. Power Energy Syst. 2019, 114, 105420. [Google Scholar] [CrossRef]
- Alam, M.N. Overcurrent protection of AC microgrids using mixed characteristic curves of relays. Comput. Electr. Eng. 2019, 74, 74–88. [Google Scholar] [CrossRef]
- Ustun, T.S.; Ozansoy, C.; Zayegh, A. Modeling and simulation of a microgrid protection system with central protection unit. In IEEE 2013 Tencon—Spring, TENCONSpring 2013—Conference Proceedings; IEEE: Piscataway, NJ, USA, 2013; pp. 5–9. [Google Scholar] [CrossRef]
- Saleh, K.; Zeineldin, H.H.; El-Saadany, E.F. Optimal Protection Coordination for Microgrids Considering N–1 Contingency. IEEE Trans. Ind. Inform. 2017, 13, 2270–2278. [Google Scholar] [CrossRef]
- Thararak, P.; Jirapong, P. Implementation of Optimal Protection Coordination for Microgrids with Distributed Generations Using Quaternary Protection Scheme. J. Electr. Comput. Eng. 2020. [CrossRef]
- De Souza, A.C.Z.; Santos, M.; Castilla, M.; Miret, J.; de Vicuña, L.G.; Marujo, D. Voltage security in AC microgrids: A power flow-based approach considering droopcontrolled inverters. IET Renew. Power Gener. 2015, 9, 954–960. [Google Scholar] [CrossRef]
- Albatsh, F.M.; Ahmad, S.; Mekhilef, S.; Mokhlis, H.; Hassan, M.A. Optimal Placement of Unified Power Flow Controllers to Improve Dynamic Voltage Stability Using Power System Variable Based Voltage Stability Indices. PLoS ONE 2015, 10, e0123802. [Google Scholar] [CrossRef]
- Guerrero, J.; Vasquez, J.C.; Matas, J.; de Vicuña, L.G.; Castilla, M. Hierarchical control of droop-controlled AC and DC microgrids—A general approach toward standardization. IEEE Trans. Ind. Electron. 2010, 58, 158–172. [Google Scholar] [CrossRef]
- Gopalakrishnan, A.; Kezunovic, M.; McKenna, S.; Hamai, D. Fault location using the distributed parameter transmission line model. IEEE Trans. Power Deliv. 2000, 15, 1169–1174. [Google Scholar] [CrossRef]
- García, L.F.R.; Londoño, S.M.P.; Flórez, J.J.M. Dynamic load modeling for small disturbances using measurement-based parameter estimation method. In Proceedings of the International Symposium on the Quality of Electrical Power, SICEL, Medellín, Colombia, 27–29 November 2013. [Google Scholar]
- Ustun, T.S.; Ozansoy, C.; Zayegh, A. A microgrid protection system with central protection unit and extensive communication. In Proceedings of the 2011 10th International Conference on Environment and Electrical Engineering, Rome, Italy, 8–11 May 2011. [Google Scholar] [CrossRef]
- Monitoring of Power System Quality eBook by Dr. Hidaia Alassouli—1230002137902|Rakuten Kobo United States. Available online: https://www.kobo.com/us/en/ebook/monitoring-of-power-system-quality (accessed on 24 November 2020).
- Andrés, H.R.F.; Hernandez, J.; Trujillo, E.R. Optimal Power Flow in Electrical Microgrids. Energy Power Eng. 2014, 6, 449–458. [Google Scholar] [CrossRef][Green Version]
- Wei, C.; Fadlullah, Z.M.; Kato, N.; Stojmenovic, I. A novel distributed algorithm for power loss minimizing in Smart Grid. In Proceedings of the 2014 IEEE International Conference on Smart Grid Communications, SmartGridComm 2014, Venice, Italy, 3–6 November 2014; pp. 290–295. [Google Scholar] [CrossRef]
- Sanseverino, E.R.; Di Silvestre, M.L.; Badalamenti, R.; Nguyen, N.Q.; Guerrero, J.M.; Meng, L.; Quang, N.N. Optimal power flow in islanded microgrids using a simple distributed algorithm. Energies 2015, 8, 11493–11514. [Google Scholar] [CrossRef]
- Bernatik, A. Risk Analysis and Management—Trends, Challenges and Emerging Issues; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- IEEE Xplore Book Abstract—Microgrid Planning and Design: A Concise Guide. Available online: https://ieeexplore.ieee.org/book/8671408 (accessed on 24 November 2020).
- Bevrani, H.; Francois, B.; Ise, T. Microgrid Dynamics and Control; John Wiley & Sons: Hoboken, NJ, USA, 2017. [Google Scholar]
- Srikanth, P.; Rajendra, O.; Yesuraj, A.; Tilak, M.; Raja, K. Load Flow Analysis of IEEE 14 Bus System Using MATLAB. Int. J. Eng. Res. Technol. 2013, 2, 149–155. [Google Scholar]
- Kim, M.-S.; Haider, R.; Cho, G.-J.; Kim, C.-H.; Won, C.-Y.; Chai, J.-S. Comprehensive Review of Islanding Detection Methods for Distributed Generation Systems. Energies 2019, 12, 837. [Google Scholar] [CrossRef]
- Reigosa, D.; Briz, F.; Blanco, C.; García, P.; Guerrero, J.M. Active islanding detection using high frequency signal injection. IEEE Trans. Ind. Appl. 2012, 48, 1588–1597. [Google Scholar] [CrossRef]
- Das, D.; Gurrala, G.; Shenoy, U.J. Transition between grid-connected mode and islanded mode in VSI-fed microgrids. Sādhanā 2017, 42, 1239–1250. [Google Scholar] [CrossRef]
- Cintuglu, M.H.; Ma, T.; Mohammed, O.A. Protection of Autonomous Microgrids Using Agent-Based Distributed Communication. IEEE Trans. Power Deliv. 2016, 32, 351–360. [Google Scholar] [CrossRef]
- Protection of Electricity Distribution Networks, 2nd Edition (Power and Energy): Juan Gers: 9780863413575: Amazon.com: Books. Available online: https://www.amazon.com/Protection-Electricity-Distribution-Networks-Energy/dp/0863413579 (accessed on 24 November 2020).
- Ahshan, R. Modeling and Analysis of a Micro-Grid System Powered by Renewable Energy Sources. Open Renew. Energy J. 2013, 6, 7–22. [Google Scholar] [CrossRef]
- Volume I, Issue II. Estimation of Line Parameters of an IEEE 14 Bus System. 2014. Available online: www.rsisinternational.org (accessed on 24 November 2020).
- Bedekar, P.P.; Bhide, S.R. Optimum coordination of directional overcurrent relays using the hybrid GA-NLP approach. IEEE Trans. Power Deliv. 2011, 26, 109–119. [Google Scholar] [CrossRef]
- Amraee, T. Coordination of directional overcurrent relays using seeker algorithm. IEEE Trans. Power Deliv. 2012, 27, 1415–1422. [Google Scholar] [CrossRef]





| Protection Strategy | Advantages | Disadvantages |
|---|---|---|
|
|
|
|
|
|
| ||
|
|
|
|
|
|
|
|
|
|
|
|
| Mode of Operation | Connected | Islanded |
|---|---|---|
| Active and reactive power (P-Q) | Constant | Variable |
| Voltage and frequency (V-F) | Variable | Constant |
| Generator’s Specification | Protection Component’s Specification | Protection Component’s Specification | |||
|---|---|---|---|---|---|
| The active power | 102.554 MW | Overcurrent relays type | Schweitzer 351, curve type IEC. | Transmission line current | 1750 Amps |
| The reactive power | 5.597 MVAR | Overcurrent relays pickup range | IDMT, 0.02-3.2 s, 1 Amp | The Apparent power rating | 100 MVA |
| The apparent power | 104.5 VA | Circuit breakers type | Standard SYM | Resistance | R = 0.0001 P.U. |
| The power factor | 95% | Circuit breakers duration | 5-cycle duration, contact parting time (CPT) of 3, and time constant of 45 s. | Reactance | X = 10 P.U. |
| Poles | 4 | Circuit breakers maximum rating | 1.01 KV | Admittance | Y = 0 P.U. |
| Speed | 1500 RPM | Transformer ratio | 500:1 | ||
| Full-Load Amperes (FLA) rating | 1925 | ||||
| Impedance | Xd” = 10%; Xd”/Ra = 19; Ra = 0.052105 Ohms | ||||
| DG No. | DG Location | Capacity of Synchronous Generators (MVA) |
|---|---|---|
| DG unit 1 | 6 | 100 |
| DG unit 2 | 8 | 100 |
| Curve Description | Standard | α | β | L |
|---|---|---|---|---|
| Standard inverse | IEC | 0.02 | 0.14 | 0 |
| Containment Zone Relays | TDS | PS | Operating Time (s) |
|---|---|---|---|
| Relay 1 | 0.026210 | 0.021952 | 0.0257 |
| Relay 2 | 0.028915 | 0.025359 | 0.0237 |
| Relay 4 | 0.028039 | 0.024171 | 0.0359 |
| Relay 15 | 0.026001 | 0.026902 | 0.2253 |
| Relay 17 | 0.528113 | 0.559173 | 0.0341 |
| Fault Location | Containment Zone Relays | Operate. Time (s) |
|---|---|---|
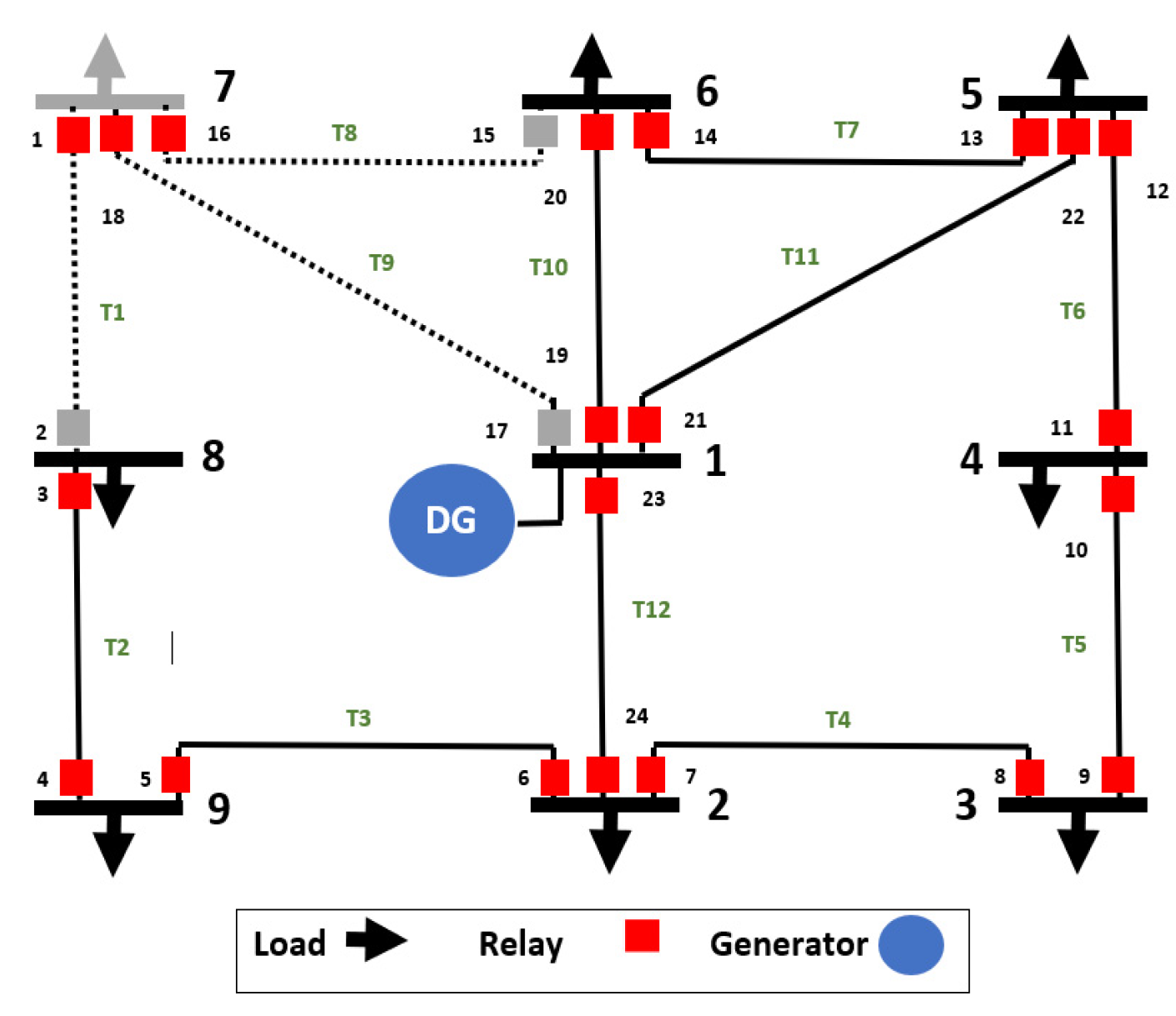
| Tran. Line (T1) | Relay (1, 2, 4, 15, 17) | 0.3447 |
| Tran. Line (T2) | Relay (2, 3, 1, 6) | 0.1029 |
| Tran. Line (T3) | Relay (4, 5, 3, 8, 23) | 0.4311 |
| Tran. Line (T4) | Relay (7, 8, 10, 23) | 0.4236 |
| Tran. Line (T5) | Relay (7, 9, 10, 22) | 0.1409 |
| Tran. Line (T6) | Relay (11, 22, 9, 14, 21) | 0.3265 |
| Tran. Line (T7) | Relay (13, 14, 11, 16, 19, 21) | 0.3461 |
| Tran. Line (T8) | Relay (15, 16, 17, 2, 19, 13) | 0.4522 |
| Tran. Line (T9) | Relay (17, 18, 2, 15) | 0.539 |
| Tran. Line (T10) | Relay (19, 20, 13, 16) | 0.4875 |
| Tran. Line (T11) | Relay (21, 22, 11, 14) | 0.5018 |
| Tran. Line (T12) | Relay (23, 24, 5, 8) | 0.3049 |
| Containment Zone Relays | TDS | PS | Operating Time (s) |
|---|---|---|---|
| Relay 17 | 0.3218 | 1.6721 | 1.3860 |
| Relay 18 | 0.1783 | 1.1743 | 1.3582 |
| Relay 20 | 0.2852 | 2.4265 | 1.3026 |
| Relay 22 | 0.4190 | 1.9308 | 1.2995 |
| Relay 27 | 0.4013 | 2.2003 | 1.3381 |
| Fault Location | Containment Zone Relays | Total Operating Time (s) |
|---|---|---|
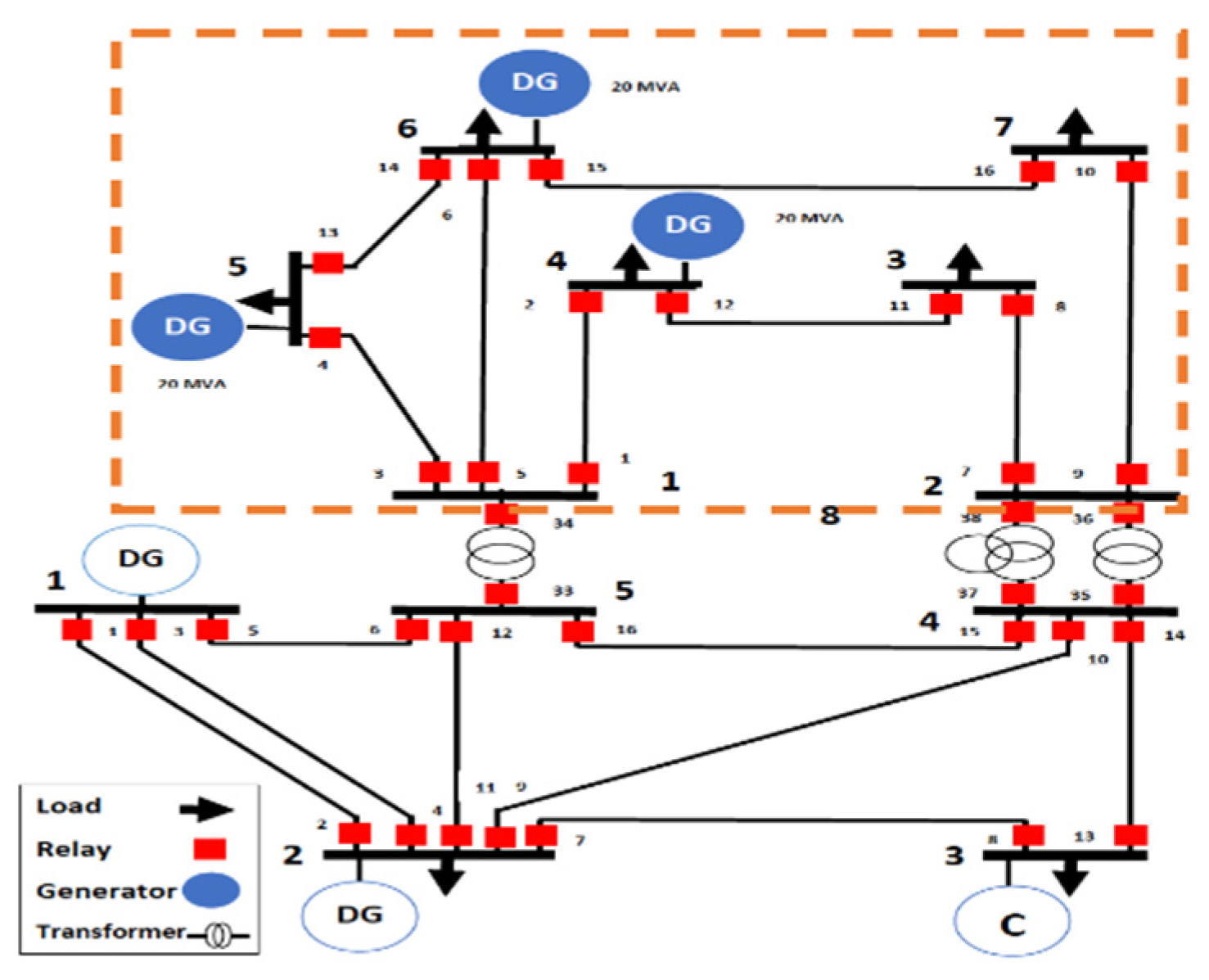
| Tran. Line (T16) | Relay (21, 25, 29, 31, 32) | 7.9531 |
| Tran. Line (T16, T12) | Relay (21, 23, 24, 25, 26, 28 29, 31, 32) | 8.8793 |
| Tran. Line (T12, T15, T16) | Relay (19, 21, 23, 24, 25, 26, 28 29, 30, 31, 32) | 9.6779 |
| Tran. Line (T12, T14, T15, T16) | Relay (17, 19, 21, 23, 24, 25, 26, 27, 28, 29, 30, 31, 32) | 10.6148 |
| Config. | Relay No. | TDS | PS | Max. Current in Normal Operation (Amps.) | Current during Dynamic Loads(Amps.) |
|---|---|---|---|---|---|
| IEEE 9 Bus System | 2 | 0.0383 | 0.0231 | 108.33 | 124.09 |
| 15 | 0.0261 | 0.0260 | 96.34 | 110.53 | |
| 17 | 0.0295 | 0.0322 | 388.91 | 403.81 | |
| IEEE 14 Bus System | 25 | 0.3962 | 2.7504 | 194.10 | 224.07 |
| 31 | 0.3885 | 2.9884 | 369.92 | 381.03 |
| Fault Location | Total Operating Time in (s) | ||
|---|---|---|---|
| [23] | Proposed Scheme | Reduction | |
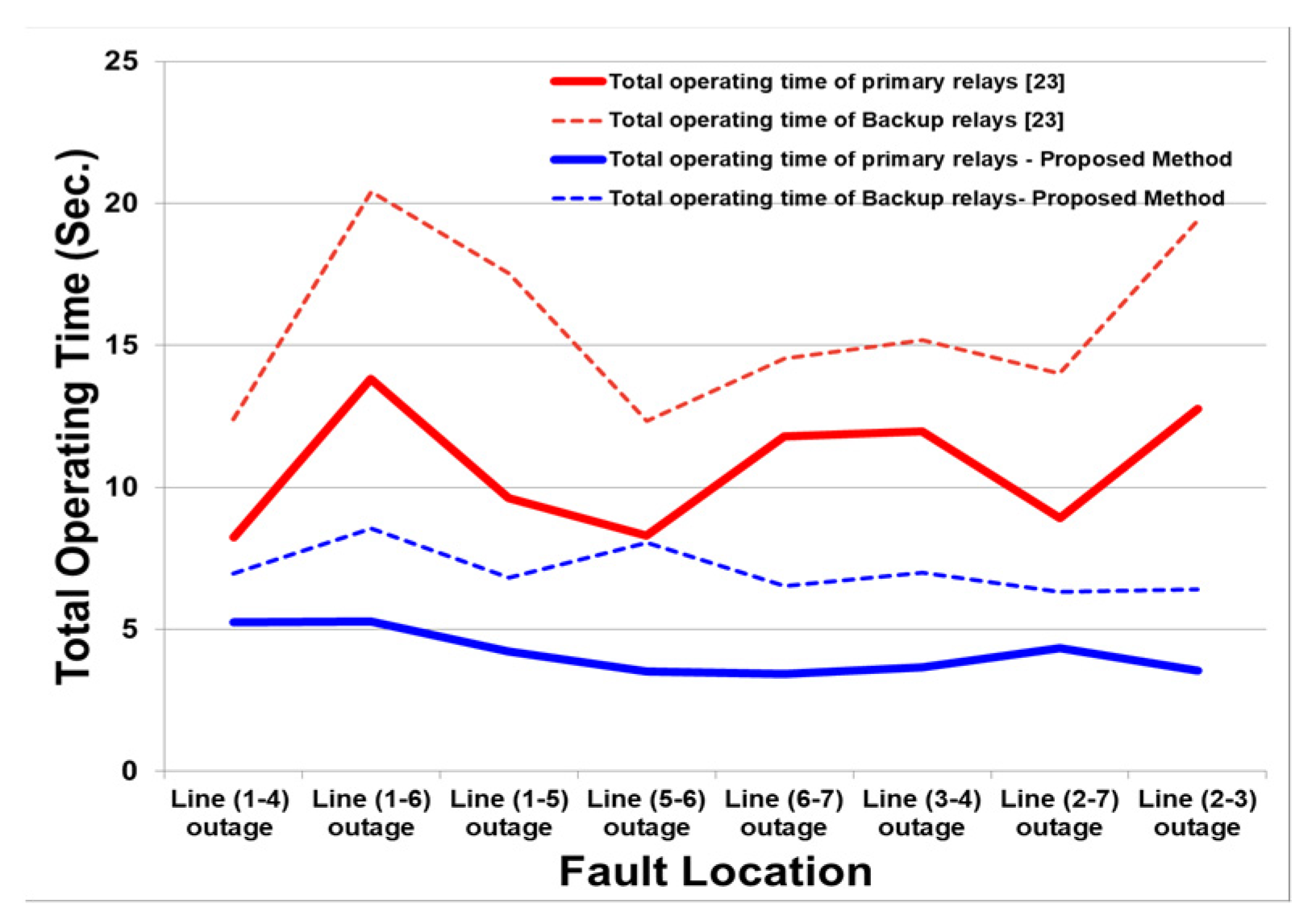
| Line (1–4) outage | 20.6281 | 13.2155 | 35.93% |
| Line (1–6) outage | 27.1609 | 14.9852 | 44.82% |
| Line (1–5) outage | 34.2278 | 14.0431 | 58.97% |
| Line (5–6) outage | 22.9395 | 10.2297 | 55.40% |
| Line (6–7) outage | 32.1598 | 9.9875 | 68.94% |
| Line (3–4) outage | 27.1606 | 10.9409 | 59.71% |
| Line (2–7) outage | 26.3331 | 9.8911 | 62.44% |
| Line (2–3) outage | 20.6281 | 12.1005 | 41.34% |
| Relay No. | TDS | PS | Operating Time (s) |
|---|---|---|---|
| 1 | 0.021525 | 0.021621 | 0.0163801 |
| 2 | 0.026352 | 0.021475 | 0.0298431 |
| 3 | 0.033039 | 0.020311 | 0.0296611 |
| 4 | 0.023705 | 0.026102 | 0.0280651 |
| 5 | 0.027107 | 0.024631 | 0.0296401 |
| 6 | 0.025357 | 0.023533 | 0.0227431 |
| 7 | 0.025357 | 0.020961 | 0.0251802 |
| 8 | 0.027012 | 0.029731 | 0.0269221 |
| 9 | 0.026041 | 0.020173 | 0.0260221 |
| 10 | 0.033461 | 0.021762 | 0.0290762 |
| 11 | 0.021752 | 0.023075 | 0.0320891 |
| 12 | 0.027093 | 0.023387 | 0.0821091 |
| 13 | 0.027201 | 0.023387 | 0.0392731 |
| 14 | 0.025441 | 0.023853 | 0.0392731 |
| 15 | 0.021359 | 0.175344 | 0.0286422 |
| 16 | 0.025991 | 0.543573 | 0.0273281 |
| 17 | 0.020836 | 0.246732 | 0.0205129 |
| 18 | 0.534511 | 0.720311 | 0.0321467 |
| 19 | 0.833215 | 0.032125 | 0.0276543 |
| 20 | 1.199532 | 0.025321 | 0.0290762 |
| 21 | 1.197021 | 0.246323 | 0.0346218 |
| 22 | 0.025012 | 0.021473 | 0.0296401 |
| 23 | 1.245122 | 0.722562 | 0.0290762 |
| 24 | 1.206718 | 1.097653 | 0.0296401 |
| Proposed Protection System | Baseline [23] | |
|---|---|---|
| Operating Time (s) | 0.3464 | 0.7446 |
| Fault Location | Operate. Time (s) | % of Total Operating Time Reduction | |
|---|---|---|---|
| Proposed Protection System | Baseline [23] | ||
| Tran. Line (T1) | 0.3464 | 0.7446 | 53.479 |
| Tran. Line (T2) | 0.1006 | 0. 2813 | 64.237 |
| Tran. Line (T3) | 0.4293 | 0.8296 | 48.252 |
| Tran. Line (T4) | 0.4292 | 0.8527 | 48.298 |
| Tran. Line (T5) | 0.1373 | 0.3011 | 54.400 |
| Tran. Line (T6) | 0.3159 | 0.6731 | 53.067 |
| Tran. Line (T7) | 0.2950 | 0.7042 | 58.108 |
| Tran. Line (T8) | 0.4494 | 0.7219 | 37.747 |
| Tran. Line (T9) | 0.5022 | 0.8633 | 41.828 |
| Tran. Line (T10) | 0.4976 | 0.8245 | 39.648 |
| Tran. Line (T11) | 0.5022 | 0.8904 | 43.591 |
| Tran. Line (T12) | 0.3024 | 0.6991 | 56.744 |
| Relay No. | TDS | PS | Operating Time (s) |
|---|---|---|---|
| 17 | 0.309864 | 1.673021 | 1.102216 |
| 18 | 0.068321 | 1.912391 | 0.108317 |
| 19 | 0.205115 | 1.037233 | 1.262101 |
| 20 | 0.054012 | 1.175339 | 0.523014 |
| 21 | 0.135661 | 1.912391 | 1.328115 |
| 22 | 0.360196 | 1.673021 | 0.122958 |
| 23 | 0.059984 | 1.292341 | 0.942339 |
| 24 | 0.124201 | 2.428312 | 0.048322 |
| 25 | 0.139448 | 1.862502 | 1.028258 |
| 26 | 0.054012 | 1.424142 | 0.202446 |
| 27 | 0.308124 | 2.428312 | 0.754291 |
| 28 | 0.092321 | 1.862502 | 1.754992 |
| 29 | 0.064983 | 1.382232 | 0.983201 |
| 30 | 0.111439 | 0.753319 | 0.212933 |
| 31 | 0.050637 | 1.673021 | 0.521991 |
| 32 | 0.055116 | 1.093202 | 1.319982 |
| 33 | 0.137887 | 1.029344 | 0.643301 |
| 34 | 0.111439 | 2.428312 | 1.411826 |
| 35 | 0.181019 | 1.091193 | 0.237662 |
| 36 | 0.124201 | 1.175339 | 1.902366 |
| 37 | 0.071998 | 1.862502 | 1.123101 |
| 38 | 0.081445 | 1.082322 | 0.927311 |
| Total Operating Time (s) | Proposed Protection System | Baseline [23] | % of Coordinated DOCRs |
|---|---|---|---|
| Single fault in line (9) | 6.6844 | 18.4610 | 36.98% |
| Multiple independent faults line (16) | 7.9531 | 19.2988 | 41.10% |
| Multiple independent faults line (12, 16) | 8.8793 | 21.7641 | 40.79% |
| Multiple independent faults line (12, 15, 16) | 9.6779 | 22.5852 | 42.61% |
| Multiple independent faults line (12, 14, 15, 16) | 10.6148 | 23.9832 | 44.37% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bawayan, H.; Younis, M. Microgrid Protection through Adaptive Overcurrent Relay Coordination. Electricity 2021, 2, 524-553. https://doi.org/10.3390/electricity2040031
Bawayan H, Younis M. Microgrid Protection through Adaptive Overcurrent Relay Coordination. Electricity. 2021; 2(4):524-553. https://doi.org/10.3390/electricity2040031
Chicago/Turabian StyleBawayan, Haneen, and Mohamed Younis. 2021. "Microgrid Protection through Adaptive Overcurrent Relay Coordination" Electricity 2, no. 4: 524-553. https://doi.org/10.3390/electricity2040031
APA StyleBawayan, H., & Younis, M. (2021). Microgrid Protection through Adaptive Overcurrent Relay Coordination. Electricity, 2(4), 524-553. https://doi.org/10.3390/electricity2040031







