Functional Gradients of the Gyroid Infill for Structural Optimization †
1. Introduction
2. Materials and Methods
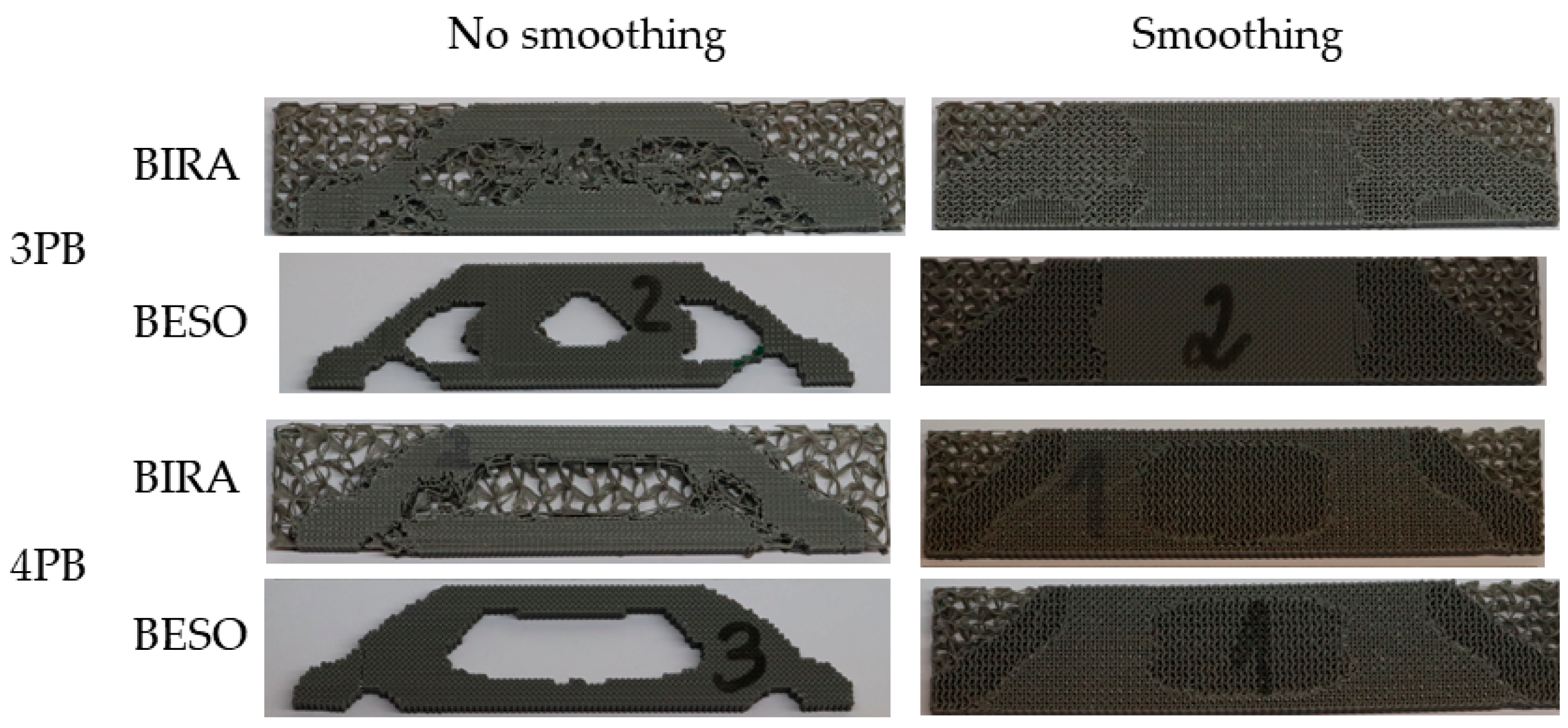
3. Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Li, D.; Dai, N.; Jiang, X.; Chen, X. Interior structural optimization based on the density-variable shape modeling of 3D printed objects. Int. J. Adv. Manuf. Technol. 2016, 83, 1627–1635. [Google Scholar] [CrossRef]
- Li, D.; Liao, W.; Dai, N.; Dong, G.; Tang, Y.; Xie, Y.M. Optimal design and modeling of gyroid-based functionally graded cellular structures for additive manufacturing. CAD Comput. Aided Des. 2018, 104, 87–99. [Google Scholar] [CrossRef]
- Tang, Y.; Kurtz, A.; Zhao, Y.F. Bidirectional Evolutionary Structural Optimization (BESO) based design method for lattice structure to be fabricated by additive manufacturing. CAD Comput. Aided Des. 2015, 69, 91–101. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J.; Sato, Y.; Yanagimoto, J. Homogenization-based topology optimization integrated with elastically isotropic lattices for additive manufacturing of ultralight and ultrastiff structures. CIRP Ann. 2021, 70, 111–114. [Google Scholar] [CrossRef]
- Zhang, P.; Toman, J.; Yu, Y.; Biyikli, E.; Kirca, M.; Chmielus, M.; To, A.C. Efficient design-optimization of variable-density hexagonal cellular structure by additive manufacturing: Theory and validation. J. Manuf. Sci. Eng. Trans. ASME 2015, 137, 41–45. [Google Scholar] [CrossRef]
- da Silva, C.M.M.; Pais, A.; Caldas, G.; Gouveia, B.P.; Alves, J.L.; Belinha, J. Study on 3D printing of gyroid based structures for superior structural behaviour. Prog. Addit. Manuf. 2021, 6, 689–703. [Google Scholar] [CrossRef]
- Belinha, J.; Jorge, R.M.N.; Dinis, L.M.J.S. A meshless microscale bone tissue trabecular remodelling analysis considering a new anisotropic bone tissue material law. Comput. Methods Biomech. Biomed. Eng. 2013, 16, 1170–1184. [Google Scholar] [CrossRef] [PubMed]
- Xie, Y.M.; Steven, G.P. Evolutionary structural optimization. In Evolutionary Structural Optimization; Springer: Berlin/Heidelberg, Germany, 1997. [Google Scholar]
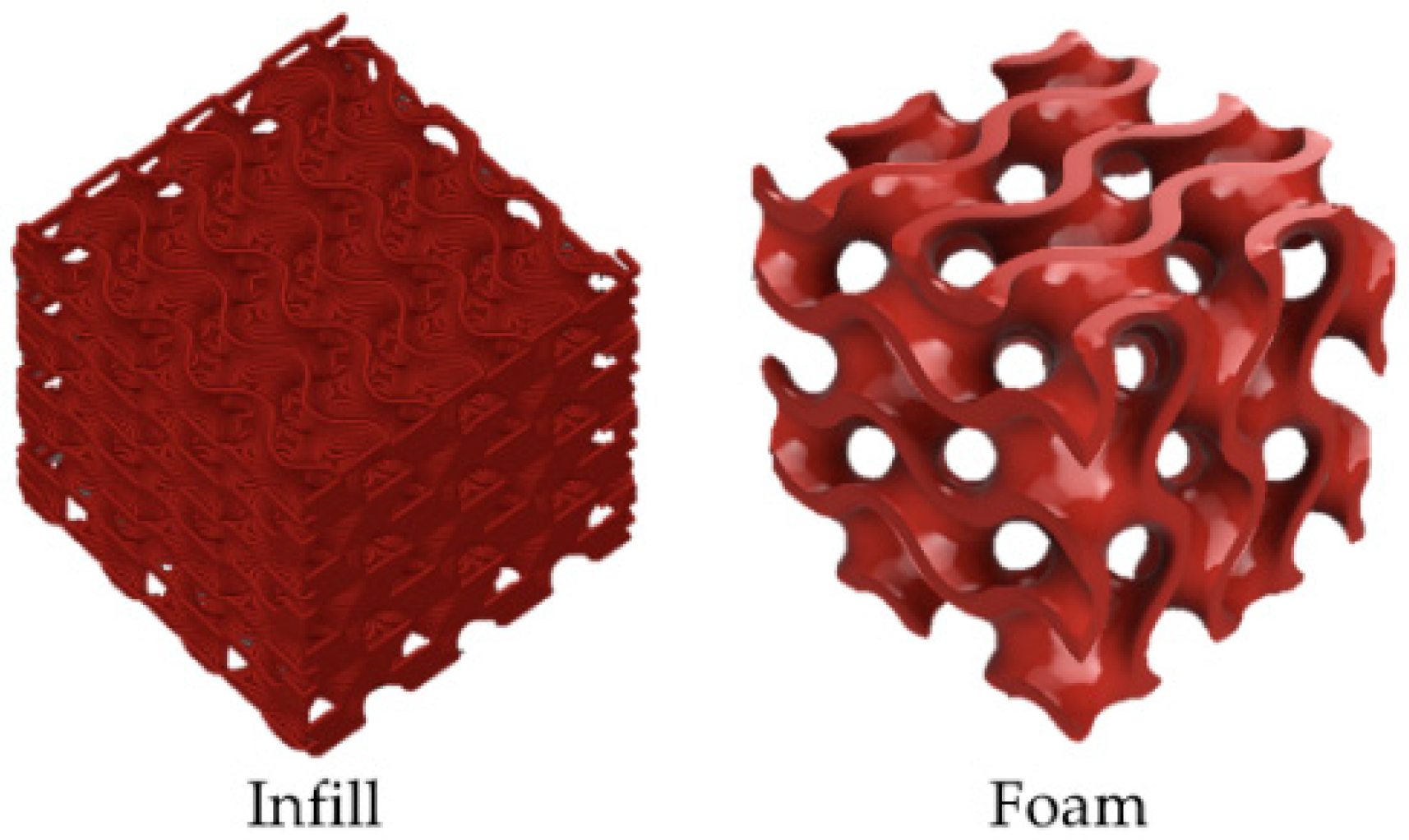
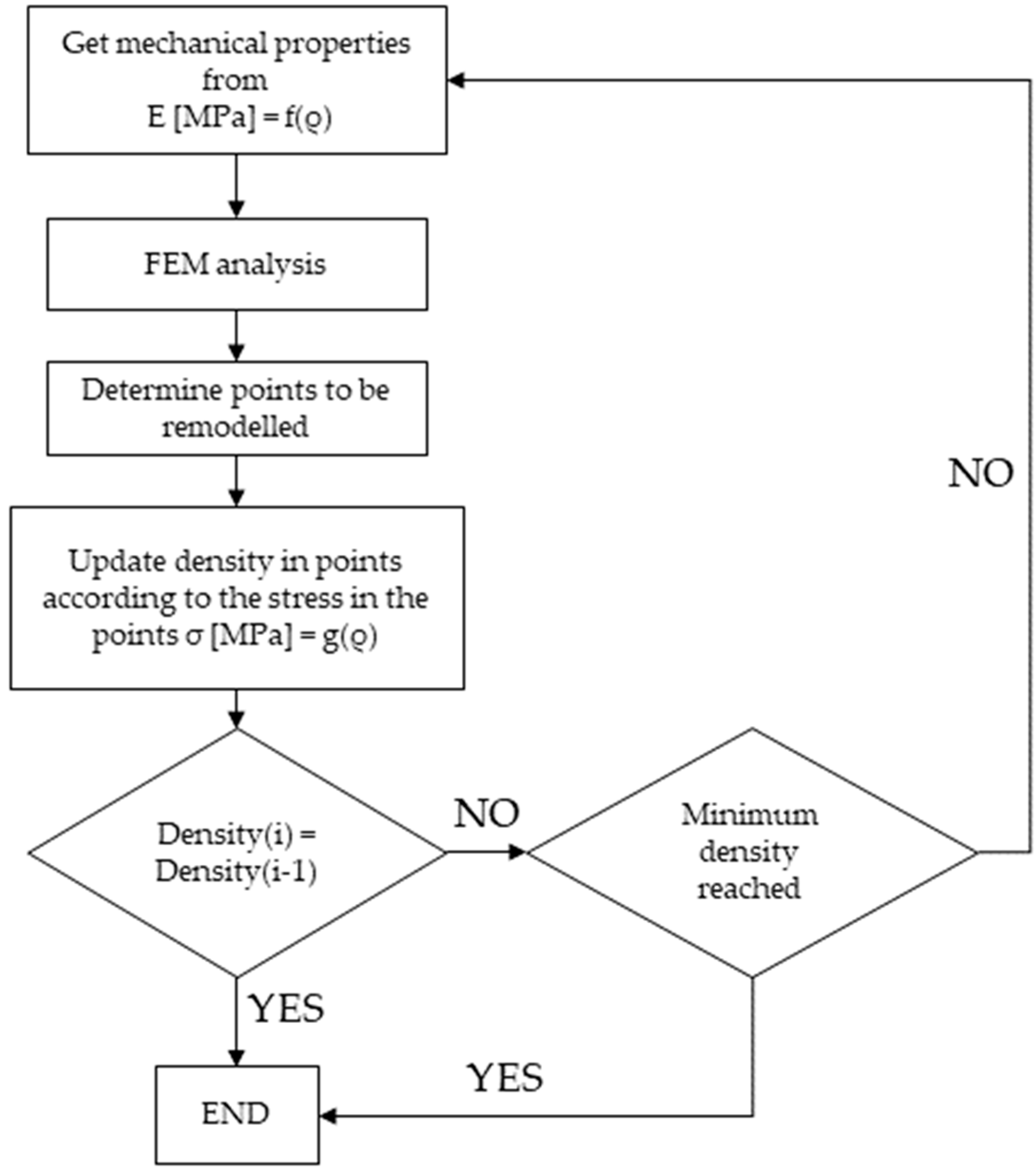
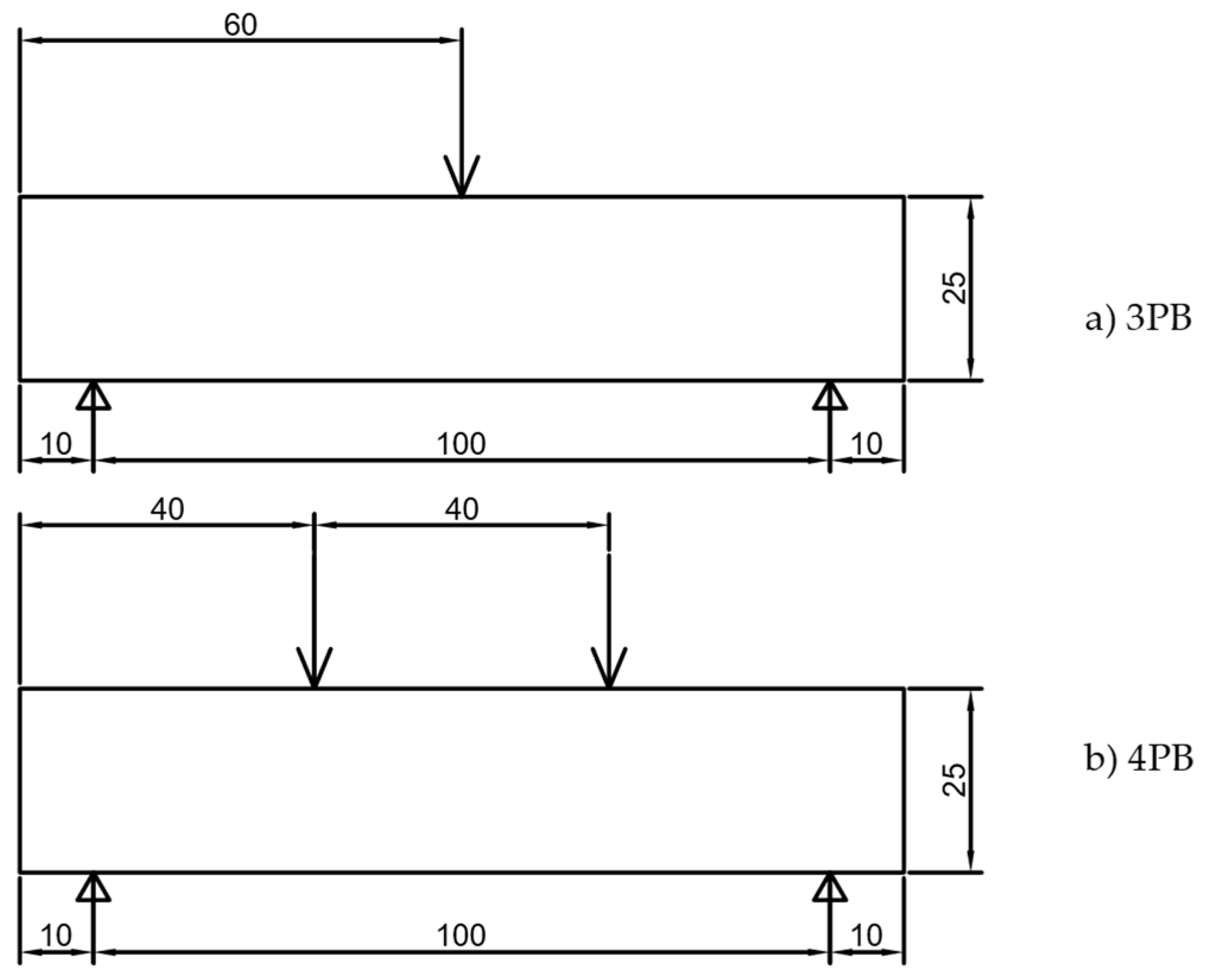
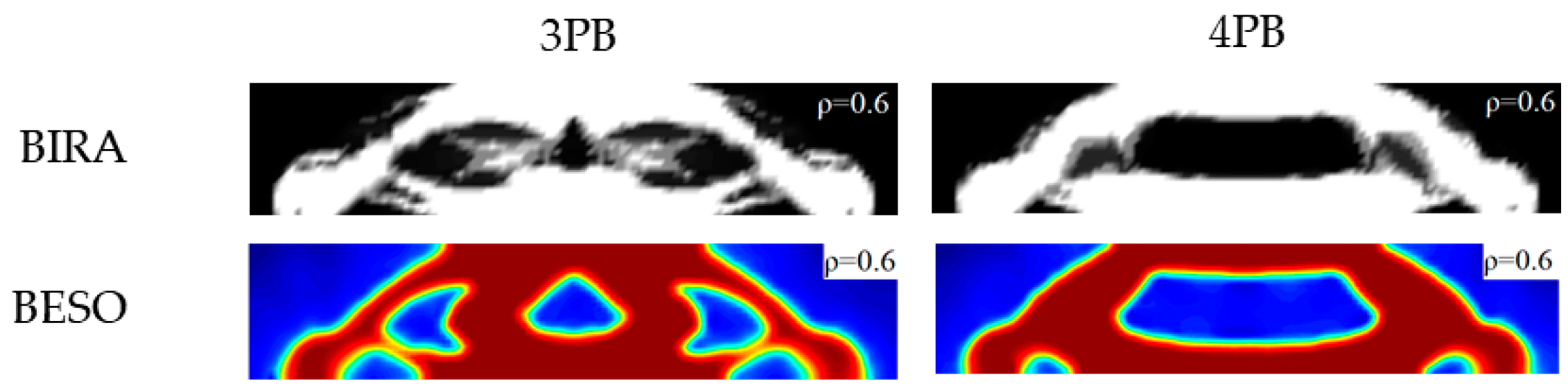
| Infill Density | Young’s Modulus (MPa) | Ultimate Stress (MPa) |
|---|---|---|
| 20% | 61 | 5 |
| 50% | 258 | 20 |
| 80% | 459 | 34 |
| Load Case | Theoretical (N/mm) | Experimental (without Smoothing) (N/mm) | Experimental (with Smoothing)(N/mm) | |
|---|---|---|---|---|
| BIRA | 3PB | 251 | 110.05 ± 0.5 | 150.04 ± 1.7 |
| 4PB | 364 | 304.65 ± 0.5 | 203.22 ± 3.8 | |
| BESO | 3PB | - | 83.77 ± 3.5 | 138.87 ± 1.2 |
| 4PB | - | 245.23 ± 6.7 | 143.42 ± 3.1 | |
| Uniform lattice | 3PB | - | 115.7 ± 2.0 | |
| 4PB | - | 176.8 ± 5.6 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pais, A.; Alves, J.L.; Belinha, J. Functional Gradients of the Gyroid Infill for Structural Optimization. Mater. Proc. 2022, 8, 72. https://doi.org/10.3390/materproc2022008072
Pais A, Alves JL, Belinha J. Functional Gradients of the Gyroid Infill for Structural Optimization. Materials Proceedings. 2022; 8(1):72. https://doi.org/10.3390/materproc2022008072
Chicago/Turabian StylePais, Ana, Jorge Lino Alves, and Jorge Belinha. 2022. "Functional Gradients of the Gyroid Infill for Structural Optimization" Materials Proceedings 8, no. 1: 72. https://doi.org/10.3390/materproc2022008072
APA StylePais, A., Alves, J. L., & Belinha, J. (2022). Functional Gradients of the Gyroid Infill for Structural Optimization. Materials Proceedings, 8(1), 72. https://doi.org/10.3390/materproc2022008072






