A Numerical Analysis on the Cyclic Behavior of 316 FR Stainless Steel and Fatigue Life Prediction †
Abstract
:1. Introduction
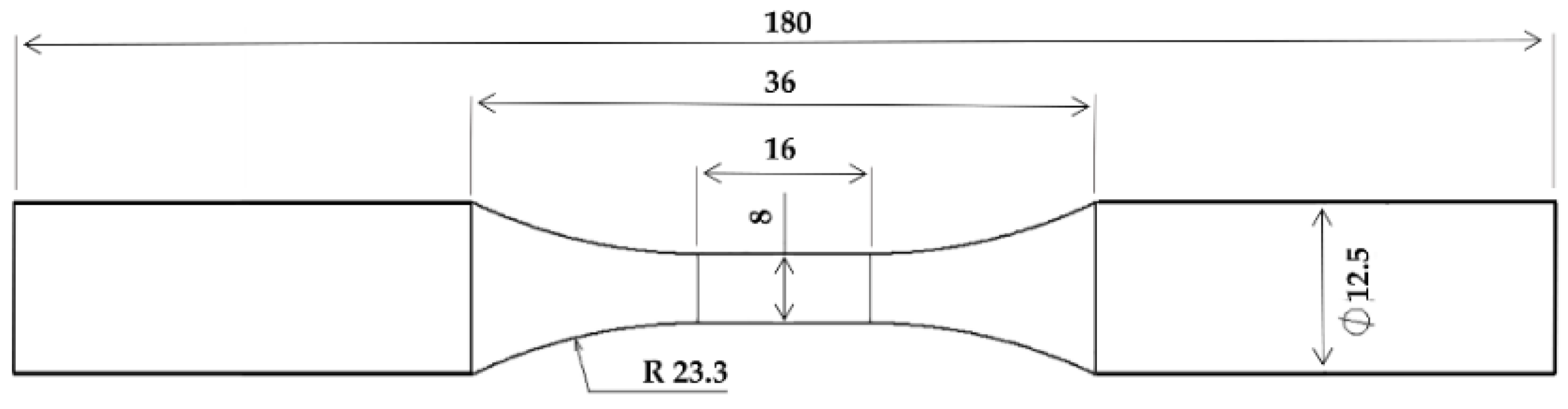
2. Experimental Conditions
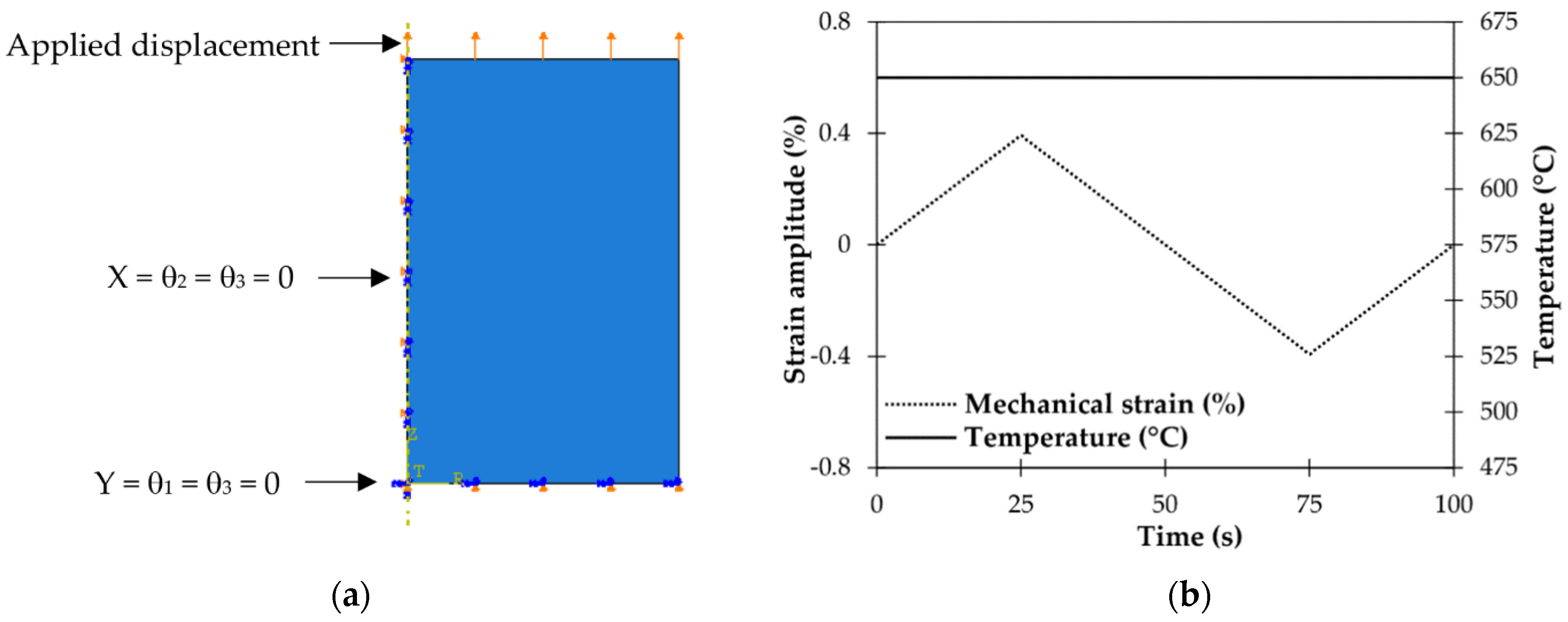
3. Finite Element Analysis
4. Results & Discussion
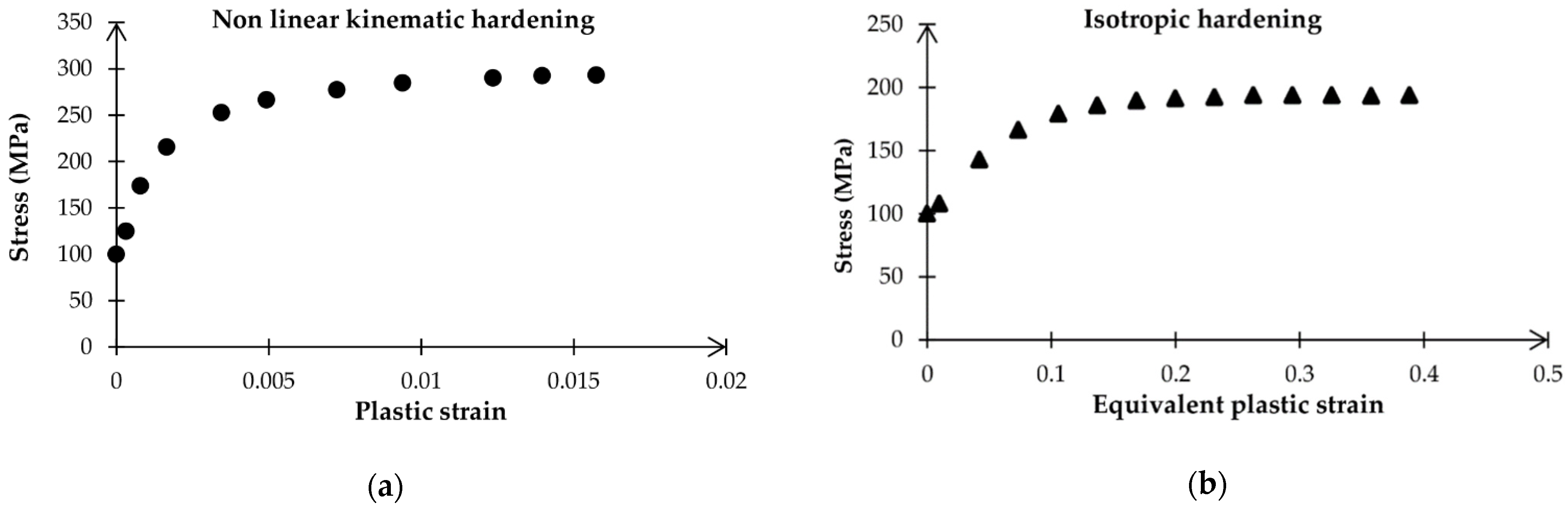
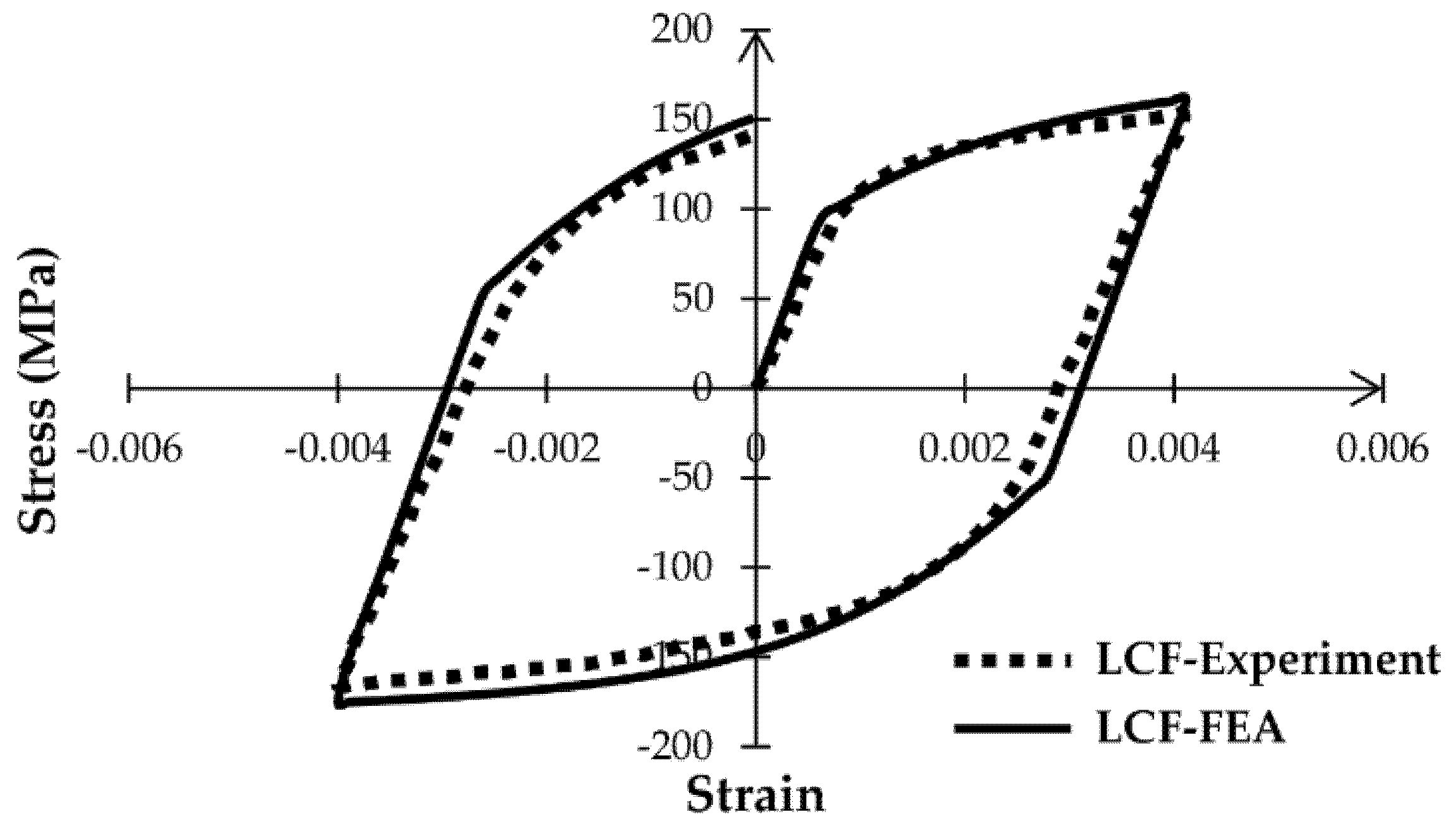
4.1. Cyclic Stress–Strain Response
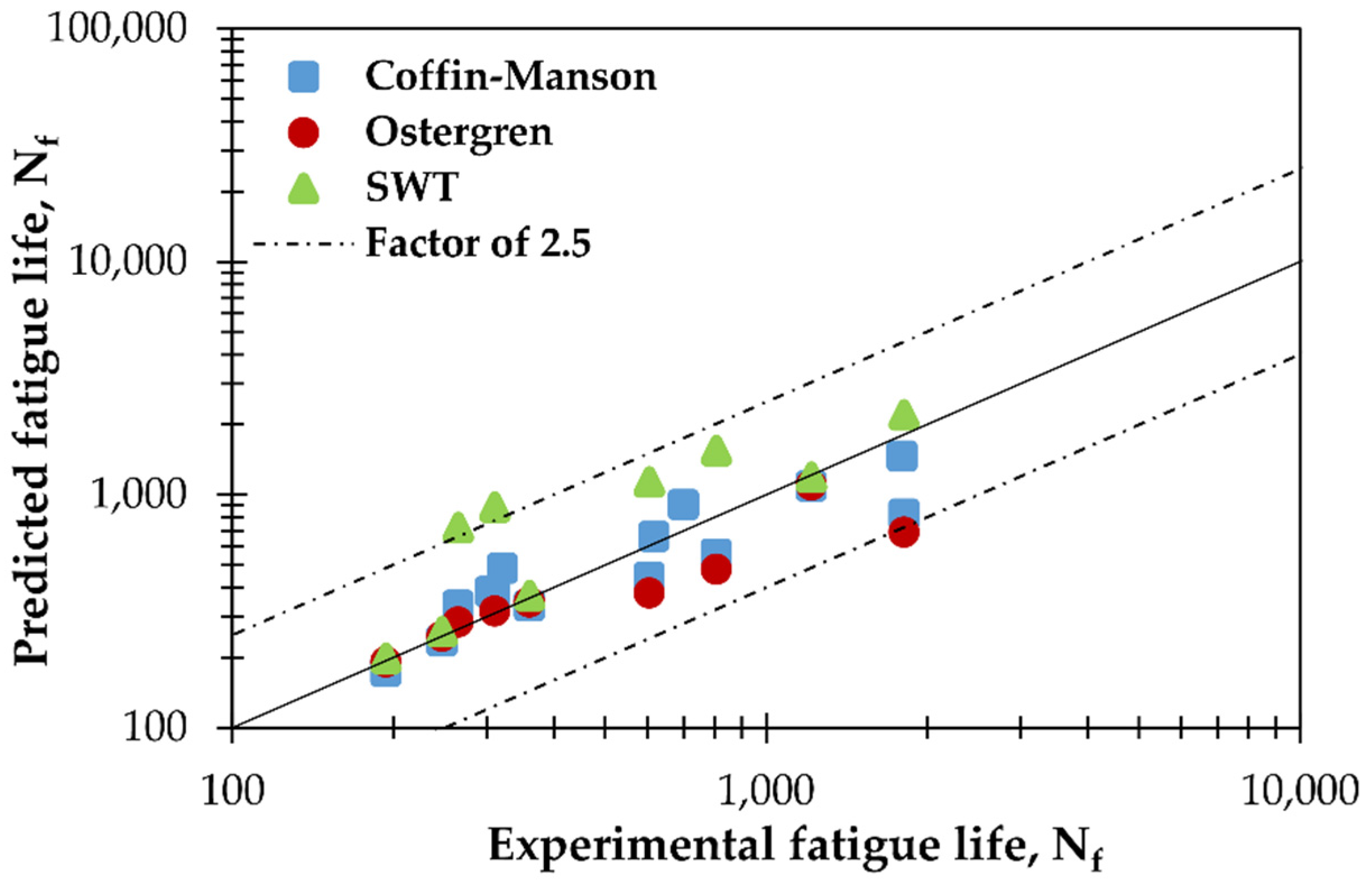
4.2. Fatigue Life Prediction
4.2.1. Coffin–Manson Model
4.2.2. Ostergren Damage Model
4.2.3. Smith–Watson–Topper Damage Model
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hormozi, R. Experimental and Numerical Simulations of Type 316 Stainless Steel Failure under LCF/TMF Loading Conditions. Ph.D. Thesis, Imperial College, London, UK, 2014. [Google Scholar]
- Coffin, L.F., Jr. A Study of the Effects of Cyclic Thermal Stresses on a Ductile Metal; Knolls Atomic Power Laboratory: Schenectady, NY, USA, 1953.
- Manson, S.S. Behavior of Materials under Conditions of Thermal Stress; National Advisory Committee for Aeronautics: Washington, DC, USA, 1954. [Google Scholar]
- Smith, K.N.; Watson, P.; Topper, T.H. A stress-strain function for the fatigue of metals. J. Mater. 1970, 5, 767–778. [Google Scholar]
- Meltzer, R.; Fiorini, Y.; Horstman, R.; Moore, I.; Batik, A.; Ostergren, W. A Damage Function and Associated Failure Equations for Predicting Hold Time and Frequency Effects in Elevated Temperature, Low Cycle Fatigue. J. Test. Eval. 1976, 4, 327. [Google Scholar] [CrossRef]
- Golos, K.; Ellyin, F. Total strain energy density as a fatigue damage parameter. In Advances in Fatigue Science and Technology; Springer: Dordrecht, The Netherlands, 1989; Volume 159, pp. 849–858. [Google Scholar] [CrossRef]
- Abarkan, I.; Shamass, R.; Achegaf, Z.; Khamlichi, A. Numerical and analytical studies of low cycle fatigue behavior of 316 LN austenitic stainless steel. J. Press. Vessel Technol. 2020. [Google Scholar] [CrossRef]
- Abarkan, I.; Khamlichi, A.; Shamass, R. A Study on low cycle fatigue life assessment of notched specimens made of 316 LN austenitic stainless steel. J. Press. Vessel. Technol. 2022, 144, 021503. [Google Scholar] [CrossRef]
- Roy, S.C.; Goyal, S.; Sandhya, R.; Ray, S. Low cycle fatigue life prediction of 316 L(N) stainless steel based on cyclic elasto-plastic response. Nucl. Eng. Des. 2012, 253, 219–225. [Google Scholar] [CrossRef]
- Dutta, A.; Dhar, S.; Acharyya, S.K. Material characterization of SS 316 in low-cycle fatigue loading. J. Mater. Sci. 2010, 45, 1782–1789. [Google Scholar] [CrossRef]
- Yuan, X.; Yu, W.; Fu, S.; Yu, D.; Chen, X. Effect of mean stress and ratcheting strain on the low cycle fatigue behavior of a wrought 316LN stainless steel. Mater. Sci. Eng. A 2016, 677, 193–202. [Google Scholar] [CrossRef]
- Hong, S.G.; Yoon, S.; Lee, S.B. The effect of temperature on low-cycle fatigue behavior of prior cold worked 316L stainless steel. J. Fatigue 2003, 25, 1293–1300. [Google Scholar] [CrossRef]
- Tak, N.H.; Kim, J.S.; Lim, J.Y. An Energy-Based Unified Approach to Predict the Low-Cycle Fatigue Life of Type 316L Stainless Steel under Various Temperatures and Strain-Rates. Materials 2019, 12, 1090. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- ABAQUS/Standard, version 6.14; Dassault Systèmes Simulia Corp.: Providence, RI, USA, 2014.
- Bartošák, M. Phenomenological Models for Lifetime Prediction under Low-Cycle Fatigue and Thermo-Mechanical Fatigue Loading Conditions. Ph.D. Thesis, Czech Technical University, Prague, Czech Republic, 2019. [Google Scholar]
| As | B | C | Co | Cr | Cu | Mn | Mo | N | Nb |
| 0.02 | 0.003 | 0.05 | 0.08 | 18.08 | 0.1 | 1.88 | 2.22 | 0.048 | 0.01 |
| Ni | P | S | Si | Sn | Ti | V | W | Zr | |
| 11.8 | 0.023 | 0.006 | 0.38 | 0.02 | 0.01 | 0.1 | 0.04 | 0.01 |
| Young’s Modulus (MPa) | Yield Strength (MPa) | Thermal Conductivity (Wm−1 °C−1) | Coefficient of Thermal Expansion (10−6 °C−1) |
|---|---|---|---|
| 160,000 | 100 | 23 | 21 |
| Coffin-Manson | Ostergren | SWT | |||
|---|---|---|---|---|---|
| εf′ | c | L (MPa) | n | C (MPa) | β |
| 0.9121 | −0.767 | 874.9 | −0.949 | 7839 | −0.378 |
| Strain Amplitude | σmax,pre | σmax,exp | RE | ∆εp,pre/2 | ∆εp,exp/2 | RE |
|---|---|---|---|---|---|---|
| (%) | (MPa) | (MPa) | (%) | (%) | (%) | (%) |
| 0.4 | 227 | 223 | 1.79 | 0.25 | 0.23 | 8.70 |
| 0.8 | 274 | 281 | −2.49 | 0.62 | 0.59 | 5.08 |
| 1 | 288 | 297 | −3.03 | 0.81 | 0.78 | 3.85 |
| 1.2 | 292 | - | - | 1.02 | - | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abarkan, I.; Khamlichi, A.; Shamass, R. A Numerical Analysis on the Cyclic Behavior of 316 FR Stainless Steel and Fatigue Life Prediction. Eng. Proc. 2021, 11, 28. https://doi.org/10.3390/ASEC2021-11116
Abarkan I, Khamlichi A, Shamass R. A Numerical Analysis on the Cyclic Behavior of 316 FR Stainless Steel and Fatigue Life Prediction. Engineering Proceedings. 2021; 11(1):28. https://doi.org/10.3390/ASEC2021-11116
Chicago/Turabian StyleAbarkan, Ikram, Abdellatif Khamlichi, and Rabee Shamass. 2021. "A Numerical Analysis on the Cyclic Behavior of 316 FR Stainless Steel and Fatigue Life Prediction" Engineering Proceedings 11, no. 1: 28. https://doi.org/10.3390/ASEC2021-11116
APA StyleAbarkan, I., Khamlichi, A., & Shamass, R. (2021). A Numerical Analysis on the Cyclic Behavior of 316 FR Stainless Steel and Fatigue Life Prediction. Engineering Proceedings, 11(1), 28. https://doi.org/10.3390/ASEC2021-11116