Theoretical Analysis of Power Conversion Efficiency of Lead-Free Double-Perovskite Cs₂TiBr₆ Solar Cells with Different Hole Transport Layers

Abstract

In recent years, there has been significant investigation into the high efficiency of perovskite solar cells. These cells have the capacity to attain efficiencies above 14%. As the perovskite materials that include lead pose a substantial environmental risk, components that are free from lead are used during the process of solar cell development. In this work, we use a lead-free double-perovskite material, namely Cs₂TiBr₆, as the main absorbing layer in perovskite solar cells to enhance power conversion efficiency (PCE). This work is centered on the development of solar cell structures with materials such as an ETL (electron transport layer) and an HTL (hole transport layer) to enhance the PCE. In this theoretical work, we perform simulations and analysis on double-perovskite Cs₂TiBr₆ to assess its efficacy as an absorber material in various HTLs like Cu₂O and CuI, with a fixed ETL of C60 using SCAPS (Solar Cell Capacitance Simulator, SCAPS 3.3.10) Software. This is a one-dimensional solar cell simulation program. In this work, the thickness of the double-perovskite material is also varied between 0.2 and 2.0 µm, and its efficiency is observed. The effect of temperature variation on efficiency in the range of 300 K to 350 K is observed. The effect of defect density on efficiency is also observed in the range of 1 × 10¹¹ to 1 × 10¹⁶. In this theoretical work, perovskite solar cells, including their absorbing layer, demonstrate outstanding ETLs and HTLs, respectively. As a result, the cells’ achieved PCE is improved. This work demonstrates the effectiveness of this lead-free double-perovskite structure that absorbs light in perovskite solar cells.

1. Introduction

The worldwide energy demand is progressively rising, requiring efficient ways of fulfilling this growing need. Renewable energy sources are highly preferred for this objective, with solar cells being one of the most effective choices. Solar cells utilize radiant energy from the sun and efficiently transform it into electrical energy. This system provides multiple benefits, such as minimal upkeep, noiseless functioning, flexibility in different weather and installation situations, portability, and an extended lifespan [1]. Photovoltaic (PV) cells, comprising thin layers of semiconductor materials like silicon, produce energy when exposed to sunlight. Subsequently, this electric current is conveyed via metallic conduits. Several cells are linked in both series and parallel configurations to form a “panel”, which can provide high current and voltage levels. PV system installation capacity surpassed 227 GW at the end of 2015. Solar cells are notable for their many useful properties, including their adaptable bandgap, low excitation binding energy, high charge mobility, and high absorption coefficient [2,3].

Solar cells made from silicon demonstrate impressive PCE, although concerns are frequently raised regarding their environmental sustainability. PCE, which is the ratio of the incident light power that is transformed into electrical energy [4]. Scientists are investigating different materials to tackle this problem. Perovskite materials are specifically intriguing in this context. The crystal structure known as perovskite, denoted by the formula ABX₃ (with X representing either an oxygen or halogen anion), is named in honor of Lev Alekseyevich Perovski. This structure consists of eight octahedrons that share corners, with A cations located in the middle cavity. Perovskite oxides are widely researched due to their remarkable ferroelectric and superconducting characteristics [5].

One new inorganic perovskite solar cell (PSC) with lead iodide as its main component was created in a recent study [6]. But, with a PCE of just 2.9%, this PSC clearly has its limitations in terms of performance. Perovskite solar cells attain PCEs of 9 to 10%, according to references [7,8,9,10,11]. The absorbing layer of these cells is typically perovskite, which is made of lead. Improving the efficiency of PSCs made of lead is a current area of study. The authors of [12] provide a detailed description of the manufacturing procedure for high-quality perovskite solar cells, including techniques for air removal during production. Impressively, a highly developed PSC with a CsPbI3 layer and a solvent-regulated process achieved a remarkable 15.7% PCE in a controlled laboratory setting [13]. Using nanoporous (np)-NiO thin films, another study [14] highlights the development of p-n-type inverted PSCs, which achieve an impressive 19% PCE. Parameter optimization and the use of new materials to increase PCE have been extensively studied in the literature. The authors of [15] emphasize the importance of modifying the absorber layer’s thickness for enhanced efficiency. A capping agent is poly diallyl dimethylammonium chloride, or PDDA [16]. Beyond that, the research looks into how various perovskite solar cell types are affected by poly(3,4-ethylenedioxythiophene)/polystyrene sulfonate (PEDOT:PSS) [5]. One study found that solution-processed planar perovskite solar cells with a polymer hole transport layer added had a PCE of 15.3% [17]. Two other crucial features of solar cells are highlighted in addition to PCE:

• Stability: Photovoltaic technologies need to meet the stability and durability standards laid out by IEC 61646 testing procedures if they are to be economically viable. Particularly for thin-film solar goods, these criteria are crucial. Hysteresis causes perovskite films to undergo metastable transformation, which drastically lowers their initial PCE. Even with consistent light intensity and temperature, this decrease can reach 50% in the first 10 h under short-circuit settings and 20% under open-circuit conditions [18].
• Toxicity: Solar cells have a long way to go before they can be mass produced due to concerns about lead poisoning, stability, and the absence of an efficient manufacturing process. There has been extensive research on the impact of lead (and tin) toxicity on human health and its dynamics. This finding has sparked significant concerns, especially about the various mechanisms through which contamination might happen. One specific area of concentration has been the impact of rainfall on PSC modules. Increased concentrations of lead can contribute to the onset of anemia, muscle weakness, as well as harmful impacts on the kidneys and brain. High levels of lead exposure can lead to mortality. Lead may traverse the placental barrier; hence, pregnant women who come into contact with lead are also subjecting their unborn child to it. Lead can have deleterious effects on the developing neurological system of an infant. Chronic lead exposure increases the risk of hypertension, cardiovascular disease, kidney disease, and reduced fertility in humans.

Prior research has demonstrated that specific solar cell devices utilizing perovskite materials including lead can attain PCEs as high as 25.2 percent [19,20,21,22]. Nevertheless, these devices utilize PSCs that incorporate lead, hence giving rise to substantial concerns regarding toxicity. Solar PV equipment toxicity is a critical issue that needs fixing right now. The commercialization of lead-based PSCs encounters obstacles owing to hazards associated with the material and worries about its stability. In light of these worries, numerous lead-free perovskite solar cell designs are currently under investigation. Recent years have seen a surge in the use of halide perovskite (HP) materials due to their exceptional optoelectronic characteristics. These include adjustable optical bandgaps, moderate mobility, broad carrier diffusion lengths, and high visible light absorption coefficients [23,24].

Both organic–inorganic and all-inorganic halide perovskites are important in several applications. The ABX3 formula describes hybrid perovskites that contain a number of different elements, including methyl ammonium (MA⁺), formamidinium (FA⁺), and inorganic cations including Cs⁺, Pb2⁺, and Sn2⁺. The halide anions denoted by X above are I, Cl, and Br, whereas the divalent cations are represented by B. The usage of ammonium cations in lead halide PSCs could lead to a PCE of 17.6% [24]. Studies [25,26] have investigated lead-based halide perovskites in a variety of combinations. Solar cells that use organic–inorganic materials and transport layers have already achieved a PCE of 26.7% according to [27]. Alternatively, [28] examines a photovoltaic solar cell with a PCE of 17.2%. Heterostructure perovskites, a type of lead halide that includes butylammonium, cesium, and formamidinium, are the subject of this investigation. In organic–inorganic halide perovskites (OHIPs), the outstanding light–matter interaction and high conductivity of the organic component combine. According to reference [29], organic–inorganic hybrid materials have notable features such as dielectric confinement and improved excitation binding energy. That after 1000 h, this solar cell still works at 80% of its original efficiency is proof of how stable it is. “However, organic cations MA⁺ and FA⁺ are very thermodynamically unstable, which makes their commercialization challenging in organic-inorganic solar cells”. One way to make a totally inorganic molecule is to replace the hygroscopic MA⁺ ion with the non-hygroscopic Cs⁺ ion [1]. Because they are less likely to quickly hydrate, lead-free halide perovskites are more stable and eco-friendlier. The focus of current research is on double-perovskite (DP) oxides, which are chemically represented as A2B′B″O6 (where A is a divalent or trivalent metal and B′ and B″ are 3d, 4d, or 5d transition metal cations). “The possibility for these materials to contribute to the development of quantum electronic/spintronic devices with low energy dissipation has prompted their investigation”. Perovskites with an ABX3 structure do not measure up to the performance of Cs₂TiBr₆, a double perovskite. The inclusion of a divalent cation B, like Cs⁺ or Cs2⁺, raises the density of charge carriers, leading to improved efficiency. Because of its great stability, non-toxicity, and abundance in nature, cesium titanium bromide (Cs₂TiBr₆) is an extremely attractive material for light absorption in integrated high-performance solar cells [30].

“The PCE of 3.3% was achieved by lead-free PSCs using Cs₂TiBr₆”. The optical, thermal, and moisture stability of Cs₂TiBr₆ was evaluated. When compared to other organic–inorganic hybrid perovskite (OIHP) materials, the results showed that Cs₂TiBr₆ performed exceptionally well. For 330 h, solar cells made of Cs₂TiBr₆ maintained a constant output [31]. The improved efficiency of Cs₂TiBr₆ double-perovskite solar cells has been the goal of numerous experimental layers of electron transport and hole transport [32].

This research aims to optimize the material choice and layer thickness of environmentally benign Cs₂TiBr₆ solar cells in order to increase their efficiency. A wide variety of electrode materials will be used to test the suggested design [33]. To overcome the impact of lead toxicity on human health and its dynamics, we consider the encapsulation of the toxic perovskite materials inside protective transparent glasses [34]. The SCAPS Device Simulator will be employed for the analysis. The outline of this paper is as follows: The research methodologies used in this study are detailed in Section 2. Section 3 displays the outcomes and conclusions that were reached. Section 4 concludes the analysis by outlining possible avenues for further investigation.

2. Materials and Methods

Numerical simulation is crucial for comprehending complicated data on complex systems and for devising and enhancing sophisticated solar cell configurations. It can be difficult to make sense of measurements without a suitable model. The performance of the specified perovskite solar cells is evaluated using the SCAPS simulator [35]. “The simulation approach relies on three fundamental equations: The Poisson equation, the hole continuity equation, and the electron continuity equation”.

Figure 1a,b depict the structures of C60/Cs₂TiBr₆/Cu₂O and C60/Cs₂TiBr₆/CuI, respectively. An energy band diagram of the proposed structures is shown in Figure 1c,d. Figure 2 illustrates the sequential processes involved in the proposed research. When the SCAPS program is started, the initial parameters, including temperature, operating voltage, and light intensity, are established. A rudimentary arrangement is subsequently replicated, comprising C60/Cs₂TiBr₆/Cu₂O and C60/Cs₂TiBr₆/CuI. The selection of this specific framework was based on the research conducted by Ahmed et al. [36].

Using Cs₂TiBr₆ as the absorber layer, this study explores the properties of perovskite solar cells, paying particular attention to the differences in the ETL and HTL. The experiment continued by utilizing many ETLs when the foundation structure was accurately represented. The best results were obtained using the C60/Cs₂TiBr₆/Cu₂O configuration. Currently, researchers are re-creating earlier trials that used C60 as an ETL in conjunction with other heterostructures.

Table 1. Parameters of different materials.

Material Properties	Cs2TiBr6	C60	Cu2O	CuI	FTO
Layer thickness (µm)	0.2 to 2	0.14	0.15	0.05	500
Optical bandgap (eV)	1.8	1.7	2.17	3.1	3.5
Affinity of electron (eV)	4.0	3.9	3.2	2.1	4
Relative permittivity	10	4.2	7.11	6.5	9
Effective DOS in the conduction band (cm−3)	6 × 1018	8 × 1019	2.2 × 1018	2.2 × 1018	2.2 × 1018
Effective DOS in the valance band (cm−3)	2.14 × 1018	8 × 1019	1.9 × 1019	1.8 × 1019	1.8 × 1019
Thermal velocity of electron (cm/s)	107	107	107	107	107
Thermal velocity of hole (cm/s)	107	107	107	107	107
Mobility of electron (cm2/Vs)	2.36 × 101	1 × 10−2	3 × 102	100	20
Hole mobility (cm2/Vs)	1.71 × 101	3.5 × 10−3	8 × 101	47.9	10
Donor density (cm−3)	−3 × 1019	2.6 × 1018	-	-	1 × 1019
Acceptor density (cm−3)	3 × 1018	-	1 × 1018	1 × 1018	-
Defect density (1/cm3)	1014	1014	1014	1014	1014
Reference	[37]	[33]	[33]	[33]	[33]

shows the parameters of different materials. The lattice of perovskites is 1 µm thick to maintain a constant structure. Using Cu₂O as a layer to increase the mobility of positive charges has not been extensively studied. Consequently, the most efficient device architecture to date has been developed, C60/Cs₂TiBr₆/Cu₂O. The next steps include fine-tuning the device’s specifications, including its thickness and defect density.

3. Results

The characteristics of the double-perovskite compound Cs₂TiBr₆ are investigated in this research. When designing our solar cells, “we kept the bandgap as mentioned. Multiple properties, such as electron affinity, relative permeability, and hole and electron mobility for different layers, are detailed in the research” [38,39]. Which ETL and which hole transport layer (HTL) are best for a given absorber layer is heavily dependent on the bandgap and electron affinity. A solar device’s PCE rises in tandem with its energy bandgap. Nevertheless, with an ideal energy bandgap, these enhancements approach saturation. Similar experimental studies have been performed for typical hybrid (organic–inorganic) PSC devices, in which charge carrier efficiency has been studied based on the selection of ETL and HTL materials, thus studying the effect of transport layer selection on the obtained PCE, i.e., towards maximizing performance and stability [40,41]. Organic semiconductor materials are also key materials for the development of solar cells [42,43,44,45].

There is no exact formula for determining the optimal ETL and HTL, and the author is aware of this. This led to the use of various materials in the simulations. Typical values for bandgap and electron affinity in the literature are between 1 and 5 eV. Using Cs₂TiBr₆ simulations, we examined the power conversion efficiency of a range of ETL and HTL materials. The solar cell configuration with the highest PCE of 8.76% was found to be C60 as the electron acceptor, Cs₂TiBr₆ as the main observing material, and Cu₂O as the hole transport layer. Curcumin as a hole transport layer (HTL) has not been extensively studied in the past. The results obtained from this setup are shown in Figure 3, showing the C60/Cs₂TiBr₆/Cu₂O structure with thickness variations in the main perovskite layer: (a) current density (Jsc) obtained at different voltages; (b) effect on open-circuit voltage (Voc) with thickness variation in main perovskite layer; (c) effect on short-circuit current density (Jsc) with thickness variation in main perovskite layer; (d) quantum efficiency; (e) Jsc with respect to different Voc; (d) effect on PCE with temperature variation.

The perovskite materials were adjusted to have a 1 µm thickness, and identical ETLs and HTLs were used for the simulations.

The quantum efficiency of a solar cell is defined as the ratio of the number of photons that reach its terminals to the number of electron–hole pairs that are captured. This proves how well photons can be converted into electric charges. A solar cell’s quantum efficiency is the amount of current it generates when exposed to photons of a specific wavelength. A higher quantum efficiency usually results in better performance. A measure of the quantity of energy transferred from photons is the photovoltaic conversion efficiency. A simple way to calculate it is to divide the amount of power that is produced by adding the current and voltage together by the amount of power that is input from sunlight. Typical values for this input power at the equator are around 1000 W/m². There is room for improvement of up to 30% based on the theoretical maximum silicon content, even though the actual efficiency is below average. An accurate measure used to evaluate the performance of solar cells is the photovoltaic conversion efficiency.

In order to determine the optimal performance, a separate experiment varied the perovskite thickness from 0.2 µm to 2 µm. Figure 3 shows that the ideal performance was attained with a thickness of 2 µm. It is important to carefully evaluate the thickness of the absorber layer in order to achieve optimal photon absorption and effectively transport the electrons and holes generated by photons to the outer contacts with minimal recombination. As a result, the power conversion efficiency increases as the thickness increases. In most cases, the perovskite layer has a thickness that is between 0.1 µm and 1 µm. At 2 µm thickness, performance improves, which is probably because photon absorption increases more than recombination losses. The effect of thickness variation on efficiency for the C60/CS₂ TiBr₆/Cu₂O structure is shown in

Table 2. Results for structure, C60/Cs₂TiBr₆/Cu₂O, thickness variation effect.

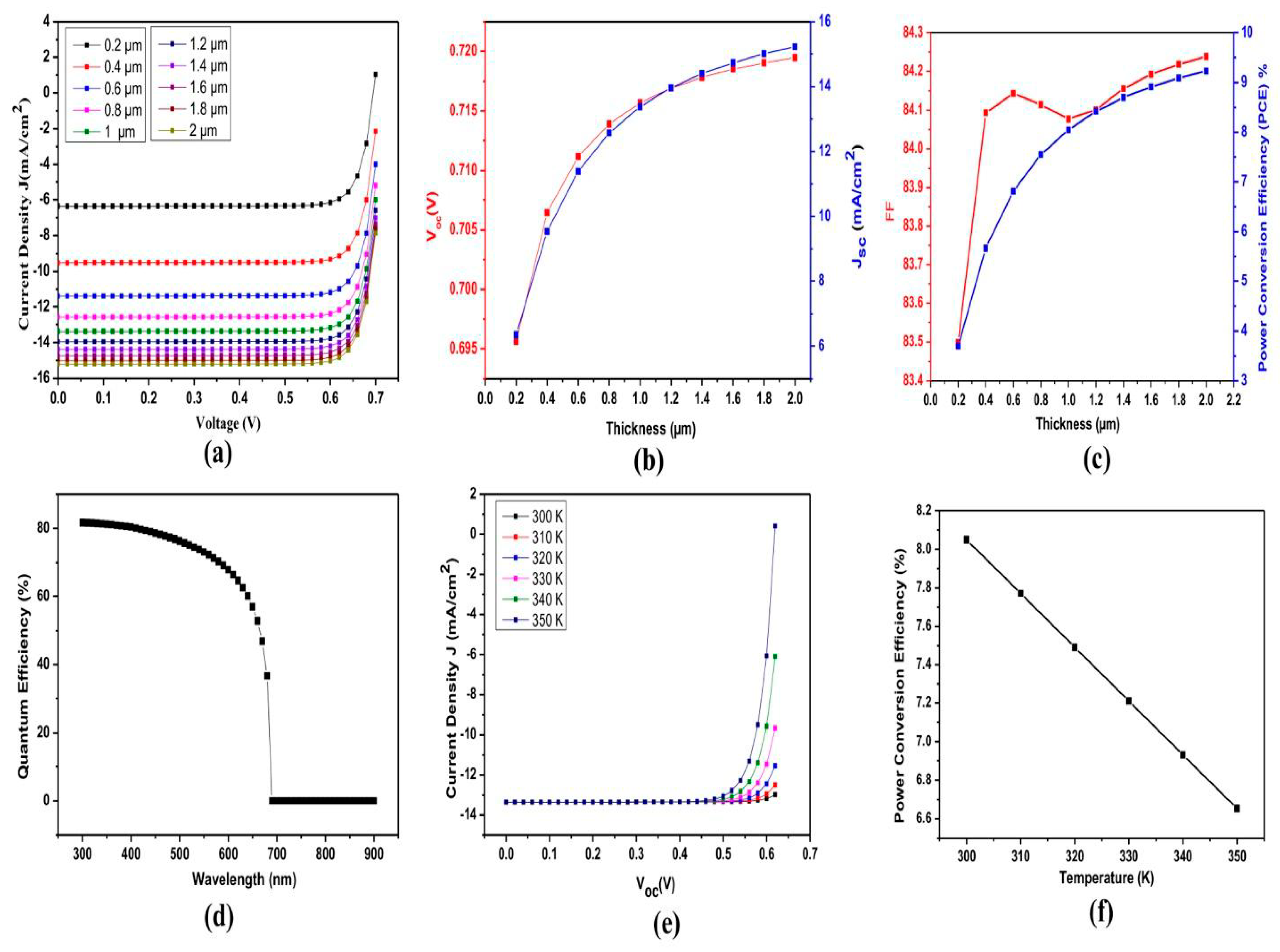
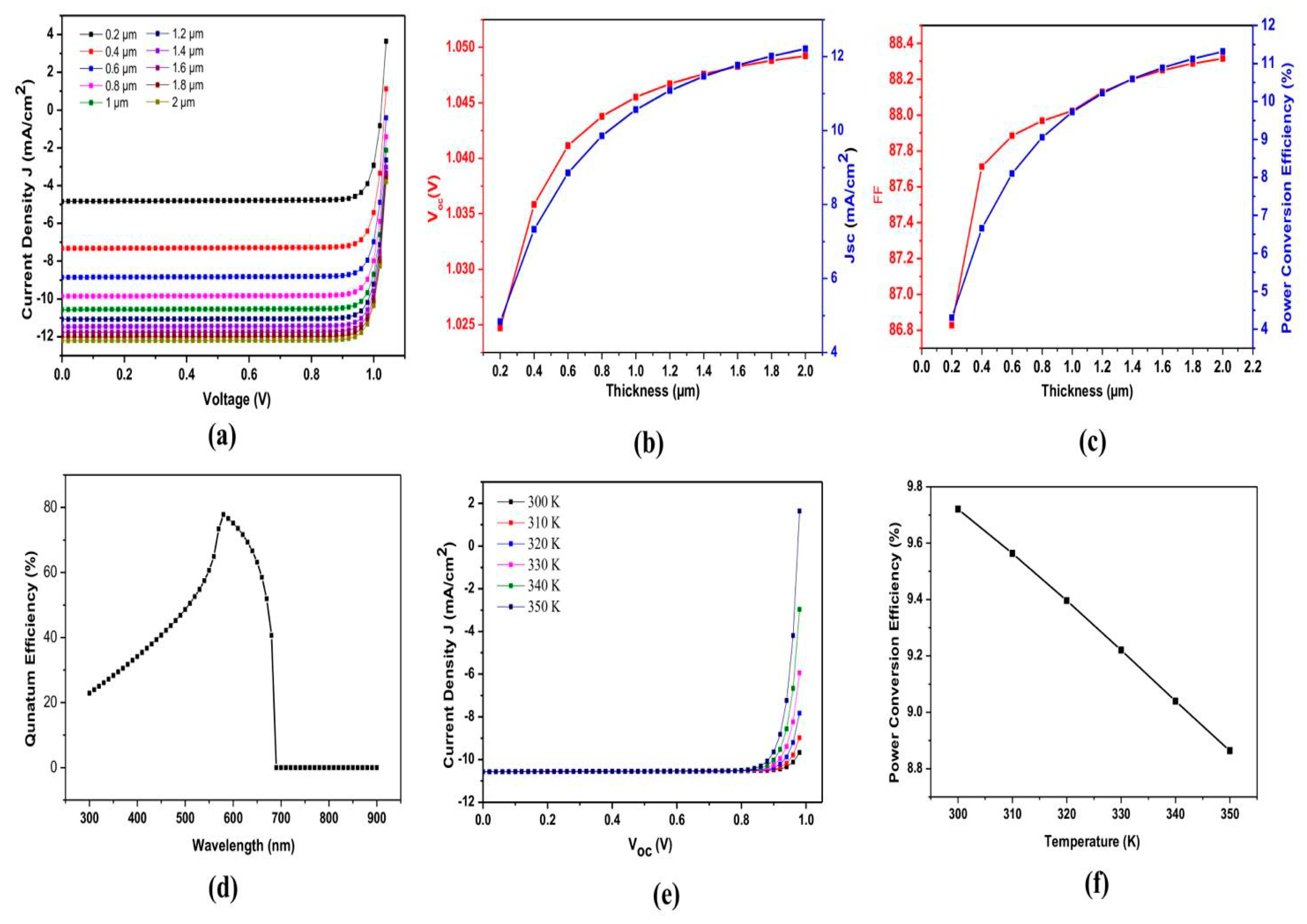
Thickness (µm)	Voc	Jsc	FF	Efficiency (%)
0.2	1.02	4.83	86.82	4.30
0.4	1.03	7.32	87.71	6.65
0.6	1.04	8.85	87.88	8.10
0.8	1.04	9.86	87.96	9.05
1	1.04	10.56	88.02	9.72
1.2	1.04	11.07	88.12	10.21
1.4	1.04	11.46	88.20	10.59
1.6	1.04	11.76	87.2	10.88
1.8	1.04	12.01	87.28	11.12
2	1.04	12.20	88.31	11.31

, and the effect of temperature variation on efficiency is shown in

Table 3. Results for structure, C60/Cs₂TiBr₆/Cu₂O, temperature variation effect.

Temperature (K)	Voc	Jsc	FF	Efficiency (%)
300	1.04	10.56	88.02	9.72
310	1.03	10.56	87.75	9.56
320	1.01	10.56	87.35	9.39
330	1.00	10.56	86.92	9.22
340	0.98	10.56	86.44	9.03
350	0.97	10.57	85.97	8.86

.

Figure 4 shows the result of the C60/Cs₂TiBr₆/CuI structure with thickness variation in the main perovskite layer: (a) current density (Jsc) obtained at different voltages; (b) effect of thickness variation in main perovskite layer on open-circuit voltage (Voc); (c) effect of thickness variation in main perovskite layer on short-circuit current density (Jsc); (d) quantum efficiency; (e) Jsc with respect to different Voc; (d) effect on PCE with temperature variation. The effect of thickness variation on efficiency for structure C60/Cs₂TiBr₆/CuI is shown in

Table 4. Results for structure, C60/Cs₂TiBr₆/CuI, temperature variation effect.

Temperature (K)	Voc	Jsc	FF	Efficiency (%)
300	0.71	13.37	84.07	8.04
310	0.69	13.37	83.38	7.77
320	0.67	13.37	82.67	7.49
330	0.65	13.37	81.96	7.21
340	0.63	13.37	81.15	6.93
350	0.61	13.37	80.34	6.65

, and the effect of temperature variation on efficiency is shown in

Table 5. Results for structure, C60/CCs₂TiBr₆/CuI, thickness variation effect.

Thickness (µm)	Voc	Jsc	FF	Efficiency (%)
0.2	0.69	6.35	83.49	3.69
0.4	0.70	9.53	84.09	5.66
0.6	0.71	11.38	84.14	6.81
0.8	0.71	12.56	84.11	7.54
1	0.71	13.37	84.07	8.04
1.2	0.71	13.96	84.10	8.41
1.4	0.71	14.39	84.15	8.69
1.6	0.71	14.73	84.19	8.91
1.8	0.71	15.00	84.21	9.08
2	0.71	15.22	84.23	9.22

.

Figure 5 shows the effect of defect density variation on the PCE of structure C60/Cs₂TiBr₆/Cu₂O and C60/Cs₂TiBr₆/CuI at 1 µm thickness.

The range of 1 × 10¹¹ to 1 × 10¹⁶ cm³ was purposefully used to alter the defect density. The effect of defect density on efficiency for structure C60/CS₂ TiBr₆/Cu₂O is shown in

Table 6. Results for structure, C60/Cs₂TiBr₆/Cu₂O, defect density effect.

Defect Density (1/cm3)	Voc	Jsc	FF	Efficiency (%)
1 × 1011	1.04	10.57	88.06	9.73
1 × 1012	1.04	10.57	88.06	9.73
1 × 1013	1.04	10.57	88.06	9.73
1 × 1014	1.04	10.56	88.02	9.72
1 × 1015	1.04	10.47	87.99	9.63
1 × 1016	1.04	9.70	87.86	8.89

, and the effect for structure C60/CS₂ TiBr₆/CuI is shown in

Table 7. Results for structure, C60/Cs₂TiBr₆/CuI, defect density effect.

Defect Density (1/cm3)	Voc	Jsc	FF	Efficiency (%)
1 × 1011	0.71	13.39	84.07	8.05
1 × 1012	0.71	13.39	84.07	8.05
1 × 1013	0.71	13.38	84.07	8.05
1 × 1014	0.71	13.37	84.07	8.04
1 × 1015	0.71	13.26	84.08	7.97
1 × 1016	0.71	12.31	84.11	7.38

. The parameters showed little change, and Jsc reached saturation at defect concentrations below 1 × 10¹⁴ cm³. An increase in defect density from 1 × 10¹¹ to 1 × 10¹⁶ cm causes a higher number of traps at recombination centers, which, in turn, causes a decline in Jsc values. Precisely 1 × 10¹⁴ cm³ was the ideal density of contact faults. Because of this, the fill factor (FF) was 88.02%, the PCE was 9.7%, the Voc was 1.04 V, and the Jsc was 10.56 mA/cm² for structure C60/CS₂ TiBr₆/Cu₂O. For structure C60/CS₂ TiBr₆/CuI, the FF was 84.07%, the PCE was 8.04, the Vocwas 1.04 V, and the Jsc was 10.56 mA/cm².

Table 8. Performance comparison.

Structure	VOC	JSC	FF	PCE (%)	Parameters Optimized
C60/Cs2TiBr6/Cu2O (Proposed) [Thickness at 2 µm]	1.04	12.20	88.31	11.31	ETL, HTL, thickness of absorbing layer, defect density
C60/LMNO/Cu2O [33]	0.19	1.92	49.5	0.43	ETL, HTL, thickness of absorbing layer, defect density

shows a performance comparison with the proposed work.

4. Conclusions

The SCAPS-1D program is utilized for conducting intricate simulations of the Cs₂TiBr₆ material, which is a lead-free double perovskite. “The primary objective of this study is to investigate the differences in the thickness, defect density, and electron affinity of the absorber, ETL, and HTL layers”. A very effective n-i-p solar cell structure is formed by utilizing a distinctive blend of C60/Cs₂TiBr₆/Cu₂O. This study investigates the influence of various HTL materials on the performance of the device and fine-tunes the thickness of the absorber layer for optimal results. The gadget has an efficiency of 9.72% and a short-circuit current density of 10.56 mA/cm² for a perovskite thickness of 1 µm and an efficiency of 11.31% and a short-circuit current density of 12.20 mA/cm² for a perovskite thickness of 2 µm. The results are consistent with prior research and have the capacity to enhance eco-friendly power conversion systems without the need for lead. These innovations have the potential to be incorporated into upcoming technology. Additional investigation could examine alternate lead-free double-perovskite materials that possess identical ETLs and HTLs, while also considering the variation in thickness for both the cases of the main perovskite materials within a range of 0.2 to 2 µm, with CuI and Cu₂O as HTLs when also considering the variation in temperature (k) on both the structures, CuI and Cu₂O, as HTLs within a range of 300 to 340. Thus, as we increase the temperature (k), the efficiency decreases, and as the thickness of the perovskite layer increases, the efficiency increases.