Finite Element Model of Concrete-Filled, Fiber-Reinforced Polymer Tubes for Small-Scale Wind Turbine Towers
Abstract
1. Introduction
2. Model Validation
2.1. Experimental Studies
2.2. Constitutive Models
2.2.1. Concrete
2.2.2. FRP
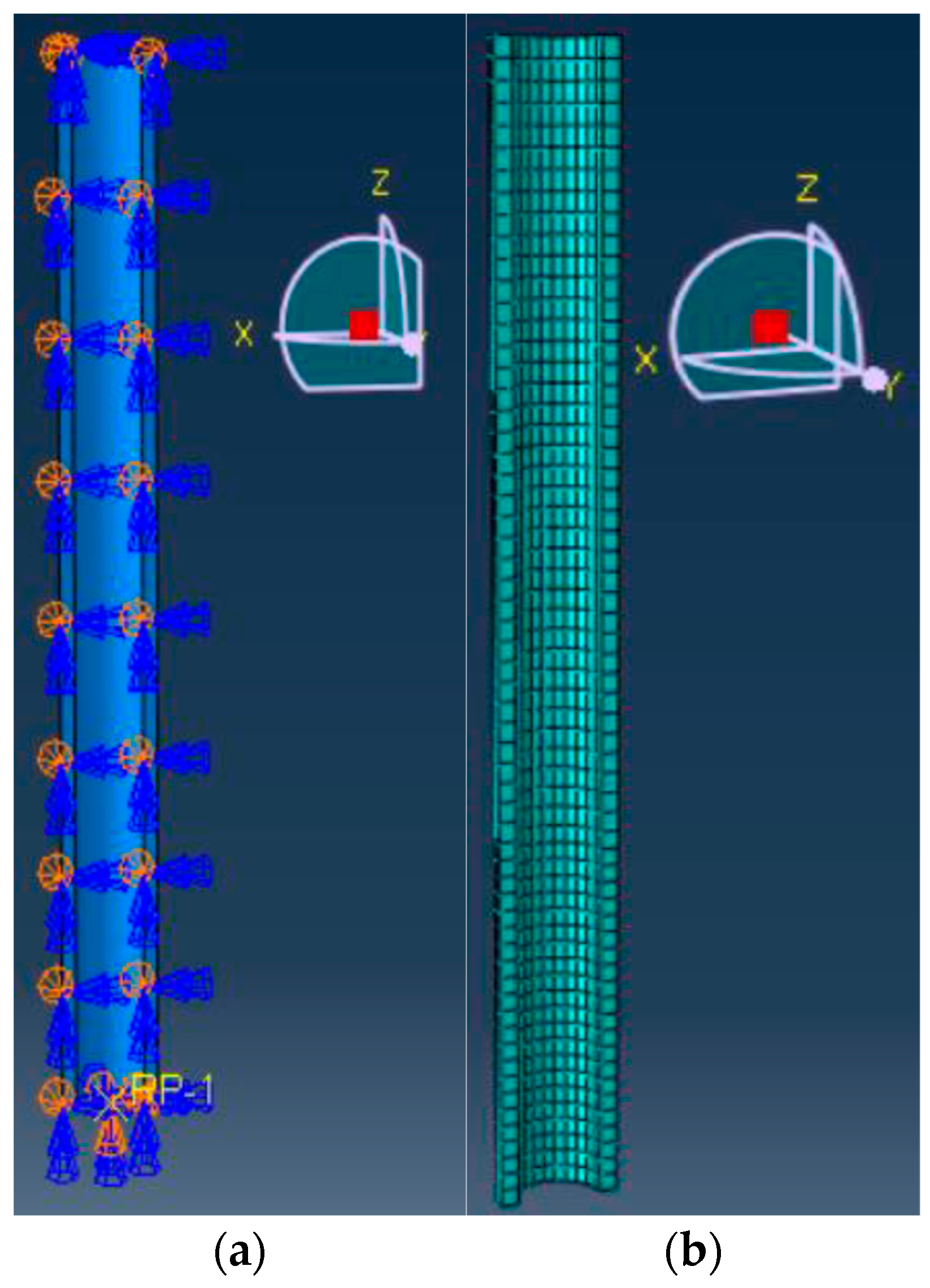
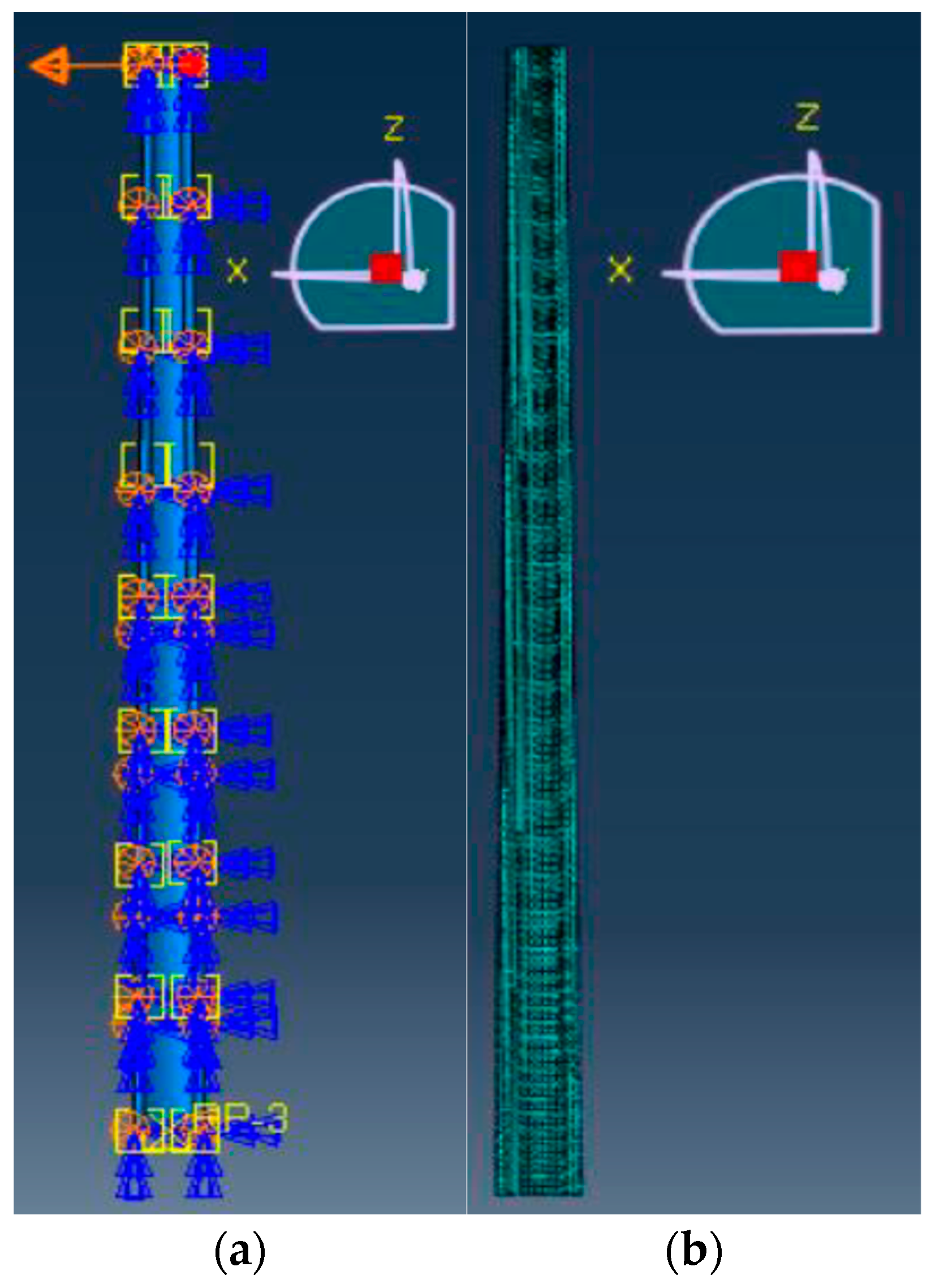
2.3. Element Type and Interaction
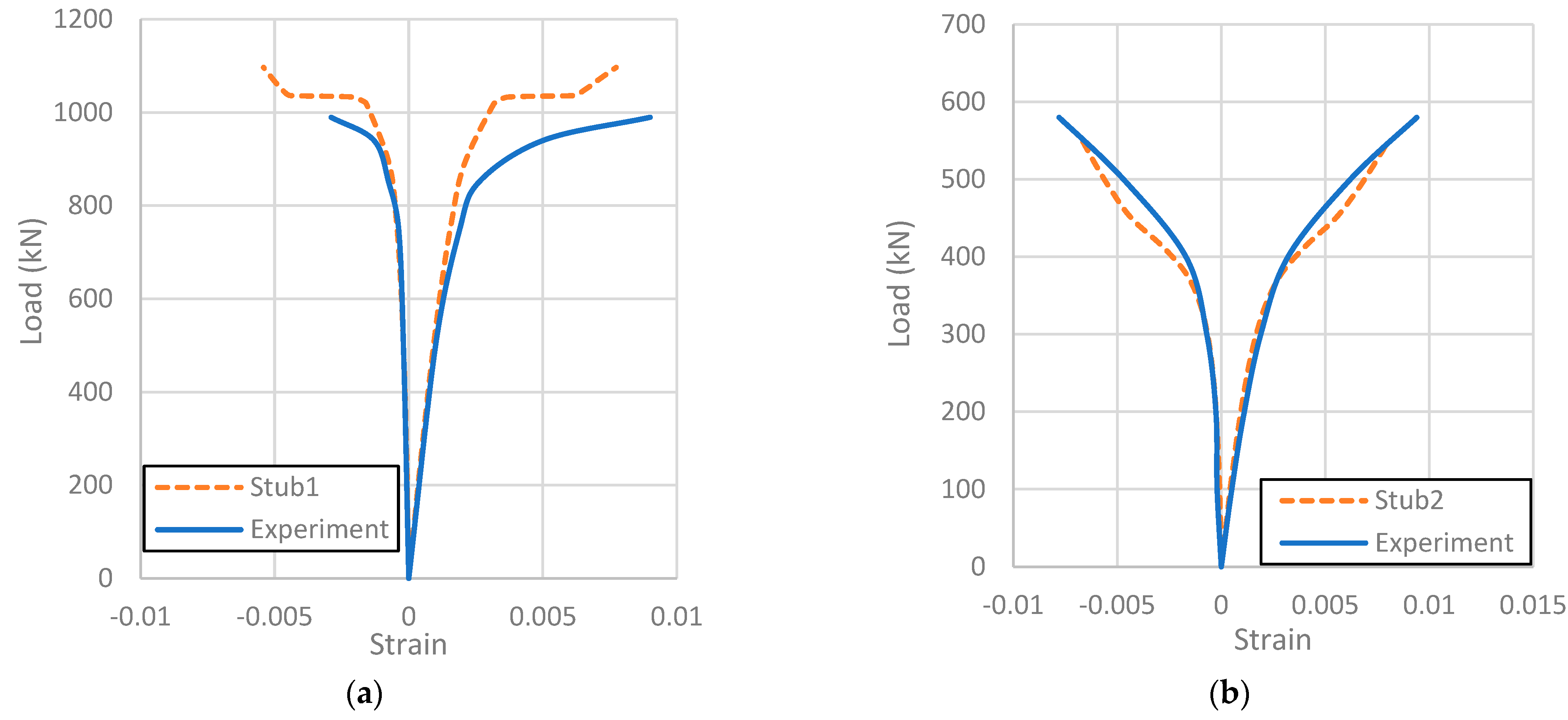
2.4. Results
3. Parametric Study
3.1. Constitutive Models and Element Type
3.2. Part A: Effect of Geometry and Reinforcement
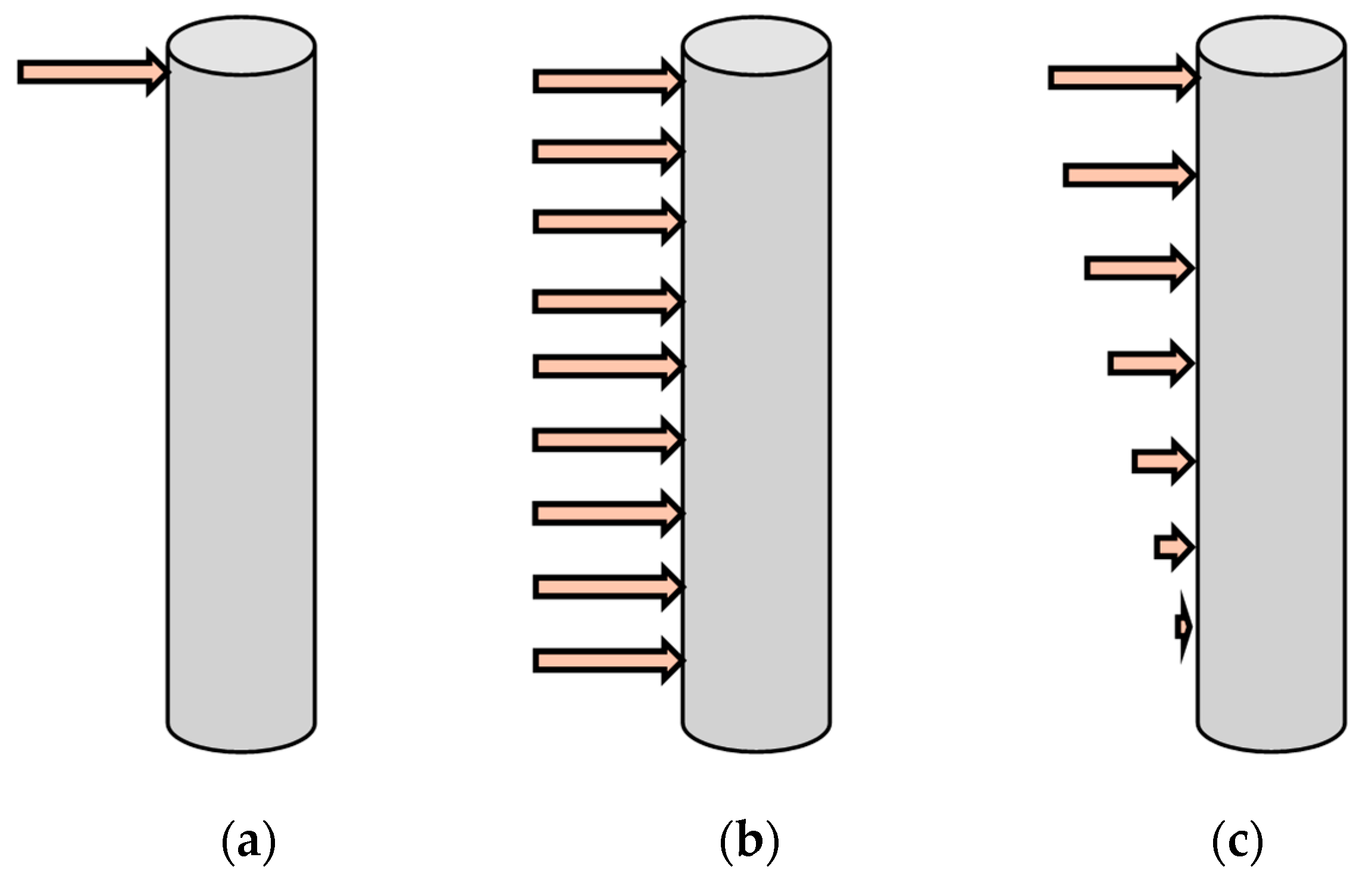
3.3. Part B: Loading Configuration
3.4. Part C: Tower Height
3.5. Part D: Tower Type
4. Results
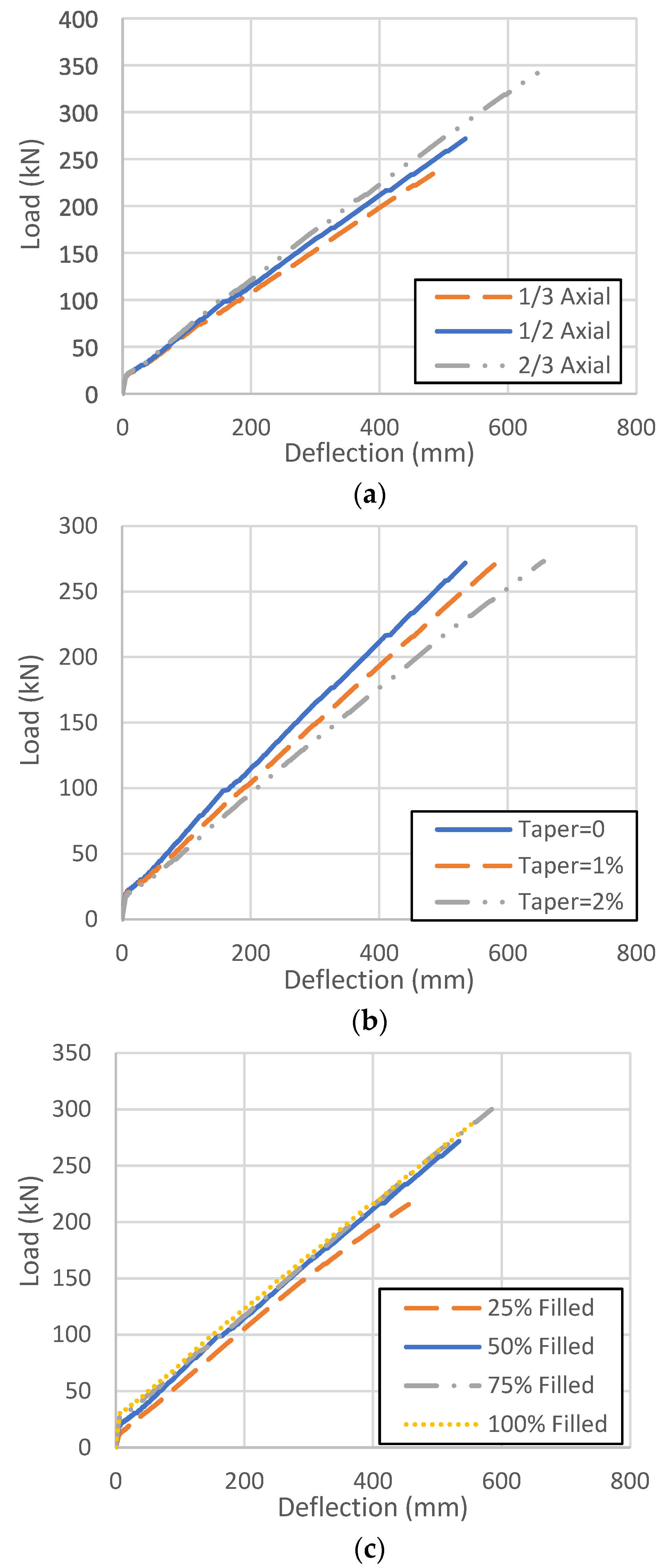
4.1. Part A: Geometry and Reinforcement
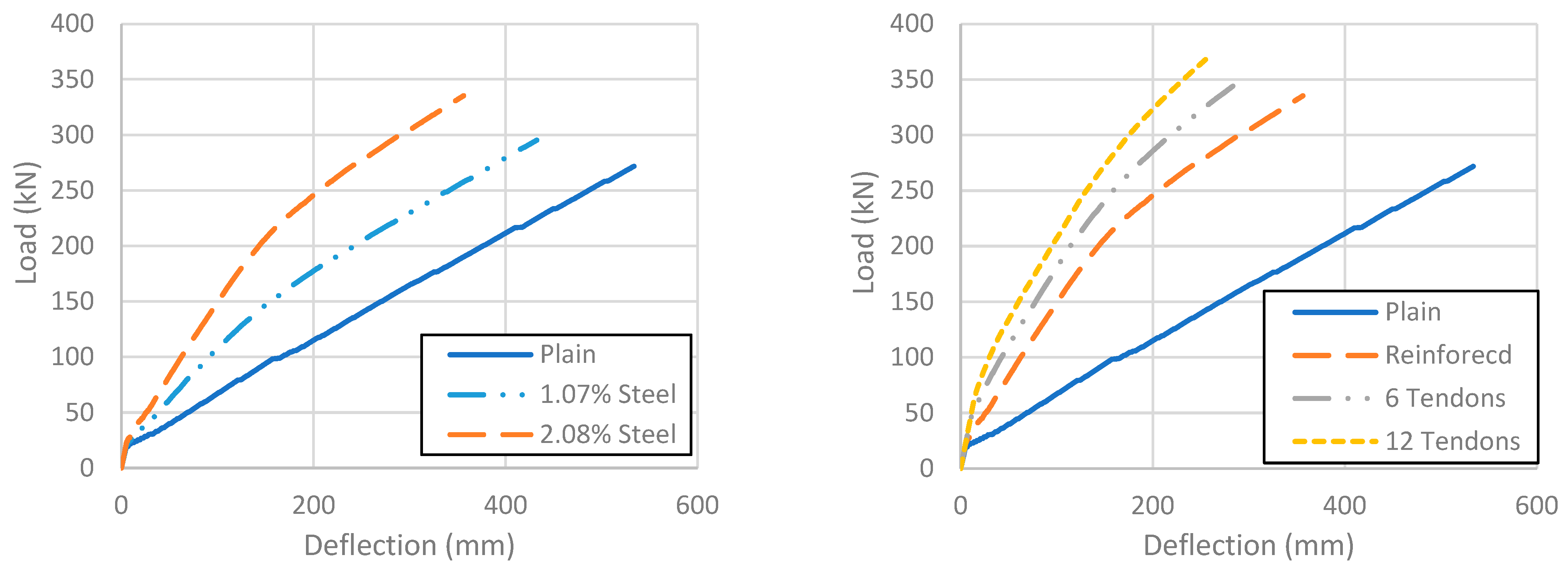
4.2. Part B: Loading Configuration
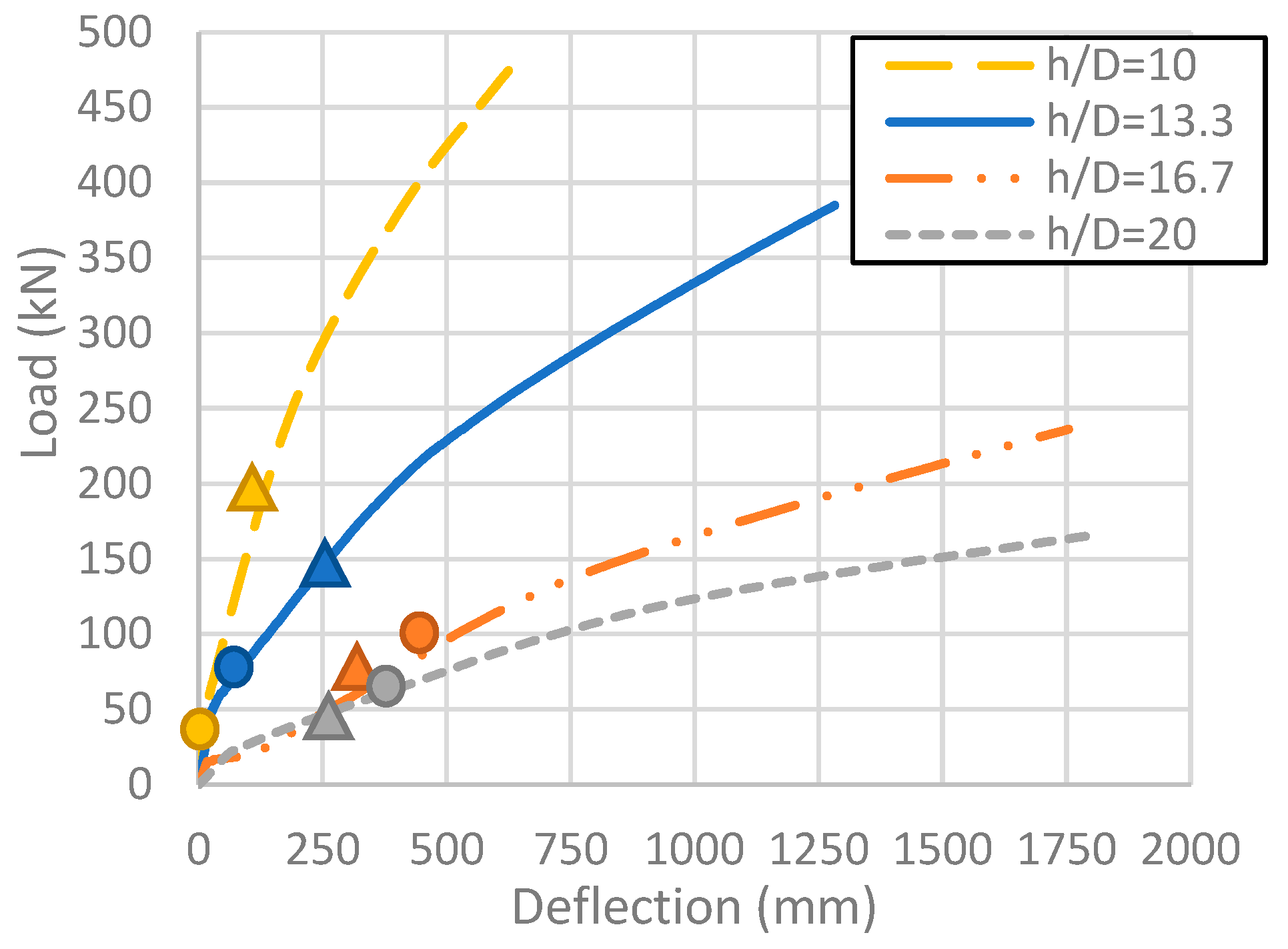
4.3. Part C: Tower Height
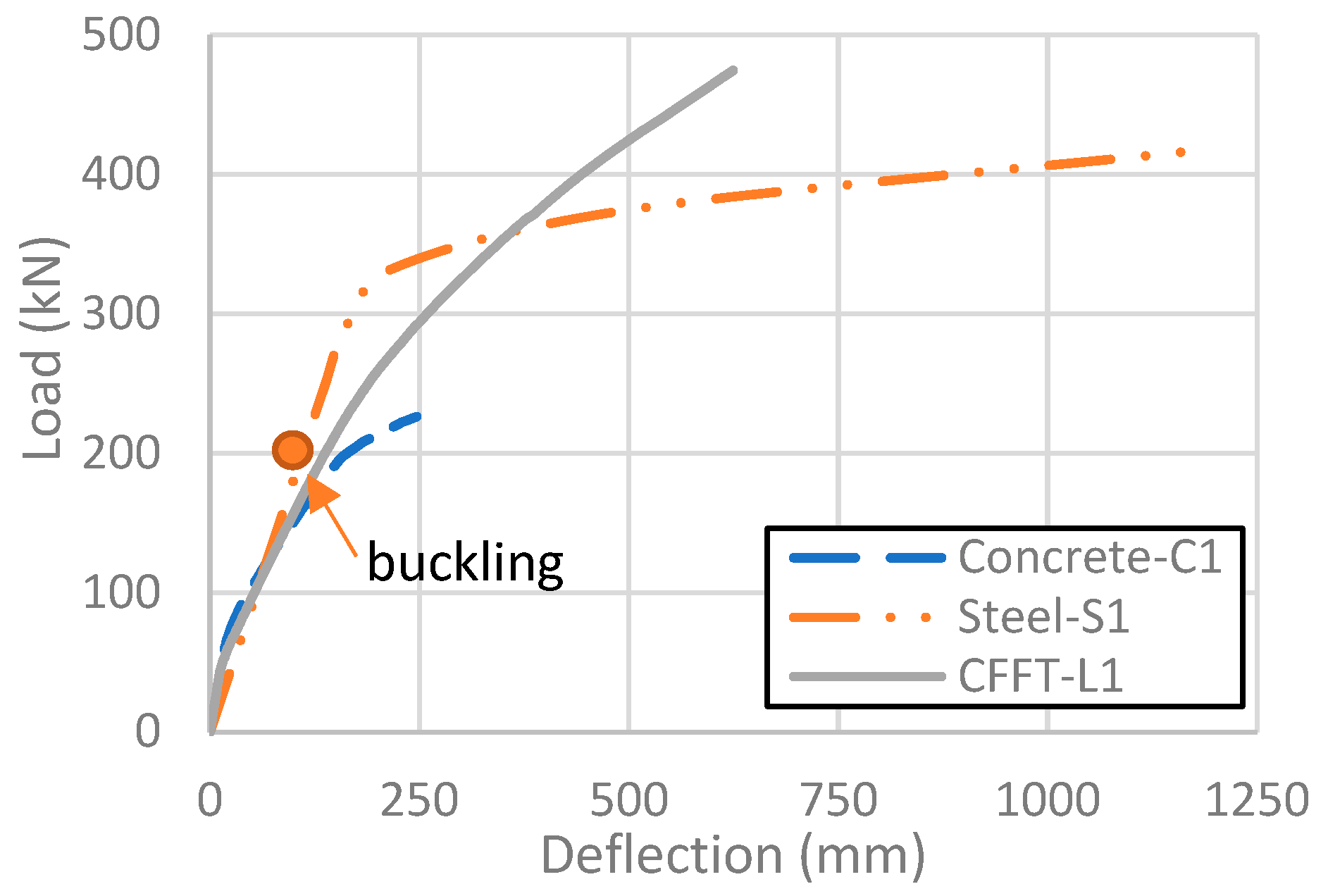
4.4. Part D: Tower Type
5. Conclusions
- Increasing the fiber ratio oriented in the longitudinal direction can increase the load capacity of wind turbine towers loaded primarily in flexure. Axial loads associated with the nacelle self-weight were negligible for the small-scale turbines considered in this study.
- The taper ratio only influences the stiffness of CFFT models and does not affect the load capacity. The use of tapered tubes is useful to facilitate segmental construction since tube segments can be “stacked” to achieve desired tower heights (the design of these segmental connections is outside the scope of the present study).
- Increasing the concrete filling ratio improves the behavior of CFFT models up to approximately 50%; however, further increases in the filling ratio had only a marginal effect on structural tower performance.
- Increasing the steel reinforcement ratio and prestressing ratio increased the load capacity and stiffness of the towers while also resulting in non-linear load–deflection behavior. The use of post-tensioned strands can potentially provide continuity for segmental tower construction (this is the subject of ongoing research).
- Wind turbine towers are subjected to combined loading that includes distributed tower loads, concentrated lateral loads, torsional moments, and axial loads due to self-weight. In this study, the simplified load case corresponding to a single concentrated load applied at the top of the tower resulted in the lowest load capacity and the highest deflection, suggesting that it can be used conservatively to develop a preliminary tower design. The effects of load eccentricity were not significant. Further research employing computational fluid dynamics simulations is recommended to confirm the dynamic response of these tower systems.
- With the same prestressing level and geometry, the CFFT model showed better behavior in load capacity, stiffness, and local stability compared with other tower types. In addition to its structural performance, the CFFT tower system is expected to be a durable and low-maintenance alternative for wind energy in remote areas.
- Increasing the h/D ratio decreases the load capacity and increases the displacement at failure. Though the deflection limit differs for different model heights, the load at the deflection limit decreases with the increase in the h/D ratio. Based on the tower configurations considered in this study, a preliminary design of a CFFT wind turbine tower is recommended to have an approximately 15 h/D ratio, 2% taper ratio, 50% concrete filling ratio, 10 mm GFRP tube thickness, and 2% steel reinforcement ratio. The prestressing level and other parameters can be refined based on the calculated wind loads and other salient factors such as location and turbine size.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
List of Symbols
| cross-sectional area of prestressing tendons | |
| projected area of wind turbine blades | |
| projected area of wind turbine nacelle | |
| projected area of wind turbine tower | |
| number of blades in the wind turbine | |
| coefficient of thermal expansion | |
| equivalent temperature to simulate prestressing in numerical model | |
| drag coefficient, assumed to be 1.5 | |
| force coefficient of nacelle, assumed to be 1.5 | |
| force coefficient of the tower, assumed to be 1.3 | |
| maximum lift coefficient at the tip, assumed to be 2.0 | |
| diameter of wind turbine tower | |
| maximum deflection of the turbine tower | |
| deflection of the turbine tower at service | |
| Young’s modulus | |
| Young’s modulus of FRP lamina parallel to the fibers | |
| Young’s modulus of FRP lamina perpendicular to the fibers | |
| Ec | Young’s modulus of concrete |
| compressive strength of concrete | |
| strength tensor of FRP in direction i | |
| applied load corresponding to deflection limit | |
| ultimate load capacity of wind turbine tower | |
| horizontal force on turbine nacelle | |
| ultimate tensile strength of steel prestressing tendons | |
| tensile strength of concrete | |
| horizontal force on wind turbine tower | |
| horizontal drag force on wind turbine | |
| yield strength of steel reinforcement | |
| shear modulus of FRP lamina | |
| wind turbine tower height | |
| Tsai–Wu failure criterion | |
| ultimate flap bending moment of the wind turbine tower | |
| applied prestressing force | |
| radius of the wind turbine | |
| extreme wind speed with a 50-year recurrence time interval | |
| concrete strain | |
| concrete strain at peak compressive stress | |
| cracking strain of concrete | |
| yield strain of steel reinforcement | |
| 50-year extreme tip steep ratio | |
| υ | Poisson’s ratio for concrete |
| Poisson’s ratio of FRP lamina | |
| air density, assumed to be equal to 1.225 kg/m3 | |
| stress in FRP in direction i, j used to calculate Tsai–Wu criterion | |
| shear strength of FRP lamina | |
| compressive strength of FRP lamina parallel to fibers | |
| compressive strength of FRP lamina perpendicular to fibers | |
| tensile strength of FRP lamina parallel to fibers | |
| tensile strength of FRP lamina perpendicular to fibers |
References
- Knowles, J. Power Shift: Electricity for Canada’s Remote Communities; The Conference Board of Canada: Ottawa, ON, Canada, 2016; p. 76. [Google Scholar]
- Shafiei, A.; Dehkordi, B.M.; Farhangi, S.; Kiyoumarsi, A. Overall power control strategy for small-scale WECS incorporating flux weakening operation. IET Renew. Power Gener. 2016, 10, 1264–1277. [Google Scholar] [CrossRef]
- Chen, H.; Xu, D.; Deng, X. Control for Power Converter of Small-Scale Switched Reluctance Wind Power Generator. IEEE Trans. Ind. Electron. 2021, 68, 3148–3158. [Google Scholar] [CrossRef]
- de Lana, J.A.; Júnior, P.A.A.M.; Magalhães, C.A.; Magalhães, A.L.M.A.; de Andrade Junior, A.C.; de Barros Ribeiro, M.S. Behavior study of prestressed concrete wind-turbine tower in circular cross-section. Eng. Struct. 2021, 227, 111403. [Google Scholar] [CrossRef]
- Veljkovic, M.; Feldmann, M.; Naumes, J.; Pak, D.; Simões, L.; da Silva; Rebelo, C. 9—Wind turbine tower design, erection and maintenance. In Wind Energy Systems; Sørensen, J.D., Sørensen, J.N., Eds.; Woodhead Publishing: Sawsto, UK, 2011; pp. 274–300. [Google Scholar] [CrossRef]
- Fam, A.; Cole, B.; Mandal, S. Composite tubes as an alternative to steel spirals for concrete members in bending and shear. Constr. Build. Mater. 2007, 21, 347–355. [Google Scholar] [CrossRef]
- Shan, B.; Gui, F.C.; Monti, G.; Xiao, Y. Effectiveness of CFRP Confinement and Compressive Strength of Square Concrete Columns. J. Compos. Constr. 2019, 23, 04019043. [Google Scholar] [CrossRef]
- Watfa, A.; Green, M.; Fam, A.; Noël, M. Segmental hollow concrete filled FRP tubes (CFFT) for wind turbine towers. In Proceedings of the 10th International Conference on FRP composites in Civil Engineering, İstanbul, Turkey, 8–10 December 2021. [Google Scholar]
- Burgoyne, C. Fiber Reinforced Polymers–Strengths, Weaknesses, Opportunities And Threats. In Proceedings of the 9th International symposium on Fiber Reinforced Polymer Reinforcement for Concrete Structures (FRPRCS-9), Sydney, Australia, 13–15 July 2009. [Google Scholar]
- Van Den Einde, L.; Zhao, L.; Seible, F. Use of FRP composites in civil structural applications. Constr. Build. Mater. 2003, 17, 389–403. [Google Scholar] [CrossRef]
- Dagher, H.J.; Bannon, D.J.; Davids, W.G.; Lopez-Anido, R.A.; Nagy, E.; Goslin, K. Bending behavior of concrete-filled tubular FRP arches for bridge structures. Constr. Build. Mater. 2012, 37, 432–439. [Google Scholar] [CrossRef]
- Fam, A.; Pando, M.; Filz, G.; Rizkalla, S. Precast Piles for Route 40 Bridge in Virginia Using Concrete Filled FRP Tubes. PCI J. 2003, 48, 32–45. [Google Scholar] [CrossRef]
- Hasak, A.; Noel, M.; Green, M.; Fam, A. Flexural behavior of post-tensioned concrete filled FRP tube for wind turbine tower applications. In Proceedings of the 11th International Conference on FRP Composites in Civil Engineering, Rio de Janeiro, Brazil, 23–26 July 2023. [Google Scholar]
- Rizzo, F.; D’Alessandro, V.; Montelpare, S.; Giammichele, L. Computational study of a bluff body aerodynamics: Impact of the laminar-to-turbulent transition modelling. Int. J. Mech. Sci. 2020, 178, 105620. [Google Scholar] [CrossRef]
- IEC 61400-2; Wind Turbines—Part 2: Design Requirements for Small Wind Turbines. International Electrotechnical Commission: Geneva, Switzerland, 2006.
- Son, J.-K.; Fam, A. Finite element modeling of hollow and concrete-filled fiber composite tubes in flexure: Model development, verification and investigation of tube parameters. Eng. Struct. 2008, 30, 2656–2666. [Google Scholar] [CrossRef]
- Fam, A.; Son, J.-K. Finite element modeling of hollow and concrete-filled fiber composite tubes in flexure: Optimization of partial filling and a design method for poles. Eng. Struct. 2008, 30, 2667–2676. [Google Scholar] [CrossRef]
- El-Nemr, A.M.; Ashour, O.; Hekal, G.M. Finite element modeling of confined concrete piles with FRP tubes in sandy soil under static loading. In Insights and Innovations in Structural Engineering, Mechanics and Computation; CRC Press: Boca Raton, FL, USA, 2016; pp. 2122–2127. [Google Scholar]
- Raza, A.; ur Rehman, A.; Masood, B.; Hussain, I. Finite element modelling and theoretical predictions of FRP-reinforced concrete columns confined with various FRP-tubes. Structures 2020, 26, 626–638. [Google Scholar] [CrossRef]
- Cao, Y.; He, M.; Ma, R.; Yang, R.; Liang, F. Beam-column modeling and seismic fragility analysis of a prestressed segmental concrete tower for wind turbines. Adv. Struct. Eng. 2020, 23, 1715–1727. [Google Scholar] [CrossRef]
- Fam, A.Z. Concrete-Filled Fiber Reinforced Polymer Tubes for Axial and Flexural Structural Members. Ph.D. Thesis, The University of Manitoba, Winnipeg, MB, USA, 2000. [Google Scholar]
- Hognestad, E. A Study of Combined Bending and Axial Load in Reinforced Concrete Members; University of Illinois: Urbana, IL, USA, 1951. [Google Scholar]
- Al-Ilani, M.; Temsah, Y. Comparative study of modeling methods used to simulate initial stresses in prestressed beams towards manual analysis. MATEC Web Conf. 2019, 281, 01014. [Google Scholar] [CrossRef]
- Ren, W.; Sneed, L.H.; Yang, Y.; He, R. Numerical Simulation of Prestressed Precast Concrete Bridge Deck Panels Using Damage Plasticity Model. Int. J. Concr. Struct. Mater. 2015, 9, 45–54. [Google Scholar] [CrossRef]
- JCSS. Probabilistic Model Code Part III—Resistance Models; Joint Committee on Structural Safety: Zürich, Switzerland, 2005. [Google Scholar]
- Khedmati, M.; Nouri, Z.; Roshanali, M. A comparative computational investigation on the effects of randomly distributed general corrosion on the post-buckling behaviour of uniaxially loaded plates. J. Mech. Sci. Technol. 2013, 26, 767–783. [Google Scholar] [CrossRef]
- Nicholson, J.C. Design of Wind Turbine Tower and Foundation Systems: Optimization Approach. Master’s Thesis, University of Iowa, Iowa City, IA, USA, 2011. [Google Scholar]

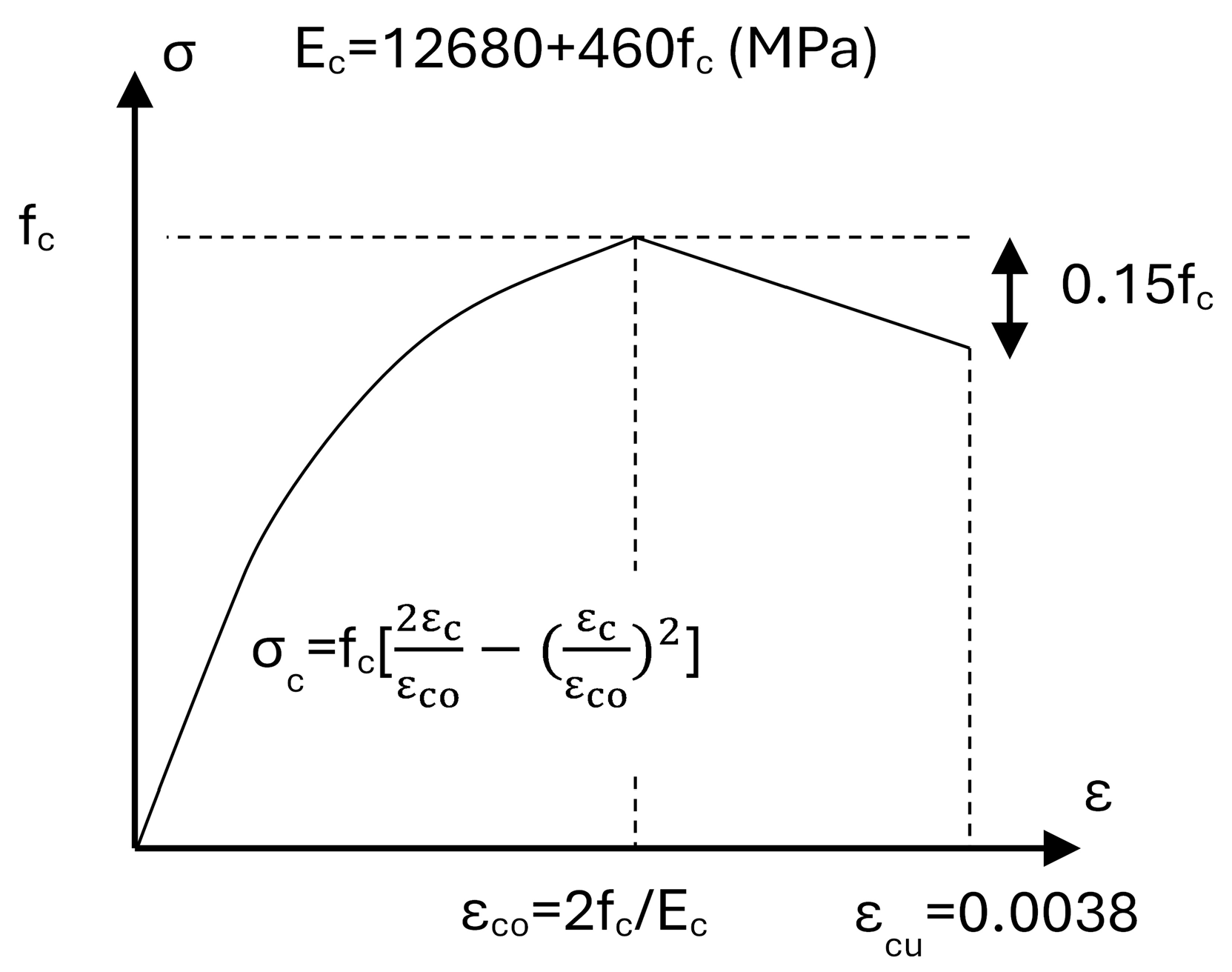

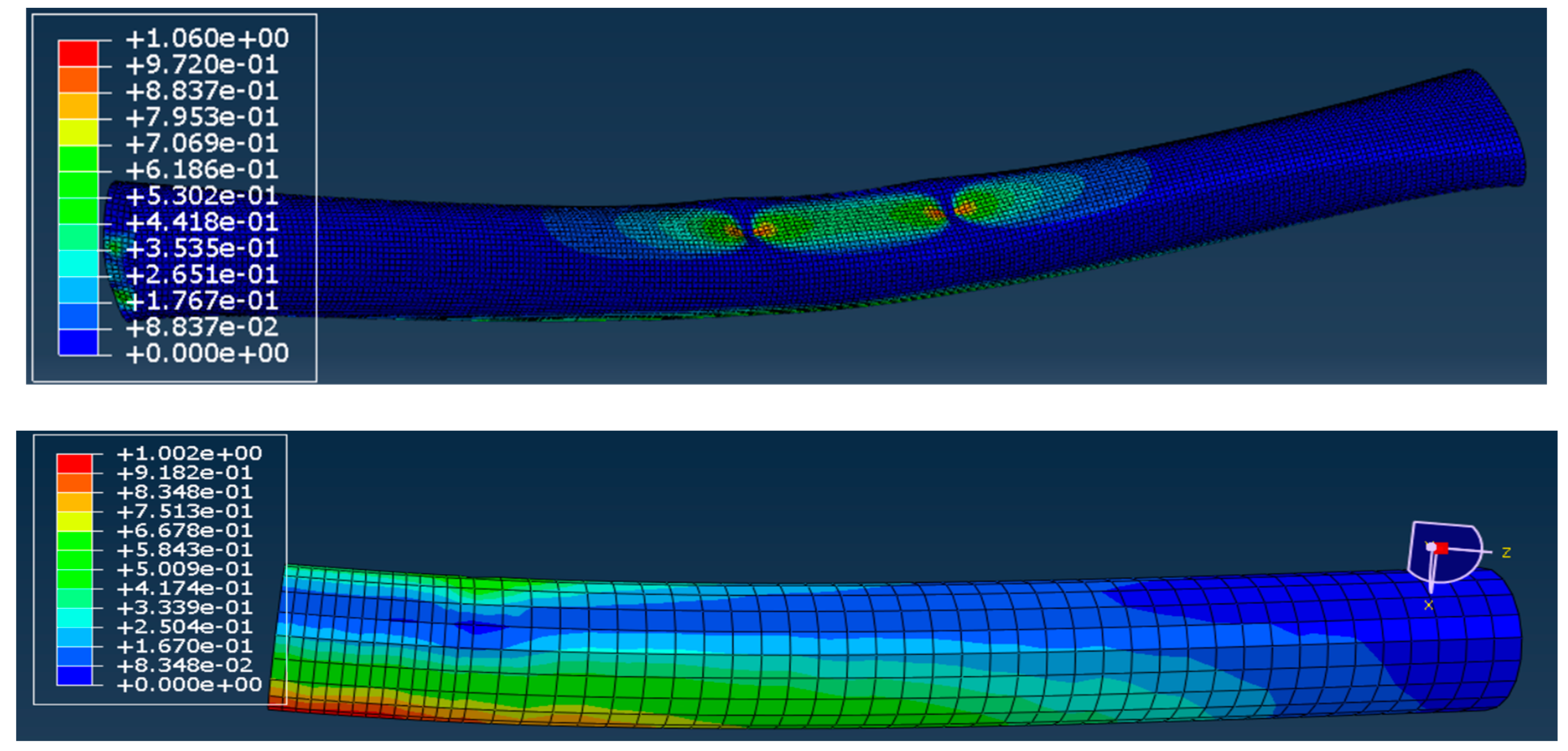
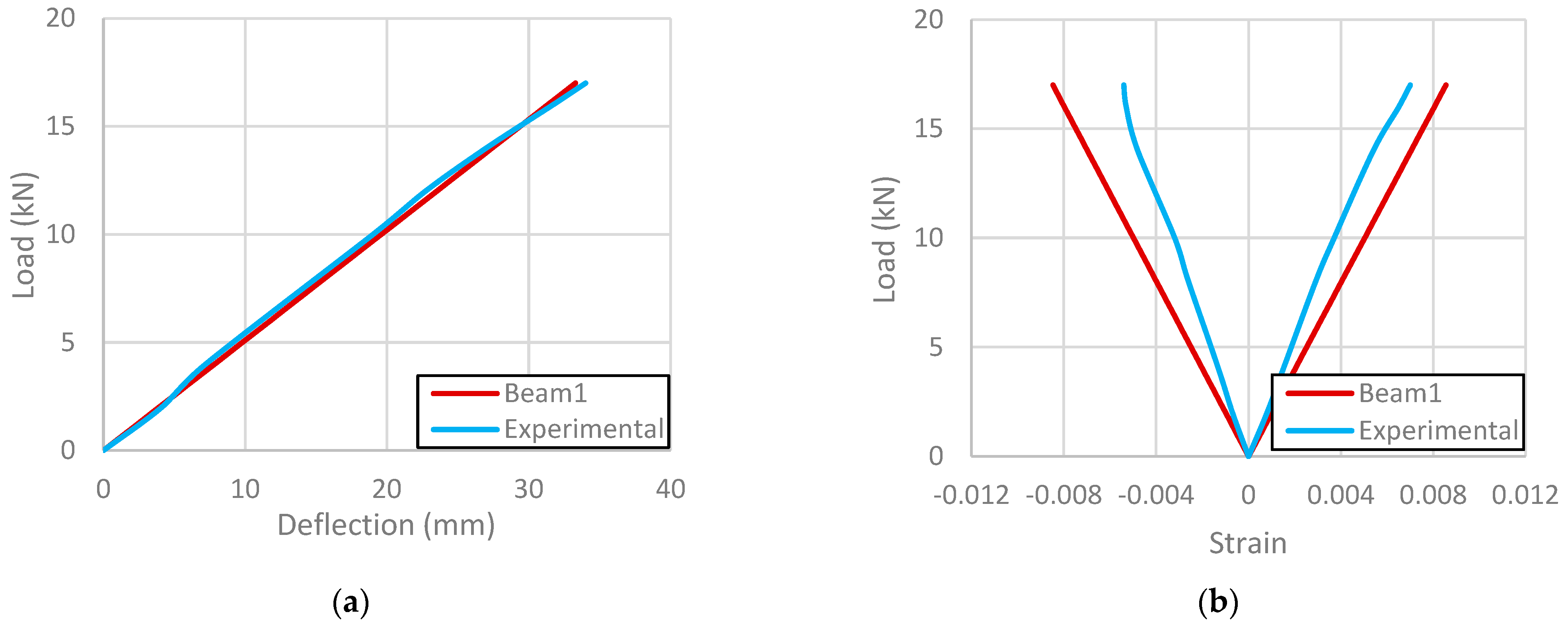
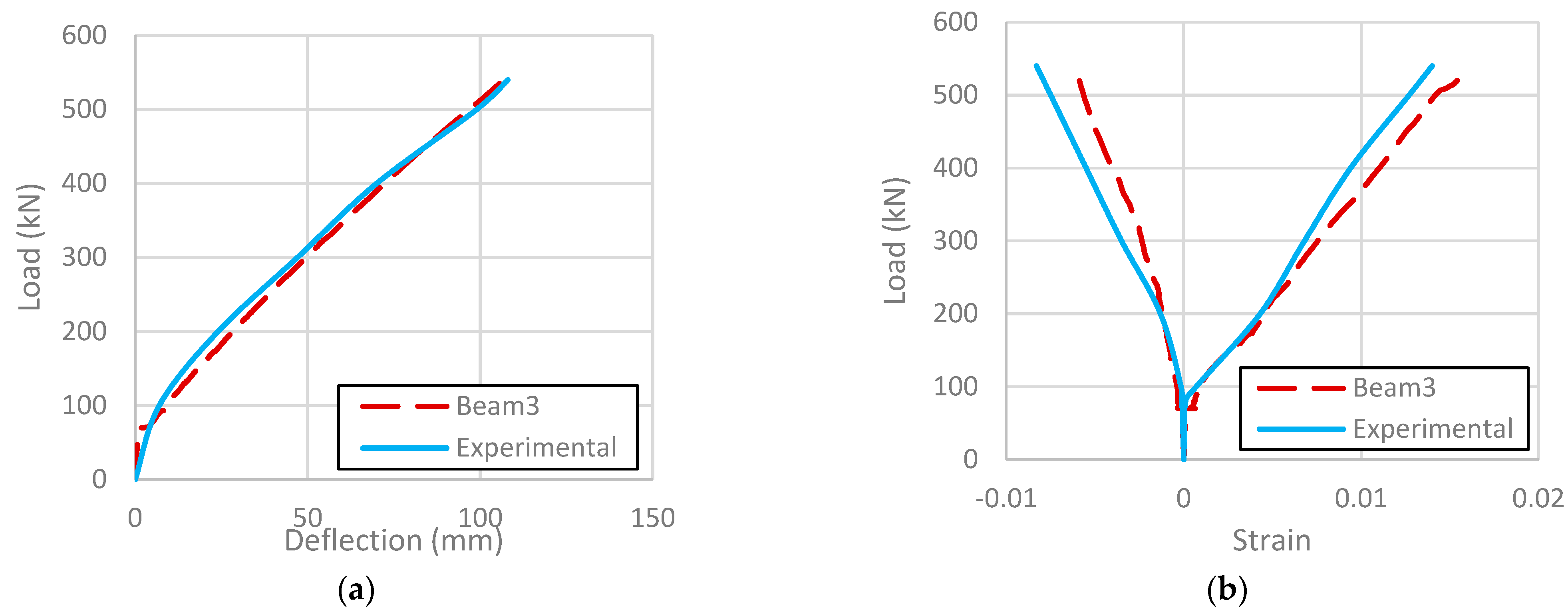


| Beam Number | Beam Type | Span (m) | Distance between Loading Points (m) | FRP Tube Thickness (mm) | FRP Ply Configuration * | FRP Elastic Modulus in Axial/Hoop Direction (GPa) | FRP Tensile Strength in Axial/Hoop Direction (MPa) | (MPa) |
|---|---|---|---|---|---|---|---|---|
| 1 | Hollow | 1.3 | 0.2 | 3.08 | A | 31/23 | 480/398 | N/A |
| 2 | CFFT | 1.3 | 0.2 | 3.08 | A | 31/23 | 480/398 | 37 |
| 3 | CFFT | 5.0 | 1.5 | 5.41 | B | 16.6/17.7 | 250/353 | 33 |
| Stub Number | Outer/Inner Diameter (mm) | Height (mm) | FRP Tube Thickness (mm) | FRP Ply Configuration * | FRP Elastic Modulus in Axial/Hoop Direction (GPa) | FRP Tensile Strength in Axial/Hoop Direction (MPa) | (MPa) |
|---|---|---|---|---|---|---|---|
| 1 | 168/95 | 336 | 2.56 | C | 17.4/27.7 | 348/547 | 58 |
| 2 | 100/0 | 200 | 3.08 | A | 31/23 | 480/398 | 37 |
| E1 (GPa) | E2 (GPa) | G12 (GPa) | ν12 | σ1tf (MPa) | σ1cf (MPa) | σ2tf (MPa) | σ2tf (MPa) | σ12f (MPa) |
|---|---|---|---|---|---|---|---|---|
| 38 | 7.8 | 3.5 | 0.28 | 795 | −533 | 39 | −128 | 89 |
| Beam 1 | Beam 2 | Beam 3 | Stub 1 | Stub 2 | |
|---|---|---|---|---|---|
| Estimated load capacity (kN) | 17 (0% *) | 46.4 (−7%) | 535.1 (−1%) | 1101 (+10%) | 555 (−4%) |
| Actual load capacity (kN) | 17 | 50.0 | 540.0 | 1000 | 580 |
| Estimated ultimate displacement (mm) | 33.3 (−2%) | 64.9 (−7%) | 105.7 (−2%) | / | / |
| Actual ultimate displacement (mm) | 34.0 | 70.0 | 108.0 | / | / |
| Estimated displacement at 40% of ultimate (mm) | 13.2 (+2%) | 21.9 (−1%) | 31.1 (+20%) | / | / |
| Actual displacement at 40% of ultimate (mm) | 13.0 | 22.0 | 26.0 | / | / |
| Estimated tensile strain at failure (10−3) | / | 22.9 (−3%) | 15.9 (+14%) | 7.9 (−12%) | 8.2 (−13%) |
| Actual tensile strain at failure (10−3) | / | 23.5 | 14.0 | 9.0 | 9.4 |
| Estimated compressive strain at failure (10−3) | / | −14.7 (−8%) | −6.1 (−27%) | −5.7 (+104%) | −6.8 (−13%) |
| Actual compressive strain at failure (10−3) | / | −16.0 | −8.3 | −2.8 | −7.8 |
| Metric Bar Size | 30 M | 45 M |
|---|---|---|
| Nominal diameter (mm) | 29.9 | 43.7 |
| Cross-sectional area (mm2) | 700 | 1500 |
| Yield stress fy (MPa) | 400 | 400 |
| Yield strain εy | 0.002 | 0.002 |
| Young’s modulus (MPa) | 200,000 | 200,000 |
| Poisson’s ratio | 0.3 | 0.3 |
| Tendon Type | Seven-Wire Strand |
|---|---|
| Ultimate stress fpu (MPa) | 1860 |
| Size designation | 15 |
| Nominal diameter (mm) | 15.24 |
| Nominal area (mm2) | 140 |
| Expansion coefficient (MPa/°C) | 1.0 × 10−5 |
| Young’s modulus (MPa) | 200,000 |
| Poisson’s ratio | 0.3 |
| D1 | Changes | |
|---|---|---|
| Height (mm) | 10,000 | / |
| Base diameter (mm) | 1000 | / |
| Top diameter (mm) | 1000 | D5 (900), D6 (800) |
| Concrete fill (%) | 50 | D2 (100), D3 (75), D4 (25) |
| Taper (%) | 0 | D5 (1%), D6 (2%) |
| FRP type | FRP1 | D7 (FRP2), D8 (FRP3) |
| Steel reinforcement | 0 | D9 (1%), D10 (2%) |
| Prestressing | 0 | D11 (6 tendons), D12 (12 tendons) at 50% stress level |
| FRP Type | Layer | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | ||
| 1 | Thickness (mm) | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Angle (°) | 0 | 90 | 0 | 90 | 0 | 90 | 0 | 90 | 0 | 90 | |
| 2 | Thickness (mm) | 1 | 1 | 1.1 | 1 | 1 | 1.1 | 1 | 1 | 1.1 | 0.7 |
| Angle (°) | 0 | 0 | 90 | 0 | 0 | 90 | 0 | 0 | 90 | 0 | |
| 3 | Thickness (mm) | 1 | 1 | 1.1 | 1 | 1 | 1.1 | 1 | 1 | 1.1 | 0.7 |
| Angle (°) | 90 | 90 | 0 | 90 | 90 | 0 | 90 | 90 | 0 | 90 | |
| Model Number | L1 to L12 |
|---|---|
| Height (mm) | 10,000 |
| Base diameter (mm) | 1000 |
| Top diameter (mm) | 800 |
| Loading Condition | Horizontal Load Distribution | Load Eccentricity (mm) | Axial Load (kN) |
|---|---|---|---|
| L1 | Concentrated load | 0 | / |
| L2 | Uniform load | / | / |
| L3 | Triangular load | / | / |
| L4 | Concentrated load | 200 | / |
| L5 | Concentrated load | 400 | / |
| L6 | / | / | 100 |
| L7 | / | / | 200 |
| L8 | / | / | 300 |
| L9 | Concentrated load | 0 | 200 |
| L10 | Concentrated load | 200 | 200 |
| L11 | Concentrated load | 200 | 300 |
| L12 | Concentrated load | 400 | 200 |
| Model Number | L13 | L14 | L15 |
|---|---|---|---|
| Height (mm) | 25,000 | 20,000 | 20,000 |
| Base diameter (mm) | 1500 | 1500 | 1000 |
| Top diameter (mm) | 1000 | 1000 | 800 |
| Model Number | C1 | S1 |
|---|---|---|
| Tower type | Concrete | Steel |
| Height (mm) | 10,000 | 10,000 |
| Base diameter (mm) | 1000 | 1000 |
| Top diameter (mm) | 800 | 800 |
| Taper (%) | 2 | 2 |
| Number of tendons | 12 | / |
| Concrete strength (MPa) | 47 | / |
| Steel reinforcement ratio (%) | 2.3 | / |
| Concrete filling ratio (%) | 50 | / |
| Steel wall thickness (mm) | / | 10 |
| L6 | L7 | L8 | |
|---|---|---|---|
| Axial load (kN) | 100 | 200 | 300 |
| Axial deflection (mm) | 0.617 | 0.687 | 0.757 |
| Compressive stress at base (MPa) | 1.87 | 2.08 | 2.30 |
| L1 | L4 | L5 | ||
|---|---|---|---|---|
| At failure | Load (kN) | 477.5 | 475.7 | 475.6 |
| Compared to L1 | / | −0.38% | −0.40% | |
| Def. * (mm) | 632.2 | 628.5 | 630.3 | |
| Comparison | / | −0.59% | −0.30% | |
| Mom. * (kN·m) | 4775 | 4759 | 4759 | |
| Comparison | / | −1.2% | −1.2% | |
| At deflection limit | Def. (mm) | 125 | 125 | 125 |
| Load (kN) | 190.5 | 185.4 | 184.6 | |
| Comparison | / | −2.7% | −3.1% | |
| Mom. (kN·m) | 1905 | 1855 | 1847 | |
| Comparison | / | −1.5% | −1.9% | |
| At service load | Load (kN) | 34.1 | 34.1 | 34.1 |
| Def. (mm) | 9.3 | 9.6 | 9.7 | |
| Comparison | / | 3.2% | 4.3% |
| L9 | L10 | L11 | L12 | ||
|---|---|---|---|---|---|
| Axial load (kN) | 200 | 200 | 300 | 200 | |
| At failure | Load (kN) | 474.7 | 474.0 | 473.9 | 473.8 |
| Comparison | / | −0.15% | −0.17% | −0.19% | |
| Def. (mm) | 614.2 | 612.5 | 606.6 | 615.3 | |
| Comparison | / | −0.28% | −1.2% | 0.18% | |
| Mom. (kN·m) | 4747 | 4741 | 4740 | 4742 | |
| Comparison | / | −0.1% | −0.1% | −0.1% | |
| At def. limit | Def. (mm) | 125 | 125 | 125 | 125 |
| Load (kN) | 191.4 | 188.6 | 191.1 | 185.8 | |
| Comparison | / | −0.57% | 0.31% | −1.1% | |
| Mom.(kN·m) | 1914 | 1886 | 1911 | 1860 | |
| Comparison | / | −1.5% | 4.0% | −2.8% | |
| At service load | Load (kN) | 34.1 | 34.1 | 34.1 | 34.1 |
| Def. (mm) | 5.6 | 9.2 | 9.2 | 9.3 | |
| Comparison | / | 64.3% | 64.3% | 66.1% |
| Model Number | (m3) | (kN) | (kN) | (kN) | (kN) |
|---|---|---|---|---|---|
| L9–L12 | 9 | 6.5 | 25.4 | 2.2 | 34.1 |
| L13 | L14 | L15 | ||
|---|---|---|---|---|
| h/D ratio | 16.7 | 13.3 | 20 | |
| At failure | Load (kN) | 263.2 | 384.9 | 165.4 |
| Comparison | −44.6% | −18.9% | −65.2% | |
| Def. (mm) | 1764.8 | 1282.4 | 1792.9 | |
| Comparison | 187.3% | 108.8% | 191.9% | |
| Mom. (kN·m) | 6580 | 7700 | 3310 | |
| Comparison | 37.8% | 61.3% | −30.7% | |
| At def. limit | Def. (mm) | 312.5 | 250 | 250 |
| Load (kN) | 59.3 | 146.3 | 46.3 | |
| Comparison | −69.3% | −24.2% | −76.0% | |
| Mom. (kN·m) | 1483 | 2926 | 927 | |
| Comparison | −22.2% | 53.6% | −51.3% | |
| At service load | Load (kN) | 96.8 | 79.2 | 59.4 |
| Def. (mm) | 500.1 | 89.6 | 362.9 | |
| Comparison | 5277% | 863% | 3802% |
| L1 | C1 | S1 | |
|---|---|---|---|
| (kN) | 477.5 | 226.2 | 417 |
| (mm) | 632.2 | 246.9 | 1169.0 |
| Buckling (kN) | / | / | 223 |
| Failure mode | FRP rupture | Flexural | Buckling |
| Def. limit (mm) | 125 | 125 | 125 |
| (kN) | 190.5 | 172.9 | 225 |
| Service load (kN) | 34.1 | 34.1 | 34.1 |
| (mm) | 9.3 | 9.83 | 18.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gong, Y.; Noël, M. Finite Element Model of Concrete-Filled, Fiber-Reinforced Polymer Tubes for Small-Scale Wind Turbine Towers. CivilEng 2024, 5, 169-190. https://doi.org/10.3390/civileng5010009
Gong Y, Noël M. Finite Element Model of Concrete-Filled, Fiber-Reinforced Polymer Tubes for Small-Scale Wind Turbine Towers. CivilEng. 2024; 5(1):169-190. https://doi.org/10.3390/civileng5010009
Chicago/Turabian StyleGong, Yikai, and Martin Noël. 2024. "Finite Element Model of Concrete-Filled, Fiber-Reinforced Polymer Tubes for Small-Scale Wind Turbine Towers" CivilEng 5, no. 1: 169-190. https://doi.org/10.3390/civileng5010009
APA StyleGong, Y., & Noël, M. (2024). Finite Element Model of Concrete-Filled, Fiber-Reinforced Polymer Tubes for Small-Scale Wind Turbine Towers. CivilEng, 5(1), 169-190. https://doi.org/10.3390/civileng5010009







