A Review of Seven Tunnel Face Stability Models
Abstract
:1. Introduction
2. Overview of Recent Advances in Tunnel Face Stability Analysis
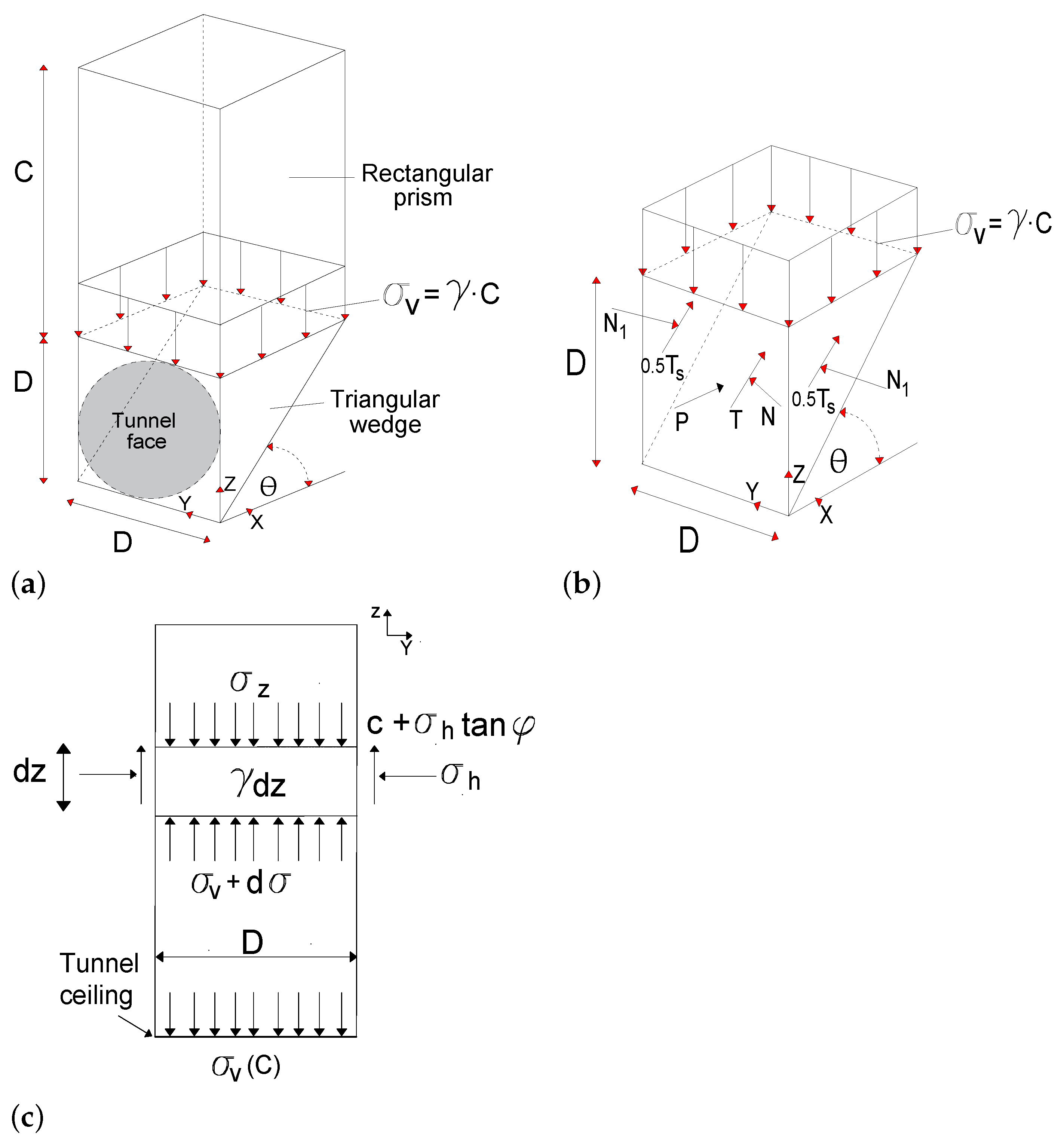
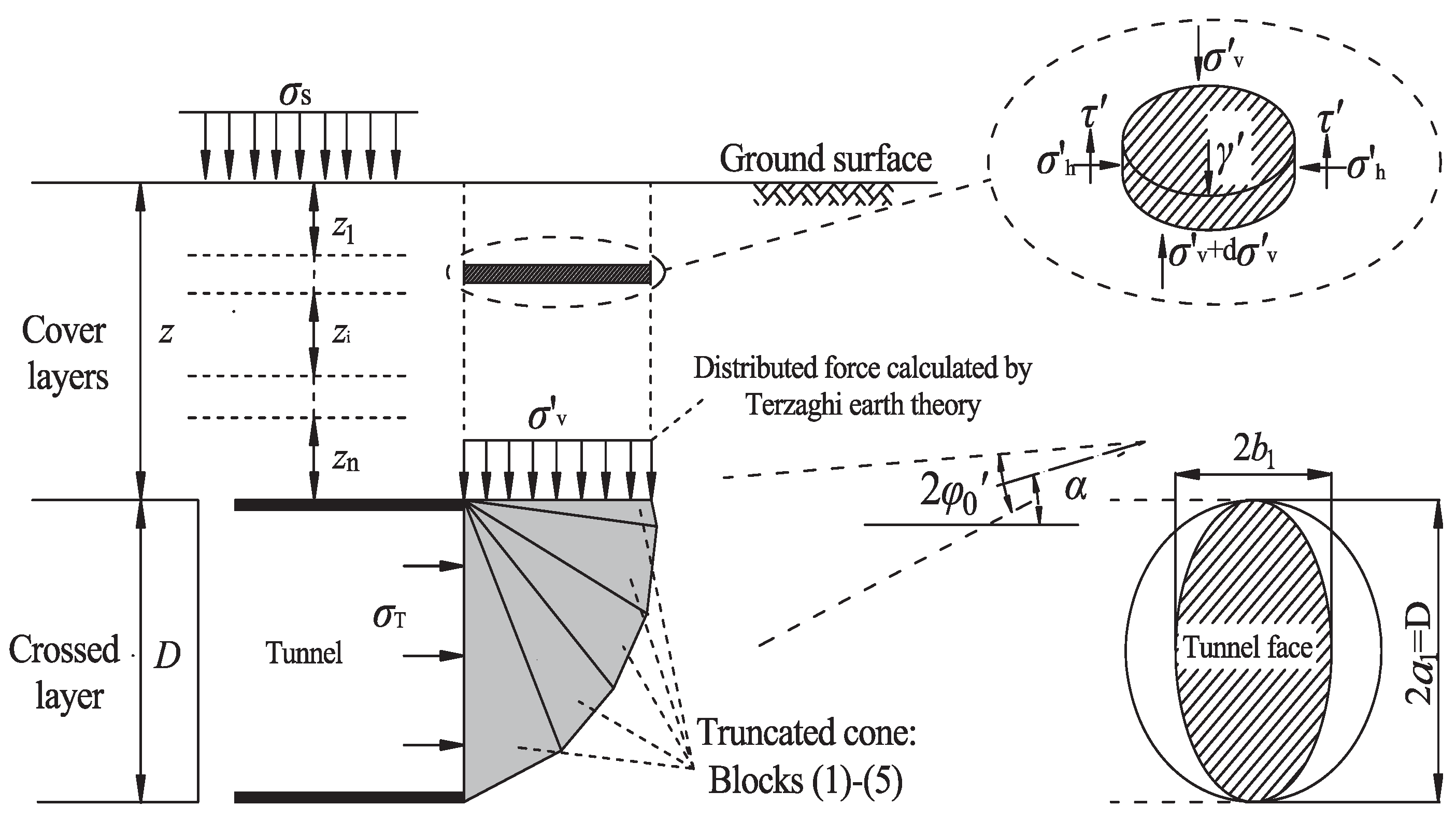
Limit Equilibrium Method (LEM)
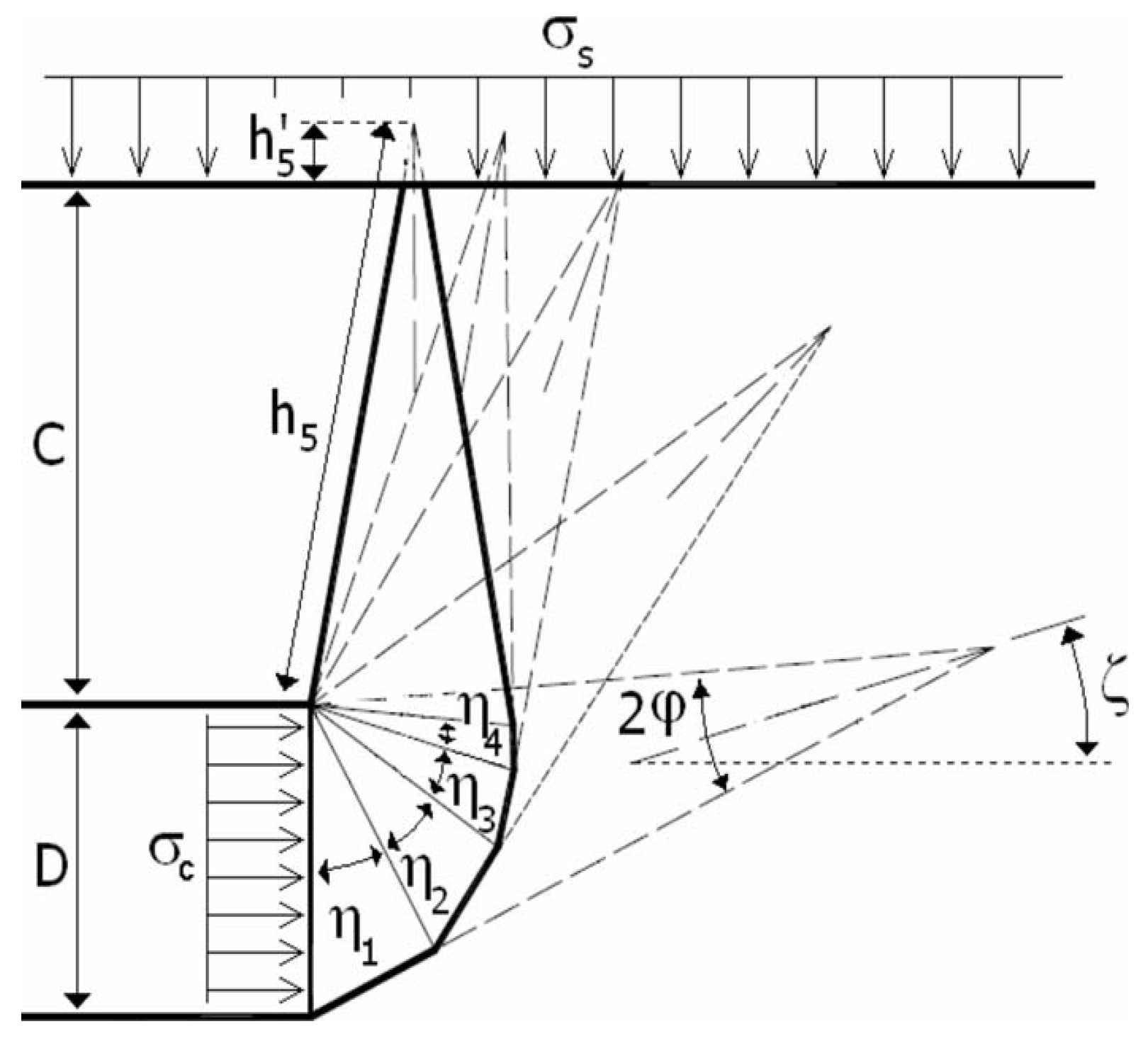
3. Limit Analysis Method (LAM)
4. Comparative Calculations Concerning Minimum Support Pressure
4.1. Homogeneous Soil
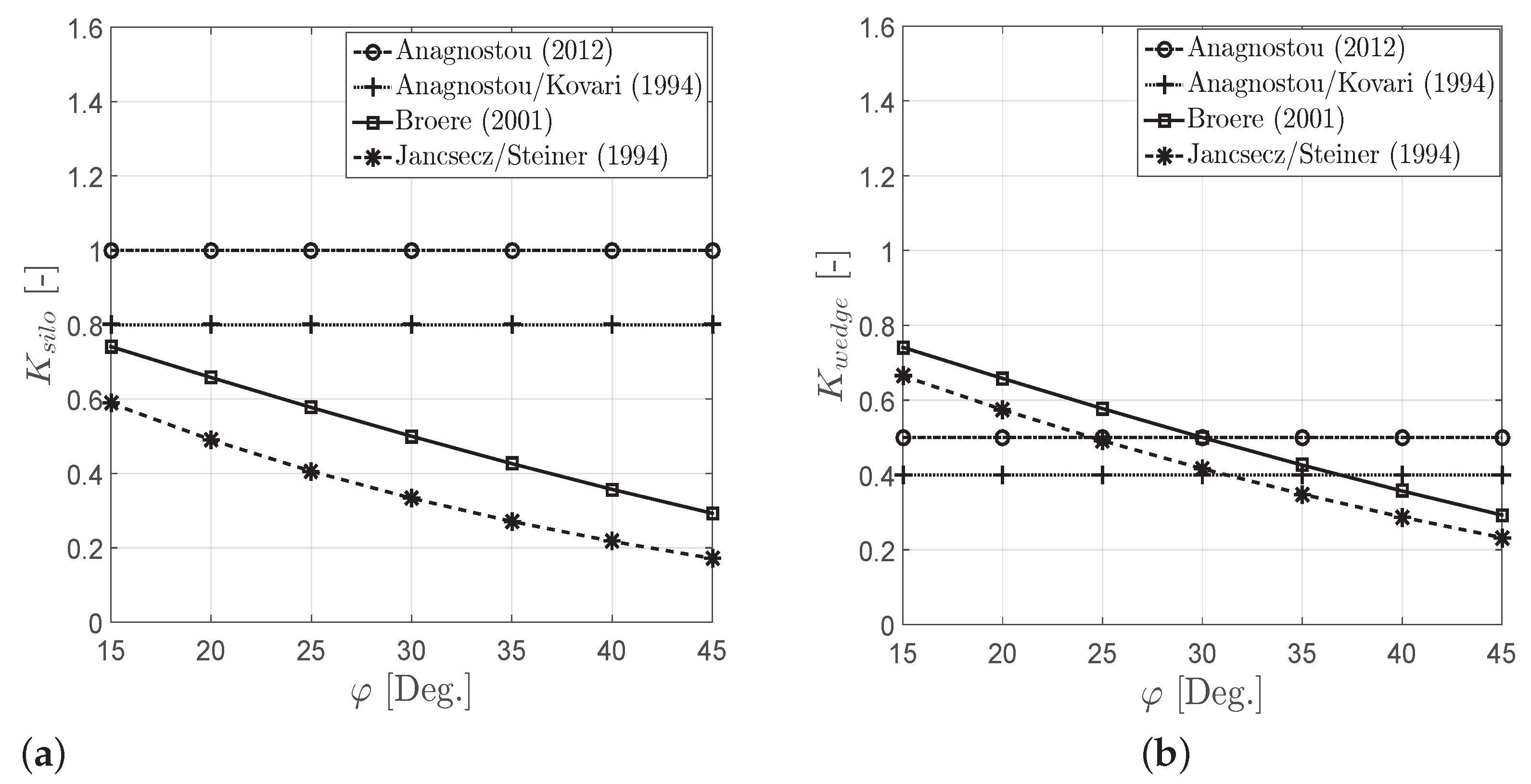
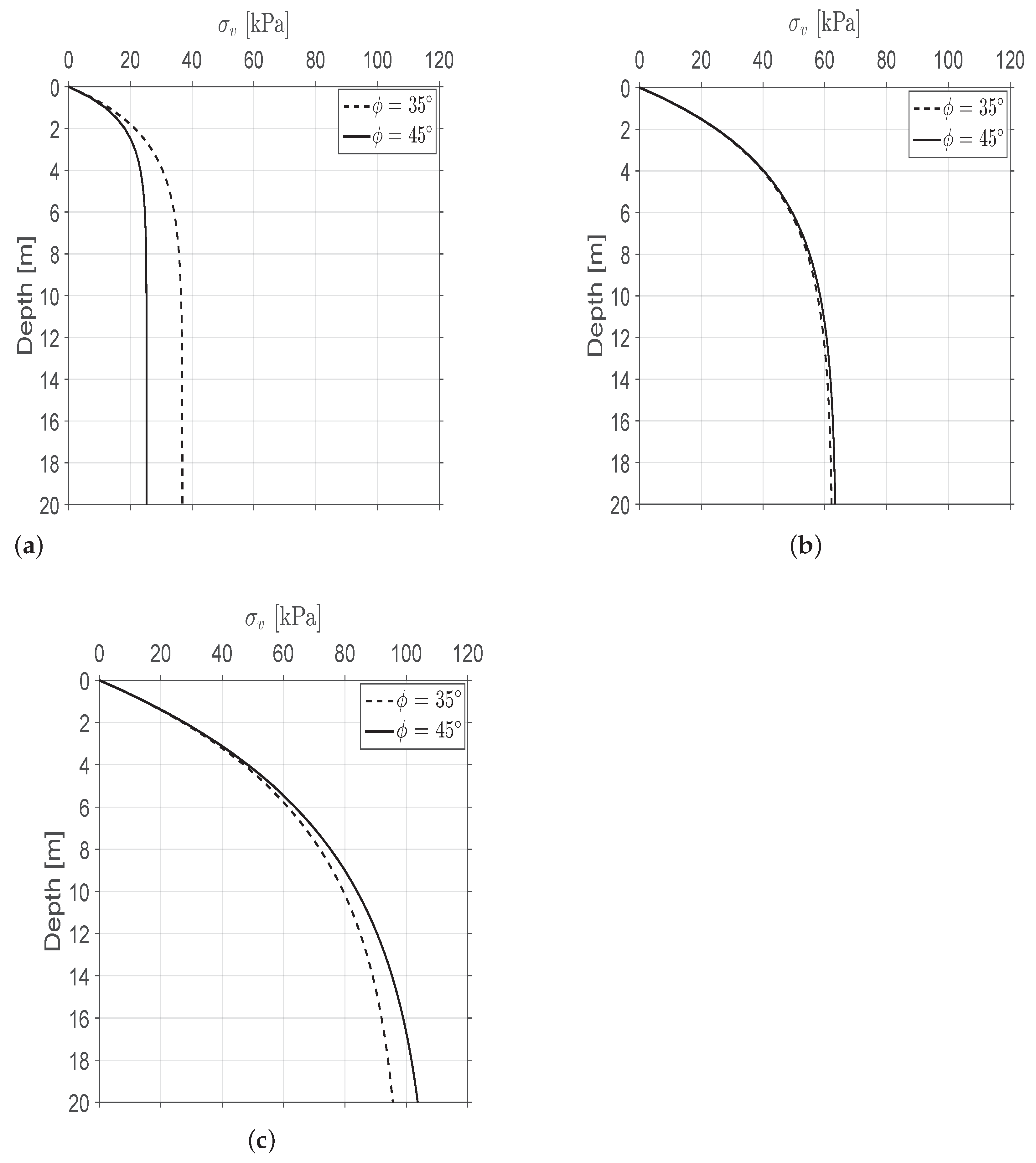
4.1.1. Influence of the Friction Angle and Cohesion
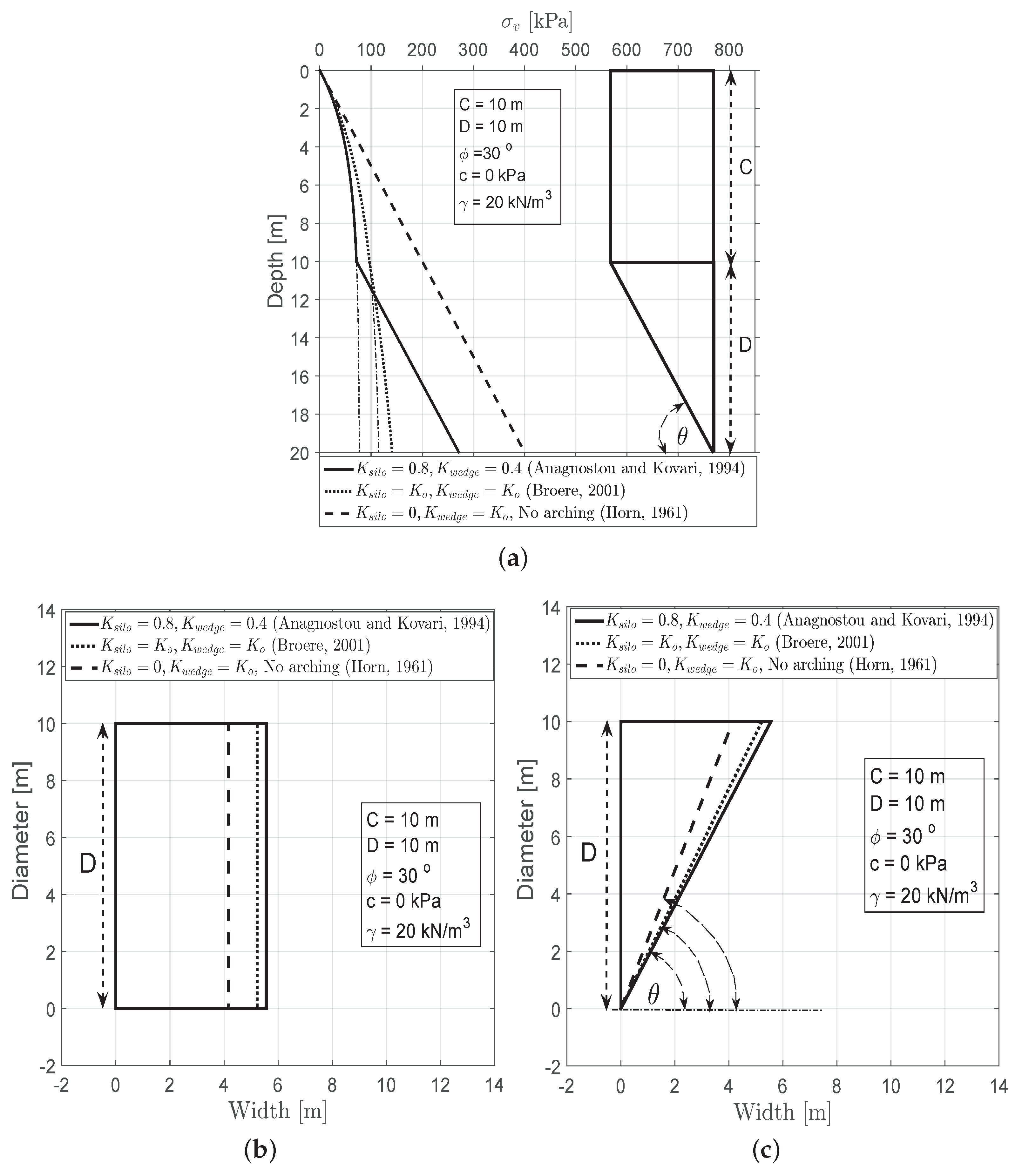
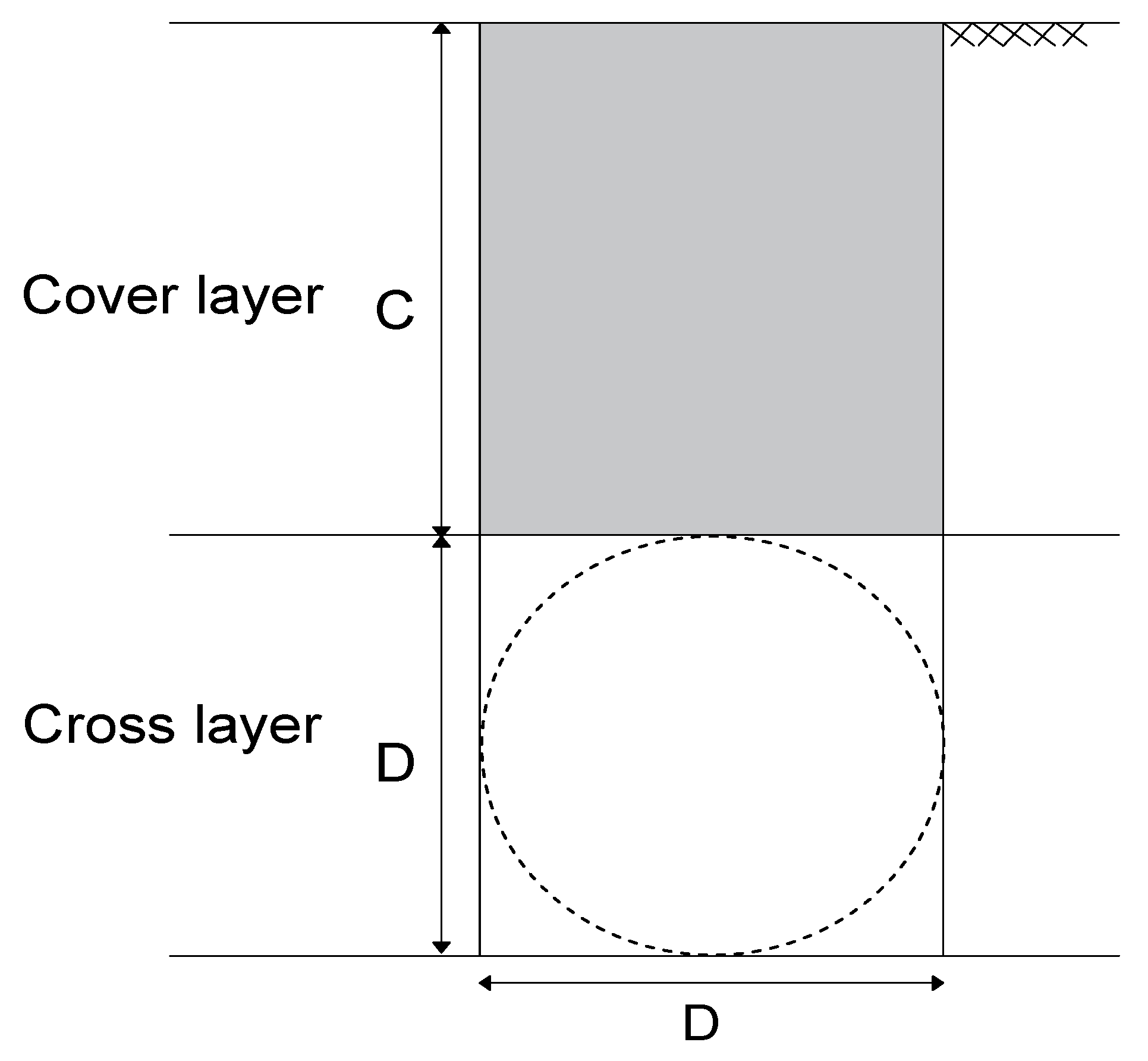
4.1.2. Influence of C/D
4.1.3. Verification by Physical Model Tests
4.2. Layered Soil
5. Conclusions
- 1.
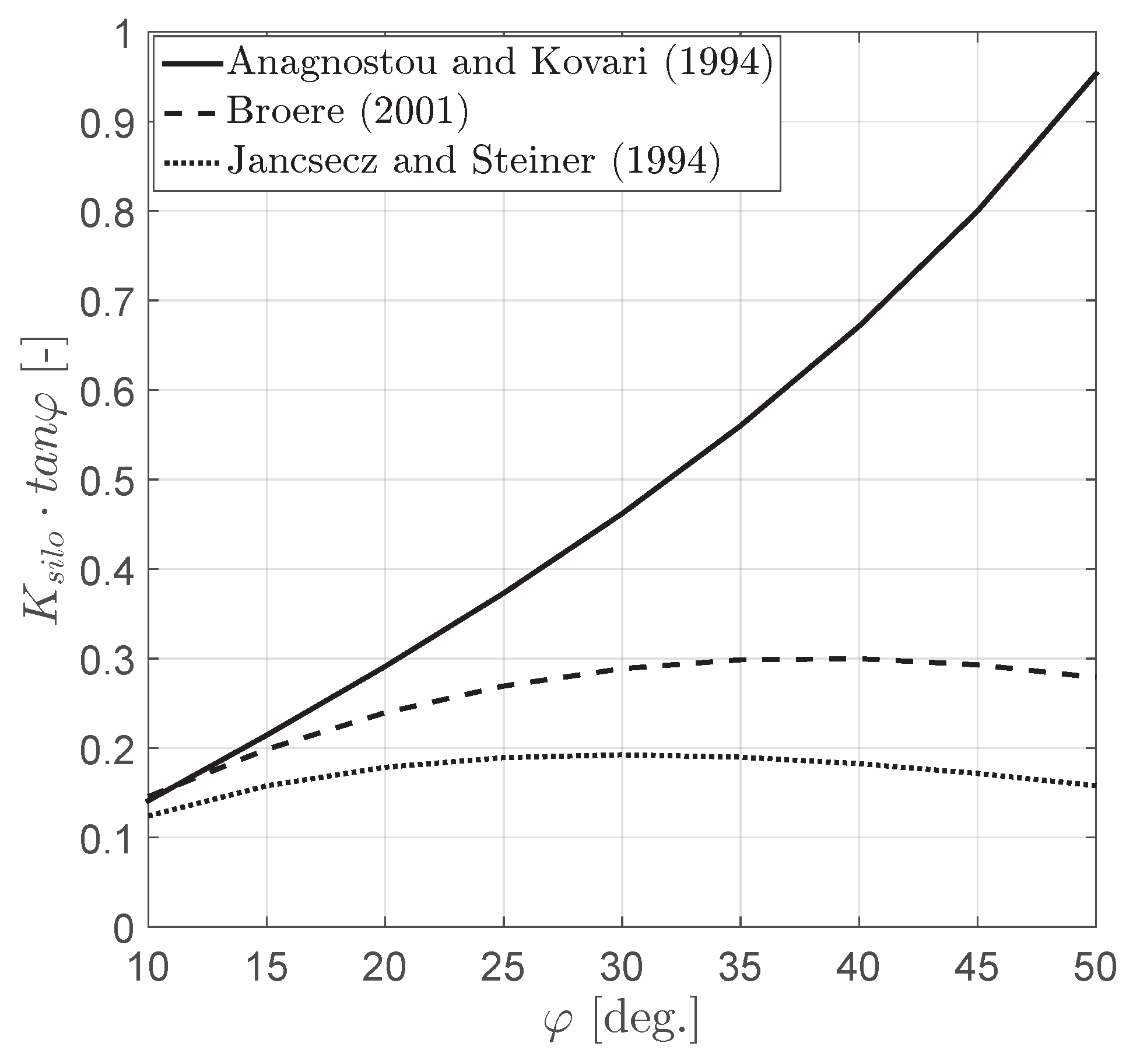
- To use Janssen’s solution in the wedge-silo model, it is required to have explicit value for the lateral earth pressure coefficient of the silo. However, the advised values of it vary in a wide range. Due to this, the face support pressures calculated by the existing wedge-silo models are quite different. So, the question about the proper value for the lateral earth pressure remains unanswered.
- 2.
- According to the results of comparative calculations, the wedge-silo models give higher support pressure than conical block models, but the differences in the two methods for the prediction of the support pressure are small for higher friction angles.
- 3.
- Comparing the results of physical model tests to the results of Anagnostou and Kovari [7] and Broere [8] models, indicating that both models are relatively conservative models for estimating the minimum support pressure. The results obtained from Vermeer et al. [21], Mollon et al. [14], and Anagnostou [11] models show a good agreement with those of the experiments. However, comparing to the experiments results, the upper bound solutions proposed by Leca and Dormieux [13] underestimate the minimum support pressure especially when soil is cohesionless.
- 4.
- The solution of the limit equilibrium method revealed that the cover to diameter ratio has significant influence on the minimum support pressure. This effect is also deduced by Chen et al. [4] and Chambon and Corte [3], whereas the solution of the upper bound theorem for the minimum support pressure is only independent, when the failure mechanism of the upper bound theorem does not intersect at the ground surface in a certain soil condition (e.g., C/D 1), this outcome has also been reported by Vermeer et al. [21].
- 5.
- In the case of a ground composed of two soil layers, the minimum support pressure obtained from wedge-silo models is higher than that predicted by the upper bound solution. In addition, the discrepancies in the results between the support pressure predicted by the different models are obvious.
- 6.
- Finally, the analytical approaches such as the Limit Equilibrium Method (LEM) and Limiting Analysis Method (LAM) are used to assess the stability of the tunnel face assuming various failure mechanisms. However, the results are quite different. Therefore, there are still considerable potential efforts for calculating the support pressure more accurately.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Terzaghi, K. Theoretical Soil Geomechanics; Wiley and Sons: New York, NY, USA, 1943. [Google Scholar]
- Terzaghi, K. Stress distribution in dry and saturated sand above a yielding trap-door. In Proceedings of the First International Conference on Soil Mechanics and Foundation Engineering, Cambridge, MA, USA, 1 August 1936; pp. 307–311. [Google Scholar]
- Chambon, P.; Corte, J. Shallow Tunnels in Cohesionless Soil: Stability of Tunnel Face. J. Geotech. Eng. 1994, 120, 1148–1165. [Google Scholar] [CrossRef]
- Chen, R.P.; Tang, L.J.; Ling, D.S.; Chen, Y.M. Face stability analysis of shallow shield tunnels in dry sandy ground using the discrete element method. Comput. Geotech. 2011, 38, 187–195. [Google Scholar] [CrossRef]
- Horn, N. Horizontaler Erddruck auf senkrechte Abschlussflächen von Tunnelröhren. In Proceedings of the National Conference of the Hungarian Civil Engineering Industry, Budapest, Hungary, 18–21 June 1961; pp. 7–16. [Google Scholar]
- Jancsecz, S.; Steiner, W. Face support for a large mix-shield in heterogeneous ground conditions. In Proceedings of the 7th International Symposium Tunnelling, London, UK, 12 June 1994; pp. 189–195. [Google Scholar]
- Anagnostou, G.; Kovari, K. The face stability of slurry-shield-driven tunnels. Tunn. Undergr. Space Technol. 1994, 9, 165–174. [Google Scholar] [CrossRef]
- Broere, W. Tunnel Face Stability and New CPT Application. Ph.D. Thesis, Delft University, Delft, The Netherlands, 2001. [Google Scholar]
- Kirsch, A.; Kolymbas, D. Theoretische Untersuchung zur Ortsbruststabilität. Bautechnik 2005, 82, 449–456. [Google Scholar] [CrossRef]
- Chen, R.P.; Tang, L.J.; Yin, X.S.; Chen, Y.M.; Bian, X.C. An improved 3D wedge prism model for the face stability analysis of the shield tunnel in cohesionless soils. Acta Geotech. 2015, 10, 683–692. [Google Scholar] [CrossRef]
- Anagnostou, G. The contribution of horizontal arching to tunnel face stability. Geotechnik 2012, 35, 34–44. [Google Scholar] [CrossRef]
- Chen, W.F. Limit Analysis and Soil Plasticity; Elsevier Scientific Publishing Company: London, UK, 1975. [Google Scholar]
- Leca, E.; Dormieux, L. Upper and lower bound solutions for the face stability of shallow circular tunnels in frictional material. Geotechnik 1990, 40, 581–606. [Google Scholar] [CrossRef] [Green Version]
- Mollon, G.; Dias, D.; Soubra, A.H. Face stability analysis of circular tunnels driven by a pressurized shield. J. Geotech. Geoenviron. Eng. 2010, 136, 215–229. [Google Scholar] [CrossRef] [Green Version]
- Tang, W.; Liu, W.; Albers, B.; Savidis, S. Upper bound analysis of tunnel face stability in layered soils. Acta Geotech. 2014, 9, 661–671. [Google Scholar] [CrossRef]
- Ibrahim, E.; Soubra, A.H.; Mollon, G.; Raphael, W.; Dias, D.; Reda, A. Three-dimensional face stability analysis of pressurized tunnels driven in a multilayered purely frictional medium. Tunn. Undergr. Space Technol. 2015, 49, 18–34. [Google Scholar] [CrossRef]
- Zou, J.; Chen, G.; Qian, Z.T. Tunnel face stability in cohesion-frictional soils considering the soil arching effect by improved failure models. Comput. Geotech. 2019, 25, 526–542. [Google Scholar] [CrossRef]
- Li, W.; Zhang, C. Face Stability Analysis for a Shield Tunnel in Anisotropic Sands. Int. J. Geomech. 2020, 20, 04020043. [Google Scholar] [CrossRef]
- Peila, D. A theoretical study of reinforcement influence on the stability of a tunnel face. Geotech. Geol. Eng. 1994, 12, 145–168. [Google Scholar] [CrossRef]
- Ohta, T.; Kiya, H. Experimental Study and Numerical Analysis on Stability of Tunnel Face in Sandy Ground; ISRM International Symposium: Tokye, Japan, 2001. [Google Scholar]
- Vermeer, P.A.; Ruse, N.M.; Marcher, T. Tunnel heading stability in drained ground. Felsbau 2002, 20, 8–18. [Google Scholar]
- Kirsch, A. On the Face Stability of Shallow Tunnels in Sand; Logos Verlag Berlin GmbH: Berlin, Germany, 2009. [Google Scholar]
- Qarmout, M.; König, D.; Gussmann, P.; Thewes, M.; Schanz, T. Tunnel face stability analysis using Kinematical Element Method. Tunn. Undergr. Space Technol. 2019, 85, 354–367. [Google Scholar] [CrossRef]
- Qarmout, M.; Schmüdderich, C.; König, D.; Thewes, M.; Wichtmann, T. Face stability of a circular tunnel excavated in dry frictional- cohesive soil. In Proceedings of the ETS Tunnelling and Underground Construction Conference & Exhibition, Luxor, Egypt, 3–5 December 2019. [Google Scholar]
- Takano, D.; Otani, J.; Nagatani, H.; Mukunoki, T. Application of X-ray CT Boundary Value Problems in Geotechnical Engineering-Research on Tunnel Face Failure; ASCE: Reston, VA, USA, 2006; pp. 1–6. [Google Scholar]
- Idinger, G.; Aklik, P.; Wu, W.; Borja, R. Centrifuge model test on the face stability of shallow tunnel. Acta Geotech. 2011, 6, 105–117. [Google Scholar] [CrossRef] [Green Version]
- Lü, X.; Zhou, Y.; Huang, M.; Zeng, S. Experimental study of the face stability of shield tunnel in sands under seepage condition. Tunn. Undergr. Space Technol. 2018, 74, 195–205. [Google Scholar] [CrossRef]
- Janssen, H. Versuche uber Getreidedruck in Silozellen. Z. Des Vereines Dtsch. Ingenieure 1895, 39, 1045–1049. [Google Scholar]
- Jaky, J. The coefficient of earth pressure at rest. J. Soc. Hung. Archit. Eng. 1944, 78, 355–358. [Google Scholar]
- Walz, B.; Prager, J. Der Nachweis der äusseren Standsicherheit suspensionsgestützter Erdwände nach der Elementscheibentheorie. In VerÖffentlichungen des Grundbauinstitutes der Technischen Universität Berlin; Grundbauinst Technology University: Berlin, Germany, 1978. [Google Scholar]
- Krause, T. Schildvortrieb Mit flüssigkeits und Erdgestützter Ortsbrust. Ph.D. Thesis, Delft University, Delft, The Netherlands, 1987. [Google Scholar]
- Perazzelli, P.; Leone, T.; Anagnostou, G. Tunnel face stability under seepage flow conditions. Tunn. Undergr. Space Technol. 2014, 43, 459–469. [Google Scholar] [CrossRef]
- Zingg, S.; Anagnostou, G. An investigation into efficient drainage layouts for the stabilization of tunnel faces in homogeneous ground. Tunn. Undergr. Space Technol. 2016, 58, 49–73. [Google Scholar] [CrossRef]
- Anagnostou, G.; Perazzelli, P. Analysis method and design charts for bolt reinforcement of the tunnel face in cohesive-frictional soils. Tunn. Undergr. Space Technol. 2015, 74, 162–181. [Google Scholar] [CrossRef]
- Anagnostou, G.; Serafeimidis, K. The dimensioning of tunnel face reinforcement. In Proceedings of the ITA-AITES World Tunnel Congress, Prague, Czech Republic, 5–10 May 2007; Volume 1, pp. 291–296. [Google Scholar]
- Dias, T.; Bezuijen, A. A Different View on TBM Face Equilibrium in Permeable Ground; ITA World Tunnel Congress-Uniting an Industry: San Francisco, CA, USA, 2016. [Google Scholar]
- Broere, W. Influence of Infiltration and Groundwater Flow on Tunnel Face Stability; Geotechnical Aspects of Underground Construction in Soft Ground: Tokyo, Japan, 2000; pp. 339–344. [Google Scholar]
- Drucker, D.; Greenberg, W.; Prager, W. The safety factor of an elastic plastic body in plane strain. Trans. ASME J. Appl. Mech. 1951, 73, 371–378. [Google Scholar] [CrossRef]
- Zhang, C.P.; Han, K.H.; Zhang, D.L. Face stability analysis of shallow circular tunnels in cohesive—Frictional soils. Tunn. Undergr. Space Technol. 2015, 50, 345–357. [Google Scholar] [CrossRef]
- Li, W.; Zhang, C.; Zhang, D.; Ye, Z.; Tan, Z. Face stability of shield tunnels considering a kinematically admissible velocity field of soil arching. J. Rock Mech. Geotech. Eng. 2021. [Google Scholar] [CrossRef]
- Senent, S.; Jimenez, R. A tunnel face failure mechanism for layered ground, considering the possibility of partial collapse. Tunn. Undergr. Space Technol. 2015, 47, 182–192. [Google Scholar] [CrossRef]
- Khezri, N.; Mohamad, H.; Hassani, M.; Fatahi, B. The stability of shallow circular tunnels in soil considering variations in cohesion with depth. Tunn. Undergr. Space Technol. 2015, 49, 230–240. [Google Scholar] [CrossRef]
- Pan, Q.J.; Dias, D. Face Stability Analysis for a Shield-Driven Tunnel in Anisotropic and Nonhomogeneous Soils by the Kinematical Approach. Int. J. Geomech. 2016, 16. [Google Scholar] [CrossRef]
- Han, K.; Zhang, C.; Zhang, D. Upper-bound solutions for the face stability of a shield tunnel in multilayered cohesive-frictional soils. Comput. Geotech. 2016, 79, 1–9. [Google Scholar] [CrossRef]
- Lee, M.; Nam, W. Effect of tunnel advance rate on seepage forces acting on the underwater tunnel face. Tunn. Undergr. Space Technol. 2004, 19, 273–281. [Google Scholar] [CrossRef]
- Pan, Q.J.; Dias, D. The effect of pore water pressure on tunnel face stability. Int. J. Numer. Anal. Meth. Geomech. 2016, 40, 2123–2136. [Google Scholar] [CrossRef]
- Chen, R.P.; Li, J.; Kong, L.G.; Tang, L.J. Experimental study on face instability of shield tunnel in sand. Tunn. Undergr. Space Technol. 2013, 33, 12–21. [Google Scholar] [CrossRef]





| Author | Model | Tested Material | c [kPa] | C/D [−] | |
|---|---|---|---|---|---|
| Chambon and Corte [3] | ng test | Fontainebleau sand | 38–42 | 0–5 | 0.5, 1, 2, 4 |
| Kirsch [22] | 1g test | Ottendorf-Okrilla sand | 32.5 | 0 | 0.5, 0.75, 1, 1.5, 2 |
| Chen et al. [47] | 1g test | Yangtze River sand | 37 | 0–0.5 | 0.5, 1, 2 |
| Model Description | Parameter | Value | Unit |
|---|---|---|---|
| Cover layer | Cover depth (C) | 9 | [m] |
| Friction angle () | 15–45 | [Deg.] | |
| Unit weight() | 18 | [kN/m] | |
| Cohesion (c) | 2.5 | [kPa] | |
| Cross layer | Tunnel diameter (D) | 6 | [m] |
| Friction angle () | 20° | [Deg.] | |
| Unit weight() | 18 | [kN/m] | |
| Cohesion (c) | 2.5 | [kPa] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qarmout, M.; König, D.; Wichtmann, T. A Review of Seven Tunnel Face Stability Models. CivilEng 2022, 3, 116-134. https://doi.org/10.3390/civileng3010008
Qarmout M, König D, Wichtmann T. A Review of Seven Tunnel Face Stability Models. CivilEng. 2022; 3(1):116-134. https://doi.org/10.3390/civileng3010008
Chicago/Turabian StyleQarmout, Mahmoud, Diethard König, and Torsten Wichtmann. 2022. "A Review of Seven Tunnel Face Stability Models" CivilEng 3, no. 1: 116-134. https://doi.org/10.3390/civileng3010008
APA StyleQarmout, M., König, D., & Wichtmann, T. (2022). A Review of Seven Tunnel Face Stability Models. CivilEng, 3(1), 116-134. https://doi.org/10.3390/civileng3010008






