Analytical Investigation of Tension Loaded Deformed Rebar Anchors in Concrete
Abstract
:1. Introduction
Current Code Provisions
2. Development of Benchmarked Analytical Models
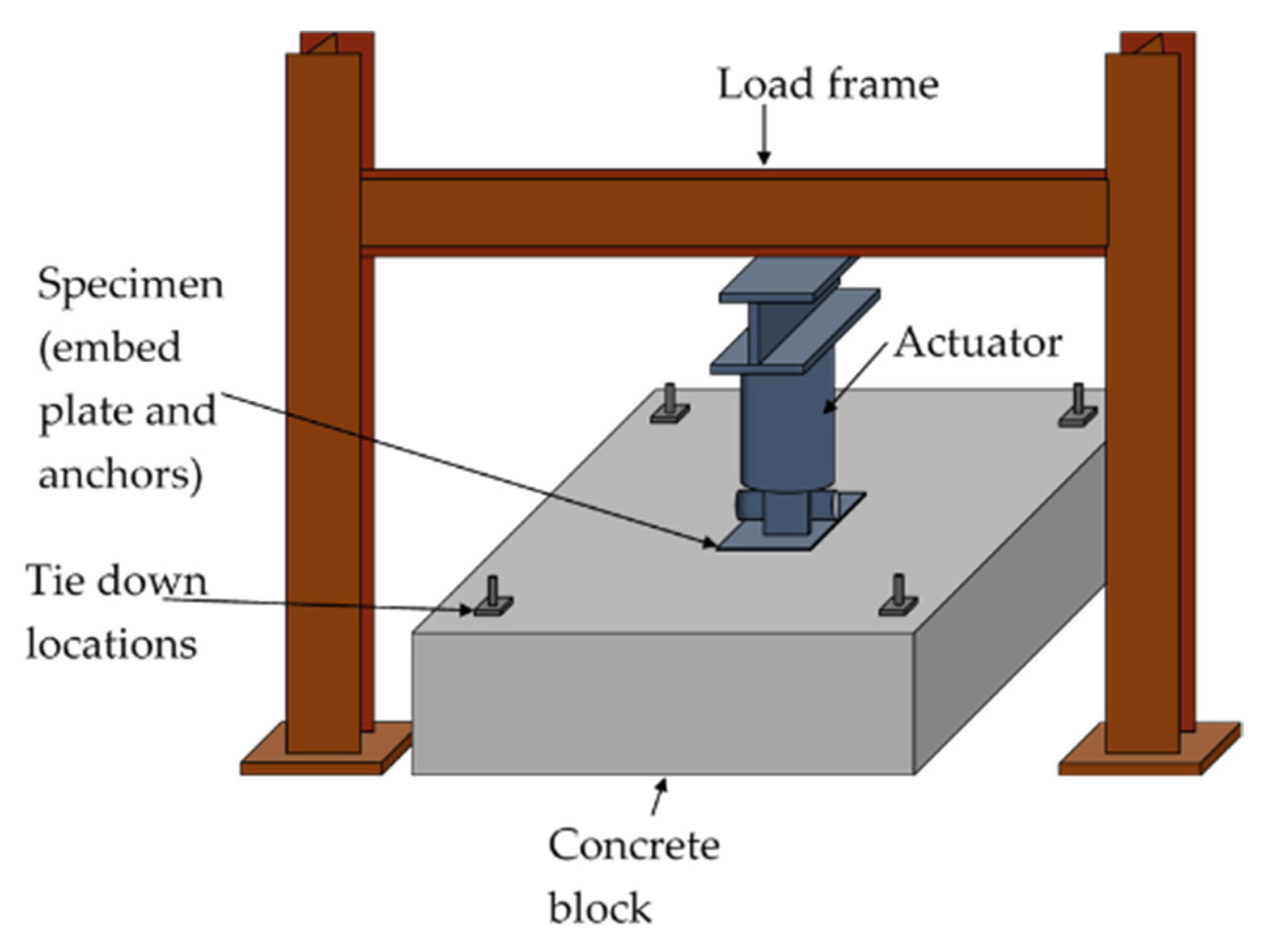
2.1. Previous Physical Experiments
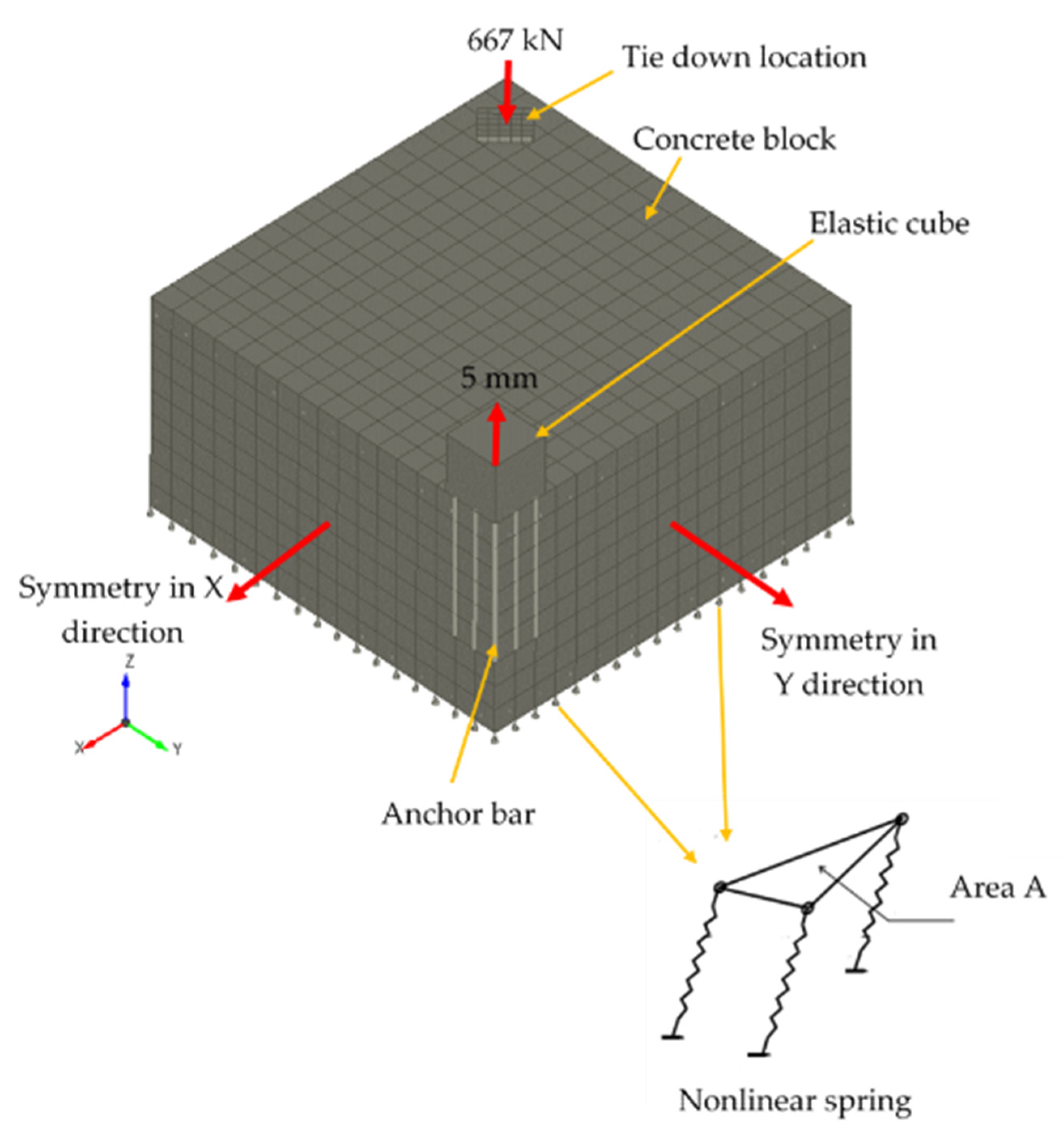
2.2. Finite Element Model
2.2.1. Concrete Material Model
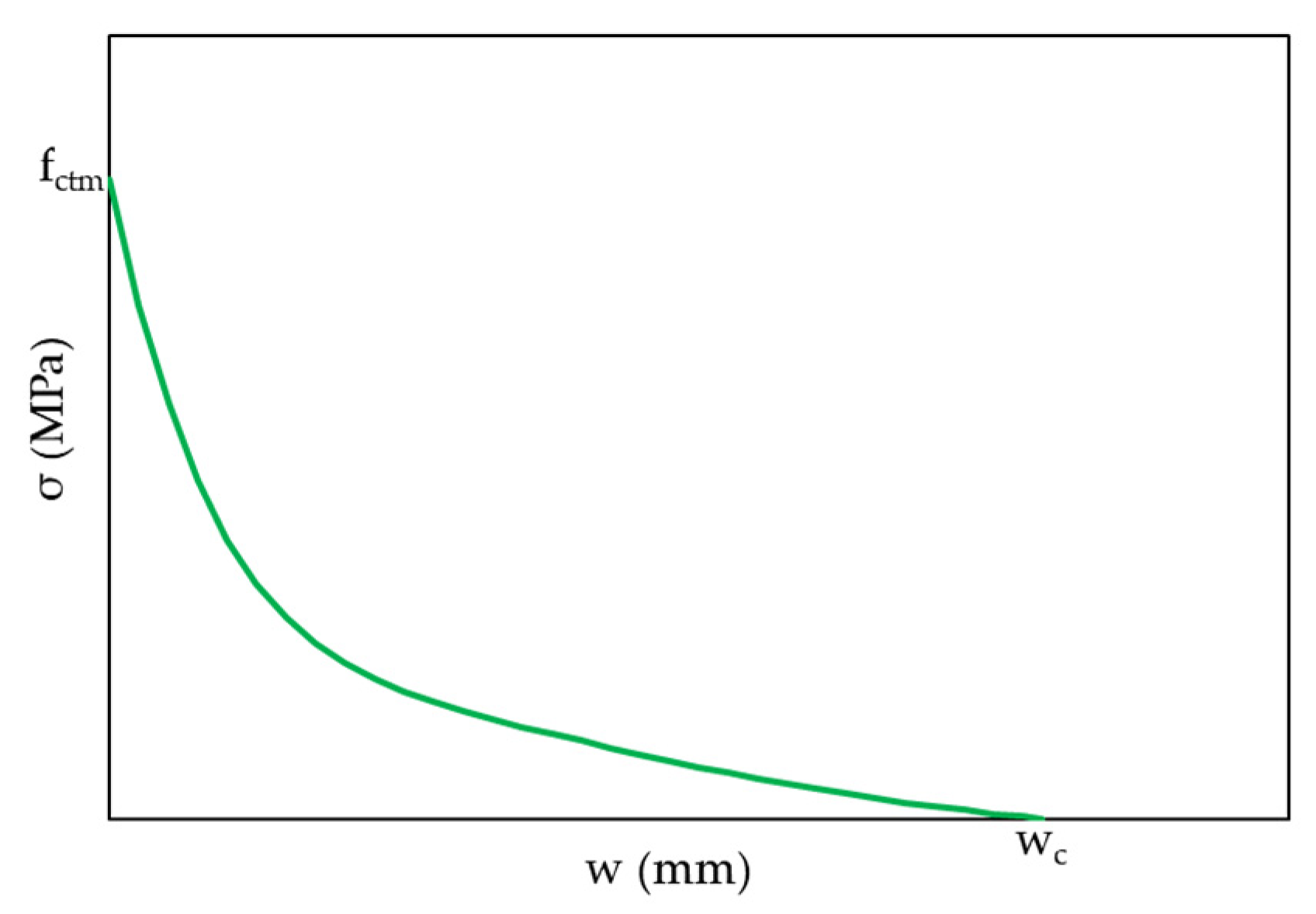
2.2.2. Tension Softening Curve for Concrete Material Model
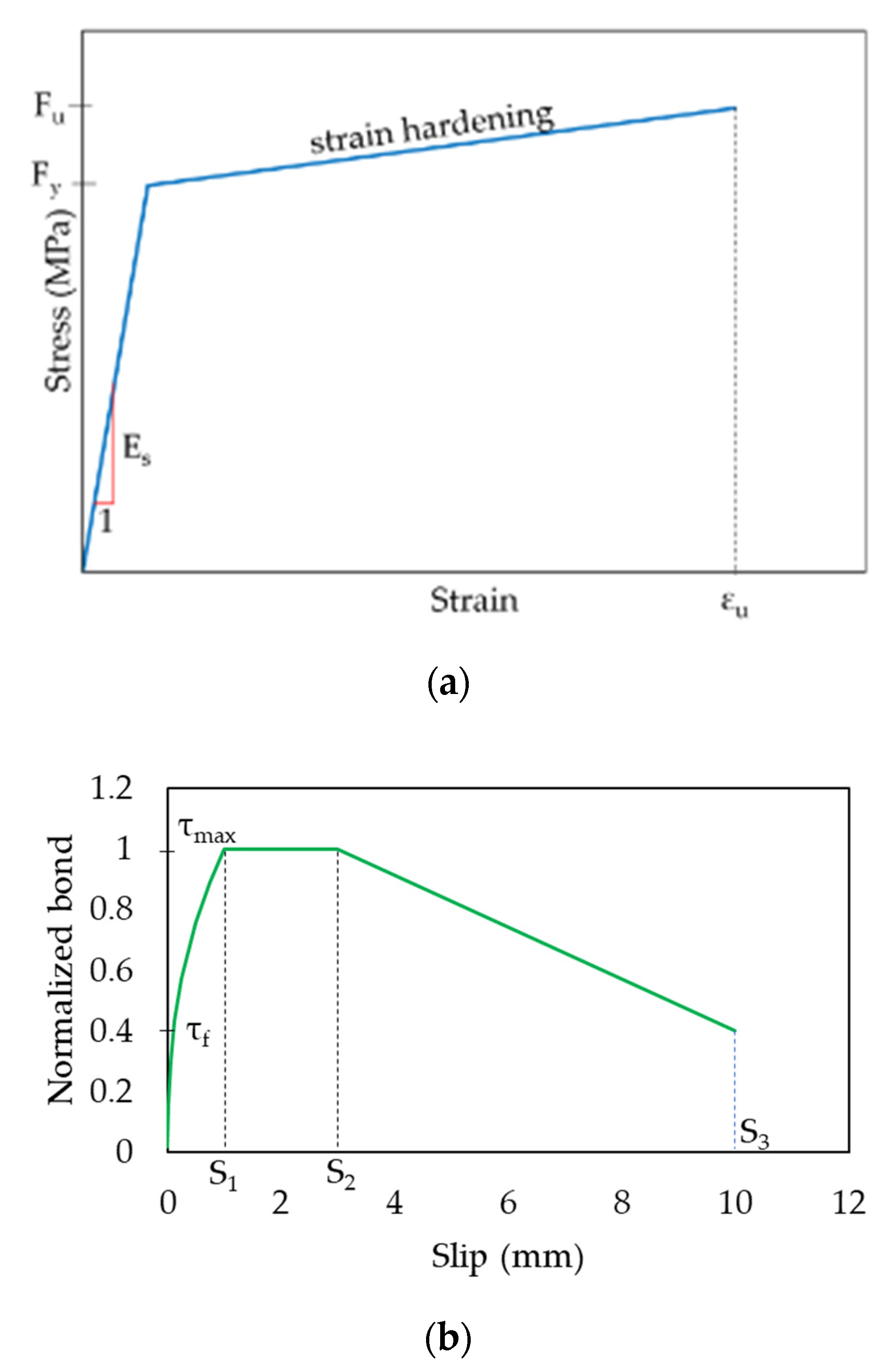
2.2.3. Steel Model and Interaction between Steel and Concrete
2.2.4. Element Type and Mesh Size
2.2.5. Benchmarked Single Anchor Model
2.3. Benchmarked Group Anchor Model
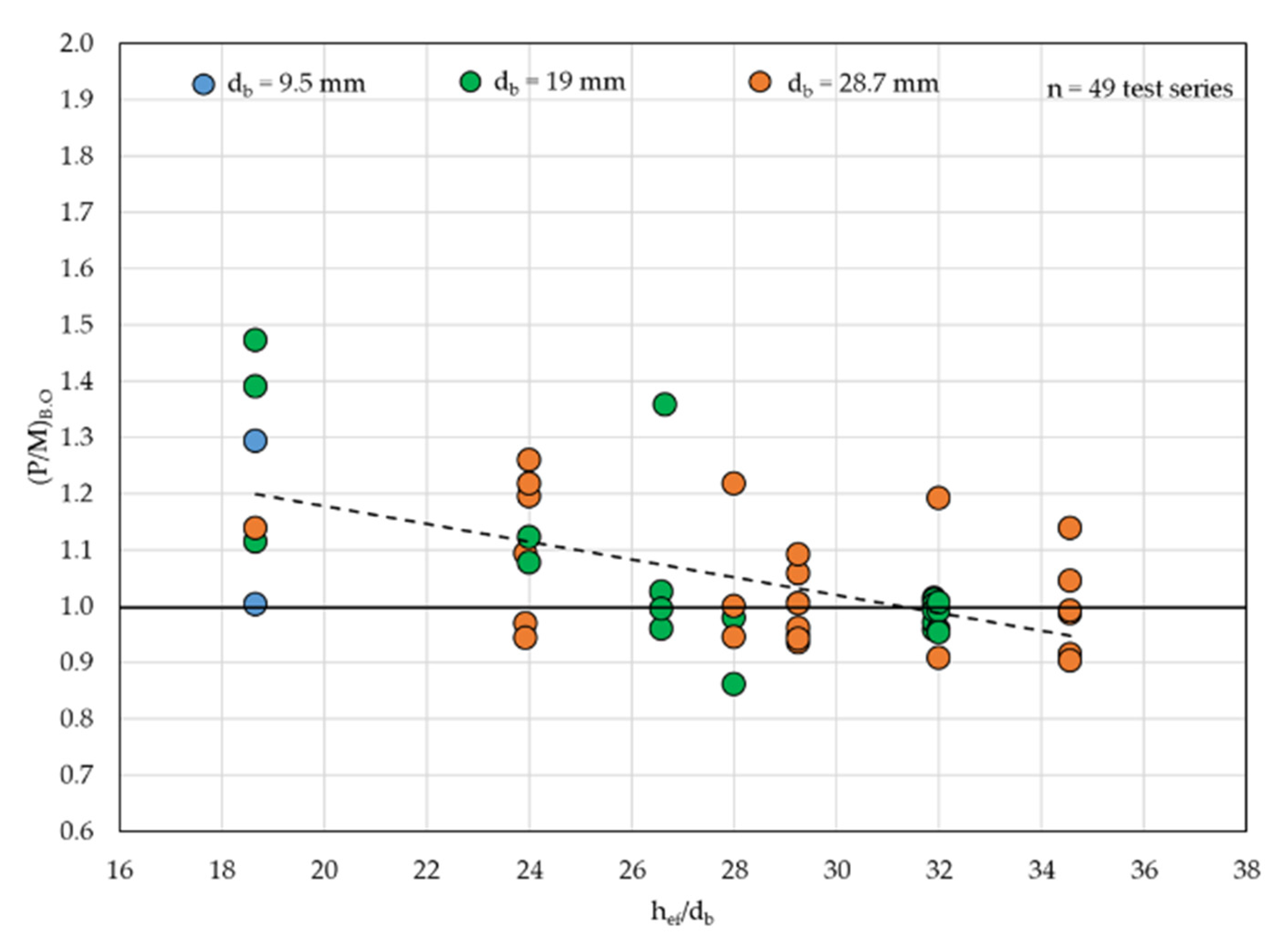
3. Parametric Study
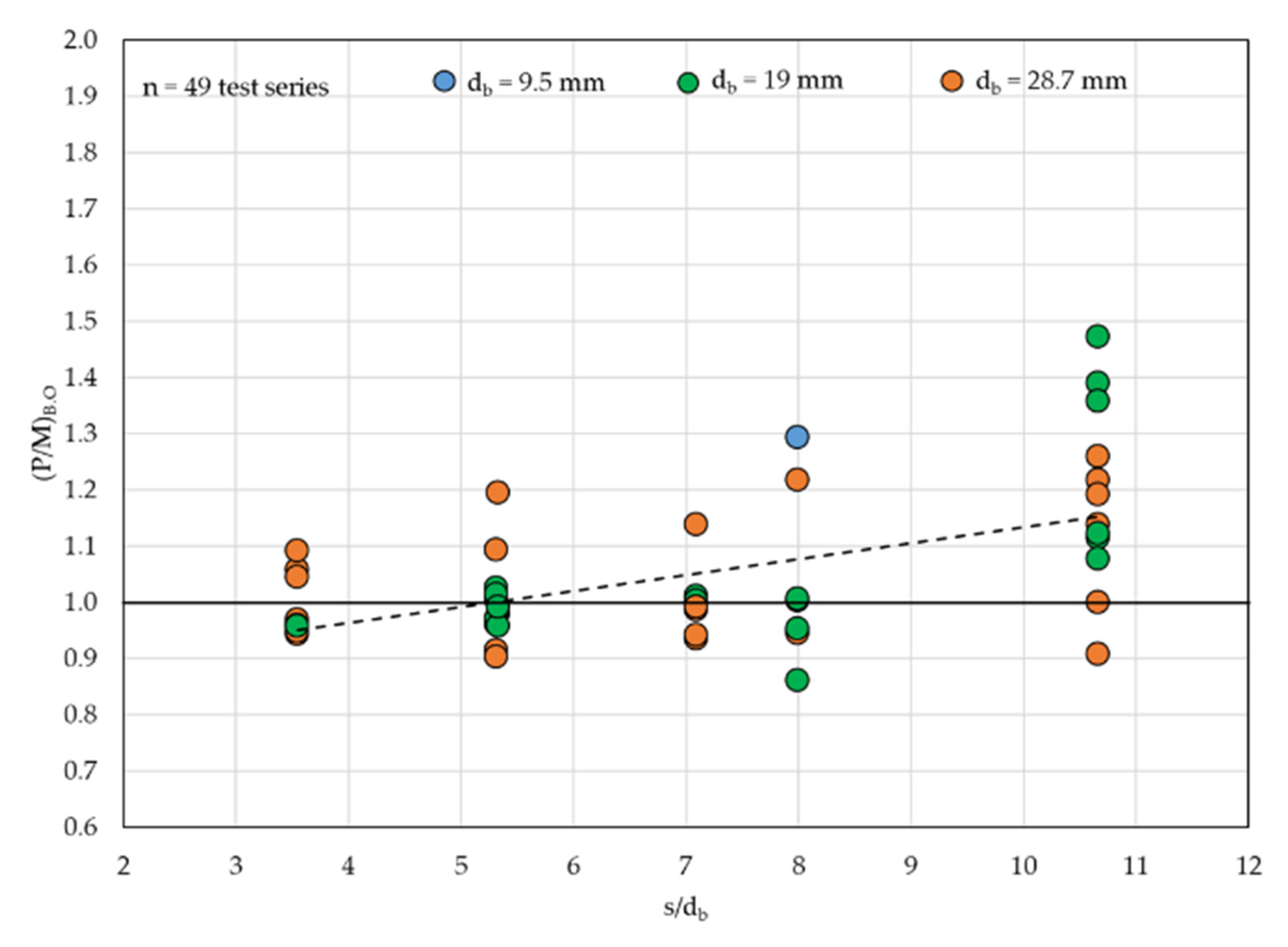
Results from Parametric Study
4. Conclusions
- Concrete breakout failure was dominant in tension loaded DRA groups with adequate development lengths (hef > Ld) and typical configurations.
- The ACI CCD formula was found to be a feasible method to estimate concrete capacity of tension loaded DRAs using a kc factor no greater than 26.
- Smaller spacing and deeper embedment of DRAs led to less conservative predictions of breakout capacity using the CCD formula.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- ACI Committee 349, American Concrete Institute; International Organization for Standardization. Code Requirements for Nuclear Safety Related Concrete Structures (ACI 349-13) and Commentary; American Concrete Institute: Farmington Hills, MI, USA, 2013. [Google Scholar]
- Chicchi, R.; Varma, A.H.; Seo, J.; Bradt, T.; McCarty, E. Experimental Testing of Tension-Loaded Deformed Anchors in Concrete. ACI Struct. J. 2020, 117, 133–146. [Google Scholar] [CrossRef]
- Mahadik, V.; Sharma, A.; Hofmann, J. Cast-in and post-installed rebar anchorage systems in RC column foundation joints: Experimental investigations. Eng. Struct. 2021, 238, 112208. [Google Scholar] [CrossRef]
- Mahrenholtz, C.; Eligehausen, R.; Reinhardt, H.W. Qualification and design of cast-in-place and post-installed reinforcing bar anchorages. ACI Struct. J. 2020, 117, 3–16. [Google Scholar] [CrossRef]
- ACI Committee 318, American Concrete Institute; International Organization for Standardization. Building Code Requirements for Structural Concrete (ACI 318-19) and Commentary; American Concrete Institute: Farmington Hills, MI, USA, 2008. [Google Scholar]
- Mahrenholtz, C.; Eligehausen, R. Groups of reinforcing bar anchorages—A comparison of the capacity calculated as end anchorage and as adhesive anchor. In Proceedings of the fib Symposium 2019, Krakow, Poland, 27–29 May 2019. [Google Scholar]
- Mahrenholtz, C.; Akguzel, U.; Eligehausen, R.; Pampanin, S. New Design Methodology for Seismic Column-to-Foundation Anchorage Connections. ACI Struct. J. 2014, 111, 1179–1190. [Google Scholar] [CrossRef]
- Fuchs, W.; Eligehausen, R.; Breen, J.E. Concrete capacity design (CCD) approach for fastening to concrete. ACI Struct. J. 1995, 92, 73–94. [Google Scholar]
- Part 4. Design of fastenings for use in concrete. In EN1992-4 Eurocode 2 Design of Concrete Structures; European Committee for Standardization: Brussels, Belgium, 2018; EN1992-4:2018 (E).
- Bokor, B.; Sharma, A.; Hofmann, J. Experimental investigations on concrete cone failure of rectangular and non-rectangular anchor groups. Eng. Struct. 2019, 188, 202–217. [Google Scholar] [CrossRef]
- Cervenka, V.; Jendele, L.; Cervenka, J. ATENA Program Documentation Part 1 Theory; Cervenka Consulting: Prague, Czech Republic, 2011; p. 231. [Google Scholar]
- Federation Internationale du Beton. fib Model Code for Concrete Structures 2010; Ernst & Sohn, a Wiley Brand: Lausanne, Switzerland, 2013. [Google Scholar]
- Vos, E. Influence of Loading Rate and Radial Pressure on Bond in Reinforced Concrete. Ph.D. Thesis, Delft University, Delft, Netherlands, 1983; pp. 219–220. [Google Scholar]
- Cornelissen, H.A.W.; Hordijk, D.A.; Reinhardt, H.W. Experimental determination of crack softening characteristics of normal-weight and lightweight concrete. Heron 1986, 31, 45–56. [Google Scholar]
- Comité Euro-International du Béton. CEB-FIP Model Code 1990: Design Code; Thomas Telford Publishing: London, UK, 1993. [Google Scholar]
- Cervenka, V.; Červenka, J.; Pukl, R.; Sajdlova, T. Prediction of Shear Failure of Large Beams Based on Fracture Mechanics. In Proceedings of the 9th International Conference on Fracture Mechanics of Concrete and Concrete Structures; International Association for Fracture Mechanics of Concrete and Concrete Structures: Berkeley, CA, USA, 2016. [Google Scholar]
- Cervenka, J.; Cervenka, V.; Laserna, S. On crack band model in finite element analysis of concrete fracture in engineering practice. Eng. Fract. Mech. 2018, 197, 27–47. [Google Scholar] [CrossRef]

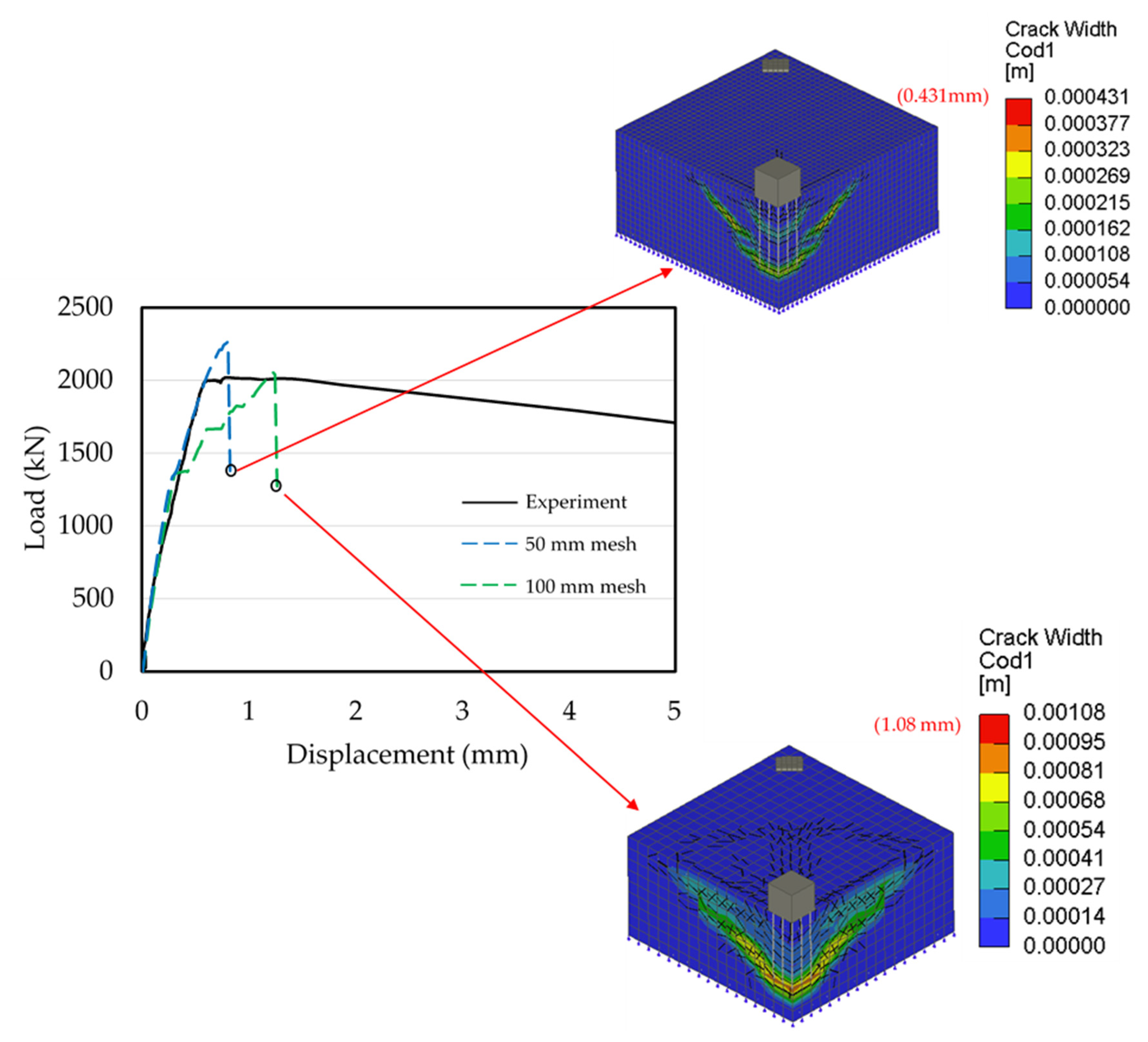

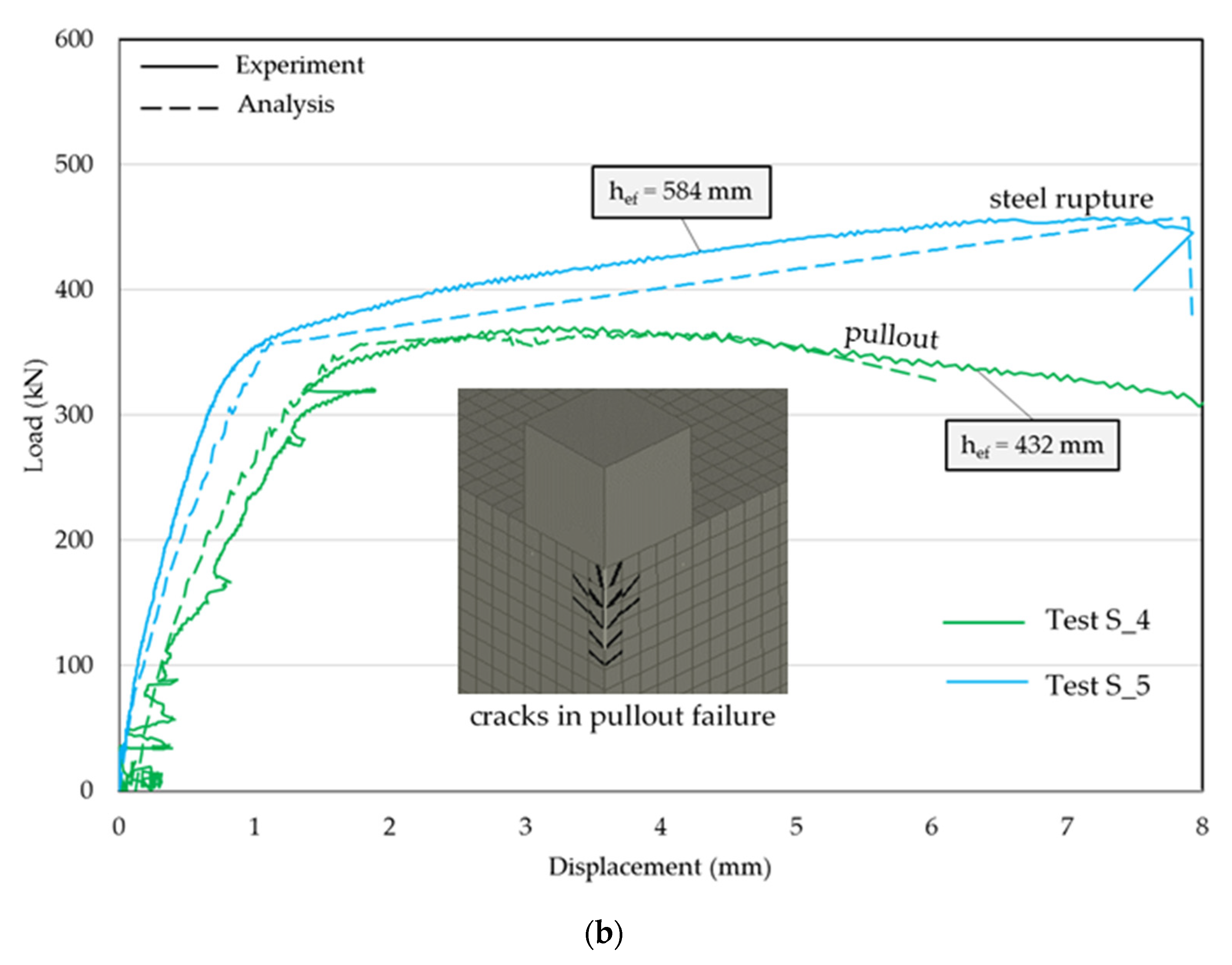
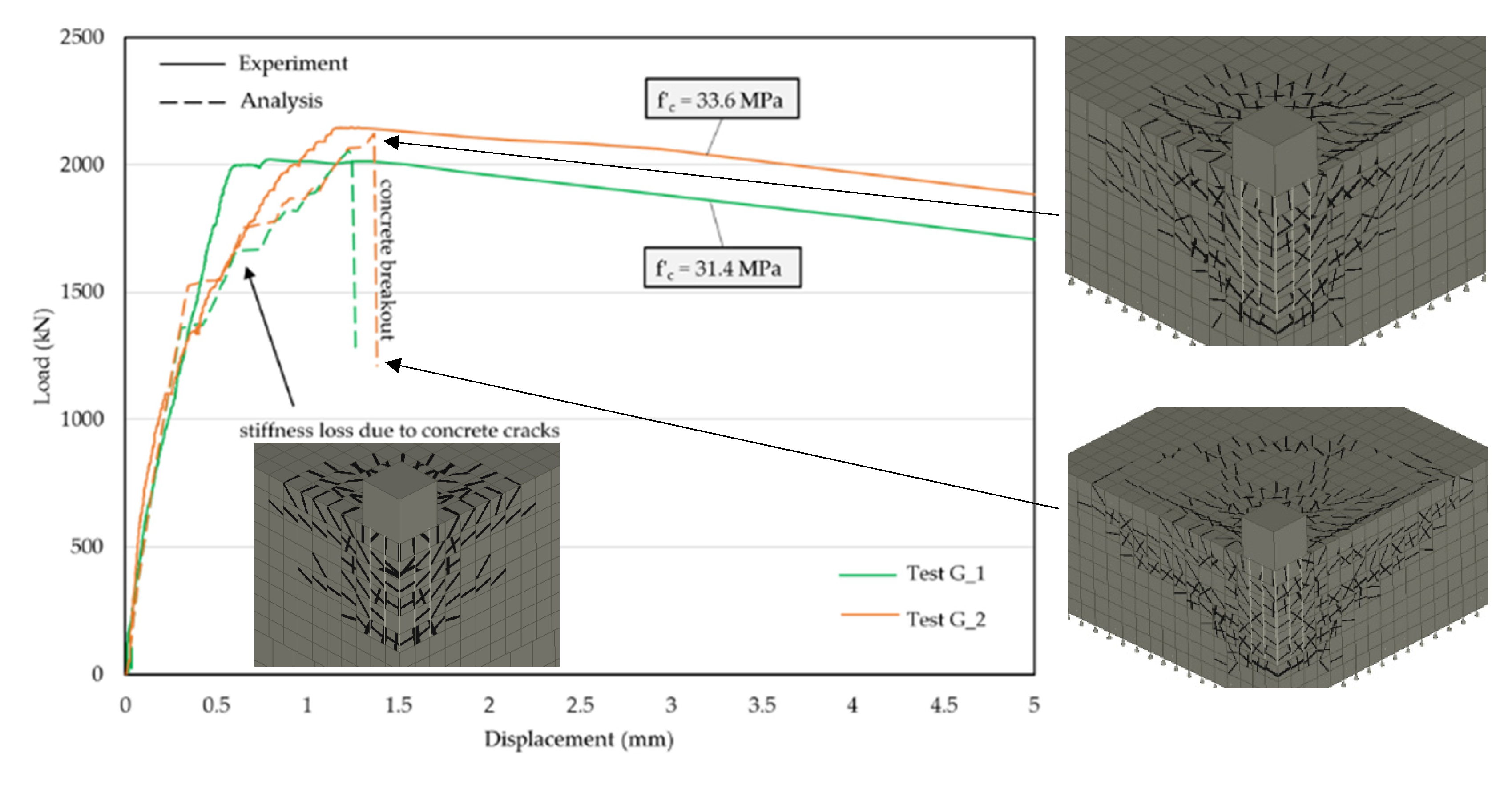


| Test | db (mm) | a × b | s (mm) | hef (mm) | f’c (MPa) |
|---|---|---|---|---|---|
| S_1 | 19 | 1 × 1 | - | 203 | 29.6 |
| S_2 | 19 | 1 × 1 | - | 279 | 29.6 |
| S_3 | 19 | 1 × 1 | - | 483 | 29.6 |
| S_4 | 28.7 | 1 × 1 | - | 432 | 26.3 |
| S_5 | 28.7 | 1 × 1 | - | 584 | 26.3 |
| G_1 | 19 | 5 × 5 | 102 | 609.6 | 31.4 |
| G_2 | 19 | 5 × 5 | 102 | 609.6 | 33.6 |
| Concrete Properties | MC 2010 [12] Formulas | For Test G_1 |
|---|---|---|
| Cylinder compressive strength | (MPa) | 31.4 MPa (from experiment [2]) |
| Tensile strength | (MPa) | 2.45 MPa |
| Tangent modulus of elasticity | (MPa) | 31,483.4 MPa |
| Fracture energy | (N/m) | 135.76 N/m 58 N/m (used) |
| Maximum crack opening | = (mm) | 0.122 mm |
| Test | Fy (MPa) | Fu (MPa) | εu | τmax (MPa) |
|---|---|---|---|---|
| S_1 | 469.1 | 710.4 | 0.09 | 8.5 |
| S_2 | 469.1 | 710.4 | 0.09 | 8.8 |
| S_3 | 469.1 | 710.4 | 0.09 | 8.8 |
| S_4 | 552 | 710.4 | 0.06 | 10.5 |
| S_5 | 552 | 710.4 | 0.06 | 10.5 |
| G_1 | 469.1 | 710.4 | 0.09 | 8.8 |
| G_2 | 469.1 | 710.4 | 0.09 | 8.8 |
| Parameters | Values |
|---|---|
| db | 9.5 mm, 19 mm, 28.7 mm |
| a × b | (4 × 4), (5 × 5), (6 × 6) |
| s | 102 mm, 152 mm, 203 mm |
| hef | Ld, (Ld + 76), (Ld + 152) mm |
| f ′c | 27.6 MPa, 41.4 MPa |
| GF | 58 N/m |
| τmax | 2 MPa [15] |
| Fy, Fu, εu | 414 MPa, 710 MPa, 0.09 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chhetri, S.; Chicchi, R.A. Analytical Investigation of Tension Loaded Deformed Rebar Anchors in Concrete. CivilEng 2021, 2, 442-458. https://doi.org/10.3390/civileng2020025
Chhetri S, Chicchi RA. Analytical Investigation of Tension Loaded Deformed Rebar Anchors in Concrete. CivilEng. 2021; 2(2):442-458. https://doi.org/10.3390/civileng2020025
Chicago/Turabian StyleChhetri, Sandip, and Rachel A. Chicchi. 2021. "Analytical Investigation of Tension Loaded Deformed Rebar Anchors in Concrete" CivilEng 2, no. 2: 442-458. https://doi.org/10.3390/civileng2020025
APA StyleChhetri, S., & Chicchi, R. A. (2021). Analytical Investigation of Tension Loaded Deformed Rebar Anchors in Concrete. CivilEng, 2(2), 442-458. https://doi.org/10.3390/civileng2020025






