Aspects Concerning Validation of Theoretical Solution of Generalised Ladder Problem
Abstract
1. Introduction
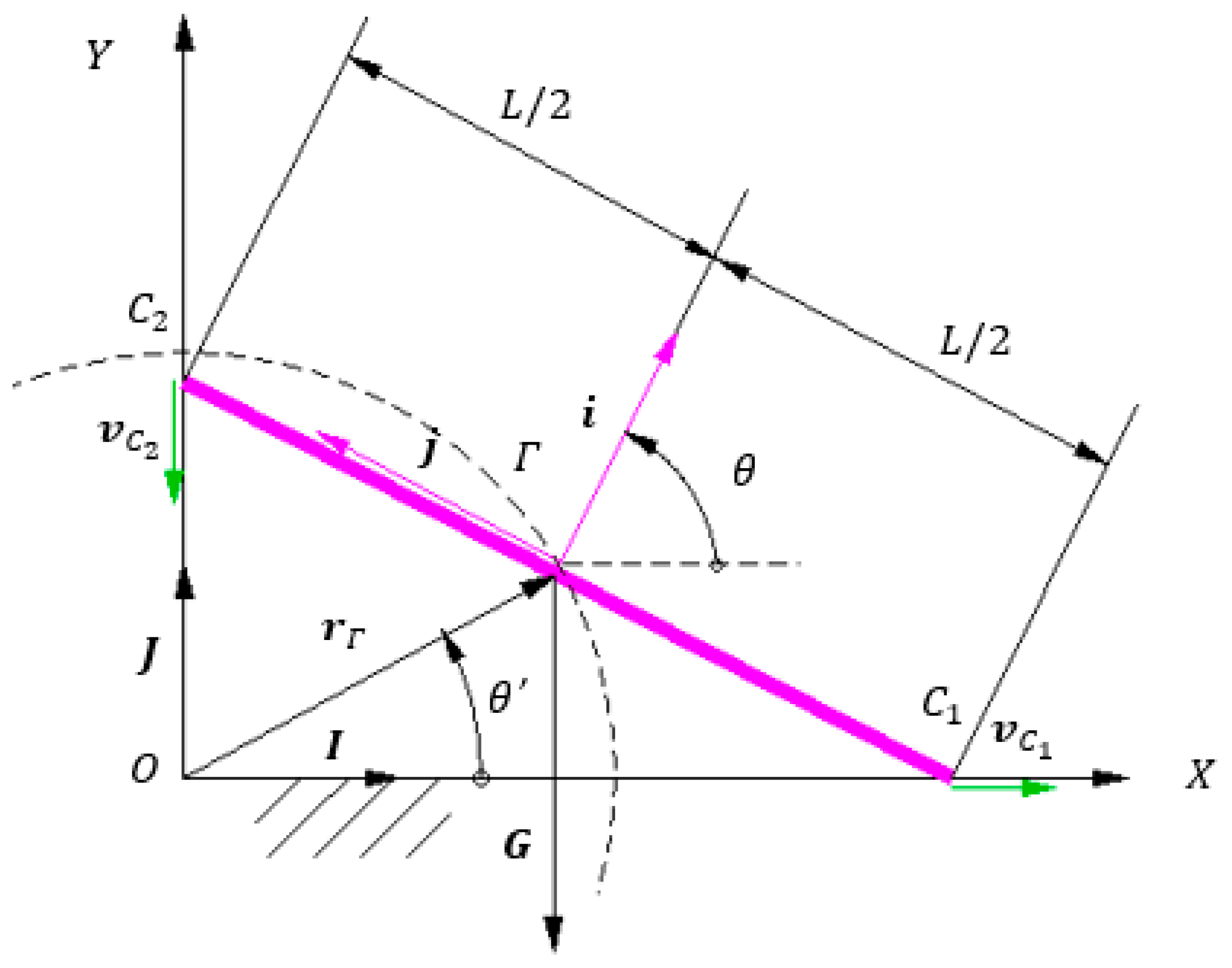
1.1. The Ladder Problem
1.2. Obtaining the Equation of Motion of the Classical Model
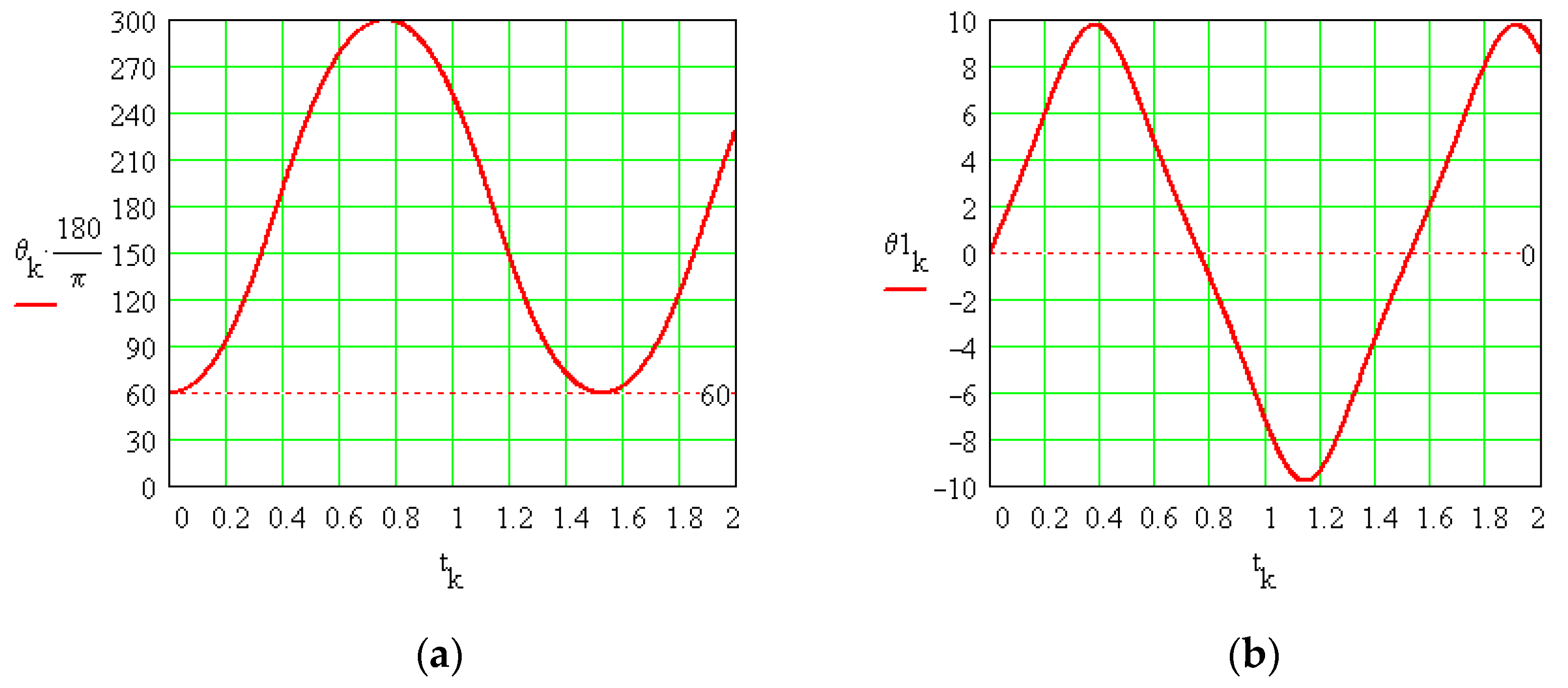
1.3. Numerical Solution of the Classical Model
2. Material and Method
- -
- The friction between the rod and the walls cannot be neglected in the actual model;
- -
- The actual rod has a certain cross-section, and the contact between the rod and the wall occurs along its edge;
- -
- The difficulty in ensuring the plane-parallel motion of the rod in the vertical plane.
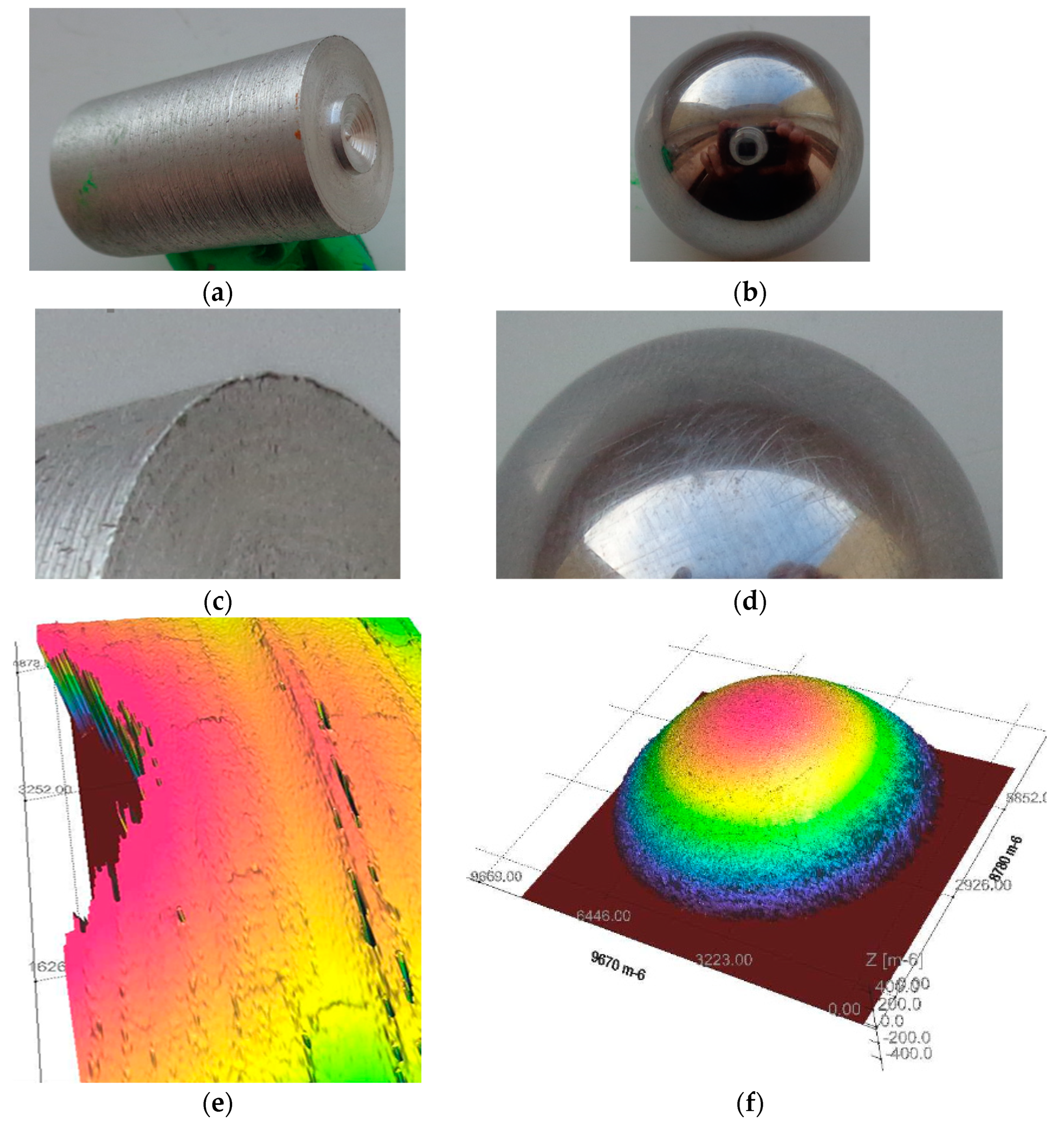
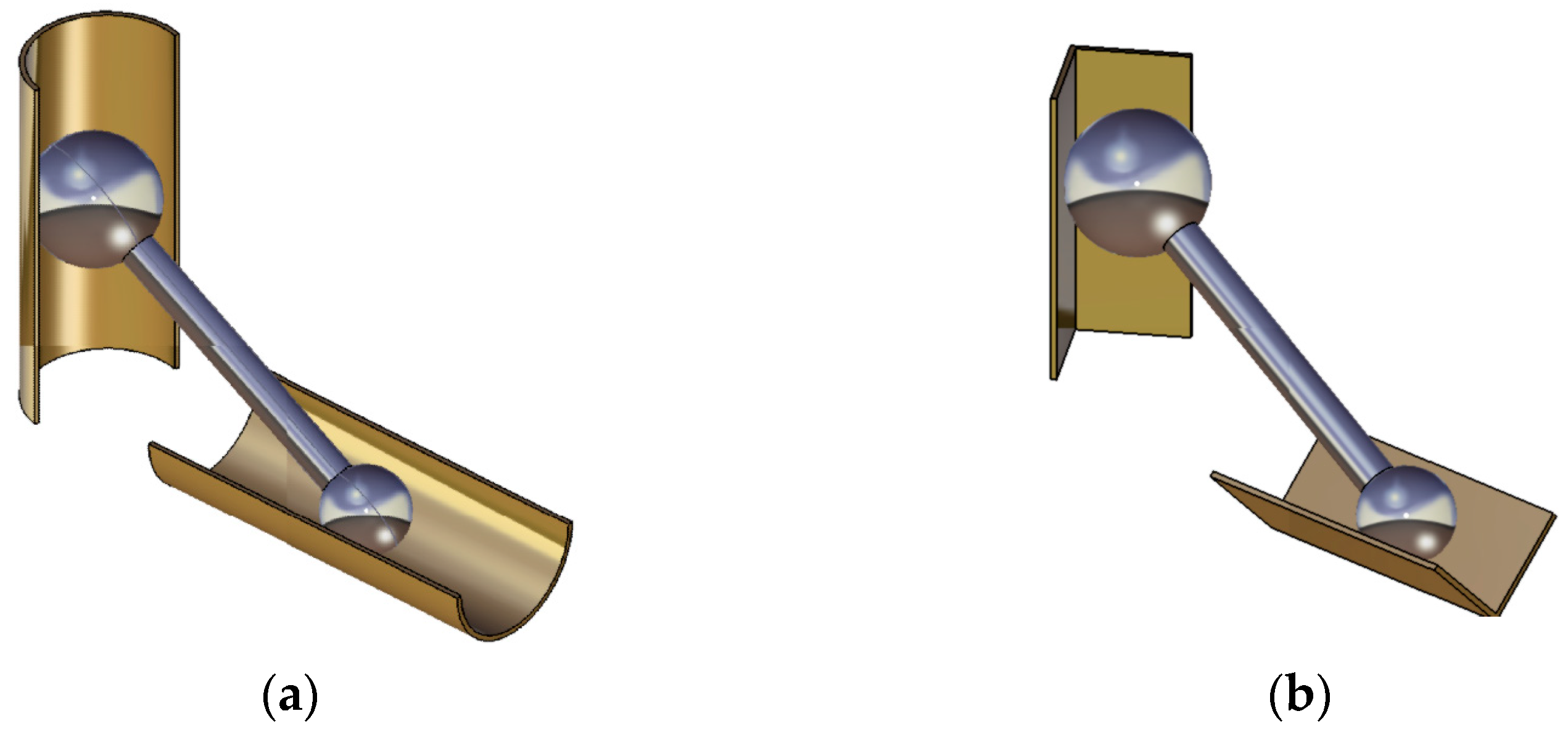
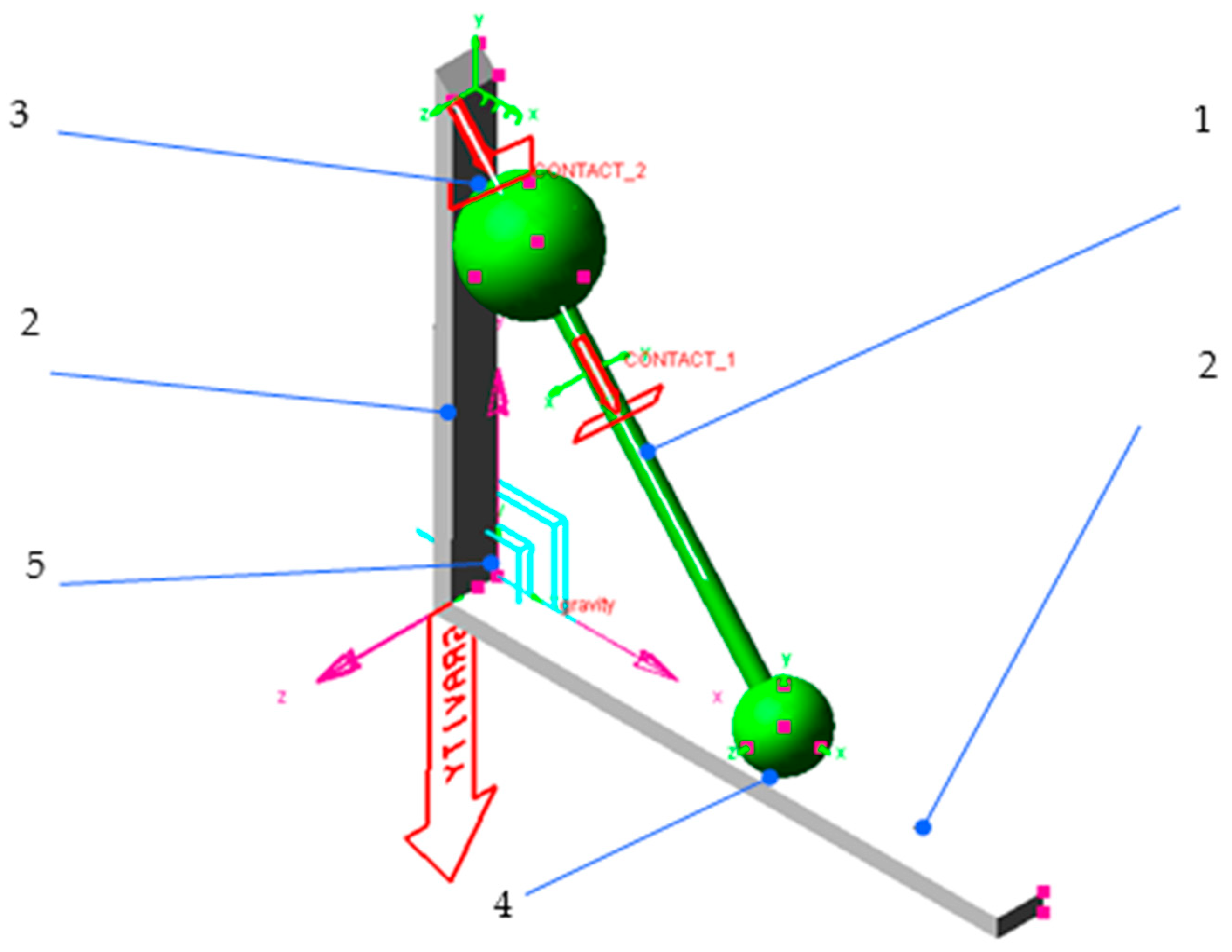
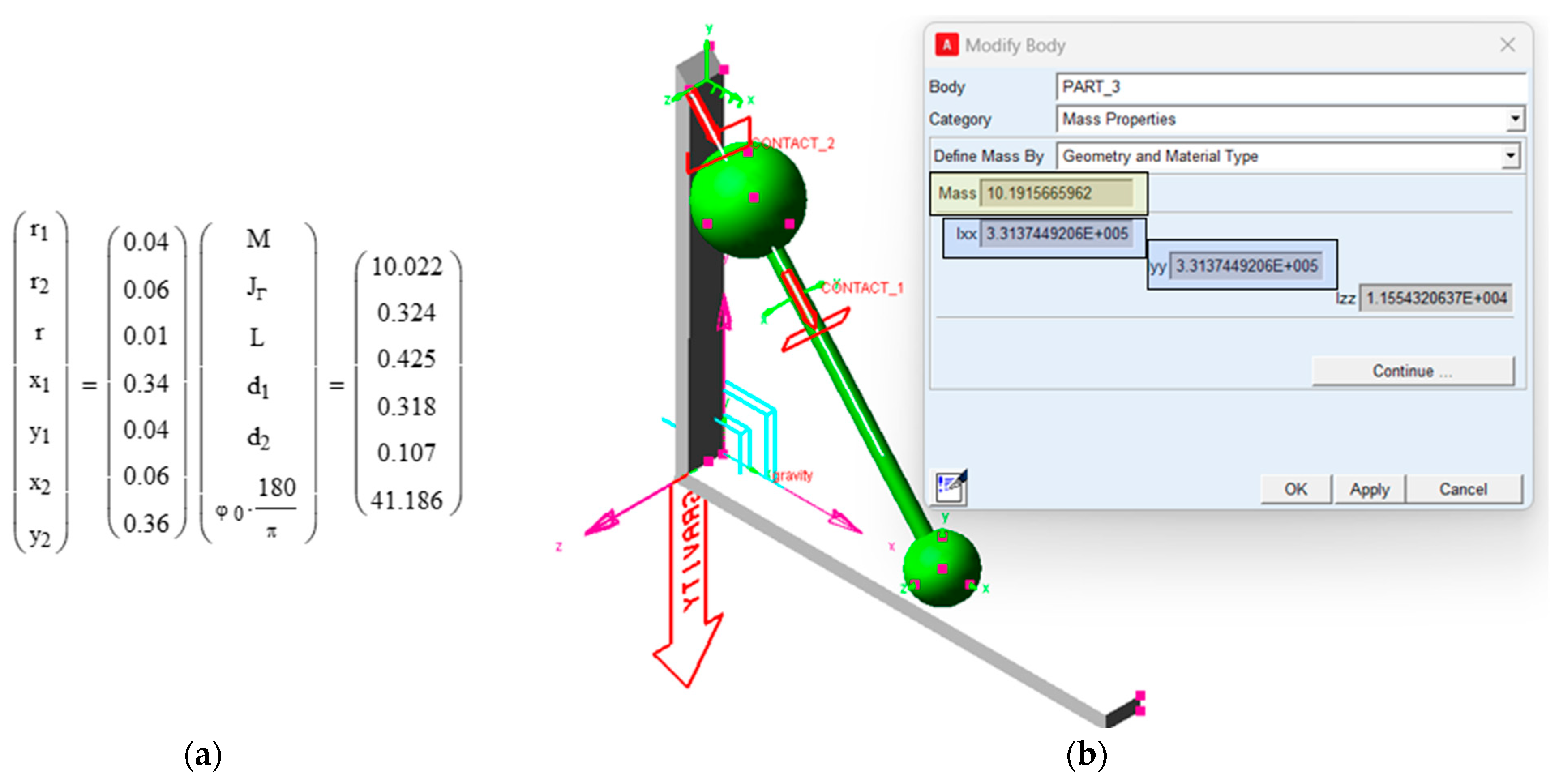
2.1. Dynamical Analysis Using Dynamic Simulation Software
2.2. Analytical Solution of the Proposed Model
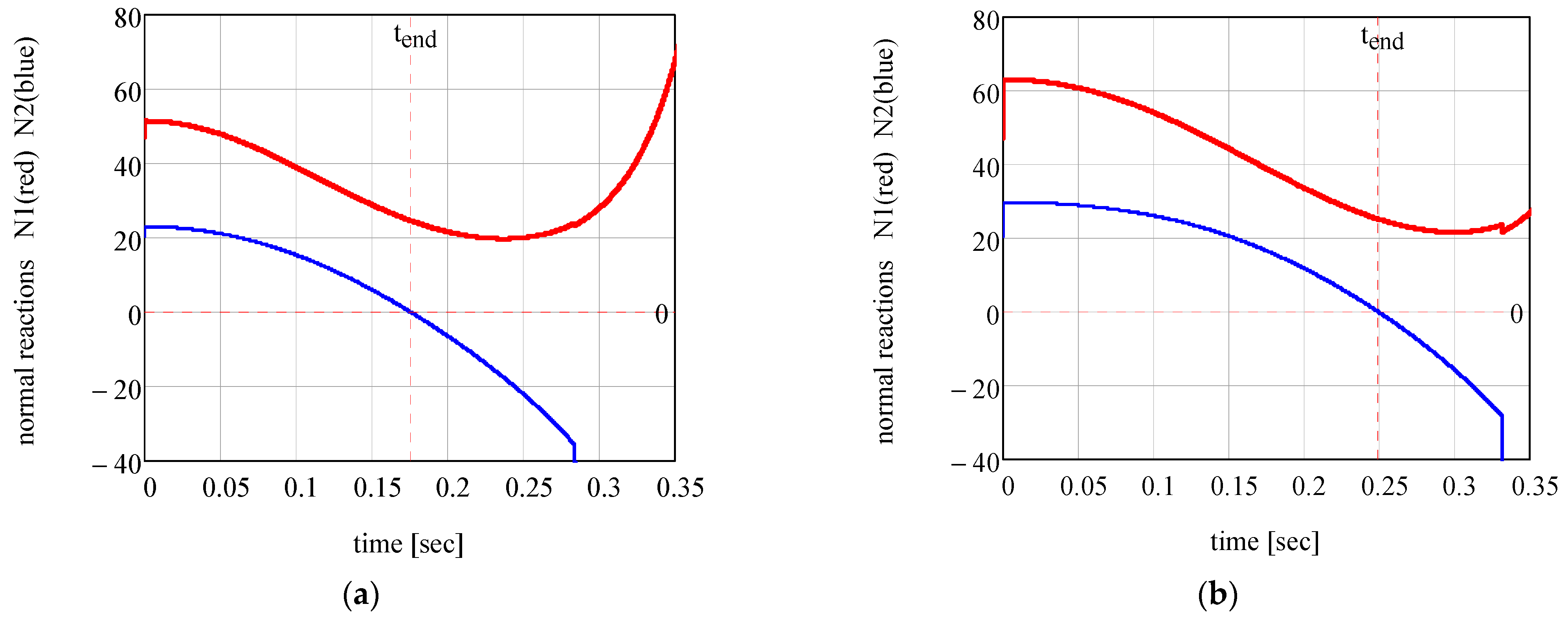
3. Results and Discussions
- Deduction of the nonlinear differential equation of motion;
- The numerical integration of the equation of motion for the specified initial conditions;
- Finding the expressions of the normal reactions from the two sphere–wall contacts;
- Finding the time when the vertical contact disrupts, imposing the condition of cancelled reaction from the vertical wall, the instant that corresponds to the transition from a 1DOF system into a 2DOF system;
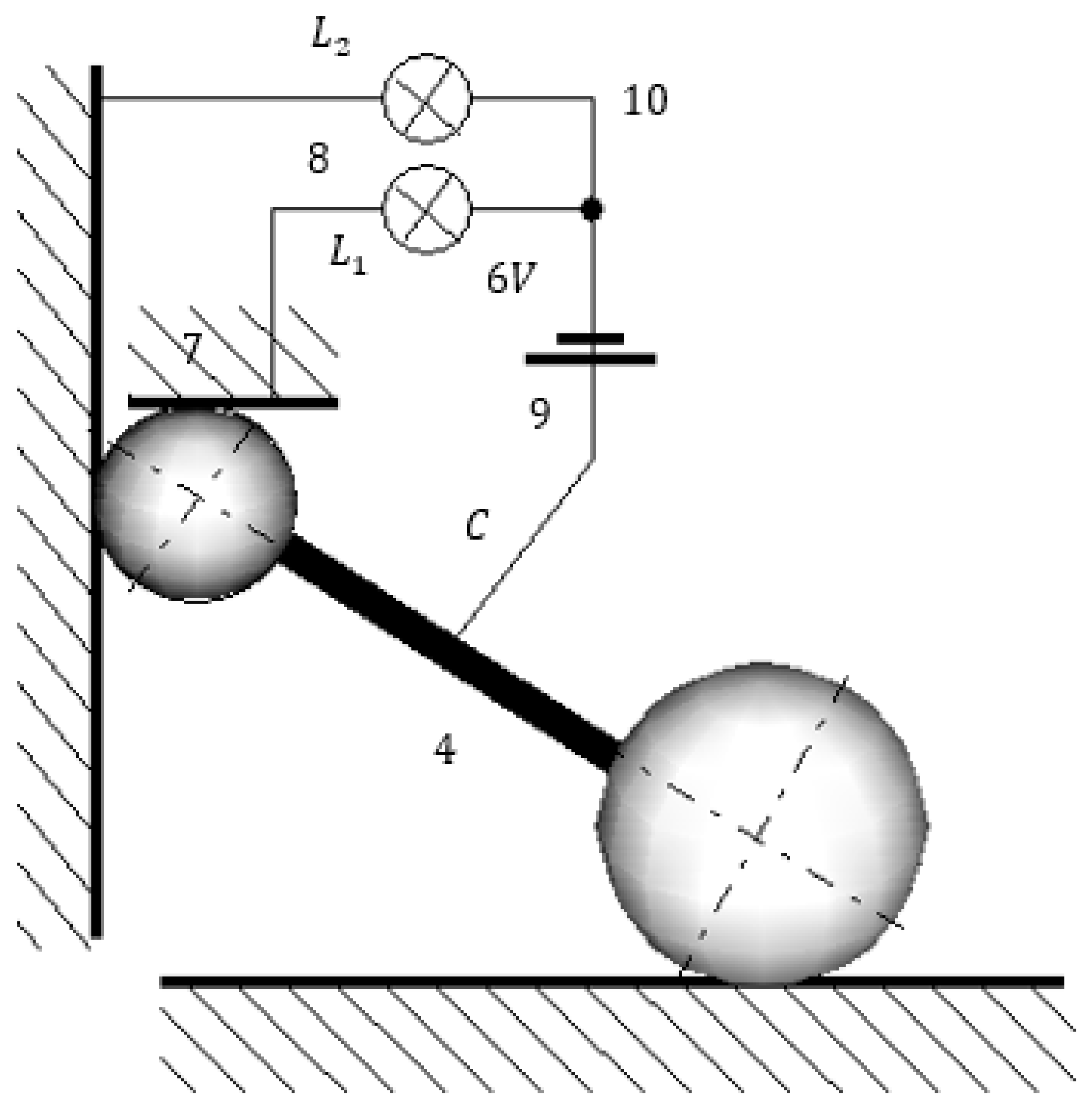
- The design and construction of a device used for experimental validation of the analytical solutions;
- The numerical simulation of the motion of the system using a software gave results in disagreement with the physical reality.
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ardema, M.D. Review of Newtonian Dynamics. In Analytical Dynamics: Theory and Applications, 1st ed.; Ardema, M.D., Ed.; Springer: New York, NY, USA, 2004; Volume 1, pp. 1–45. [Google Scholar]
- Butcher, J.C. Numerical Methods for Ordinary Differential Equations, 2nd ed.; John Wiley & Sons: Chichester, UK, 2008; pp. 93–104. [Google Scholar]
- Armanini, C.; Dal Corso, F.; Misseroni, D.; Bigoni, D. Configurational forces and nonlinear structural dynamics. J. Mech. Phys. Solids 2019, 130, 82–100. [Google Scholar] [CrossRef]
- Popova, E.; Popov, V. The research works of Coulomb and Amontons and generalized laws of friction. Friction 2015, 3, 183–190. [Google Scholar] [CrossRef]
- Coulomb, C.A. Théorie des Machines Simples, en Ayant Égard au Frottement de Leurs Parties, et à la Roideur des Cordages; Bachelier, Libraire: Paris, France, 1821; pp. 212–245. [Google Scholar]
- Marques, F.; Flores, P.; Claro, J.C.; Lankarani, H. A survey and comparison of several friction force models for dynamic analysis of multibody mechanical systems. Nonlinear Dyn. 2016, 86, 1407–1443. [Google Scholar]
- Marques, F.; Flores, P.; Claro, J.C.P.; Lankarani, H.M. Modeling and analysis of friction including rolling effects in multibody dynamics: A review. Multibody Syst. Dyn. 2019, 45, 223–244. [Google Scholar]
- Bengisu, M.T.; Akay, A. Stability of friction-induced vibrations in multi-degree-of-freedom systems. J. Sound Vibr. 1994, 171, 557–570. [Google Scholar]
- Dahl, P.R. Solid friction damping in mechanical vibrations. AIAA J. 1976, 14, 1675–1682. [Google Scholar]
- Dahl, P.R. A Solid Friction Model; Technical Report; The Aerospace Corporation: El Segundo, CA, USA, 1968; pp. 12–17. [Google Scholar]
- Pennestrì, E.; Valentini, P.P.; Vita, L. Multibody dynamics simulation of planar linkages with Dahl friction. Multibody Syst. Dyn. 2007, 17, 321–347. [Google Scholar] [CrossRef]
- Armstrong-Hélouvry, B.; Dupont, P.; de Wit Canudas, C. A survey of models, analysis tools and compensation methods for the control of machines with friction. Automatica 1994, 30, 1083–1138. [Google Scholar] [CrossRef]
- Alaci, S.; Alexandru, C.; Ciornei, F.-C.; Doroftei, I.; Irimescu, L. Chaos illustrations in dynamics of mechanisms. In New Trends in Mechanism and Machine Science; Mechanisms and Machine Science; Springer: Cham, Switzerland, 2020; Volume 89, pp. 297–304. [Google Scholar] [CrossRef]
- Available online: https://www.laddersukdirect.co.uk/latest-news/?tag=history+of+ladders (accessed on 10 January 2025).
- Available online: https://www.rcet.org.in/uploads/academics/regulation2021/rohini_14042492967.pdf (accessed on 12 January 2025).
- Available online: https://physique.merici.ca/mechanics/chap13mech.pdf (accessed on 8 January 2025).
- Mendelson, K.S. Statics of a ladder leaning against a rough wall. Am. J. Phys. 1995, 63, 148–150. [Google Scholar] [CrossRef]
- Glane, S. Combining dynamics and numerics using the falling ladder problem. Eur. J. Phys. 2019, 40, 055001. [Google Scholar] [CrossRef]
- Silverman, M. Reaction Forces on a Fixed Ladder in Static Equilibrium: Analysis and Definitive Experimental Test of the Ladder Problem. World J. Mech. 2018, 8, 311–342. [Google Scholar] [CrossRef]
- The Static Ladder Problem with Two Sources of Friction. Available online: https://wikis.mit.edu/confluence/display/RELATE/The+Ladder+Problem (accessed on 15 January 2025).
- Davidson, J.K.; Hunt, K.H. Robots and Screw Theory: Applications of Kinematics and Statics to Robotics, 1st ed.; Oxford University Press: New York, NY, USA, 2004; pp. 134–191. [Google Scholar]
- Atkinson, K.; Han, W.; Stewart, D. Numerical Solution of Ordinary Differential Equations; John Wiley & Sons: Hoboken, NJ, USA, 2009; pp. 67–94. [Google Scholar]
- Greenwood, J.; Tripp, J. Contact of two nominally flat rough surfaces. Proc. Inst. Mech. Eng. 1970, 185, 625–633. [Google Scholar]
- Giesbers, J. Contact Mechanics in MSC Adams—A Technical Evaluation of the Contact Models in Multibody Dynamics Software MSC Adams. Bachelor’s Thesis, University Twente, Enschede, The Netherland, 2012; pp. 13–19. [Google Scholar]
- Flores, P.; Lankarani, H.M. Contact Force Models for Multibody Dynamics; Solid Mechanics and Its Applications Series; Springer International Publishing: Cham, Switzerland, 2016; Volume 226, p. 171. [Google Scholar]
- Ghorashi, M. New insights on the falling ladder problem. Meccanica 2022, 57, 2281–2292. [Google Scholar] [CrossRef]
- Glane, S.; Müller, W.H. The sliding ladder problem revisited in phase space. Am. J. Phys. 2019, 87, 444–448. [Google Scholar]
- Kapranidis, S.; Koo, R. Variations of the sliding ladder problem. Coll. Math. J. 2008, 39, 374–379. [Google Scholar]
- Silverman, M. The Role of Friction in the Static Equilibrium of a Fixed Ladder: Theoretical Analysis and Experimental Test. World J. Mech. 2018, 8, 445–463. [Google Scholar] [CrossRef]
- Salu, Y. Revisiting the ladder on a wall problem. Phys. Teach. 2011, 49, 289–290. [Google Scholar]
- González, A.G.; Gratton, J. Reaction forces on a ladder leaning against a rough wall. Am. J. Phys. 1996, 64, 1001–1005. [Google Scholar]
- Oliveira, J.B.; Carvalho, P.S.; Mota, M.F.; Quintas, M.J. Dynamics of a sliding ladder leaning against a wall. Phys. Educ. 2015, 50, 329–334. [Google Scholar]
- Ardema, M.D. Newton-Euler Dynamics; Springer: New York, NY, USA, 2006; pp. 231–260. ISBN 978-0-387-23276-8. [Google Scholar]
- Mangeron, D.; Irimiciuc, N. Mecanica Rigidelor cu Aplicaţii în Inginerie; Editura Tehnică: Bucureşti, Romania, 1978; Volume I, pp. 304–319. (In Romanian) [Google Scholar]
- Venetis, J. An Explicit Form of Signum Function. Mathematics 2024, 12, 3246. [Google Scholar] [CrossRef]
- Maxfield, B. Engineering with Mathcad; Elsevier Linacre House: Oxford, UK, 2006; pp. 317–335. [Google Scholar]
- Alaci, S.; Ciornei, F.-C.; Lupascu, C.; Romanu, I.-C. Mathematical Model of the Evolution of a Simple Dynamic System with Dry Friction. Axioms 2024, 13, 372. [Google Scholar] [CrossRef]
- Eren, H. 193 Specialty Displacement and Angle Sensors. In Handbook of Measuring System Design; Sydenham, P.H., Thorn, R., Eds.; Part 13, Section 2. Displacement and Angle Measurement; John Wiley & Sons: Hoboken, NJ, USA, 2005; Volume 3. [Google Scholar]
- Wei, Y. Applications of Ultrasonic Sensors: A Review. Appl. Comp. Eng. 2024, 99, 144–148. [Google Scholar] [CrossRef]
- Zamani, N.; Weaver, G.M. CATIA V5 Tutorials Mechanism Design & Animation, 1st ed.; SDC Publications: Mission, KS, USA, 2012. [Google Scholar]
- Arnoux, J.J.; Sutter, G.; List, G.; Molinari, A. Friction experiments for dynamical coefficient measurement. Adv. Tribol. 2011, 2011, 613581. [Google Scholar] [CrossRef][Green Version]
- Marino, L.; Cicirello, A. Experimental investigation of a single-degree-of-freedom system with Coulomb friction. Nonlinear Dyn. 2020, 99, 1781–1799. [Google Scholar] [CrossRef]
- Rill, G.; Schaeffer, T.; Schuderer, M. LuGre or not LuGre. Multibody Syst. Dyn. 2023, 60, 191–218. [Google Scholar] [CrossRef]
- Schuderer, M.; Rill, G.; Schaeffer, T.; Carsten, S. Friction modeling from a practical point of view. Multibody Syst. Dyn. 2024, 63, 141–158. [Google Scholar] [CrossRef]








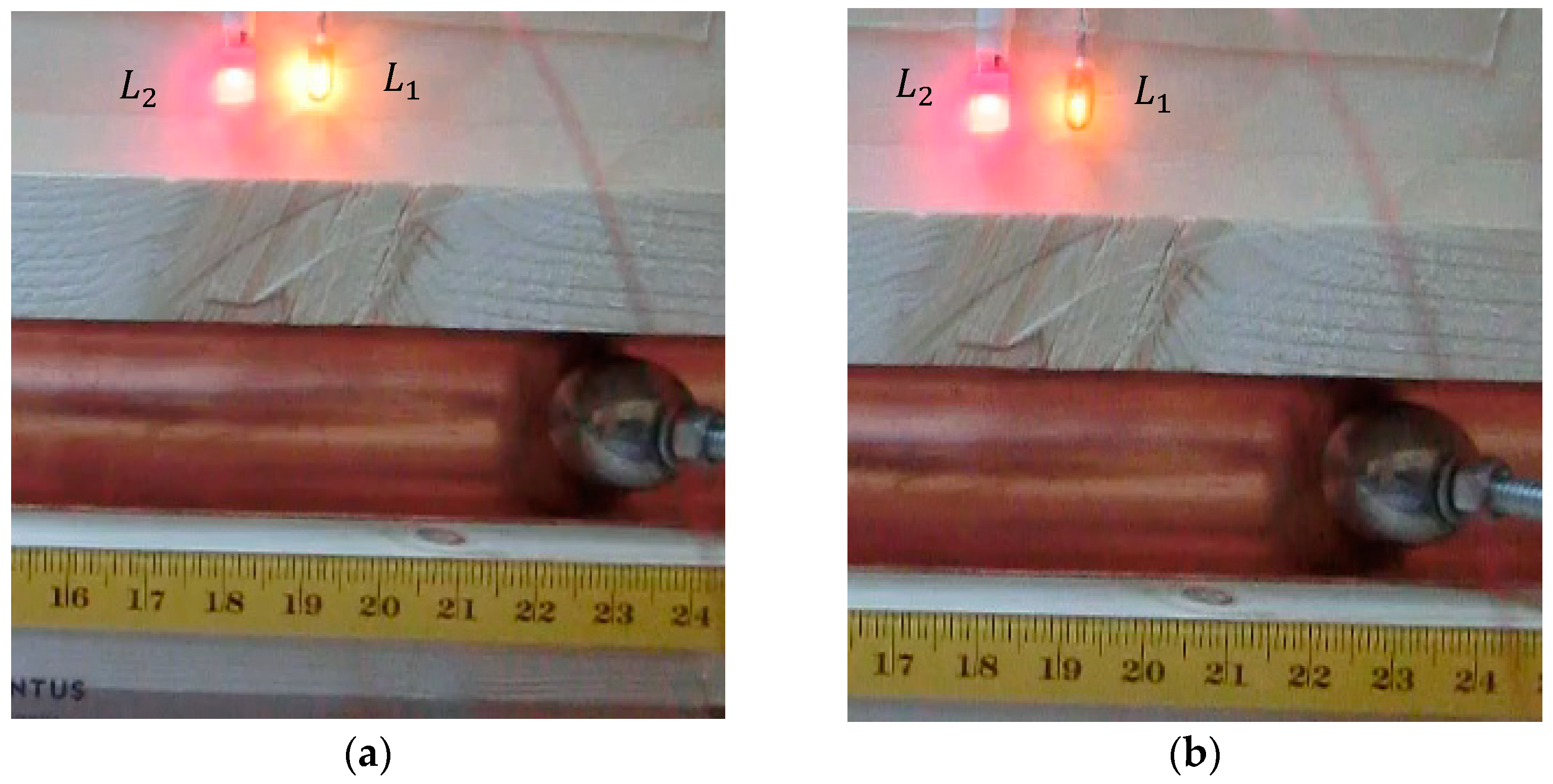
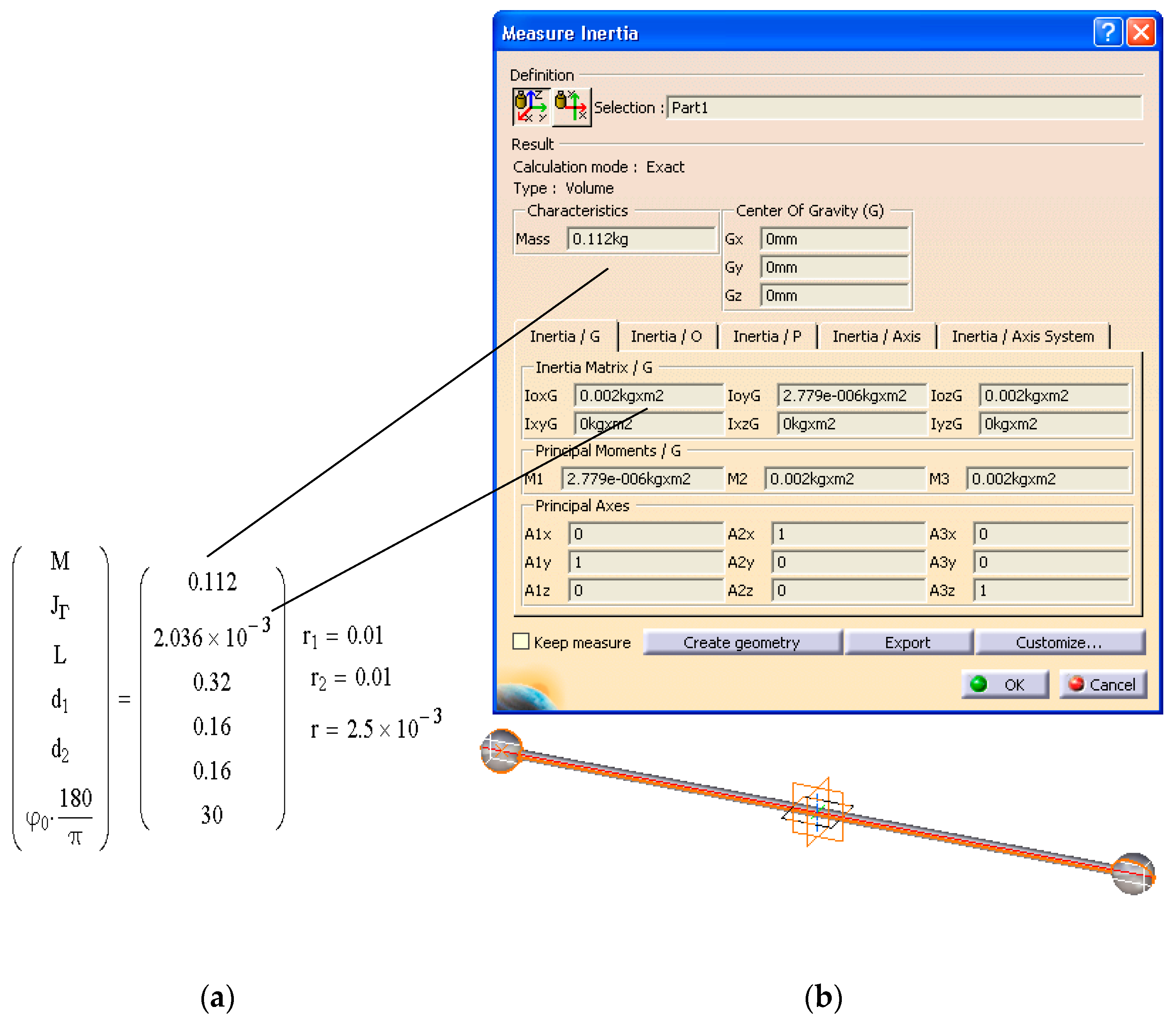
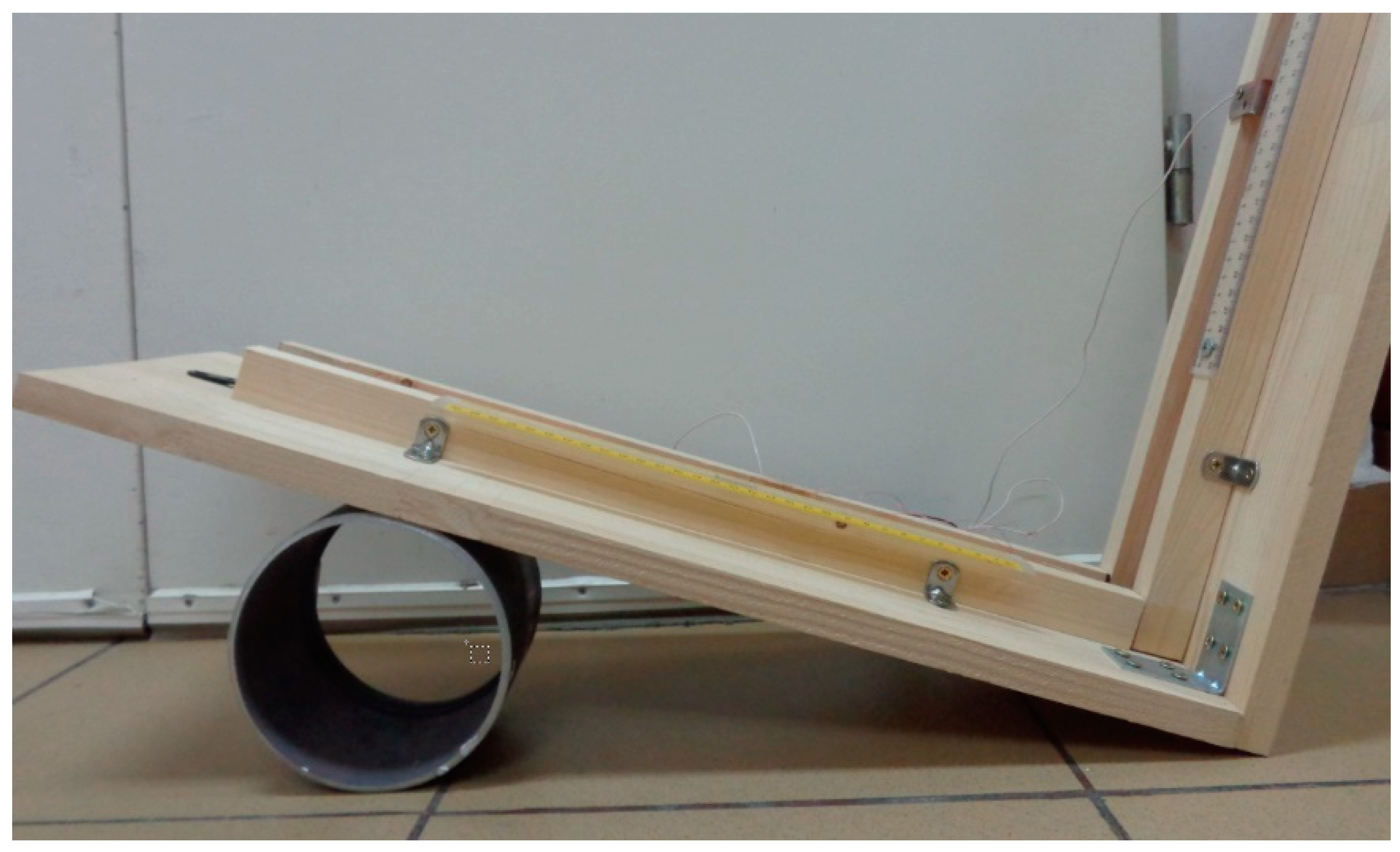
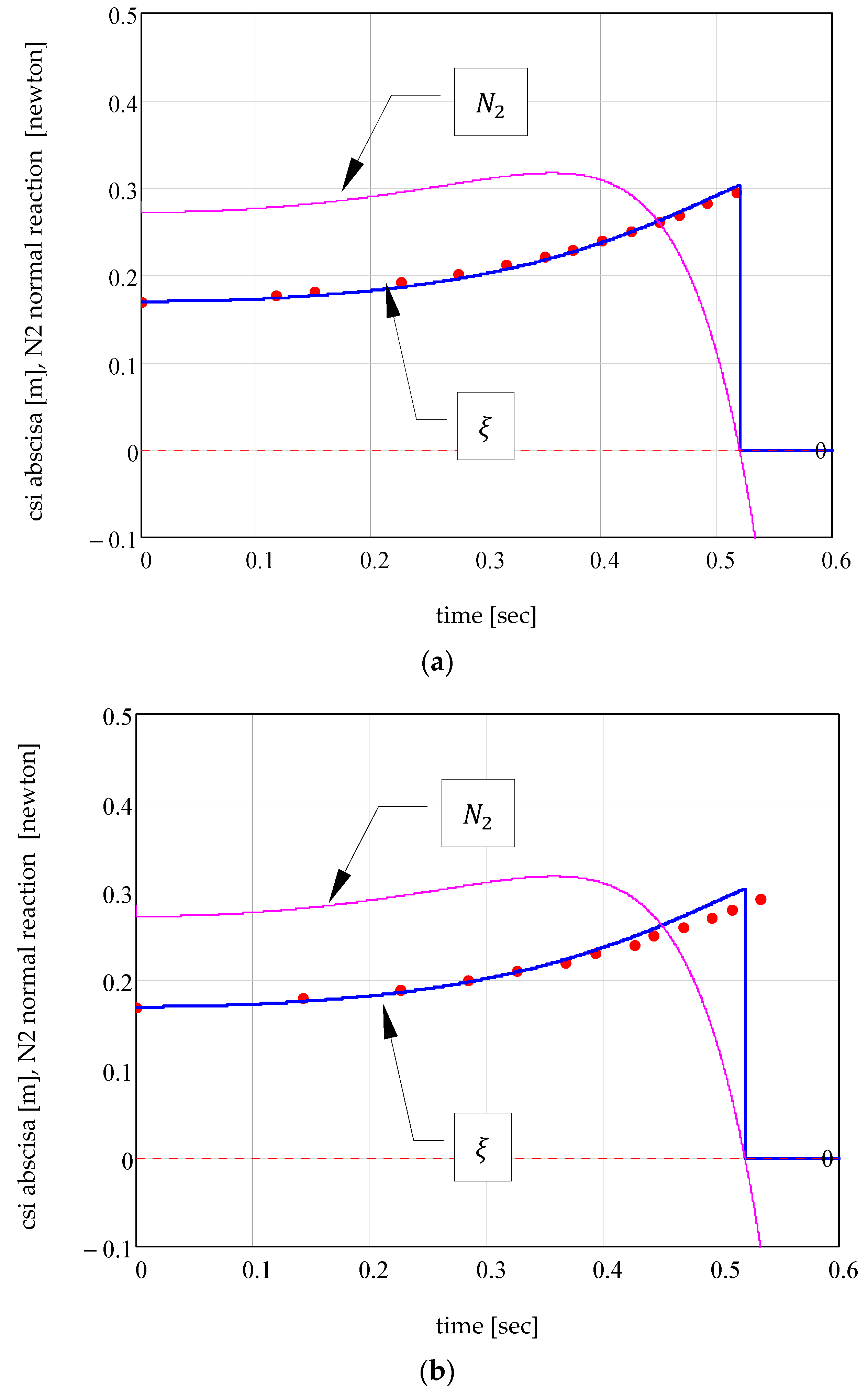
| Launching | Frame Number [−] | Displacement [mm] |
|---|---|---|
| set 1 | 3488 | 232 |
| 3502 | 225 | |
| 3506 | 220 | |
| 3515 | 210 | |
| 3521 | 200 | |
| 3526 | 190 | |
| 3530 | 180 | |
| 3533 | 173 | |
| 3536 | 162 | |
| 3539 | 152 | |
| 3542 | 141 | |
| 3544 | 133 | |
| 3547 | 120 | |
| 3550 | 107 | |
| set 2 | 4535 | 230 |
| 4552 | 220 | |
| 4562 | 210 | |
| 4569 | 200 | |
| 4574 | 190 | |
| 4579 | 180 | |
| 4582 | 170 | |
| 4586 | 160 | |
| 4588 | 150 | |
| 4591 | 140 | |
| 4594 | 130 | |
| 4596 | 120 | |
| 4599 | 108 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lupascu, C.; Alaci, S.; Ciornei, F.-C.; Romanu, I.-C.; Cerlinca, D.-A.; Bujoreanu, C. Aspects Concerning Validation of Theoretical Solution of Generalised Ladder Problem. Modelling 2025, 6, 28. https://doi.org/10.3390/modelling6020028
Lupascu C, Alaci S, Ciornei F-C, Romanu I-C, Cerlinca D-A, Bujoreanu C. Aspects Concerning Validation of Theoretical Solution of Generalised Ladder Problem. Modelling. 2025; 6(2):28. https://doi.org/10.3390/modelling6020028
Chicago/Turabian StyleLupascu, Costica, Stelian Alaci, Florina-Carmen Ciornei, Ionut-Cristian Romanu, Delia-Aurora Cerlinca, and Carmen Bujoreanu. 2025. "Aspects Concerning Validation of Theoretical Solution of Generalised Ladder Problem" Modelling 6, no. 2: 28. https://doi.org/10.3390/modelling6020028
APA StyleLupascu, C., Alaci, S., Ciornei, F.-C., Romanu, I.-C., Cerlinca, D.-A., & Bujoreanu, C. (2025). Aspects Concerning Validation of Theoretical Solution of Generalised Ladder Problem. Modelling, 6(2), 28. https://doi.org/10.3390/modelling6020028






