The Difference in the Effects of IR-Drop from the Negative Capacitance of Fast Cyclic Voltammograms
Abstract
:1. Introduction
2. Materials and Methods
2.1. Chemicals
2.2. Voltammetry
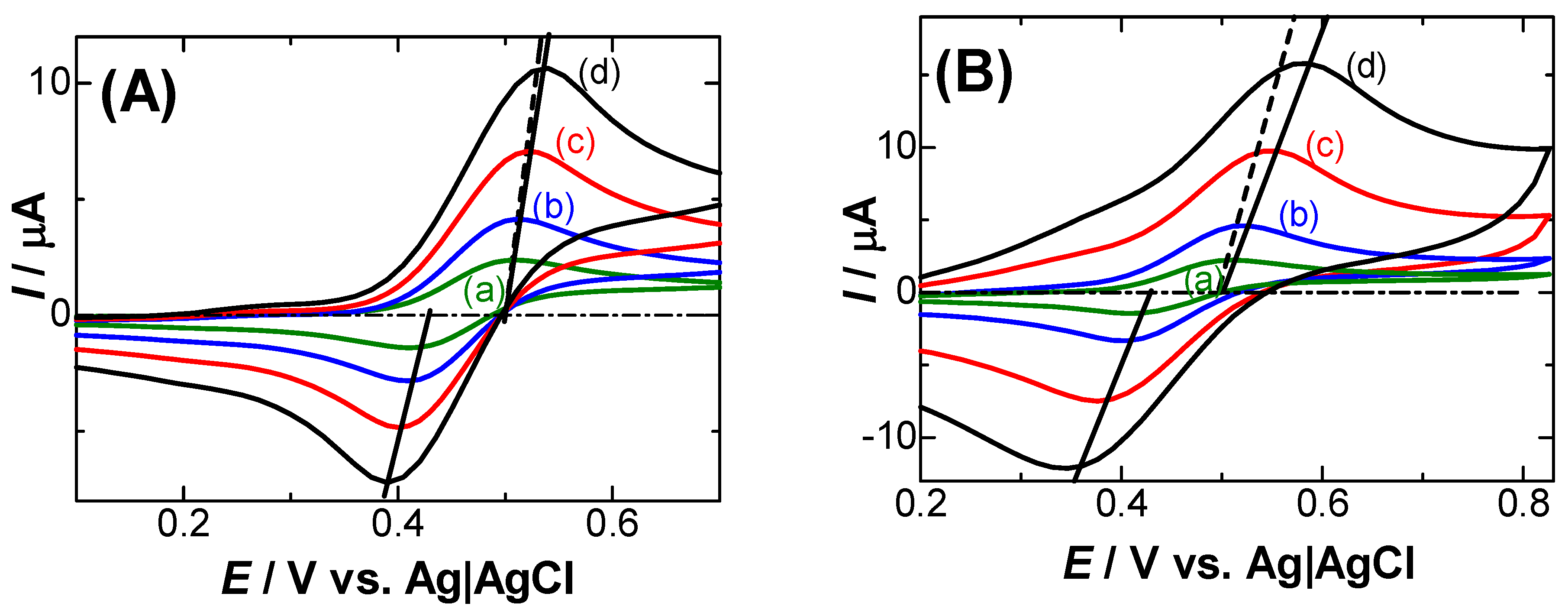
3. Results
- (i)
- The flux of a univalent cationic redox species controlled both by diffusion and electric migration is expressed by the Nernst–Planck equation, j/F = −Ddc/dx − (DF/RT)c(dϕ/dx) [1], where c is its concentration, D is the diffusion coefficient, and ϕ is the potential in the solution at a distance from the electrode of x. It can be approximated to be j/F = −Dc*/δ − (DF/RT)c*(Δϕ/δ), on the basis of the concept of a diffusion layer [33]. Here, its thickness at the peak current, Ip, is given by δ = Dc*FA/Ip, where A is the electrode area. The ratio of the current component of the migration to that of the diffusion is (F/RT)Δϕ. When Δϕ was replaced by RsIp, the ratio became less than 0.5% under our experimental conditions. Therefore, migration had no contribution to the potential shift in our case.
- (ii)
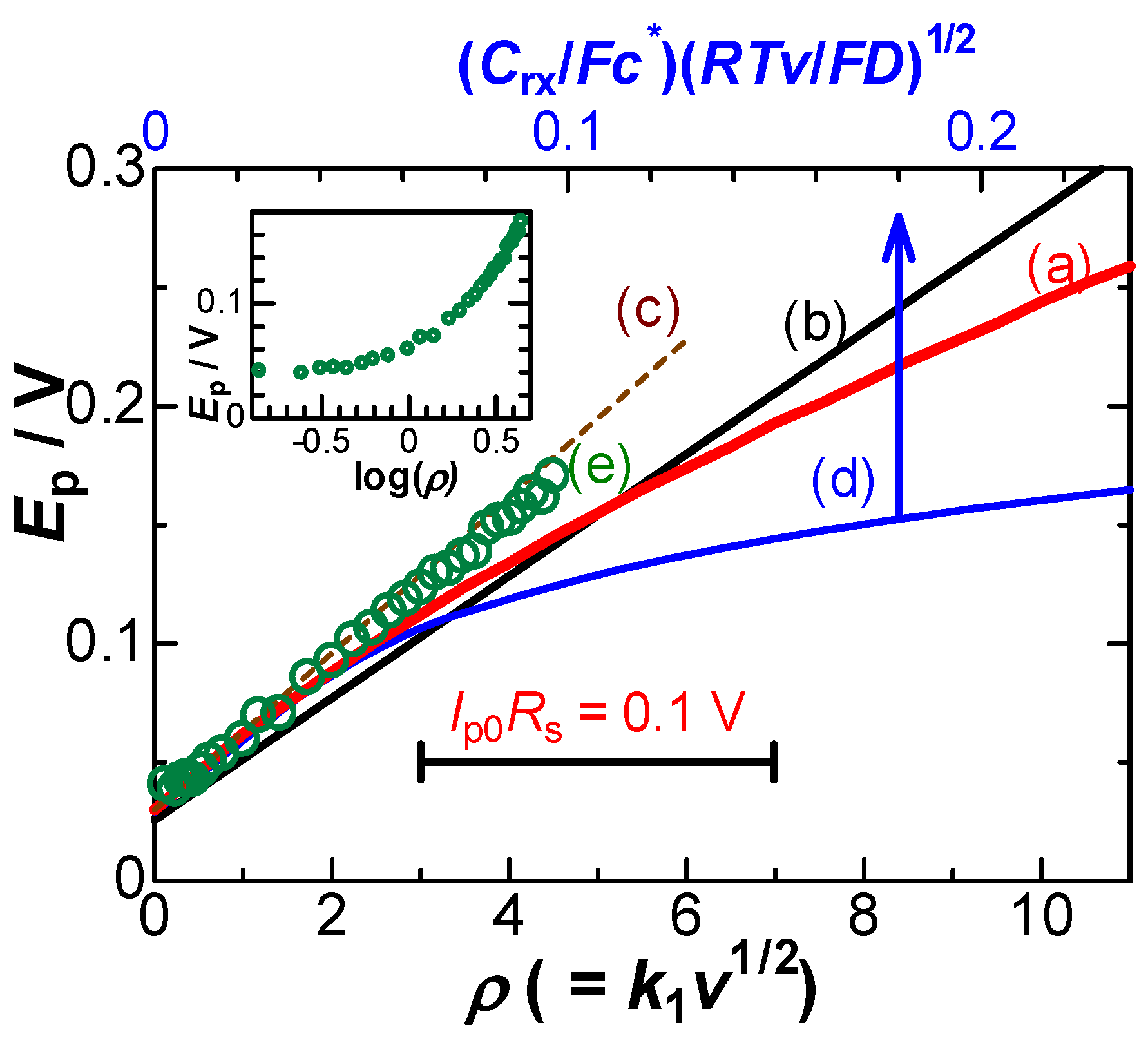
- Electrode kinetics often cause potential shifts. Quantitatively accessible kinetics are represented by the Butler–Volmer equation. The theoretical peak currents and potentials for different scan rates can be obtained from the analytical equation at a given transfer coefficient α and a heterogeneous rate constant k0 [17]. Figure 3(c–e) shows the variation in Ip with Ep for several scan rates at α = 0.5 and some values of k0 via the use of our software for the kinetics, so that Ip vs. Ep were close to the experimental ones (a). However, the experimental plots (a) were different from the theoretical variations for any different values of k0. The inconsistency was also applied to other values of Rs (b). Since no potential shift was found in the 0.1 M KCl solution (b), it is not reasonable to explain the shift in terms of the heterogeneous kinetics. However, an explanation only due to kinetics has often been reported [2,3,4,5,6,7,8,9].
- (iii)
- A following chemical reaction causes a potential shift, as can be understood from the Nernst equation for reaction rates faster than the voltammetric rates. Ferrocenyl compounds, however, are not satisfied with the condition of the rates; hence, item (iii) is unsuitable for explaining the present potential shifts.
- (iv)
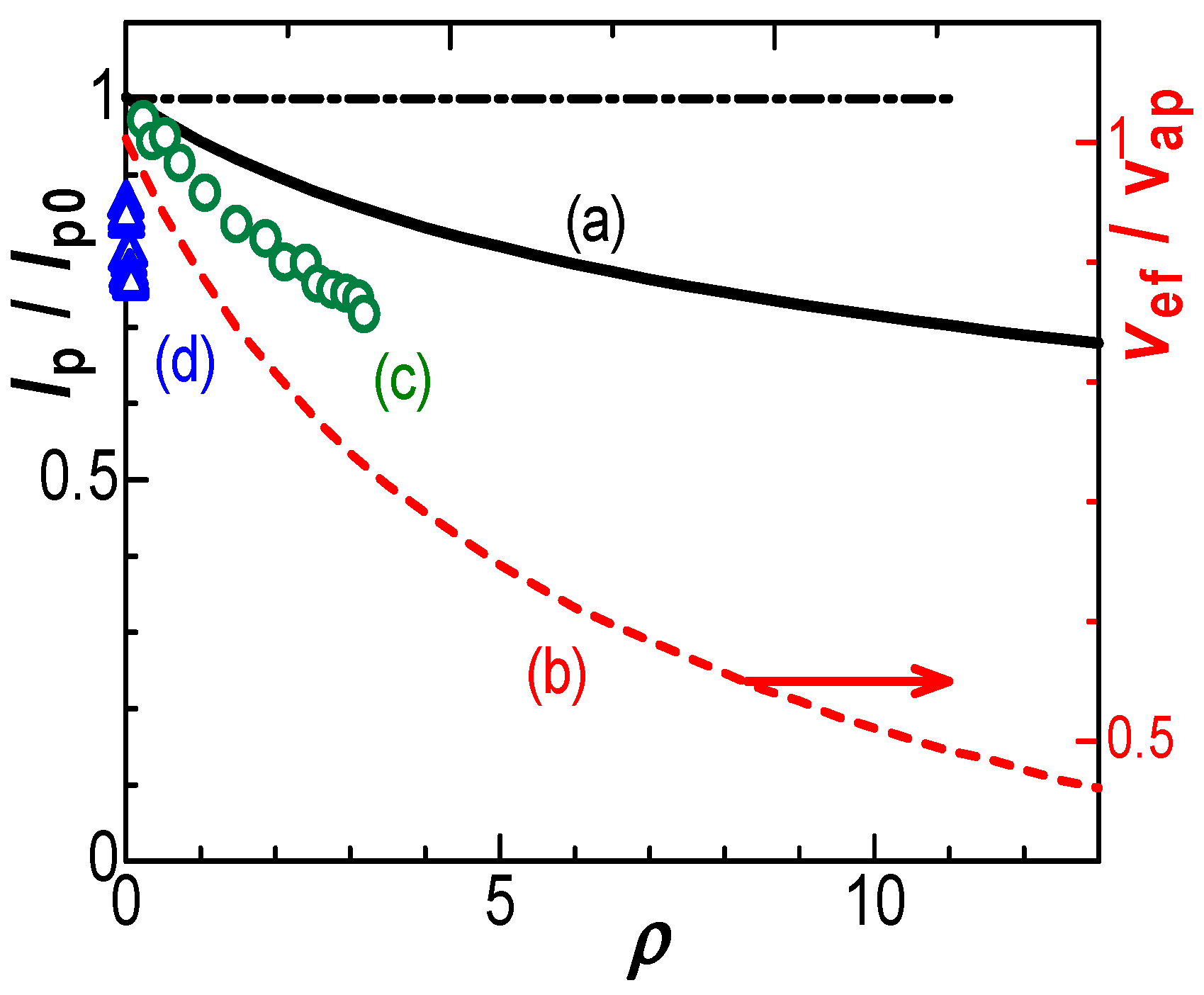
- The negative capacitance was brought about through the following steps according to Figure 4: a charge-transferred redox species (Fc) was coupled with a counterion (Cl−) for electric neutrality by responding to the externally applied field Eap to yield an electric dipole (Fc+-Cl−), where Cl− came from the supporting electrolyte because of the highest concentration of anions: the dipole with the dipole moment p was oriented in the direction for enhancing the external field by -pc/ε0 to yield the effective field Eef, which generated capacitance with a sign opposite to double-layer capacitance [16,34]. Negative capacitance has been obtained for ferrocenyl derivatives [18], ruthenium complex, iridium complex, and hemin [34] with ac-impedance. Since the voltammetric current from the negative capacitance was proportional to the scan rate, it depressed the diffusion tail to cause the potential shift [16]. The negative capacitance varied with electrode areas and scan rates, as the IR-drop did. The similarity in the properties stimulated us to distinguish their properties theoretically.



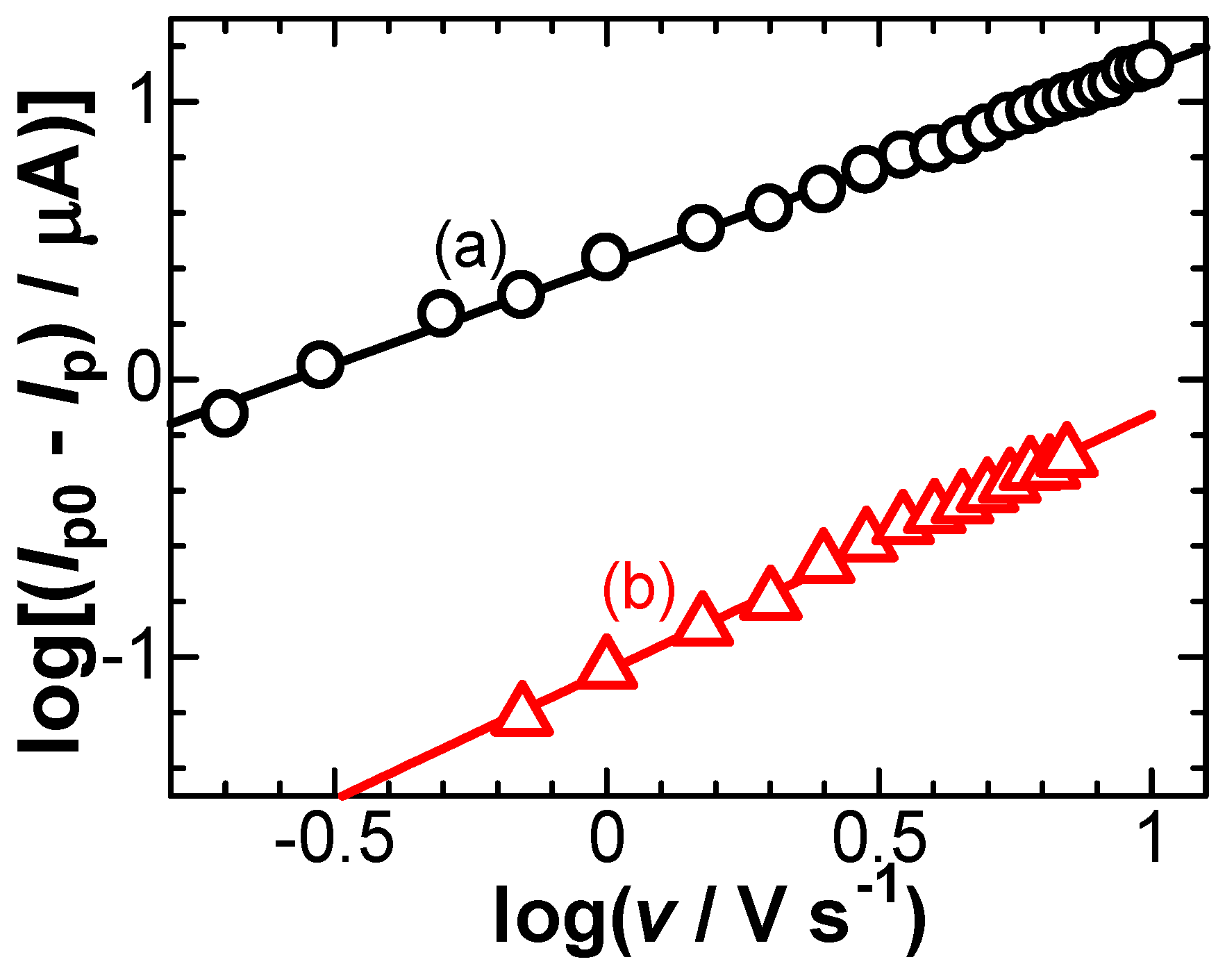
4. Theory of Effects of IR-Drop
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Bard, A.J.; Faulkner, L.R. Electrochemical Methods; Fundamentals and Applications, 2nd ed.; John Wiley & Sons: New York, NY, USA, 2001; pp. 29, 170–176, 331–332. [Google Scholar]
- Howell, J.O.; Goncalves, J.M.; Amatore, C.; Klasinc, L.; Wightman, R.M.; Kochi, J.K. Electron transfer from aromatic hydrocarbons and their.pi.-complexes with metals. Comparison of the standard oxidation potentials and vertical ionization potentials. J. Am. Chem. Sot. 1984, 106, 3968–3976. [Google Scholar] [CrossRef]
- Amatore, C.; Lefrou, C. Is cyclic voltammetry above a few hundred kilovolts per second still cyclic voltammetry? J. Electroanal. Chem. 1990, 296, 335–358. [Google Scholar] [CrossRef]
- Montenegro, M.I.; Pletcher, D. The determination of the kinetics of electron transfer using fast sweep cyclic voltammetry at microdisc electrodes. J. Electroanal. Chem. 1986, 200, 371–374. [Google Scholar] [CrossRef]
- Fitch, A.; Evans, D.H. Use of microelectrodes for the study of a fast chemical step in an electrode reaction. J. ElectroanaI. Chem. 1986, 202, 83–92. [Google Scholar] [CrossRef]
- Amatore, C.; Maisonhaute, E.; Simonneau, G. Ultrafast cyclic voltammetry: Performing in the few megavolts per second range without ohmic drop. Electrochem. Commun. 2000, 2, 81–84. [Google Scholar] [CrossRef]
- Andrieux, C.P.; Garreau, D.; Hapiot, P.; Pinson, J.; Saveant, J.M. Fast Sweep Cyclic Voltammetry at Ultra-Microelectrodes Evaluation of the Method for Fast Electron-Transfer Kinetic Measurements. J. Eleetroanal. Chem. 1988, 243, 321–335. [Google Scholar] [CrossRef]
- Wosiak, G.; Coelho, D.; Carneiro-Neto, E.B.; Pereira, E.C.; Lopes, M.C. Numerical Resolving of Net Faradaic Current in Fast-Scan Cyclic Voltammetry Considering Induced Charging Currents. Anal. Chem. 2020, 92, 15412–15419. [Google Scholar] [CrossRef]
- Amatore, C.; Oleinick, A.; Svir, I. Theoretical Analysis of Microscopic Ohmic Drop Effects on Steady-State and Transient Voltammetry at the Disk Microelectrode: A Quasi-Conformal Mapping Modeling and Simulation. Anal. Chem. 2008, 80, 7947–7956. [Google Scholar] [CrossRef]
- Roberts, J.G.; Sombers, L.A. Fast-Scan Cyclic Voltammetry: Chemical Sensing in the Brain and Beyond. Anal. Chem. 2018, 90, 490–504. [Google Scholar] [CrossRef]
- Baur, J.E.; Kristensen, E.W.; May, L.J.; Wiedemann, D.J.; Wightman, R.M. Fast-Scan Voltammetry of Biogenic Amines. Anal. Chem. 1988, 60, 1268–1272. [Google Scholar] [CrossRef]
- Venton, B.J.; Cao, Q. Fundamentals of fast-scan cyclic voltammetry for dopamine detection. Analyst 2020, 145, 1158–1168. [Google Scholar] [CrossRef]
- Puthongkham, P.; Venton, B.J. Recent advances in fast-scan cyclic voltammetry, doi.org/10.1039/C9AN01925A. Analyst 2020, 145, 1087–1102. [Google Scholar] [CrossRef]
- CV Sim: Simulation of Simple Redox Reaction (E) Part 2 Ohmic Drop Effect & Double Layer Capacitance–Application Note 41-2, pp. 1–4. Available online: https://www.biologic.net/wp-content/uploads/2019/08/cv-simulation_electrochemistry-an41-2.pdf (accessed on 5 November 2018).
- Aoki, K.J.; Chen, J. Tips of Voltammetry. pp. 1–19. Available online: https://www.intechopen.com/chapters/63917 (accessed on 5 November 2018).
- Aoki, K.J.; Chen, J.; Liu, Y.; Jia, B. Peak potential shift for fast cyclic voltammograms owing to capacitance of redox reactions. J. Electroanal. Chem. 2020, 856, 113609. [Google Scholar] [CrossRef]
- Matsuda, H.; Ayabe, Y.Z. The theory of the cathode-ray polarography of Randles-Sevcik. Elektrochmie 1955, 59, 494–503. [Google Scholar]
- Aoki, K.J.; Chen, J.; Zeng, X.; Wang, Z. Decrease in double layer capacitance by Faradaic current. RSC Adv. 2017, 7, 22501. [Google Scholar] [CrossRef]
- Wang, R.; Aoki, K.J.; Chen, J. Enhancement of the Negative Capacitance Associated with the Dissolution of Silver by Salt Concentrations by Means of Anodic Stripping Voltammetry. J. Electrochem. 2022, 3, 397–406. [Google Scholar] [CrossRef]
- Aoki, K.J.; Zhang, C.; Chen, J.; Nishiumi, T. Heterogeneous reaction rate constants by steady-state microelectrode techniques and fast scan voltammetry. J. Electroanal. Chem. 2013, 706, 40–47. [Google Scholar] [CrossRef]
- Sun, P.; Mirkin, M.V. Kinetics of electron-transfer reactions at nanoelectrodes. Anal. Chem. 2006, 78, 6526–6534. [Google Scholar] [CrossRef]
- Velmurugan, J.; Sun, P.; Mirkin, M.V. Scanning Electrochemical Microscopy with Gold Nanotips: The Effect of Electrode Material on Electron Transfer Rates. J. Phys. Chem. C 2009, 113, 459–464. [Google Scholar] [CrossRef]
- Smalley, J.F.; Feldberg, S.W.; Chidsey, C.E.D.; Linford, M.R.; Newton, M.D.; Liu, Y.-P. The Kinetics of Electron Transfer Through Ferrocene-Terminated Alkanethiol Monolayers on Gold. J. Phys. Chem. 1995, 99, 13141–13149. [Google Scholar] [CrossRef]
- Nioradze, N.; Kim, J.; Amemiya, S. Quasi-Steady-State Voltammetry of Rapid Electron Transfer Reactions at the Macroscopic Substrate of the Scanning Electrochemical Microscope. Anal. Chem. 2011, 83, 828–835. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Bergman, D.; Zhang, B. Preparation and Electrochemical Response of 1−3 nm Pt Disk Electrodes. Anal. Chem. 2009, 81, 5496–5502. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Zhou, J.; Lin, L.; Lin, Z. Determination of Electrochemical Electron-Transfer Reaction Standard Rate Constants at Nanoelectrodes: Standard Rate Constants for Ferrocenylmethyltrimethylammonium(III)/(II) and Hexacyanoferrate(III)/(II). Electroanal 2008, 20, 1490–1494. [Google Scholar] [CrossRef]
- Atkins, P.; Paula, J.D. Atkin’s Physical Chemistry, 10th ed.; Oxford University Press: Oxford, UK, 2014; pp. 799–802. [Google Scholar]
- Zhou, Y.; Yang, P.; Yuan, C.; Huo, Y. Electrochemical migration failure of the copper trace on printed circuit board driven by immersion silver finish. Chem. Eng. Trans. 2013, 33, 559–564. [Google Scholar]
- Sorbello, R.S. Microscopic driving forces for electromigration. Mater. Res. Soc. Conf. Proc. 1996, 427, 73–81. [Google Scholar] [CrossRef]
- Putra, B.R.; Aoki, K.J.; Chen, J.; Marken, F. A cationic rectifier based on a graphene oxide covered microhole: Theory and experiment. Langmuir 2019, 35, 2055–2065. [Google Scholar] [CrossRef]
- Song, P.; Fisher, A.C.; Wadhawan, J.D.; Cooper, J.J.; Ward, H.J.; Lawrence, N.S. A mechanistic study of the EC’ mechanism–the split wave in cyclic voltammetry and square wave voltammetry. RSC Adv. 2016, 6, 70237–70242. [Google Scholar] [CrossRef]
- Nassef, H.M.; Radi, A.-E.; O’Sullivan, C.K. Electrocatalytic sensing of NADH on a glassy carbon electrode modified with electrografted o-aminophenol film. Electrochem. Commun. 2006, 8, 1719–1725. [Google Scholar]
- Molina, A.; Gonzalez, J.; Laborda, E.; Compton, R.G. On the meaning of the diffusion layer thickness for slow electrode reactions. Phys. Chem. Chem. Phys. 2013, 15, 2381–2388. [Google Scholar] [CrossRef]
- Aoki, K.J.; Taniguchi, S.; Chen, J. Participation in negative capacitance of diffusion-controlled voltammograms of hemin. Omega ACS 2020, 5, 29447–29452. [Google Scholar] [CrossRef]
- Aoki, K. Is voltammetric current proportional to the number of transferring electrons of multi-charged ions or 3/2 powers of the number? Electroanalysis 2005, 17, 1379–1383. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions; U.S. Government Printing Office: Washington, DC, USA, 1964; p. 11. Available online: https://personal.math.ubc.ca/~cbm/aands/frameindex.htm (accessed on 5 November 2018).
- Newman, J.S. Electrochemical Systems; Prentice-Hall Inc.: Englewood Cliff, NJ, USA, 1991; Chapter 18; pp. 340–345. [Google Scholar]


| Range of v | Advantages | Risks |
|---|---|---|
| (I) <0.2 V s−1 | Small background currents Easy extraction of diffusion currents Usage of low-cost potentiostats Possibility of theoretical analysis | Misleading kinetic reaction mechanisms Time consumption |
| (II) <5 V s−1 | Possibility of subtraction of IR-drop Possibility of determining reaction mechanisms Evaluation of heterogeneous kinetics Comparison of the results with those by other rapid electrochemical methods Commercially available potentiostats | Discussion required for peak shifts Deformation of waveform Limited to microelectrodes in order to prevent large currents |
| (III) <500 V s−1 | Detection of kinetics with milli-second orders such as neurotransmitters | Empirical search for detecting conditions A loss of theoretical support |
| Variables | Ep | Ip0−Ip | ||
|---|---|---|---|---|
| IR | NC | IR | NC | |
| c* | c* | 1 | c*3/2 | c* |
| A(disk) | A1/2 | 1 | A5/4 | A |
| v | v1/2 | v1/2 | v3/4 | v |
| Rs | Rs | 1 | Rs1/2 | 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Aoki, K.J.; Chen, J. The Difference in the Effects of IR-Drop from the Negative Capacitance of Fast Cyclic Voltammograms. Electrochem 2023, 4, 460-472. https://doi.org/10.3390/electrochem4040030
Liu Y, Aoki KJ, Chen J. The Difference in the Effects of IR-Drop from the Negative Capacitance of Fast Cyclic Voltammograms. Electrochem. 2023; 4(4):460-472. https://doi.org/10.3390/electrochem4040030
Chicago/Turabian StyleLiu, Yuanyuan, Koichi Jeremiah Aoki, and Jingyuan Chen. 2023. "The Difference in the Effects of IR-Drop from the Negative Capacitance of Fast Cyclic Voltammograms" Electrochem 4, no. 4: 460-472. https://doi.org/10.3390/electrochem4040030
APA StyleLiu, Y., Aoki, K. J., & Chen, J. (2023). The Difference in the Effects of IR-Drop from the Negative Capacitance of Fast Cyclic Voltammograms. Electrochem, 4(4), 460-472. https://doi.org/10.3390/electrochem4040030








