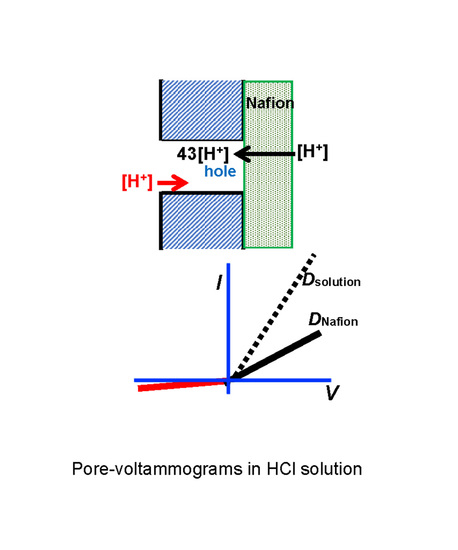
Electric Migration of Hydrogen Ion in Pore-Voltammetry Suppressed by Nafion Film
Abstract
1. Introduction
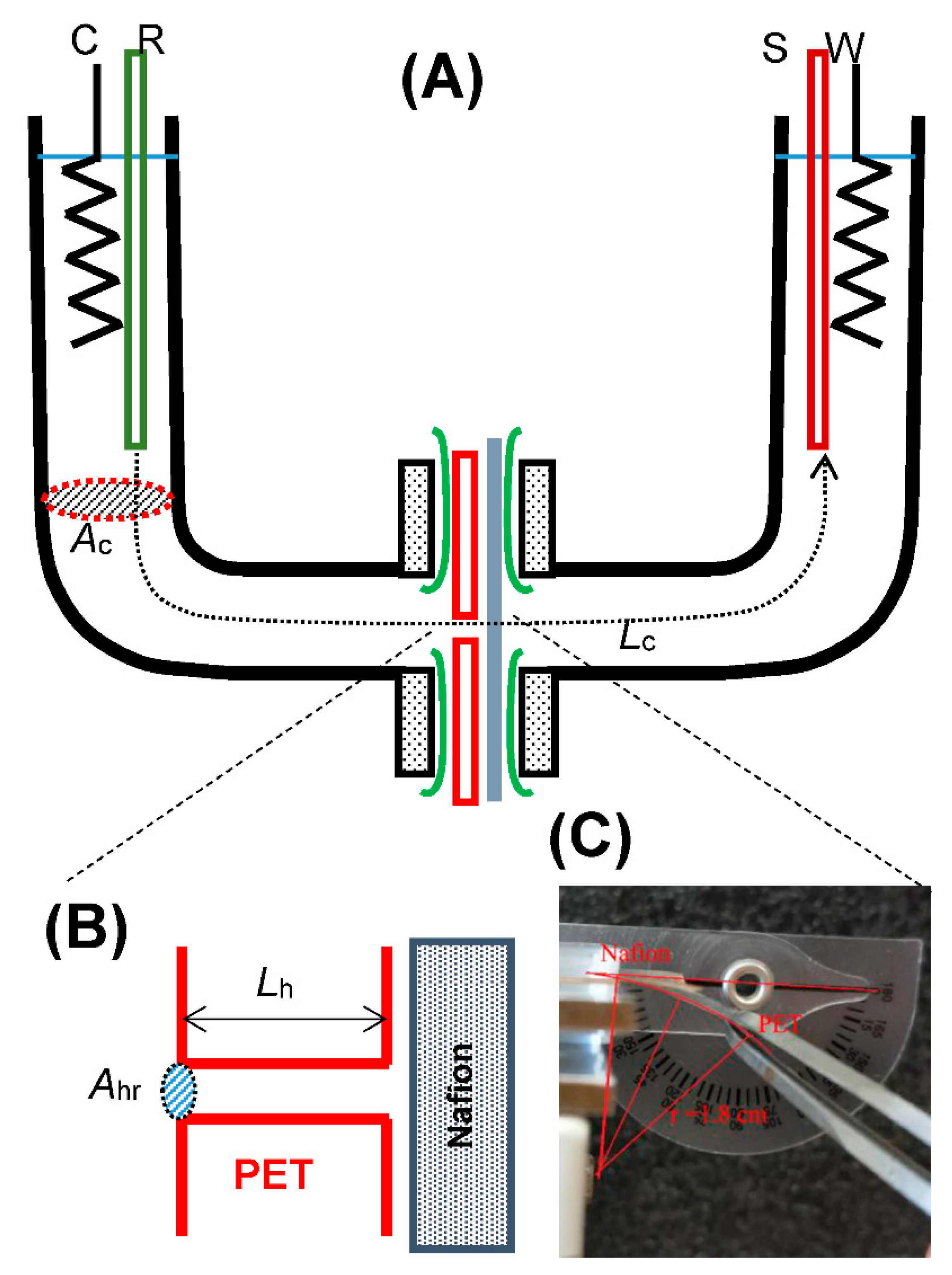
2. Materials and Methods
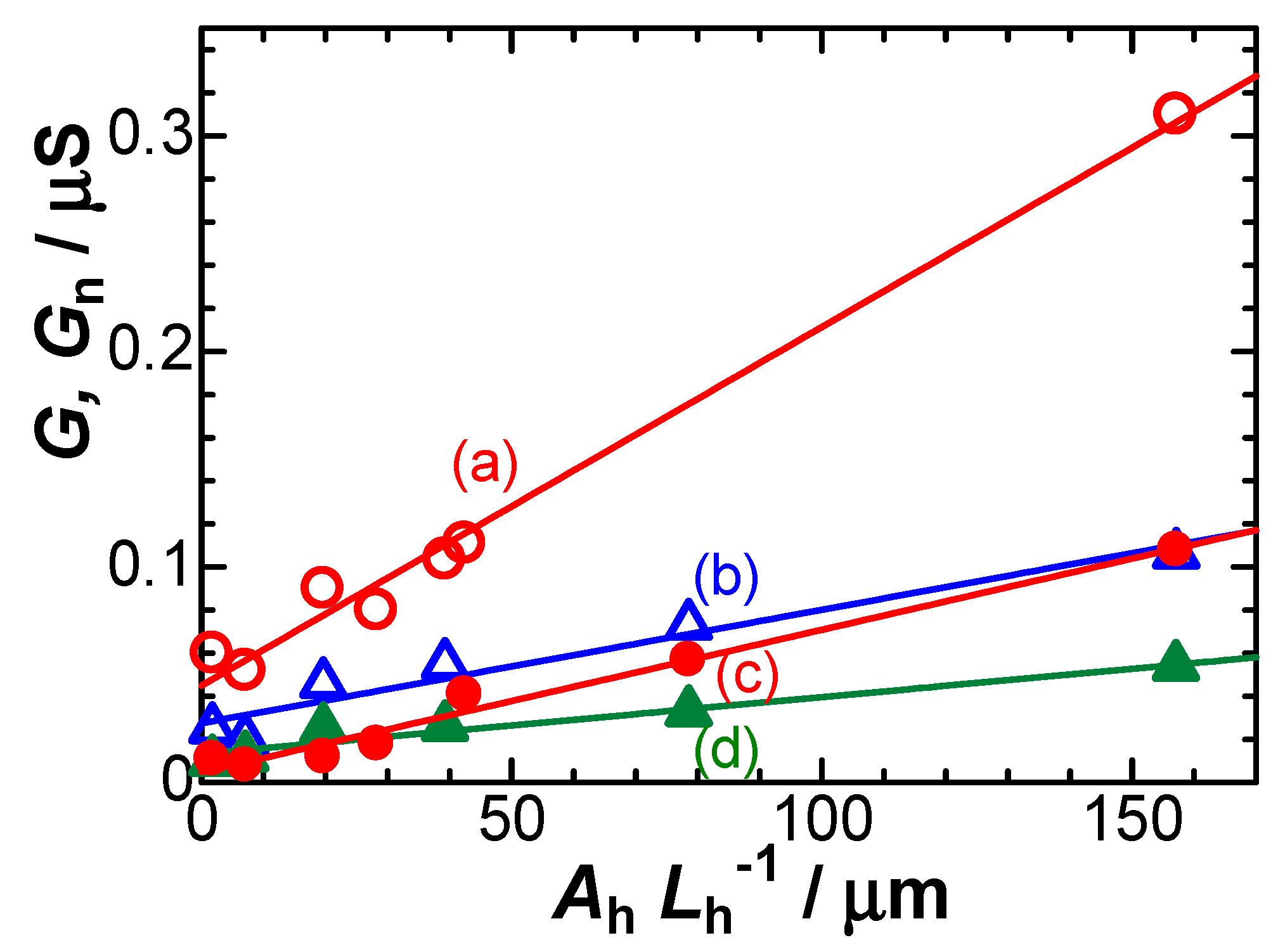
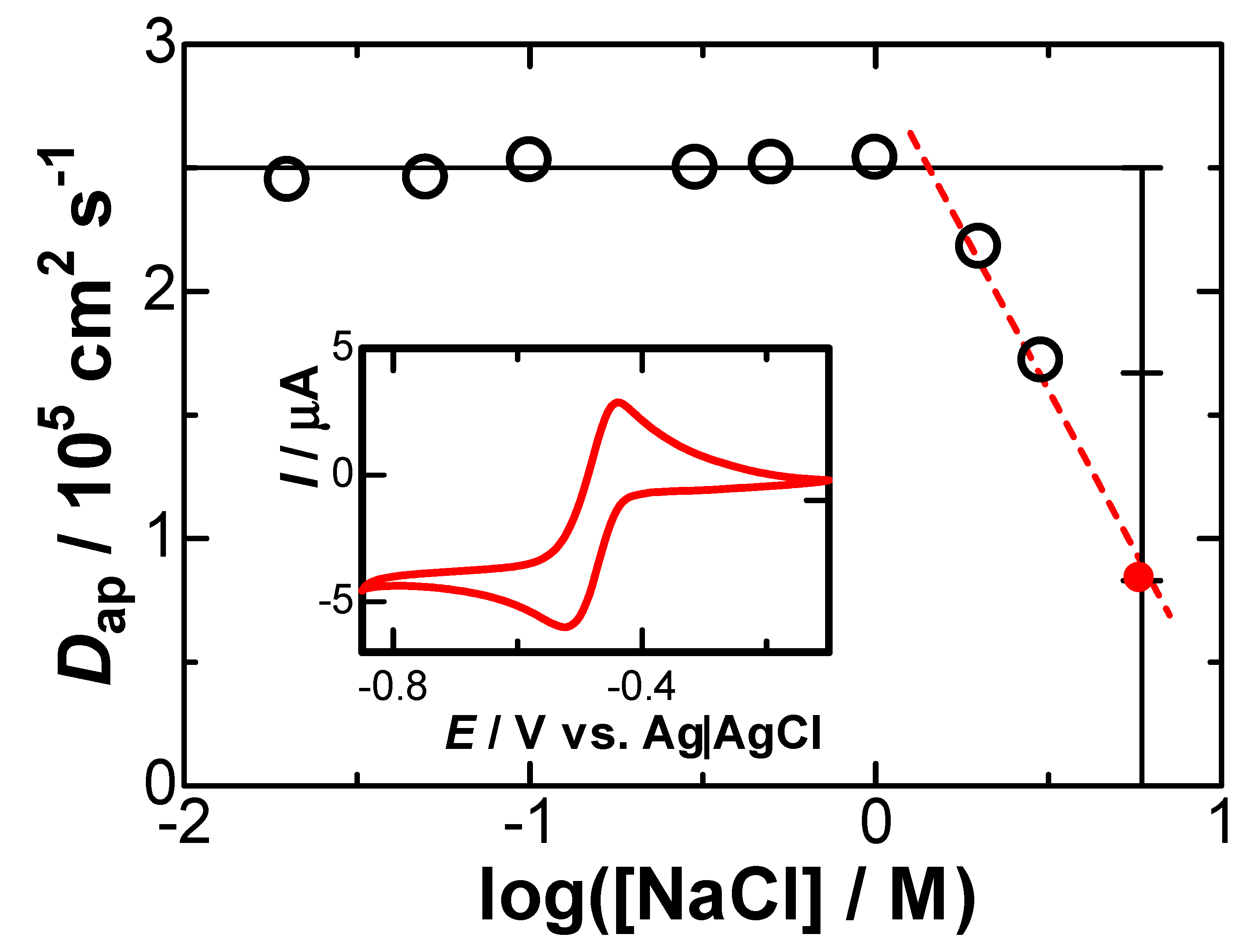
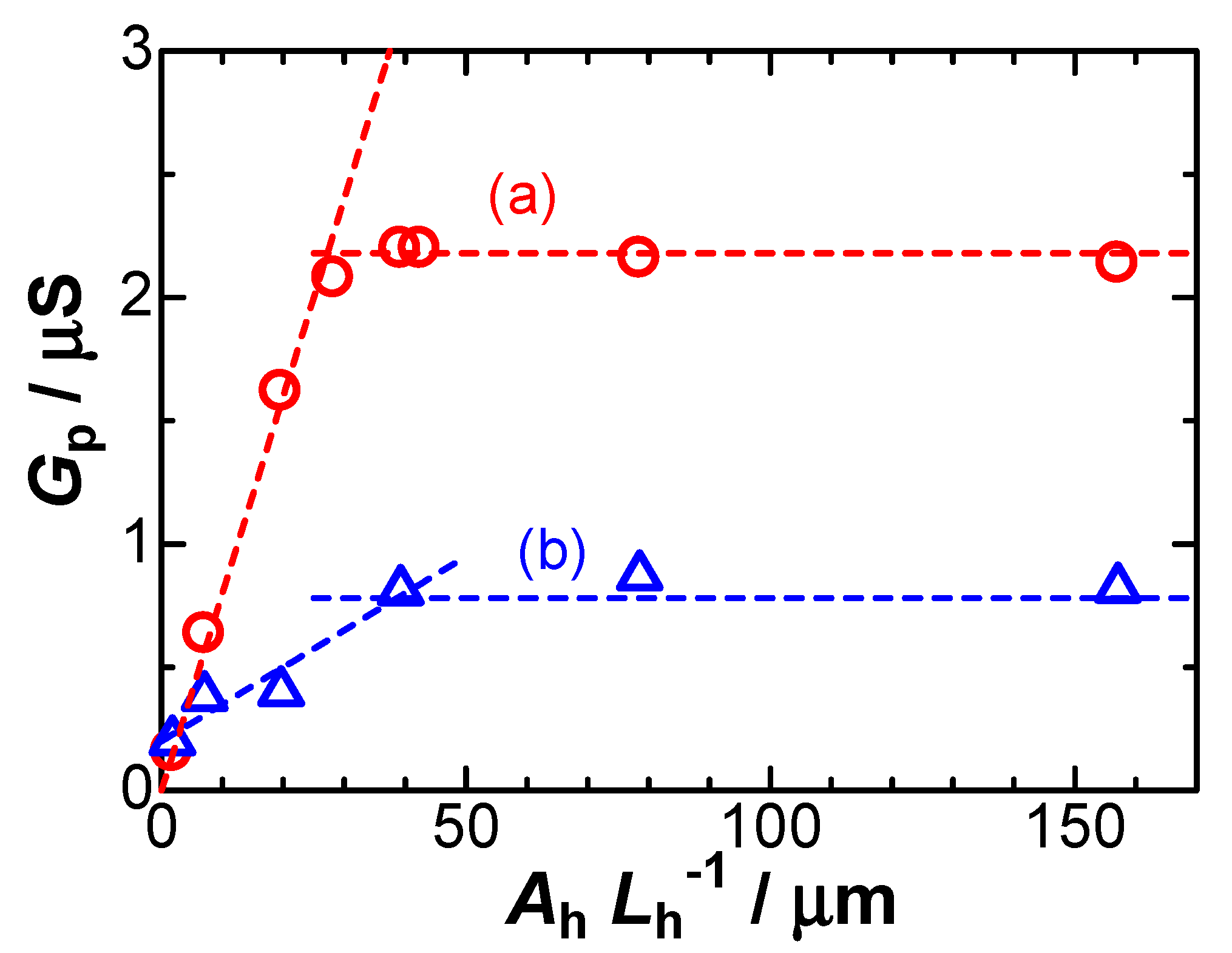
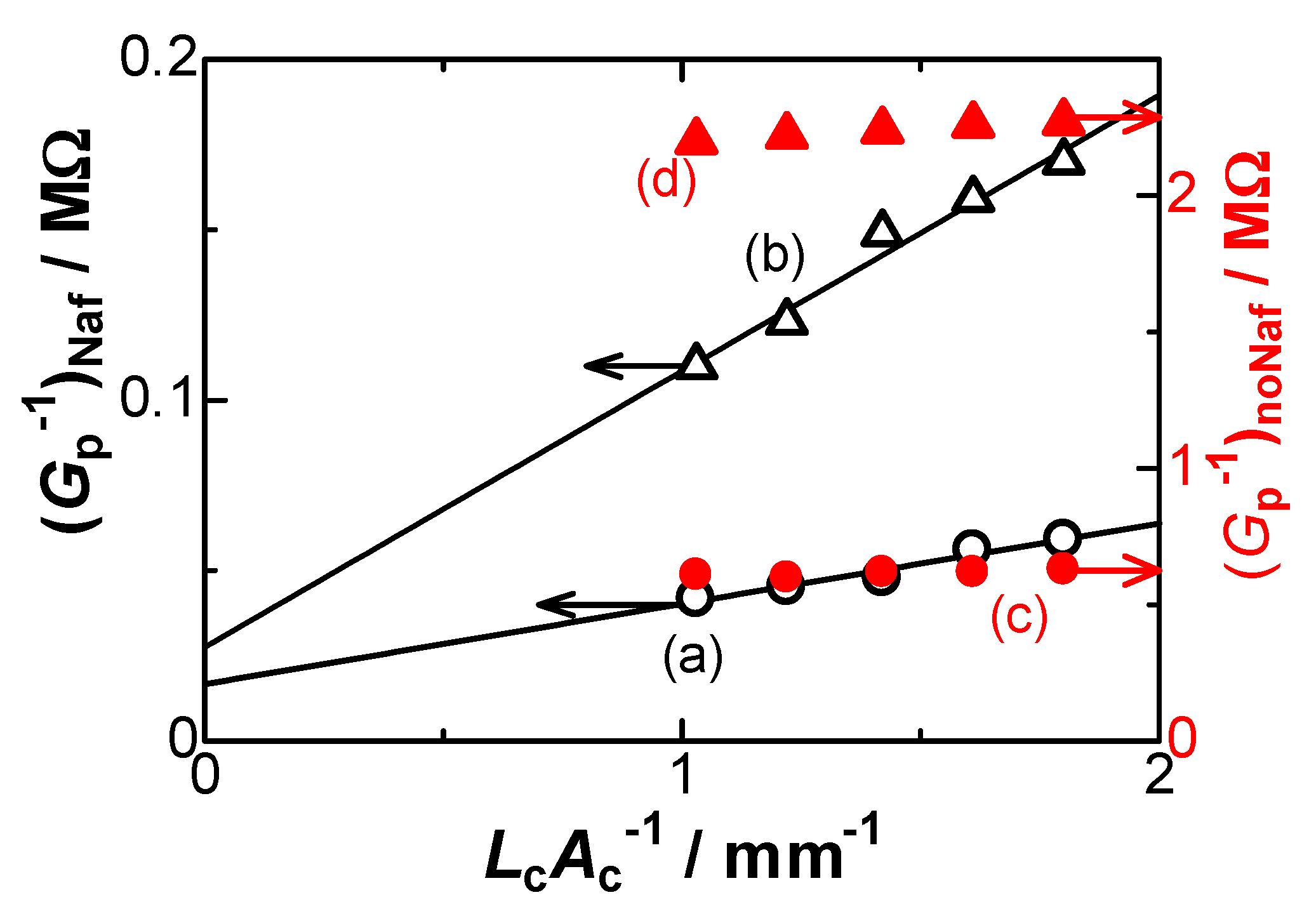
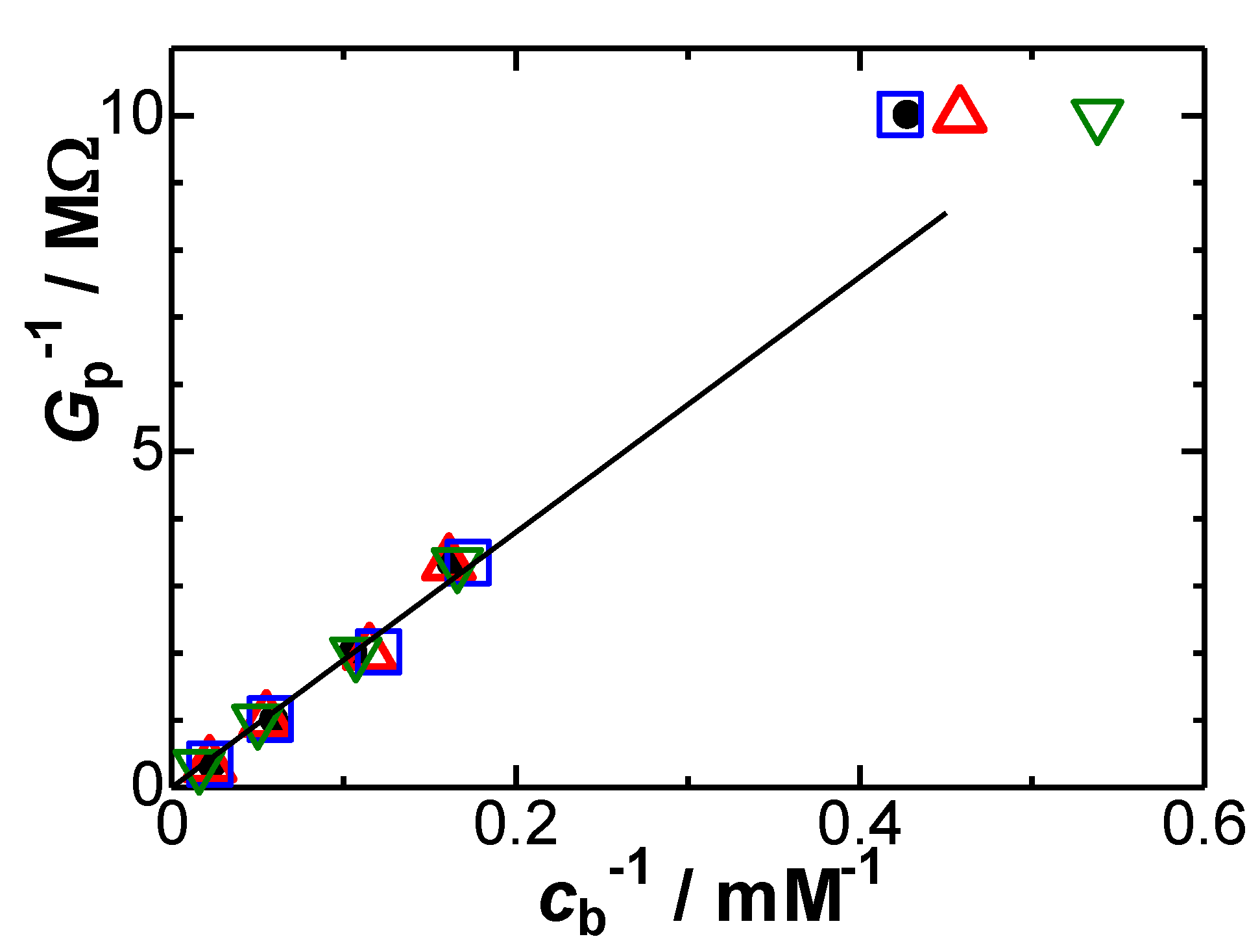
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zhang, H.C.; Tian, Y.; Jiang, L. Fundamental studies and practical applications of bio-inspired smart solid-state nanopores and nanochannels. Nano Today 2016, 11, 61–81. [Google Scholar] [CrossRef]
- Slouka, Z.; Senapati, S.; Chang, H.C. Microfluidic systems with ion-selective membranes. Annu. Rev. Anal. Chem. 2014, 7, 317–335. [Google Scholar] [CrossRef]
- Miles, B.N.; Ivanov, A.P.; Wilson, K.A.; Doğan, F.; Japrung, D.; Edel, J.B. Single molecule sensing with solid-state nanopores: Novel materials, methods, and applications. Chem. Soc. Rev. 2013, 42, 15–28. [Google Scholar] [CrossRef] [PubMed]
- Koo, H.J.; Velev, O.D. Ionic current devices-Recent progress in the merging of electronic, microfluidic, and biomimetic structures. Biomicrofluidics 2013, 7, 031501. [Google Scholar] [CrossRef] [PubMed]
- Wang, D.; Wang, G. Dynamics of ion transport and electric double layer in single conical nanopores. J. Electroanal. Chem. 2016, 779, 39–46. [Google Scholar] [CrossRef]
- Rong, Y.; Song, Q.; Mathwig, K.; Madrid, E.; He, D.; Niemann, R.G.; Cameron, P.J.; Dale, S.E.; Bending, S.; Carta, M.; et al. pH-induced reversal of ionic diode polarity in 300 nm thin membranes based on a polymer of intrinsic microporosity. Electrochem. Commun. 2016, 69, 41–45. [Google Scholar] [CrossRef]
- Guo, W.; Tian, Y.; Jiang, L. Asymmetric ion transport through ion-channel-mimetic solid-state nanopores. Acc. Chem. Res. 2013, 46, 2834–2846. [Google Scholar] [CrossRef]
- Madrid, E.; Cottis, P.; Rong, Y.; Rogers, A.T.; Stone, J.M.; Malpass-Evans, R.; Carta, M.; McKeown, N.B.; Marken, F. Water desalination concept using an ionic rectifier based on a polymer of intrinsic microporosity (PIM). J. Mater. Chem. A 2015, 3, 15849–15853. [Google Scholar] [CrossRef]
- Gao, J.; Guo, W.; Feng, D.; Wang, H.; Zhao, D.; Jiang, L. High-performance ionic diode membrane for salinity gradient power generation. J. Am. Chem. Soc. 2014, 136, 12265–12272. [Google Scholar] [CrossRef] [PubMed]
- Zhu, X.; Hao, J.; Bao, B.; Zhou, Y.; Zhang, H.; Pang, J.; Jiang, Z.; Jiang, L. Unique ion rectification in hypersaline environment: A high-performance and sustainable power generator system. Sci. Adv. 2018, 4, eaau1665. [Google Scholar] [CrossRef]
- Han, J.; Bae, C.; Chae, S.; Choi, D.; Lee, S.; Nam, Y.; Lee, C. High-efficiency power generation in hyper-saline environment using conventional nanoporous membrane. Electrochim. Acta 2019, 319, 366–374. [Google Scholar] [CrossRef]
- Putra, B.R.; Madrid, E.; Tshwenya, L.; Arotiba, O.A.; Marken, F. An AC-driven desalination/salination system based on a Nafion cationic rectifier. Desalination 2020, 480, 114351. [Google Scholar] [CrossRef]
- Jia, Z.; Wang, B.; Song, S.; Fan, Y. Blue energy: Current technologies for sustainable power generation from water salinity gradient. Renew. Sustain. Energy Rev. 2014, 31, 91–100. [Google Scholar] [CrossRef]
- Liu, Y.; Yobas, L. Cylindrical glass nanocapillaries patterned via coarse lithography (>1 μm) for biomicrofluidic applications. Biomicrofluidics 2012, 6, 046502. [Google Scholar] [CrossRef]
- Cheng, L.J.; Guo, L.J. Nanofluidic diodes. Chem. Soc. Rev. 2010, 39, 923–938. [Google Scholar] [CrossRef]
- Bearden, S.; Simpanen, E.; Zhang, G. Active current gating in electrically biased conical nanopores. Nanotechnology 2015, 26, 185502. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, H.; Yang, Z.; Zhou, J.; Wen, L.; Li, L.; Jiang, L. Fabrication of hydrogel-coated single conical nanochannels exhibiting controllable ion rectification characteristics. Phys. Chem. Chem. Phys. 2015, 17, 6367–6373. [Google Scholar] [CrossRef] [PubMed]
- McKeown, N.B.; Budd, P.M. Exploitation of intrinsic microporosity in polymer-based materials. Macromolecules 2010, 43, 5163–5176. [Google Scholar] [CrossRef]
- Madrid, E.; Rong, Y.; Carta, M.; McKeown, N.B.; Malpass-Evans, R.; Attard, G.A.; Clarke, T.J.; Taylor, S.H.; Long, Y.T.; Marken, F. Metastable Ionic Diodes Derived from an Amine-Based Polymer of Intrinsic Microporosity. Angew. Chem. 2014, 126, 10927–10930. [Google Scholar] [CrossRef]
- Madrid, E.; Buckingham, M.A.; Stone, J.M.; Rogers, A.T.; Gee, W.J.; Burrows, A.D.; Raithby, P.R.; Celorrio, V.; Fermin, D.J.; Marken, F. Ion flow in a zeolitic imidazolate framework results in ionic diode phenomena. Chem. Commun. 2016, 52, 2792–2794. [Google Scholar] [CrossRef]
- Li, T.; He, X.; Yu, P.; Mao, L. Nonlinear dependence of the ion current rectification factor on bias voltage in conical nanopipettes. J. Electroanal. Chem. 2016, 779, 106–111. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, Z.; Su, Z.; Cai, S. Stretchable and transparent ionic diode and logic gates. Extrem. Mech. Lett. 2019, 28, 81–86. [Google Scholar] [CrossRef]
- Tshwenya, L.; Arotiba, O.; Putra, B.R.; Madrid, E.; Mathwig, K.; Marken, F. Cationic diodes by hot-pressing of Fumasep FKS-30 ionomer film onto a microhole in polyethylene terephthalate (PET). J. Electroanal. Chem. 2018, 815, 114–122. [Google Scholar] [CrossRef]
- Xiao, K.; Xie, G.; Zhang, Z.; Kong, X.Y.; Liu, Q.; Li, P.; Wen, L.; Jiang, L. Enhanced Stability and Controllability of an Ionic Diode Based on Funnel-Shaped Nanochannels with an Extended Critical Region. Adv. Mater. 2016, 28, 3345–3350. [Google Scholar] [CrossRef]
- Wei, C.; Bard, A.J.; Feldberg, S.W. Current rectification at quartz nanopipet electrodes. Anal. Chem. 1997, 69, 4627–4633. [Google Scholar] [CrossRef]
- Hu, K.; Wang, Y.; Cai, H.; Mirkin, M.V.; Gao, Y.; Friedman, G.; Gogotsi, Y. Open carbon nanopipettes as resistive-pulse sensors, rectification sensors, and electrochemical nanoprobes. Anal. Chem. 2014, 86, 8897–8901. [Google Scholar] [CrossRef]
- Yan, F.; Yao, L.; Yang, Q.; Chen, K.; Su, B. Ionic current rectification by laminated bipolar silica isoporous membrane. Anal. Chem. 2018, 91, 1227–1231. [Google Scholar] [CrossRef]
- Yin, X.; Zhang, S.; Dong, Y.; Liu, S.; Gu, J.; Chen, Y.; Zhang, X.; Zhang, X.; Shao, Y. Ionic current rectification in organic solutions with quartz nanopipettes. Anal. Chem. 2015, 87, 9070–9077. [Google Scholar] [CrossRef] [PubMed]
- Mathwig, K.; Aaronson, B.D.; Marken, F. Ionic transport in microhole fluidic diodes based on asymmetric ionomer film deposits. ChemElectroChem 2018, 5, 897–901. [Google Scholar] [CrossRef]
- He, D.; Madrid, E.; Aaronson, B.D.; Fan, L.; Doughty, J.; Mathwig, K.; Bond, A.M.; McKeown, N.B.; Marken, F. A cationic diode based on asymmetric Nafion film deposits. ACS Appl. Mater. Interfaces 2017, 9, 11272–11278. [Google Scholar] [CrossRef] [PubMed]
- Karnik, R.; Duan, C.; Castelino, K.; Daiguji, H.; Majumdar, A. Rectification of ionic current in a nanofluidic diode. Nano Lett. 2007, 7, 547–551. [Google Scholar] [CrossRef]
- Xiao, K.; Chen, L.; Zhang, Z.; Xie, G.; Li, P.; Kong, X.Y.; Wen, L.; Jiang, L. A Tunable Ionic Diode Based on a Biomimetic Structure-Tailorable Nanochannel. Angew. Chem. Int. Ed. 2017, 56, 8168–8172. [Google Scholar] [CrossRef]
- Liu, G.C.; Gao, M.J.; Chen, W.; Hu, X.Y.; Song, L.B.; Liu, B.; Zhao, Y.D. pH-modulated ion-current rectification in a cysteine-functionalized glass nanopipette. Electrochem. Commun. 2018, 97, 6–10. [Google Scholar] [CrossRef]
- Liu, G.C.; Chen, W.; Gao, M.J.; Song, L.B.; Hu, X.Y.; Zhao, Y.D. Ion-current-rectification-based customizable pH response in glass nanopipettes via silanization. Electrochem. Commun. 2018, 93, 95–99. [Google Scholar] [CrossRef]
- Khalid, W.; Abbasi, M.A.; Ali, M.; Ali, Z.; Atif, M.; Trautmann, C.; Ensinger, W. Zinc ion driven ionic conduction through single asymmetric nanochannels functionalized with nanocomposites. Electrochim. Acta 2020, 337, 135810. [Google Scholar] [CrossRef]
- Ali, M.; Ramirez, P.; Nasir, S.; Cervera, J.; Mafe, S.; Ensinger, W. Tetraalkylammonium Cations Conduction through a Single Nanofluidic Diode: Experimental and Theoretical Studies. Electrochim. Acta 2017, 250, 302–308. [Google Scholar] [CrossRef]
- Cervera, J.; Ramirez, P.; Mafe, S.; Stroeve, P. Asymmetric nanopore rectification for ion pumping, electrical power generation, and information processing applications. Electrochim. Acta 2011, 56, 4504–4511. [Google Scholar] [CrossRef]
- Chang, H.C.; Yossifon, G.; Demekhin, E.A. Nanoscale electrokinetics and microvortices: How microhydrodynamics affects nanofluidic ion flux. Annu. Rev. Fluid Mech. 2012, 44, 401–426. [Google Scholar] [CrossRef]
- Yossifon, G.; Chang, Y.C.; Chang, H.C. Rectification, gating voltage, and interchannel communication of nanoslot arrays due to asymmetric entrance space charge polarization. Phys. Rev. Lett. 2009, 103, 154502. [Google Scholar] [CrossRef]
- Sorbello, R.S. Microscopic driving forces for electromigration. MRS Online Proc. Libr. Arch. 1996, 73–81. [Google Scholar] [CrossRef]
- Putra, B.R.; Aoki, K.J.; Chen, J.; Marken, F. Cationic Rectifier Based on a Graphene Oxide-Covered Microhole: Theory and Experiment. Langmuir 2019, 35, 2055–2065. [Google Scholar] [CrossRef]
- Aoki, K.J.; Liu, L.; Marken, F.; Chen, J. Rectification effects of Nafion-backed micropore-voltammograms by difference in migrational modes. Electrochim. Acta 2020, 358, 136839. [Google Scholar] [CrossRef]
- de Grotthuss, C.J.T. Sur la décomposition de l’eau et des corps qu’elle tient en dissolution à l’aide de l’électricité galvanique. Ann. Chim. 1806, 58, 54–73. [Google Scholar]
- Gileadi, E.; Kirowa-Eisner, E. Electrolytic conductivity—The hopping mechanism of the proton and beyond. Electrochim. Acta 2006, 51, 6003–6011. [Google Scholar] [CrossRef]
- Pauliukaite, R.; Juodkazytė, J.; Ramanauskas, R. Theodor von Grotthuss’ Contribution to Electrochemistry. Electrochim. Acta 2017, 236, 28–32. [Google Scholar] [CrossRef]
- Zhang, H.; Aoki, K.; Chen, J.; Nishiumi, T.; Toda, H.; Torita, E. Voltammetric Determination of Both Concentration and Diffusion Coefficient by Combinational Use of Regular and Microelectrodes. Electroanal 2011, 23, 947–952. [Google Scholar] [CrossRef]
- Aoki, K.; Toda, H.; Yamamoto, J.; Chen, J.; Nishiumi, T. Is hydrogen gas in water present as bubbles or hydrated form? J. Electroanal. Chem. 2012, 668, 83–89. [Google Scholar] [CrossRef]
- Unnikrishnan, E.K.; Kumar, S.D.; Maiti, B. Permeation of inorganic anions through Nafion ionomer membrane. J. Membr. Sci. 1997, 24, 133–137. [Google Scholar] [CrossRef]
- Putra, B.R.; Karpińska, K.S.; Kudła, P.; Yin, H.; Boswell, J.A.; Squires, A.M.; Da Silva, M.A.; Edler, K.J.; Fletcher, P.J.; Parker, S.C.; et al. Bacteriophage M13 Aggregation on a Microhole Poly(ethylene terephthalate) Substrate Produces an Anionic Current Rectifier: Sensitivity toward Anionic versus Cationic Guests. ACS Appl. Bio Mater. 2019, 3, 512–521. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, L.; Aoki, K.J.; Chen, J. Electric Migration of Hydrogen Ion in Pore-Voltammetry Suppressed by Nafion Film. Electrochem 2020, 1, 400-409. https://doi.org/10.3390/electrochem1040027
Liu L, Aoki KJ, Chen J. Electric Migration of Hydrogen Ion in Pore-Voltammetry Suppressed by Nafion Film. Electrochem. 2020; 1(4):400-409. https://doi.org/10.3390/electrochem1040027
Chicago/Turabian StyleLiu, Ling, Koichi Jeremiah Aoki, and Jingyuan Chen. 2020. "Electric Migration of Hydrogen Ion in Pore-Voltammetry Suppressed by Nafion Film" Electrochem 1, no. 4: 400-409. https://doi.org/10.3390/electrochem1040027
APA StyleLiu, L., Aoki, K. J., & Chen, J. (2020). Electric Migration of Hydrogen Ion in Pore-Voltammetry Suppressed by Nafion Film. Electrochem, 1(4), 400-409. https://doi.org/10.3390/electrochem1040027