Backscattering Estimation of a Tilted Spherical Cap for Different Kinds of Optical Scattering
Abstract
:1. Introduction
2. Theoretical Background
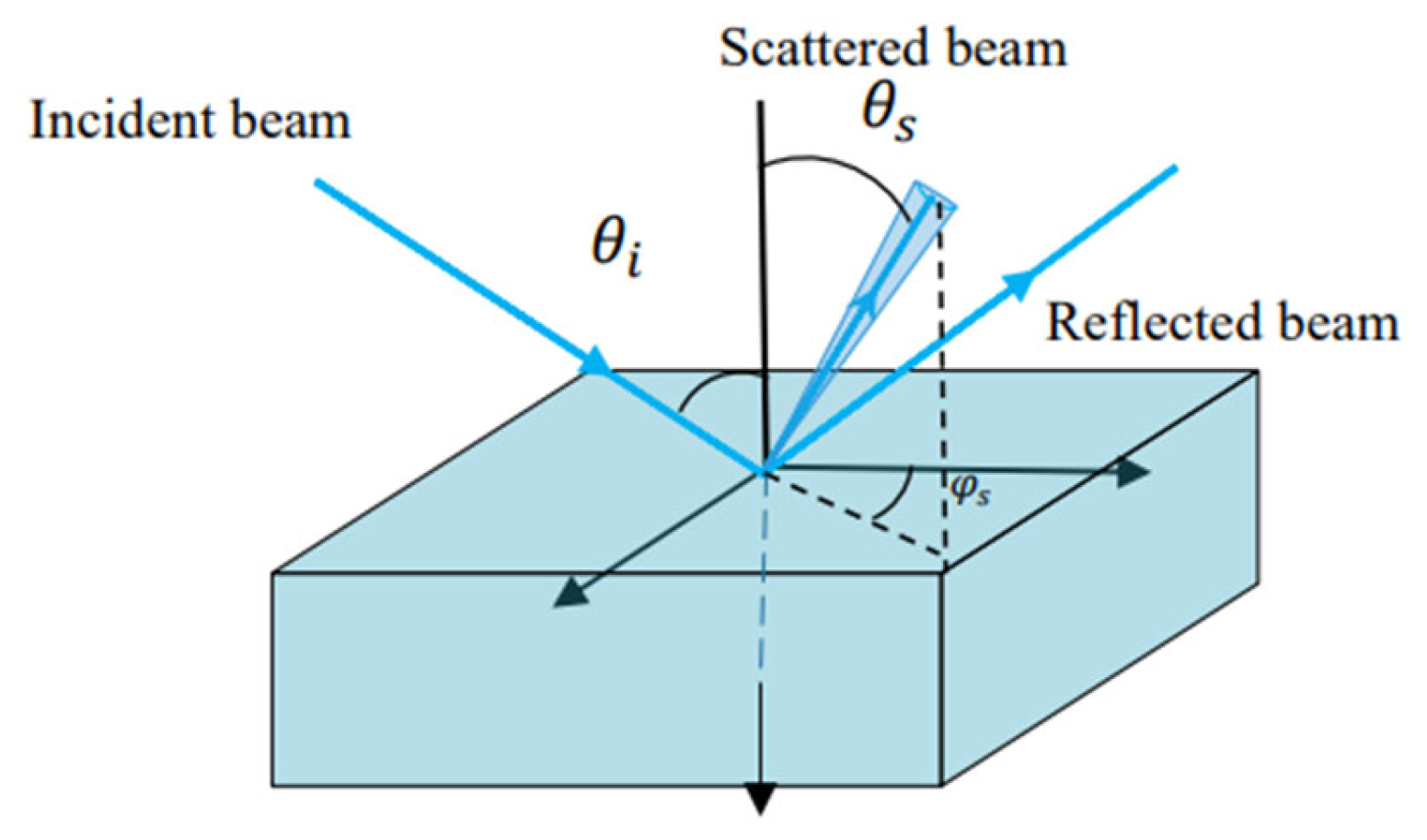
2.1. Definitions
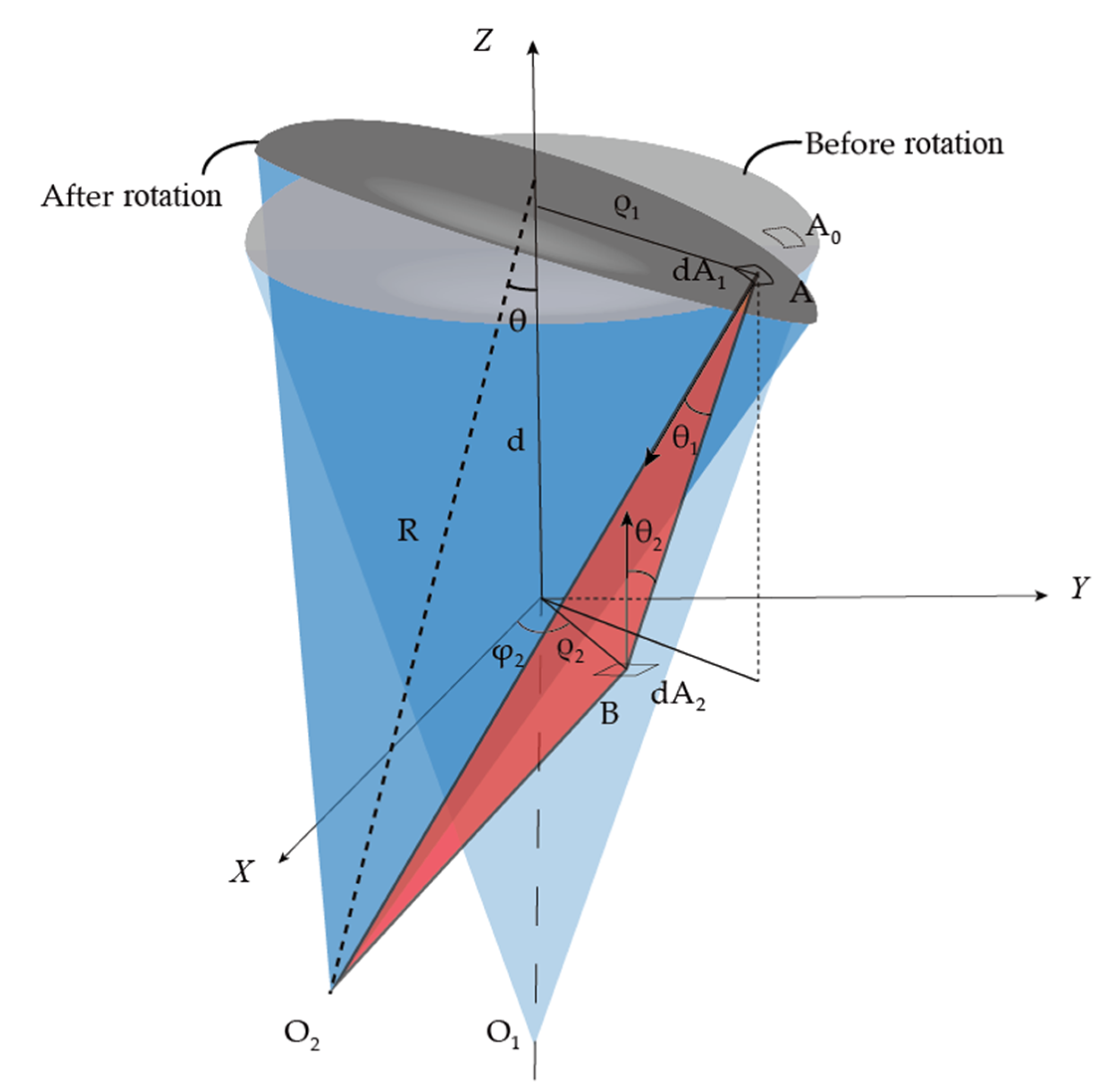
2.2. Mathmetical Modeling
3. Results
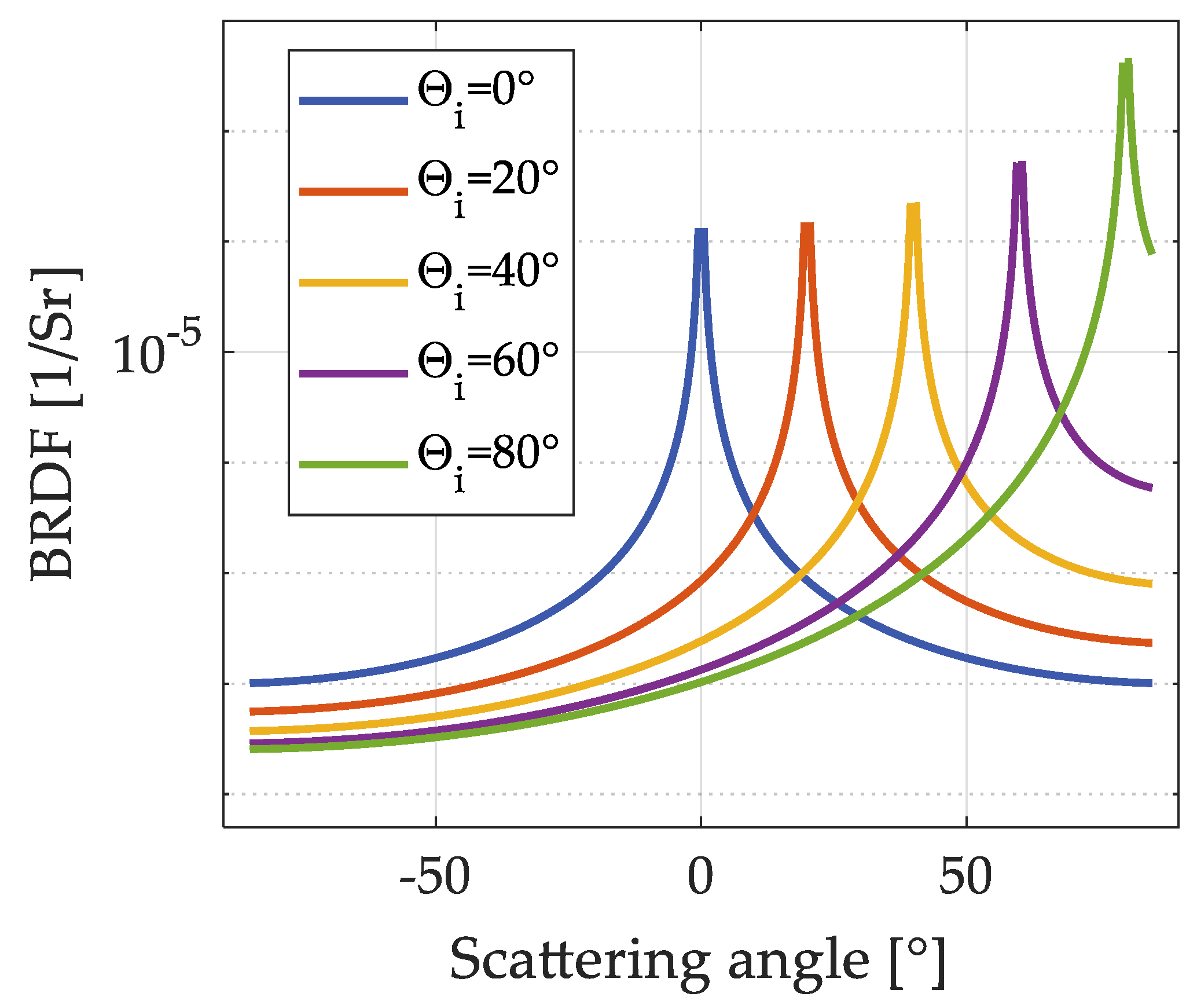
3.1. Diffuse Samples
- Direct analytical method.
- Statistical method, since the scattering is independent from direction, the configuration factor approximates the number of rays received by the detector divided by the number of rays emitted from the scattering source.
- Algebraic method, the laws of closeness, reciprocity, distribution and composition can be applied to derive the target configuration factor.
- Projection method, under some special layouts, the target surface can be projected on a unit sphere in order to simplify the calculation.
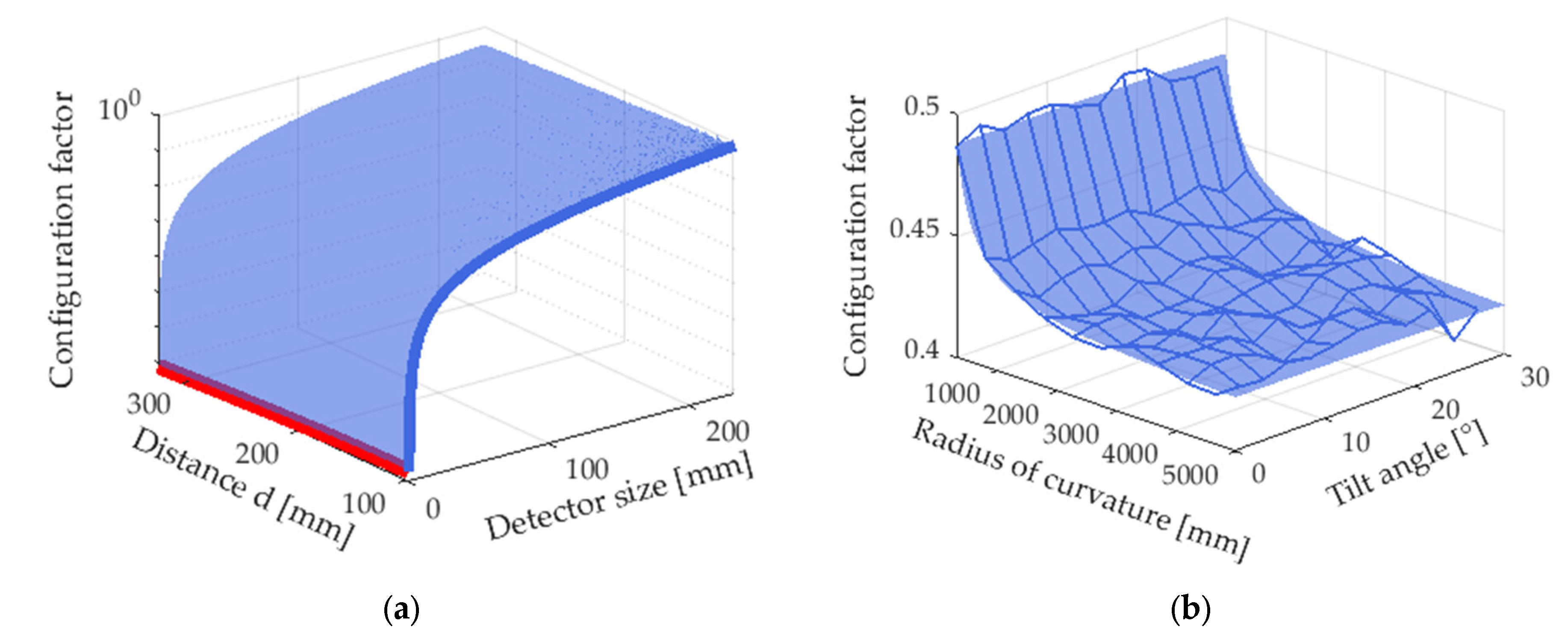
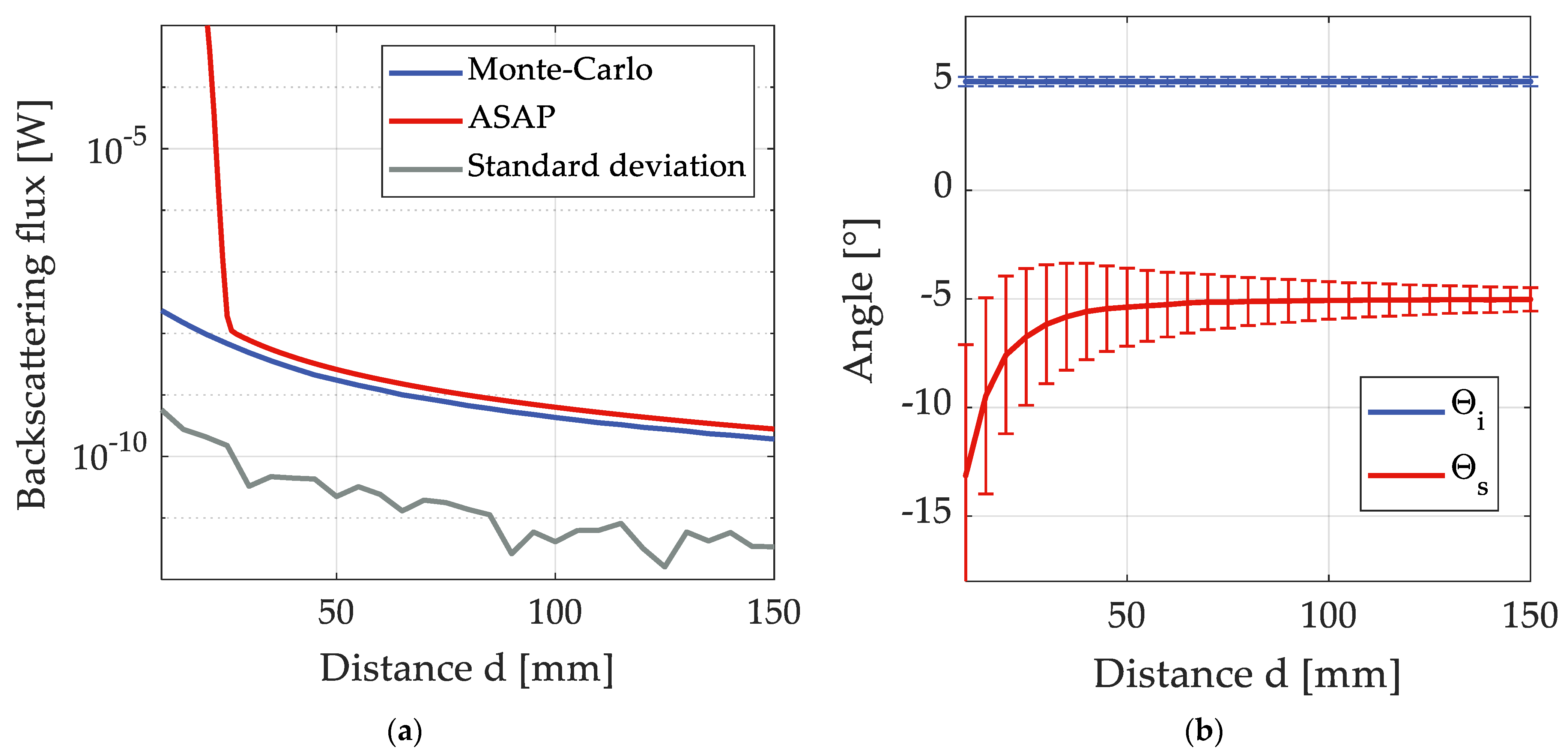
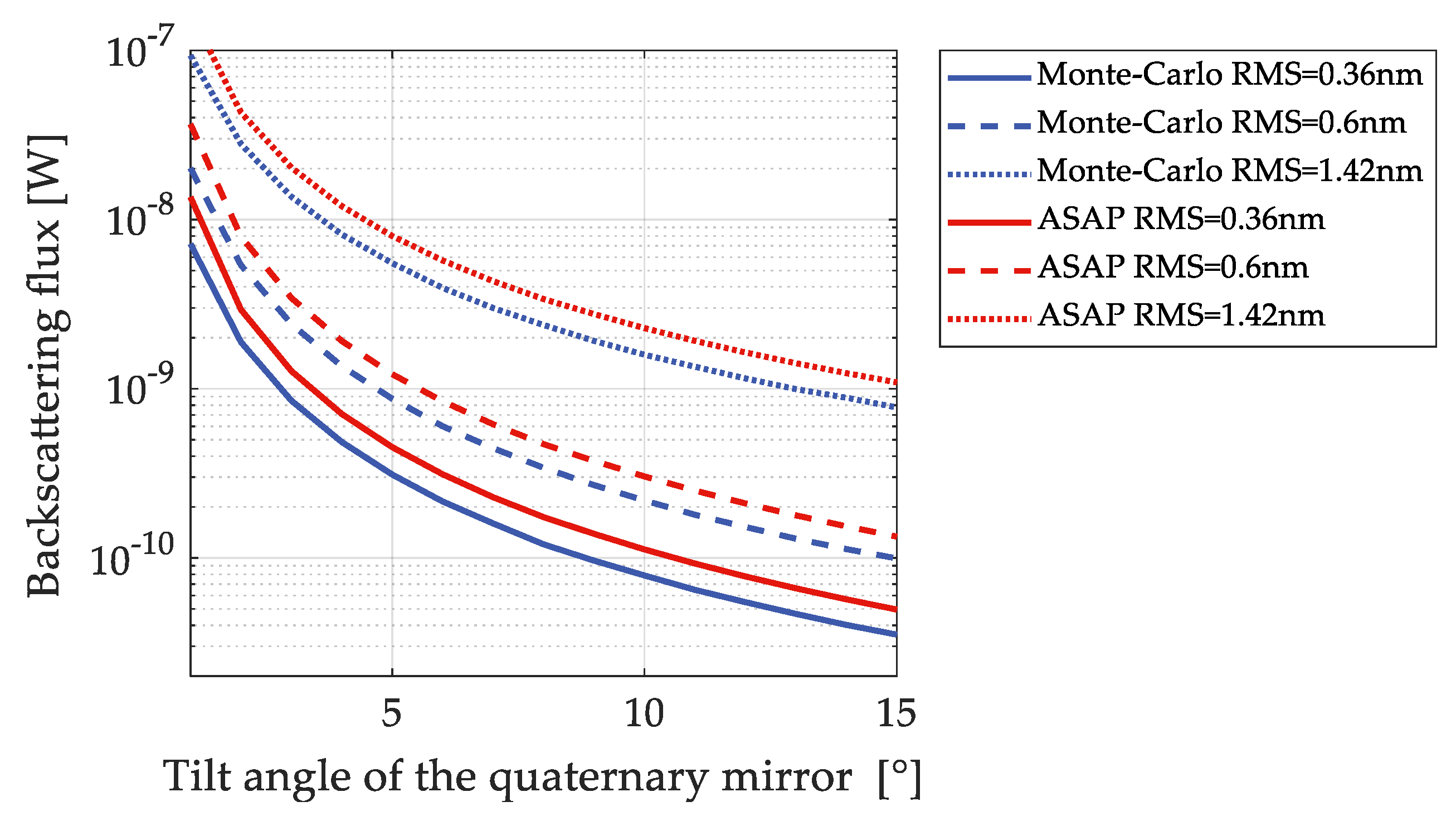
3.2. Backscattering of the Quaternary Mirror of Taiji GW Telescope
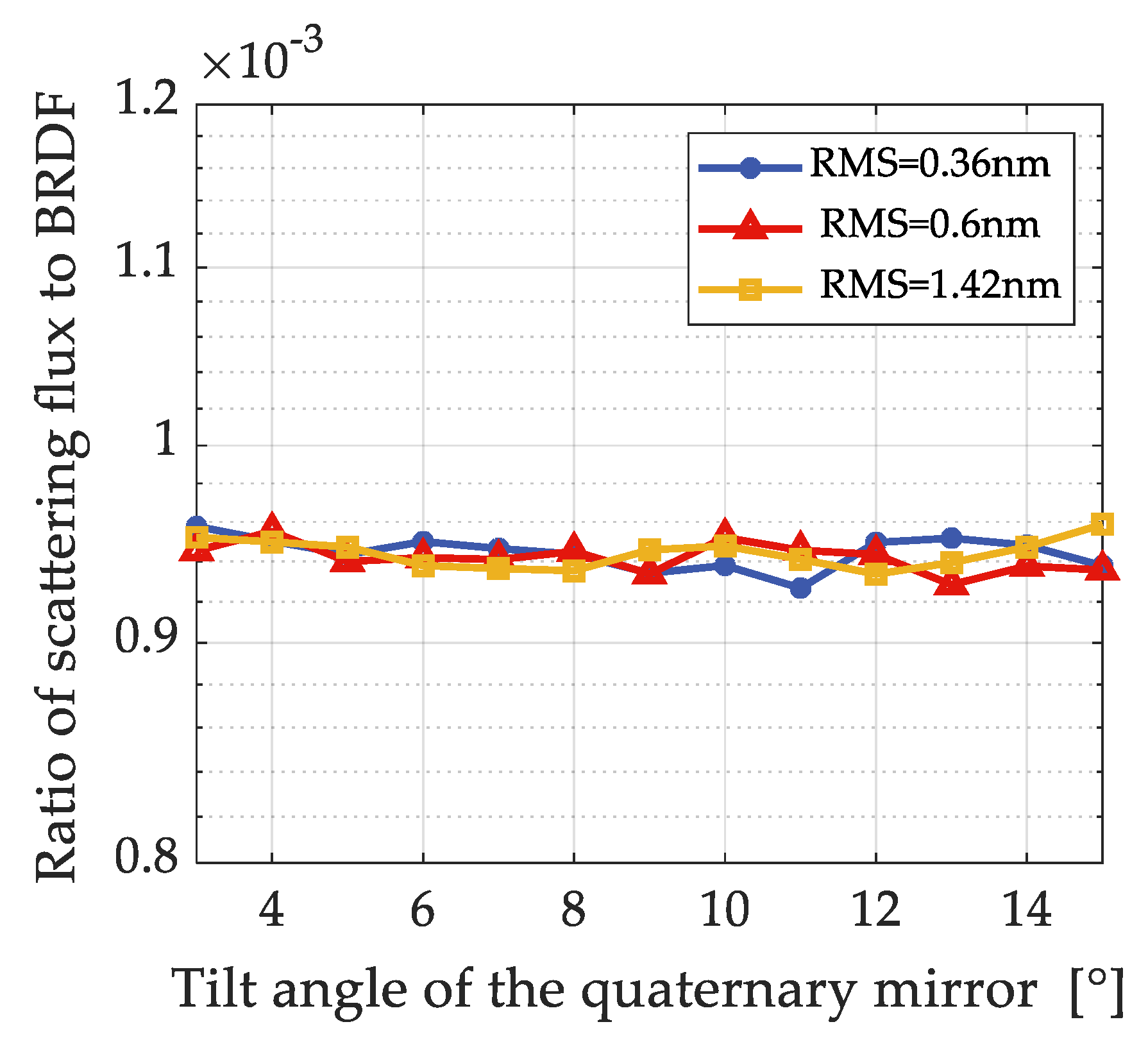
3.3. Error Analysis
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Fest, E.C. Stray Light Analysis and Control; SPIE Press: Bellingham, Washington, USA, 2013; pp. 13–58. [Google Scholar]
- Church, E.; Jenkinson, H.; Zavada, J. Measurement of the finish of diamond-turned metal surfaces by differential light scattering. Opt. Eng. 1977, 16, 360–374. [Google Scholar] [CrossRef]
- Church, E.; Takacs, P. Optimal estimation of finish parameters. In Proceedings of SPIE—Optical Scatter: Applications, Measurement, and Theory, San Diego, CA, USA, 1 December 1991; Volume 1530. [Google Scholar]
- Spyak, P. Scatter from particulate-contaminated mirrors—Part 2: Theory and experiment for dust and lambda = 0.6328 um. Opt. Eng. 1992, 31, 1757–1763. [Google Scholar] [CrossRef]
- Spyak, P.; Wolfe, W. Scatter from particulate-contaminated mirrors—Part 3: Theory and experiment for dust and lambda = 10.6 um. Opt. Eng. 1992, 31, 1764–1774. [Google Scholar] [CrossRef]
- Trost, M.; Herffurth, T.; Schmitz, D.; Schröder, S.; Duparré, A.; Tünnermann, A. Evaluation of subsurface damage by light scattering techniques. Appl. Opt. 2013, 52, 6579–6588. [Google Scholar] [CrossRef] [PubMed]
- Stauder, J. Stray Light Comparison of Off-Axis and On-Axis Telescopes. Ph.D. Thesis, Utah State University, Logan, UT, USA, 2000. [Google Scholar]
- Peterson, G. Analytic expression for in-field scattered light distribution. Opt. Modeling Perform. Predict. 2004, 5178, 184–193. [Google Scholar] [CrossRef]
- Harvey, J.E.; Krywonos, A.; Peterson, G.; Bruner, M. Image degradation due to scattering effects in two-mirror telescopes. Opt. Eng. 2010, 49, 063202–063207. [Google Scholar] [CrossRef]
- Luo, Z.; Wang, Y.; Wu, Y.; Hu, W.; Jin, G. The Taiji program: A concise overview. Prog. Theor. Exp. Phys. 2021, 2021, 05A108. [Google Scholar] [CrossRef]
- Sankar, S.; Livas, J. Optical Telescope Design for a Space-Based Gravitational-Wave Mission. In Proceedings of SPIE—Optical, Infrared and Millimeter Wave, Montréal, QC, Canada, 22–27 June 2014; Volume 9143. [Google Scholar]
- Livas, J.; Sankar, S. Optical Telescope System-Level Design Considerations for a Space-Based Gravitational Wave Mission. In Proceedings of SPIE—Optical, Infrared, and Millimeter Wave, Edinburgh, UK, 26 June–1 July 2016; Volume 9904. [Google Scholar]
- Canuel, B.; Genin, E.; Vajente, G.; Marque, J. Displacement noise from back scattering and specular reflection of input optics in advanced gravitational wave detectors. Opt. Express 2013, 21, 10546–10562. [Google Scholar] [CrossRef] [PubMed]
- Khan, I.; Lequime, M.; Zerrad, M.; Amra, C. Coherent Detection of the Light Backscattered by an Optical Surface. In Proceedings of SPIE—International Conference on Space Optics—ICSO 2020, Online, 11 June 2021; Volume 11852. [Google Scholar]
- Khodnevych, V.; Pace, S.; Vinet, J.-Y.; Dinu-Jaeger, N.; Lintz, M. Study of the Coherent Perturbation of a Michelson Interferometer Due to the Return from a Scattering Surface. In Proceedings of SPIE—International Conference on Space Optics—ICSO 2018, Chania, Greece, 9–12 October 2018; Volume 11807. [Google Scholar]
- Lequime, M.; Lumeau, J. Accurate determination of the optical performances of antireflective coatings by low coherence reflectometry. Appl. Optics 2007, 46, 5635–5644. [Google Scholar] [CrossRef] [PubMed]
- Stover, J.C. Optical Scattering—Measurement and Analysis; SPIE Press: Bellingham, WA, USA, 1990. [Google Scholar]
- Duparré, A.; Ferre-Borrull, J.; Gliech, S.; Notni, G.; Steinert, J.; Bennett, J. Surface Characterization Techniques for Determining the Root-Mean-Square Roughness and Power Spectral Densities of Optical Components. Appl. Optics 2002, 41, 154–171. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Harvey, J. Light-Scattering Characteristics of Optical Surfaces. In Proceedings of SPIE—Stray Light Problems in Optical Systems, Reston, USA, 26 September 1977; Volume 0107. [Google Scholar]
- Michael, G.D. K-correlation power spectral density and surface scatter model. In Proceedings of the SPIE—Optical Systems Degradation, Contamination, and Stray Light: Effects Measurements, and Control II, San Diego, CA, USA, 7 September 2006; Volume 6291. [Google Scholar]
- Freniere, E.R. Interactive software for optomechanical modeling. In Proceedings of the SPIE—Lens Design, Illumination, and Optomechanical Modeling, San Diego, CA, USA, 25 September 1997; Volume 3130. [Google Scholar]
- A Catalog of Radiation Heat Transfer Configuration Factors. Available online: http://www.thermalradiation.net/tablecon.html (accessed on 19 January 2022).
- Sasaki, K.; Sznajder, M. Analytical view factor solutions of a spherical cap from an infinitesimal surface. Int. J. Heat Mass Transf. 2020, 163, 120477. [Google Scholar] [CrossRef]
- Wolfe, W. Radiometry and the detection of optical radiation. J. Opt. Soc. Am. A 1984, 1, 565. [Google Scholar]
- Koshel, R. Illumination Engineering; Wiley-IEEE Press: Hoboken, NJ, USA, 2013; pp. 31–44. [Google Scholar]
- Veach, E. Robust Monte Carlo Methods for Light Transport Simulation. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 1997. [Google Scholar]
- Acernese, F.; Alshourbagy, M.; Amico, P.; Antonucci, F.; Aoudia, S.; Arun, K.; Astone, P.; Avino, S.; Baggio, L.; Ballardin, G. Noise studies during the first Virgo science run and after. Class. Quantum Gravity 2008, 25, 184003. [Google Scholar] [CrossRef]
- Acernese, F.; Alshourbagy, M.; Antonucci, F.; Aoudia, S.; Arun, K.; Astone, P.; Ballardin, G.; Barone, F.; Barsuglia, M.; Bauer, T.S.; et al. Control of the Laser Frequency of the Virgo Gravitational Wave Interferometer with an in-Loop Relative Frequency Stability of 1.0 × 10–21 on a 100 ms Time Scale. In Proceedings of IEEE—International Frequency Control Symposium Joint with the 22nd European Frequency and Time Forum, Besancon, France, 20–24 April 2009. [Google Scholar]
- Feingold, A. A New Look at Radiation Configuration Factors between Disks. J. Heat Transf. 1978, 100, 742–744. [Google Scholar] [CrossRef]
- ASAP—Breault Research Organization. Available online: https://bro.com/asap/ (accessed on 19 January 2022).
- Technical Guide: Scattering in ASAP; Breault Research Organization: Tucson, AZ, USA, 2014.
- Marsaglia, G. Choosing a Point from the Surface of a Sphere. Ann. Math. Stat. 1972, 43, 645–646. [Google Scholar] [CrossRef]
- Neumann, V. Various techniques used in connection with random digits. Natl. Bur. Stand. Appl. Math Ser. 1950, 12, 36–38. [Google Scholar]
- Shirley, P. Physically Based Lighting Calculations for Computer Graphics. Ph.D. Thesis, University of Illinois at Urbana-Champaign, Champaign, IL, USA, 1991. [Google Scholar]
- Chiu, K.; Wang, C.; Shirley, P. V.4.-Multi-Jittered Sampling. In Graphics Gems; Heckbert, P.S., Ed.; Academic Press: Cambridge, MA, USA, 1994; pp. 370–374. [Google Scholar]


| Nomenclature | |
|---|---|
| R | Radius of curvature |
| Tilt angle | |
| d | Distance between the detector and vertex of the spherical cap |
| N | Number of random point pairs |
| The center of spherical cap before rotation | |
| The center of spherical cap after rotation | |
| Cartesian coordinates of the sample before rotation | |
| Cylinder coordinates of the sample before rotation | |
| Cartesian coordinates of the sample after rotation | |
| Cartesian coordinates of the detector | |
| r | Distance between two differential patches. |
| A1, A2 | Area of spherical cap and the detector |
| dA1, dA2 | The differential area of A1, A2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leng, R.; Wang, Z.; Fang, C.; Liu, L.; Chen, Z.; Cui, X. Backscattering Estimation of a Tilted Spherical Cap for Different Kinds of Optical Scattering. Optics 2022, 3, 177-190. https://doi.org/10.3390/opt3020018
Leng R, Wang Z, Fang C, Liu L, Chen Z, Cui X. Backscattering Estimation of a Tilted Spherical Cap for Different Kinds of Optical Scattering. Optics. 2022; 3(2):177-190. https://doi.org/10.3390/opt3020018
Chicago/Turabian StyleLeng, Rongkuan, Zhi Wang, Chao Fang, Lei Liu, Zhiwei Chen, and Xinxu Cui. 2022. "Backscattering Estimation of a Tilted Spherical Cap for Different Kinds of Optical Scattering" Optics 3, no. 2: 177-190. https://doi.org/10.3390/opt3020018
APA StyleLeng, R., Wang, Z., Fang, C., Liu, L., Chen, Z., & Cui, X. (2022). Backscattering Estimation of a Tilted Spherical Cap for Different Kinds of Optical Scattering. Optics, 3(2), 177-190. https://doi.org/10.3390/opt3020018







