Synthesizing General Electromagnetic Partially Coherent Sources from Random, Correlated Complex Screens
Abstract
1. Introduction
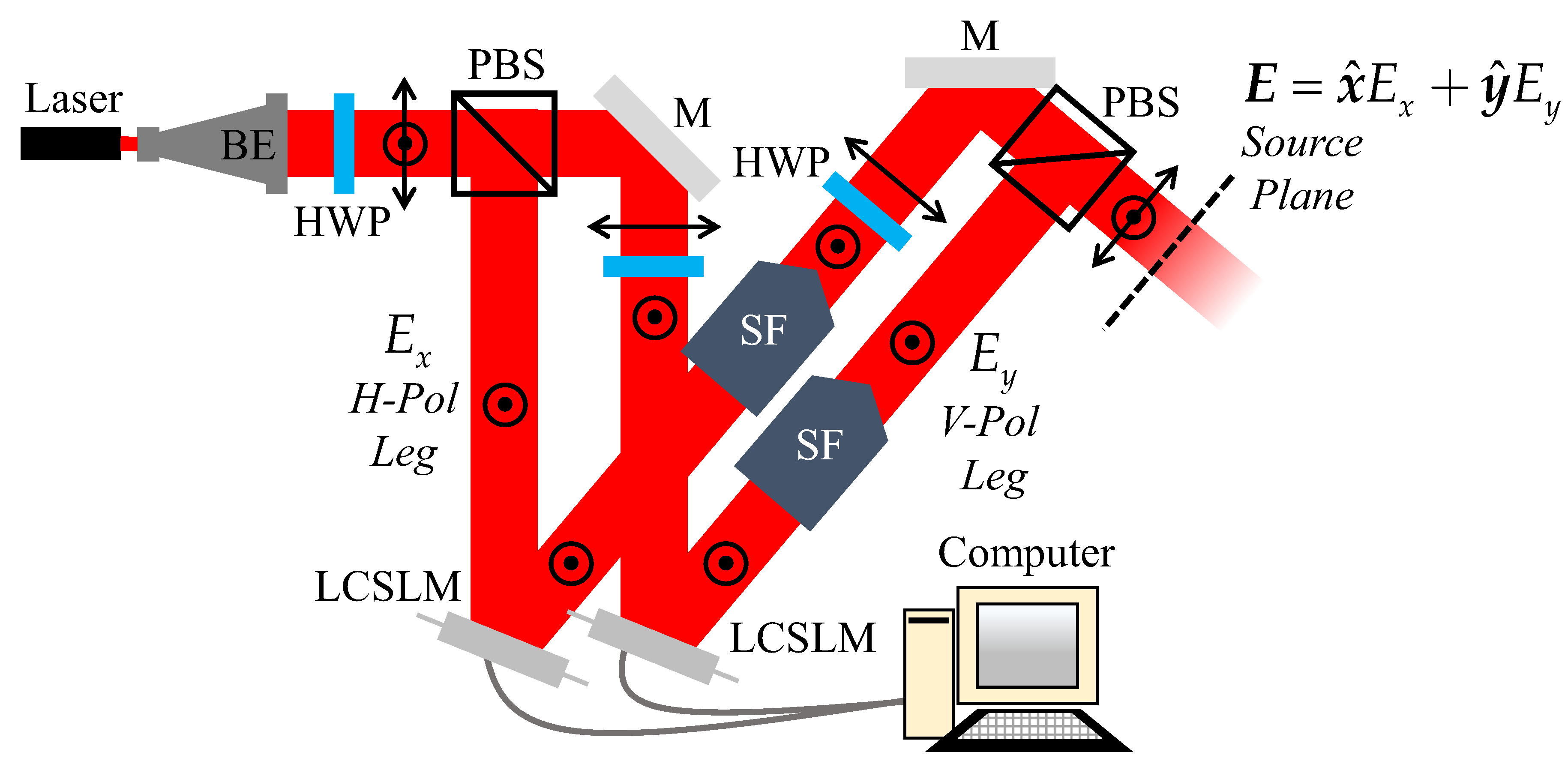
2. Materials and Methods
- Identify , , , , , , and associated with the desired .
- Compute and using Equation (14).
- Generate and . The means of and are zero, and the covariance matrix isUsing the Cholesky factor [65] of , i.e., andwe generate and using the following relations:where , , , and are mutually-independent, delta-correlated, Gaussian random functions.
- Create a field realization using Equation (7).
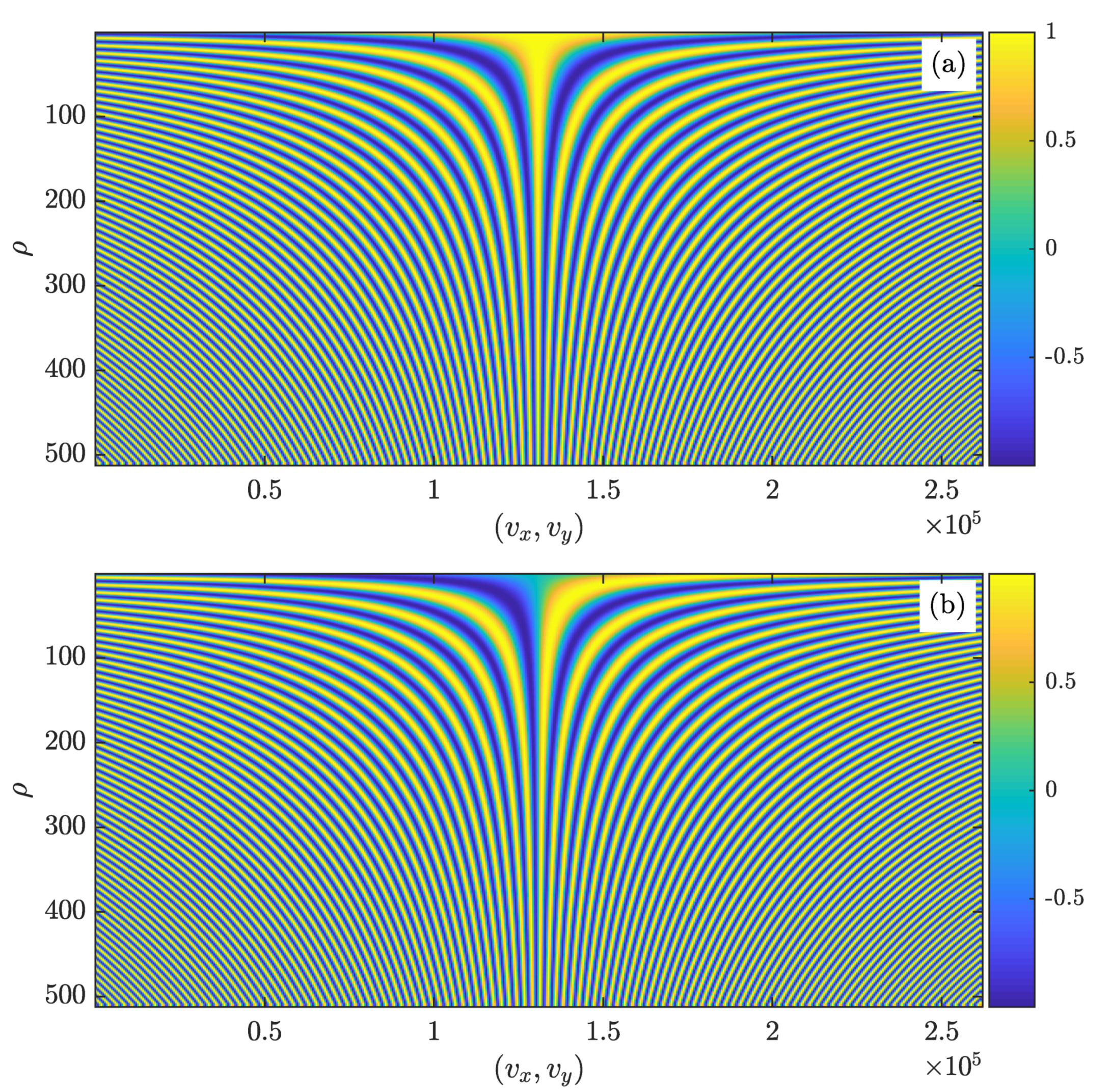
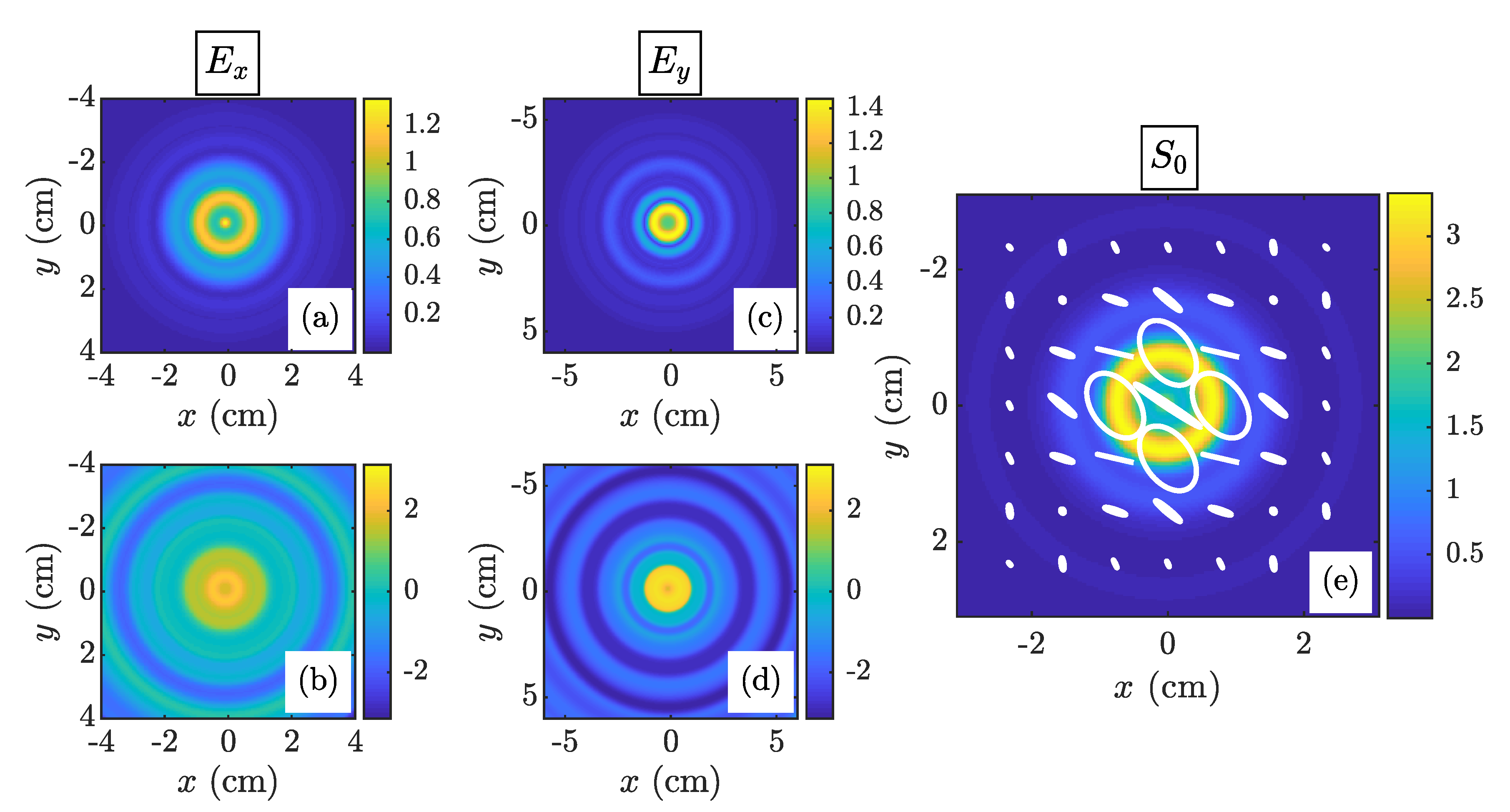
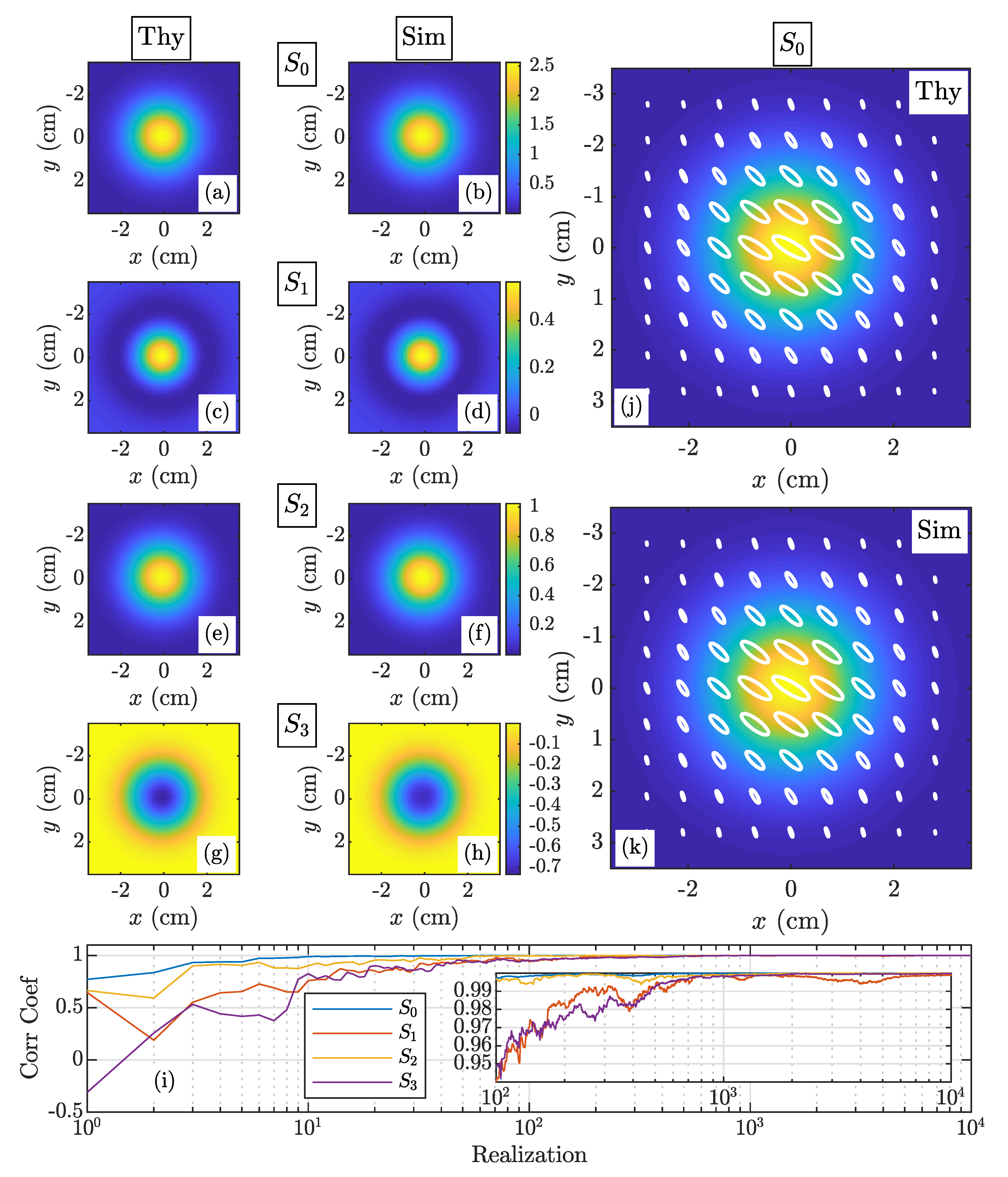
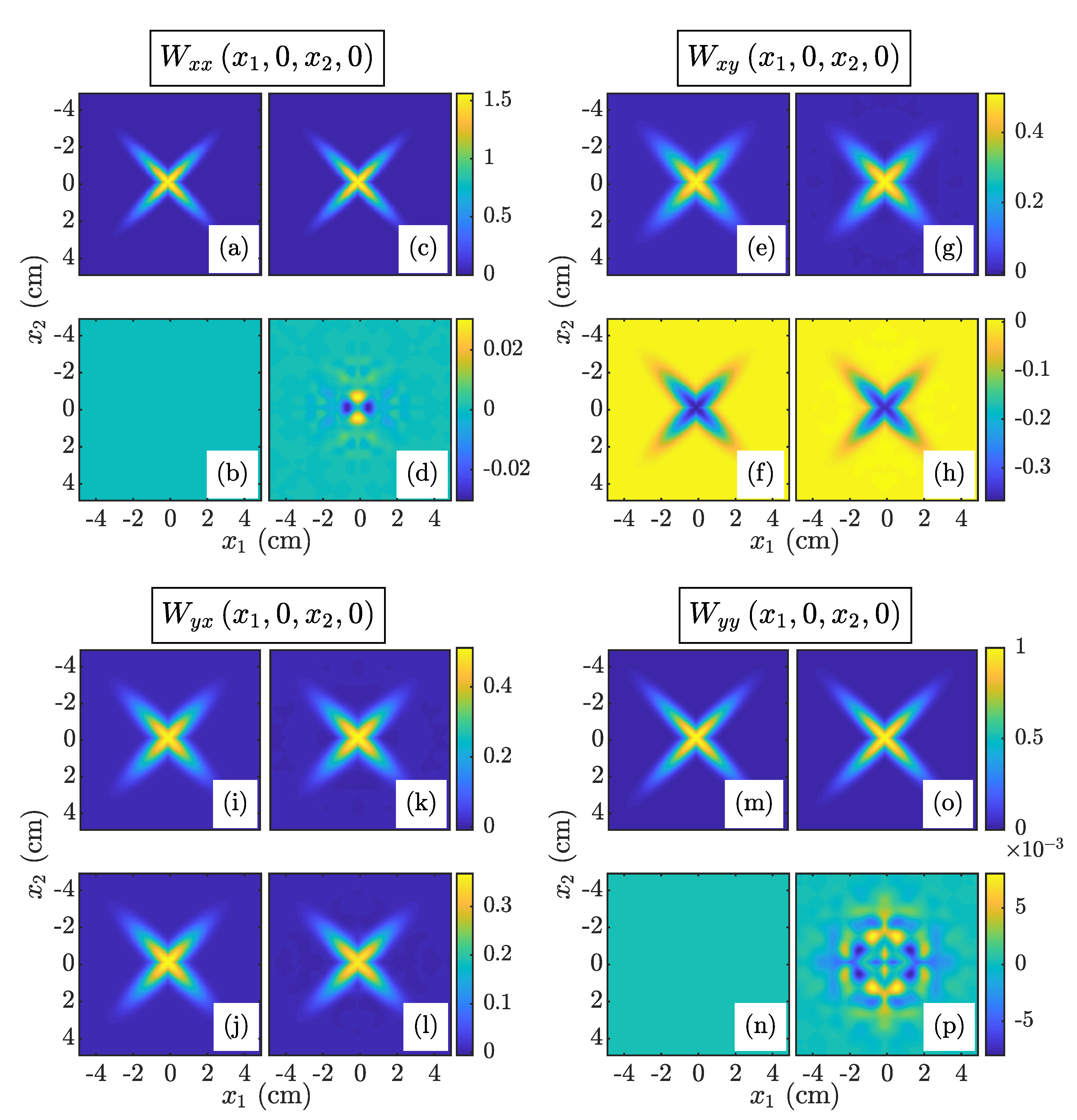
3. Results and Discussion
- Generate four vectors of mutually-independent, zero-mean, unit-variance Gaussian random numbers, i.e., , , , and .
- Produce and using item 1 and Equation (17), where and are represented as vectors.
- Multiply (element-wise) by (represented as vectors).
- Interpolate vectors (discrete functions of ) to matrices (2D discrete functions of ).
- Multiply (element-wise) by to form realizations.
4. Conclusions
Supplementary Materials
Funding
Acknowledgments
Conflicts of Interest
References
- Wolf, E. Unified theory of coherence and polarization of random electromagnetic beams. Phys. Lett. A 2003, 312, 263–267. [Google Scholar] [CrossRef]
- Mandel, L.; Wolf, E. Optical Coherence and Quantum Optics; Cambridge University: New York, NY, USA, 1995. [Google Scholar]
- Wolf, E. Introduction to the Theory of Coherence and Polarization of Light; Cambridge University: Cambridge, UK, 2007. [Google Scholar]
- Agarwal, G.S.; Classen, A. Partial coherence in modern optics: Emil Wolf’s legacy in the 21st century. Prog. Opt. 2019. [Google Scholar] [CrossRef]
- Korotkova, O. Random Light Beams: Theory and Applications; CRC: Boca Raton, FL, USA, 2014. [Google Scholar]
- Gbur, G. Partially coherent beam propagation in atmospheric turbulence. J. Opt. Soc. Am. A 2014, 31, 2038–2045. [Google Scholar] [CrossRef] [PubMed]
- Zhan, Q. (Ed.) Vectorial Optical Fields: Fundamentals and Applications; World Scientific: Hackensack, NJ, USA, 2014. [Google Scholar]
- Efimov, A. Gigabit per second modulation and transmission of a partially coherent beam through laboratory turbulence. Proc. SPIE 2016, 9739, 163–168. [Google Scholar] [CrossRef]
- Korotkova, O. Light propagation in a turbulent ocean. Prog. Opt. 2019, 64, 1–43. [Google Scholar] [CrossRef]
- Korotkova, O.; Gbur, G. Applications of optical coherence theory. Prog. Opt. 2020. [Google Scholar] [CrossRef]
- Borghi, R.; Gori, F.; Ponomarenko, S.A. On a class of electromagnetic diffraction-free beams. J. Opt. Soc. Am. A 2009, 26, 2275–2281. [Google Scholar] [CrossRef]
- Cai, Y.; Chen, Y.; Wang, F. Generation and propagation of partially coherent beams with nonconventional correlation functions: A review. J. Opt. Soc. Am. A 2014, 31, 2083–2096. [Google Scholar] [CrossRef]
- Voelz, D.; Xiao, X.; Korotkova, O. Numerical modeling of Schell-model beams with arbitrary far-field patterns. Opt. Lett. 2015, 40, 352–355. [Google Scholar] [CrossRef]
- Hyde, M.W.; Basu, S.; Voelz, D.G.; Xiao, X. Experimentally generating any desired partially coherent Schell-model source using phase-only control. J. Appl. Phys. 2015, 118, 093102. [Google Scholar] [CrossRef]
- Lajunen, H.; Saastamoinen, T. Propagation characteristics of partially coherent beams with spatially varying correlations. Opt. Lett. 2011, 36, 4104–4106. [Google Scholar] [CrossRef] [PubMed]
- Santarsiero, M.; Martínez-Herrero, R.; Maluenda, D.; de Sande, J.C.G.; Piquero, G.; Gori, F. Partially coherent sources with circular coherence. Opt. Lett. 2017, 42, 1512–1515. [Google Scholar] [CrossRef] [PubMed]
- Piquero, G.; Santarsiero, M.; Martínez-Herrero, R.; de Sande, J.C.G.; Alonzo, M.; Gori, F. Partially coherent sources with radial coherence. Opt. Lett. 2018, 43, 2376–2379. [Google Scholar] [CrossRef]
- Yu, J.; Cai, Y.; Gbur, G. Rectangular Hermite non-uniformly correlated beams and its propagation properties. Opt. Express 2018, 26, 27894–27906. [Google Scholar] [CrossRef] [PubMed]
- Mei, Z.; Korotkova, O. Random sources for rotating spectral densities. Opt. Lett. 2017, 42, 255–258. [Google Scholar] [CrossRef]
- Hyde, M.W. Generating electromagnetic Schell-model sources using complex screens with spatially varying auto- and cross-correlation functions. Results Phys. 2019, 15, 102663. [Google Scholar] [CrossRef]
- Mei, Z.; Korotkova, O. Electromagnetic Schell-model sources generating far fields with stable and flexible concentric rings profiles. Opt. Express 2016, 24, 5572–5583. [Google Scholar] [CrossRef] [PubMed]
- Liang, C.; Mi, C.; Wang, F.; Zhao, C.; Cai, Y.; Ponomarenko, S.A. Vector optical coherence lattices generating controllable far-field beam profiles. Opt. Express 2017, 25, 9872–9885. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Wang, F.; Yu, J.; Liu, L.; Cai, Y. Vector Hermite-Gaussian correlated Schell-model beam. Opt. Express 2016, 24, 15232–15250. [Google Scholar] [CrossRef] [PubMed]
- Tong, Z.; Korotkova, O. Electromagnetic nonuniformly correlated beams. J. Opt. Soc. Am. A 2012, 29, 2154–2158. [Google Scholar] [CrossRef] [PubMed]
- Ostrovsky, A.S.; Olvera, M.A.; Rickenstorff, C.; Martínez-Niconoff, G.; Arrizón, V. Generation of a secondary electromagnetic source with desired statistical properties. Opt. Commun. 2010, 283, 4490–4493. [Google Scholar] [CrossRef]
- Hyde, M.W.; Bose-Pillai, S.; Voelz, D.G.; Xiao, X. Generation of vector partially coherent optical sources using phase-only spatial light modulators. Phys. Rev. Appl. 2016, 6, 064030. [Google Scholar] [CrossRef]
- Hyde, M.W. Shaping the far-zone intensity, degree of polarization, angle of polarization, and ellipticity angle using vector Schell-model sources. Results Phys. 2019, 12, 2242–2250. [Google Scholar] [CrossRef]
- Mei, Z.; Korotkova, O.; Shchepakina, E. Electromagnetic multi-Gaussian Schell-model beams. J. Opt. 2013, 15, 025705. [Google Scholar] [CrossRef]
- Mao, H.; Chen, Y.; Liang, C.; Chen, L.; Cai, Y.; Ponomarenko, S.A. Self-steering partially coherent vector beams. Opt. Express 2019, 27, 14353–14368. [Google Scholar] [CrossRef]
- Mao, Y.; Wang, Y.; Mei, Z. Electromagnetic fractional multi-Gaussian Schell-model beams and their statistical properties. J. Opt. 2019, 21, 035601. [Google Scholar] [CrossRef]
- Mishra, S.; Gautam, S.K.; Naik, D.N.; Chen, Z.; Pu, J.; Singh, R.K. Tailoring and analysis of vectorial coherence. J. Opt. 2018, 20, 125605. [Google Scholar] [CrossRef]
- Shirai, T. Interpretation of the recipe for synthesizing genuine cross-spectral density matrices. Opt. Commun. 2010, 283, 4478–4483. [Google Scholar] [CrossRef]
- Gori, F.; Ramírez-Sánchez, V.; Santarsiero, M.; Shirai, T. On genuine cross-spectral density matrices. J. Opt. A Pure Appl. Opt. 2009, 11, 085706. [Google Scholar] [CrossRef]
- Grella, R. Synthesis of generalized Collett-Wolf sources. J. Opt. 1982, 13, 127–131. [Google Scholar] [CrossRef]
- Santarsiero, M.; Borghi, R.; Ramírez-Sánchez, V. Synthesis of electromagnetic Schell-model sources. J. Opt. Soc. Am. A 2009, 26, 1437–1443. [Google Scholar] [CrossRef] [PubMed]
- Hyde, M.W. Controlling the spatial coherence of an optical source using a spatial filter. Appl. Sci. 2018, 8, 1465. [Google Scholar] [CrossRef]
- Cai, Y.; Chen, Y.; Yu, J.; Liu, X.; Liu, L. Generation of partially coherent beams. Prog. Opt. 2017, 62, 157–223. [Google Scholar] [CrossRef]
- Wang, H.; Peng, X.; Liu, L.; Wang, F.; Cai, Y.; Ponomarenko, S.A. Generating bona fide twisted Gaussian Schell-model beams. Opt. Lett. 2019, 44, 3709–3712. [Google Scholar] [CrossRef] [PubMed]
- Friberg, A.T.; Tervonen, E.; Turunen, J. Interpretation and experimental demonstration of twisted Gaussian Schell-model beams. J. Opt. Soc. Am. A 1994, 11, 1818–1826. [Google Scholar] [CrossRef]
- Wang, F.; Liang, C.; Yuan, Y.; Cai, Y. Generalized multi-Gaussian correlated Schell-model beam: From theory to experiment. Opt. Express 2014, 22, 23456–23464. [Google Scholar] [CrossRef]
- Wang, F.; Wu, G.; Liu, X.; Zhu, S.; Cai, Y. Experimental measurement of the beam parameters of an electromagnetic Gaussian Schell-model source. Opt. Lett. 2011, 36, 2722–2724. [Google Scholar] [CrossRef]
- Liu, X.; Wang, F.; Liu, L.; Zhao, C.; Cai, Y. Generation and propagation of an electromagnetic Gaussian Schell-model vortex beam. J. Opt. Soc. Am. A 2015, 32, 2058–2065. [Google Scholar] [CrossRef]
- Piquero, G.; Gori, F.; Romanini, P.; Santarsiero, M.; Borghi, R.; Mondello, A. Synthesis of partially polarized Gaussian Schell-model sources. Opt. Commun. 2002, 208, 9–16. [Google Scholar] [CrossRef]
- Shirai, T.; Korotkova, O.; Wolf, E. A method of generating electromagnetic Gaussian Schell-model beams. J. Opt. A Pure Appl. Opt. 2005, 7, 232–237. [Google Scholar] [CrossRef]
- Hyde, M.W.; Bose-Pillai, S.R.; Wood, R.A. Synthesis of non-uniformly correlated partially coherent sources using a deformable mirror. Appl. Phys. Lett. 2017, 111, 101106. [Google Scholar] [CrossRef]
- Shirai, T.; Wolf, E. Coherence and polarization of electromagnetic beams modulated by random phase screens and their changes on propagation in free space. J. Opt. Soc. Am. A 2004, 21, 1907–1916. [Google Scholar] [CrossRef] [PubMed]
- Ostrovsky, A.S.; Martínez-Niconoff, G.; Arrizón, V.; Martínez-Vara, P.; Olvera-Santamaría, M.A.; Rickenstorff-Parrao, C. Modulation of coherence and polarization using liquid crystal spatial light modulators. Opt. Express 2009, 17, 5257–5264. [Google Scholar] [CrossRef] [PubMed]
- Ostrovsky, A.S.; Rodríguez-Zurita, G.; Meneses-Fabián, C.; Olvera-Santamaría, M.A.; Rickenstorff-Parrao, C. Experimental generating the partially coherent and partially polarized electromagnetic source. Opt. Express 2010, 18, 12864–12871. [Google Scholar] [CrossRef] [PubMed]
- Basu, S.; Hyde, M.W.; Xiao, X.; Voelz, D.G.; Korotkova, O. Computational approaches for generating electromagnetic Gaussian Schell-model sources. Opt. Express 2014, 22, 31691–31707. [Google Scholar] [CrossRef] [PubMed]
- Hyde, M.W. Stochastic complex transmittance screens for synthesizing general partially coherent sources. J. Opt. Soc. Am. A 2020, 37, 257–264. [Google Scholar] [CrossRef] [PubMed]
- Rosales-Guzmán, C.; Ndagano, B.; Forbes, A. A review of complex vector light fields and their applications. J. Opt. 2018, 20, 123001. [Google Scholar] [CrossRef]
- Yu, Z.; Chen, H.; Chen, Z.; Hao, J.; Ding, J. Simultaneous tailoring of complete polarization, amplitude and phase of vector beams. Opt. Commun. 2015, 345, 135–140. [Google Scholar] [CrossRef]
- Chen, Z.; Zeng, T.; Qian, B.; Ding, J. Complete shaping of optical vector beams. Opt. Express 2015, 23, 17701–17710. [Google Scholar] [CrossRef]
- Mitchell, K.J.; Turtaev, S.; Padgett, M.J.; Čižmár, T.; Phillips, D.B. High-speed spatial control of the intensity, phase and polarisation of vector beams using a digital micro-mirror device. Opt. Express 2016, 24, 29269–29282. [Google Scholar] [CrossRef]
- Rosales-Guzmán, C.; Bhebhe, N.; Forbes, A. Simultaneous generation of multiple vector beams on a single SLM. Opt. Express 2017, 25, 25697–25706. [Google Scholar] [CrossRef]
- Rosales-Guzmán, C.; Bhebhe, N.; Mahonisi, N.; Forbes, A. Multiplexing 200 spatial modes with a single hologram. J. Opt. 2017, 19, 113501. [Google Scholar] [CrossRef]
- Fu, S.; Zhai, Y.; Wang, T.; Yin, C.; Gao, C. Tailoring arbitrary hybrid Poincarè beams through a single hologram. Appl. Phys. Lett. 2017, 111, 211101. [Google Scholar] [CrossRef]
- Scholes, S.; Kara, R.; Pinnell, J.; Rodríguez-Fajardo, V.; Forbes, A. Structured light with digital micromirror devices: A guide to best practice. Opt. Eng. 2019, 59, 1–12. [Google Scholar] [CrossRef]
- Restaino, S.R.; Teare, S.W. Introduction to Liquid Crystals for Optical Design and Engineering; SPIE Press: Bellingham, WA, USA, 2015. [Google Scholar]
- Zhang, Z.; You, Z.; Chu, D. Fundamentals of phase-only liquid crystal on silicon (LCOS) devices. Light Sci. Appl. 2014, 3, e213. [Google Scholar] [CrossRef]
- Rosales-Guzmán, C.; Forbes, A. How to Shape Light with Spatial Light Modulators; SPIE Press: Bellingham, WA, USA, 2017. [Google Scholar]
- Gori, F.; Santarsiero, M.; Borghi, R.; Ramírez-Sánchez, V. Realizability condition for electromagnetic Schell-model sources. J. Opt. Soc. Am. A 2008, 25, 1016–1021. [Google Scholar] [CrossRef] [PubMed]
- Goodman, J.W. Introduction to Fourier Optics, 3rd ed.; Roberts & Company: Englewood, CO, USA, 2005. [Google Scholar]
- Hyde, M.W.; Xiao, X.; Voelz, D.G. Generating electromagnetic nonuniformly correlated beams. Opt. Lett. 2019, 44, 5719–5722. [Google Scholar] [CrossRef]
- Watkins, D.S. Fundamentals of Matrix Computations, 2nd ed.; Wiley: New York, NY, USA, 2002. [Google Scholar]
- Goodman, J.W. Statistical Optics, 2nd ed.; Wiley: Hoboken, NJ, USA, 2015. [Google Scholar]
- de Sande, J.C.G.; Martínez-Herrero, R.; Piquero, G.; Santarsiero, M.; Gori, F. Pseudo-Schell model sources. Opt. Express 2019, 27, 3963–3977. [Google Scholar] [CrossRef]
| 1.25 | |
| 1 | |
| 1 cm | |
| 1.25 cm | |
| 0.5 | |
| 3.33 mm | |
| 4.17 mm | |
| 5.12 mm |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
IV, M.W.H. Synthesizing General Electromagnetic Partially Coherent Sources from Random, Correlated Complex Screens. Optics 2020, 1, 97-113. https://doi.org/10.3390/opt1010008
IV MWH. Synthesizing General Electromagnetic Partially Coherent Sources from Random, Correlated Complex Screens. Optics. 2020; 1(1):97-113. https://doi.org/10.3390/opt1010008
Chicago/Turabian StyleIV, Milo W. Hyde. 2020. "Synthesizing General Electromagnetic Partially Coherent Sources from Random, Correlated Complex Screens" Optics 1, no. 1: 97-113. https://doi.org/10.3390/opt1010008
APA StyleIV, M. W. H. (2020). Synthesizing General Electromagnetic Partially Coherent Sources from Random, Correlated Complex Screens. Optics, 1(1), 97-113. https://doi.org/10.3390/opt1010008




