Experimental Investigation on Local and Global Texture Evolution in Drawing Seamless Copper Tubes
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Drawing Process
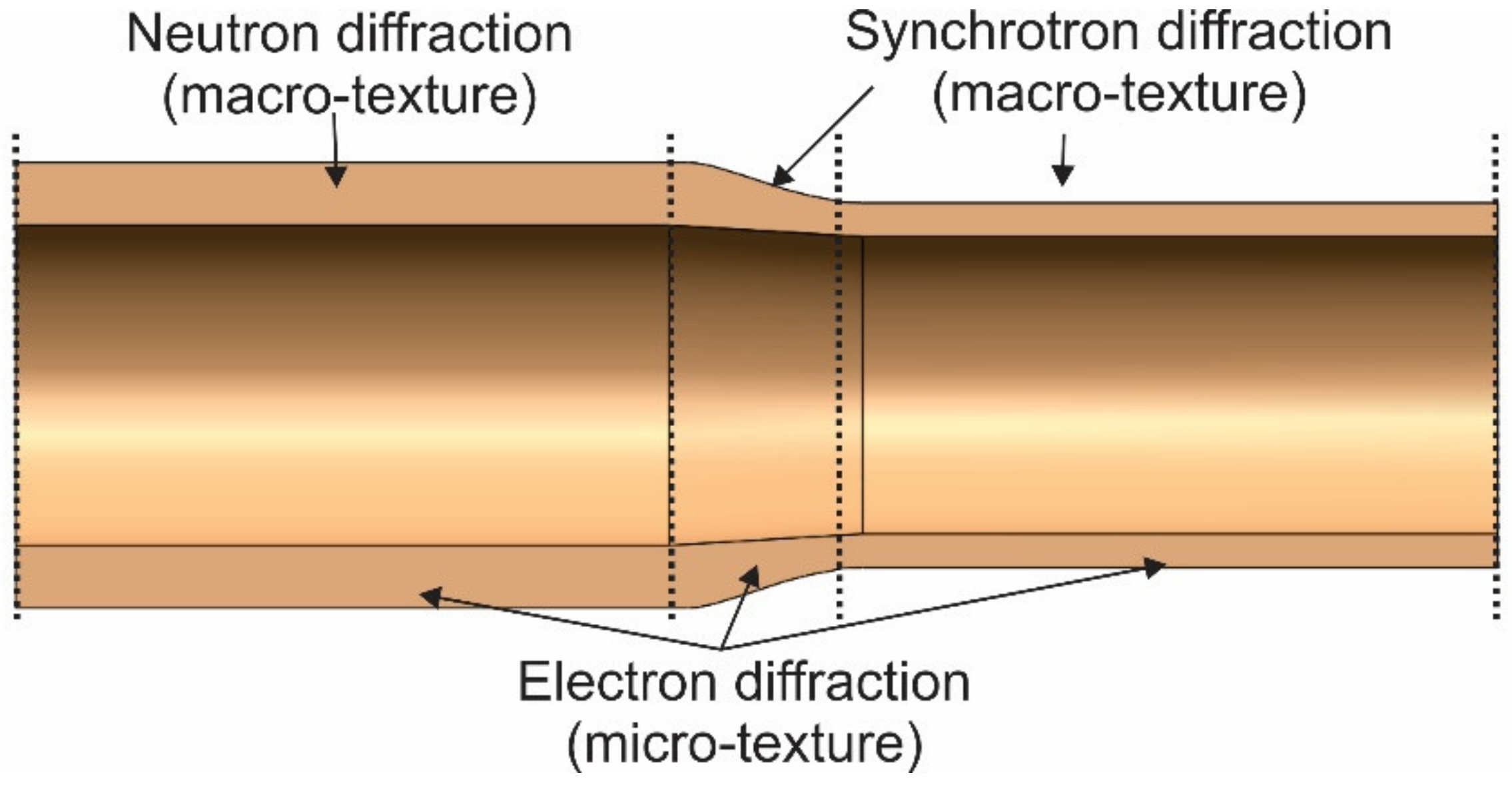
2.3. Texture Analysis
2.3.1. Texture Measurements by Neutron Diffraction
2.3.2. Measurements of Texture with Synchrotron Diffraction
2.3.3. Texture Measurements by Electron Diffraction
3. Results and Discussions
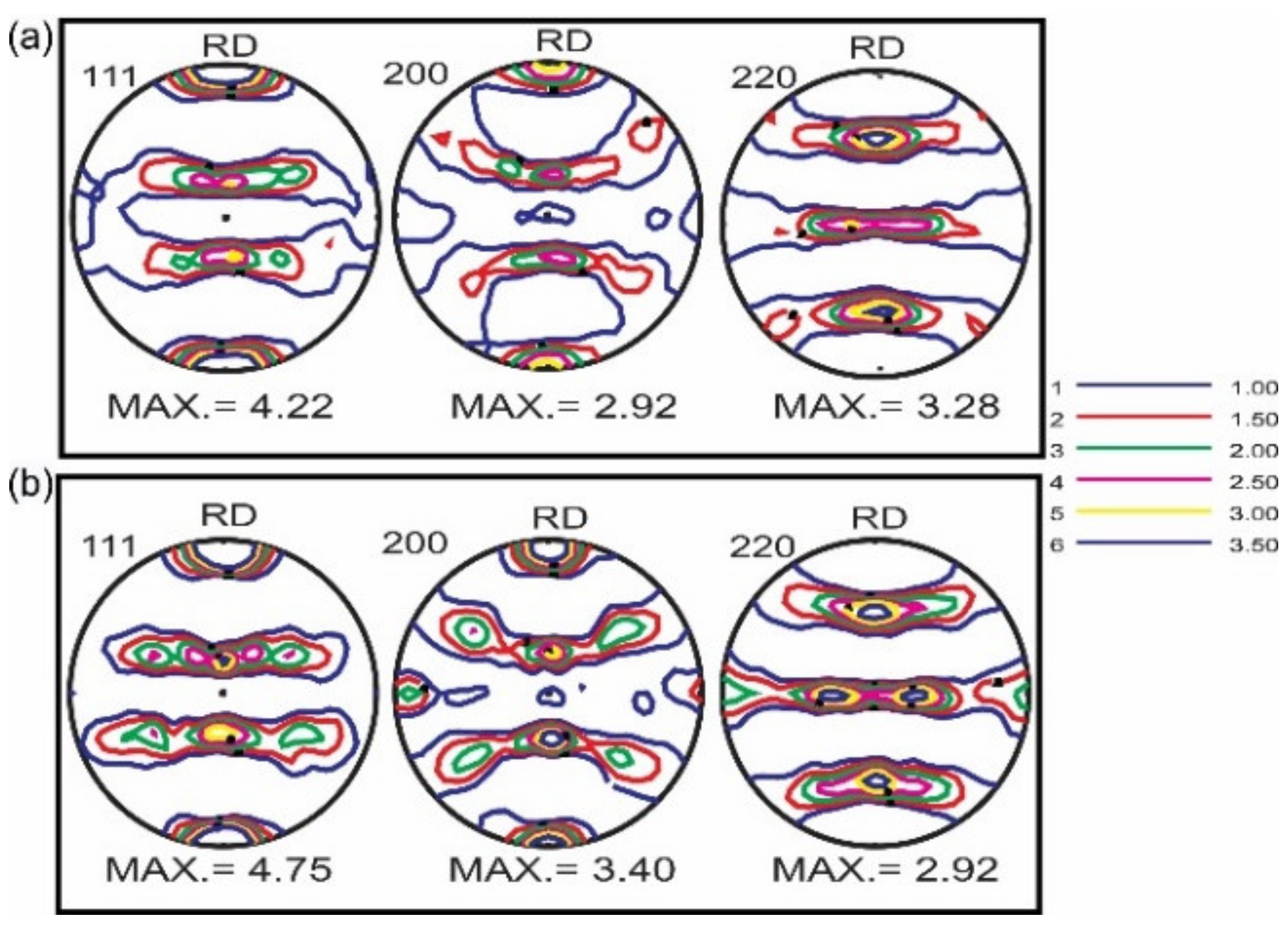
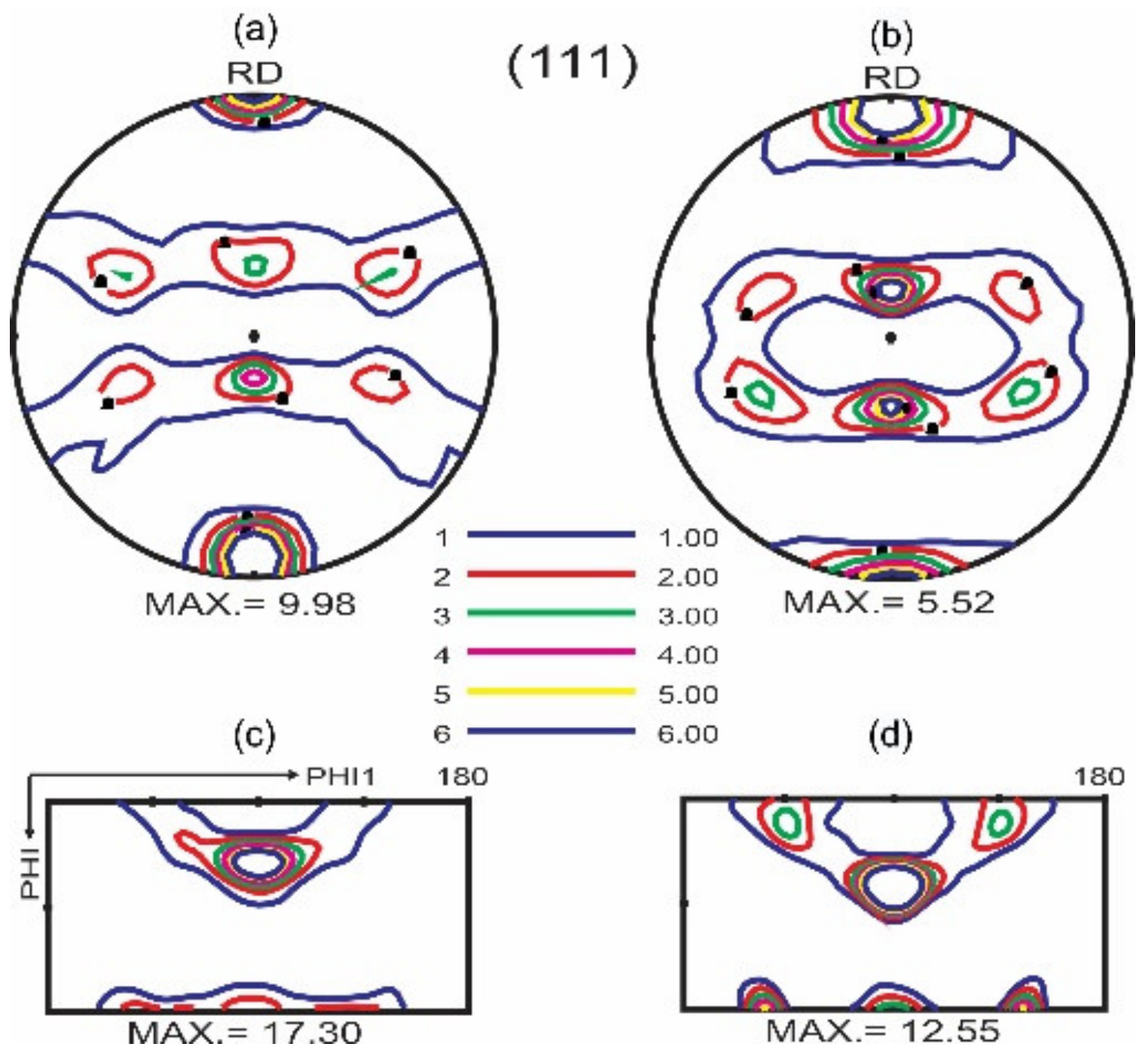
3.1. Macro-Texture Analysis
3.1.1. As-Received Tubes
3.1.2. Texture Development following the First Drawing Step
3.1.3. Texture Evolution after the Second Drawing Step
3.1.4. Texture Gradient
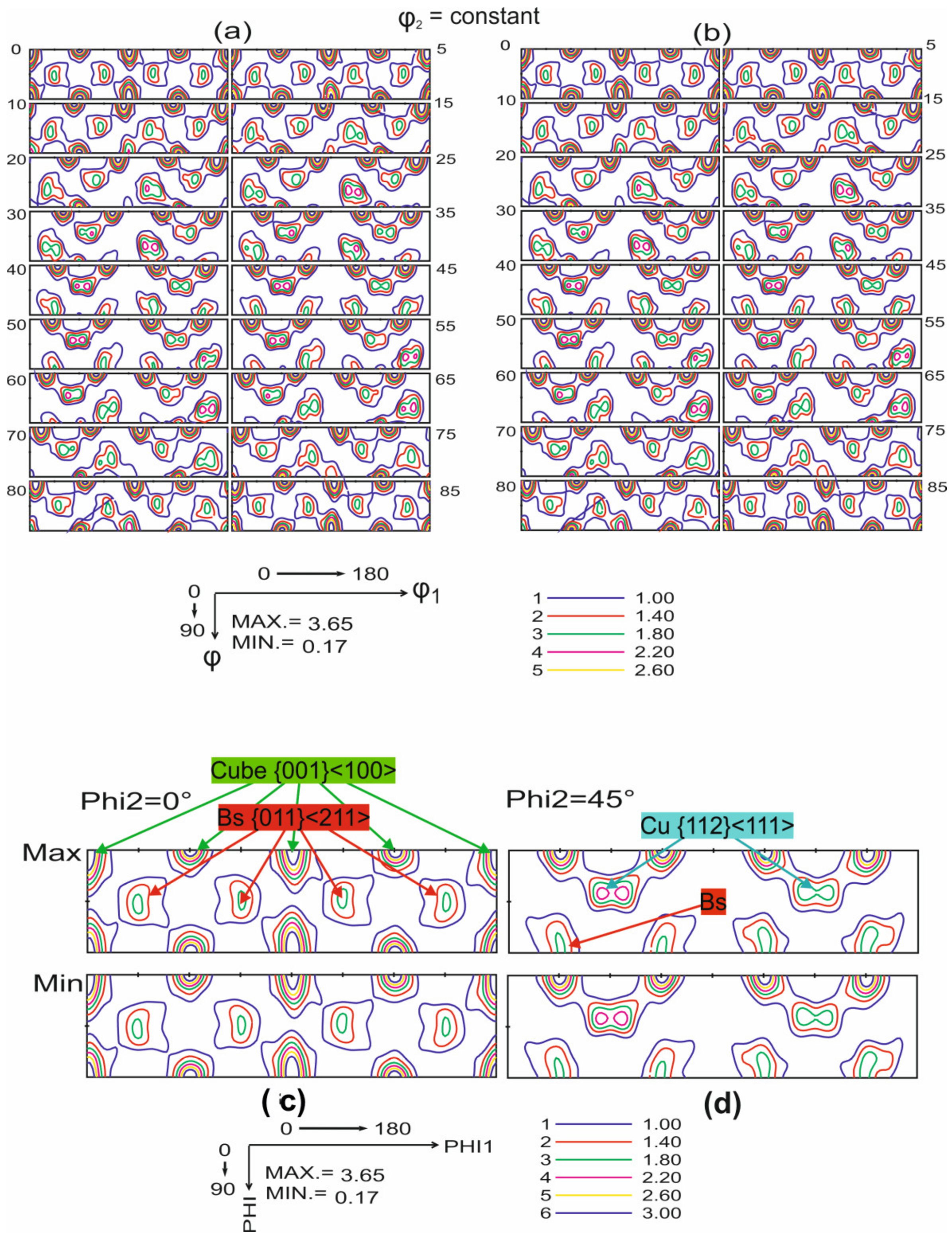
3.2. Micro-Texture Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Symbol | Definition | Unit |
| Bs | Brass orientation | [-] |
| CP | Crystal plasticity | [-] |
| Cu | Copper orientation | [-] |
| DESY | German electron synchrotron | [-] |
| E | Eccentricity | [%] |
| EBSD | Electron backscatter scanning diffraction | [-] |
| FSD | Forward scattered detector | [-] |
| IDT | Interrupted drawn tube | [-] |
| Max | Maximum | [-] |
| Min | Minimum | [-] |
| ND | Normal direction | [-] |
| ODF | Orientation distribution function | [-] |
| OIM | Orientation imaging microscopy | [-] |
| PE | Perkin Elmer | [-] |
| PF | Pole figure | [-] |
| RD | Rolling direction | [-] |
| RS | Residual stress | [MPa] |
| SGV | Sample gauge volume | [-] |
| TD | Transverse direction | [-] |
References
- Foadian, F.; Carradó, A.; Palkowski, H. Precision tube production: Influencing the eccentricity and residual stresses by tilting and shifting. J. Mater. Process. Technol. 2015, 222, 155–162. [Google Scholar] [CrossRef]
- Foadian, F.; Carradó, A.; Pirling, T.; Palkowski, H. Residual stresses evolution in Cu tubes, cold drawn with tilted dies—Neutron diffraction measurements and finite element simulation. Mater. Des. 2016, 107, 163–170. [Google Scholar] [CrossRef]
- Tenckhoff, E. Deformation Mechanisms, Texture, and Anisotropy in Zirconium and Zircaloy; ASTM: Philadelphia, PA, USA, 1988. [Google Scholar]
- Mainprice, D.; Nicolas, A. Development of shape and lattice preferred orientations: Application to the seismic anisotropy of the lower crust. J. Struct. Geol. 1989, 11, 175–189. [Google Scholar] [CrossRef]
- Engler, O.; Schäfer, C.; Brinkman, H.-J. Crystal-plasticity simulation of the correlation of microtexture and roping in AA 6xxx Al–Mg–Si sheet alloys for automotive applications. Acta Mater. 2012, 60, 5217–5232. [Google Scholar] [CrossRef]
- Suwas, S.; Ray, R.K. Crystallographic Texture of Materials; Springer: London, UK, 2014. [Google Scholar]
- Al-Hamdany, N.; Brokmeier, H.-G.; Salih, M.; Zhong, Z.; Schwebke, B.; Schell, N.; Gan, W. Crystallographic texture gradient along the wall thickness of an SF-copper tube. Mater. Charact. 2018, 139, 125–133. [Google Scholar] [CrossRef]
- Cho, J.H.; Park, S.J.; Choi, S.; Oh, K.H. Deformation Texture of Cold Drawn Al6063 Tube. Mater. Sci. Forum 2002, 408–412, 565–570. [Google Scholar] [CrossRef]
- Brokmeier, H.-G.; Carradò, A.; Al-Hamdany, N.; Pirling, T.; Wimpory, R.; Schell, N.; Palkowski, H. Texture gradient in a copper tube at maximum and minimum wall thickness. IOP Conf. Ser. Mater. Sci. Eng. 2015, 82, 12102. [Google Scholar] [CrossRef]
- Carradò, A.; Brokmeier, H.-G.; Pirling, T.; Wimpory, R.C.; Schell, N.; Palkowski, H. Development of Residual Stresses and Texture in Drawn Copper Tubes. Adv. Eng. Mater. 2013, 15, 469–475. [Google Scholar] [CrossRef]
- Schläfer, D.; Bunge, H.J. The Development of the Rolling Texture of Iron Determined by Neutron-Diffraction. Texture 1974, 1, 157–171. [Google Scholar] [CrossRef]
- Bunge, H. Three-dimensional texture analysis. Int. Mater. Rev. 1987, 32, 265–291. [Google Scholar] [CrossRef]
- Kocks, U.F.; Tomé, C.N.; Wenk, H.-R. (Eds.) Texture and Anisotropy: Preferred Orientations in Polycrystals and Their Effect on Materials Properties; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Truszkowski, W.; Krol, J.; Major, B. On Penetration of Shear Texture into the Rolled Aluminum and Copper. Met. Mater. Trans. A 1982, 13, 665–669. [Google Scholar] [CrossRef]
- Park, H.; Lee, D.N. Deformation and annealing textures of drawn Al–Mg–Si alloy tubes. J. Mater. Process. Technol. 2001, 113, 551–555. [Google Scholar] [CrossRef]
- Chen, J.; Yan, W.; Li, W.; Miao, J.; Fan, X.-H. Texture evolution and its simulation of cold drawing copper wires produced by continuous casting. Trans. Nonferrous Met. Soc. China 2011, 21, 152–158. [Google Scholar] [CrossRef]
- Hofmann, M.; Gan, W.; Rebelo-Kornmeier, J. STRESS-SPEC: Materials science diffractometer. J. Large-Scale Res. Facil. JLSRF 2015, 1, A6. [Google Scholar] [CrossRef]
- Foadian, F.; Carradó, A.; Brokmeier, H.; Gan, W.; Schell, N.; Al-Hamdany, N.; Palkowski, H. Evolution of texture in precision seamless tubes investigated by synchrotron and neutron radiation measurement. Mater. Charact. 2019, 151, 582–589. [Google Scholar] [CrossRef]
- Suwas, S.; Singh, A.K.; Narasimha Rao, K.; Singh, T. Effect of modes of rolling on evolution of texture in pure copper and some copper base alloys. Part III. Yield locus anisotropy. Z. Fuer Met. 2003, 94, 1313–1319. [Google Scholar]
- Engler, O.; Randke, V. Introduction to Texture Analysis: Macrotexture, Microtexture, and Orientation Mapping, 2nd ed.; CRC Press, Taylor & Francis Group: Boca Raton, FL, USA, 2009. [Google Scholar]
- Ridha, A.; Hutchinson, W. Recrystallisation mechanisms and the origin of cube texture in copper. Acta Met. 1982, 30, 1929–1939. [Google Scholar] [CrossRef]
- Hatherly, M.; Hutchinson, W.B. An Introduction to Textures in Metals; Chameleon Press: London, UK, 1979. [Google Scholar]
- Wassermann, G.; Grewen, J. Texturen Metallischer Werkstoffe, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 1962. [Google Scholar]





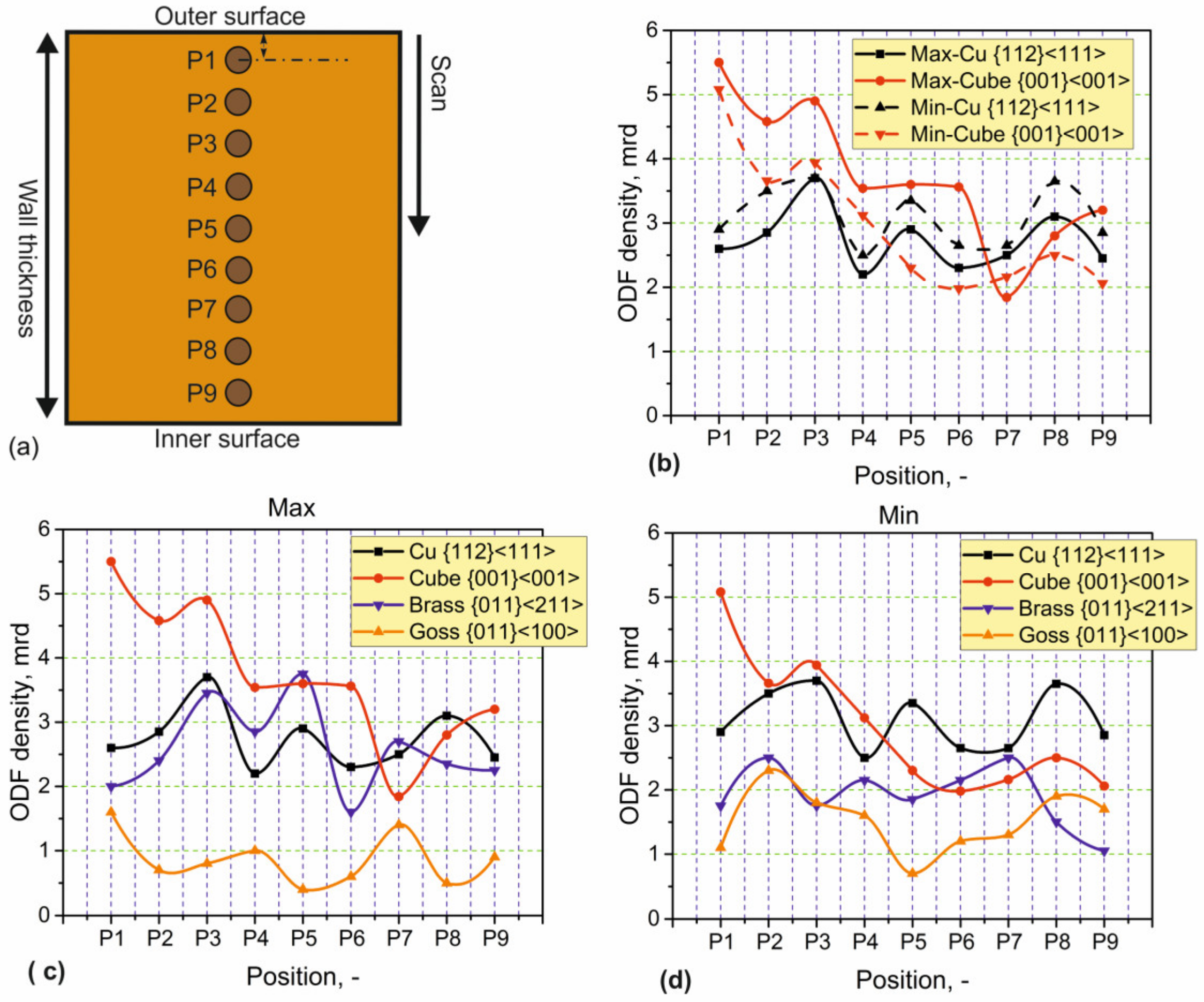




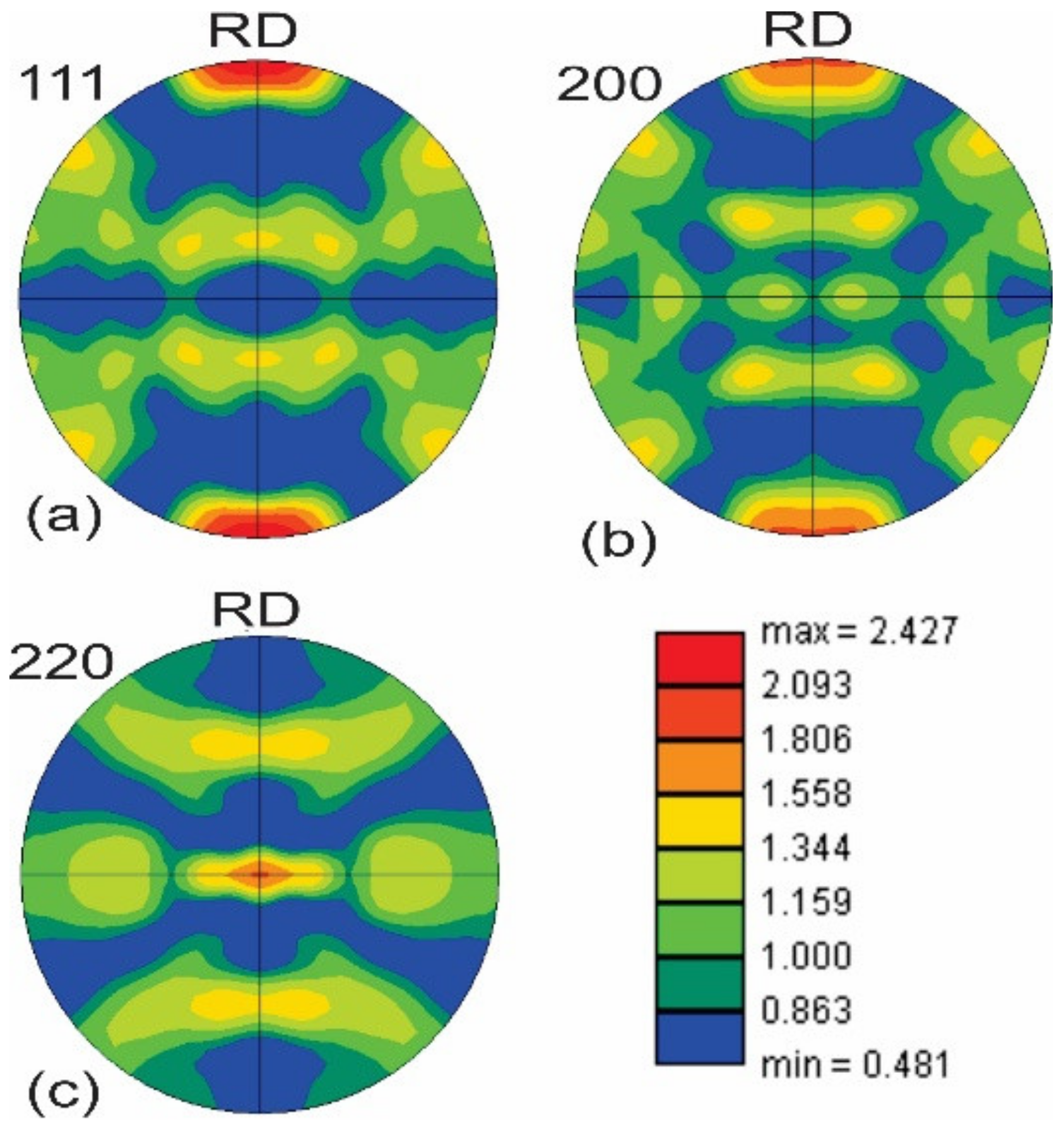

| Step | Initial Size, mm | Die ∅, mm | Plug ∅, mm | Q Value | Final Size, ∅ × t, mm | ||
|---|---|---|---|---|---|---|---|
| First | 65.0 × 5.5 | 60.0 | 51.0 | 0.20 | 0.08 | 2.5 | 60.0 × 4.5 |
| Second | 60.0 × 4.5 | 50.0 | 44.0 | 0.22 | 0.11 | 2.0 | × 4.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khani, S.; Palkowski, H.; Carradò, A.; Foadian, F. Experimental Investigation on Local and Global Texture Evolution in Drawing Seamless Copper Tubes. Appl. Mech. 2023, 4, 93-108. https://doi.org/10.3390/applmech4010007
Khani S, Palkowski H, Carradò A, Foadian F. Experimental Investigation on Local and Global Texture Evolution in Drawing Seamless Copper Tubes. Applied Mechanics. 2023; 4(1):93-108. https://doi.org/10.3390/applmech4010007
Chicago/Turabian StyleKhani, Somayeh, Heinz Palkowski, Adele Carradò, and Farzad Foadian. 2023. "Experimental Investigation on Local and Global Texture Evolution in Drawing Seamless Copper Tubes" Applied Mechanics 4, no. 1: 93-108. https://doi.org/10.3390/applmech4010007
APA StyleKhani, S., Palkowski, H., Carradò, A., & Foadian, F. (2023). Experimental Investigation on Local and Global Texture Evolution in Drawing Seamless Copper Tubes. Applied Mechanics, 4(1), 93-108. https://doi.org/10.3390/applmech4010007






