Apparent Randomness of the Normal-Force Dependence of the Coefficient of Friction between a Bare Finger and Artificial Skin under Active Tactile Exploration
Abstract
:1. Introduction
2. Methods
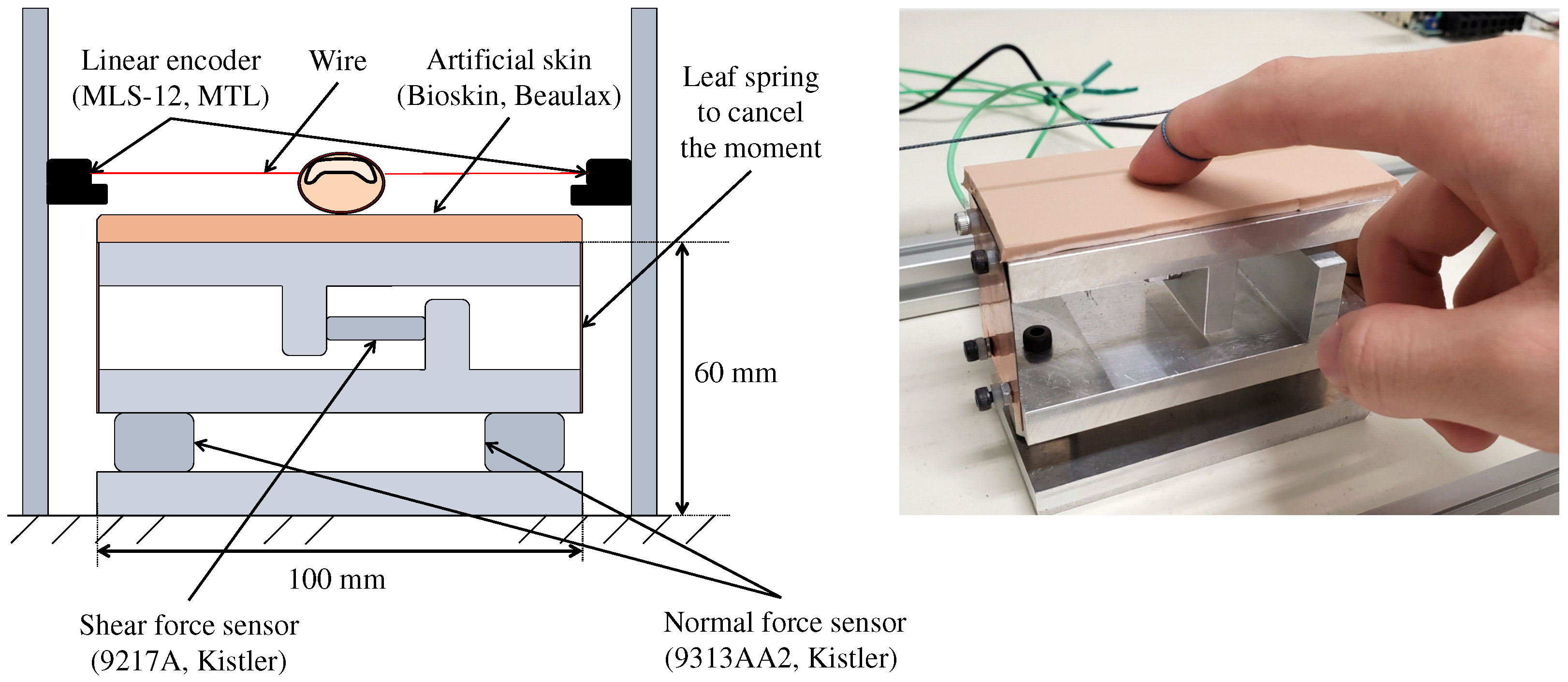
2.1. Measurement of the Two-Axial Forces and Sliding Speed
2.2. Artificial Skins Used in the Experiment
2.3. Task
2.4. Participants
2.5. Analysis
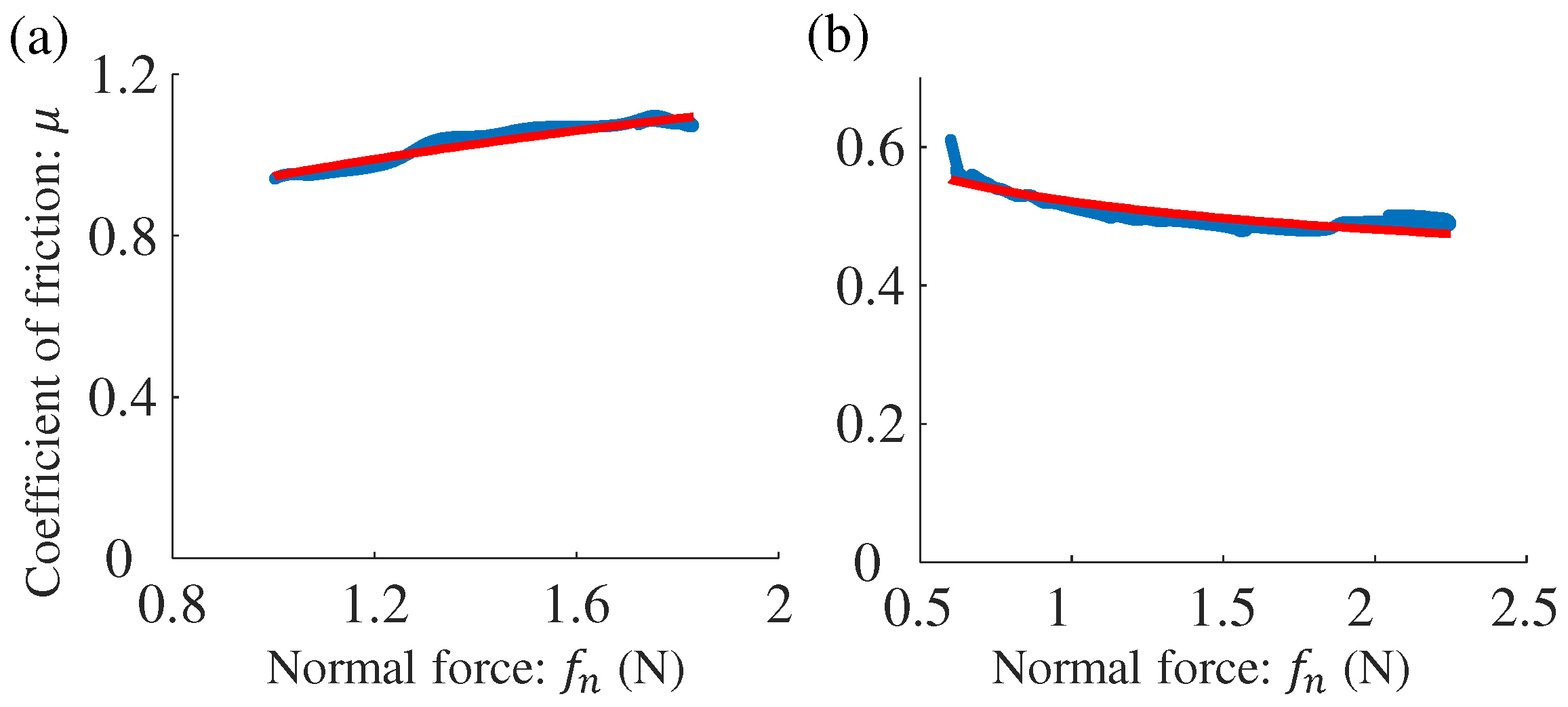
3. Results
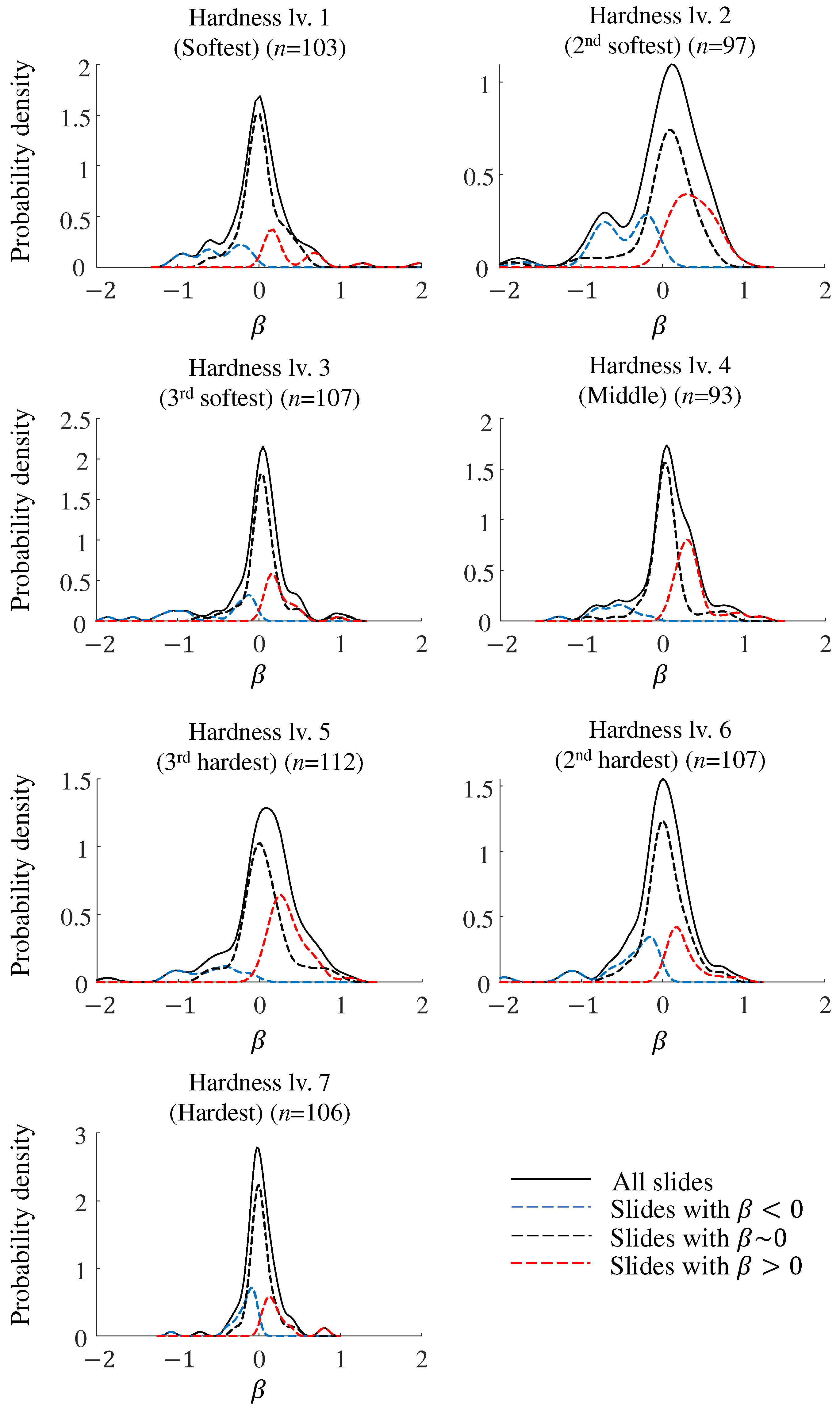
3.1. Probability Density Functions of
3.2. Second Moments and Interquartile Range of
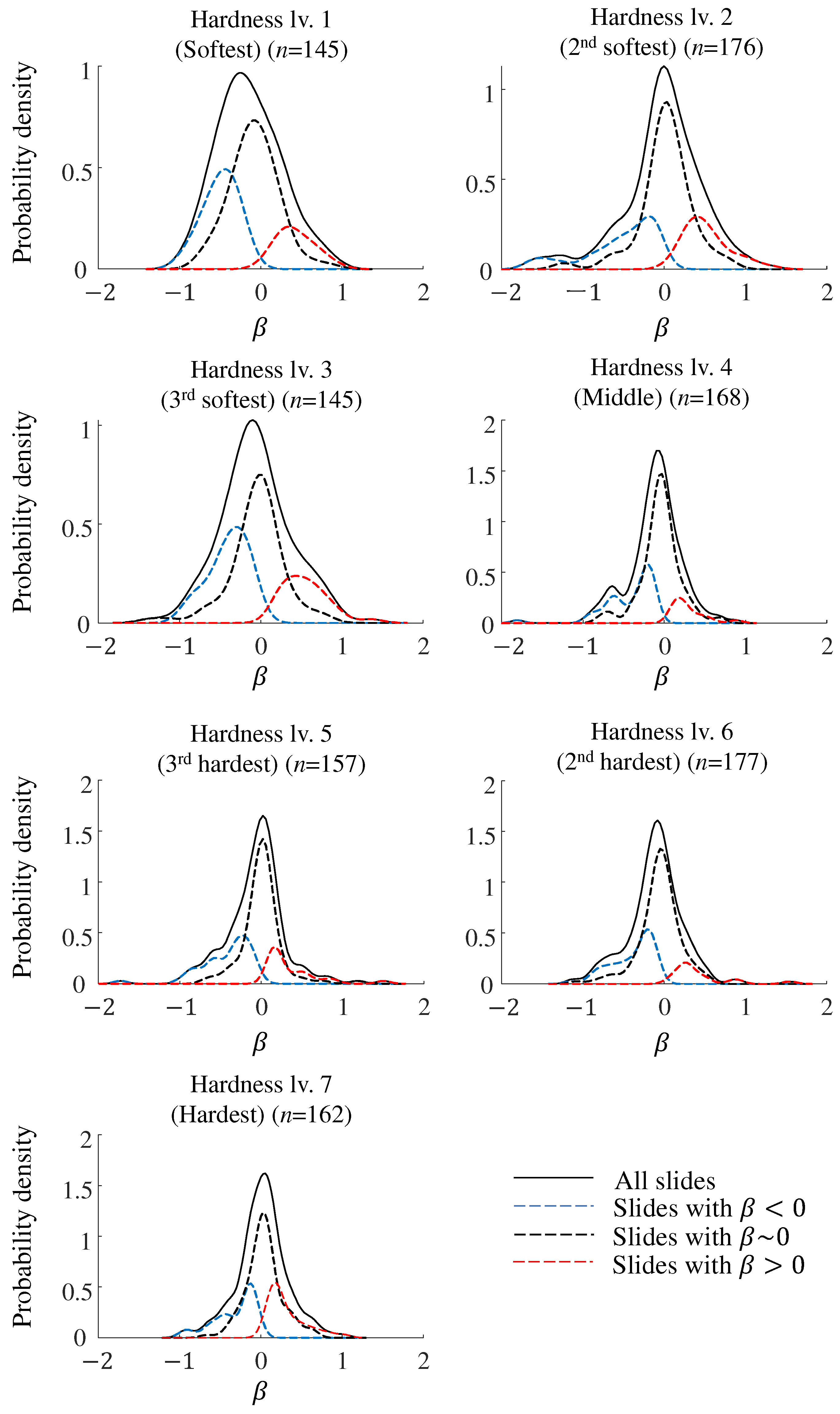
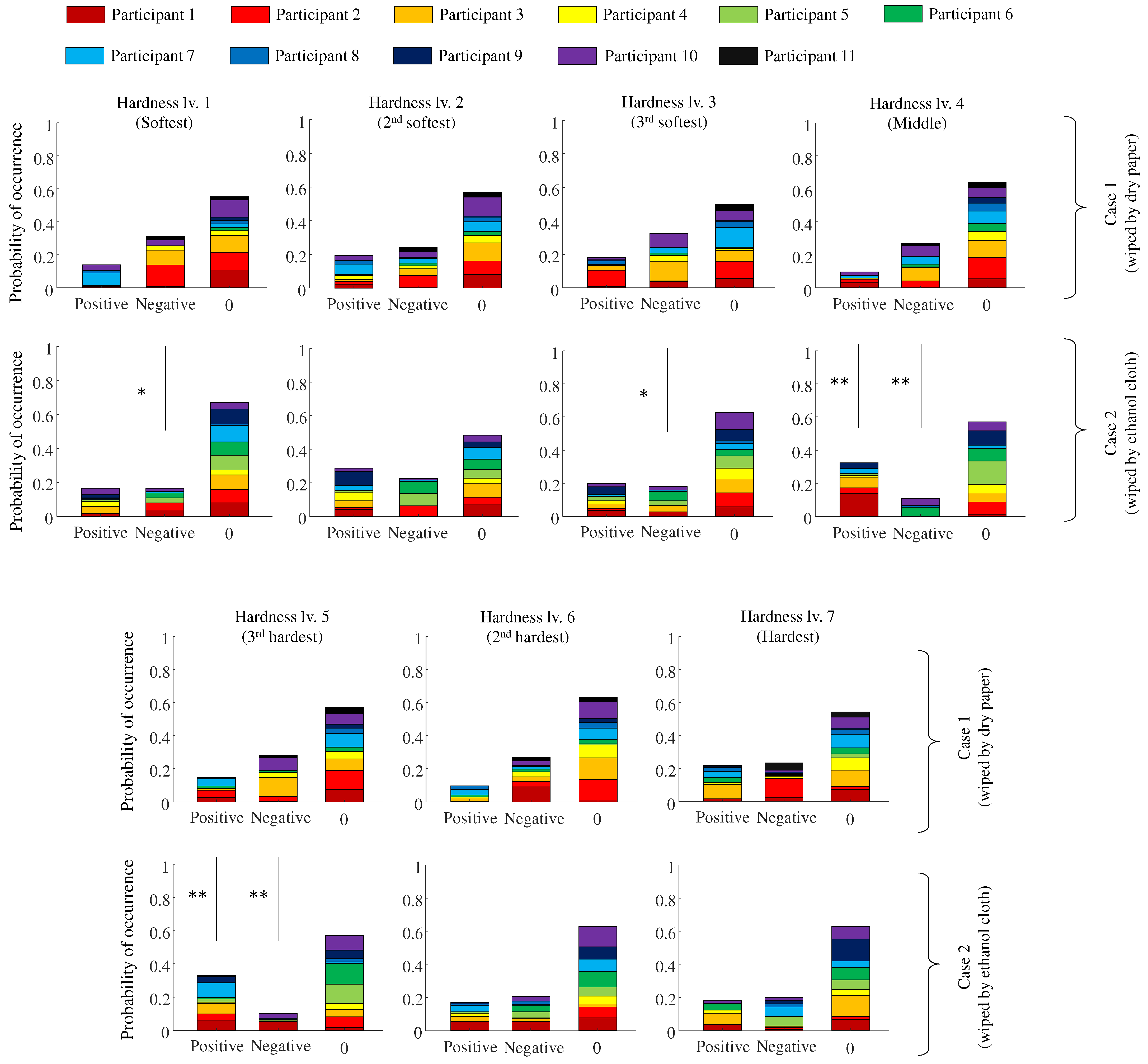
3.3. Proportions of Positive, Negative, and Insignificant Values
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Klöcker, A.; Oddo, C.M.; Camboni, D.; Penta, M.; Thonnard, J.L. Physical factors influencing pleasant touch during passive fingertip stimulation. PLoS ONE 2014, 9, e101361. [Google Scholar] [CrossRef] [PubMed]
- Klöcker, A.; Wiertlewski, M.; Théate, V.; Hayward, V.; Thonnard, J.L. Physical factors influencing pleasant touch during tactile exploration. PLoS ONE 2013, 8, e79085. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Skedung, L.; Harris, K.; Collier, E.S.; Arvidsson, M.; Wäckerlin, A.; Haag, W.; Bieri, M.; Romanyuk, A.; Rutland, M.W. Feeling Smooth: Psychotribological Probing of Molecular Composition. Tribol. Lett. 2018, 66, 138. [Google Scholar] [CrossRef] [Green Version]
- Koudine, A.A.; Barquins, M.; Anthoine, P.; Aubert, L.; Leveque, J.L. Frictional properties of skin: Proposal of a new approach. Int. J. Cosmet. Sci. 2000, 22, 11–20. [Google Scholar] [CrossRef]
- Schallamach, A. The Load Dependence of Rubber Friction. Proc. Phys. Soc. Sect. B 1952, 65, 657–661. [Google Scholar] [CrossRef]
- Zhang, M.; Mak, A. In vivo friction properties of human skin. Prosthetics Orthot. Int. 1999, 23, 135–141. [Google Scholar] [CrossRef] [Green Version]
- Adams, M.J.; Briscoe, B.J.; Johnson, S.A. Friction and lubrication of human skin. Tribol. Lett. 2007, 26, 239–253. [Google Scholar] [CrossRef]
- Derler, S.; Gerhardt, L.C. Tribology of skin: Review and analysis of experimental results for the friction coefficient of human skin. Tribol. Lett. 2012, 45, 1–27. [Google Scholar] [CrossRef] [Green Version]
- Dowson, D. Tribology and the skin surface. In Bioengineering of the Skin: Skin Imaging and Analysis, 2nd ed.; Wilhelm, K.P., Elsner, P., Berardesca, E., Maivadh, H.I., Eds.; CRC Press: Boca Raton, FL, USA, 1997; pp. 159–179. [Google Scholar]
- Greenwood, J.A.; Tabor, D. The friction of hard sliders on lubricated rubber: The importance of deformation losses. Proc. Phys. Soc. 1958, 71, 989–1001. [Google Scholar] [CrossRef]
- Derler, S.; Gerhardt, L.C.; Lenz, A.; Bertaux, E.; Hadad, M. Friction of human skin against smooth and rough glass as a function of the contact pressure. Tribol. Int. 2009, 42, 1565–1574. [Google Scholar] [CrossRef]
- Dzidek, B.; Adams, M.; Zhang, Z.; Johnson, S.; Bochereau, S.; Hayward, V. Role of occlusion in non-Coulombic slip of the finger pad. In Haptics: Neuroscience, Devices, Modeling, and Applications; Springer: Berlin/Heidelberg, Germany, 2014; pp. 109–116. [Google Scholar]
- Fagiani, R.; Massi, F.; Chatelet, E.; Costes, J.P.; Berthier, Y. Contact of a finger on rigid surfaces and textiles: Friction coefficient and induced vibrations. Tribol. Lett. 2012, 48, 145–158. [Google Scholar] [CrossRef]
- Han, H.Y.; Shimada, A.; Kawamura, S. Analysis of friction on human fingers and design of artificial fingers. In Proceedings of the IEEE International Conference on Robotics and Automation, Minneapolis, MN, USA, 22–28 April 1996; Volume 4, pp. 3061–3066. [Google Scholar]
- van Kuilenburg, J.; Masen, M.A.; van der Heide, E. A review of fingerpad contact mechanics and friction and how this affects tactile perception. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2015, 229, 243–258. [Google Scholar] [CrossRef]
- Sivamani, R.K.; Goodman, J.; Gitis, N.V.; Maibach, H.I. Friction coefficient of skin in real-time. Skin Res. Technol. 2003, 9, 235–239. [Google Scholar] [CrossRef]
- Ules, T.; Hausberger, A.; Grießer, M.; Schlögl, S.; Gruber, D.P. Introduction of a new in-situ measurement system for the study of touch-feel relevant surface properties. Polymers 2020, 12, 1380. [Google Scholar] [CrossRef] [PubMed]
- Duvefelt, K.; Olofsson, U.; Johannesson, C.M.; Skedung, L. Model for contact between finger and sinusoidal plane to evaluate adhesion and deformation component of friction. Tribol. Int. 2016, 96, 389–394. [Google Scholar] [CrossRef]
- Fujii, Y.; Okamoto, S.; Yamada, Y. Friction model of fingertip sliding over wavy surface for friction-variable tactile feedback panel. Adv. Robot. 2016, 30, 1–13. [Google Scholar] [CrossRef]
- Mahdi, D.; Riches, A.; Gester, M.; Yeomans, J.; Smith, P. Rolling and sliding: Separation of adhesion and deformation friction and their relative contribution to total friction. Tribol. Int. 2015, 89, 128–134. [Google Scholar] [CrossRef]
- Liu, X.; Carré, M.; Zhang, Q.; Lu, Z.; Matcher, S.; Lewis, R. Measuring Contact Area in a Sliding Human Finger-Pad Contact. Skin Res. Technol. 2018, 24, 31–44. [Google Scholar] [CrossRef]
- Nonomura, Y.; Ouchi, H. Friction Dynamics on Human Skin Surfaces. J. Oleo Sci. 2020, 69, 461–465. [Google Scholar] [CrossRef] [Green Version]
- Tang, W.; Zhang, J.; Chen, S.; Chen, N.; Zhu, H.; Ge, S.; Zhang, S. Tactile perception of skin and skin cream. Tribol. Lett. 2015, 59, 24. [Google Scholar] [CrossRef]
- Sakata, Y.; Mayama, H.; Nonomura, Y. Friction dynamics of moisturized human skin under non-linear motion. Int. J. Cosmet. Sci. 2021, 44, 20–29. [Google Scholar] [CrossRef] [PubMed]
- Inoue, K.; Okamoto, S.; Akiyama, Y.; Yamada, Y. Random switch of adhesion and deformation friction depending on material hardness. In Proceedings of the IEEE 2nd Global Conference on Life Sciences and Technologies, Kyoto, Japan, 10–12 March 2020; pp. 204–206. [Google Scholar]
- Pasumarty, S.M.; Johnson, S.A.; Watson, S.A.; Adams, M.J. Friction of the Human Finger Pad: Influence of Moisture, Occlusion and Velocity. Tribol. Lett. 2011, 44, 117–137. [Google Scholar] [CrossRef]
- Tomlinson, S.E.; Lewis, R.; Liu, X.; Texier, C.; Carré, M. Understanding the Friction Mechanisms between the Human Finger and Flat Contacting Surfaces in Moist Conditions. Tribol. Lett. 2011, 41, 283–294. [Google Scholar] [CrossRef] [Green Version]
- Inoue, K.; Okamoto, S.; Akiyama, Y.; Yamada, Y. Effect of Material Hardness on Friction between a Bare Finger and Dry and Lubricated Artificial Skin. IEEE Trans. Haptics 2020, 13, 123–129. [Google Scholar] [CrossRef] [PubMed]
- Hasegawa, H.; Okamoto, S.; Yamada, Y. Phase difference between normal and shear forces during tactile exploration represents textural features. IEEE Trans. Haptics 2020, 13, 11–17. [Google Scholar] [CrossRef] [PubMed]
- Shimizu, R.; Nonomura, Y. Preparation of artificial skin that mimics human skin surface and mechanical properties. J. Oleo Sci. 2018, 67, 47–54. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tanaka, Y.; Bergmann Tiest, W.M.; Kappers, A.M.L.; Sano, A. Contact force and scanning velocity during active roughness perception. PLoS One 2014, 9, e93363. [Google Scholar] [CrossRef]
- Adams, M.J.; Johnson, S.A.; Lefèvre, P.; Lévesque, V.; Hayward, V.; André, T.; Thonnard, J.L. Finger pad friction and its role in grip and touch. J. R. Soc. Interface 2013, 10, 20120467. [Google Scholar] [CrossRef] [Green Version]
- Cohen, J. Statistical Power Analysis for the Behavioral Sciences, 2nd ed.; Lawrence Erlbaum Associates: Mahwah, NJ, USA, 1988. [Google Scholar]
- Mukaka, M.M. Statistics corner: A guide to appropriate use of correlation coefficient in medical research. Malawi Med. J. 2012, 24, 69–71. [Google Scholar]
- Persson, B.N.J.; Kovalev, A.; Gorb, S.N. Contact mechanics and friction on dry and wet human skin. Tribol. Lett. 2013, 50, 17–30. [Google Scholar] [CrossRef]
- Dzidek, B.M.; Adams, M.J.; Andrews, J.W.; Zhang, Z.; Johnson, S.A. Contact mechanics of the human finger pad under compressive loads. J. R. Soc. Interface 2017, 14, 20160935. [Google Scholar] [CrossRef] [PubMed]
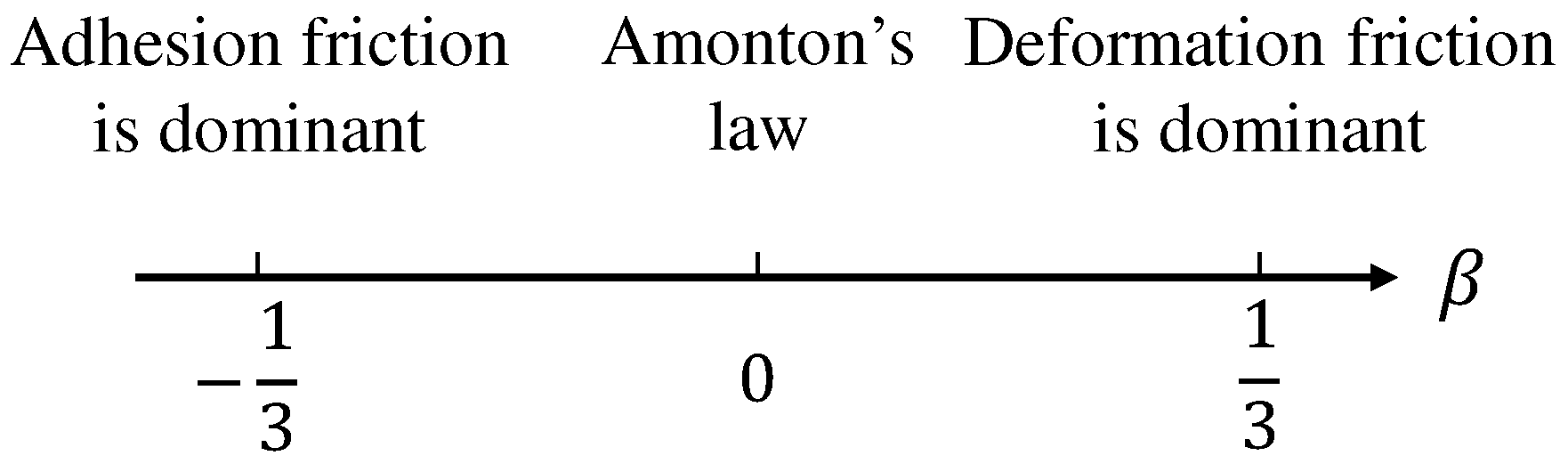


| Hardness level | 1 | 2 | 3 | 4 |
| Shore AO Hardness | 3.2 | 7.8 | 9.6 | 11.0 |
| E (kPa) | 69.4 | 74.2 | 79.6 | 85.2 |
| Hardness level | 5 | 6 | 7 | Finger |
| Shore AO Hardness | 16.9 | 18.0 | 19.1 | 7.9 ± 2.1 |
| E (kPa) | 122.4 | 131.7 | 141.8 | 74.4 ± 18.6 |
| Lv. | AO Hardness | Case 1 | Case 2 |
|---|---|---|---|
| 1 | 3.2 | 0.15 (0.54) | 0.32 (0.30) |
| 2 | 7.8 | 0.24 (0.47) | 0.23 (0.50) |
| 3 | 9.6 | 0.19 (0.50) | 0.19 (0.23) |
| 4 | 11.0 | 0.11 (0.29) | 0.15 (0.31) |
| 5 | 16.9 | 0.15 (0.35) | 0.18 (0.36) |
| 6 | 18.0 | 0.12 (0.33) | 0.15 (0.32) |
| 7 | 19.1 | 0.10 (0.31) | 0.053 (0.18) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Inoue, K.; Okamoto, S.; Akiyama, Y.; Yamada, Y. Apparent Randomness of the Normal-Force Dependence of the Coefficient of Friction between a Bare Finger and Artificial Skin under Active Tactile Exploration. Appl. Mech. 2022, 3, 649-662. https://doi.org/10.3390/applmech3020038
Inoue K, Okamoto S, Akiyama Y, Yamada Y. Apparent Randomness of the Normal-Force Dependence of the Coefficient of Friction between a Bare Finger and Artificial Skin under Active Tactile Exploration. Applied Mechanics. 2022; 3(2):649-662. https://doi.org/10.3390/applmech3020038
Chicago/Turabian StyleInoue, Koki, Shogo Okamoto, Yasuhiro Akiyama, and Yoji Yamada. 2022. "Apparent Randomness of the Normal-Force Dependence of the Coefficient of Friction between a Bare Finger and Artificial Skin under Active Tactile Exploration" Applied Mechanics 3, no. 2: 649-662. https://doi.org/10.3390/applmech3020038
APA StyleInoue, K., Okamoto, S., Akiyama, Y., & Yamada, Y. (2022). Apparent Randomness of the Normal-Force Dependence of the Coefficient of Friction between a Bare Finger and Artificial Skin under Active Tactile Exploration. Applied Mechanics, 3(2), 649-662. https://doi.org/10.3390/applmech3020038





