Evaluating Angled Abutments: Three-Dimensional Finite Element Stress Analysis of Anterior Maxillary Implants
Abstract
1. Introduction
2. Materials and Methods
2.1. Modelling
2.2. Meshing and Boundary Conditions
3. Results
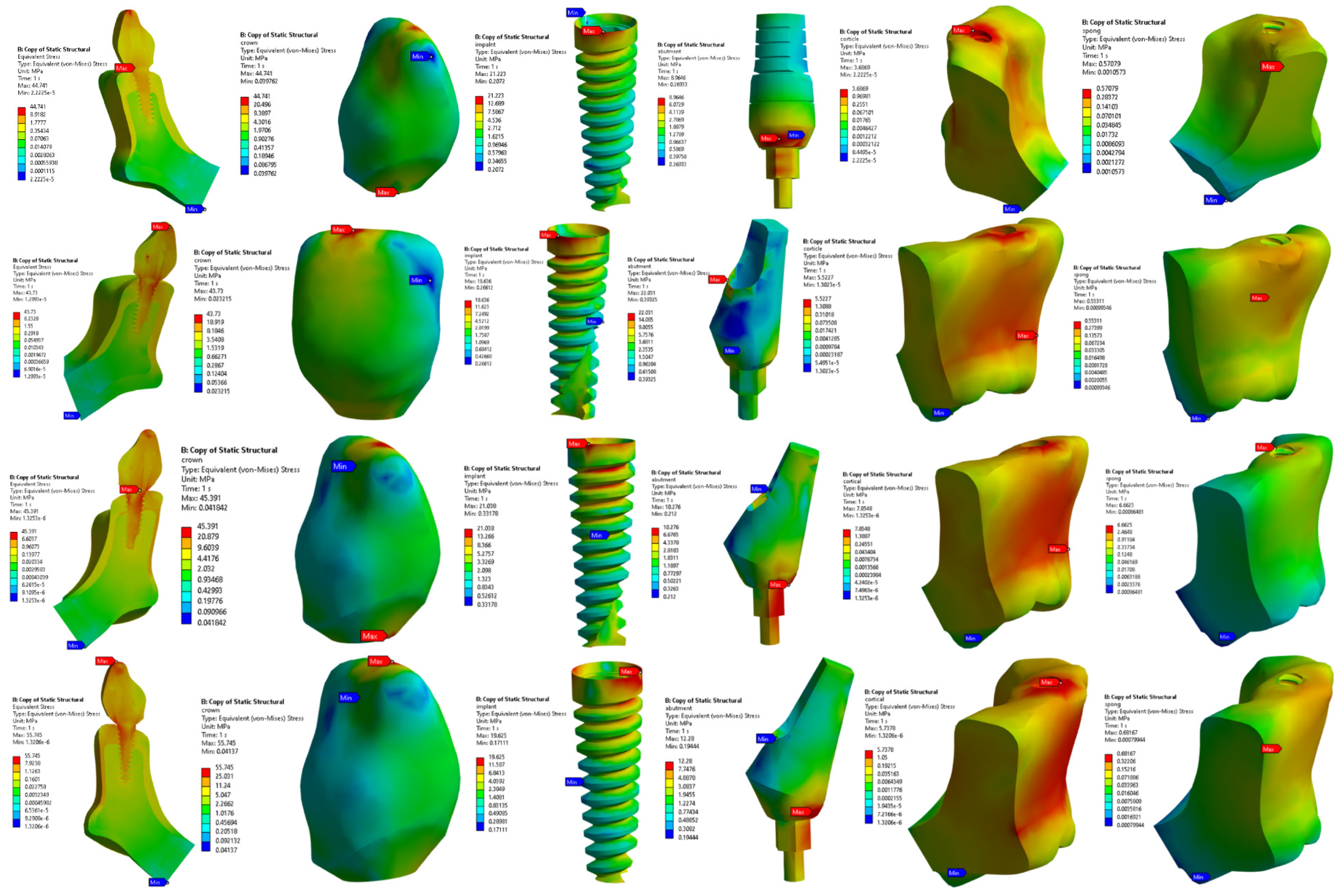
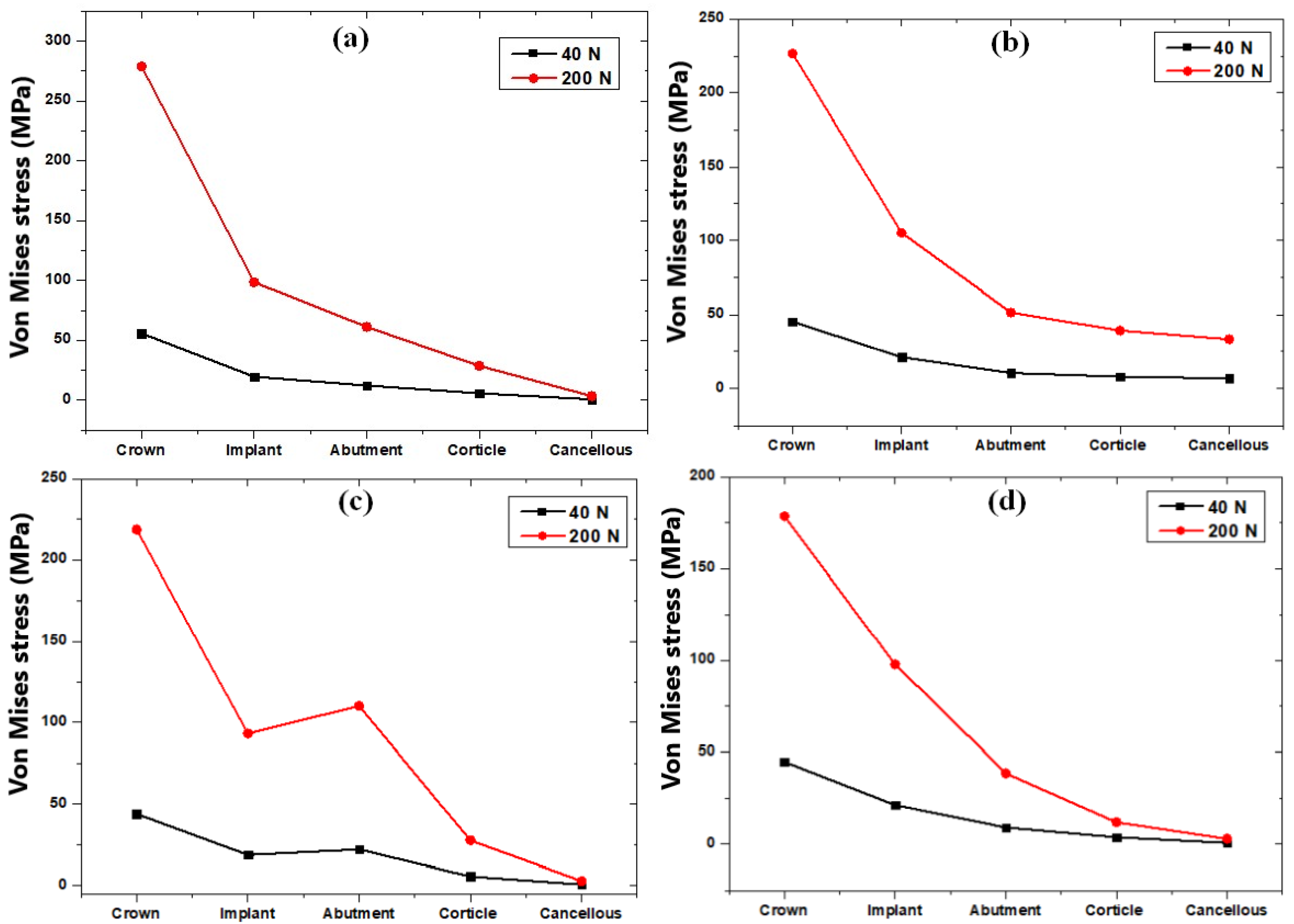
3.1. Stress Distribution in the Implant System
3.2. Validation of the Obtained Result with the Literature
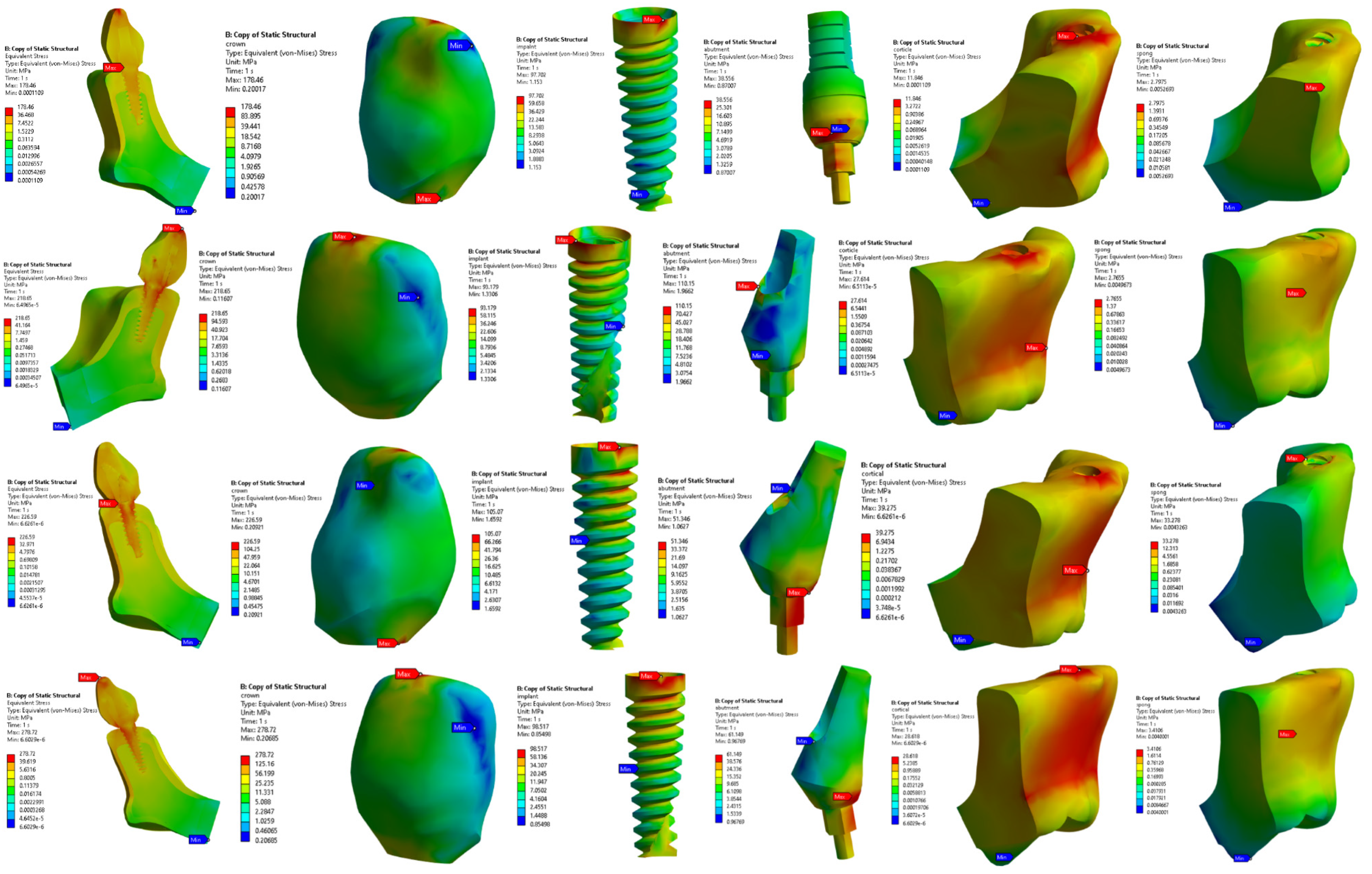
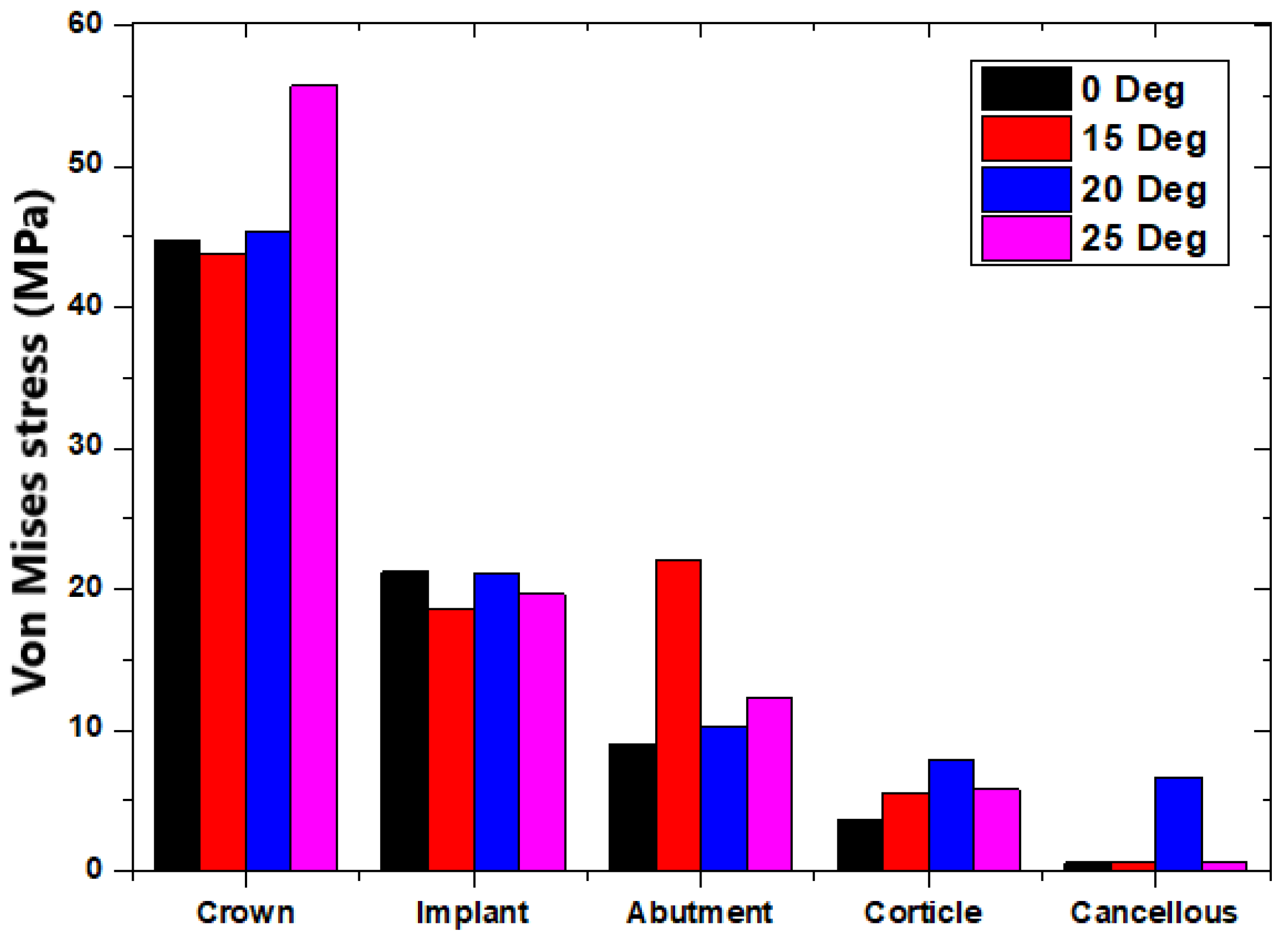
3.3. Comparison of Different Loading Conditions on the Angled Abutment
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shortall, A.C.; Shaini, F.J. A study into the performance of a gallium-based restorative material. Br. Dent. J. 2000, 189, 306. [Google Scholar] [CrossRef]
- Shehab, N.M.; Abdelhady, A.A.; Noaman, K.M.; Tawfeek, H.E.M. 3D finite element analysis assessment of maxillary premolar restored with various restorative materials. Int. J. Health Sci. 2022, 6, 907–920. [Google Scholar] [CrossRef]
- Pacheco, R.R.; Vitti, R.P.; Andrada, A.C.; Martins, C.M.; Catelan, A.; Khurshid, Z. Restorative materials used in endodontics. In Biomaterials in Endodontics; Elsevier: Amsterdam, The Netherlands, 2022; pp. 99–112. [Google Scholar]
- Frese, C.; Leciejewski, F.; Specht, R.; Wohlrab, T.; Büsch, C.; Boemicke, W.; Probst, K.; Katsikogianni, E.N.; Wolff, D. The dental esthetic screening index: A new tool for assessment of dento-facial esthetics in restorative dentistry. J. Esthet. Restor. Dent. 2019, 31, 572–582. [Google Scholar] [CrossRef] [PubMed]
- Saeed, F.; Muhammad, N.; Khan, A.S.; Sharif, F.; Rahim, A.; Ahmad, P.; Irfan, M. Prosthodontics dental materials: From conventional to unconventional. Mater. Sci. Eng. C 2020, 106, 110167. [Google Scholar] [CrossRef]
- Le Révérend, B.J.D.; Edelson, L.R.; Loret, C. Anatomical, functional, physiological and behavioural aspects of the development of mastication in early childhood. Br. J. Nutr. 2014, 111, 403–414. [Google Scholar] [CrossRef]
- Heinemann, F.; Mundt, T.; Biffar, R. Retrospective evaluation of temporary cemented, tooth and implant supported fixed partial dentures. J. Craniomaxillofac. Surg. 2006, 34 (Suppl. 2), 86–90. [Google Scholar] [CrossRef]
- Mozaffari, A.; Hashtbaran, D.; Moghadam, A.; Aalaei, S. Stress Distribution in Peri-implant Bone in the Replacement of Molars with One or Two Implants: A Finite Element Analysis. J. Dent. 2023, 24, 132–137. [Google Scholar] [CrossRef]
- Hebel, K.; Gajjar, R.; Hofstede, T. Single-tooth replacement: Bridge vs. implant-supported restoration. J. Can. Dent. Assoc. 2000, 66, 435–438. [Google Scholar]
- Coutsiers Morell, G.F.; Berlin-Broner, Y.; Flores-Mir, C.; Heo, G. Tooth and root size as determined from 0.25- and 0.30-mm voxel size cone-beam CT imaging when contrasted to micro-CT scans (0.06 mm): An ex vivo study. J. Orthod. 2022, 49, 174–178. [Google Scholar] [CrossRef] [PubMed]
- Xia, Z.; Gan, Y.; Chang, L.; Xiong, J.; Zhao, Q. Individual tooth segmentation from CT images scanned with contacts of maxillary and mandible teeth. Comput. Methods Programs Biomed. 2017, 138, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Tian, K.; Chen, J.; Han, L.; Yang, J.; Huang, W.; Wu, D. Angled abutments result in increased or decreased stress on surrounding bone of single-unit dental implants: A finite element analysis. Med. Eng. Phys. 2012, 34, 1526–1531. [Google Scholar] [CrossRef]
- Mesnard, M.; Ramos, A.; Ballu, A.; Morlier, J.; Cid, M.; Simoes, J.A. Biomechanical analysis comparing natural and alloplastic temporomandibular joint replacement using a finite element model. J. Oral Maxillofac. Surg. 2011, 69, 1008–1017. [Google Scholar] [CrossRef] [PubMed]
- Turkyilmaz, I. Restoring severely angled implants with custom abutments and a screw-retained fixed dental prosthesis. J. Dent. Sci. 2019, 14, 107–108. [Google Scholar] [CrossRef] [PubMed]
- Gutmann, C.; Shaikh, N.; Shenoy, B.S.; Shaymasunder Bhat, N.; Keni, L.G. Chethan Wear estimation of hip implants with varying chamfer geometry at the trunnion junction: A finite element analysis. Biomed. Phys. Eng. Express 2023, 9, 035004. [Google Scholar] [CrossRef] [PubMed]
- Shaikh, N.; Shenoy, B.S.; Bhat, N.S.; Shetty, S.; Chethan, K.N. Wear estimation at the contact surfaces of oval shaped hip implants using finite element analysis. Cogent Eng. 2023, 10, 222298. [Google Scholar] [CrossRef]
- Göktaş, H.; Subaşi, E.; Uzkut, M.; Kara, M.; Biçici, H.; Shirazi, H.; Chethan, K.N.; Mihçin, Ş. Optimization of Hip Implant Designs Based on Its Mechanical Behaviour; Springer: Berlin/Heidelberg, Germany, 2022; pp. 37–43. [Google Scholar]
- Keni, L.G.; Hayoz, M.J.; Mohammad, S.; Khader, A.; Hegde, P.; Prakashini, K.; Tamagawa, M.; Satish Shenoy, B.; Hameed, B.M.Z.; Zuber, M. Computational Flow Analysis of a Single Peristaltic Wave Propagation in the Ureter. Comput. Methods Programs Biomed. 2021, 210, 106378. [Google Scholar] [CrossRef] [PubMed]
- Baggi, L.; Cappelloni, I.; Di Girolamo, M.; Maceri, F.; Vairo, G. The influence of implant diameter and length on stress distribution of osseointegrated implants related to crestal bone geometry: A three-dimensional finite element analysis. J. Prosthet. Dent. 2008, 100, 422–431. [Google Scholar] [CrossRef]
- Sannino, G.; Pozzi, A.; Schiavetti, R.; Barlattani, A. Stress distribution on a three-unit implant-supported zirconia framework. A 3D finite element analysis and fatigue test. Oral Implantol. 2012, 5, 11–20. [Google Scholar]
- Cibirka, R.M.; Razzoog, M.E.; Lang, B.R.; Stohler, C.S. Determining the force absorption quotient for restorative materials used in implant occlusal surfaces. J. Prosthet. Dent. 1992, 67, 361–364. [Google Scholar] [CrossRef]
- Pellizzer, E.P.; Verri, F.R.; de Moraes, S.L.D.; Falcón-Antenucci, R.M.; de Carvalho, P.S.P.; Noritomi, P.Y. Influence of the Implant Diameter With Different Sizes of Hexagon: Analysis by 3-Dimensional Finite Element Method. J. Oral Implantol. 2013, 39, 425–431. [Google Scholar] [CrossRef]
- Morneburg, T.R.; Pröschel, P.A. Predicted incidence of occlusal errors in centric closing around arbitrary axes. Int. J. Prosthodont. 2002, 15, 358–364. [Google Scholar]
- Costa, C.; Peixinho, N.; Silva, J.P.; Carvalho, S. Study and characterization of the crest module design: A 3D finite element analysis. J. Prosthet. Dent. 2015, 113, 541–547. [Google Scholar] [CrossRef]
- Faegh, S.; Müftü, S. Load transfer along the bone-dental implant interface. J. Biomech. 2010, 43, 1761–1770. [Google Scholar] [CrossRef]
- Leblebicioğlu Kurtuluş, I.; Kilic, K.; Bal, B.; Kilavuz, A. Finite Element Analysis of the Stress Distribution Associated With Different Implant Designs for Different Bone Densities. J. Prosthodont. 2022, 31, 614–622. [Google Scholar] [CrossRef] [PubMed]
- Datte, C.-E.; Tribst, J.-P.-M.; Dal Piva, A.-M.O.; Nishioka, R.-S.; Bottino, M.-A.; Evangelhista, A.-D.M.; de Monteiro, F.M.; Borges, A.-L.-S. Influence of different restorative materials on the stress distribution in dental implants. J. Clin. Exp. Dent. 2018, 10, e439–e444. [Google Scholar] [CrossRef] [PubMed]
- Cardelli, P.; Montani, M.; Gallio, M.; Biancolini, M.; Brutti, C.; Barlattani, A. Angulated abutments and perimplants stress: F.E.M. analysis. Oral Implantol. 2009, 2, 3–10. [Google Scholar]
- Alemayehu, D.B.; Jeng, Y.R. Three-dimensional finite element investigation into effects of implant thread design and loading rate on stress distribution in dental implants and anisotropic bone. Materials 2021, 14, 6974. [Google Scholar] [CrossRef] [PubMed]
- Kapoor, S.; Rodrigues, S.; Mahesh, M.; Shetty, T.; Pai, U.; Saldanha, S.; Hedge, P.; Shenoy, S. Evaluation of Stress Generated with Different Abutment Materials and Angulations under Axial and Oblique Loading in the Anterior Maxilla: Three-Dimensional Finite Element Analysis. Int. J. Dent. 2021, 2021, 9205930. [Google Scholar] [CrossRef] [PubMed]
- Jo, L.; Vamsi, K.; Ariga, P.; Bholla, P. Influence of occlusal loading on stress patterns at the bone-implant interface by angulated abutments in the anterior maxilla: A three-dimensional finite-element study. J. Dent. Implant. 2014, 4, 3. [Google Scholar] [CrossRef]
- Kao, H.-C.; Gung, Y.-W.; Chung, T.-F.; Hsu, M.-L. The influence of abutment angulation on micromotion level for immediately loaded dental implants: A 3-D finite element analysis. Int. J. Oral Maxillofac. Implants 2008, 23, 623–630. [Google Scholar]
- Clelland, N.L.; Lee, J.K.; Bimbenet, O.C.; Brantley, W.A. A Three-Dimensional Finite Element Stress Analysis of Angled Abutments for an Implant Placed in the Anterior Maxilla. J. Prosthodont. 1995, 4, 95–100. [Google Scholar] [CrossRef] [PubMed]
- Papavasiliou, G.; Kamposiora, P.; Bayne, S.C.; Felton, D.A. Three-dimensional finite element analysis of stress-distribution around single tooth implants as a function of bony support, prosthesis type, and loading during function. J. Prosthet. Dent. 1996, 76, 633–640. [Google Scholar] [CrossRef] [PubMed]
- Wu, D.; Tian, K.; Chen, J.; Jin, H.; Huang, W.; Liu, Y. A Further Finite Element Stress Analysis of Angled Abutments for an Implant Placed in the Anterior Maxilla. Comput. Math. Methods Med. 2015, 2015, 560645. [Google Scholar] [CrossRef] [PubMed]
- Chun, H.-J.; Shin, H.-S.; Han, C.-H.; Lee, S.-H. Influence of implant abutment type on stress distribution in bone under various loading conditions using finite element analysis. Int. J. Oral Maxillofac. Implants 2006, 21, 195–202. [Google Scholar]
- Lin, C.-L.; Wang, J.-C.; Ramp, L.C.; Liu, P.-R. Biomechanical response of implant systems placed in the maxillary posterior region under various conditions of angulation, bone density, and loading. Int. J. Oral Maxillofac. Implants 2008, 23, 57–64. [Google Scholar]
- Badalia, I.; Kumar, M.; Bansal, A.; Mehra, S.; Batra, R. Evaluation of Stress Patterns in Bone Around Implants for Different Abutment Angulations Under Axial and Oblique Loading in Anterior Maxillary Region—A Finite Element Analysis. Dent. J. Adv. Stud. 2020, 8, 60–64. [Google Scholar] [CrossRef]
- Mahajan, S.; Patil, R. Application of finite element analysis to optimizing dental implant. Int. Res. J. Eng. Technol. 2016, 03, 850–856. [Google Scholar]
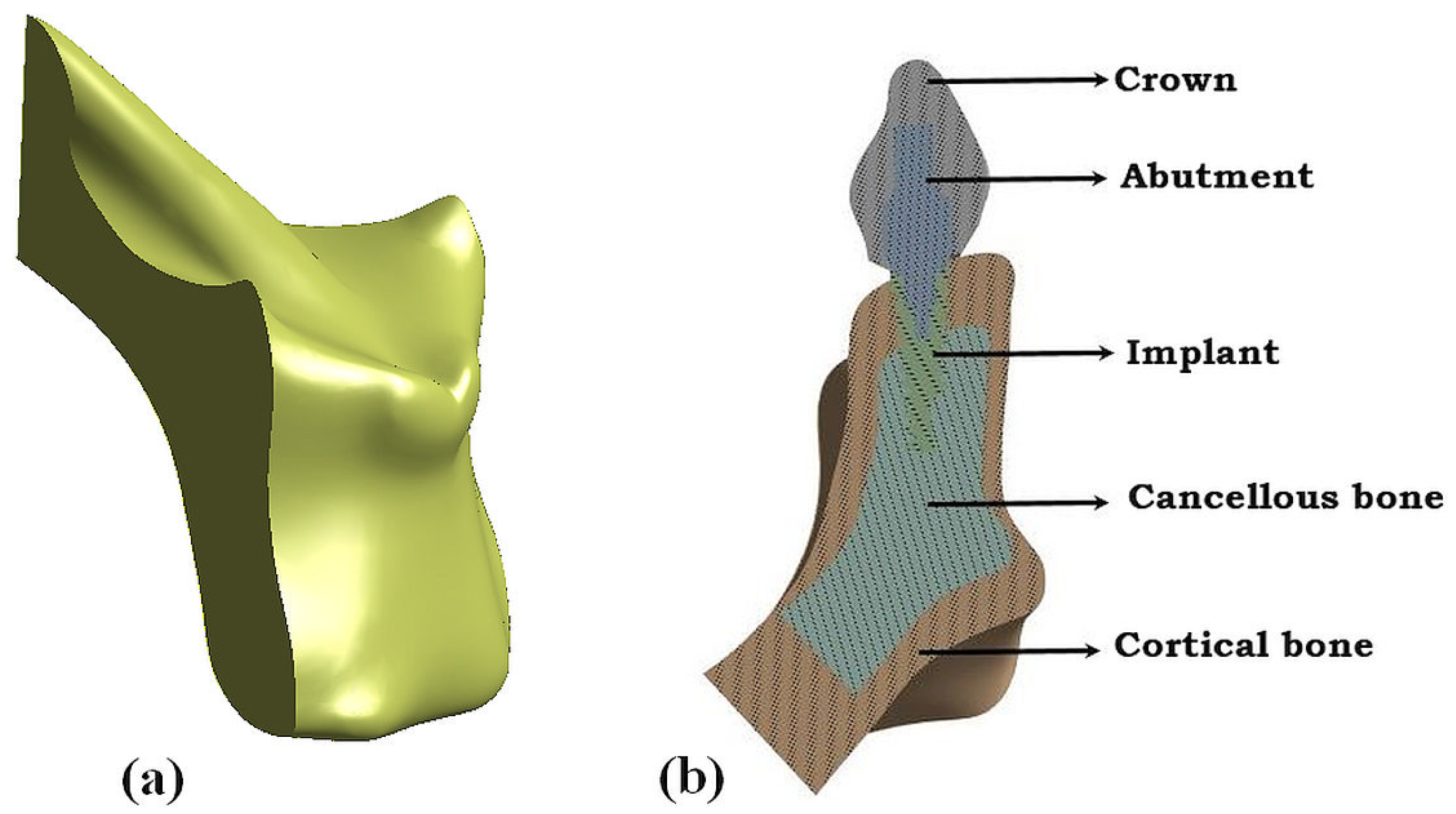
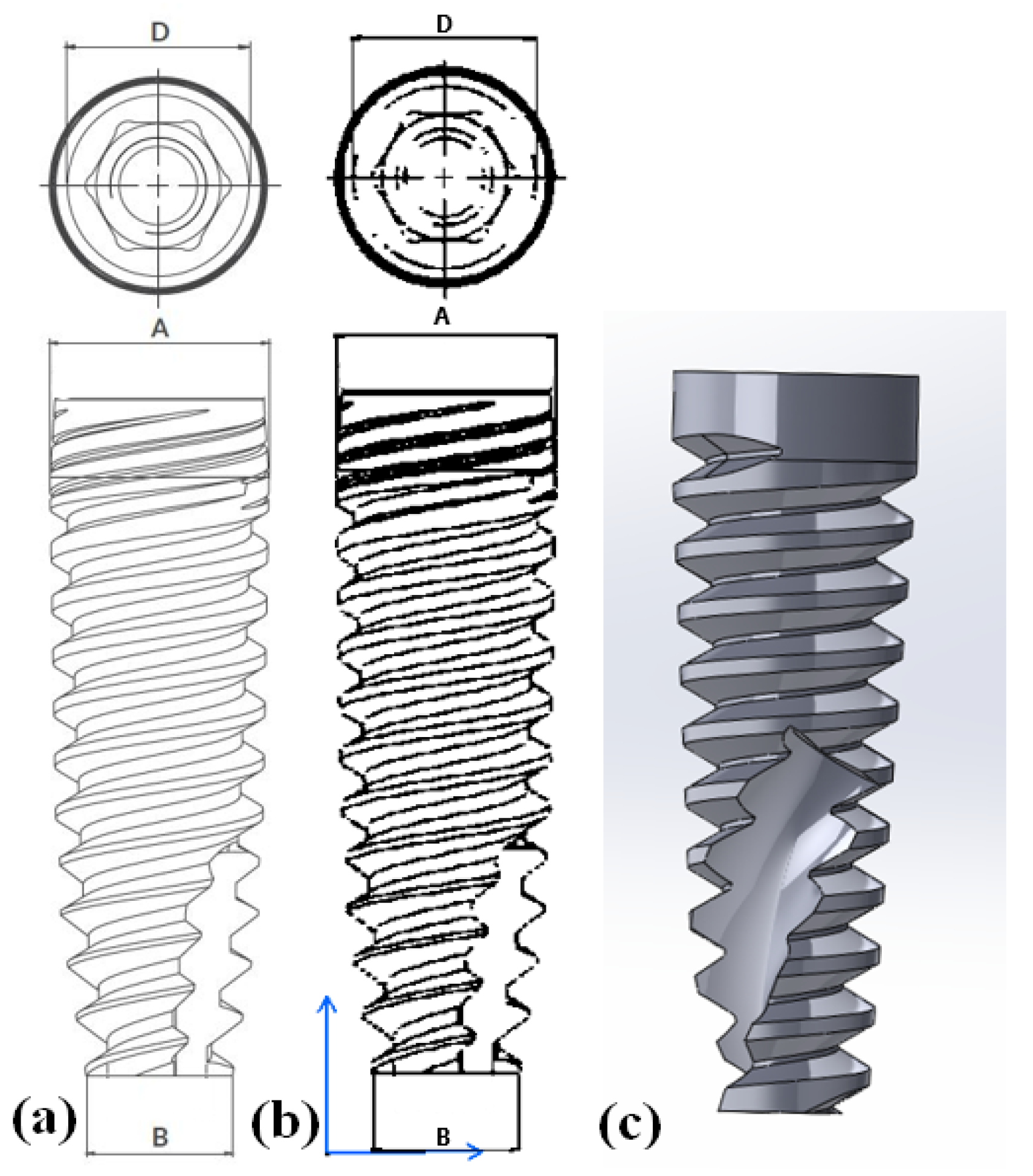
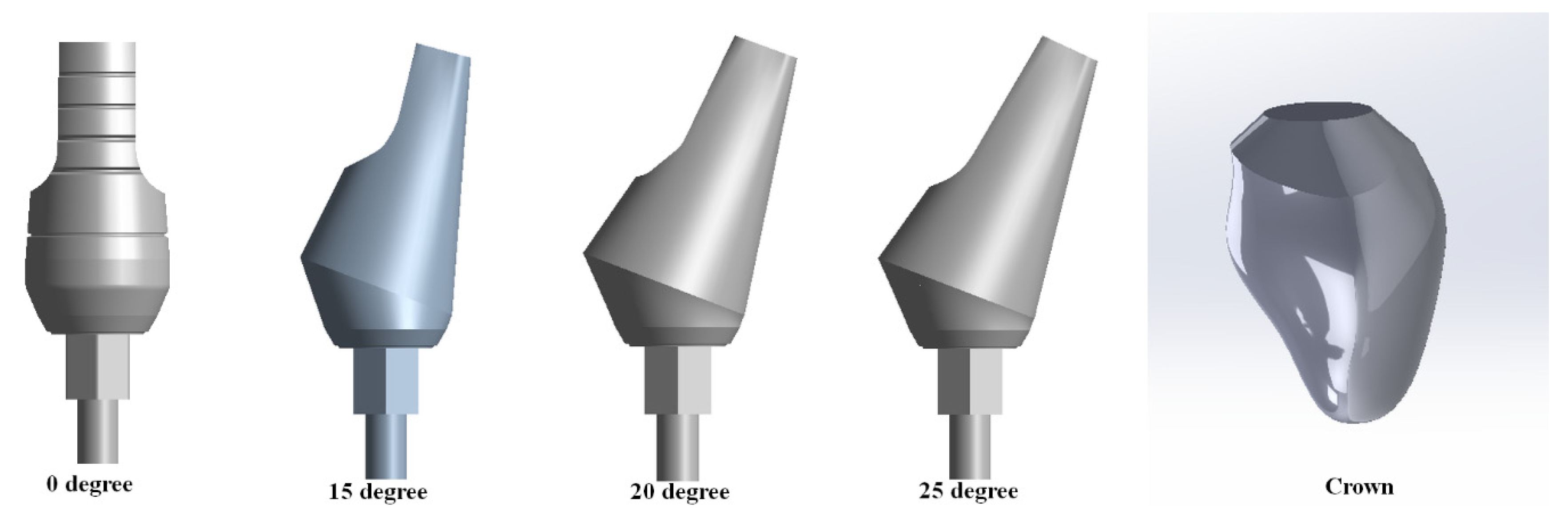
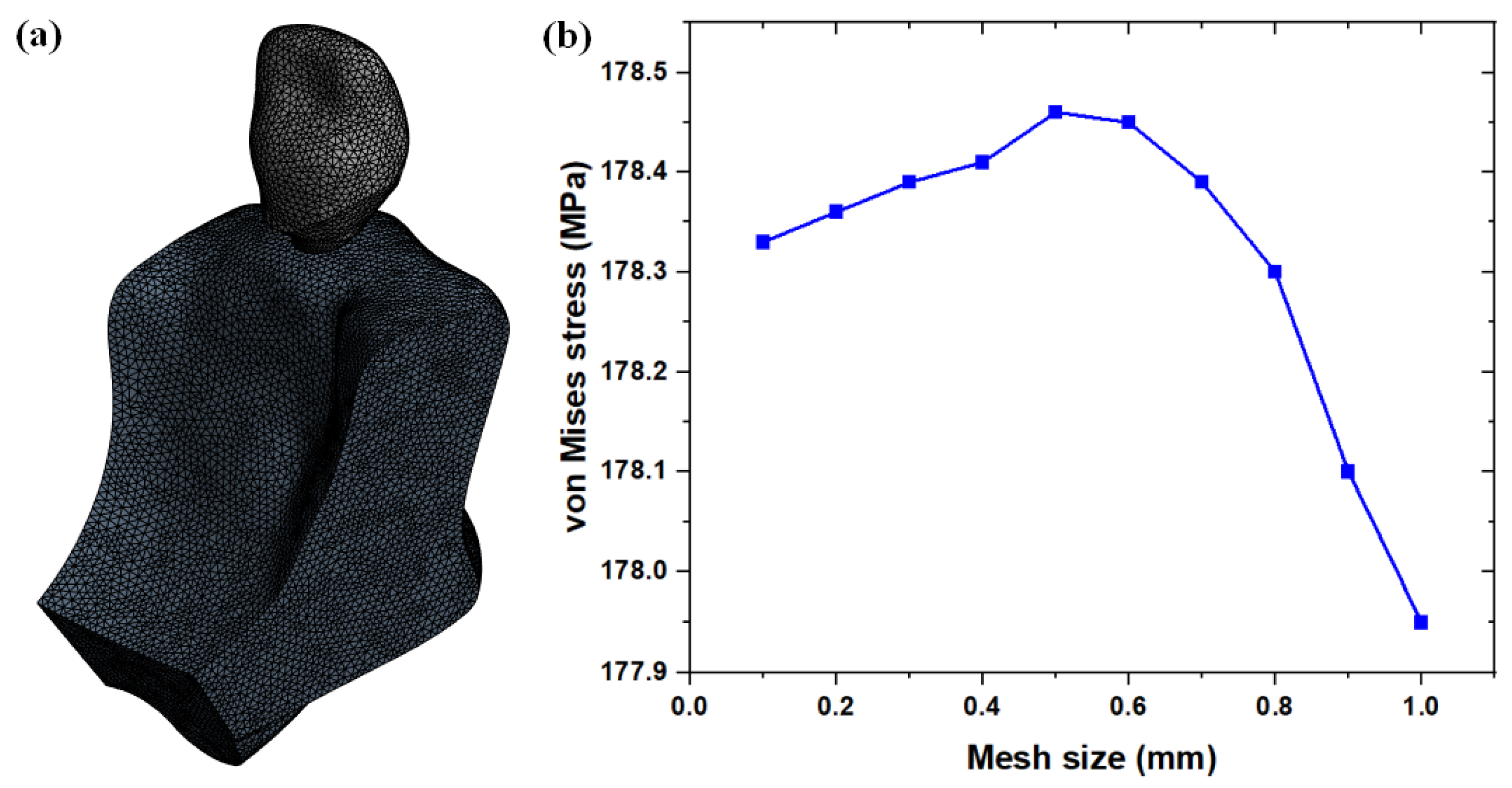
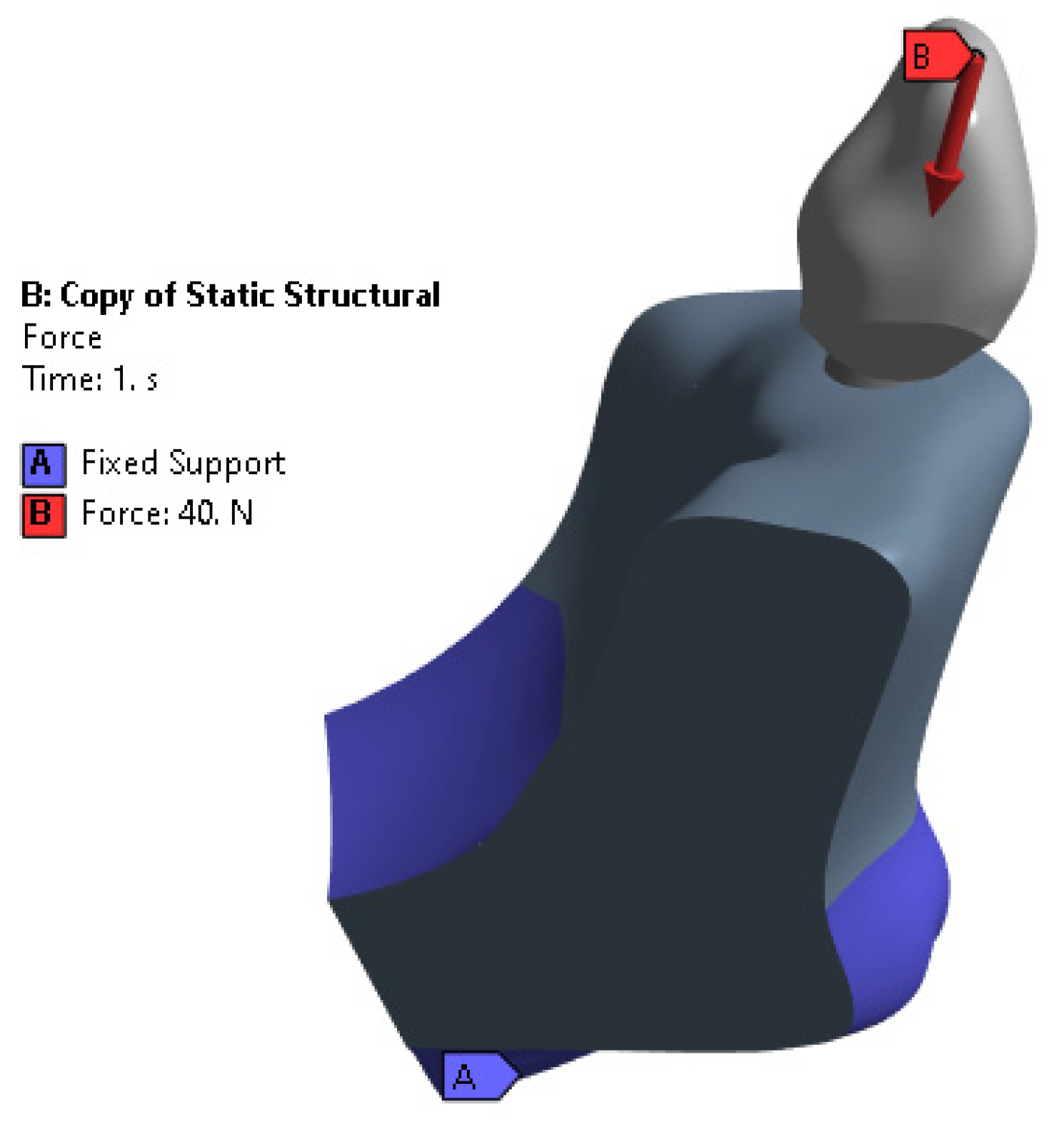


| Components | Node | Elements |
|---|---|---|
| Crown | 47,575 | 31,658 |
| Abutment | 91,614 | 63,194 |
| Implant | 55,416 | 35,432 |
| Cortical bone | 363,346 | 251,040 |
| Cancellous bone | 162,859 | 114,354 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
K N, C.; Eram, A.; Shetty, N.; Shetty, D.D.; Futane, M.; Keni, L.G. Evaluating Angled Abutments: Three-Dimensional Finite Element Stress Analysis of Anterior Maxillary Implants. Prosthesis 2024, 6, 315-328. https://doi.org/10.3390/prosthesis6020024
K N C, Eram A, Shetty N, Shetty DD, Futane M, Keni LG. Evaluating Angled Abutments: Three-Dimensional Finite Element Stress Analysis of Anterior Maxillary Implants. Prosthesis. 2024; 6(2):315-328. https://doi.org/10.3390/prosthesis6020024
Chicago/Turabian StyleK N, Chethan, Afiya Eram, Nisha Shetty, Divya D. Shetty, Mohan Futane, and Laxmikant G. Keni. 2024. "Evaluating Angled Abutments: Three-Dimensional Finite Element Stress Analysis of Anterior Maxillary Implants" Prosthesis 6, no. 2: 315-328. https://doi.org/10.3390/prosthesis6020024
APA StyleK N, C., Eram, A., Shetty, N., Shetty, D. D., Futane, M., & Keni, L. G. (2024). Evaluating Angled Abutments: Three-Dimensional Finite Element Stress Analysis of Anterior Maxillary Implants. Prosthesis, 6(2), 315-328. https://doi.org/10.3390/prosthesis6020024







